Abstract
We have measured low-intensity, polarized one-color pump-probe traces in the B800 band of the light-harvesting complex LH2 of Rhodospirillum molischianum at 77 K. The excitation/detection wavelength was tuned through the B800 band. A single-wavelength and a global target analysis of the data were performed with a model that accounts for excitation energy transfer among the B800 molecules and from B800 to B850. By including the anisotropy of the signals into the fitting procedure, both transfer processes could be separated. It was estimated in the global target analysis that the intra-B800 energy transfer, i.e., the hopping of the excitation from one B800 to another B800 molecule, takes ∼0.5 ps at 77 K. This transfer time increases with the excitation/detection wavelength from 0.3 ps on the blue side of the B800 band to ∼0.8 ps on the red side. The residual B800 anisotropy shows a wavelength dependence as expected for energy transfer within an inhomogeneously broadened cluster of weakly coupled pigments. In the global target analysis, the transfer time from B800 to B850 was determined to be ∼1.7 ps at 77 K. In the single-wavelength analysis, a speeding-up of the B800 → B850 energy transfer rate toward the blue edge of the B800 band was found. This nicely correlates with the proposed position of the suggested high-exciton component of the B850 band acting as an additional decay channel for B800 excitations.
INTRODUCTION
Photosynthesis is the synthesis of organic compounds by the use of photons. The primary steps of photosynthesis are light harvesting and charge separation. For light harvesting, all photosynthetic organisms have so-called antennae or light-harvesting complexes. The function of these pigment(-protein) complexes is to absorb light and to transport the excited state energy finally to a special pigment-protein complex—the photosynthetic reaction center—where charge separation occurs (van Grondelle et al., 1994; Sundström et al., 1999; Hu et al., 2002).
In the mid-1990s, the crystal structure of the peripheral light-harvesting complex LH2 of the purple bacterium Rhodopseudomonas (Rps.) acidophila was resolved to high resolution by x-ray diffraction (McDermott et al., 1995), followed by the crystal structure of LH2 of Rhodospirillum (Rs.) molischianum (Koepke et al., 1996). The latter structure is known at a resolution of 2.4 Å; the complex consists of eight α- and eight β-transmembrane helices, 24 bacteriochlorophyll (BChl) a molecules, and eight lycopenes arranged in a ringlike structure with C8 symmetry. Sixteen of the BChl a molecules are bound to histidines of the α-, β-polypeptides in their transmembrane α-helical part (Zuber and Brunisholz, 1991) and form a ring structure that is responsible for the strong Qy absorption band around 850 nm at room temperature (the B850 band). The remaining eight BChl a molecules are bound to the α-polypeptides near the cytoplasmic surface, and are responsible for a second Qy absorption band around 800 nm (the B800 band). Therefore the LH2 complex is also commonly referred to as B800-850 complex. Whereas the electronic interaction between neighboring B850 pigments is quite strong (∼300 cm−1) and gives rise to excitonic behavior (Koolhaas et al., 1998), the interaction between adjacent BChl a in the B800 ring is only ∼10–20 cm−1. Those pigments can be regarded as monomers, inasmuch as this coupling is much smaller than the inhomogeneous broadening of the B800 band (∼100–200 cm−1 (Reddy et al., 1991; Wu et al. 1996a; Scholes and Fleming, 2000; Ihalainen et al., 2001)).
Excitation of the B800 band is followed by relaxation of the prepared electronic and vibrational states and by energy transfer processes between the B800 pigments, from B800 to B850, between the B850s and eventually by decay of the excited state, mostly by nonradiative decay. This energy transfer within B800-850 complexes of different purple bacteria has been studied by time-resolved measurements (Hess et al., 1995; Monshouwer et al., 1995; Jimenez et al., 1996; Joo et al., 1996; Wu et al., 1996a; Kennis et al., 1997; Pullerits et al., 1997; Herek et al., 2000; Salverda et al., 2000; Agarwal et al., 2001; Ihalainen et al., 2001), hole-burning spectroscopy (van der Laan et al., 1990; Reddy et al., 1991; de Caro et al., 1994; Wu et al., 1996a, 1996b; Matsuzaki et al., 2001; Zazubovich et al., 2002) and also by single-molecule experiments (van Oijen et al., 2000); the subject has been reviewed by Sundström et al. (1999). For the events taking place in the B800 band, de Caro et al. (1994) proposed a two-pool model. In this model the B800 pigments can be divided into two groups, one on the blue side and one on the red side of the B800 band. Pigments of the red pool can transfer exclusively to B850. In the blue pool, competition occurs between B800 → B850 transfer and transfer to the red B800 pool. De Caro et al. (1994) interpreted their hole-burning results at 1.2 K on Rhodobacter (Rb.) sphaeroides in terms of a wavelength-independent B800 → B850 transfer time of 2.5 ps and a wavelength-dependent B800 → B800 transfer time, which can be as fast as 0.85 ps for excitation in the blue wing of the B800 band.
Monshouwer et al. (1995) measured energy transfer in the LH2 complex of Rb. sphaeroides by one-color pump-probe spectroscopy at 77 K. In the red part of the spectrum, the population decays monoexponentially with a time constant of 1.2 ps. This was attributed to B800 → B850 energy transfer. When the excitation/detection wavelength was tuned to the blue, a second lifetime of 0.45 ps with increasing amplitude was necessary to fit the experimental data. It was concluded that blue B800s could transfer both to B850, in 1.2 ps, and to more red-absorbing B800s, with a hopping time of 0.7 ps. Note that in these experiments the intra-B800 transfer time was wavelength independent.
In one-color pump-probe experiments on Rb. sphaeroides at 77 K, Hess et al. (1995) found biexponential decays with wavelength-dependent time constants. For 790, 800, and 810 nm excitation/detection, fast components of 0.33, 0.46, and 0.60 ps, and slow components of 1.67, 1.80, and 1.90 ps, respectively, were measured. It was argued that the slow component (∼1.8 ps) corresponds to B800 → B850 transfer.
In two-color pump-probe experiments on Rb. sphaeroides at 77 K Pullerits et al. (1997) took a slightly different approach. By exciting at 795 nm and detecting at 800 nm, two decay times (0.3 ps and 1.2 ps) were found. The latter was attributed to B800 → B850 transfer and the former to energy equilibration within the inhomogeneously broadened B800 band. (The faster decay time corresponds in our perception to the sum of rates for B800 → B800 and B800 → B850 energy transfer (see below). The true B800 → B800 transfer time would therefore be ∼0.5 ps). It was concluded that energy transfer within B800 could quantitatively be described by the Förster mechanism, but estimates for the B800 → B850 transfer based on Förster theory yielded slower transfer times than experimentally observed (see also Herek et al., 2000).
Pump-probe and hole-burning data on the B800-850 complex of Rps. acidophila have been reported by Wu et al. (1996a). For excitation at or to the red of the B800 absorption maximum, the pump-probe experiments at 19 K yielded a lifetime of 1.6 ps, which was attributed to B800 → B850 energy transfer. Hole-burning at 4.2 K in this spectral region gave a transfer time of 1.8 ps. When the excitation was located to the blue of the B800 absorption maximum, both techniques revealed a second decay channel for B800 with a rate constant of (400 fs)−1.
In Rs. molischianum, a B800 → B850 transfer time of 1.9 ps at 4.2 K has been determined by hole-burning (Wu et al., 1996b). But so far, only room temperature kinetics were published for that species (Salverda et al., 2000; Ihalainen et al., 2001). By photon echo measurements Salverda et al. (2000) estimated that the B800 → B850 transfer time is ∼0.7 ps and that the pairwise hopping time between B800 molecules is 1.6 ps. Two-color pump-probe experiments yielded a range of B800 → B850 transfer times, 0.9–1.2 ps, depending on the excitation wavelength (Ihalainen et al., 2001).
Generally spoken, the experiments agree on the observation that states on the blue side of the B800 band decay faster than those in the middle and on the red side. Besides the intra-B800 energy transfer (as in the model of de Caro et al., 1994), additional explanations for this effect have been proposed. It has been shown that it is not due to vibrational relaxation of BChl a modes that build on B800 or B850 (Wu et al., 1996a; Matsuzaki et al., 2001). The involvement of carotenoid molecules has been considered theoretically (Pullerits et al., 1997; Krueger et al., 1998a, 1998b; Mukai et al., 1999; Scholes and Fleming, 2000), but still awaits a more direct experimental support. Mixed B800-B850 states have been put forward (Wu et al., 1996a; Matsuzaki et al., 2001), but are now considered unlikely (Zazubovich et al., 2002). Finally, it has been suggested that the upper exciton levels of the B850 ring, lying in the vicinity of the B800 band, might play a role (Pullerits et al., 1997; Sundström et al., 1999; Scholes and Fleming, 2000).
With the present study, we want to remove the lack of low-temperature kinetic measurements for Rs. molischianum by reporting low-intensity, polarized one-color pump-probe traces in the B800 band at 77 K. Furthermore, we want to characterize the energy transfer involving the B800 BChl a molecules in more detail and obtain some information about the suggested role of the upper exciton levels of the B850 band in the B800 → B850 excitation energy transfer.
The analysis of kinetic measurements performed on the B800 band is inherently complex due to the variety of energy transfer processes taking place (see above). Moreover, all the bands are inhomogeneously broadened, and spectrally relatively broad pulses have to be used for the time-resolved measurements. Furthermore, the measured signal consists of ground state bleaching/stimulated emission from the B800s and excited state absorption from B850. Instead of only fitting the traces with a sum of exponentials, we have therefore based the analysis of our experimental results on a model that directly accounts for intra-B800 transfer and B800 → B850 transfer. The anisotropy of the transient signals is directly included in the modeling procedure. This approach proved to be powerful and allows for a separation of both transfer processes.
MATERIALS AND METHODS
Measurements
The measurements were performed on detergent isolated LH2 complexes of Rs. molischianum DSM 119. The samples were prepared as described before (Visschers et al., 1995).
A 0.5 cm cuvette was used, which consisted of a plastic spacer with two quartz plates loosely glued to the sides. The experiments were performed in a liquid nitrogen cryostat (Oxford) at 77 K. The maximum optical density in the B800 band was 0.6 at 798 nm. The absorption was measured on a home-built spectrophotometer.
For the time-resolved measurements a titanium:sapphire laser (Mira-SEED, Coherent Laser Group, Santa Clara, CA, modified for cavity dumping), pumped by an argon ion laser (Coherent) was used, operated at a repetition rate of 125 kHz. The autocorrelation of the light pulses had a full width at half-maximum (fwhm) of ∼60 fs. The chirp of the pulse was corrected by a prism compensator.
The desired pump/probe wavelengths were selected by a filter. The spectral fwhm of the pulse after the filter was ∼8 nm and the autocorrelation had a fwhm of 180 fs. The pulse was split into a pump and a probe beam with average powers of less than 40 and 30 μW, respectively. The beam diameter was ∼50 μm in the focus, determined as fwhm of a Gaussian intensity profile. The measurements were performed at magic angle (54.7°), parallel and perpendicular polarizations of pump versus probe pulse.
Under our experimental conditions, about one out of 100 BChl a molecules is excited per pulse. Because eight BChl a molecules per complex contribute to the B800 band of Rs. molischianum, every LH2 complex receives ∼8/100 excitations. By assuming that the number of excitations absorbed by each complex is governed by Poisson statistics, ∼0.3% of all complexes will get two excitations. Therefore, any annihilation effects can safely be neglected in our experiments. It has also been demonstrated that high excitation intensities can cause photodamage to LH2, thereby lengthening the B800 → B850 energy transfer times (Monshouwer et al., 1995). Our experimental conditions are comparable to the low-intensity measurements by Monshouwer et al. (1995).
Data analysis
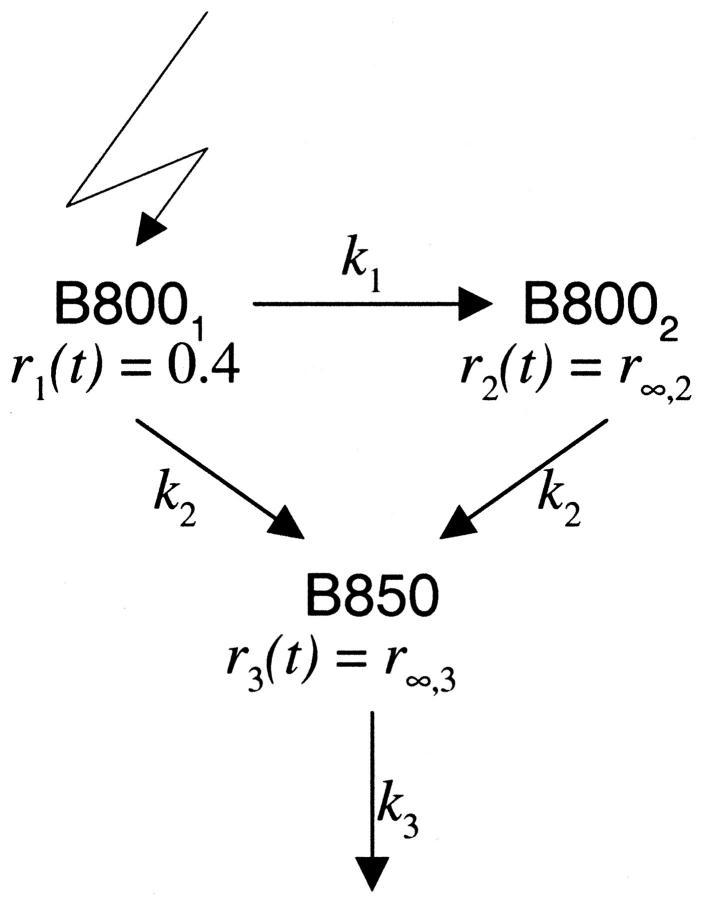
In the target analysis (Holzwarth, 1996) the measured traces were analyzed individually as well as globally using a branched model with three compartments, which is illustrated in Fig. 1. It is an extension of the two-pool model by de Caro et al. (1994), described above. The B800 molecules are split into two pools. The first pool is excited directly and the second is not. The directly excited pool can decay by energy transfer to the second pool of B800s with rate k1 or by energy transfer to B850 with rate k2. The second pool can only decay to B850 (with rate k2). The decay rate of B850, k3, was fixed to 1 ns−1. On the timescale of the experiment, this corresponds to an infinite time constant. One can think about the directly excited pool of B800 pigments as “blue” and the second pool as “red” B800s. However, one should keep in mind that in our experiment also excitation is performed into the red wing and that at 77 K also uphill energy transfer among the B800s is likely to occur. Therefore we have chosen the term “(not-)directly excited” pool - in an adaptation of the original two-pool model of de Caro et al. (1994).
FIGURE 1.
Model used to analyze the data (for details see text). B8001 is the directly excited and B8002 is the not-directly excited B800 pool.
It is assumed that the measured signal consists of contributions from all compartments: ground state bleaching/stimulated emission from each of the B800 pools and excited state absorption from B850. The magic angle decay mak(t) of each compartment k is given by the solution to the set of differential equations describing the model. The polarized model decay curves of compartment k for vertical excitation/vertical detection vvk(t) and for vertical excitation/horizontal detection vhk(t) are related to the magic angle model decay curve mak(t) of compartment k by:
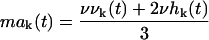 |
(1) |
and define the anisotropy curve of compartment k:
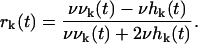 |
(2) |
As indicated in Fig. 1, the anisotropy rk(t) of each of the compartments k is set to a constant in the model (see also below).
Using Eqs. 1 and 2, the polarized model traces can be described in terms of magic angle mak(t) and anisotropy rk(t), and the contribution of each compartment k to the experimental traces for magic angle decay MA(t), for vertical excitation/vertical detection VV(t) and for vertical excitation/horizontal detection VH(t) is expressed in the following equation:
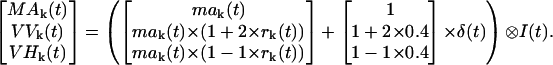 |
(3) |
The second term in the sum with the δ-function accounts for the coherent coupling artifact, which was assumed to have an anisotropy of 0.4. The model functions have to be convolved with the instrument response I(t), which was modeled as a Gaussian (fwhm was fitted to 160 ± 30 fs).
As mentioned, the measured signal is a superposition of contributions from all compartments k. The respective experimental traces at time t and wavelength λ are described by:
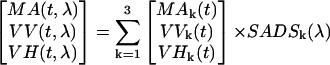 |
(4) |
SADSk is the species associated difference spectrum of compartment k. The SADS were constructed by normalizing the traces to a constant excited state absorption for B850, which is known to be flat in the B800 region (Kennis et al., 1997).
In the fitting procedure, time 0 for each of the measured traces was determined. Furthermore, relative scaling parameters of the respective traces MA(t, λ), VV(t, λ), and VH(t, λ) were estimated, allowing correction for long-term variations of the laser intensity.
RESULTS
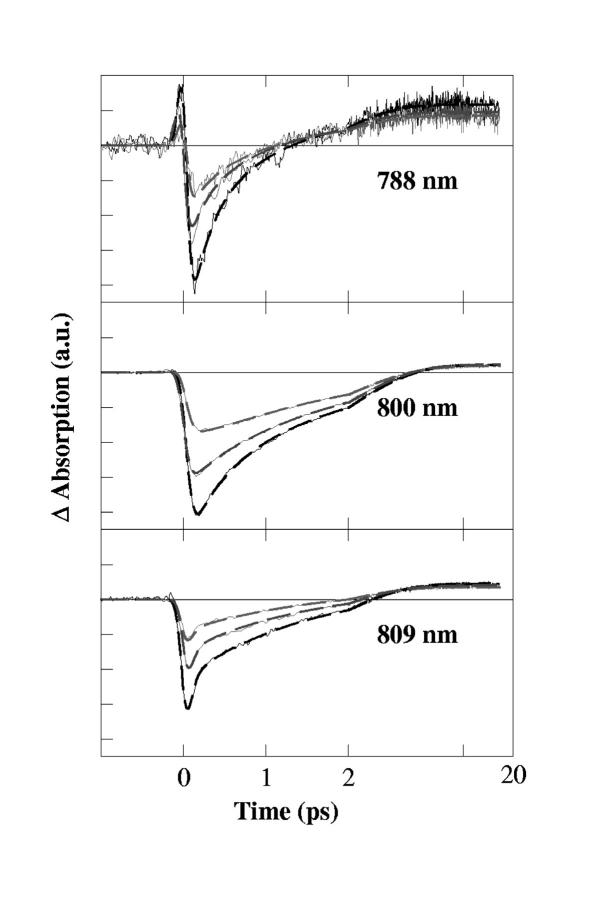
In Fig. 2, one-color pump-probe traces measured in the LH2 complex of Rs. molischianum at 77 K for excitation/detection in the blue wing, in the middle, and in the red wing of the B800 band are shown. The qualitative appearance of these traces is similar to those reported before for Rb. sphaeroides (Monshouwer et al., 1995; Hess et al., 1995; Pullerits et al., 1997). Excitation results in an instantaneous (pulse-width limited) bleaching/stimulated emission signal. This signal decays and changes into an induced-absorption signal with an infinite lifetime (on the experimental timescale). Around time 0 the signal is distorted by the coherent coupling artifact. In the fitting procedure this was accounted for as described in the section Materials and Methods.
FIGURE 2.
VV, VH, and MA traces (in black, red, and blue, respectively) are shown for excitation/detection at 788 nm, 800 nm, and 809 nm. In every subpanel the measured trace (solid line) and the single-wavelength fit (dashed line) are show. The timescale is linear between 0 and 2 ps and logarithmic between 2 and 20 ps.
At each individual wavelength, the traces detected with different polarizations were fitted to the model depicted in Fig. 1. The fits are included in Fig. 2 to illustrate the quality of the data and the fits. We also performed a global target analysis of all the traces using the model described above. The results of the single-wavelength and the global data analysis are summarized in Table 1 and visualized in Fig. 3.
TABLE 1.
Polarized, one-color pump-probe measurements in LH2 of Rs. molischianum at 77 K
| 1/k1 (ps)
|
1/k2 (ps)
|
r∞,2†
|
r∞,3
|
|||||
|---|---|---|---|---|---|---|---|---|
| λ (nm)* | Single | Global | Single | Global | Single | Global | Single | Global |
| 788 | 0.31 | 1.3 | 0.23 | 0.14 | ||||
| 793 | 0.44 | 1.5 | 0.17 | 0.09 | ||||
| 794 | 0.52 | 1.7 | 0.16 | 0.17 | 0.11 | |||
| 797 | 0.47 | 1.8 | 0.14 | 0.09 | ||||
| 800 | 0.48 | 0.53 | 1.9 | 1.7 | 0.16 | 0.08 | 0.11 | |
| 803 | 0.53 | 1.8 | 0.24 | 0.23 | 0.12 | |||
| 806 | 0.80 | 1.7 | 0.28 | 0.30 | 0.11 | |||
| 809 | 0.06‡ | 1.6 | 0.36 | 0.33 | 0.12 | |||
Shown are the results from the single-wavelength and the global target analysis using the model depicted in Fig. 1. The values are illustrated in Fig. 3. The estimated uncertainties for the rates (lifetimes) are ∼10%.
Excitation/detection wavelength.
In the global target analysis, separate values for the residual anisotropy r∞,2 were allowed for excitation/detection at 803, 806, and 809 nm (see text).
This value is irrelevant here as a monoexponential fit results in the same residual sum of squares and the same value for k2 (see text).
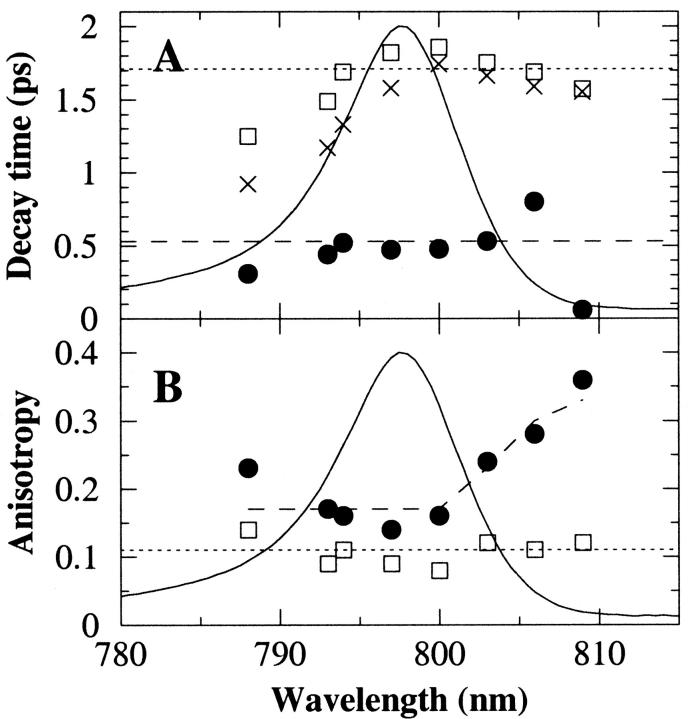
FIGURE 3.
Wavelength dependence of the estimated parameters from the single-wavelength target analysis (symbols) and estimated values from the global target analysis (broken lines) (see Table 1). The absorption spectrum is shown as solid line. (A) The energy transfer times 1/k1 (solid circles, dashed line), 1/k2 (squares, dotted line), and the decay times from a monoexponential fit (crosses) are shown. (B) The anisotropies of the not-directly excited B800 pool (r∞,2, solid circles, dashed line) and of B850 (r∞,3, squares, dotted line) are shown.
The bleaching/stimulated emission is attributed to the excitation of B800 molecules. In our model the B800 excited-state population decays by (pairwise) energy transfer within B800 and by energy transfer to B850. The latter transfer results in excited B850 states, which contribute to the measured signal in the 800 nm region by their excited-state absorption. The intra-B800 energy transfer will result in a decay of the B800 anisotropy (see below). Because of our modeling procedure (target analysis), the estimated time constants are indeed energy transfer times. From the global analysis the B800 → B850 energy transfer time 1/k2 is determined to be 1.7 ps. However, some wavelength dependence can be observed (Fig. 3 A). The B800 → B850 transfer time is 1.3 ps for excitation/detection on the blue side of the B800 band (788 nm), has a maximum of 1.9 ps for excitation/detection at 800 nm, and is 1.6 ps for red excitation/detection at 809 nm.
The intra-B800 energy transfer time 1/k1, estimated from the global target analysis, is ∼0.5 ps. From the single-wavelength analysis we find that this transfer is fast in the blue, 0.3 ps, and slows down with increasing excitation/detection wavelength to 0.8 ps at 806 nm (Fig. 3 A). The estimated time constant for 809 nm excitation/detection is very short. However, a monoexponential fit results in the same residual sum of squares and gives the same value k2 for B800 → B850 transfer. Therefore, if one wants to describe the data with a minimal model, the measurement at 809 nm is characterized by one exponent only and k1 is irrelevant here. The conclusion is, in other words, that at this wavelength only B800 → B850 energy transfer occurs. The anisotropy characterizing the B850 excited-state absorption, r∞,3, is ∼0.1 for all individual single-wavelength experiments, which is also reflected in the global analysis (Fig. 3 B). The anisotropy of the not-directly excited B800s, r∞,2, is found in the single-wavelength analysis to be large in the wings (0.23 and 0.36 for 788 nm and 809 nm, respectively; in the monoexponential fit to the 809 nm experiment as described above, the anisotropy value is of course 0.4) and lower in the middle of the B800 band (Fig. 3 B). When in the global analysis all traces are fitted with the same value for r∞,2, the fits to traces measured in the red wing of the B800 band are not good. Therefore we have decided to allow separate r∞,2 values for 803, 806, and 809 nm excitation/detection. One might argue based on Fig. 3 B that this procedure should also be followed for 788 nm excitation/detection. However, this does not result in a lower value of the residual sum of squares; because of relatively larger noise in that trace compared to the others, r∞,2 has a relatively large error here. The described procedure results in a global r∞,2 value of ∼0.17 for blue side and middle of the B800 band, which increases toward the red up to 0.33 for excitation/detection at 809 nm.
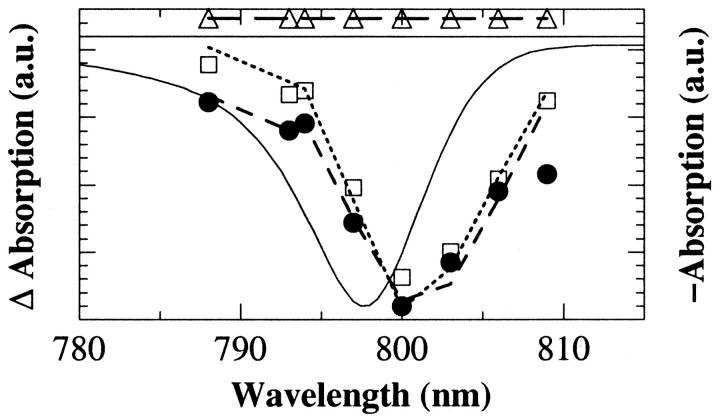
SADS were constructed as described in the section Materials and Methods for the individually as well as the globally analyzed data, which results in approximately the same sets of SADS (see Fig. 4). The SADS for the directly excited B800 and the not-directly excited B800 are essentially the same and red-shifted relative to the absorption spectrum.
FIGURE 4.
Species associated difference spectra (SADS) were constructed from the single-wavelength (symbols) and the global target analysis (broken lines) by assuming a constant excited-state absorption for B850 (triangles, long-dashed line). The SADS for the directly excited B800 is shown as filled circles/dashed line, and the SADS for the not-directly excited B800 as squares/dotted line. The inverse absorption spectrum is included in the figure (thin solid line).
DISCUSSION
B800 → B850 energy transfer
We determined the B800 → B850 energy transfer time in Rs. molischianum at 77 K to be ∼1.7 ps with some wavelength dependence through the B800 band. At 4.2 K, a transfer time of 1.9 ps was determined by hole-burning spectroscopy (Wu et al., 1996b), which is in good agreement with our result.
No time-resolved data for Rs. molischianum at low temperature are available. At room temperature, the B800 → B850 transfer time is ∼0.7–1.2 ps (Salverda et al., 2000; Ihalainen et al., 2001). The increase of the rate with higher temperature is comparable to that observed for LH2 of Rb. sphaeroides. For that species a low-intensity B800 → B850 energy transfer time of 1.2 ps (wavelength independent) has been reported at 77 K (Monshouwer et al., 1995), whereas the transfer speeds up to ∼0.7 ps at room temperature (Hess et al., 1995).
There is an ongoing discussion about the mechanism of the B800 → B850 energy transfer in LH2 (Mukai et al., 1999; Sumi, 1999; Sundström et al., 1999; Sundström, 2000; Scholes and Fleming, 2000). Förster overlap calculations for the energy transfer rate between B800 and B850 yielded considerably longer transfer times than experimentally determined (Pullerits et al., 1997; Herek et al., 2000). This discrepancy has led to the assumption that also other decay mechanisms might play a role (see Introduction). It was noted that the upper exciton levels of the B850 ring are in the vicinity of the B800 band. This high-exciton band of B850, which is hidden under the B800 Qy absorption and which is supposed to be located at ∼780 nm (Wu et al., 1997; Koolhaas et al., 1998; Georgakopoulou et al., 2002), is probably such an extra decay route for excited B800 states.
Scholes and Fleming (2000) have extended the Förster model for excitation energy transfer between a donor and an acceptor to complex, coupled multichromophoric systems like LH2. Their method allows to calculate ensemble average transfer rates. It was found that, by taking energetic disorder of the excitonic B850 band into account, the B800 → B850 energy transfer rate increases considerably. Furthermore, it is worth mentioning that although the upper exciton levels of B850 have small transition dipole moments (in a perfect ring they are even optically forbidden!), excitation energy from B800 can be transferred to them with a large rate (see also Mukai et al., 1999). Compared to earlier attempts (Pullerits et al., 1997; Herek et al., 2000) the calculated rates are closer to the experimental values but still a factor of 2 too slow (note, however, that no correction for the dielectric screening was performed). With respect to our observations, it is important to notice that the major band of the B850 density of states is located at ∼780 nm and that the overlap integral (weighted by the coupling between B800 and B850 and ensemble averaged) not only peaks below 800 nm but also has a center of gravity below 800 nm (see Scholes and Fleming (2000): Fig. 7, Fig. 9, and Fig. 16). The calculations were done for Rb. sphaeroides and Rps. acidophila. The ringlike structure of the latter species is very similar to that of Rs. molischianum. Moreover, the energy gaps between B800 and B850 in Rps. acidophila and Rs. molischianum are approximately equal (see Wu et al., 1996b). It is therefore reasonable to conclude that also in Rs. molischianum, the density of states of the B850 ring will have a major peak on the blue side of the B800 band. This is indeed indicated by linear dichroism measurements on LH2 complexes of both species (not shown) and by calculations of the exciton dynamics within the LH2 of Rs. molischianum using a Redfield approach (Novoderezhkin, V., M. Wendling, and R. van Grondelle. In preparation).
Although our measurements can very well be described by two global rates, k1 and k2, we see a wavelength-dependent variation of the B800 → B850 transfer time in the single-wavelength analysis. Roughly speaking, the B800 → B850 transfer is faster in the blue than in the middle and red of the B800 band. This nicely correlates with the model of Scholes and Fleming (2000), where the wavelength dependence of the overlap integral suggests a faster rate on the blue side of the B800 band due to a better overlap of it with the high-exciton band of B850. Energetic disorder in both the B800 and B850 band results in a distribution of B800 → B850 rates, with the faster rates on the blue side of the B800 band. This is reflected in the observed wavelength dependence of the B800 → B850 transfer time. A variation in the B800 → B850 transfer times was also reported for Rb. sphaeroides at 77 K (Hess et al., 1995). However, in these experiments the B800 → B850 energy transfer time nearly linearly increases with the excitation/detection wavelength.
The trend for Rs. molischianum at room temperature (Ihalainen et al., 2001) is opposite to the one observed by us at 77 K. It is fast for excitation at 800 nm (0.9 ps) and slows down for 790 and 810 nm excitation (1.2 and 1.0 ps, respectively). One should note, however, that in that study in the analysis of the isotropic data, no intra-B800 transfer was allowed for, which probably obscures the estimated transfer times.
In a recent hole-burning study, Matsuzaki et al. (2001) used an LH2 complex of Rps. acidophila containing only one B800 molecule. In this B800-deficient LH2 the intra-B800 energy transfer was elegantly switched off. A wavelength-independent hole width was measured in the B800 band corresponding to a time of ∼3.2 ps. This time was attributed to B800 → B850 energy transfer, but it is much longer than B800 → B850 transfer takes in intact LH2, where for Rps. acidophila time constants were determined of 1.8 ps by hole-burning at 4.2 K and of 1.6 ps by pump-probe at 19 K (Wu et al., 1996a). Matsuzaki et al. (2001) explain the hole-burning results on intact LH2 and B800-deficient LH2 by mixed B800-B850 states. These states are responsible for the shorter lifetimes on the blue side of the B800 band in intact LH2. In the B800-deficient sample, those states are not present and therefore a constant lifetime over the B800 band is expected. However, from high-pressure hole-burning data it was concluded that mixing between B800 and B850 states is unimportant (Zazubovich et al., 2002).
Independent of the actual mechanism, it is instructive to reflect about the difference in the estimated B800 → B850 transfer times between hole-burning on the B800-deficient LH2 and (our) pump-probe measurements on intact LH2. The hole-burning and the pump-probe experiments each have their own merits and flaws, and neither of them directly yields unbiased results. Therefore it is very worthwhile to explore possible explanations for the discrepancy between the experiments. Let us therefore consider what would be the consequences if the transfer rates for direct transfer from each of the B800 pigments to B850 are actually taken from a broad distribution due to the disorder in the B850 ring.
In the intact B800 ring, B800 molecules with a slow transfer rate to B850 can still be well-connected to B850 through their neighboring B800 molecules, and therefore the slow part of the rate distribution will never show up—in contrast to the B800-deficient sample, where the longer lifetimes are visible. These longer lifetimes from the distribution are even extra emphasized in a hole-burning experiment, where naturally narrow holes are more easily detected than broad holes, which results, in the case of a distribution of lifetimes, in a bias toward narrower holes, i.e., longer times (see also Pullerits et al., 1997). This might be an explanation why the estimated B800 → B850 transfer time in the LH2 complex with only one B800 molecule is longer than in intact LH2. On the other hand, the explanation might be more biochemical. The spectrum of the B800-deficient sample obviously misses the usual steep red flank of the B800 band and shows a more Gaussian-like B800 absorption profile (see Fig. 1 in Matsuzaki et al., 2001). One might wonder if this is caused by some structural change of the B800 bounding to the protein. Taking this and the above-mentioned arguments into account, we think that the hole-burning results on the B800-deficient sample should not directly be compared to our experiments on intact LH2.
Intra-B800 energy transfer
From the global target analysis, the intra-B800 energy transfer time in Rs. molischianum at 77 K is estimated to be ∼0.5 ps. The intra-B800 energy transfer slows down from 0.3 ps to 0.8 ps for excitation/detection on the blue and the red side of the B800 band, respectively (Fig. 3 A). For Rb. sphaeroides, Monshouwer et al. (1995) reported a wavelength-independent 0.7 ps for the B800 → B800 transfer. If we want to compare our values with those of Hess et al. (1995) on Rb. sphaeroides, we have to consider that the fast times in those experiments correspond to the sum of rates for intra-B800 and B800 → B850 transfer. When this is taken into account, we can calculate for the study of Hess et al. (1995), that the intra-B800 energy transfer time in Rb. sphaeroides at 77 K slows down from 0.4 ps to 0.9 ps for blue versus red side excitation of the B800 band, which is comparable to our measured values for Rs. molischianum.
The increase of the energy transfer time with a factor of 2 while going from blue to red excitation/detection is “understandable” within a “mechanistic” (Matsuzaki et al., 2001) picture. If a blue pigment is excited, there is a high probability that its two neighboring pigments absorb “downhill”—in contrast to red excitation where this probability is low. In this picture one expects a factor of 2. Therefore, we arrive at a hopping time within B800 of ∼0.8 ps for Rs. molischianum at 77 K. The 0.5 ps from the global analysis should be seen as average of one- and two-step transfers.
At room temperature, a value of 1.6 ps was found for the hopping time between adjacent pigments in B800 based on three-pulse-photon echo experiments (Salverda et al., 2000). At first sight it might be surprising that we find this hopping time to be faster at 77 K (0.8 ps). For simplicity, let us assume Gaussian homogeneous absorption and emission spectra with a fwhm of kT and a temperature-dependent Stokes shift between absorption and emission. Furthermore we take a Gaussian inhomogeneous distribution function with fwhm of 130 cm−1. By using Gaussian arithmetic (see Appendix) it is then straightforward to calculate that the average rate will be 1.8 times slower at room temperature than at 77 K. If we consider the simplicity of the estimates, this is in very good agreement with the experimental results.
Anisotropy
Anisotropy signals are inherently complex in case the measured signal consists of several contributions. Even if the isotropic decay is exponential, the anisotropy will then in general display a nonexponential decay.
If the initial excited state population disappears from the probe window, such as in a one-color pump-probe experiment, the anisotropy will not decay. Decay of the anisotropy is only observed when excitations are transferred to pigments in the probe window. Taking this into account but keeping the model simple, we haven chosen constant anisotropies for all compartments (Fig. 1). As a result of this approach, the anisotropy of the B800s will approximately (appear to) decay with the rate constant k1 of the intra-B800 transfer from 0.4 to r∞,2. From measurements in Rb. sphaeroides (Jimenez et al., 1996) it was concluded that the anisotropy in B850 decays very fast, so that in good approximation we may assume an immediate end value r∞,3. Moreover, we analyzed separately the kinetics measured at magic angle versus the parallel/perpendicular components. The obtained rate constants do agree within experimental uncertainty (smaller than 10%). This justifies our target analysis.
Globally, we find a constant value for r∞,2 for excitation/detection in the blue and in the center of the band and an increase of this residual anisotropy of B800 toward the red side (Fig. 3 B). These results are similar to those of fluorescence site selection experiments performed in the B850 band of LH2 and in the light-harvesting complex 1 of purple bacteria (van Mourik et al., 1992; Visschers et al., 1995; Wendling et al., 2002). A quantitative explanation of this effect was given by van Mourik et al. (1992) in terms of energy transfer in a cluster of weakly coupled pigments, which have inhomogeneously distributed site energies. When excitation is performed into the blue wing and the center of the absorption band, excitation energy will be transferred to the pigment with the lowest energy per cluster. This energy transfer results in a low anisotropy due to the different orientations of the transition dipole moments of the pigments in each cluster. When excitation is performed into the red wing of the absorption band, an increasing fraction of lowest-energy pigments will be directly excited. Therefore less energy transfer will occur, resulting in a high anisotropy. It has been shown that this description remains also valid when the pigments' pure electronic states are replaced by exciton states (Westerhuis et al.,1999; Wendling et al., 2002).
Keeping in mind that in a one-color pump-probe experiment we see the excited state population disappear from the probe window, the trend of the measured residual anisotropy r∞,2 in B800 can nevertheless be described by the same scenario. When excitation is done into the blue wing of the band, energy is transferred away to the center of the B800 band (and of course directly to B850). But because of the phonon wing the accepting B800 pigments will still be visible in a blue probe window and the energy transfer that occurred will show up as a relatively low anisotropy. For excitation/detection in the middle of the band, the extensive energy transfer steps are directly monitored, resulting as well in a low anisotropy. Tuning the excitation/detection to the red, more and more lowest-energy pigments will be excited directly and less energy transfer will occur, increasing the anisotropy.
Even though we are using spectrally broad excitation/detection pulses that smear out some of the wavelength-dependent effects, it can be seen that the measured anisotropy effect can qualitatively be described by our model. Moreover, this anisotropy effect is expected in an inhomogeneously broadened absorption band. That it can be derived from the data, although it is hidden under the B800 → B850 contributions, is a powerful demonstration of how a precise modeling procedure can unravel these effects.
Model
Strictly speaking, our model is not very realistic because the situation in B800 is much more complex. The B800 band is inhomogeneously broadened and the width of this distribution is on the order of 2…3 kT. Furthermore, spectrally broad pulses have to be used to unravel the fast kinetics. On the other hand it is a minimal model sufficient to describe the measured data.
The SADS for directly and not-directly excited B800s are very similar (see Fig. 4). As both B800 pools characterize the same states, this is as expected and demonstrates the self-consistency of our model. The red shift of the B800 SADS compared to the absorption spectrum we attribute to the fact that the B800 SADS are a combination of bleaching and stimulated emission. The B800 (aselective-excited) emission is known to have a large Stokes shift of ∼6–8 nm (van Grondelle et al., 1982; de Caro et al., 1994) at liquid helium temperature.
CONCLUSIONS
We presented low-intensity, polarized one-color pump-probe traces in the B800 band of the LH2 complex from Rs. molischianum at 77 K. By using a two-pool model for B800 accounting for intra-B800 and B800 → B850 excitation energy transfer and also anisotropy effects, the fitting procedure proved to be powerful to separate both transfer processes. Intra-B800 transfer can be described by pairwise energy transfer in a system of weakly coupled pigments. Globally, intra-B800 energy transfer takes place within ∼0.5 ps and the time constant for transfer from B800 → B850 is ∼1.7 ps. The speeding up of the latter transfer rate with blue side excitation of the B800 band is in nice agreement with the suggested position of the high-exciton component of the B850 band.
Acknowledgments
The research was supported by the Netherlands Foundation for Scientific Research via the Foundation for Earth and Life Sciences. M.W. received a Marie Curie Fellowship (European Commission grant ERB FMBICT 960842).
APPENDIX
According to Förster theory, the energy transfer rate k(νD, νA) from a donor D absorbing at νD to an acceptor A absorbing at νA is proportional to the overlap integral of the homogeneous emission and absorption spectra of the two pigments, eD(ν) and aA(ν), respectively:
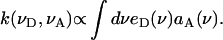 |
(5) |
We assume a Gaussian for the homogeneous absorption spectrum:
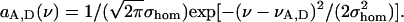 |
(6) |
σhom is the width (given as standard deviation) of the homogeneous absorption spectrum.
The emission of the donor eD(ν) at temperature T is then given by the Stepanov relation (Stepanov, 1957):
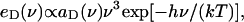 |
(7) |
giving the normalized emission spectrum (neglecting ν3):
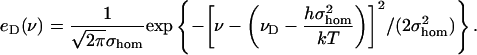 |
(8) |
This is the same Gaussian as for the absorption but shifted to the red by 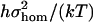 . Further we assume that the inhomogeneous distribution function IDF is given by a Gaussian of width σIDF:
. Further we assume that the inhomogeneous distribution function IDF is given by a Gaussian of width σIDF:
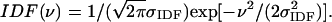 |
(9) |
Averaging the rate k(νD, νA) over all donors and acceptors gives:
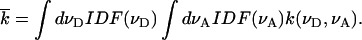 |
(10) |
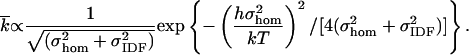 |
(11) |
References
- Agarwal, R., M. Yang, Q.-H. Xu, and G. R. Fleming. 2001. Three pulse photon echo peak shift study of the B800 band of the LH2 complex of Rps. acidophila at room temperature: a coupled master equation and nonlinear optical response function approach. J. Phys. Chem. B. 105:1887–1894. [Google Scholar]
- de Caro, C., R. W. Visschers, R. van Grondelle, and S. Völker. 1994. Inter- and intraband energy transfer in LH2-antenna complexes of purple bacteria. A fluorescence line-narrowing and hole-burning study. J. Phys. Chem. 98:10584–10590. [Google Scholar]
- Georgakopoulou, S., R. N. Frese, E. Johnson, M. H. C. Koolhaas, R. J. Cogdell, R. van Grondelle, and G. van der Zwan. 2002. Absorption and CD spectroscopy and modeling of various LH2 complexes from purple bacteria. Biophys. J. 82:2184–2197. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Herek, J. L., N. J. Fraser, T. Pullerits, P. Martinsson, T. Polívka, H. Scheer, R. J. Cogdell, and V. Sundström. 2000. B800 → B850 energy transfer mechanism in bacterial LH2 complexes investigated by B800 pigment exchange. Biophys. J. 78:2590–2596. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Hess, S., E. Åkesson, R. J. Cogdell, T. Pullerits, and V. Sundström. 1995. Energy transfer in spectrally inhomogeneous light-harvesting pigment-protein complexes of purple bacteria. Biophys. J. 69:2211–2225. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Holzwarth, A. R. 1996. Data analysis of time-resolved measurements. In Biophysical Techniques in Photosynthesis. J. Amesz, and A. J. Hoff, editors. Kluwer Academic Publishers, Dordrecht, The Netherlands. 75–92.
- Hu, X., T. Ritz, A. Damjanović, F. Autenrieth, and K. Schulten. 2002. Photosynthetic apparatus of purple bacteria. Q. Rev. Biophys. 35:1–62. [DOI] [PubMed] [Google Scholar]
- Ihalainen, J. A., J. Linnanto, P. Myllyperkiö, I. H. M. van Stokkum, B. Ücker, H. Scheer, and J. E. I. Korppi-Tommola. 2001. Energy transfer in LH2 of Rhodospirillum molischianum, studied by subpicosecond spectroscopy and configuration interaction exciton calculations. J. Phys. Chem. B. 105:9849–9856. [Google Scholar]
- Jimenez, R., S. N. Dikshit, S. E. Bradforth, and G. R. Fleming. 1996. Electronic excitation transfer in the LH2 complex of Rhodobacter sphaeroides. J. Phys. Chem. 100:6825–6834. [Google Scholar]
- Joo, T., Y. Jia, J.-Y. Yu, D. M. Jonas, and G. R. Fleming. 1996. Dynamics in isolated bacterial light harvesting antenna (LH2) of Rhodobacter sphaeroides at room temperature. J. Phys. Chem. 100:2399–2409. [Google Scholar]
- Kennis, J. T. M., A. M. Streltsov, H. Permentier, T. J. Aartsma, and J. Amesz. 1997. Exciton coherence and energy transfer in the LH2 antenna complex of Rhodopseudomonas acidophila at low temperature. J. Phys. Chem. B. 101:8369–8374. [Google Scholar]
- Koepke, J., X. Hu, C. Muenke, K. Schulten, and H. Michel. 1996. The crystal structure of the light-harvesting complex II (B800–850) from Rhodospirillum molischianum. Structure. 4:581–597. [DOI] [PubMed] [Google Scholar]
- Koolhaas, M. H. C., R. N. Frese, G. J. S. Fowler, T. S. Bibby, S. Georgakopoulou, G. van der Zwan, C. N. Hunter, and R. van Grondelle. 1998. Identification of the upper exciton component of the B850 bacteriochlorophylls of the LH2 antenna complex, using a B800-free mutant of Rhodobacter sphaeroides. Biochemistry. 37:4693–4698. [DOI] [PubMed] [Google Scholar]
- Krueger, B. P., G. D. Scholes, and G. R. Fleming. 1998a. Calculation of couplings and energy-transfer pathways between the pigments of LH2 by the ab initio transition density cube method. J. Phys. Chem. B. 102:5378–5386. [Google Scholar]
- Krueger, B. P., G. D. Scholes, and G. R. Fleming. 1998b. Calculation of couplings and energy-transfer pathways between the pigments of LH2 by the ab initio transition density cube method. J. Phys. Chem. B. 102B:9603. [Google Scholar]
- Matsuzaki, S., V. Zazubovich, N. J. Fraser, R. J. Cogdell, and G. J. Small. 2001. Energy transfer dynamics in LH2 complexes of Rhodopseudomonas acidophila containing only one B800 molecule. J. Phys. Chem. B. 105:7049–7056. [Google Scholar]
- McDermott, G., S. M. Prince, A. A. Freer, A. M. Hawthornthwaite-Lawless, M. Z. Papiz, R. J. Cogdell, and N. W. Isaacs. 1995. Crystal structure of an integral membrane light-harvesting complex from photosynthetic bacteria. Nature. 374:517–521. [Google Scholar]
- Monshouwer, R., I. Ortiz de Zarate, F. van Mourik, and R. van Grondelle. 1995. Low-intensity pump-probe spectroscopy on the B800 to B850 transfer in the light harvesting 2 complex of Rhodobacter sphaeroides. Chem. Phys. Lett. 246:341–346. [Google Scholar]
- Mukai, K., S. Abe, and H. Sumi. 1999. Theory of rapid excitation-energy transfer from B800 to optically-forbidden exciton states of B850 in the antenna system LH2 of photosynthetic purple bacteria. J. Phys. Chem. B. 103:6096–6102. [Google Scholar]
- Pullerits, T., S. Hess, J. L. Herek, and V. Sundström. 1997. Temperature dependence of excitation transfer in LH2 of Rhodobacter sphaeroides. J. Phys. Chem. B. 101:10560–10567. [Google Scholar]
- Reddy, N. R. S., G. J. Small, M. Seibert, and R. Picorel. 1991. Energy transfer dynamics of the B800–B850 antenna complex of Rhodobacter sphaeroides: a hole burning study. Chem. Phys. Lett. 181:391–399. [Google Scholar]
- Salverda, J. M., F. van Mourik, G. van der Zwan, and R. van Grondelle. 2000. Energy transfer in the B800 rings of the peripheral bacterial light-harvesting complexes of Rhodopseudomonas acidophila and Rhodospirillum molischianum studied with photon echo techniques. J. Phys. Chem. B. 104:11395–11408. [Google Scholar]
- Scholes, G. D., and G. R. Fleming. 2000. On the mechanism of light harvesting in photosynthetic purple bacteria: B800 to B850 energy transfer. J. Phys. Chem. B. 104:1854–1868. [Google Scholar]
- Stepanov, B. I. 1957. Universal relationship between absorption and luminescence spectra of complex molecules. Dokl. Acad. Nauk SSSR. 112:839–841. [Google Scholar]
- Sumi, H. 1999. Theory on rates of excitation-energy transfer between molecular aggregates through distributed transition dipoles with application to the antenna system in bacterial photosynthesis. J. Phys. Chem. B. 103:252–260. [Google Scholar]
- Sundström, V., T. Pullerits, and R. van Grondelle. 1999. Photosynthetic light-harvesting: reconciling dynamics and structure of purple bacterial LH2 reveals function of photosynthetic unit. J. Phys. Chem. B. 103:2327–2346. [Google Scholar]
- Sundström, V. 2000. Light in elementary biological reactions. Progress in Quantum Electronics. 24:187–238. [Google Scholar]
- van der Laan, H., T. Schmidt, R. W. Visschers, K. J. Visscher, R. van Grondelle, and S. Völker. 1990. Energy transfer in the B800–850 antenna complex of purple bacteria Rhodobacter sphaeroides: a study by spectral hole-burning. Chem. Phys. Lett. 170:231–238. [Google Scholar]
- van Grondelle, R., H. J. M. Kramer, and C. P. Rijgersberg. 1982. Energy transfer in the B800-850-carotenoid light-harvesting complex of various mutants of Rhodopseudomonas sphaeroides and of Rhodopseudomonas capsulata. Biochim. Biophys. Acta. 682:208–215. [Google Scholar]
- van Grondelle, R., J. P. Dekker, T. Gillbro, and V. Sundström. 1994. Energy transfer and trapping in photosynthesis. Biochim. Biophys. Acta. 1187:1–65. [Google Scholar]
- van Mourik, F., R. W. Visschers, and R. van Grondelle. 1992. Energy transfer and aggregate size effects in the inhomogeneously broadened core light-harvesting complex of Rhodobacter sphaeroides. Chem. Phys. Lett. 193:1–7. [Google Scholar]
- van Oijen, A. M., M. Ketelaars, J. Köhler, T. J. Aartsma, and J. Schmidt. 2000. Spectroscopy of individual light-harvesting 2 complexes of Rhodopseudomonas acidophila: diagonal disorder, intercomplex heterogeneity, spectral diffusion, and energy transfer in the B800 band. Biophys. J. 78:1570–1577. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Visschers, R. W., L. Germeroth, H. Michel, R. Monshouwer, and R. van Grondelle. 1995. Spectroscopic properties of the light-harvesting complexes from Rhodospirillum molischianum. Biochim. Biophys. Acta. 1230:147–154. [DOI] [PubMed] [Google Scholar]
- Wendling, M., K. Lapouge, F. van Mourik, V. Novoderezhkin, B. Robert, and R. van Grondelle. 2002. Steady-state spectroscopy of zinc-bacteriopheophytin containing LH1—an in vitro and in silico study. Chem. Phys. 275:31–45. [Google Scholar]
- Westerhuis, W. H. J., C. N. Hunter, R. van Grondelle, and R. A. Niederman. 1999. Modeling of oligomeric-state dependent spectral heterogeneity in the B875 light-harvesting complex of Rhodobacter sphaeroides by numerical simulation. J. Phys. Chem. B. 103:7733–7742. [Google Scholar]
- Wu, H.-M., S. Savikhin, N. R. S. Reddy, R. Jankowiak, R. J. Cogdell, W. S. Struve, and G. J. Small. 1996a. Femtosecond and hole-burning studies of B800's excitation energy relaxation dynamics in the LH2 antenna complex of Rhodopseudomonas acidophila (strain 10050). J. Phys. Chem. 100:12022–12033. [Google Scholar]
- Wu, H.-M., N. R. S. Reddy, R. J. Cogdell, C. Muenke, H. Michel, and G. J. Small. 1996b. A comparison of the LH2 antenna complex of three purple bacteria by hole-burning and absorption spectroscopies. Mol. Cryst. Liq. Cryst. 291:163–173. [Google Scholar]
- Wu, H.-M., M. Rätsep, I.-J. Lee, R. J. Cogdell, and G. J. Small. 1997. Exciton level structure and energy disorder of the B850 ring of the LH2 antenna complex. J. Phys. Chem. B. 101:7654–7663. [Google Scholar]
- Zazubovich, V., R. Jankowiak, and G. J. Small. 2002. On B800 → B800 energy transfer in the LH2 complex of purple bacteria. J. Lumin. 98:123–129. [Google Scholar]
- Zuber, H., and R. A. Brunisholz. 1991. Structure and function of antenna polypeptides and chlorophyll-protein complexes: principles and variability. In Chlorophylls. H. Scheer, editor. CRC Press, Boca Raton, FL. 627–703.