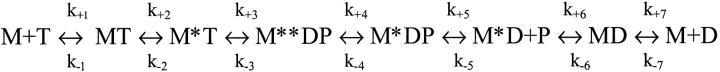Ionic Interactions Play a Role in the Regulatory Mechanism of Scallop Heavy Meromyosin

Abstract

Heavy meromyosin from scallop (scHMM) striated muscle is regulated by calcium binding to the essential light chain. The regulation can be modeled with a calcium-dependent equilibrium between on and off scHMM conformations. The observed rate constant for mant-ADP dissociation from scHMM is calcium dependent, and we show here that it can be used to define the equilibrium constant (K_eq) between on and off conformations. The data show that K_eq is markedly ionic strength dependent, with high salt (≥200 mM) abolishing the off state even in the absence of calcium and low salt (<50 mM) favoring the off state even in the presence of calcium. Debye-Huckel plots of the equilibrium constant (K_eq) for the on and off forms gave parallel slopes (5.94 ± 0.33 and 6.36 ± 0.17 M^−0.5) in the presence and absence of calcium. The presence of an equilibrium mixture of two conformations was confirmed by sedimentation data and the effects of ADP, calcium and ionic strength were in qualitative agreement. Thus scHMM exists in two conformations that can be distinguished in sedimentation profiles and by the rate of release of mant-ADP. Increasing salt concentrations biases the system toward the on state, suggesting a role for ionic interactions in stabilizing the off state.

INTRODUCTION

The regulation of molluscan myosins is achieved by the direct binding of calcium to the essential light chain of the regulatory domain (Chantler and Szent-Györgyi, 1980, 1999). Whereas both the full-length myosin and the double-headed heavy meromyosin (HMM) preserve the regulatory mechanisms, the Mg-ATPase activity of the single-headed subfragment-1 (S1) is calcium independent. Full regulation appears to require the presence of an intact junction between the two heads formed by a stable coiled-coil region and may involve direct interactions between the two heads (Kalabokis and Szent-Györgyi, 1997).

In addition to calcium, the binding of nucleotides can also play an important role in regulating the activity of scHMM heads (Kalabokis and Szent-Györgyi, 1997; Jackson and Bagshaw, 1988a; Nyitrai et al., 2002). Based on the results of detailed rapid kinetic experiments, we recently proposed that the calcium-mediated regulation of the scallop HMM could be described by a cooperative kinetic model (Nyitrai et al., 2002). The model assumes that scHMM can exist in two conformations—the on and off conformations—and that the Mg-ATPase activity of the on state is more than 50× greater than that of the off conformation. Under equilibrium conditions, the transition between the on and off states is rapid, and the dynamic equilibrium between the conformations is controlled by the binding of calcium and nucleotides. Calcium binding favors the on state, whereas the binding of ADP to the nucleotide-binding pocket shifts the equilibrium toward the off conformation. The kinetic results also suggested that in the off conformation only one of the scHMM heads is able to bind ADP.

In the absence of actin, the phosphate release is the rate-limiting step in the ATPase cycle. However, the rate constant for dissociation of the bound ADP was also found to be calcium dependent (Jackson and Bagshaw, 1988a,b; Nyitrai et al., 2002). The dissociation rate constant observed in the presence of calcium (15 s⁻¹) was reduced by a factor of ∼50 in the absence of calcium, to 0.3 s⁻¹ at 100 mM KCl. Both kinetic and equilibrium methods suggested that the interaction of scHMM with nucleotides was influenced by the ionic strength. The dissociation rate constants for ADP release measured at 100 mM KCl (0.3 s⁻¹) (Nyitrai et al., 2002) was reduced by a factor of ∼10–30 at 20 mM KCl to 0.01–0.03 s⁻¹ (Jackson and Bagshaw, 1988a,b). Similarly, the Mg-ATPase activity in the presence of calcium and the calcium sensitivity of the ATPase were found to be ionic strength dependent in steady-state assays (Kalabokis and Szent-Györgyi, 1997; Wells et al., 1985). At low ionic strength the steady-state Mg-ATPase activity of scHMM increased 10- to 15-fold upon calcium binding to the essential light chain. At very high ionic strength (0.5 M KCl), the calcium regulation was abolished, but the ATPase activity was increased relative to that at low ionic strength.

The structural differences between the on and off conformations are not understood. The interpretation of sedimentation experiments implicated two distinct structural states of the scHMM. In one of the conformations the heads were up relative to the tail, and could rotate independently of each other, whereas the other conformational state contained the heads in the down conformation (Stafford et al., 2001).

Smooth muscle myosin HMM appears to have some similarities to the regulatory mechanism of scallop HMM (Trybus, 1991) and like scHMM can bind only one ADP with high affinity in the off state (Berger et al., 2001; although see also Ellison et al., 2002). For the smooth muscle myosin, the transition from the on to the off conformation involves the formation of an asymmetric structure in which the two heads interact (Wendt et al., 2001).

In the present work we investigated the ionic strength dependence of the mechanism of regulation of scHMM. We propose that the on/off equilibrium involves charge-to-charge interactions between the two heads and is therefore sensitive to the ionic strength of the medium. In support of this hypothesis, we demonstrate that the ADP release from scHMM is ionic strength dependent and is compatible with salt affecting the equilibrium between the two conformations with very different rates of ADP release. To corroborate this argument we show that sedimentation of scHMM is consistent with an equilibrium mixture of two conformations, and the effects of ADP, calcium, and ionic strength on the equilibrium position are in qualitative agreement with the kinetic results.

MATERIALS AND METHODS

HMM preparation and characterization

HMM was prepared from 4–5 g striated scallop muscle myosin (Argopecten irradians) according to Stafford et al. (2001). The scHMM was routinely checked by measuring its Mg-ATPase activity in the absence of actin using the coupled assay method (Kalabokis and Szent-Györgyi, 1997). The turnover rate per scHMM head was in the range of 0.32–0.36 s⁻¹ in the presence of calcium and the calcium sensitivity, i.e., (ATPase^+Ca − ATPase^−Ca) × 100/ATPase^+Ca, of the preparations was between 85% and 92%. The samples used in the kinetic assays were heterogeneous, consisting of 40% short and 60% long molecules that differed in tail length by 20 nm. Differences in tail lengths did not affect ATPase activity or calcium sensitivity (Stafford et al., 2001).

Kinetic experiments

All experiments were carried out at 20°C in a standard buffer of 20 mM MOPS, pH 7.0, 5 mM MgCl₂, and either 100 μM EGTA or 100 μM CaCl₂, with additional KCl to adjust to the desired ionic strength. When measurements were recorded as a function of pCa, the calcium concentration was buffered by the addition of the appropriate amount of 2 mM EGTA and 2 mM Ca-EGTA (Harrison and Bers, 1987). Fluorescence transients were recorded with a standard Hi-Tech SF-61DX2 stopped-flow spectrophotometer. Mant-ADP fluorescence was excited at 365 nm, using a 75 W Xe/Hg lamp and monochromator. Fluorescence emission was monitored through a KV389 cutoff filter. The concentrations used to describe the experimental conditions are those established after mixing the reactants in the stopped-flow (dilution by 2, 1:1 mixing). The kinetic parameters obtained were interpreted in terms of the seven-step Bagshaw-Trentham mechanism of the myosin ATPase (see Scheme 1; see also Bagshaw and Trentham, 1974).

SCHEME 1.

The seven-step kinetic scheme for the interaction of myosin with nucleotides (Bagshaw and Trentham, 1974). In the model, the forward (k_+i) and reverse (k_−i) rate constants are presented.

The measured mant-fluorescence amplitudes (A_m) were analyzed with:

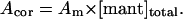(1)

The calculated amplitudes are directly proportional to the concentration of the bound mant-nucleotide (Nyitrai et al., 2002) and the corrected values (A_cor, in arbitrary units) are presented throughout the article.

Sedimentation studies

Sedimentation velocity experiments were carried out on a Beckman Instruments Optima XL-I Analytical Ultra-centrifuge equipped with Rayleigh optics and analyzed as described earlier (Stafford et al., 2001). Protein concentrations were typically in the range 0.1–1.0 mg/ml. All protein solutions were dialyzed against their respective buffers; dialysates and buffers were adjusted identically for different conditions. Runs were performed at 20°C and 50,000 rpm. Sedimentation coefficient values were taken from the peak positions of the g(s^*) plots and were corrected for density and viscosity to standard condition of water at 20°.

RESULTS

Working hypothesis

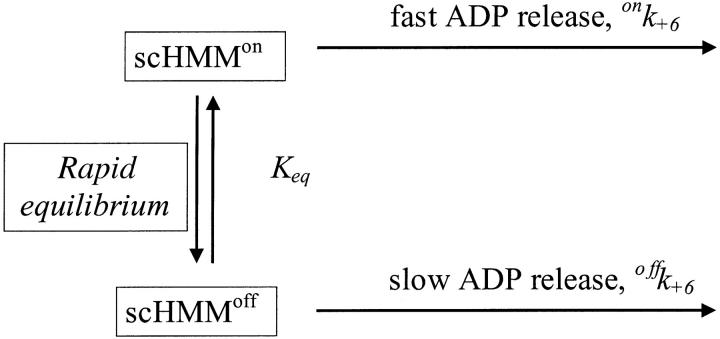
Our working hypothesis is based on measurements of the calcium dependence of the rate constant for mant-ADP dissociation from scHMM (k₊₆). This was measured previously at 100 mM KCl (Nyitrai et al., 2002) and is presented in Fig. 1. The analysis of the data with the Hill equation showed that k_obs changed between its extreme values of 0.3 s⁻¹ and 15 s⁻¹ with half-saturation at 0.78 ± 0.03 μM free calcium and a Hill coefficient of 1.9 ± 0.1. The simplest interpretation of the data is that the protein can exist in two conformations, which are in rapid equilibrium on the timescale of ADP release and that addition of calcium shifts the equilibrium between the two conformations. The off state has a slow rate constant for ADP release (^offk₊₆) and the on state a fast rate constant of ADP release (^onk₊₆) (Scheme 2).

FIGURE 1.

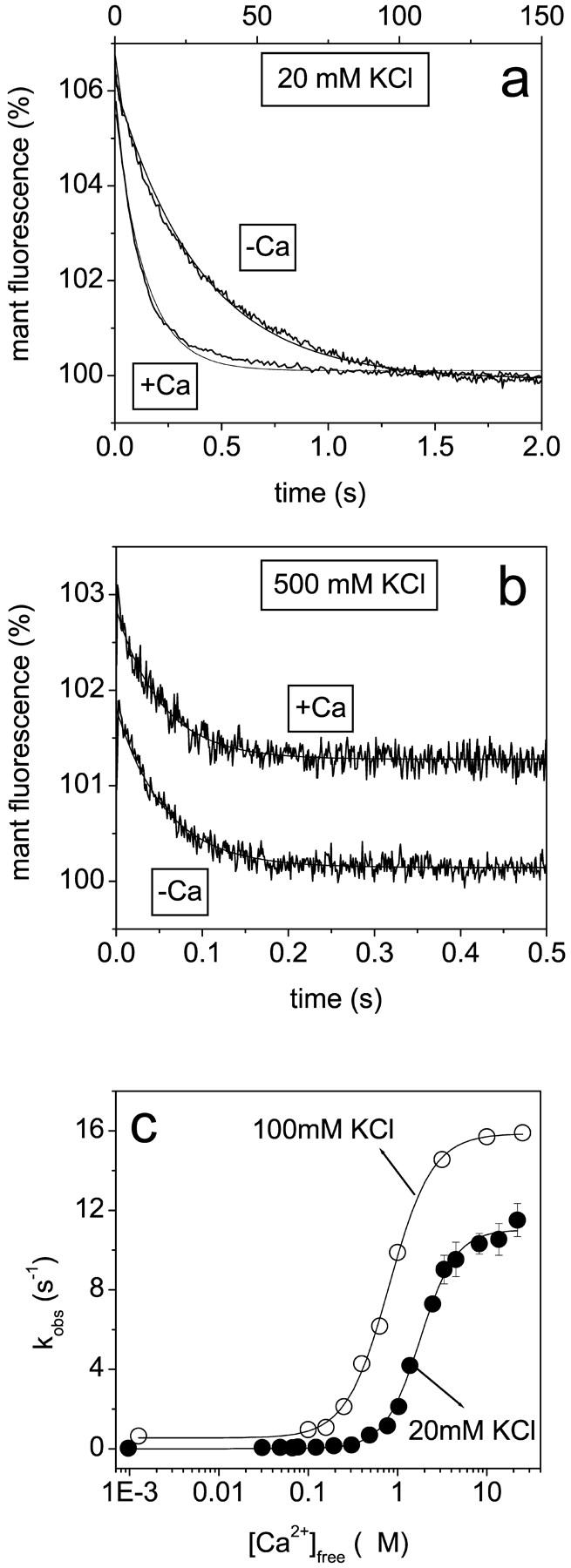
The calcium dependence of the mant-ADP dissociation rate constant. Scallop HMM (0.5 μM) and 10 μM mant-ADP were mixed with large excess of ATP (300 μM). Mant-fluorescence transients at 20 mM KCl, a, or at 500 mM KCl, b, in the presence or absence of calcium (indicated on the figures). (a) +Ca: single-exponential fit; k_obs = 7.2 s⁻¹; 5.6% amplitude. −Ca: double-exponential fit; k_obs = 8.0 s⁻¹ and 0.03 s⁻¹; 0.7% and 6.3% amplitudes. (b) +Ca: single-exponential fit; k_obs = 20.3 s⁻¹; 1.57% amplitude. −Ca: k_obs = 19.0 s⁻¹; 1.66% amplitude. The +Ca transient is shown with an offset to provide clearer presentation. (c) The calcium dependence of the mant-ADP dissociation rate constant. The solid lines represent the results of Hill analysis, which gave half-saturation calcium concentration of 1.8 ± 0.4 μM and Hill coefficient of 2.2 ± 0.7 at 20 mM KCl. The results measured at 100 mM KCl (empty circles) are from Nyitrai et al. (2002), with Hill parameters of 0.78 μM and 1.9 at 100 mM KCl.

SCHEME 2.

The kinetic scheme for the dissociation of ADP from scHMM.

In this model, the values of the observed rate constants are defined by

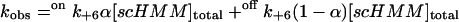(2)

and

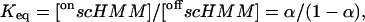(3)

where α is the fraction of the total scHMM heads in the on conformation. In support of this interpretation we previously showed that the transition between the on and off conformations in the presence of ADP occurred faster than 150 s⁻¹ (Nyitrai et al., 2002).

To test if this model was valid across a range of salt concentrations we repeated the mant-ADP dissociation experiments at 20 mM KCl and 0.5 M KCl. As in the previous work, mant-ADP (10 μM) was displaced from scHMM by addition of excess of ATP (300 μM) and the decrease in mant-fluorescence was recorded (Fig. 1, a and b). At 20 mM KCl the fluorescence decrease was well-described by a single exponential at high [calcium] but became biphasic as the [calcium] was reduced (Fig. 1 a). At low calcium the k_obs of the faster component was ∼8.0 s⁻¹ and had an amplitude of ∼15–20% of the total signal change, and both k_obs and its relative contribution to the total amplitude were independent of [calcium]. This is consistent with previous studies that showed the presence of 15–20% of unregulated HMM which behaves as though it is always on. The k_obs of the slower component was dependent upon the calcium concentration as shown in Fig. 1 c. The k_obs values at 20 mM KCl were all lower than those at 0.1 M KCl but still showed a similar calcium dependence (Fig. 1 c). Analysis by the Hill equation gave a Hill coefficient of 2.2 ± 0.7 and half-saturation at 1.8 ± 0.4 μM calcium. The cooperativity of the transition shows little change but there was a weakening of the calcium affinity from 0.78 μM at 0.1 M KCl. The model of Scheme 2 therefore remains valid at low ionic strength.

The model was not valid at high ionic strength. At 0.5 M KCl the decrease in fluorescence (1.6%) could be described by a single exponential (Fig. 1 b) and the k_obs for mant-ADP release was calcium independent (20.3 s⁻¹, +Ca; and 19.0 s⁻¹, −Ca). Thus the scHMM appears to be in the on state and removal of calcium did not produce the off conformation. This is consistent with previous studies that showed a high ATPase activity and loss of the calcium regulation at high ionic strength (Kalabokis and Szent-Györgyi, 1997). These studies also showed that calcium regulation was increased at low ionic strength but the low salt reduced ATPase activity. We proposed that the lower k_obs values for mant-ADP release at low ionic strength could represent a small shift in equilibrium toward the off state even in the presence of calcium.

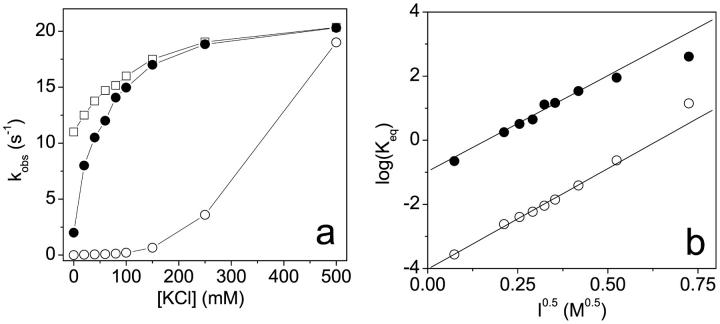
To test this idea we examined the ionic strength dependence of the k_obs values in the presence and absence of calcium. The results of such a measurement are shown in Fig. 2 a together with a similar measurement using scS1, which lacks any calcium regulation of its ATPase activity. At 0.5 M KCl the k_obs values were similar for scS1 (20.4 s⁻¹) and scHMM, and were calcium independent, again consistent with only the presence of the on state of the proteins. In the case of scS1 the k_obs value decreased as the salt concentration was reduced to a limiting value of 11 s⁻¹ at zero [KCl] indicating the intrinsic ionic strength dependence of mant-ADP release from the on state of the proteins. In the case of scHMM the k_obs values showed a more marked reduction as the ionic strength was reduced to a limiting value of 2 s⁻¹ in the presence of calcium and to 0.01–0.03 s⁻¹ in the absence of calcium for 0 mM KCl. In our working hypothesis this salt dependence is the result of the ionic strength dependence of the on-off equilibrium constant. If the model of Scheme 2 is correct, then if we know the intrinsic value of the rate constant of mant-ADP release from the on state (provided by the values for S1) and assume that the rate constant of mant-ADP release from the off state is negligible, we can calculate the value of K_eq (Eq. 1 and 2). This was done for the data both in the presence and absence of calcium and the results are presented in a Debye-Huckel plot in Fig. 2 b. The Debye-Huckle plot shows the +Ca and −Ca data as two almost parallel lines with slopes of 5.94 ± 0.33 and 6.36 ± 0.17 M^−0.5, respectively. This is consistent with a common mechanism for the effect of salt in the presence and absence of calcium. The limiting values of the equilibrium constant (K_eq) at zero ionic strength are 0.14 in the presence of calcium and 10⁻⁴ in the absence of calcium. The calculated values of K_eq at 20 mM KCl and 0.1 M KCl are given in Table 2.

FIGURE 2.

The ionic strength dependence of the mant-ADP dissociation rate constant. Scallop HMM (0.5 μM) or scS1 (0.5 μM) and 10 μM mant-ADP were mixed with ATP (300 μM) at different [KCl]. (a) Dependence of k_obs values on the [KCl] for scS1 (empty squares) and scHMM in the presence (filled circles) or absence (empty circles) of calcium. (b) The Debye-Huckel plots (log(K_eq) vs. I^0.5) in the presence (filled circles) and absence (empty circles) of calcium. The slopes were 5.94 ± 0.33 and 6.36 ± 0.17 M^−0.5 and the value of K_eq at infinite dilution was found to be 0.14 and 10⁻⁴ in the presence and absence of calcium, respectively.

TABLE 2.

The summary of equilibrium association constants defining the equilibrium between the on and off conformations of scHMM in the presence and absence of calcium

		Value
Conditions	Parameter	+Ca2+	−Ca2+
100 mM KCl	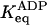*	15 (94% on)	10−2 (<1% on)
	Keq†	v. large (>99% on)	∼2 (∼67% on)
20 mM KCl	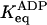*	2 (67% on)	2 × 10−3 (<0.1% on)
	Keq†	20 (>94% on)	∼2 (∼67% on)

^*Data from the analysis of mant-ADP replacement with ATP experiments.

^†Estimations from the amplitudes measured in mant-ADP binding experiments. K_eq = [on]/[off], in the absence (K_eq) or presence 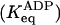of ADP. The contribution of on heads to the total scHMM population was calculated from K_eq as: on/(on + off) = K_eq/(1 + K_eq).

The on/off equilibrium in the absence of ADP

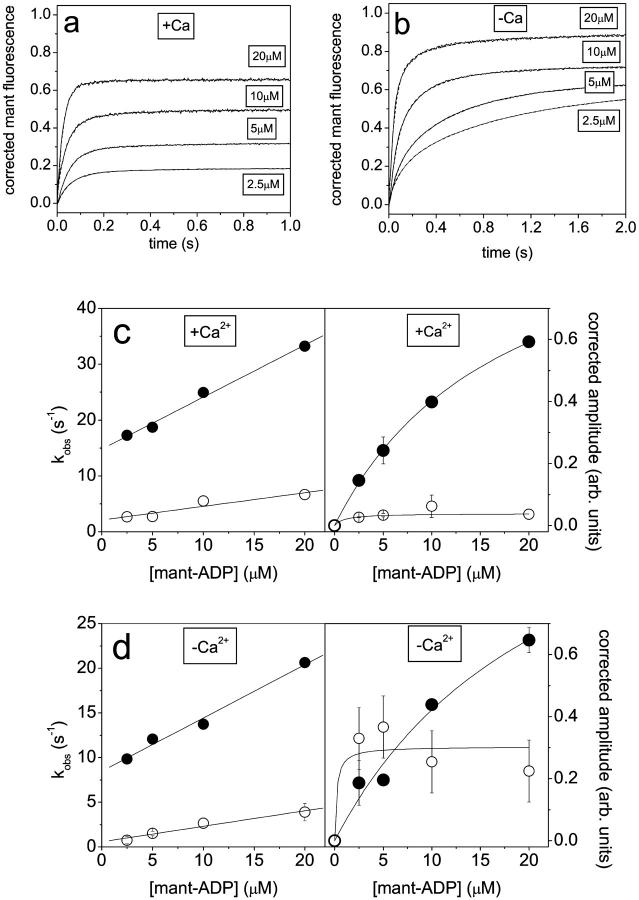
In our previous study we demonstrated that the rate of mant-ADP binding to scHMM could be used to estimate the fraction of scHMM in the off and on conformations in nucleotide-free states. The binding of mant-ADP to scHMM can be followed by the increase of mant-fluorescence. At 100 mM KCl the observed reaction for mant-ADP binding to scHMM was single exponential in the presence of calcium consistent with a single population of on state heads. In the absence of calcium the transients were described by two exponentials. The faster phase was attributed to the scHMM in on conformation, whereas the slower phase reflects the binding of mant-ADP to the off heads. The ratio of the two forms was estimated to be 7:4 in favor of the on conformation from amplitudes. This experiment was repeated here in 20 mM KCl. Fig. 3, a and b, show the mant-fluorescence traces obtained in the experiments, which were two-exponential in either the presence or absence of calcium, suggesting the presence of both on and off conformations at 20 mM KCl even in the presence of calcium. The results of the two-exponential fits are presented in Fig. 3, c and d.

FIGURE 3.

The binding of mant-ADP to scHMM in the presence and absence of calcium. The mant-fluorescence changes on mixing scHMM (0.5 μM) with mant-ADP (2.5–20 μM) in the presence (a), or absence (b), of calcium at 20 mM KCl. The mant-intensities were corrected using F_corr = (F_m − F_i)/[mant], where F_m and F_i are the measured and initial intensities. Double-exponential fits are presented as dashed lines in the figure. The mant-ADP dependence of k_obs and amplitudes in the presence, c, and absence, d, of calcium. Filled and empty circles show the data obtained for the fast and slow phase, respectively. (c) +Ca: linear fits to k_obs values gave slopes of 9 × 10⁵ M⁻¹s⁻¹ and 2.4 × 10⁵ M⁻¹s⁻¹, with intercepts of 15 s⁻¹ and 2 s⁻¹. Hyperbola fits to the amplitudes gave K_0.5 of 17.9 μM and <2 μM and A_max of 1.1 and 0.05. (d) −Ca: slopes were 6 × 10⁵ M⁻¹s⁻¹ and 2 × 10⁵ M⁻¹s⁻¹ with intercepts of 8.5 s⁻¹ and 1 s⁻¹. Hyperbola fits to the amplitudes gave K_0.5 of 20 μM and <2.5 μM, and A_max of 1.2 and 0.3.

In the presence of calcium the k_obs vs. [mant-ADP] plot was linear for both phases and gave an apparent second-order rate constant of 9 × 10⁵ M⁻¹s⁻¹ for the faster phase and 2.4 × 10⁵ M⁻¹s⁻¹ for the slower phase (Fig. 3 c). The intercept was ∼15 s⁻¹ for the faster phase and 2 s⁻¹ for the slower phase, although this latter value was not well-defined. The mant-ADP concentration dependence of the amplitudes was fitted to hyperbolae and gave half-saturation at 18 μM mant-ADP for the faster phase. The half-saturation mant-ADP concentration gives an estimation of the dissociation equilibrium constant for ADP binding to the on state (K₆K₇). For the slower phase, half-saturation occurred below 2 μM mant-ADP, giving an upper limit for K₆K₇ for the off state. The maximum amplitudes values of 1.1 and ∼0.03 were resolved for the faster and slower phases, respectively. At saturation the maximum amplitudes are proportional to the bound [mant-ADP] and the fast and slow phase amplitudes provide an estimate of the contribution of on and off states after correcting for the observation that scHMM can bind two mant-ADP in the on, and only one mant-ADP in the off conformation (see Fig. 5, and Nyitrai et al., 2002). Although the presence of scHMM heads in the off conformation was detected in these experiments, the maximum amplitudes indicate that besides the ∼15–20% of unregulated scHMM, 6% of the heads are off and most of the heads (∼74–79%) are on in the absence of ADP. At 100 mM KCl the presence of off heads was not detected in the presence of calcium previously—which suggests that the decrease of the ionic strength had only a small effect on the on-off equilibrium.

FIGURE 5.

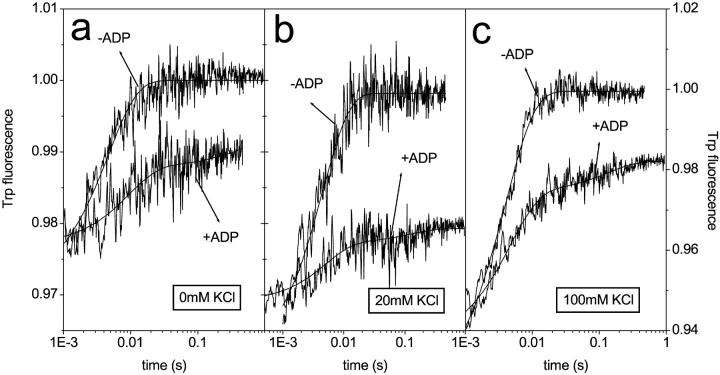
Tryptophan signals on the displacement of ADP by ATP in the absence of calcium. Scallop HMM (0.5 μM) and ±7.5 μM ADP were mixed with ATP (300) at 0 mM (a), 0 mM (b), and 100 mM (c) KCl. In the absence of ADP the traces were single exponential with amplitudes and k_obs values of 2.5%, 3.0%, and 7.5%, and 180 s⁻¹, 210 s⁻¹, and 250 s⁻¹ at 0 mM, 20 mM, and 100 mM KCl, respectively. In the presence of ADP, the traces were double exponential. The fast phases gave amplitudes and k_obs values of 1.1%, 1%, and 3.7%, and 160 s⁻¹, 220 s⁻¹, and 220 s⁻¹, respectively. The amplitude of the slow phase was small (<1%) at all ionic strengths, and determination of k_obs values was not possible.

In the absence of calcium (Fig. 3 d) k_obs vs. [mant-ADP] plots were linear and gave apparent second-order binding constants (k₋₆/K₇) of 6 × 10⁵ M⁻¹s⁻¹ and 2 × 10⁵ M⁻¹s⁻¹ for the fast and slow phases, respectively, similar to the values found in the presence of calcium. The intercept (k₊₆) was 8.5 s⁻¹ in the fast phase and <1 s⁻¹ but not well-determined for the slow phase. Hyperbolic analysis of the amplitudes indicated that half-saturation occurred at ∼20 μM mant-ADP for the faster phase, which gave an estimation of K₆K₇ for the on heads. In the slower phase the half-saturation mant-ADP concentration was 2.5 μM, giving an upper limit of K₆K₇ for the off heads. Hyperbola fits gave maximum amplitudes of 1.3 for the faster phase and 0.3 for the slower one. These maximum amplitudes suggest that approximately one-third of the scHMM heads were off in the absence of calcium, i.e., ∼67% of the heads were on, when correction was made for unregulated heads. A similar contribution of the on heads were determined at 100 mM KCl (64%), which indicates that the change of ionic strength has little effect on the on-off equilibrium in the absence of calcium and ADP.

The dissociation equilibrium constant of mant-ADP for scHMM (K₆K₇) at 20 mM KCl can be estimated by calculating the ratio of the dissociation rate constants (k₊₆; Fig. 1) to the second-order binding constants (k₋₆/K₇; Fig. 3). The affinity (K₆K₇) of mant-ADP for the scHMM on heads is determined from the fast phase to be ∼14–21 μM at 20 mM KCl, in agreement with the half-saturation mant-ADP concentrations (18 μM from Fig. 3 c and 20 μM from Fig. 3 d) for the faster phase in mant-ADP binding experiments and similar to that measured at 100 mM KCl (15 μM; see Table 1.) (Nyitrai et al., 2002). For the off heads a similar calculation gave dissociation equilibrium constant of ∼0.1 μM at 20 mM KCl, 140–210× tighter than that determined for the on heads. This value for K₆K₇ is in agreement with the half-saturation mant-ADP concentration determined for the slow phases in mant-ADP binding experiments (Fig. 3, c and d).

TABLE 1.

The comparison of kinetic rate and equilibrium binding constants determined experimentally at 20 mM and 100 mM KCl for the on and off conformations of scHMM heads

			Value
Conditions	Parameter	Units	onHMM	offHMM	scS1*
100 mM KCl	k−6/K7	106 M−1s−1	1	0.5–0.8	1.5
	k+6	s−1	15	0.3–0.7	17
	K6K7	μM	15	1	11
20 mM KCl	k−6/K7†	106 M−1s−1	0.6–0.9	0.2–0.3	–
	k+6	s−1	12.5‡	0.02–0.03	12.5‡
	K6K7§	μM	15–20	0.1	–

The data at 100 mM KCl is from the previous work (Nyitrai et al., 2002).

^*The data for scS1 were cited from Kurzawa-Goertz et al. (1998).

^†From mant-ADP binding experiments.

^‡From mant-ADP dissociation from scS1 experiments (Fig. 1). The intercepts in mant-ADP binding plots were in agreement with these data giving 8.5–15 s⁻¹ and less than ∼1 s⁻¹ for the on and off forms, respectively.

^§Calculated as k₊₆/k₋₆/K₇.

NB Plots of k_obs for fast and slow phase are similar +/− Ca, validating that the properties of the on and off states are independent of calcium but the occupancy of the two states varies with calcium.

The ionic strength dependence of the sedimentation of scHMM

Previous studies have shown that the sedimentation velocity of scHMM is sensitive to the conformations of scHMM and is calcium sensitive (Stafford et al., 2001). In the absence of calcium scHMM adopts a compact structure leading to fast sedimentation. In the presence of calcium, the sedimentation was slower than in EGTA due to a more extended conformation attributed to the independent rotation of the two heads. To corroborate the conclusions from kinetic experiments we used the method of analytical ultracentrifugation to determine the sedimentation of scHMM over a range of ionic strengths in either the presence or absence of calcium and ADP. The results obtained in these experiments are shown in Fig. 4. The appearance of a single peak at each ionic strength (Fig. 4 a) suggests that the on and off conformations of the scHMM are in a rapid equilibrium during the time course of the sedimentation experiment. This conclusion is in agreement with the rapid kinetic results (Nyitrai et al., 2002). The peaks are not single Gaussians since the scHMM preparation consists of a nearly equal ratio of short and long scHMM molecules differing in their tail portion by 20 nm. The peak value reflects the weight average value for the mixture. A reduction in ionic strength results in a faster sedimentation of scHMM under all conditions. The ionic strength dependence of the sedimentation peaks position in the presence or absence of ADP is shown in Fig. 4, b and c. The dependence of s_peak on the square root of ionic strength is almost linear for the data in the presence of both ADP and calcium (Fig. 4 b). The identical line is drawn on Fig. 4 c to aid comparison between the two data sets.

FIGURE 4.

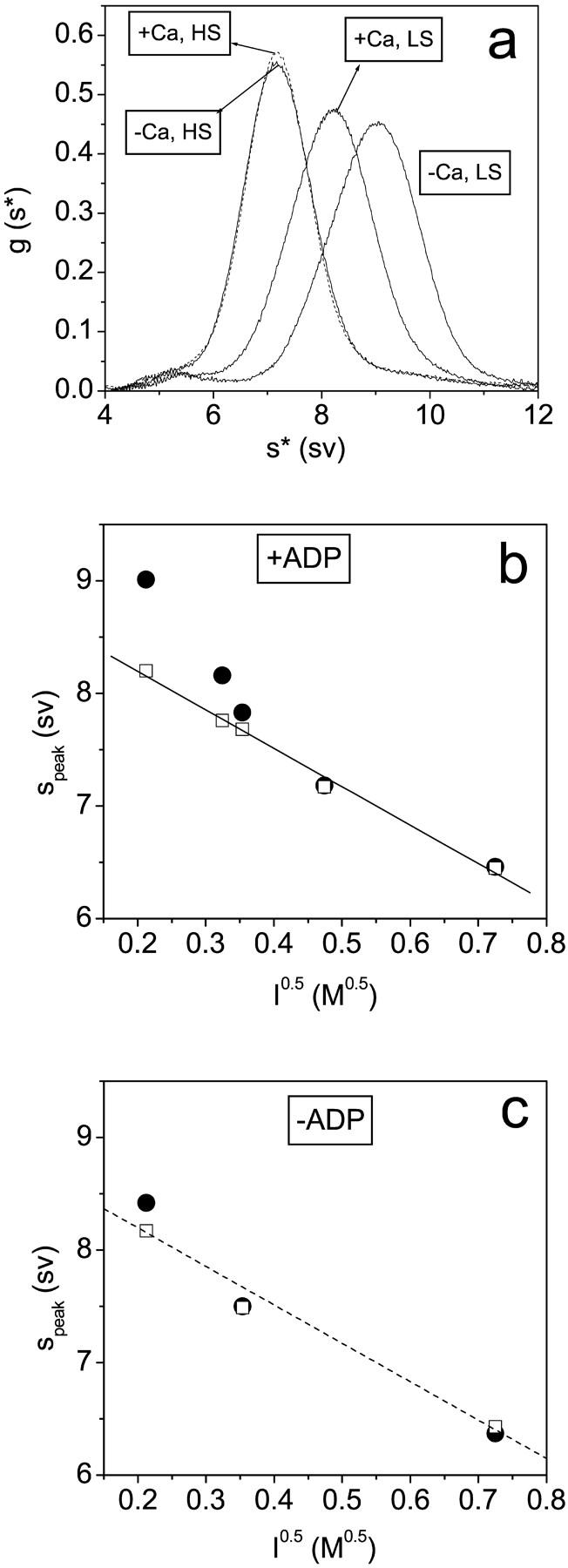
The ionic strength dependence of the sedimentation of scHMM. (a) Sedimentation results in the presence of 100 μM ADP with and without 100 μM calcium (as indicated) at 20 mM NaCl (LS) and at 200 mM NaCl (HS). (b) The dependence of sedimentation on √(ionic strength) in 100 μM ADP with a best fit line drawn through the data in the presence of calcium. (c) Data in the absence of nucleotides with the line reproduced from b to aid comparison. The peak positions are shown in the presence (empty squares) and absence (filled circles) of calcium (100 μM). Protein concentrations were typically in the range 0.1–1.0 mg/ml. No concentration dependence of the sedimentation patterns was seen, indicating the absence of any reversible aggregation.

If ADP is bound to scHMM at low salt in the presence of calcium, the sedimentation is slower than that in the absence of calcium (Fig. 4, a and b). As the salt concentration is increased, this difference is reduced and disappears at ionic strengths >0.2 M. This is consistent with loss of the off state at higher ionic strengths. In the absence of ADP, calcium has little effect on the sedimentation at any ionic strength (Fig. 4 c), consistent with the off state requiring the presence of nucleotide.

At both 100 and 20 mM NaCl the binding of ADP to scHMM shifted the peak positions toward greater values, indicating a more compact structure, in the absence of calcium but not in its presence (Fig. 4 b). This is consistent with the kinetic data which shows that in the absence of calcium ADP addition results in a shift toward the more compact, faster sedimenting off state. In the presence of calcium the addition of ADP has little effect on the sedimentation (Fig. 4, b and c). This is consistent with the kinetic analysis where the system is substantially in the on state in the presence of calcium, independent of the presence of ADP, and independent of ionic strength. The salt concentrations in sedimentation and kinetic experiments were adjusted with NaCl and KCl, respectively. We repeated the rapid kinetic measurements in the presence of NaCl and the results showed that the rate constants for mant-ADP dissociation from scHMM were independent of the nature of the salt at either 20 mM salt or 100 mM salt, and in either the presence or absence of calcium.

The ionic strength dependence of the kinetics of the dissociation of calcium from scHMM in the absence of nucleotides

The affinity of the calcium for scHMM depends on both the binding and dissociation kinetics. The determination of the binding of calcium and its dissociation in the presence of ADP was not possible as these processes are too fast to be measured in a stopped-flow experiment. The kinetics of calcium release in the absence of ADP, however, proved to be slower and could be determined by monitoring Trp fluorescence (Jackson and Bagshaw, 1988b; Nyitrai et al., 2002). As these two studies were carried out on different scallop species the direct comparison of the data obtained at 20 mM KCl (25 s⁻¹ from Jackson and Bagshaw, 1988b) and at 100 mM KCl (75 s⁻¹ from Nyitrai et al., 2002) is not appropriate. Therefore, in this study experiments were carried out at different KCl concentrations (20 mM, 60 mM, 100 mM, and 500 mM) using Trp fluorescence to provide information regarding the ionic strength dependence of the calcium release step. When scHMM (0.5 μM) and calcium (10 μM) was mixed with a large excess of EGTA (500 μM) the fluorescence of Trp decreased by ∼3% regardless of the ionic strength. The traces (not shown) were single exponential and gave apparently KCl-independent k_obs values between 50 s⁻¹ and 80 s⁻¹.

The displacement of ADP with ATP

It was shown previously that only one of the scHMM heads could bind ADP in the absence of calcium at 100 mM KCl. This property of scHMM was observed when ADP was displaced with ATP and the changes in Trp fluorescence were recorded. The appearance of a fast component showed that even at 40 μM ADP (∼20× of the K₆K₇) approximately half of the heads bound ATP very quickly, indicating that they did not bind ADP. To gain further information about this specific behavior of scHMM the ADP displacement experiments were repeated at lower KCl concentrations (0 mM or 20 mM KCl) where the binding of ADP to scHMM is expected to be tighter. In the absence of ADP, the fluorescence transients were single exponential and gave amplitudes of 2.5% and 3.6% at 0 mM and 20 mM KCl, respectively (Fig. 5, a and b). These values are a factor of 2–3 smaller than the one (7.5%) measured at 100 mM KCl (Fig. 5 c). The resolved k_obs values were 180 s⁻¹, 210 s⁻¹, and 250 s⁻¹ at 0 mM KCl, 20 mM KCl, and 100 mM KCl, respectively, and were attributed to the fast binding of ATP to the nucleotide pocket. When scHMM was incubated with 15 μM ADP (more than 10× that of the K₆K₇, Table 1) before mixing with ATP, the traces were double exponential. The slow phase in these transients represents scHMM heads with bound ADP, which must dissociate from the heads before ATP binds. The amplitude of these slow components were <1%, which is consistent with the fact that the fluorescence changes are similar for the binding of ATP and ADP, and thus the replacement of ADP with ATP contributes little to the total fluorescence change. Fast phases were observed in the presence of ADP regardless of the KCl concentration (Fig. 5). The resolved k_obs values were 160 s⁻¹, 220 s⁻¹, and 220 s⁻¹ at 0 mM, 20 mM, and 100 mM KCl, similar to those measured in the absence of ADP. The fast phases therefore represent the scHMM heads with no bound ADP. The amplitudes of these fast phases were 1.1%, 1.0%, and 3.7% at 0 mM, 20 mM, and 100 mM KCl, respectively, with 44%, 28%, and 50% of the fast phase measured in the absence of ADP. Although the error attributed to the determination of the amplitudes is greater at lower ionic strength (0 mM or 20 mM KCl), these values are compatible with the observation that approximately half of the scHMM cannot bind ADP in the absence of calcium.

DISCUSSION

The data present here are all consistent with our previous proposal (Nyitrai et al., 2002) that scHMM exists in two conformations off and on, and that the equilibrium position between the two conformations is modulated by both calcium and ADP. In addition, we show here that the equilibrium is also influenced by ionic strength suggesting a major role for ionic interactions in stabilizing the off conformation. We have presented two lines of evidence to support this view from kinetic and sedimentations studies.

The kinetic evidence is based on the observation that the affinity of scHMM for mant-ADP (K₆K₇) is calcium dependent, and that this is due to a calcium-dependent rate constant for ADP release (k₊₆). Analysis of the k_obs for mant-ADP release as a function of calcium and ionic strength were interpreted in terms of the model shown in Scheme 2 and the results of this analysis are summarized in Tables 1 and 2.

The calcium dependence of the k_obs for ADP displacement shows the classic behavior for a cooperative switching system where the binding of two calcium ions are required to switch between the high and low ADP affinity states (Fig. 1 c). This cooperative switching behavior is apparent at all ionic strengths <0.2 M; above this ionic strength, the switching is no longer apparent and the scHMM is in the low ADP affinity on state independent of calcium. The calcium affinity (0.78 to 1.8 μM from 0.1 to 0.02 M KCl) and the Hill coefficient (1.9–2.2) show little ionic strength dependence within the limits of the measurements. The calcium and salt dependence of the ADP release rate constant can be explained by the model shown in Scheme 2. The detailed interpretation in terms of Scheme 2 depends upon the assumption that behavior of scS1 provides a reasonable model for the intrinsic salt dependence of the ADP release rate constant from the scHMM^on. The k_obs for ADP release from S1 showed only a twofold reduction as the [KCl] was reduced from 0.5 M to 0 M, indicating a minor dependence upon ionic strength. In contrast, the scHMM showed an almost 10-fold reduction in the presence of calcium and a 1000-fold reduction in the absence of calcium. Both reductions can be an indication of increased occupancy of the off state as the ionic strength is reduced.

Interpretation of the data terms of Scheme 2 and Eqs. 2 and 3 suggests that the reduction in the k_obs values with reduced calcium concentrations and reduced ionic strength is due to a combination of increasing occupancy of the off conformation and the lower value of ^offk₊₆ in the off state. If ^offk₊₆ has the same low salt dependence as the k₊₆ value for scS1 (twofold) then the limiting value of ^offk₊₆ is 0.01 s⁻¹. It remains possible, however, that ADP is not released at all from the off state, and that ^offk₊₆ is zero. In this case, Eq. 2 becomes

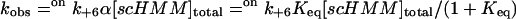(4)

Either interpretation of the model suggests that the effects of both calcium and salt are primarily on the equilibrium between the on and off states in the presence of ADP, with both low calcium and low salt favoring the off state. The influence of low calcium is reduced at high salt concentrations and reciprocally the influence of salt is diminished in the presence of calcium. These effects are only clearly seen in the presence of ADP.

The conformation of the on and off states can also be followed by sedimentation studies. Previous work has shown that in the presence of ADP or ATP analogs but no calcium, scHMM takes up a more compact structure and sediments faster than in the state induced by calcium (Stafford et al., 2001). We show here that in the presence of ADP, the calcium-induced difference in sedimentation is greater at low salt concentration and disappears at ionic strengths exceeding 0.2 M (Fig. 4 c). This is consistent with the estimates of the equilibrium between on and off states in Table 2. Scallop HMM sediments always as a single peak at different salt concentrations indicating that the on and off conformations are in an ionic strength-dependent equilibrium. There is also a marked salt dependence of sedimentation independent of the on/off equilibrium position shown most markedly in the −ADP +Ca data which is expected to be >95% on at all salts conditions except the very lowest. The relationship between S and I ^0.5 was almost linear under these conditions and ADP had little effect, except at the lowest ionic strengths used—again consistent with the K_eq values in Table 2.

This marked ionic strength dependence of sedimentation properties under conditions where the scHMM is expected to be predominantly on suggest that the state cannot be associated with precise structural states. It is consistent with significant conformational heterogeneity in the on state which is sensitive to the ionic strength. This may be expected for the on state which is thought to be one in which the two heads have independent movement, and therefore disorder, around the head-S2 junction. Such heterogeneity is not expected for an off state in which the two heads are docked together onto the myosin tail. The marked salt-dependent behavior of the sedimentation of the on state caused by changes in the conformational heterogeneity may be larger than subtle changes in the on/off equilibrium under some conditions. This precludes a more detailed comparison of the sedimentation and kinetic data. The overall conclusion is that both the kinetic and sedimentation data are consistent with a dynamic Ca-dependent equilibrium between two primary conformations of scHMM with the equilibrium position being influenced by salt and nucleotide. Low calcium, ADP, and low salt all favor the off conformation. The kinetic data allow the best definition of the equilibrium constant in the presence of ADP at salt concentration <0.1 M. No off state is detected at salts >0.2 M independent of calcium, and little on state is detected at low salt in the absence of Ca.

The evidence for on and off states in the absence of ADP is not as clear in the presence of ADP and relies on estimates of the relative amplitudes of the two phases in the mant-ADP binding data which are Ca dependent. This interpretation in terms of two populations of on and off states requires that the two states are kinetically distinct populations on the timescale of mant-ADP binding and therefore the on/off equilibration must be slower than the rate of mant-ADP binding, i.e., in the absence of ADP the on/off equilibration is slower than in the presence of ADP. In support of a difference in the equilibrium behavior in the absence of nucleotide is the observation that both the sedimentation and the mant-ADP binding data are consistent with a much smaller influence of salt on the on/off equilibrium in the absence of nucleotide.

All of the data presented here are consistent with our previous observation that the HMM^off binds only a single ADP. The off state therefore must have an asymmetric structure as reported for smHMM in both kinetic studies (Berger et al., 2001) and in EM images of smHMM and myosin (Wendt et al., 2001). There is nothing in our sedimentation data which rules out the type of structure reported by Wendt et al. (2001) for smHMM, but other asymmetric structural models remain possible for scHMM. Whatever the structure of the off state, it must account for the observation that only a single head can bind ADP.

A significant observation is that the off state, discussed here, is a characteristic of regulated myosins, and requires the interaction between two heads of myosin. Sedimentation data are consistent with a model where the two heads interact with each other and form a rigid structure. In case of scallop HMM and myosin, calcium disrupts the interaction between the heads which become mobile and can enter the ATPase cycle (Stafford et al., 2001).

The nature and dynamics of the off conformation

Atomic models from crystallographic studies suggest that there is direct coupling between the conformation of the structural element known as switch II which contacts the P_i of ATP, the converter domain and the orientation of the light-chain-binding domain (or tail or lever arm). For convenience this overall conformational switch is here referred to as the switch II open/closed conformation change. The structures determined in different nucleotide states (Fisher et al., 1995; Smith and Rayment, 1996; Dominguez et al., 1998; Geeves and Holmes, 1999; Houdusse et al., 1999; Bauer et al., 2000) suggested that hydrolysis of ATP on myosin only happens in the closed conformation of the motor domain, while the power stroke and the associated P_i and ADP release require the structure to return to the open conformation. If the off state involves a “docking” of one head of HMM onto the partner head (as in Wendt et al., 2001), or one or both heads onto S2, this would restrict the ability of the head to go through the major conformational changes associated with the open/closed tail swing and therefore would produce an inhibition of the cycle. For some myosins, notably smooth muscle myosin, there is an additional movement of the light-chain-binding domain (LCBD) on binding and release of ADP (Whittaker et al., 1995; Jontes et al., 1995). The potential for a direct communication between the regulatory LCBD and nucleotide therefore clearly exists. Any change in the LCBD which restricts the movement of the LCBD relative to the converter domain therefore has the potential to influence the open-closed transition and ATP hydrolysis and the overall cycle.