Skip to main content
PMC home page
Biophysical Journal logoLink to Biophysical Journal
. 1974 Dec;14(12):923–931. doi: 10.1016/S0006-3495(74)85959-X

Bending Resistance and Chemically Induced Moments in Membrane Bilayers

E A Evans
PMCID: PMC1334588  PMID: 4429770

Abstract

Pure bending of a membrane bilayer is developed including different properties for each membrane half. Both connected and unconnected bilayer surfaces are treated. The bilayer bending resistance is the resultant of parallel surface compression “resistances.” The neutral surface is a function of the upper and lower surface compressibility moduli and does not necessarily coincide with the mid-surface. Alterations in the interfacial chemical free energy density (surface tension) on either face can create induced bending moments and produce curvature; even small changes can have a pronounced curvature effect. Chemically induced moments are considered as a possible mechanism for crenation of red blood cells.

Full text

PDF
923

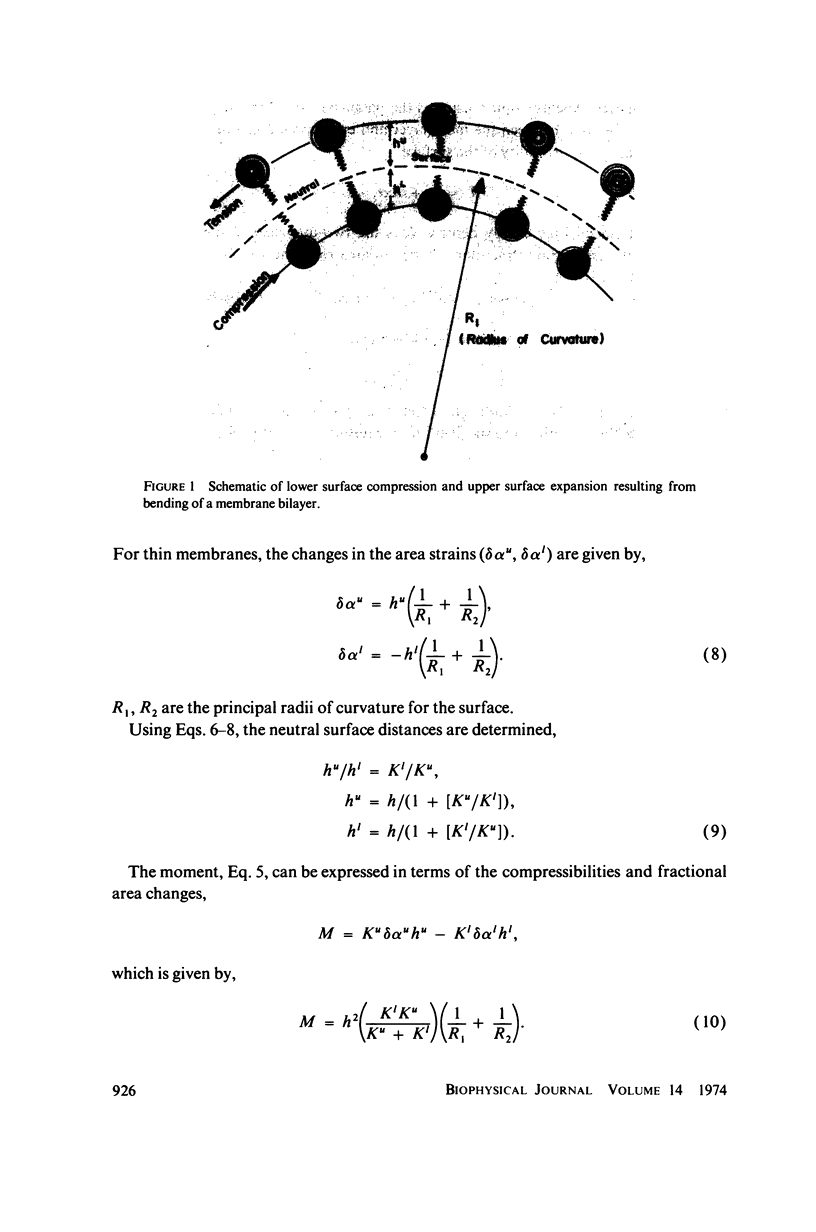
Selected References

These references are in PubMed. This may not be the complete list of references from this article.

  1. Bretscher M. S. Membrane structure: some general principles. Science. 1973 Aug 17;181(4100):622–629. doi: 10.1126/science.181.4100.622. [DOI] [PubMed] [Google Scholar]
  2. Canham P. B. The minimum energy of bending as a possible explanation of the biconcave shape of the human red blood cell. J Theor Biol. 1970 Jan;26(1):61–81. doi: 10.1016/s0022-5193(70)80032-7. [DOI] [PubMed] [Google Scholar]
  3. Evans E. A. A new material concept for the red cell membrane. Biophys J. 1973 Sep;13(9):926–940. doi: 10.1016/S0006-3495(73)86035-7. [DOI] [PMC free article] [PubMed] [Google Scholar]
  4. Fung Y. C. Theoretical considerations of the elasticity of red cells and small blood vessels. Fed Proc. 1966 Nov-Dec;25(6):1761–1772. [PubMed] [Google Scholar]
  5. Hochmuth R. M., Mohandas N., Blackshear P. L., Jr Measurement of the elastic modulus for red cell membrane using a fluid mechanical technique. Biophys J. 1973 Aug;13(8):747–762. doi: 10.1016/S0006-3495(73)86021-7. [DOI] [PMC free article] [PubMed] [Google Scholar]
  6. Hochmuth R. M., Mohandas N. Uniaxial loading of the red-cell membrane. J Biomech. 1972 Sep;5(5):501–509. doi: 10.1016/0021-9290(72)90007-3. [DOI] [PubMed] [Google Scholar]
  7. Lew H. S. Electro-tension and torque in biological membranes modeled as a dipole sheet in fluid conductors. J Biomech. 1972 Jul;5(4):399–408. doi: 10.1016/0021-9290(72)90067-x. [DOI] [PubMed] [Google Scholar]
  8. Mateu L., Luzzati V., London Y., Gould R. M., Vosseberg F. G. X-ray diffraction and electron microscope study of the interactions of myelin components. The structure of a lamellar phase with a 150 to 180 A repeat distance containing basic proteins and acidic lipids. J Mol Biol. 1973 Apr 25;75(4):697–709. doi: 10.1016/0022-2836(73)90302-1. [DOI] [PubMed] [Google Scholar]
  9. RAND R. P., BURTON A. C. MECHANICAL PROPERTIES OF THE RED CELL MEMBRANE. I. MEMBRANE STIFFNESS AND INTRACELLULAR PRESSURE. Biophys J. 1964 Mar;4:115–135. doi: 10.1016/s0006-3495(64)86773-4. [DOI] [PMC free article] [PubMed] [Google Scholar]
  10. RAND R. P. MECHANICAL PROPERTIES OF THE RED CELL MEMBRANE. II. VISCOELASTIC BREAKDOWN OF THE MEMBRANE. Biophys J. 1964 Jul;4:303–316. doi: 10.1016/s0006-3495(64)86784-9. [DOI] [PMC free article] [PubMed] [Google Scholar]
  11. Skalak R., Tozeren A., Zarda R. P., Chien S. Strain energy function of red blood cell membranes. Biophys J. 1973 Mar;13(3):245–264. doi: 10.1016/S0006-3495(73)85983-1. [DOI] [PMC free article] [PubMed] [Google Scholar]
  12. Tardieu A., Luzzati V., Reman F. C. Structure and polymorphism of the hydrocarbon chains of lipids: a study of lecithin-water phases. J Mol Biol. 1973 Apr 25;75(4):711–733. doi: 10.1016/0022-2836(73)90303-3. [DOI] [PubMed] [Google Scholar]

Articles from Biophysical Journal are provided here courtesy of The Biophysical Society

RESOURCES