The Collapse of Monolayers Containing Pulmonary Surfactant Phospholipids Is Kinetically Determined

Abstract

Prior studies have shown that during and after slow compressions of monomolecular films containing the complete set of purified phospholipids (PPL) from calf surfactant at an air/water interface, surface pressures (π) reach and sustain values that are remarkably high relative to expectations from simple systems with model lipids. Microscopy shows that the liquid-expanded, tilted-condensed, and collapsed phases are present together in the PPL films between 45 and 65 mN/m. The Gibbs phase rule restricts equilibrium coexistence of three phases to a single π for films with two components but not for more constituents. We therefore determined if the surprising stability of PPL reflects release from the thermodynamic restrictions of simple model systems by the presence of multiple components. Experiments with binary films containing dioleoyl phosphatidylcholine and dipalmitoyl phosphatidylcholine first tested the predictions of the phase rule. The onset of three-phase coexistence, determined by fluorescence microscopy, and its termination, established by relaxation of collapsing films on a captive bubble, occurred at similar π. Experiments for PPL using the same methods suggested that the three phases might coexist over a range of π, but limited to ∼2 mN/m, and extending below rather than above the coexistence π for the binary films. Our results show that the PPL films at high π must deviate from equilibrium and that they must then be metastable.

INTRODUCTION

Films of pulmonary surfactant in the lungs reach exceptionally high surface pressures (π). When compressed by the shrinking alveolar surface area during exhalation, films in the alveolus achieve π approaching 70 mN/m (1–5), well above the π at which single-component monolayers undergo a phase transition to form a three-dimensional bulk phase. For individual phospholipids, the equilibrium spreading pressure (π_e), defined by the coexistence of the film and the bulk phase, occurs at or below π ∼ 46 mN/m (6,7). The ability of the alveolar films to sustain higher π has traditionally been explained in terms of interfacial phases based on the behavior of the individual phospholipids. At physiological temperatures, most phospholipids in pulmonary surfactant form the disordered liquid-expanded (LE) phase, and at or above π_e, single-component films collapse completely. Dipalmitoyl phosphatidylcholine (DPPC), however, which represents an unusually large fraction of pulmonary surfactant relative to other biological phospholipids, can form the ordered tilted-condensed (TC) phase that remains at the interface above π_e. One widely held view has been that at π_e, the LE phase collapses from the surface, leaving a TC film that can replicate the performance of films in the lungs.

This scenario is based fundamentally on the thermodynamics of equilibrium phase behavior. Here we raise an issue not previously considered concerning the presence of multiple constituents in the biological system and the extent to which they might alter the behavior of systems with fewer components. For simple systems, the Gibbs phase rule limits the conditions at which multiple phases can coexist at equilibrium. The degrees of freedom f for coexistence of p phases in a system with c components and n undetermined intensive variables is given by f = c – p + n (8). For isothermal films containing either a single component or two components with two interfacial phases, coexistence with the collapsed phase is limited to a unique π, and π_e is single valued (9,10). A multicomponent mixture faces no such constraints. In contrast to the binary mixtures often used to model pulmonary surfactant, for the biological mixture, extension of π_e over a range of π, although not required, is possible.

The studies here consider the extent to which the presence of multiple constituents alters the phase behavior of simple model systems and allows the LE, TC, and collapsed phases to persist together at high π. We first use binary mixtures of dioleoyl phosphatidylcholine (DOPC) and DPPC to confirm the implications of the phase rule concerning collapse. We then use the complete mix of purified phospholipids (PPL) obtained from calf surfactant to determine the range of π over which the film and collapsed structures can coexist.

MATERIALS AND METHODS

Materials

DPPC and DOPC (Avanti Polar Lipids, Alabaster, AL) and N-(lissamine rhodamine-B sulfonyl)-dipalmitoyl phosphatidylethanolamine (Rh-DPPE; Molecular Probes, Eugene, OR) were purchased and used without further purification or analysis. Extracted calf surfactant (calf lung surfactant extract (CLSE)), purified as described previously (11), was obtained from Dr. Edmund Egan, ONY Inc. (Amherst, NY). The complete set of PPL was separated from CLSE by gel permeation chromatography (12,13).

All experiments used a subphase containing 10 mM Hepes pH 7.0, 150 mM NaCl, and 1.5 mM CaCl₂ (HSC). All chemicals were purchased from Sigma (St. Louis, MO). Water purified using a multicartridge system (Barnstead, Dubuque, IA) had a resistivity >17 mΩ/cm. Glassware was acid cleaned.

Methods

Phospholipid concentrations were determined by measuring the phosphate content of measured aliquots (14).

Fluorescence microscopy

Films containing PPL or binary mixtures of DOPC and DPPC at a series of mol fractions were studied microscopically. Monolayers containing 0.5 mol % of Rh-DPPE were spread in chloroform on a Langmuir trough with a continuous Teflon ribbon barrier (Labcon, Darlington, UK) to an initial π of <5 mN/m. After 30 min for solvent evaporation, films were compressed at ∼3 Ų/(molecule × min), with stops at selected π below 45 mN/m to ensure that static films would remain isobaric, indicating the absence of leakage along or around the confining barriers. A Teflon C-shaped mask minimized thermal drift of the monolayer while fluorescence micrographs were obtained using a Nikon fluorescence microscope equipped with a SIT camera (C2400; Hamamatsu Corp., Hamamatsu City, Japan). Images of monolayers were captured using a frame grabber at an automated rate of 1 image per 0.3 mN/m change in π, or 1 image/s when traversing a plateau of relatively constant π. Experiments were performed at ambient temperature of 22 ± 1°C and replicated at least three times for each composition.

Images were analyzed using programs constructed with the graphical user interface LabVIEW (National Instruments, Austin, TX). After choosing the appropriate area of interest, pixels were counted with grayscale above and below an assigned threshold value. The threshold was selected to provide close matching between the images with binary and full grayscales.

Relaxation experiments

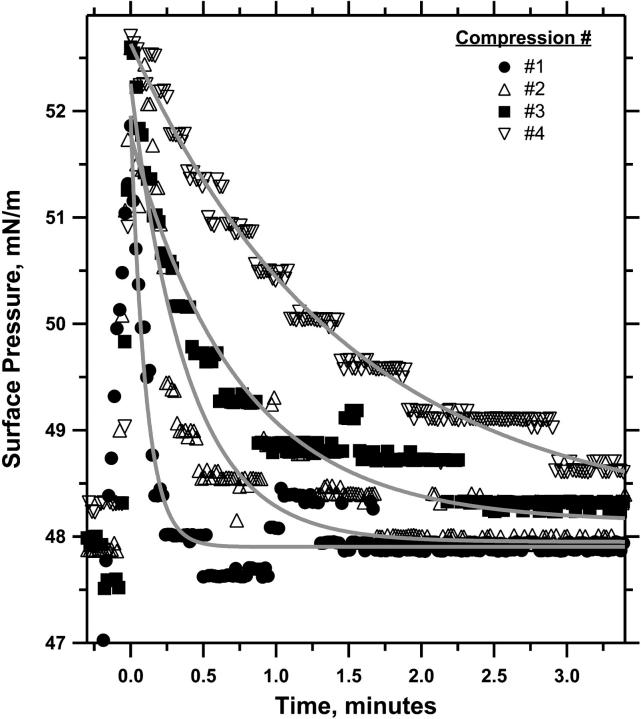
Measurements of the π to which static films collapse used a captive bubble as a surface balance (15,16). The continuous interface of the bubble eliminates confining barriers and any artifact from leakage of the compressed film (17). Small amounts of the phospholipids in chloroform/methanol (1:1, v/v) were deposited at the surface of a bubble floating below an agarose dome in aqueous solution (16). The spreading solvent was removed by exhaustive replacement of the subphase (16). The films were then compressed by applying hydrostatic pressure to the subphase and shrinking the bubble at 0.70%–1.36% volume/s to the desired π. Charge-coupled device cameras monitored the bubble along the vertical axis to ensure an axisymmetric shape and along the horizontal axis to capture images through a frame grabber to computer, which calculated surface tension, π, and surface area from the measured height and width according to previously published semiempirical algorithms (18). When π reached 52 mN/m, compression was halted and hydrostatic pressure was manipulated to hold surface area constant, while the film relaxed toward equilibrium.

RESULTS

Binary mixtures

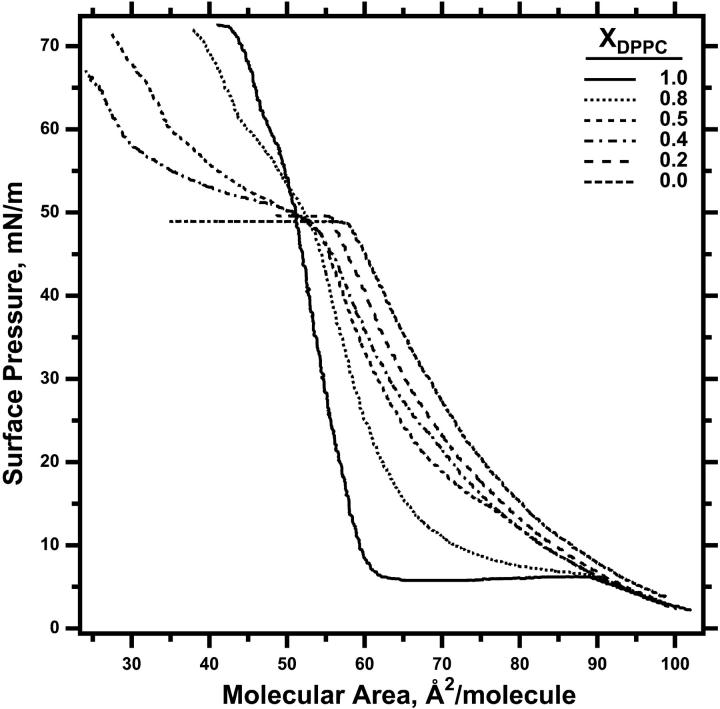
π-Area isotherms obtained on a Langmuir trough for single-component films containing either DOPC or DPPC showed the features expected for known phase behavior (Fig. 1). With DPPC, the long isobaric plateau at ∼6 mN/m corresponded with the expected coexistence of LE and TC phases, which is restricted by the phase rule to a single π. For DOPC, the isotherms rose smoothly from lift-off to the abrupt onset of a plateau at ∼47 mN/m that persisted until the end of compression and that fit with the expected coexistence of the film with the collapsed phase. Isotherms for binary mixtures of these two phospholipids in some cases showed vestiges of the plateaus observed with the individual compounds, but determining the onset and termination of any coexistence was difficult (Fig. 1).

FIGURE 1.

π-Area isotherms for compression of DOPC-DPPC binary mixtures at different mole fractions. Films at ambient temperatures of 22°C were compressed on a Langmuir trough at a constant rate of 3 Ų/(molecule × min). Curves are representative of three experiments in each case. The change in slope that occurs for DPPC at ∼60 mN/m is most consistent with creep of the film onto the barriers of the Langmuir trough (20). Isotherms for some mol fractions were omitted for clarity of presentation.

Previous studies have shown that phospholipids labeled with fluorescent chromophores partition into lipid structures according to their different levels of disorder. Fluorescence microscopy with the single-component films used here distinguished three distinct structures that corresponded to the expected LE, TC, and collapsed phases. At the lower π, films containing either pure DOPC or pure DPPC both showed a fluorescent LE phase. In films of DPPC, the nonfluorescent TC domains demonstrated previously in numerous studies appeared at the beginning of the coexistence plateau in the π-area isotherm and grew during compression across the plateau to occupy most of the surface. For monolayers of DOPC, the films remained homogeneously fluorescent until at ∼47 mN/m, brightly fluorescent spots appeared that increased in size and number during compression across the collapse plateau. In prior studies with DPPC-dihydrocholesterol mixtures and CLSE, light scattering microscopy and Brewster angle microscopy demonstrated that similar structures, which emerge at roughly the same π and have the same appearance by fluorescence microscopy, are three dimensional (19). We therefore interpreted these spots in the current studies as the collapsed phase.

For films containing both phospholipids, microscopy defined the conditions at which the LE and TC phases were present together (Fig. 2). Small amounts of DOPC broadened the range of π over which these two phases coexisted. For pure DPPC, the nonfluorescent phase occupied most of the interface within a few mN/m of its initial appearance, but with as little as 5% DOPC, both phases were readily apparent from 5 to 46 mN/m. This effect of DOPC fits with the prediction of the phase rule that addition of a second constituent would release an isothermal single-component film from the constraints which limit LE-TC coexistence ideally to a single π.

FIGURE 2.

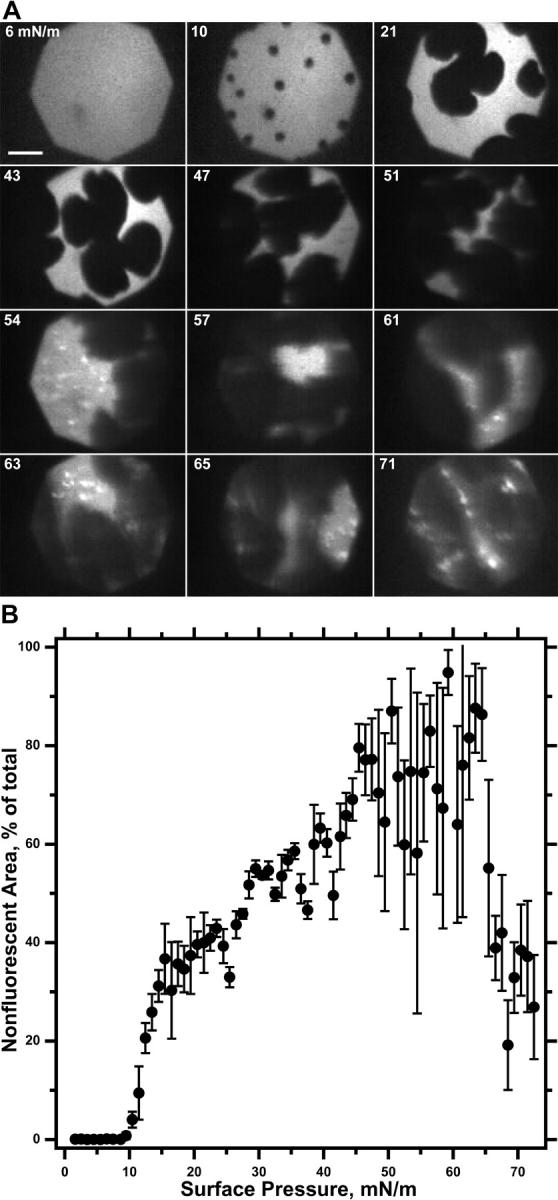
Variation of coexisting phases in DOPC-DPPC binary mixtures during compression at a constant rate. Monolayers containing DOPC-DPPC with X_DPPC = 0.70 DPPC and 0.5 mol % Rh-DPPE were compressed at 3 Ų/(molecule × min) and 22°C. (A) Fluorescence micrographs recorded at the π indicated on each frame. Camera gain was automatically controlled according to the intensity of the visual field. Scale bar represents 50 μm. Images are representative of micrographs recorded after each 0.3 mN/m increment in π. (B) Fractional area occupied by the nonfluorescent phase as a function of π. Data are the fraction of pixels in a micrograph that are darker than a threshold value, box-car averaged for images obtained over an interval of 1 mN/m, and representative of three experiments.

In addition to extending LE-TC coexistence over a broader range of π, added DOPC also shifted its onset to progressively higher π. When the DPPC mol fraction (X_DPPC) reached 0.05, the nonfluorescent phase was never apparent. At X_DPPC = 0.10, the TC phase emerged just below 46 mN/m, immediately before the collapsed structures appeared. Therefore at ∼46 mN/m, the coexisting light and dark phases were detected for all X_DPPC from 0.10 to 0.95. Immediately before the onset of collapse, the coexistence region for the two monomolecular phases extended over at least that range of compositions.

To confirm the prediction of the phase rule that three phases should coexist only at a single π, we tested the alternative hypothesis that the LE, TC, and collapsed phases might coexist over a range of π. For the series of films with 0.1 ≤ X_DPPC ≤ 1.0 for which the two monolayer phases were present at 46 mN/m, separate methods established the upper and lower boundaries for this hypothetical range of π. We used microscopy to determine the lowest π at which the collapsed phase first became apparent. At progressively higher X_DPPC, the π at which the brightly fluorescent spots emerged increased slightly from ∼47 mN/m for X_DPPC ≤ 0.30 to 56 mN/m at X_DPPC = 0.70. Because detection of the collapsed phase required structures above a certain minimum size, we considered that these microscopic experiments established only an estimated upper limit to the onset of collapse, which might begin at lower π.

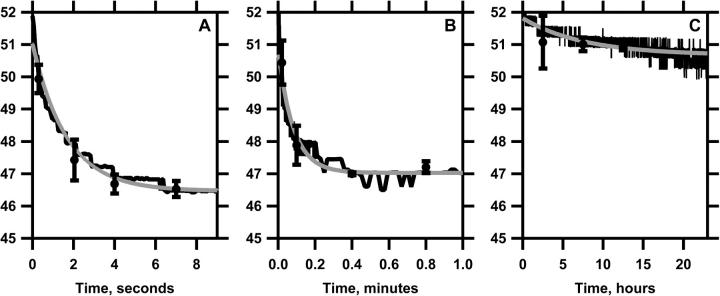
To determine the upper limit of the hypothetical range of π over which the collapsed structures and two monomolecular phases can coexist, we measured the π to which films relaxed after collapse began (Fig. 3). Films were rapidly compressed on a captive bubble from 45 to 52 mN/m and then held at constant area while π decayed from an initial π_o toward a final π_e. The measurements of π versus time (t) were fit to a simple exponential function, 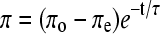, which yielded values of π_e. Composition had relatively little effect on π_e obtained by this approach. Over the range of LE-TC coexistence, π_e varied from 46.2 ± 0.1 mN/m at X_DPPC = 0.10 to 46.9 ± 0.1 mN/m for X_DPPC = 0.90, with an average value of 46.7 ± 0.3 mN/m over that range. (For films at X_DPPC = 0.95, π fell too slowly to assess accurately the value of π_e.) These values, which established the upper limit at the different X_DPPC of the π over which the collapsed phase and film could coexist, therefore in each case fell below the lower limit estimated by microscopy.

FIGURE 3.

Relaxation of DOPC-DPPC films at constant area after compression to 52 mN/m. Films with different compositions (X_DPPC = 0.10, 0.50, and 1.00 for panels A, B, and C, respectively) were compressed at the ambient temperature of 22°C on a captive bubble from <46 mN/m to 52 mN/m and then maintained at constant area, while π relaxed. Measured data (black lines) were fit to simple exponential functions of time (shaded lines). Each curve is representative of at least three experiments, with symbols providing mean ± SD for all experiments at selected points.

We then considered whether the collapsed and condensed phases could coexist at π > 46 mN/m. Relative to the LE phase, the TC phase collapsed at rates that were negligible (Fig. 3). These experiments used films with the compositions at 46 mN/m of each monomolecular phase, established by microscopy as X_DPPC ∼ 0.10 and ∼ 1.00 for the LE and TC phases, respectively. When compressed to 52 mN/m and then held at constant area, films with X_DPPC = 0.10 relaxed to 46.2 ± 0.1 mN/m within seconds. In contrast, films with X_DPPC = 1.00 held π constant at 52.2 ± 0.1 mN/m for hours. When monitored for sufficiently long durations, π did fall (Fig. 3 C), indicating that the films of DPPC were metastable rather than at true equilibrium. This behavior was consistent with the prediction of the phase rule that for an isothermal single-component film, coexistence of a collapsed and monomolecular phase should be limited to a single π. The condensed and collapsed phases persisted together at high π over the timescale on which the LE phase collapsed, but not indefinitely.

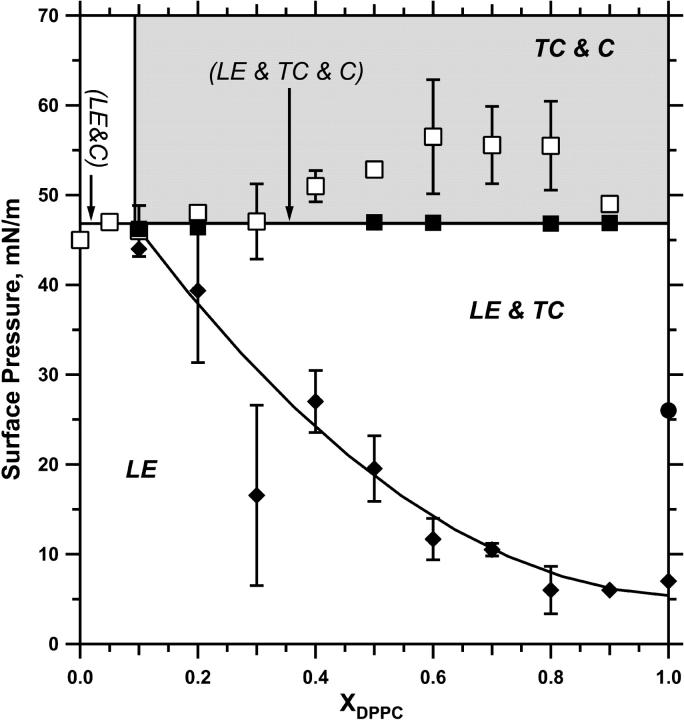
The phase diagram constructed on the basis of microscopic and relaxation experiments (Fig. 4) predicted the behavior of films compressed along specific pathways. The most important issues concerned changes during continuous compression at π ≥ 46 mN/m. For films with X_DPPC < ∼0.1, which contained a single LE phase, the films should collapse completely and π should rise above 46 mN/m only transiently. For any film with X_DPPC ≥ 0.1, collapse should begin at 46 mN/m and occur exclusively from the LE phase. The composition of the monolayer should change accordingly, but the compositions of the coexisting monomolecular phases should remain fixed, and collapse of the LE phase should begin at the same π_e and continue until the film is homogeneously TC. In all films compressed beyond the onset of collapse, contrast between the LE and TC phases decreased, presumably because of the progressive loss of the fluorescent probe. With compression either at a constant rate (Fig. 2 B) or isobarically at 52 mN/m (Fig. 5), however, contrast remained sufficient to show the predicted loss of the LE phase and expansion of the TC phase to occupy most of the interface, along with the increase in number and size of the collapsed nuclei.

FIGURE 4.

Phase diagram for monolayers of DOPC/DPPC. For films with different X_DPPC compressed on a Langmuir trough at a constant rate of 3 Ų/(molecule × min), (♦) indicate the π at which fluorescence microscopy first detected the nonfluorescent phase. The curved line through these points was drawn by hand. (□) show the π at which brightly fluorescent spots, consistent with collapsed structures, first appeared. (▪) indicate the π to which films relaxed on a captive bubble when held at constant area after compression to 52 mN/m. The horizontal line through these points represents the average π for the filled squares. The (•) indicates the only point at which microscopy indicated the completion of transition from the fluorescent phase to a homogeneously nonfluorescent film. Labels indicate conditions at which the LE, TC, and collapsed (C) phases are present. Labels in parentheses represent coexistence that occurs along the isobaric line indicated by the arrow. Labels in bold without parentheses represent the phases present in the region bounded by solid lines. The shaded zone indicates conditions at which the film is metastable rather than at true equilibrium. Data are mean ± SD for at least three measurements.

FIGURE 5.

Variation of coexisting phases during continuous and then isobaric compression of a DOPC-DPPC monolayer. Films containing DOPC-DPPC at X_DPPC = 0.80 mixed with 0.5 mol % Rh-DPPE at 22°C were compressed continuously at 3 Ų/(molecule × min) to 52 mN/m and then isobarically using simple feedback to hold π constant. Images were recorded at regular intervals with the gain of the camera held constant and with the same magnification. Scale bar represents 50 μm. (A) Fluorescence micrographs during the initial compression at a constant rate from 0 to 52 mN/m. Labels indicate π (mN/m) at which the images were obtained. (B) Images during the isobaric compression at 52 mN/m. Labels indicate time (minutes) after reaching 52 mN/m. (C) Area of nonfluorescent domains during isobaric compression. Area is expressed as the fraction of total area, obtained by counting pixels with grayscale below a threshold value in images captured at a rate of 1 Hz. Data are mean ± SD from values boxcar-averaged over a series of successive images and representative of three experiments.

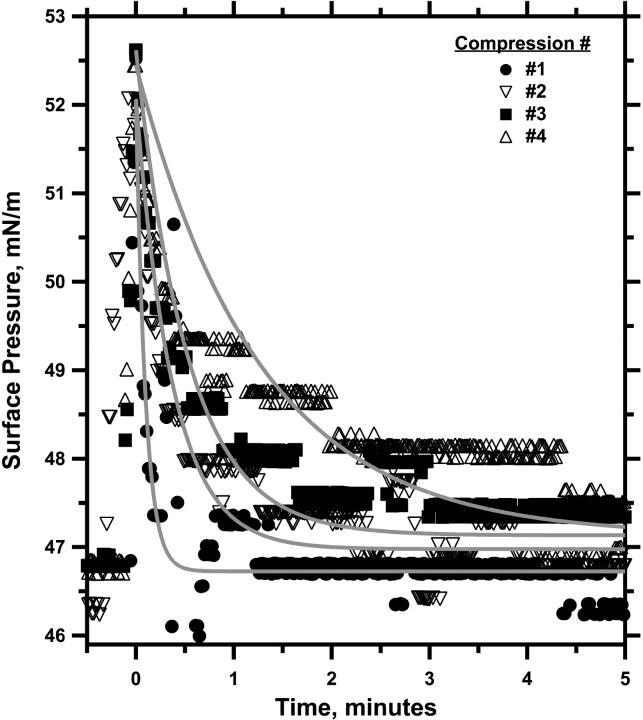
The phase diagram also predicted that collapse, which changes the composition of the monolayer, should have the same effect as varying the composition by spreading different initial films. Relaxation at constant area after a series of repetitive compressions should reduce the LE area and slow the kinetics of collapse, but π should always return to the same final π_e. Experiments with the captive bubble confirmed the expected behavior. Films with X_DPPC = 0.50 were compressed sequentially to 52 mN/m and then held at constant area while π was allowed to relax (Fig. 6). π again fell after each compression as an exponential function of time. The rate of relaxation decreased with each successive compression, but the extrapolated value of π_e changed between the first and fourth relaxation by only 0.2 ± 0.1 mN/m. After each successive compression, π returned toward the common π_e obtained during the first compression of films with different compositions.

FIGURE 6.

Relaxation of a DOPC-DPPC monolayer after sequential compressions. After spreading at the continuous interface of a captive bubble, monolayers with X_DPPC = 0.50 were slowly compressed at ∼2 Ų/(molecule × min) to 45 mN/m and then rapidly to 52 mN/m. Interfacial area was then held constant, while π relaxed. The pulsed compression and relaxation were repeated with time in each case set to zero at the beginning of relaxation. The superimposed shaded curves give the best fit to the data of a simple exponential function. Representative of three experiments.

Surfactant phospholipids

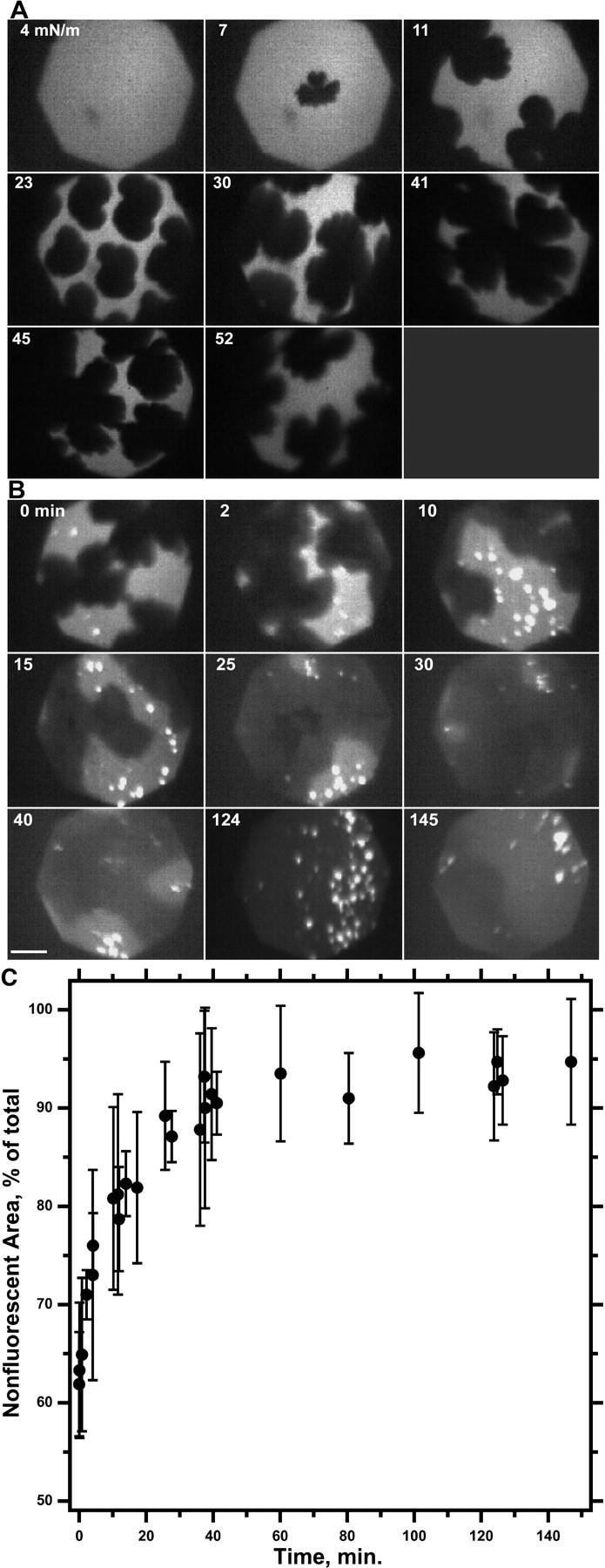
We used the same methods to determine the behavior of the three phases in PPL. Microscopic measurements during compression of the PPL monolayer showed that the film was initially homogeneously fluorescent and that at higher π a nonfluorescent phase appeared first, followed by the brightly fluorescent spots, all in agreement with previous observations (13,20). The fluorescent spots emerged at 45.3 ± 0.7 mN/m, providing an estimate of the lower limit of the π at which all three phases coexist (Fig. 7). To establish the upper limit of the coexistence region, films of PPL were compressed on the captive bubble to 52 mN/m and then allowed to relax. π again fell along an exponential function of time to an extrapolated final value of 47.6 ± 0.3 mN/m (Fig. 8). In contrast to the binary mixtures, this upper limit of the coexistence region, obtained by relaxation, exceeded the lower limit established by microscopy. These results suggested that coexistence of the interfacial and collapsed phases did in fact extend over a narrow range of π and that π_e was not single valued. The experiments also showed, however, that despite release from the constraints of the phase rule by the presence of multiple constituents, coexistence of the collapsed and monomolecular phases persisted to π no higher than for the binary mixtures.

FIGURE 7.

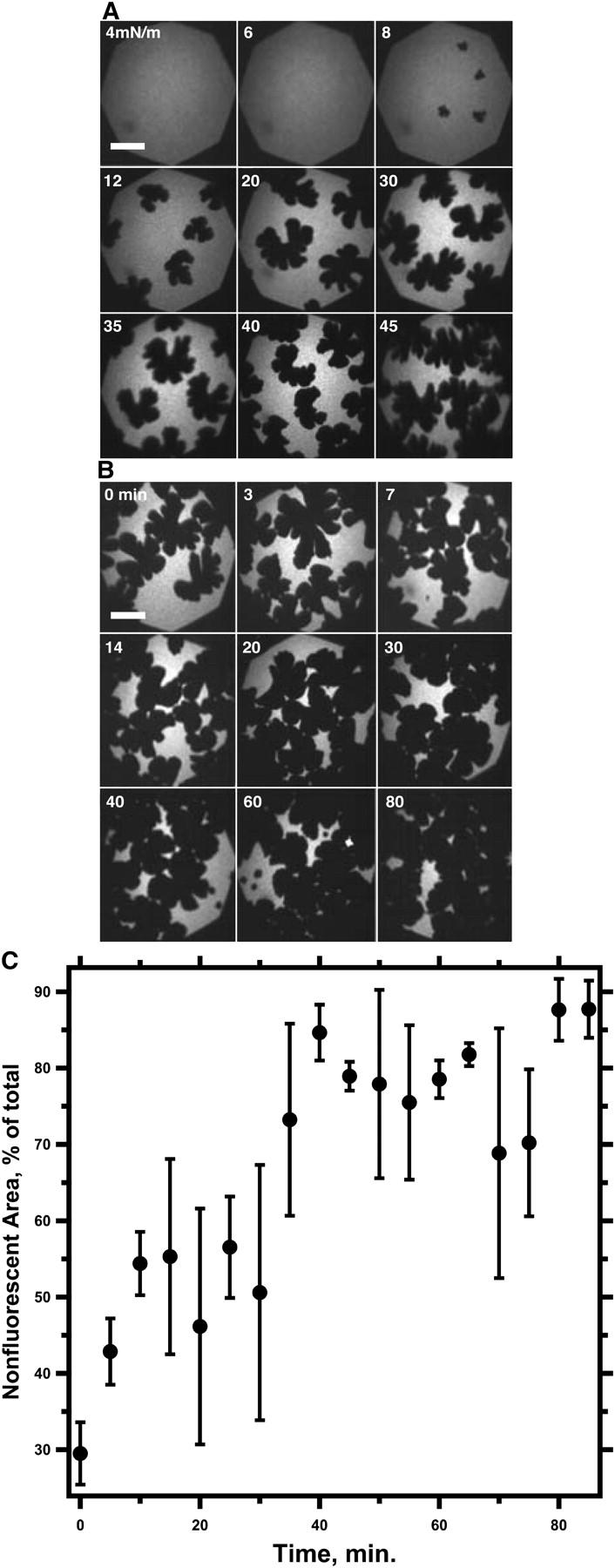
Fluorescence micrographs of PPL monolayers during continuous and then isobaric compression. (A) Fluorescence micrographs during the initial compression at a constant rate up to 45 mN/m, at which the intensely fluorescent spots of the collapsed structures were first observed. (B) Images during the subsequent isobaric compression. Labels indicate time (minutes) after reaching 45 mN/m. Scale bars represent 50 μm. (Note that the magnification differs from Figs. 2 and 5). Gain of the camera was constant throughout the experiment. (C) Area of nonfluorescent domains, expressed as the fraction of total area, obtained by counting pixels with grayscale below a threshold value in images captured at a rate of 1 Hz during the isobaric compression. Data are mean ± SD from values boxcar-averaged over a series of successive images obtained during three experiments.

FIGURE 8.

Relaxation of PPL monolayers after sequential compressions. After spreading on a captive bubble, monolayers of PPL were compressed initially at ∼2 Ų/(molecule × min) to 45 mN/m and then more rapidly to 52 mN/m. Interfacial area was then held constant, while π relaxed. After achieving a π that remained constant for 30 min, the compression and relaxation were repeated, with time recorded for each cycle relative to the moment at which relaxation began. Data are representative of three experiments.

PPL films showed behavior similar to that of binary films during compressions beyond the onset of collapse. During isobaric compressions at 45 mN/m after the appearance of the intensely fluorescence spots, the collapsed structures became progressively more evident, and the fraction of the interface occupied by the nonfluorescent phase grew continuously toward 1 (Fig. 7). During sequential cycles of compressions to 52 mN/m followed by relaxation at constant area, π fell more slowly with each compression but toward a π_e that remained constant (Fig. 8). The difference in π_e, obtained by extrapolating fitted exponential curves to infinite time, between the fourth and first successive compressions was 0.3 ± 0.1 mN/m for PPL and 0.2 ± 0.1 mN/m for DOPC-DPPC with X_DPPC = 0.50. PPL after the onset of collapse therefore behaved like the binary mixtures of DOPC and DPPC.

DISCUSSION

Previous studies in our laboratory have shown that in monolayers containing the complete set of surfactant phospholipids, collapse begins as expected at ∼45 mN/m (21). During slow continuous compression, however, rather than the anticipated complete exclusion of the LE phase before π can rise further, all three phases remain readily evident over the full range of π to values approaching 70 mN/m. When compression stops above 65 mN/m, π remains constant for prolonged periods, indicating the absence of further detectable collapse. The studies here distinguish between two basic explanations for this behavior. The films at high π could exist in a kinetically achieved metastable state, produced by compressions that, although slow, nonetheless deviate from equilibrium. Conversely, the presence of the three phases could reflect a true thermodynamic equilibrium. Such an equilibrium of three phases should be impossible for the simple two-component mixtures often used to model pulmonary surfactant because of the constraints of the phase rule. For a mixture of multiple components, however, those constraints would not apply, and the three phases might coexist over a range of π.

The binary films of DOPC-DPPC provide a simple test of the phase rule. Although the Gibbs rule might be accepted as established, we agree with recognized authorities that the testing of basic thermodynamic relationships may reveal unexpected complexities and is therefore worthwhile (22). The Gibbs rule predicts that the three phases can coexist in a two-component isothermal film only at a single π. The highest and lowest π for the three-phase coexistence, rather than defining a range, should therefore have the same value. In our studies, the lower limit, detected by microscopy, actually lies above the upper boundary established by relaxation of films compressed to the onset of collapse. The simplest explanation for this disparity is that the microscopic detection of the collapsed phase is delayed beyond the true onset of three-phase coexistence. Our experiments probably overestimate the π that represents the lower limit of coexistence, which may well coincide with the upper limiting π. Although our results therefore fall short of a true confirmation of the phase rule, they are entirely consistent with its predictions.

The microscopic measurements might overestimate the π at which coexistence of the collapsed and monomolecular phases becomes possible for two reasons. First, microscopy detects only structures above a certain minimum size, and during continuous compression, π might rise appreciably while collapsed nuclei grow to the limits of detection. Second, nucleation of the collapsed phase might be delayed to conditions beyond the true phase boundary, similar to the behavior of other phase transitions (23). The emergence of collapsed nuclei might therefore represent an inaccurate indication of the limits of the coexistence region in the phase diagram. On both counts, the conclusion that the microscopically determined lower limiting π might be artifactually high seems quite reasonable.

The phase diagram established for the DOPC-DPPC films up to the onset of collapse makes further predictions. In addition to having a single value for any particular film, π_e should be the same for all compositions that extend over the LE-TC coexistence region. Between X_DPPC ≈ 0.10 and 0.95, the films contain an LE phase with a fixed composition that should therefore collapse at the same π. Our results confirm that prediction. The different films relax at different rates, presumably because of the different LE areas, but they eventually reach a π_e that for films with six different compositions is equivalent within experimental error.

The phase diagram also predicts that above ∼47 mN/m, the binary films should initially be unstable. Although the isotherms obtained during continuous compression establish that DOPC-DPPC films can reach higher π, they should be unstable as long as the monolayer contains two phases. If π remains above 47 mN/m, the LE regions should continue to collapse until that phase is eliminated. The microscopic images during isobaric compressions (Fig. 5) confirm that prediction.

For PPL, prior studies have established that the films up to the onset of collapse generally behave like the DOPC-DPPC mixtures (20). Compression of the PPL monolayers induces the separation of TC domains from a surrounding LE film, and at the temperatures used here, the two phases coexist over a broad range of π. The TC domains have a X_DPPC that exceeds 0.95, and the constituents other than DPPC are confined to the LE phase. Our studies here show that the similarity of the PPL and DOPC-DPPC films extends in several respects to higher π. After compression to the π at which collapse begins, static films relax to 47.6 mN/m, similar to the value of 46.6 mN/m achieved by the binary films. When compressed isobarically just after the onset of collapse, the LE regions in PPL are progressively excluded from the interface until that phase is eliminated, similar to the behavior of binary mixtures. These experiments with PPL therefore establish an upper limit of coexistence for the three phases at a level similar to that of the binary mixtures. At higher π, the films deviate from equilibrium.

The multiple constituents in PPL may in fact allow the coexistence of the three phases to extend beyond a single π. In contrast to the binary mixtures, microscopy first detects collapsed structures ∼2 mN/m below the level reached during relaxation from higher π. These experiments suggest that the three phases may coexist over the interval between these upper and lower boundaries and that π_e may represent a range of π rather than a single value. The extent of this range is of course limited and possibly explained by overestimation of the upper limiting π because of incomplete relaxation. If real, the range of π over which the three phases coexist might explain the slope of the compression isotherms for pulmonary surfactant films, for which the horizontal collapse plateau begins with a rounded shoulder rather than the sharp discontinuity that occurs for simple fluid monolayers (13,19,24). A true difference between the upper and lower limits of coexistence, however, would nonetheless be inconsequential for the question addressed here. The narrow range over which the three phases could coexist would extend below π_e for the binary mixtures rather than to higher π. The thermodynamic effects of multiple constituents would therefore provide no explanation for persistence of the three phases to higher π.

Because equilibrium coexistence of three phases terminates at ∼46 mN/m for PPL as well as for the binary mixtures, the LE phase in PPL at higher π must deviate from equilibrium. When effectively no collapse occurs at very high π, as demonstrated in our previous studies (21), the films must be metastable. The LE phase in the PPL films with multiple components must behave at high π like supercompressed fluid films containing a single phospholipid. Those single-component films become metastable, analogous in several respects to supercooled three-dimensional liquids, with rates of the transition to form the collapsed phase becoming slower at progressively higher π despite further deviation from equilibrium conditions (7,25–27). The best explanation for the persistent LE phase in static PPL films at high π is that it becomes jammed into nonequilibrium solid structures.

In summary, our results show that for binary films of DOPC-DPPC, which form two monomolecular phases, collapse is consistent with the constraints of the phase rule that limits three-phase coexistence to a single-valued π_e. For films of PPL, which contain multiple constituents, coexistence of two monolayer phases with collapsed structures may extend over a narrow range of π, but the upper limit is similar to π_e for the binary mixtures. PPL films at higher π must deviate from equilibrium.