From Shut to Open: What Can We Learn from Linear Free Energy Relationships?

There is much to be said for having a model, even an oversimplified model, to focus ideas. To avoid confusion, I should say that by model, I mean a quantitative reaction scheme that can make testable predictions, not the multicolored icons of proteomics (or should it be called blobomics?). In the area of ligand-gated ion channels, this is what Bernard Katz (1) did in 1957, when he proposed the first mechanism for a channel that separated the ligand binding step from the channel opening (gating) step. Thinking about this model is what showed the errors in the woollier thinking about affinity (binding) and efficacy (gating) that were prevalent in the 1950s (actually it had all been done earlier, in a different context, by Jeffries Wyman, but that is another story).

In this issue, Zhou, Pearson, and Auerbach (2) have done something similar. Their model may well be oversimplified but it contains the guts of the problem, and lends concreteness to arguments that previously had been somewhat vague. Before describing their results, a bit of background is needed.

Now that some, albeit incomplete, structures are available for ion channels, it is natural to expand Bernard Katz's mechanism by asking how it is that the binding of an agonist causes the channel to open. How does the signal caused by ligand binding get transmitted to the gate which is some distance away? It is a question not so much about physiology as about the internal machinery of protein molecules. But it is an important question because unless it can be answered, we cannot hope to achieve the goal of being able to predict the activity of a ligand, or predict the effect of a mutation in the receptor. It must be admitted at the outset, that despite great advances in both structure and function in the last 40 years, our ability to predict is essentially the same as it was in the 1950s—i.e., essentially zero.

Attempts to fit mechanisms started in 1972 (3), but the macroscopic methods available then did not have the necessary resolution. In 1977, it was predicted (4) that channel openings should usually not occur singly, but in bursts of openings separated by short shuttings—the whole burst, or activation, being what a synapse sees as an effective opening. This prediction was soon verified experimentally (5,6), and the fine structure of the bursts allowed the separate estimation of the rate constants for binding of agonist and for gating of the channel (or, in pharmacological terms, the separate estimation of affinity and efficacy). It was a first attempt at solution of the “binding-gating problem” (7). Many others have contributed since then (review in (8)). At that stage there was optimism that this separation of the rate constants for individual steps, rather than the crude use of macroscopic binding and EC₅₀ measurements which allow no such separation, would allow more sense to be made of the structure-activity relationships of agonists and, later, of the effect of mutations. It has to be said that this aim is far from being achieved yet. For example, some mutations in positions that are certainly far from the agonist binding site appear to affect mainly the microscopic binding rates to the shut channel (e.g., αN217K, ɛL221F (9,10); both in M1). This might happen if the thing described as “microscopic affinity for the resting binding site on the shut channel” was actually the affinity for a state that had altered conformation substantially. Any reaction that involves a conformation change is, of course, potentially affected by mutations anywhere in the parts of the molecule that move.

This state of affairs concentrated attention on what happens between the time when the agonist molecule first binds, and the time when the channel eventually opens. Is it possible to detect intermediate states between initial binding and eventual opening? Perhaps there are states that are still shut, but which have undergone a substantial conformation change—such states could, for example, correspond to domain closure around an agonist molecule, as suggested by structural studies. Two approaches have been made to this problem recently. One approach was to attempt to fit single-channel results with mechanisms that include a conformation change while the channel is still shut (11). The other attempt, from Auerbach's lab in Buffalo, used the theory of linear free-energy relationships, imported from physical chemistry, via protein folding, and first applied to receptors by Grosman et al. (12).

Auerbach's lab now uses the more descriptive term “rate-equilibrium free energy relationship” (REFER) to describe their approach. It is applied, usually, to the gating step, the transition from shut to open. The opening rate constant is usually denoted β, and the shutting rate constant is α, so the gating equilibrium constant is β/α, a quantity variously denoted Θ or L or E (for efficacy) in experimental articles. The basic idea is very simple. If the value of Θ changes (because of a perturbation, e.g., a mutation, or a change in membrane potential for example), we observe whether the change results from a change in the numerator, β, or in the denominator, α. For example, over a series of mutations, log(β) is plotted against log(Θ) and the slope of this plot is called Φ. Thus, if Φ = 1, only β is changed, whereas if Φ = 0 it is the shutting rate constant, α, that is changed by the perturbation. By dint of testing a heroic number of mutations, Auerbach and his colleagues observed that mutations made in the outer part of the molecule, near the agonist binding site, gave Φ-values close to one (β-changes), but as mutations were made deeper, toward the channel gate, Φ-values decreased progressively toward zero (α-changes). Furthermore, groups of amino acids that are close to each other appeared to have similar Φ-values, which was taken as suggesting that these groups (or blocks) move together as a rigid body as the channel opens. This systematic change was very striking, and itself quite enough to persuade one that the authors were on to something important. But what exactly does it mean in terms of mechanisms?

The classical interpretation of the slope, Φ, of the REFER plot refers to the transition state, the energetic peak that must be climbed to move between shut and open. When Φ = 1 it means that the transition state resembles the open state, whereas small values of Φ suggest the transition state resembles the shut state. For example, use of a series of different agonists, or a series of mutations in the binding site region, both give Φ-values close to 0.93 (12). The fact that these residues are in an openlike state when the receptor reaches its transition state might be taken as a suggestion that this part of the molecule changed shape before other parts.

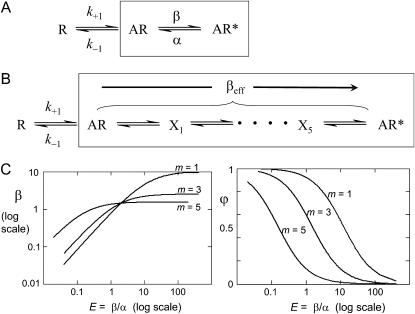
This temporal interpretation of transition state analysis, and the very existence of fractional values of Φ, has given rise to much debate (e.g., (13)). To me, at least, the arguments have always seemed too woolly to be entirely convincing. I have never quite been able to bridge the gap between concept and full understanding. But now concrete reaction mechanism comes to the rescue. In this article (2), and in a previous simulation study (14), it is postulated that there are several very short-lived intermediate states (X₁–X₅ in Fig. 1), which must be passed through in sequence en route from the shut to the open state. So, for example, the del Castillo-Katz mechanism in Fig. 1 A would be extended as shown in Fig. 1 B. Usually attention is focused on the fully liganded receptor so the binding step on the left can be ignored.

FIGURE 1.

The simple del Castillo-Katz mechanism (A = agonist, R = receptor), and its extension by addition of five intermediate preopening states. Boxed regions are fully liganded. The REFER plots calculated from the numerical example in Zhou et al. (2) are shown (bottom left) and the corresponding Φ-values are plotted against the gating constant (bottom right). Calculations are shown for perturbation of the first (m = 1), third (m = 3), and fifth (m = 5) intermediate states (X_m), as in Zhou et al. (2).

In his simulation study, Auerbach (14) showed that changing the rate constants for leaving X₁ produced a large (near 1) value of Φ, that perturbing the second intermediate in the sequence X₂ resulted in a smaller value of Φ, and so on—the smallest values being produced by perturbation of the last intermediate X₅. For this sort of scheme at least, the value of Φ does have a temporal significance. In this article (2), they go further by finding an approximate analytical solution for the reaction scheme in Fig. 1 B. To calculate the REFER plot, the abscissa is not a problem: it has values for the overall equilibrium constant for opening, the product of all the separate equilibrium constants for the intermediates. But how do we find the ordinate, the effective opening rate constant, β_eff in Fig. 1 B? Well, when completely saturated (see boxed region in Fig. 1 B), the mean shut time is 1/β in Fig. 1 A, so it is sensible to take 1/β_eff as the mean shut time conditional on the shut time starting in AR (also known as the mean first-passage time from AR to AR*). A simple matrix expression for this was given by Colquhoun and Hawkes (15), but this article (2) has now come up with a nice approximation to the exact result, which holds when the two end states (AR and AR*) have much longer lifetimes that all the intermediate states (X_i). This approximation does not hold well with the fast rate of leaving AR that is needed to describe acetylcholine itself (14), but it is accurate under the conditions where Θ is smaller (many of the experiments were done with choline as agonist rather than acetylcholine). The model is undoubtedly oversimplified. REFER plots calculated from it have slopes (Φ) that fall from one to zero as Θ is increased, regardless of which intermediate is perturbed, as shown in Fig. 1 (bottom). This is consistent with the observation that unliganded openings (which have very low Θ) always give Φ = 1 (16). And experimental REFER plots seem be more nearly linear than the model predicts. The important point, however, is that for any given Θ, the slope, Φ, is always lower when the rate constants are changed for later steps in the chain than for earlier ones. The idea that Φ is a measure of the temporal sequence of intermediate events now has a concrete basis that heretofore it has lacked. Although one can quibble about the extent to which Φ-values occur in blocks that define rigid movements of groups of amino acids, the basic idea is now well founded, and has made a major contribution to understanding of how the ion channel works.

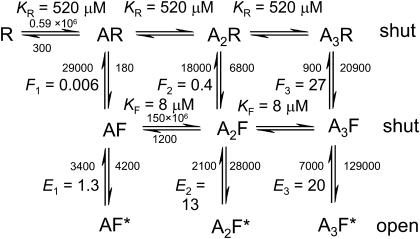
An alternative approach is to try to identify intermediate states, short-lived shut states that occur before the channel opens, by more conventional methods. This has been tried with Ca²⁺ as agonist (17), and it has not proved easy for the nicotinic receptor, although ultra-high-resolution methods give a hint that it might be possible (18). In the case of the heteromeric glycine receptor, however, single-channel measurements can be fitted with an intermediate shut state, a flipped conformation, between the resting and the open states of the receptor (11). This is shown in Fig. 2. The triply liganded flipped state (A₃F, Fig. 2) is short-lived (∼8 μs), and thus resembles one of Auerbach's X states.

FIGURE 2.

The flip model for the glycine receptor. The agonist A (glycine) binds to the resting state of the receptor (R), which then flips to a higher-affinity shut state (F) before the channel opens (F*). The binding sites on any one conformation do not interact, so the microscopic binding constant is the same for all three bindings, but the affinity for the flipped conformation is ∼65-times higher than the affinity for the resting conformation. Consequently the flipping equilibrium constant (F₁, F₂, F₃) increases 65-fold for each agonist molecule bound. This contributes more than the changes in gating constants (E₁, E₂, E₃) to the increase in efficacy as more agonist molecules bind.

This interpretation, although not unique, is attractive because it explains also the apparent cooperativity of binding without having to suppose that the binding sites interact with each other. The advantage of this sort of approach is that it is more direct than using Φ-values, and that it may be possible to identify all the relevant rate constants (as in Fig. 2). But it is hard to imagine that more than one preopening shut state could be identified in this way, so, although more quantitative, the picture can never be as detailed as that which is now emerging from Φ-analysis.