Abstract
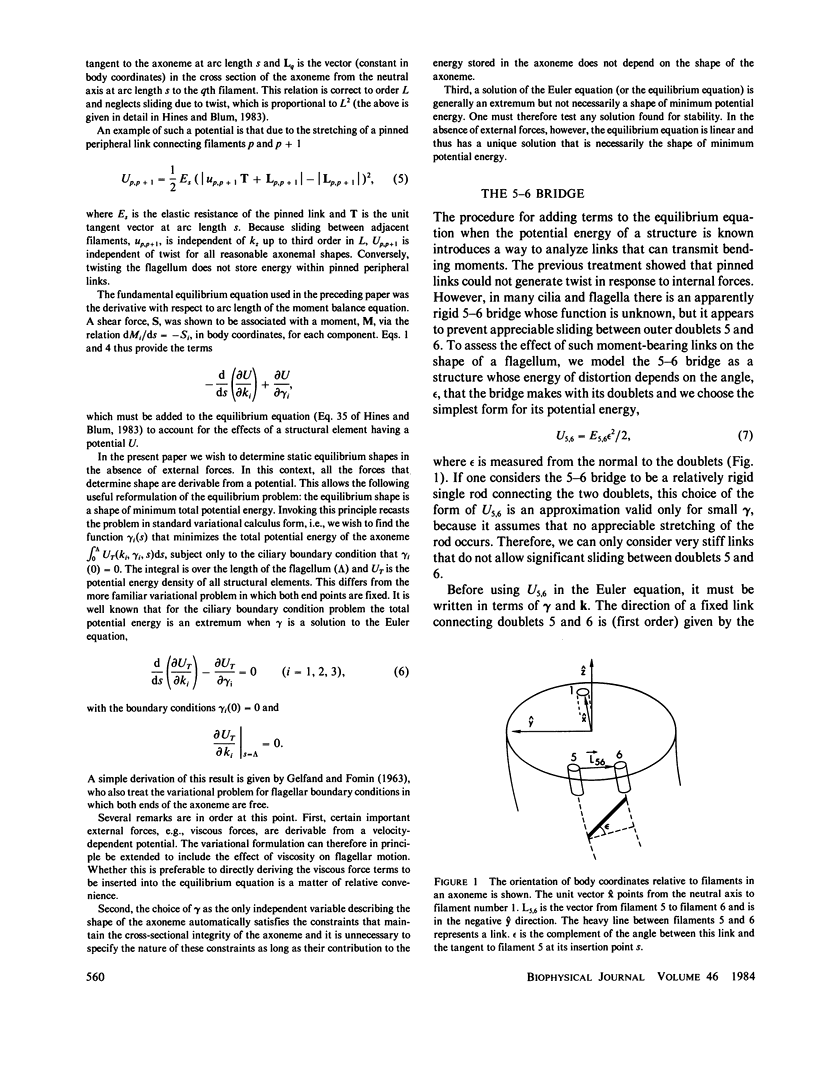
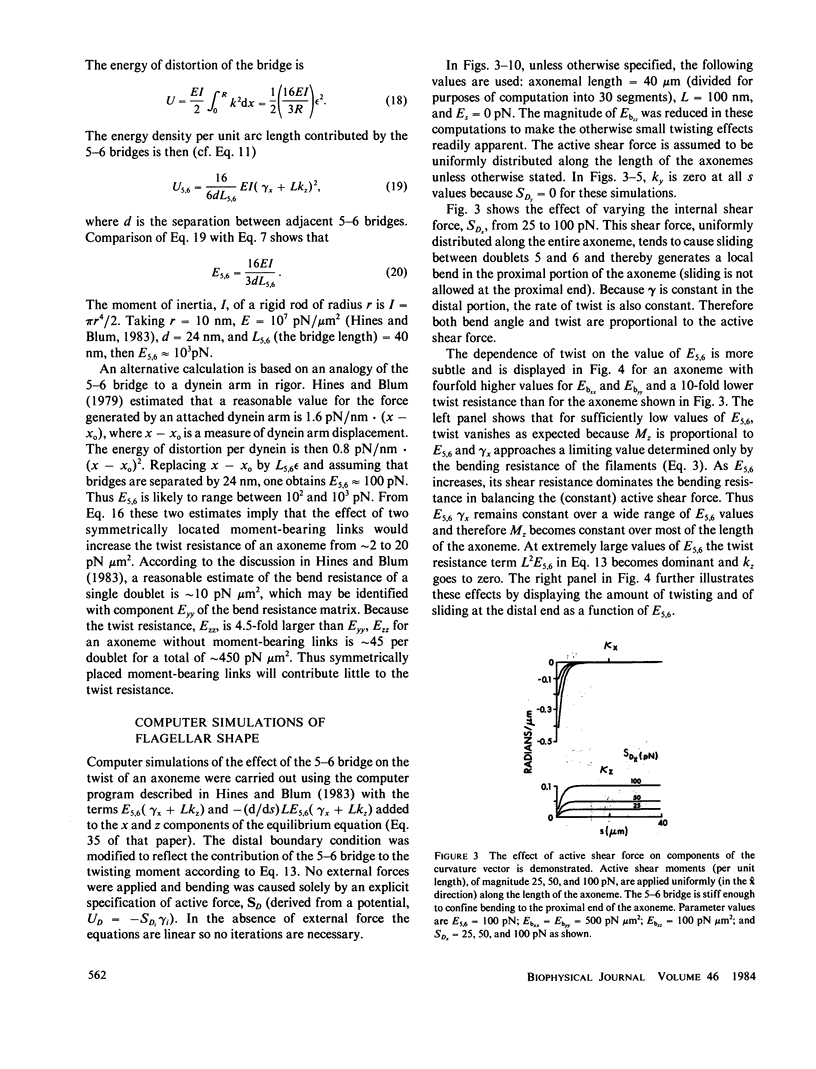
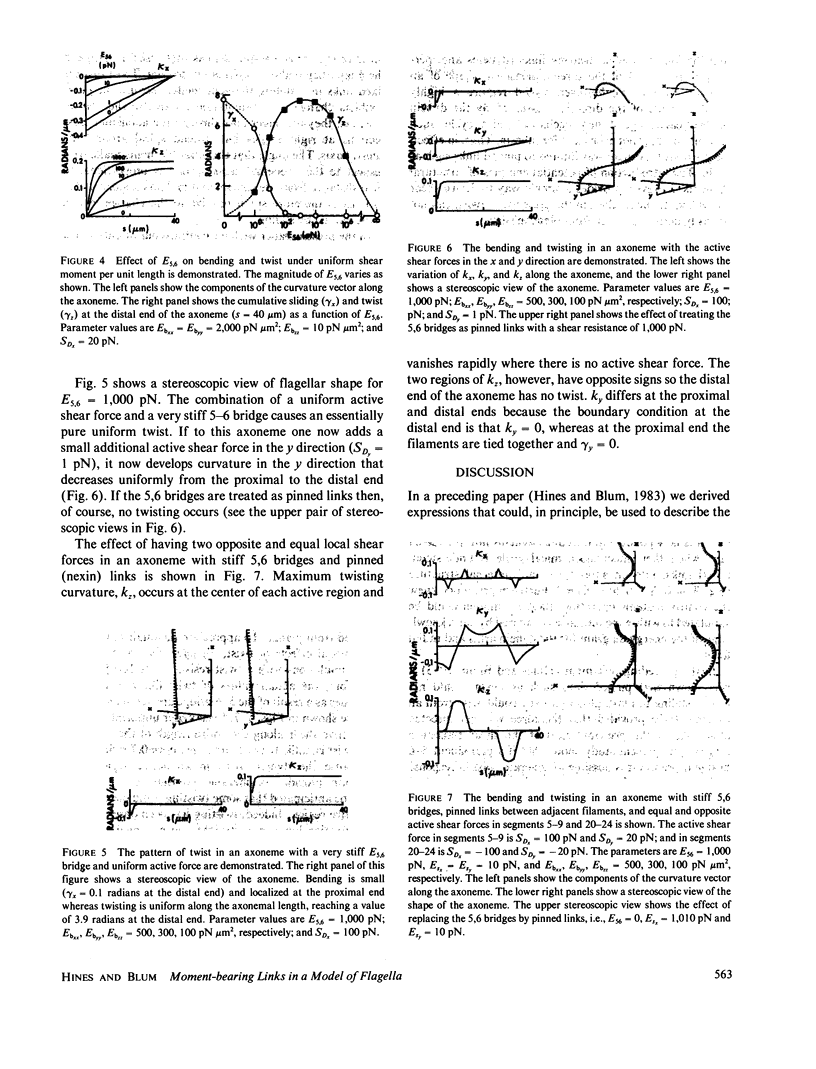
Previously (Hines, M., and J.J. Blum 1983, Biophys. J., 41:67-79), a method was developed that allowed one to compute curvature and twist for a three-dimensional sliding filament model. In that formalism it was difficult to specify the shear and bending moments arising from moment-bearing interfilament links such as fixed 5-6 bridges or dyneins. Euler's equation offers a straightforward method for computing these bending and shear moments when the potential energy stored in the links as a function of axonemal shape is specified. We used this approach to examine the effect of 5-6 bridges on curvature and twist for several distributions of internal shear moments. Twist changes the angle that a link makes with a doublet and thus in some circumstances may reduce the potential energy stored in those links. Twist is a second-order effect proportional to the square of the distance between an outer doublet and the neutral axis. Fixed links will not generate twist if they are symmetrically located around the axoneme.
Full text
PDF



Selected References
These references are in PubMed. This may not be the complete list of references from this article.
- Brokaw C. J. Molecular mechanism for oscillation in flagella and muscle. Proc Natl Acad Sci U S A. 1975 Aug;72(8):3102–3106. doi: 10.1073/pnas.72.8.3102. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Gibbons I. R. The molecular basis of flagellar motility in sea urchin spermatozoa. Soc Gen Physiol Ser. 1975;30:207–232. [PubMed] [Google Scholar]
- Hill T. L. Theoretical formalism for the sliding filament model of contraction of striated muscle. Part I. Prog Biophys Mol Biol. 1974;28:267–340. doi: 10.1016/0079-6107(74)90020-0. [DOI] [PubMed] [Google Scholar]
- Hines M., Blum J. J. Bend propagation in flagella. II. Incorporation of dynein cross-bridge kinetics into the equations of motion. Biophys J. 1979 Mar;25(3):421–441. doi: 10.1016/S0006-3495(79)85313-8. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Hines M., Blum J. J. Three-dimensional mechanics of eukaryotic flagella. Biophys J. 1983 Jan;41(1):67–79. doi: 10.1016/S0006-3495(83)84406-3. [DOI] [PMC free article] [PubMed] [Google Scholar]


