Abstract
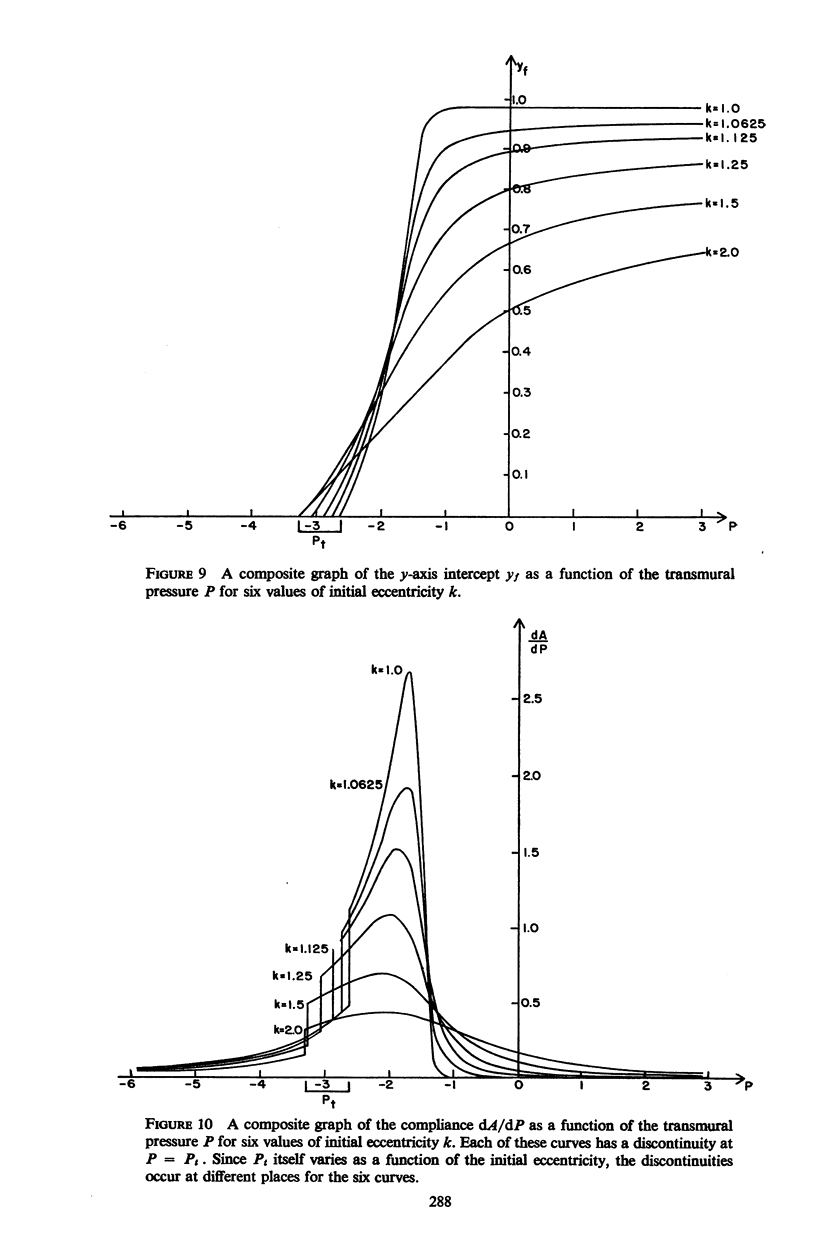
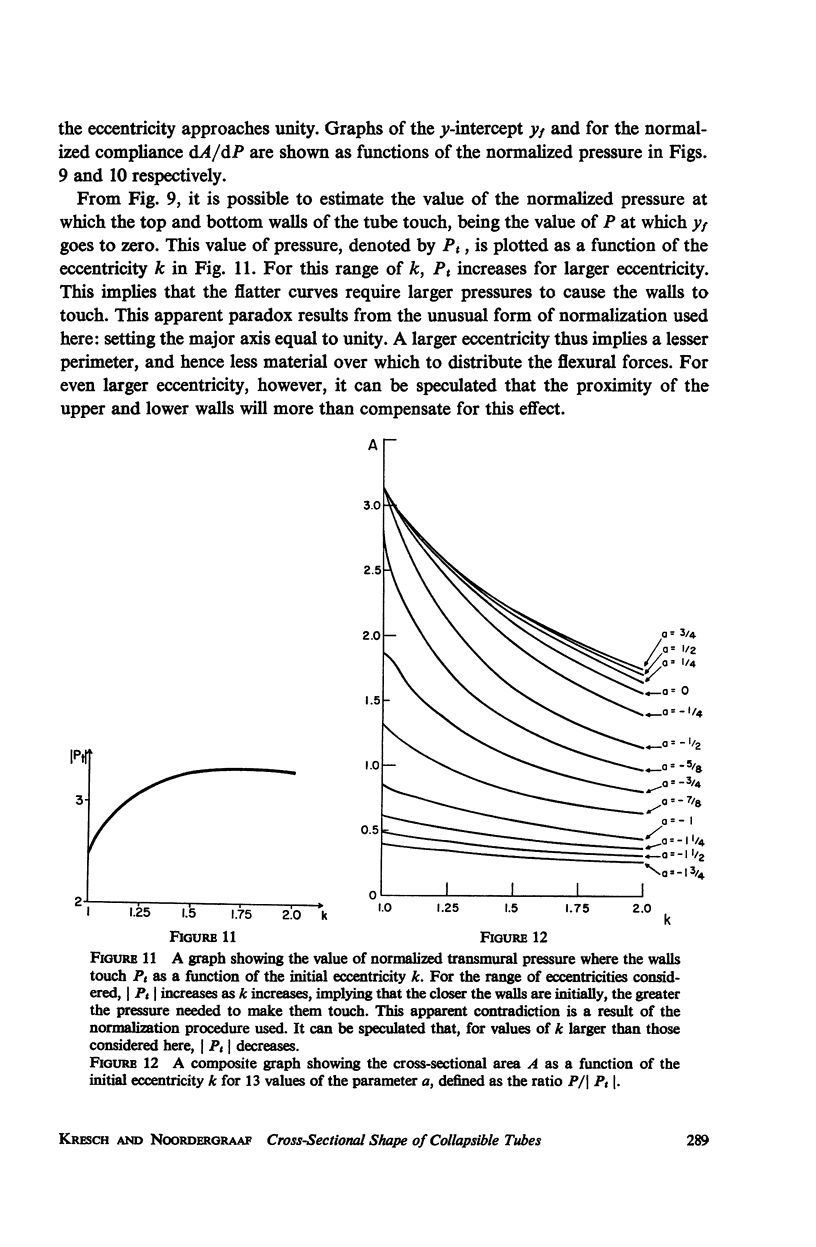
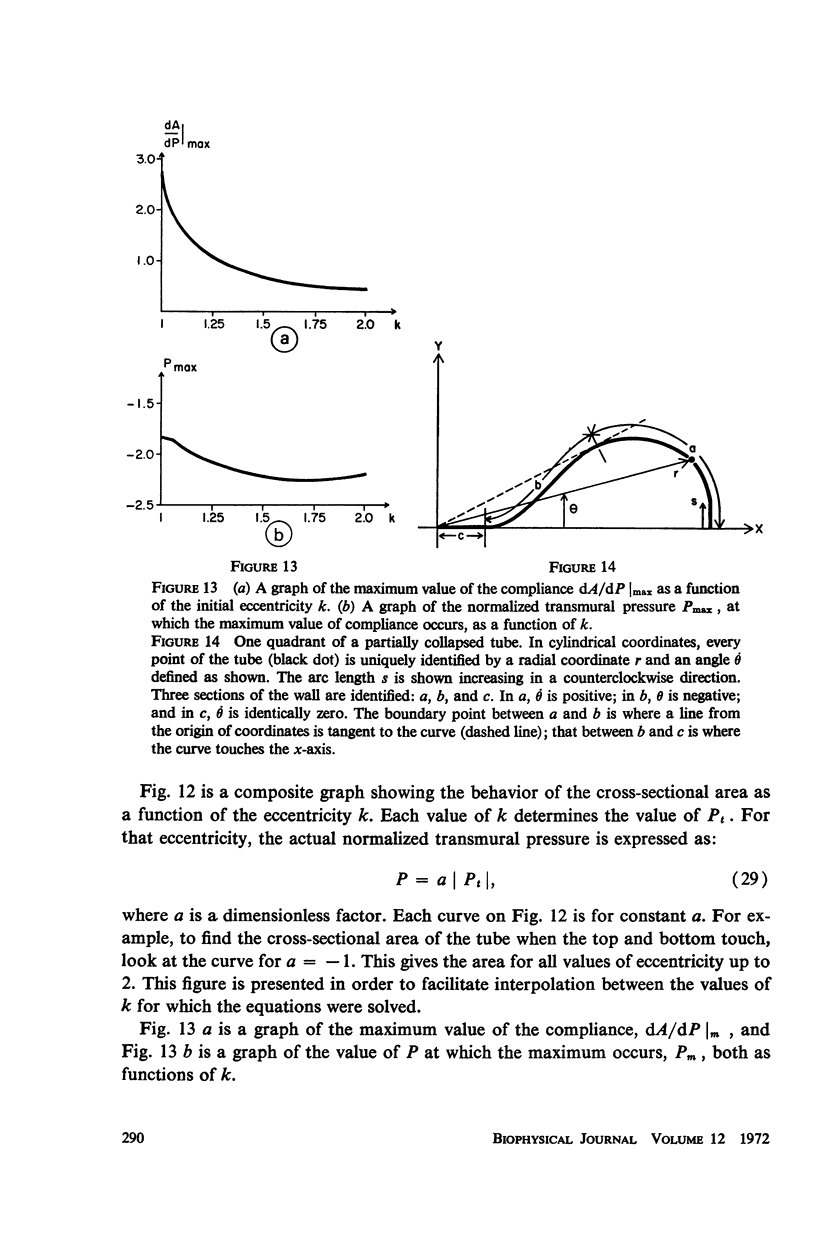
In order to quantify the collapse phenomenon in veins, this paper presents a mathematical analysis of the cross-sectional shape of a flexible tube as its internal pressure varies. Quantitative results are presented in terms of the physical parameters of the tube, such as wall thickness and Young's modulus. It is assumed that the tube is thin walled, that no stretching occurs, that the cross-sectional shape is elliptical when the transmural pressure is zero, and that the longitudinal prestress is zero. The equations were solved on a digital computer which displayed the cross-sectional shapes on an oscilloscope, which were then photographed. A selection of these photographs is presented. Curves are shown which give the cross-sectional area and compliance as functions of transmural pressure. Other curves are shown which are useful for interpolation, and for use in the experimental determination of the physical parameters which may otherwise be difficult or impossible to measure accurately.
Full text
PDF

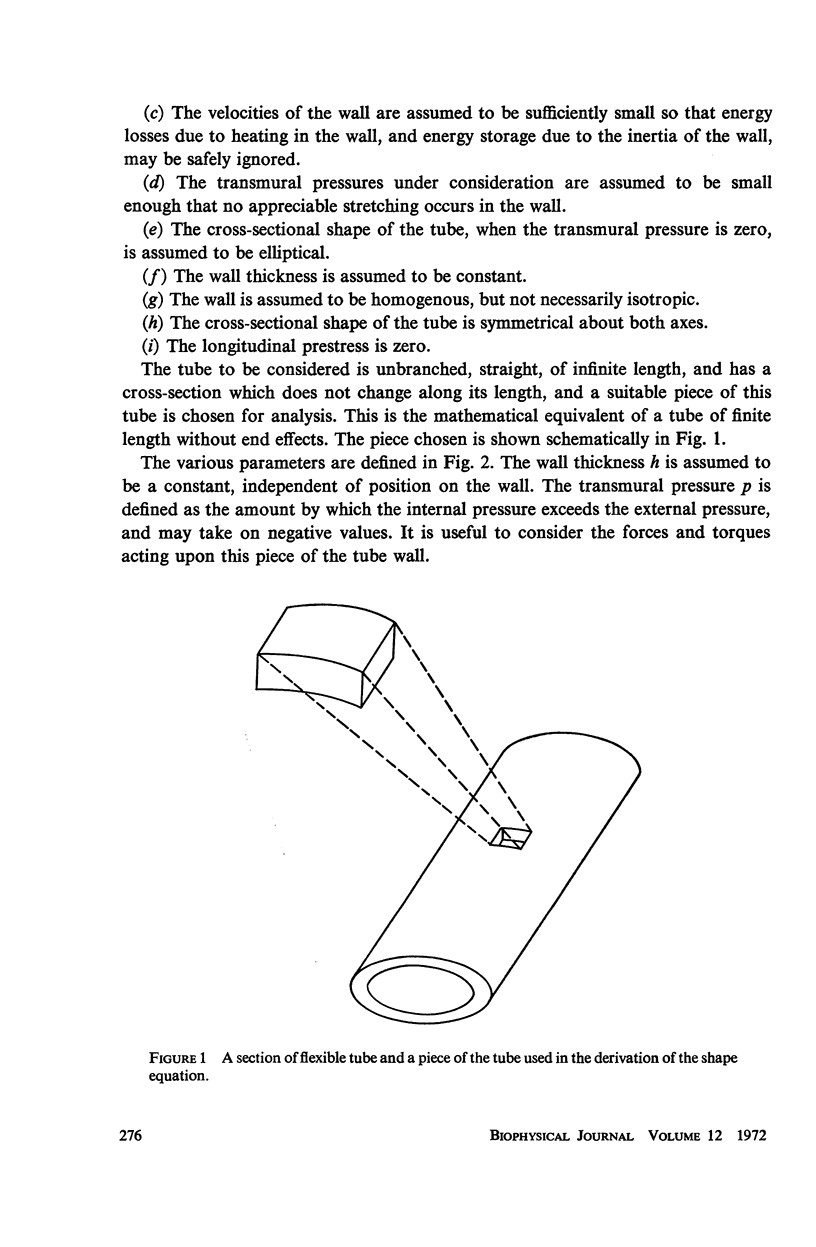
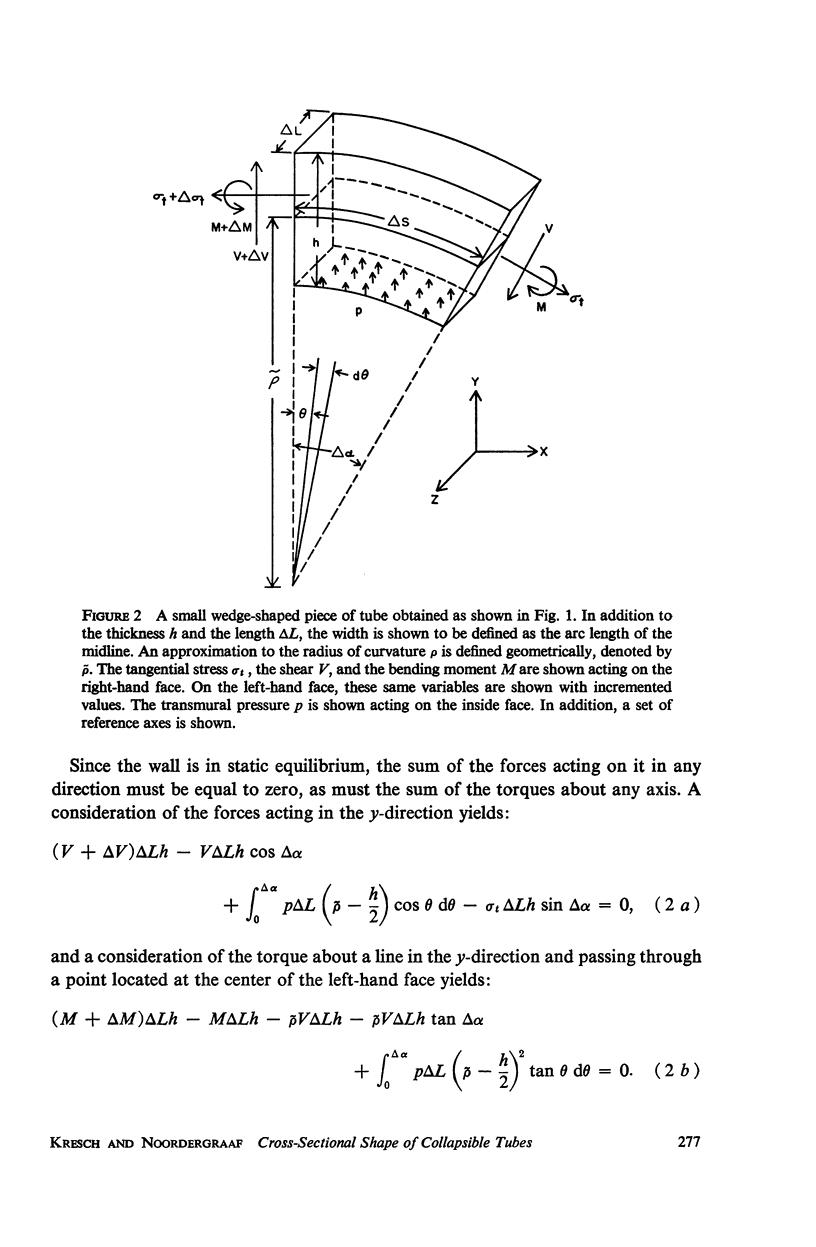

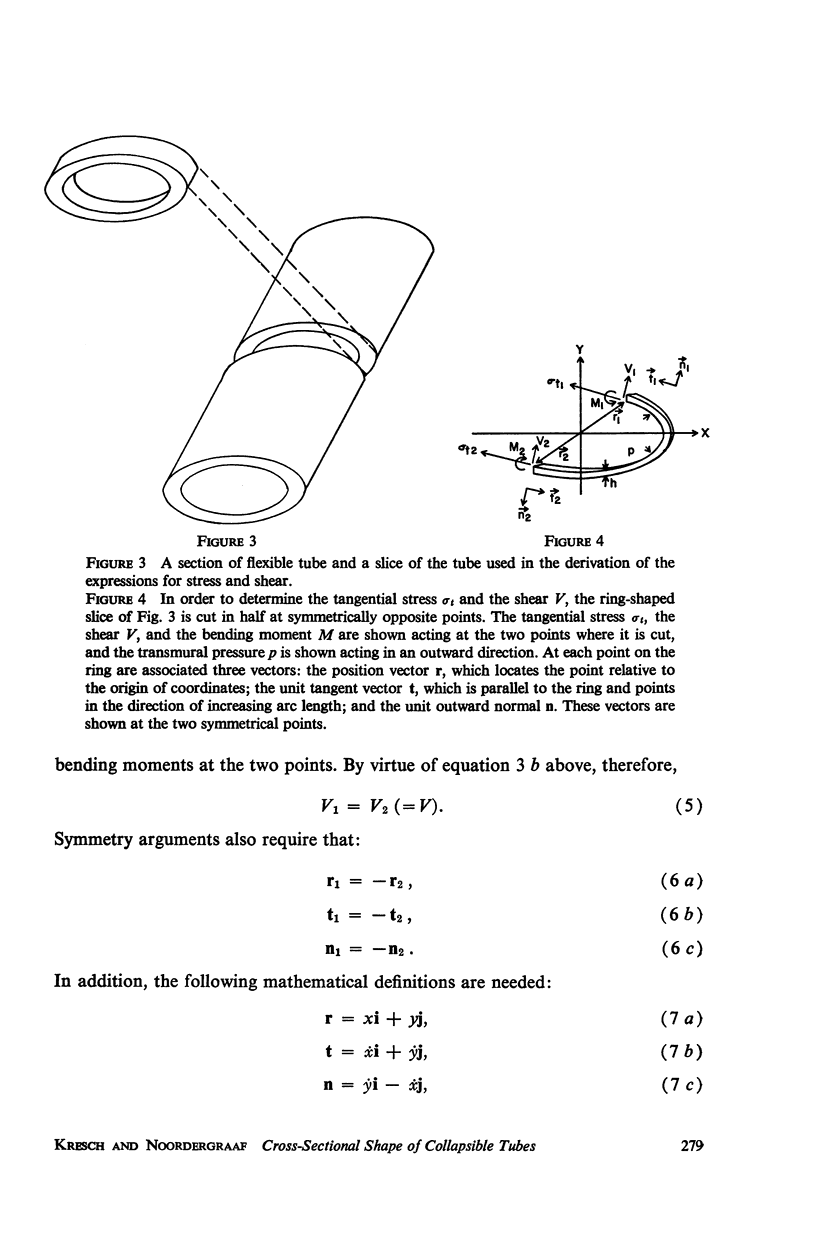


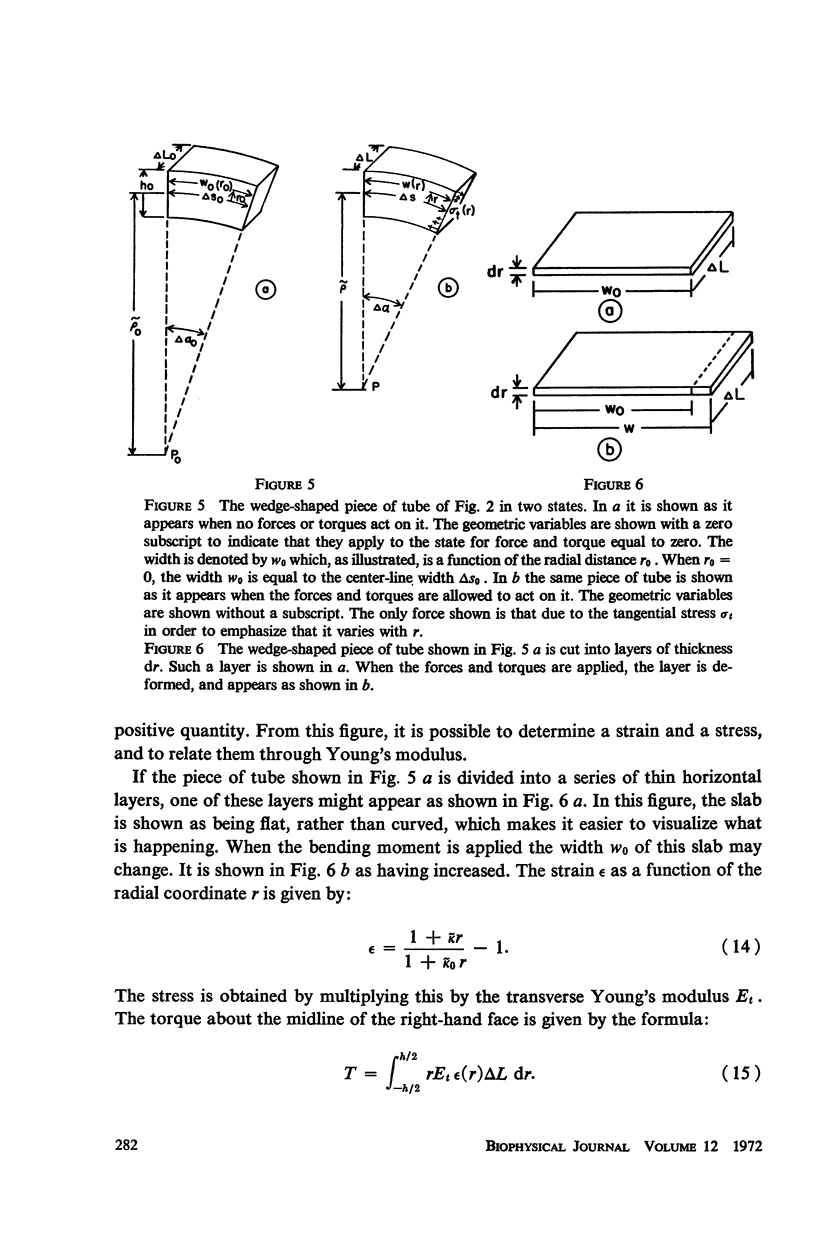





Selected References
These references are in PubMed. This may not be the complete list of references from this article.
- Brecher G. A. History of venous research. IEEE Trans Biomed Eng. 1969 Oct;16(4):236–247. doi: 10.1109/tbme.1969.4502655. [DOI] [PubMed] [Google Scholar]
- Conrad W. A. Pressure--flow relationships in collapsible tubes. IEEE Trans Biomed Eng. 1969 Oct;16(4):284–295. doi: 10.1109/tbme.1969.4502660. [DOI] [PubMed] [Google Scholar]
- Holt J. P. Flow through collapsible tubes and through in situ veins. IEEE Trans Biomed Eng. 1969 Oct;16(4):274–283. doi: 10.1109/tbme.1969.4502659. [DOI] [PubMed] [Google Scholar]
- Moreno A. H., Katz A. I., Gold L. D., Reddy R. V. Mechanics of distension of dog veins and other very thin-walled tubular structures. Circ Res. 1970 Dec;27(6):1069–1080. doi: 10.1161/01.res.27.6.1069. [DOI] [PubMed] [Google Scholar]


