Skip to main content
NCBI home page
Biophysical Journal logoLink to Biophysical Journal
. 1972 Oct;12(10):1302–1315. doi: 10.1016/S0006-3495(72)86164-2

Dissipative Structures for an Allosteric Model

Application to Glycolytic Oscillations

A Goldbeter, R Lefever
PMCID: PMC1484224  PMID: 4263005

Abstract

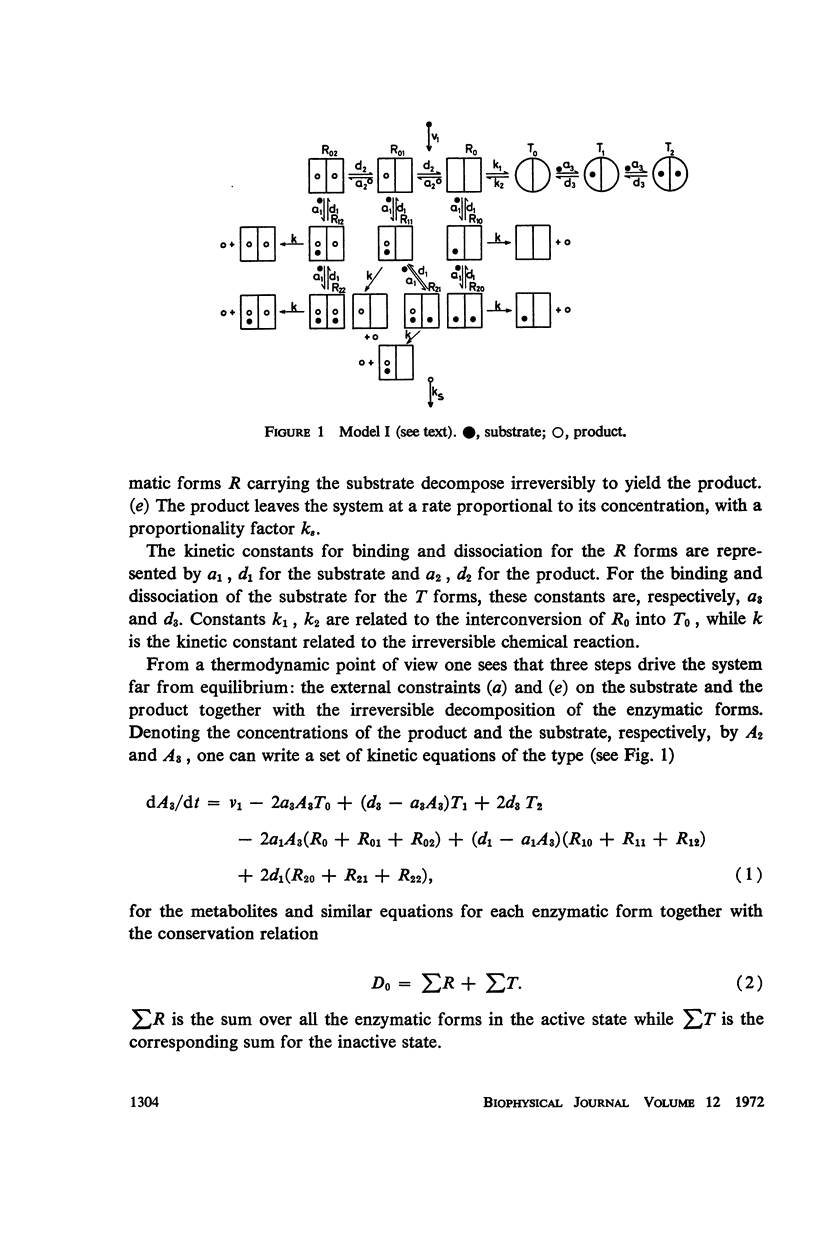
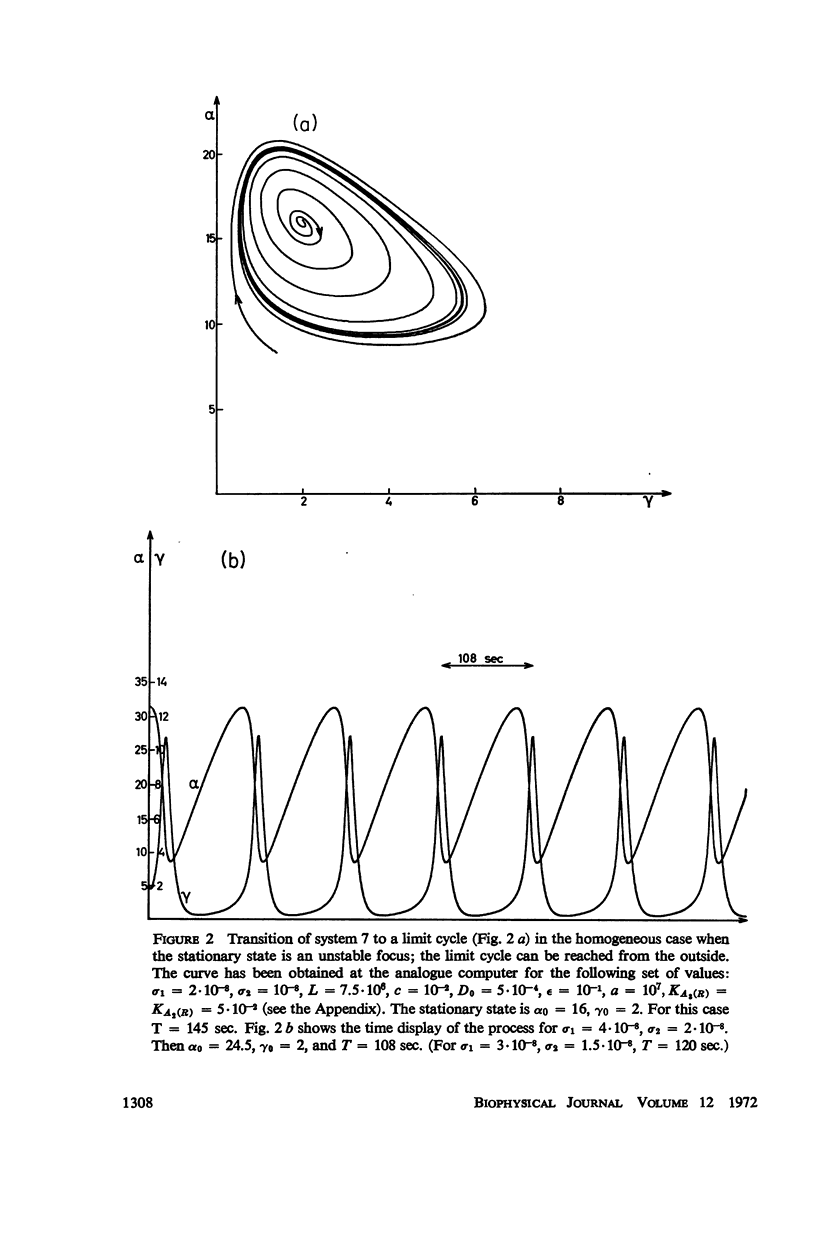
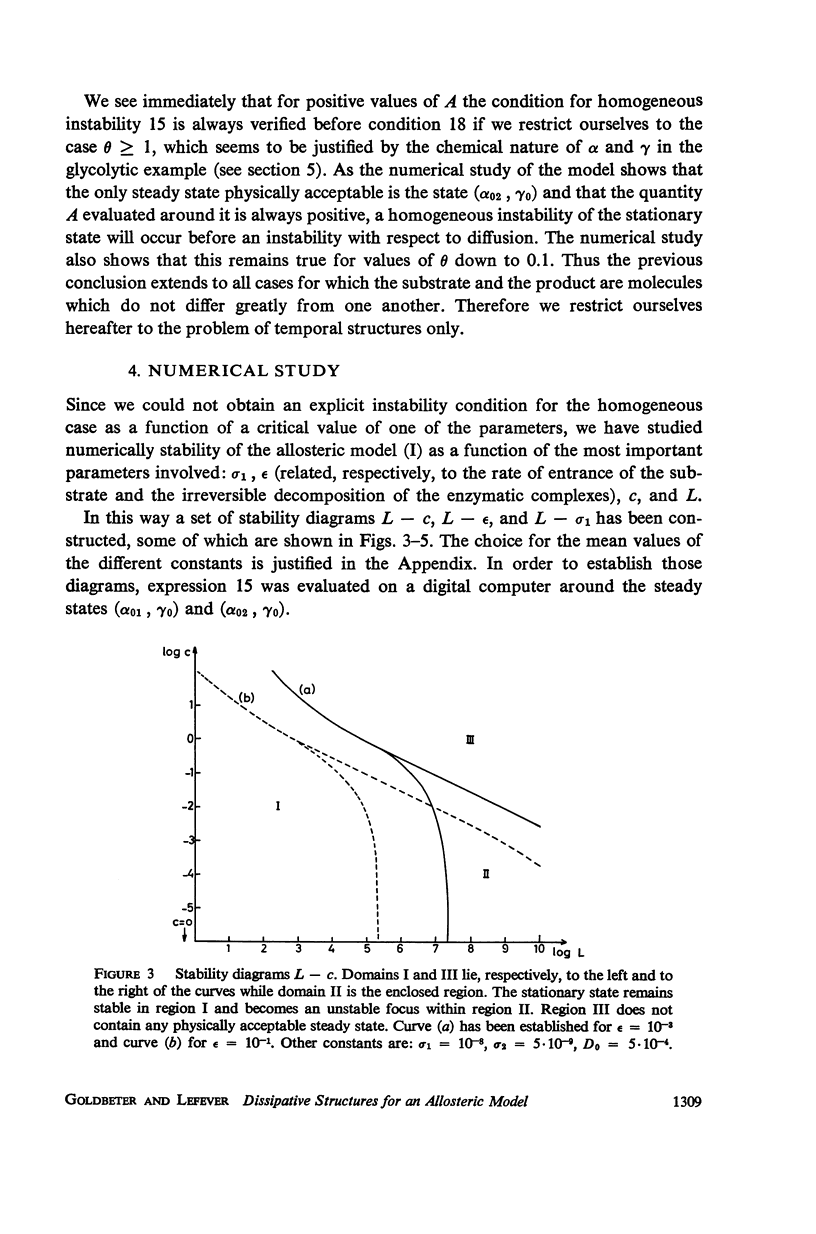
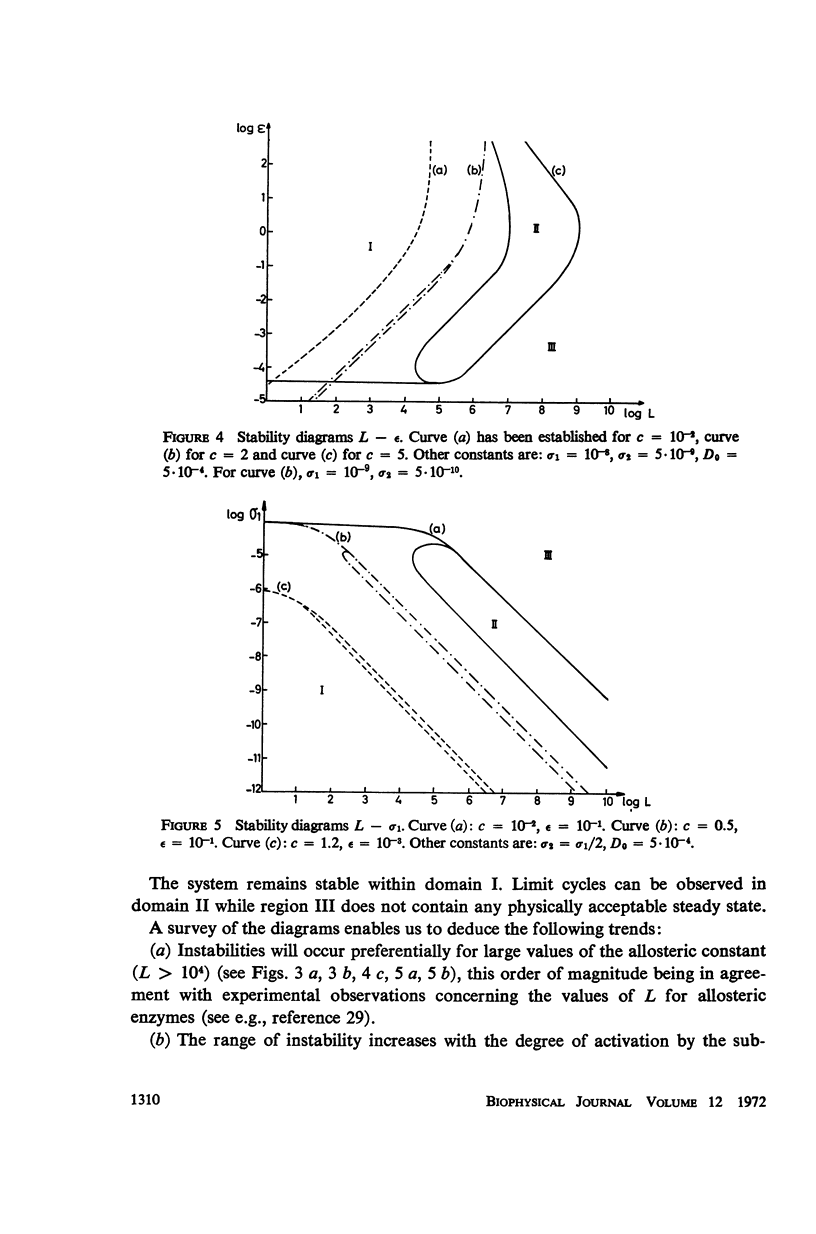
An allosteric model of an open monosubstrate enzyme reaction is analyzed for the case where the enzyme, containing two protomers, is activated by the product. It is shown that this system can lead to instabilities beyond which a new state organized in time or in space (dissipative structure) can be reached. The conditions for both types of instabilities are presented and the occurrence of a temporal structure, consisting of a limit cycle behavior, is determined numerically as a function of the important parameters involved in the system. Sustained oscillations in the product and substrate concentrations are shown to occur for acceptable values of the allosteric and kinetic constants; moreover, they seem to be favored by substrate activation. The model is applied to phosphofructokinase, which is the enzyme chiefly responsible for glycolytic oscillations and which presents the same pattern of regulation as the allosteric enzyme appearing in the model. A qualitative and quantitative agreement is obtained with the experimental observations concerning glycolytic self-oscillations.

Full text

PDF
1300

Selected References

These references are in PubMed. This may not be the complete list of references from this article.

  1. BETZ A., CHANCE B. PHASE RELATIONSHIP OF GLYCOLYTIC INTERMEDIATES IN YEAST CELLS WITH OSCILLATORY METABOLIC CONTROL. Arch Biochem Biophys. 1965 Mar;109:585–594. doi: 10.1016/0003-9861(65)90404-2. [DOI] [PubMed] [Google Scholar]
  2. Betz A., Moore C. Fluctuating metabolite levels in yeast cells and extracts, and the control of phosphofructokinase activity in vitro. Arch Biochem Biophys. 1967 May;120(2):268–273. doi: 10.1016/0003-9861(67)90238-x. [DOI] [PubMed] [Google Scholar]
  3. Blangy D., Buc H., Monod J. Kinetics of the allosteric interactions of phosphofructokinase from Escherichia coli. J Mol Biol. 1968 Jan 14;31(1):13–35. doi: 10.1016/0022-2836(68)90051-x. [DOI] [PubMed] [Google Scholar]
  4. CHANCE B., ESTABROOK R. W., GHOSH A. DAMPED SINUSOIDAL OSCILLATIONS OF CYTOPLASMIC REDUCED PYRIDINE NUCLEOTIDE IN YEAST CELLS. Proc Natl Acad Sci U S A. 1964 Jun;51:1244–1251. doi: 10.1073/pnas.51.6.1244. [DOI] [PMC free article] [PubMed] [Google Scholar]
  5. Ghosh A., Chance B. Oscillations of glycolytic intermediates in yeast cells. Biochem Biophys Res Commun. 1964 Jun 1;16(2):174–181. doi: 10.1016/0006-291x(64)90357-2. [DOI] [PubMed] [Google Scholar]
  6. Goodwin B. C., Cohen M. H. A phase-shift model for the spatial and temporal organization of developing systems. J Theor Biol. 1969 Oct;25(1):49–107. doi: 10.1016/s0022-5193(69)80017-2. [DOI] [PubMed] [Google Scholar]
  7. Goodwin B. C. Oscillatory behavior in enzymatic control processes. Adv Enzyme Regul. 1965;3:425–438. doi: 10.1016/0065-2571(65)90067-1. [DOI] [PubMed] [Google Scholar]
  8. HIGGINS J. A CHEMICAL MECHANISM FOR OSCILLATION OF GLYCOLYTIC INTERMEDIATES IN YEAST CELLS. Proc Natl Acad Sci U S A. 1964 Jun;51:989–994. doi: 10.1073/pnas.51.6.989. [DOI] [PMC free article] [PubMed] [Google Scholar]
  9. Hess B., Boiteux A., Krüger J. Cooperation of glycolytic enzymes. Adv Enzyme Regul. 1969;7:149–167. doi: 10.1016/0065-2571(69)90016-8. [DOI] [PubMed] [Google Scholar]
  10. Hess B., Brand K., Pye K. Continuous oscillations in a cell-free extract of S. carlsbergensis. Biochem Biophys Res Commun. 1966 Apr 6;23(1):102–108. doi: 10.1016/0006-291x(66)90276-2. [DOI] [PubMed] [Google Scholar]
  11. Keller E. F., Segel L. A. Initiation of slime mold aggregation viewed as an instability. J Theor Biol. 1970 Mar;26(3):399–415. doi: 10.1016/0022-5193(70)90092-5. [DOI] [PubMed] [Google Scholar]
  12. Landahl H. D. Some conditions for sustained oscillations in biochemical chains with feedback inhibition. Bull Math Biophys. 1969 Dec;31(4):775–787. doi: 10.1007/BF02477786. [DOI] [PubMed] [Google Scholar]
  13. Lefever R., Nicolis G. Chemical instabilities and sustained oscillations. J Theor Biol. 1971 Feb;30(2):267–284. doi: 10.1016/0022-5193(71)90054-3. [DOI] [PubMed] [Google Scholar]
  14. MONOD J., WYMAN J., CHANGEUX J. P. ON THE NATURE OF ALLOSTERIC TRANSITIONS: A PLAUSIBLE MODEL. J Mol Biol. 1965 May;12:88–118. doi: 10.1016/s0022-2836(65)80285-6. [DOI] [PubMed] [Google Scholar]
  15. Morales M., McKay D. Biochemical oscillations in "controlled" systems. Biophys J. 1967 Sep;7(5):621–625. doi: 10.1016/S0006-3495(67)86611-6. [DOI] [PMC free article] [PubMed] [Google Scholar]
  16. Nakamura S., Yokota K., Yamazaki I. Sustained oscillations in a lactoperoxidase. NADPH and O2 system. Nature. 1969 May 24;222(5195):794–794. doi: 10.1038/222794a0. [DOI] [PubMed] [Google Scholar]
  17. Prigogine I., Lefever R., Goldbeter A., Herschkowitz-Kaufman M. Symmetry breaking instabilities in biological systems. Nature. 1969 Aug 30;223(5209):913–916. doi: 10.1038/223913a0. [DOI] [PubMed] [Google Scholar]
  18. Prigogine I., Nicolis G. Biological order, structure and instabilities. Q Rev Biophys. 1971 Aug;4(2):107–148. doi: 10.1017/s0033583500000615. [DOI] [PubMed] [Google Scholar]
  19. Pye K., Chance B. Sustained sinusoidal oscillations of reduced pyridine nucleotide in a cell-free extract of Saccharomyces carlsbergensis. Proc Natl Acad Sci U S A. 1966 Apr;55(4):888–894. doi: 10.1073/pnas.55.4.888. [DOI] [PMC free article] [PubMed] [Google Scholar]
  20. Waddington C. H. Autogenous cellular periodicities as (a) temporal templates and (b) the basis of "morphogenetic fields". J Theor Biol. 1965 Mar;8(2):367–369. doi: 10.1016/0022-5193(65)90084-6. [DOI] [PubMed] [Google Scholar]
  21. Walter C. Stability of controlled biological systems. J Theor Biol. 1969 Apr;23(1):23–38. doi: 10.1016/0022-5193(69)90065-4. [DOI] [PubMed] [Google Scholar]

Articles from Biophysical Journal are provided here courtesy of The Biophysical Society

RESOURCES