Reinterpretation of gizzard sizes of red knots world-wide emphasises overriding importance of prey quality at migratory stopover sites

Abstract

The size of digestive organs can be rapidly and reversibly adjusted to ecological circumstances, but such phenotypic flexibility comes at a cost. Here, we test how the gizzard mass of a long-distance migrant, the red knot (Calidris canutus), is adjusted to (i) local climate, (ii) prey quality and (iii) migratory fuelling demands. For eight sites around the world (both wintering and stopover sites), we assembled data on gizzard masses of free-living red knots, the quality of their prey and the local climate. Using an energetic cost–benefit approach, we predicted the gizzard size required for fastest fuelling (net rate-maximization, i.e. expected during migration) and the gizzard size required to balance daily energy budgets (satisficing, expected in wintering birds) at each site. The measured gizzards matched the net rate-maximizing predictions at stopover sites and the satisficing predictions at wintering sites. To our surprise, owing to the fact that red knots selected stopover sites with prey of particularly high quality, gizzard sizes at stopovers and at wintering sites were nevertheless similar. To quantify the benefit of minimizing size changes in the gizzard, we constructed a model incorporating the size-dependent energy costs of maintaining and carrying a gizzard. The model showed that by selecting stopovers containing high-quality prey, metabolic rates are kept at a minimum, potentially reducing the spring migratory period by a full week. By inference, red knots appear to time their stopovers so that they hit local peaks in prey quality, which occur during the reproductive seasons of the intertidal benthic invertebrates.

1. Introduction

The realization that size, structure and function of digestive organs can be fine-tuned rapidly and reversibly to local/temporal ecological circumstances (phenotypic flexibility; reviewed by Piersma & Lindström 1997 and Piersma & Drent 2003), raises the question of costs that such adjustments entail. For example, as a response to increased energy demands through cold exposure, house wrens (Troglodytes aedon) increased the length of their small intestines (Dykstra & Karasov 1992). Quail (Coturnix japonica) increased gizzard size, intestine length and mucosal surface when food quality was reduced (Starck & Rahmaan 2003; and see López-Calleja & Bozinovic 2003 for a similar effect in hummingbirds, Sephanoides sephanoides). When fuelling for migration, garden warblers (Sylvia borin) increased their digestive tract size (Hume & Biebach 1996).

Long-distance migrants encounter a variety of climates and food qualities throughout the year and have different energy demands during non-breeding and migration seasons. These factors result in seasonal changes in food requirements (e.g. Piersma 2002). Given the existing empirical relationships between food processing rate and digestive organ size (Van Gils et al. 2003), migrants provide an excellent model for studying how organ size is optimized in relation to external and internal energy demands.

It is increasingly realized that much of the reproductive success of long-distance migrant birds may depend on the ecological conditions encountered long before arrival on the breeding grounds (Ebbinge & Spaans 1995; Drent et al. 2003; Baker et al. 2004). In a world where many stopover sites are under threat, this gives the study of the selection pressures during migration more than academic interest. Arguments for the safeguarding of stopover sites usually come from observations of large concentrations (Wetlands International 2002), their provision of ample food (Myers et al. 1987) or theoretical arguments based on issues of speed of migration (Alerstam & Hedenström 1998). Here we combine these factors in a single argument based on the organ architecture of a shorebird species during different times of the year in relation to diet quality, energy expenditure and migratory phase.

We examine gizzard flexibility in the red knot (Calidris canutus), a medium-sized shorebird that undertakes several non-stop long-distance flights (1 400–6 500 km) between high-arctic breeding grounds and temperate, or tropical non-breeding grounds (Piersma et al. 2005). At these intertidal wintering sites, red knots feed primarily on hard-shelled prey that are generally of poor quality (i.e. low ratio of digestible to indigestible matter). Over the past decade, studies on red knots have quantified (i) the energetic costs of living in (Wiersma & Piersma 1994; Bruinzeel & Piersma 1998) and travelling between (Kvist et al. 2001) sites with contrasting climates (reviewed by Piersma 2002), (ii) the energetic costs and benefits of feeding on prey of different quality (Piersma et al. 2003; Van Gils et al. 2003, 2005a,b) and (iii) fuelling rates at different sites around the world (Gudmundsson et al. 1991; González et al. 1996; Piersma et al. 2005). Recent advances in a non-invasive technique (ultrasonography; see Dietz et al. 1999) enabled us to reveal flexibility in the size of the gizzard enforced by experimental changes in prey quality (Dekinga et al. 2001; Van Gils et al. 2003). This organ is vital in the feeding ecology of the knot as the hard-shelled prey, which provide most of their diet, are ingested whole and crushed in the muscular gizzard (Piersma et al. 1993b; Battley & Piersma 2005). Changes in gizzard size, which can occur rapidly and reversibly (50% within a week; Dekinga et al. 2001), are likely to have an impact on the knot's energy budget (Piersma et al. 2003; Van Gils et al. 2003, 2005b). As rates of shell crushing increase with gizzard size, energetic benefits increase with gizzard size (Van Gils et al. 2003). However, as larger gizzards require larger maintenance and transport costs, energetic costs should also increase with gizzard size (Piersma et al. 2003; Van Gils et al. 2003).

In this paper we predict optimal gizzard sizes at different sites based on energy demand and prey quality. Energy demand varies with (i) local climate and (ii) ‘internal’ energy demands for migratory fuelling. Prey quality affects the amount of bulk material that must be processed to meet the daily energy demands. Optimal gizzard size depends on whether birds are balancing gross energy intake and expenditure (‘satisficing’; Nonacs & Dill 1993; expected during winter when there is no change in body mass), or maximizing net energetic benefits (gross intake minus expenditure; ‘net rate-maximization’; Stephens & Krebs 1986; expected during spring when rapidly fuelling for migration). We tested this for five of the six recognized subspecies of knot (Piersma & Davidson 1992; Tomkovich 2001), for which data on climate, prey quality and gizzard size are available. These are canutus breeding at Taymyr Peninsula and wintering in west and southwest Africa, islandica breeding in north Greenland and northeast Canada and wintering in northwest Europe, rufa breeding in the central Canadian arctic and wintering in southern Patagonia and Tierra del Fuego, rogersi breeding at Chukotskiy Peninsula and wintering in New Zealand and southeast Australia and piersmai breeding on the New Siberia islands and wintering in northwest Australia (figure 1). We considered shellfish-eaters only, but we will discuss exceptions later (horseshoecrab-egg-eating rufa knots during stopover in Delaware Bay; see Castro & Myers 1993). The links not specifically covered in this paper, owing to lack of data, are the movements of roselaari and the 6 900-km journey linking the South Africa canutus wintering sites with West African sites.

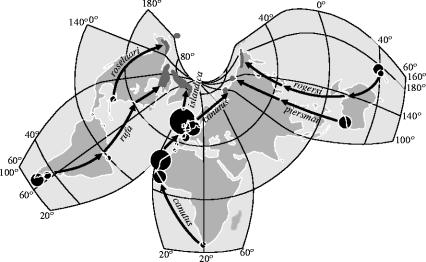
Figure 1.

A map of the world with the knot's flyways (arrows), breeding grounds (dark grey surfaces) and wintering grounds (dots, scaled to estimated wintering population sizes). Distances (km) travelled between main wintering and breeding grounds are 9 000 (canutus), 4 680 (islandica), 15 000 (rufa), 15 000 (rogersi) and 10 400 (piersmai).

2. Material and Methods

(a) Modelling cost-benefit as a function of gizzard size

We modelled two types of gizzards. (i) The so-called ‘satisficing’ gizzards balance gross energy income with energy expenditure on a daily basis. (ii) The so-called ‘net-rate maximizing’ gizzards maximize net energy income on a daily basis (gross energy income minus energy expenditure). Of what size these two types of gizzard turn out to be, depends on how energetic benefits and energetic costs scale with gizzard size G. These relations are clarified in figure 2a and parameterized below.

Figure 2.

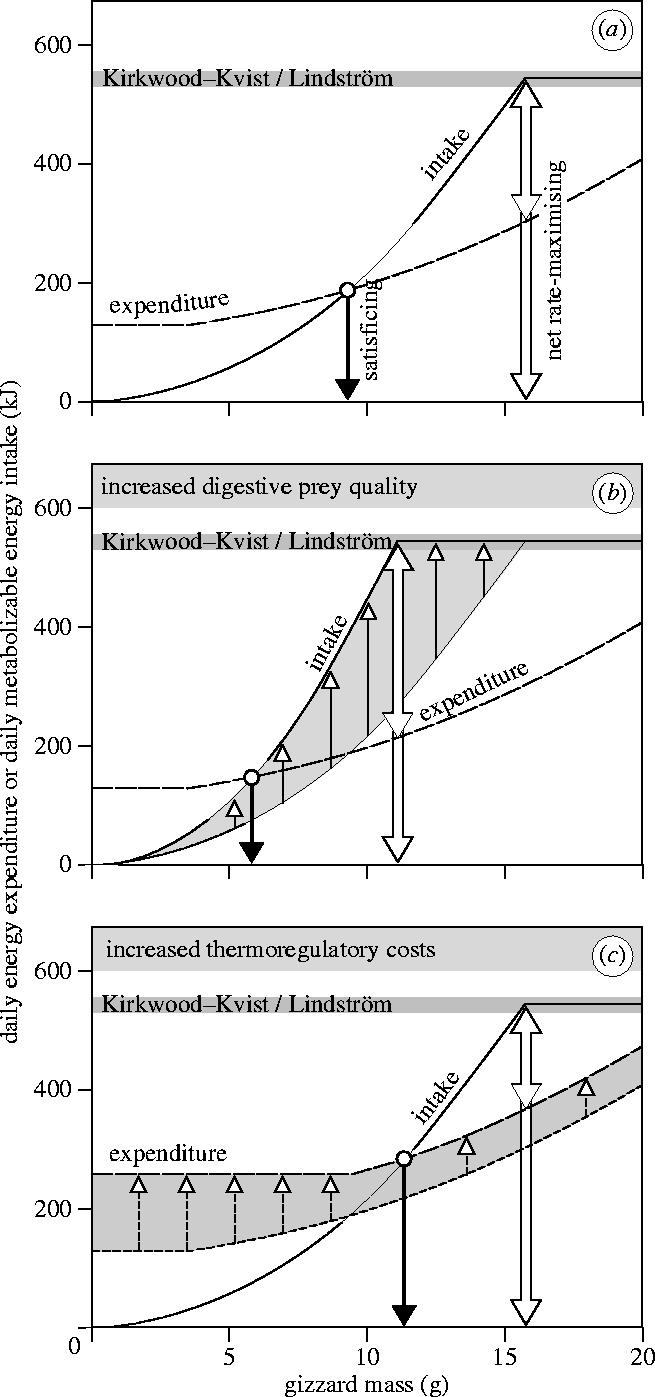
Daily energy expenditure and daily metabolizable energy intake as a function of gizzard size, to demonstrate the two optimal gizzard sizes. (a) Intake increases quadratically with gizzard mass up to a level constrained by other processes in the physiology of digestion (Kirkwood–Kvist/Lindström bar). The optimal satisficing gizzard mass is at the intersection of the two curves, indicated by the solid, left arrow. Net daily energy intake is maximized when (gross) daily intake hits the Kirkwood–Kvist/Lindström constraint; the open, right arrow points at the optimal net rate-maximizing gizzard mass (where the upper part of the arrow indicates the maximum net daily energy intake). The parameters in this example are given standard values for TRC (1 W) and prey quality (1 kJ g⁻¹ DM_shell). (b) An enhanced intake (through a higher digestive prey quality of 2 kJ g⁻¹ DM_shell) decreases both the satisficing and the net rate-maximizing gizzard mass. (c) An increased expenditure level (through a higher TRC of 2.5 W) increases the satisficing gizzard mass but not the net rate-maximizing gizzard mass. Note that costs scarcely increase at the lowest gizzard sizes because all of the HIF produced by small gizzards is assumed to substitute for TRC.

We emphasize that we have followed the lead of optimal foraging theory (Stephens & Krebs 1986) by explicitly modelling energetic currencies in order to understand variation in gizzard mass. We realize that this somewhat limited approach ignores associated non-energetic costs, for example risk of predation, which may increase with gizzard size due to mass-dependent predation risk (MacLeod et al. 2005). Moreover, we neglect additional mortality costs that generally come with increased rates of energy assimilation (Yearsley et al. 2002) or expenditure (Daan et al. 1996), for example through an increased release of free radicals (Finkel & Holbrook 2000). We simply assume that birds either balance or maximize their energy budget, no matter the amount of energy this takes (an energetic currency that could partly account for expenditure-induced costs would be (the modified form of) efficiency, which expresses gross energy gained per unit of energy expended (Houston 1995; Hedenström & Alerstam 1995)). The reasons that, in spite of these notions, we have focused on two energetic currencies only are: (i) simplicity and, most importantly, (ii) knowledge about parameter-values. In contrast to the body of knowledge that exists on energetic cost functions in knots (reviewed in §1), we have no clue how non-energetic mortality costs scale with gizzard size, although studies on predation-related cost functions in knots are being made (Van den Hout, in preparation). Thus, in order to make tractable and reliable predictions, we restricted ourselves to a cost-benefit model based on energetics only.

When modelling energetic benefits as a function of G we relied on the observation that the amount of shell material that can be crushed and processed per unit time increases quadratically with gizzard mass (10^−4.293 g shell mass s⁻¹ g⁻² gizzard mass; Van Gils et al. 2003). Rates of metabolizable energy intake (MEIR; W) can therefore be lifted by increasing gizzard size (G; g) and/or selecting prey with high flesh-to-shell ratios (prey quality Q; J metabolizable energy g⁻¹ shell mass). This can be formalized to MEIR=10^−4.293 G²Q (Van Gils et al. 2003). See figure 2b for effects of prey quality on optimal gizzard size.

Prey quality Q was determined as precisely as possible by selecting only prey species and sizes fed upon by knots (see electronic supplementary material for diet composition and table 1 for resulting values of Q). Since knots ingest their shelled, largely indigestible prey whole, their faeces reveal much about their diet. Shell fragments can usually be identified to species-level and hinge-sizes allow reconstructions of prey size (Dekinga & Piersma 1993). In this way, hard parts of occasionally ingested crustaceans can also be found and identified (Van Gils et al. 2003, 2005b). Following this methodology, we reconstructed digestive prey quality for each subspecies at most sites, except for three where Q was extracted from the literature (islandica at the wintering site from Van Gils et al. (2003) and at stopover from Alerstam et al. (1992) and canutus and rufa at stopover from Van Gils et al. (2003) and González et al. (1996), respectively). Flesh and shell mass for each bivalve prey species and where possible each relevant size class, were determined. This was done by removing the flesh from the shells, drying both flesh and shell for three days to constant mass at 60°C and finally measuring dry masses to the nearest 0.1 mg (yielding DM_flesh and DM_shell, respectively). Next, the dried flesh was incinerated for 2 h at 550°C to determine ash-free dry mass (AFDM_flesh). After averaging AFDM_flesh and DM_shell, we calculated Q as $da{\text{AFDM}}_{\text{flesh}}/{\text{DM}}_{\text{shell}}$, where d is energetic density of the flesh (22 kJ g⁻¹ AFDM_flesh; Zwarts & Wanink 1993) and a is assimilation efficiency (0.8; Kersten & Piersma 1987; Piersma 1994). Note that Piersma (1994) found that assimilation efficiencies did not vary between individual birds or between prey species.

Table 1.

Site and sample data for the five subspecies of red knot, with predicted TRCs (W;±s.e.), average digestive prey quality (kJ g⁻¹ DM_shell;±s.e.), expected satisficing and net rate-maximizing gizzard masses (g; s.e.-confidence interval in brackets), the number of gizzards sampled and the observed gizzard masses (g; mean±s.e.).

subspecies	migratory phase	site	month	TRC (W)	prey quality (kJ g−1 DMshell)	exp. satisficing gizzard mass (g)	exp. net rate-maximizing gizzard mass (g)	N gizzards sampled	obs. mean gizzard mass±s.e. (g)	reference
canutus	wintering	Banc d'Arguin, Mauritania	December	1.49±0.27	0.89±0.07	10.51 (9.70–11.38)	16.64 (15.71–17.67)	6	9.89±0.53	this study
canutus	stopover	Wadden Sea, NW-Europe	May	1.99±0.03	3.74±0.03	5.73 (5.68–5.78)	8.14 (7.93–8.34)	2	8.00±0.30	Van Gils et al. (2003)
islandica	wintering	Wadden Sea, NW-Europe	January	2.93±0.02	2.10±0.03	8.49 (8.40–8.58)	10.86 (10.55–11.17)	60	8.75±0.18	Van Gils et al. (2003)
islandica	stopover	Selvogur, SW-Iceland	May	2.26±0.04	2.35±0.31	6.33 (5.91–6.85)	9.45 (8.70–10.35)	8	7.95±0.48	Piersma et al. (1999), Alerstam et al. (1992)
rufa	wintering	Tierra del Fuego, S-Argentina	February	1.82±0.06	2.03±0.14	7.21 (6.92–7.53)	11.04 (10.47–11.66)	13	8.09±0.20	this study
rufa	stopover	San Antonio Oeste, E-Argentina	March	1.35±0.05	3.50±0.23	5.09 (4.89–5.31)	8.41 (7.97–8.87)	7	8.17±0.20	González et al. (1996)
rogersi	wintering	Northern New Zealand	March	1.30±0.03	0.82±0.04	9.55 (9.29–9.84)	15.49 (14.82–16.20)	5	9.31±0.64	Battley & Piersma (1997)
piersmai	wintering	Roebuck Bay, NW-Australia	January–April	0.67±0.04	2.22±0.30	5.91 (5.53–6.39)	10.55 (9.70–11.59)	24	5.94±0.36	this study

References are to detailed studies at the particular site.

In order to model energetic costs as a function of G, we used the linear relation between basal metabolic rate BMR (W) and lean body mass L (g) as observed by Piersma et al. (1996; BMR=0.0081 L – 0.046) and assumed that L equals 100 g+G+ intestine mass (Van der Meer & Piersma 1994) and that gizzard mass scales to intestine mass in a one-to-one isomorphic relationship (as observed by Piersma et al. 2003), or formally L=100+2G. The metabolic rate due to flying MR_fly (W) scales to body mass B (g) as MR_fly=10^0.39 B^0.35−0.95 (Kvist et al. 2001). Metabolic rate due to walking MR_walk (W) scales to body mass B (g) in the following manner: ${\text{MR}}_{\text{walk}}=v840{(B/100)}^{2.90}$(Bruinzeel et al. 1999), where v is a walking speed of 0.072 m s⁻¹ (Piersma et al. 2003). In order to equate these mass-dependent equations as a function of gizzard mass, we assume that B=L (i.e. no fat is assumed; as fat is metabolically inactive this assumption hardly affects the calculations through the relatively small transport costs). Metabolic rate owing to probing equals 0.47 W (Piersma et al. 2003) and heat increment of feeding (HIF) amounts to 5.2 kJ g⁻¹ AFDM_flesh digested (Piersma et al. 2003).

Finally, thermoregulatory cost (TRC) is estimated on the basis of Wiersma & Piersma (1994), who provide (i) direct estimates of TRC for islandica and canutus and (ii) an estimate of thermal conductance that we used to translate ambient temperatures into TRC for the other three subspecies (listed in table 1; using data in Stans et al. 1972). This aspect of cost is exemplified in figure 2c. We assume that some heat-generating sources can contribute towards TRC, which makes life in the cold considerably cheaper. Walking generates most of its heat close to the skin and therefore only 30% of it can be ‘kept’ and used as a substitute for TRC (Bruinzeel & Piersma 1998). Since HIF represents heat generated in the core of the body, 100% of it can potentially substitute for TRC (and does, K. Jalvingh & F. Vézina unpublished data). The ‘maintenance cost’ as mostly used by Wiersma & Piersma (1994) and Piersma (2002) is embodied in the well known ‘Scholander-curve’ (Scholander et al. 1950) which equals TRC below and BMR above the lower critical temperature (Scholander et al. 1950).

To complete the calculations on the daily energy budget, we multiplied these expenditure and intake rates with daily time allocations to foraging, flying and resting. Red knots feed at exposed intertidal mudflats during day and night and therefore have daily foraging periods of 12 h on average (Piersma et al. 1994; Van Gils & Piersma 1999; Van Gils et al. 2000). This value was used for all subspecies at all sites except for islandica stopping over at Iceland (14.2 h; Alerstam et al. 1992) and for rogersi wintering in New Zealand (15 h; P.F.B. unpublished data). For all subspecies at all sites we further assumed that 0.5 h day⁻¹ was spent in flight (mainly to commute between roosts and feeding grounds; Rogers 2003), except for knots living in the Wadden Sea (islandica in winter and canutus during stopover) that were assumed to fly for 1 h day⁻¹ owing to larger daily foraging ranges compared to other sites (Piersma et al. 1993a). The remainder of the day was spent at rest.

Satisficing gizzard sizes were predicted from these parameters by solving for G while equating total income and expenditure on a daily basis (i.e. net energy gain=0; solid arrow in figure 2). By contrast, net energy gain is maximized at a gizzard size G above which gross energy gain has stopped to increase with G while the energetic costs still do (open arrow in figure 2). This is the smallest gizzard at which MEIR= MEIR_max, where MEIR_max represents the ultimate limit to food intake as reviewed by Kirkwood (1983) and measured by Kvist & Lindström (2003; i.e. 12.6 W (s.e.=0.5 W) when feeding for 12 h a day or 544 kJ day⁻¹ (s.e.=22 kJ day⁻¹) as indicated by the shaded bar in figure 2). Both studies showed that food intake is subject to an upper limit, which generally scales with a species' body size. This upper limit may either be set centrally by the rate at which energy can be absorbed by the gut, or set peripherally by the rate at which organs can expend energy (Kirkwood 1983; Weiner 1992; Hammond & Diamond 1997; Karasov & McWilliams 2005). Until now, this issue has remained unanswered and for reasons of parsimony we therefore assumed that MEIR_max is unaffected by gizzard size (figure 2; whether this assumption holds needs to be explored in future studies). Then, net-rate maximizing gizzard size can simply be found by rewriting the quadratic intake-rate function (given above), while setting MEIR to MEIR_max: $G=\sqrt{({\text{MEIR}}_{\text{max}}/Q{10}^{-4.293})}$.

(b) Observed gizzard sizes

Fresh masses of gizzards were determined either directly through dissections of carcasses (N=45), or indirectly through ultrasonography on live birds (N=80). Both methods have been calibrated extensively (Dietz et al. 1999), which gave us the opportunity to apply ultrasonography in both experimental (Dekinga et al. 2001; Van Gils et al. 2003, 2005a) and field (Van Gils et al. 2005b) studies. Most carcasses were collected as catching casualties (N=32); rogersi birds (N=5) were recovered from poachers, while a few were obtained by shooting under license (N=8; islandica at the Iceland stopover analysed by Piersma et al. (1999); collection was justified because, at that time (1994), casualties were unavailable, while the technique of ultrasonography had yet to be developed). The birds that were measured by ultrasound were caught with mistnets (N=60; islandica at its wintering site) or with cannon-nets (N=20; the majority of the piersmai individuals). Table 1 gives the details on catch site, sample size and date (indicated by month, but note that the birds have been sampled in different years ranging from 1987 to 2002). For islandica stopping over on northward migration in Iceland, to ensure that only actively fuelling birds were analysed, we selected birds from the middle of the stopover period (10 May). Because rogersi in New Zealand have been shown to dramatically reduce gizzard size before departure on migration (Battley & Piersma 1997), we only analysed resident non-fuelling juvenile birds.

3. Results

(a) Model parameters and predictions

For each site, we predicted the optimal satisficing and rate-maximizing gizzard mass based on climate, energy demand and prey quality (table 1; illustrated in figure 3), while taking standard errors in the parameter values into account (see table 1). In figure 3, optimal gizzard size (right axis) follows from the daily amount of shell material that must be processed (left axis), which, in turn, follows from the daily amount of energy that is required (horizontal axis; equal to the Kirkwood–Kvist/Lindström constraint for fuelling birds) and the amount of metabolizable energy per gram of shell material (=prey quality given by diagonal lines). Due to low prey qualities for wintering sites, we predict relatively large satisficing gizzards, especially so in the case of canutus and rogersi (around 10 g: figure 3b). Satisficing gizzards of overwintering islandica are predicted to be relatively large owing to the combination of low quality prey at high TRC (figure 3b). By contrast, due to high prey qualities for stopover sites, we predict relatively small net-rate maximizing gizzards. This pattern holds for each subspecies for which we have stopover data available (islandica, canutus and rufa: table 1; figure 3b). Through this shift in prey quality, knots are predicted to keep their gizzards more or less at the ‘satisficing-in-winter size’ when fuelling at stopovers (arrows in figure 3b).

Figure 3.

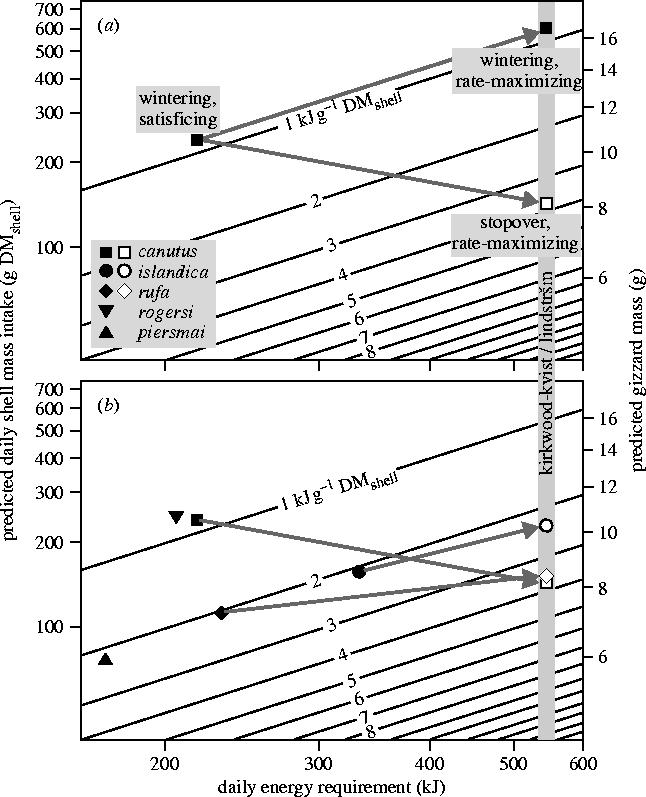
Daily energy requirement (horizontal axis) and prey quality (diagonal lines) predict the daily mass of shell material processed (left vertical axis) and thus predict the required gizzard size (right vertical axis). (a) The prediction for satisficing and rate-maximizing C. c. canutus, both at wintering (filled squares) and stopover sites (open squares). Arrows indicate the expected change in gizzard mass when changing from wintering/satisficing conditions to wintering/rate-maximizing or to stopover/rate-maximizing conditions. (b) Predictions for all subspecies (ignoring rate-maximization during winter). Due to an increase in prey quality, little change in gizzard size is required when shifting from wintering to stopover sites (arrows). Note that the gizzard scale is based on 12 h of foraging per day, which holds for all cases except for wintering rogersi (15 h) and stopping-over islandica (14.2 h). In these two cases, predicted gizzard mass is actually somewhat smaller than plotted here ($\sqrt{12/15}$ and $\sqrt{12/14.2}$ times the plotted gizzard mass).

(b) Observed gizzard sizes

Observed individual gizzard masses varied between 3.7 and 12.7 g and were smallest in wintering piersmai and largest in wintering canutus (figure 4; table 1). Gizzards at wintering sites did not differ from predicted satisficing sizes (p>0.15, using the ‘combined probability test’ as proposed by Sokal & Rohlf (1995); we compared test statistic −2Σln p=13.74 with χ²_2×5) and were smaller than the predicted net rate-maximizing sizes (p<0.0005, i.e. comparing −2Σln p=63.58 with χ²_2×3: figure 4; table 1). Conversely, gizzards at stopover sites did not differ from predicted net rate-maximizing sizes (p>0.4, i.e. comparing −2Σln p=5.90 with χ²_2×3) and were larger than the predicted satisficing sizes (p<0.0005, i.e. comparing −2Σln p=129.03 with χ²_2×5: figure 4; table 1). However, stopover gizzards were of similar size to the wintering gizzards (p>0.1, taking differences between subspecies into account, N=125, R²=0.41).

Figure 4.

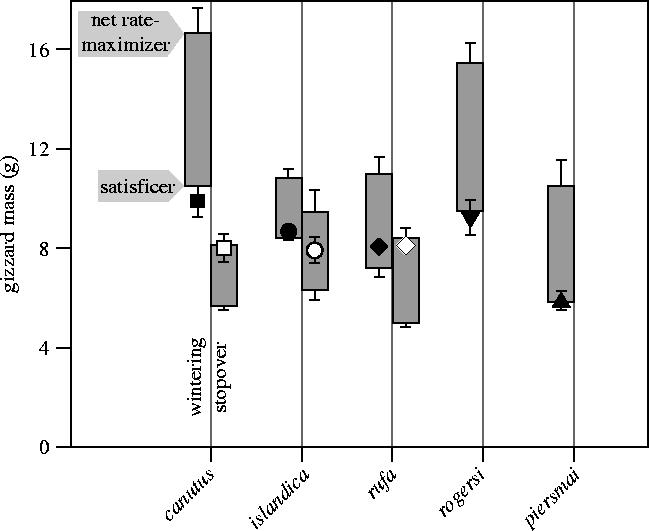
Observed gizzard masses (mean±s.e.) for each subspecies at its wintering site (closed symbol) and stopover site (open symbol, where data are available). Boxes in the background give range in expected gizzard mass (± s.e.), where each box spans the expected gizzard mass of satisficing (bottom) and net rate-maximizing birds (top).

4. Discussion

Gizzards at stopovers and gizzards at wintering sites were of similar size (figure 4). This may seem counterintuitive, especially since ‘stopover gizzards’ were of rate-maximizing size while ‘wintering gizzards’ were of satisficing size (figure 4; table 1). However, prey qualities at stopovers were on average twice those at wintering sites (figure 3b; N=8; p<0.05). This enabled gizzards of the same size to accommodate a maximal fuelling mode (arrows in figure 3b). When migrating northwards, the increase in prey quality for canutus is of such magnitude (from 0.89 to 3.74 kJ g⁻¹ DM_shell) that fuelling ‘spring gizzards’ are predicted and observed to be 2 g smaller than satisficing ‘winter gizzards’. With an increase in prey quality from 2.03 to 3.50 kJ g⁻¹ DM_shell, it seems as if rufa uses its relatively nearby stopover in San Antonio Oeste (‘only’ 1 400 km away from the wintering grounds at Tierra del Fuego) as a springboard to escape from the Patagonian wintering grounds. The increase in prey quality is smallest in the temperate-zone wintering islandica (from 2.10 to 2.35 kJ g⁻¹ DM_shell), but in this case fuelling in Iceland with relatively small gizzards seems feasible by foraging longer than 12 h (Alerstam et al. 1992), although not at maximal rates (see figure 4).

Apparently, red knots only utilize the stopover sites that harbour prey of high quality. This agrees with theoretical predictions (Alerstam & Lindström 1990) and other empirical studies on red knots (those listed by Gudmundsson et al. 1991; Van Gils et al. 2005b): poor-quality stopovers ought to be skipped and indeed are. In an attempt to quantify the benefits of selecting high-quality stopovers, we modelled total duration of migration as a function of stopover selection for a (theoretical) knot migrating 15 000 km (figure 5). The model builds on our previous calculations, but now includes fattening. Fuelling birds deposit up to 100 g of fat at a biosynthesis-cost of 0.33 J per J of tissue (Ricklefs 1974). The next step in our model is their long-distance flight. During long-distance flight, the burning of 1 g of fat yields 40 kJ. To take into account the instantaneous changes in energetic costs with changing amounts of fat (increasing when fuelling and decreasing when flying), the model runs at discrete time steps of 1 s. Across any prey quality, net-rate maximizing gizzards always yield a gross rate of energy gain that equals the upper physiological limit as empirically derived by Kirkwood (1983) and Kvist & Lindström (2003; grey bars in figures 2 and 3). However, as net-rate maximizing gizzard size declines with increasing prey quality (figure 5a cf. figure 2b), the overall cost of transport and maintenance declines likewise with increasing prey quality (figure 5b).

Figure 5.

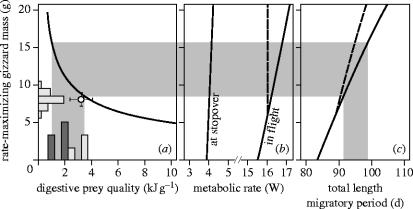
The predicted effect of digestive prey quality at stopovers on overall speed for a theoretical, but realistic, knot population to migrate 15 000 km from wintering to breeding grounds. (a) Expected net-rate maximizing gizzard mass declines with prey quality (solid curved line; see eq. given in §2). Given the observed frequency distribution of prey qualities among sites (bars on horizontal axis), knots only select stopovers that harbour the highest prey qualities (light grey bars; dark grey bars represent wintering sites). As predicted, gizzards observed at stopovers are distributed around 8 g (bars on vertical axis). Mean stopover gizzard mass versus mean stopover prey quality is given by open dot (bars are 95% confidence intervals). (b) Metabolic rates are predicted to increase as a function of gizzard size, especially during flight. (c) Through these higher energetic costs, the total length of the migratory period increases with increasing gizzard size (i.e. declines with increasing prey quality). Grey band across the three figures shows that, according to the model, stopping over in the best instead of in the worst sites should reduce length of migratory period by about a week. Downsizing the gizzard before flight (to 6 g with no time or energy costs of transformation), results in only a minor reduction of the migratory period (dashed line in b and c).

This cost-reduction by minimizing gizzard size brings two advantages that should, according to the model, reduce overall duration of migration (by about one day per gram decline in gizzard mass; figure 5c). First, at the stopovers, higher net rates of energy gain (= gross gain minus costs) are achieved, leading to shorter stopover times (P_dep increases, using the terminology of Hedenström & Alerstam 1998). Second, during flight, metabolic rate is reduced and thus longer distances can be travelled per gram of stored fat, which reduces required stopover time per distance travelled (P_flight decreases, using the terminology of Hedenström & Alerstam 1998). Even though this second effect is stronger than the first on an instantaneous basis (given by differences in slopes in figure 5b), overall, most energy is saved at stopovers, since much more time and thus energy, is spent at stopovers than in flight (cf. Wikelski et al. 2003). Summarizing, overall speed of migration should improve by selecting stopovers that harbour high-quality prey. The overall speed of migration expected by the model (15 000 km in 91 days=165 km day⁻¹) approaches the value of 175 km day⁻¹ actually observed in red knots (Hedenström & Alerstam 1998).

We extended the model by including the flexibility to further reduce gizzard size just before the onset of a long-distance flight down to 6 g (as observed by Battley & Piersma 1997; Piersma et al. 1999). We assumed such transformations incur no time or energy costs. Recent evidence suggests that the energetic costs involved may indeed be minor. Overgaard et al. (2002) found that growth of gastrointestinal organs in pythons is associated with vast increases in metabolic rate, but showed that such increases are merely owing to the act of processing a meal rather than to the organ growth itself. Starck & Rahmaan (2003) found that (resting) metabolic rates in a bird species, quail, remain unaltered during size changes in gizzard and small intestine. Although energetic costs involved in size changes in both gizzard and intestine may thus be minute, Starck & Rahmaan additionally showed that the cellular processes underlying such changes differ substantially between both organs. Gizzards grow because of cell growth while intestines grow because of cell proliferation.

Due to the fact that fuelling gizzards are relatively small (8 g), the 25% downscaling to 6 g only marginally improves overall speed of migration through small reductions in P_flight (by only a single day over the full distance of 15 000 km, so the overall speed of migration=167 km day⁻¹: dashed line in figure 5b,c). The far greater effect of prey quality remains.

Prey is of best quality (high amounts of flesh) in the reproductive phase just before the release of gametes (Gabbot 1983; Zwarts 1991; Honkoop & Van der Meer 1997). In temperate zones, reproduction of marine invertebrates is seasonally synchronized and takes place in spring (see references in Zwarts 1991), which is reflected in the peak in prey quality during spring (Van Gils et al. 2003). Keeping in mind that the boreal spring and thus prey reproduction starts later in the year with increasing latitude (Piersma et al. 1994), spring-migrating knots seem to follow a northwards ‘wave’ in prey quality. We therefore suggest that knots locate and time their stopovers to coincide with local peaks in prey reproductive periods. This idea has been suggested earlier with respect to increased availability of benthic prey (Piersma et al. 1994) and for Arctic-breeding waterfowl tuning their migration to plant growth (‘the green wave hypothesis’; Drent et al. 1978).

In tropical regions, prey reproduction is much less synchronized and occurs throughout the year so that a distinct seasonal peak in prey quality may be absent (De Goeij et al. 2003). Therefore, rapid fuelling may be impossible around the equator. This might explain why tropical regions are probably skipped as stopovers by knots wintering in the Southern Hemisphere (rufa and rogersi). Tropically wintering knots (canutus and piersmai) may find fuelling and leaving these sites difficult, a suggestion that has been made before (Ens et al. 1990; Piersma et al. 2005). Indeed, rates of fuelling are lower (N=14 sites, R²=0.29, p<0.05) and length of fuelling periods are longer (N=14 sites, R²=0.61, p=0.001) in tropical than in temperate regions (data from Piersma et al. 2005). West-African wintering canutus may face the severest problems. If their prey in spring had the same low quality observed in winter (0.89 kJ g⁻¹ DM_shell), then the maximum fuelling rate would require a gizzard of approximately 17 g (figure 3a; table 1). In addition to higher maintenance and transport costs associated with such huge gizzards, ‘space’ for fat deposition might be limited in such muscular bodies. Indeed, knots departing from Banc d'Arguin are relatively light (average 168 g, p<0.05 when comparing this with 13 other average departure masses given by Piersma et al. (2005)). Possibly, canutus manages with gizzards smaller than 17 g by extending the low tide period beyond the ‘usual’ 12 h day⁻¹ (Zwarts et al. (1990); this has been observed for islandica in the Wadden Sea (Van Gils et al. 2005b) and Iceland (Alerstam et al. 1992)).

Fuelling in the Southern Hemisphere takes place during the austral autumn, a time of year long past the peak in prey quality in the (austral) spring (Wilson & Hodgkin 1967; Wilson 1969). Our ‘prey–reproduction’ hypothesis would therefore predict that among sites in temperate regions, fuelling and consequently speed of northward migration should proceed faster in the Northern Hemisphere than in the Southern Hemisphere. Using data published by Piersma et al. (2005), this prediction is upheld, both with respect to fuelling rates (N=11 sites, R²=0.39, p<0.05) and with respect to lengths of fuelling periods (N=11 sites, R²=0.36, p=0.05).

The approach used here, predicting optimal gizzard sizes from a prey's flesh-to-ballast ratio, is only applicable to knots that feed on hard-shelled mollusc prey. Applying the model to rufa feeding on the super-high quality eggs of horseshoe crabs (Limulus polyphemus) at its stopover in Delaware Bay (North America), predicted net-rate maximizing gizzards of about 1 g. The fact that observed gizzards were much larger than that (mean±s.e.=7.0±0.2 g, N=61), hints that the grinding of horseshoe crab eggs is a fundamentally different process when compared to crushing the outer shells of molluscs. Only Delaware Bay red knots have small stones in their gizzards, presumably to grind the leathery surface of the eggs (T.P. unpubl. data; see Piersma et al. 1993b). Direct experimentation is called for to clarify this.

5. Conclusion

Variation in gizzard mass of wintering red knots is large (from 6 g in piersmai to almost 10 g in canutus and rogersi). The variation appears to reflect the best possible size adjustments to global variations in prey quality and climate in order to balance energy budgets on a daily basis. Variation in gizzard mass is, by contrast, small in migrating knots (around 8 g in all populations). By the selection of stopover sites with high-quality prey, red knots maximize fuelling rates and overall speed of migration using relatively small gizzards. This idea generates testable predictions on the prey quality at as yet unexplored stopover sites, such as those of rogersi and piersmai along the East Asian–Australasian Flyway. Given the extremely late departure from their northwest Australian wintering site (Battley et al. 2005), piersmai is likely to encounter ‘super-food’ at its stopover in the Yellow Sea, enabling gizzards to be kept small and migration sped up.