Evolution in group-structured populations can resolve the tragedy of the commons

Abstract

Public goods are the key features of all human societies and are also important in many animal societies. Collaborative hunting and collective defence are but two examples of public goods that have played a crucial role in the development of human societies and still play an important role in many animal societies. Public goods allow societies composed largely of cooperators to outperform societies composed mainly of non-cooperators. However, public goods also provide an incentive for individuals to be selfish by benefiting from the public good without contributing to it. This is the essential paradox of cooperation—known variously as the Tragedy of the Commons, Multi-person Prisoner's Dilemma or Social Dilemma. Here, we show that a new model for evolution in group-structured populations provides a simple and effective mechanism for the emergence and maintenance of cooperation in such a social dilemma. This model does not depend on kin selection, direct or indirect reciprocity, punishment, optional participation or trait-group selection. Since this mechanism depends only on population dynamics and requires no cognitive abilities on the part of the agents concerned, it potentially applies to organisms at all levels of complexity.

1. Introduction

Achieving a satisfactory understanding of the evolution of cooperation in social dilemmas is fundamental for elucidating many important problems in biology and the social sciences, such as the stability of human and animal societies and the sustainability of public resources (Hamilton 1964; Hardin 1968; Wilson 1975b; Axelrod & Hamilton 1981; Alexander 1987; Berkes et al. 1989; Frank 1998; Richerson & Boyd 1998; Ostrom et al. 1999). Social dilemmas also emerge in many other key problems in biology, including the major transitions in evolution (Maynard Smith & Szathmary 1995), the evolution of pre-biotic replicators (Eigen & Schuster 1979), viral evolution (Turner & Chao 1999) and the evolution of metabolic pathways (Pfeiffer et al. 2001).

Many theoretical and experimental investigations of cooperative behaviour have employed public goods games as simple models of social dilemmas (Ledyard 1995; Gintis 2000; Fehr & Gachter 2002; Hauert et al. 2002; Milinski et al. 2002; Semmann et al. 2003). Public goods games capture the essential features of social dilemmas—groups of cooperators outperform groups of non-cooperators, but selfish individuals always do better than cooperators in their group (Gintis 2000). In a typical public goods game (Ledyard 1995), an experimenter gives each of the four subjects an endowment of £10. Each player is offered the opportunity to invest some or all of their £10 in a common pool. The experimenter then collects the money in the pool, doubles it and divides it equally among the four individuals. Game theory predicts that, since each £1 invested yields a return of £0.50 to the investor, no one should ever contribute to the common pool. This situation is a social dilemma—if no one contributes to the common pool then each individual keeps their initial endowment of £10, however, if all individuals contribute their £10 endowment to the pool then they each end up with £20. In public goods experiments, the initial high level of investment drops quickly to low levels (Ledyard 1995). High levels of investment can be maintained by allowing individuals to punish non-cooperators (Fehr & Gachter 2002), by the need for individuals to maintain a good reputation (Milinski et al. 2002) or by including optional participation in the game (Hauert et al. 2002; Semmann et al. 2003).

Here, we focus on a simple alternative mechanism in which evolutionary dynamics in a group-structured population results in the evolution of high levels of cooperative investment, which are maintained indefinitely. This mechanism is fundamentally distinct from previous approaches for studying the evolution of cooperation in group-structured populations which have depended on kin selection (Maynard Smith 1964; Wade 1985; Queller 1992; Taylor 1992a,b; Wilson et al. 1992; West et al. 2002; Rousset 2004), classical group selection (Maynard Smith 1964; Wilson 1975a), trait-group selection (Wilson 1975a, 1980) or spatial game theory (Nowak & May 1992; Killingback et al. 1999). In addition, our model does not depend on the agents having any capacity to recognize other agents or remember past actions, which is essential for mechanisms based on reputation (Milinski et al. 2002) or punishing (Fehr & Gachter 2002); or on individuals being able to opt out of the public goods game, which is crucial for mechanisms based on volunteering (Hauert et al. 2002; Semmann et al. 2003).

2. Model and results

We use the following public goods game to study the evolution of cooperation in social dilemmas. We assume that n individuals each make an investment x_i in a public good, where each x_i (i=1, …, n) is a real number between 0 and some positive maximum value V. The payoff to individual i is given by $E(x_i;\overline{x})=(k/n){\sum }_{j=1}^n{x}_j-x_i$, where k is a positive constant (which can be viewed as the ‘interest rate’), and $\overline{x}=(x_1,\dots ,x_n)$ denotes the strategy profile of the individuals in the group. The first term in this expression represents the benefit that the individual gets from the public good, while the second term represents the cost of making the investment. This definition extends the notion of a public goods game that is used in experimental situations to a general game with continuous investments. In our formulation of the public goods game, we have assumed that the interest rate k is a constant, independent of the group size n. This is a standard assumption in work on the public goods game: however, we note that, in principle, we could also consider a variant of our model in which k depends on n. We also note that in our formulation of the game the strategy is an arbitrary real number between 0 and V. This definition extends the standard discrete strategy public goods game. In principle, it is also possible to consider more complex strategies that depend explicitly on the group size n, but we will not do this here.

Since the payoff can be written as $E(x_i;\overline{x})=(k/n)\sum _{1\le j\ne i\le n}{x}_j-(1-(k/n))x_i$, it is clear that, for $1<k<n$, every individual will maximize its payoff by making zero investment, irrespective of the investments made by the other individuals (i.e. for 1<k<n defection is the dominant strategy). However, if all the players make zero investment, they each receive a payoff E₀=0, while if every player instead invested V they would each receive the larger payoff E_V=(k−1)V. This is a social dilemma: groups of cooperators outperform groups of non-cooperators, but it is always individually advantageous to cheat by not cooperating.

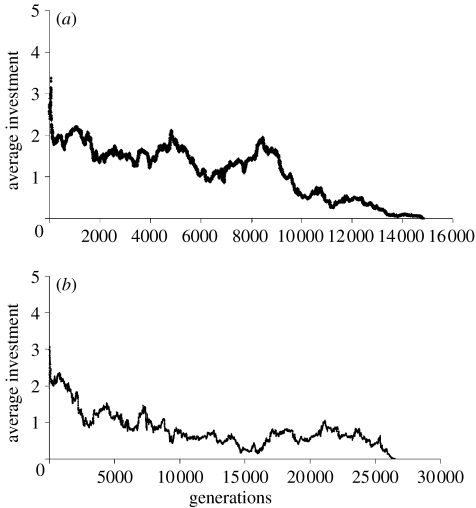
For 1<k<n, the public goods game is a social dilemma and zero investment is the individually optimal strategy. More generally, if the public goods game is considered as an evolutionary game (Maynard Smith 1982), then selection will always result in individuals making zero investment, even for k>n. This follows from the fact that, in any group, for any k, low investors obtain a greater payoff than higher investors. In the evolutionary context, we consider a population of N individuals, each making an investment x_i. We assume that all individuals in the population participate in the public goods game. The fitness of individual i is taken to be $W(x_i)=V+E(x_i;\overline{x})$, which is always positive. We assume that individuals reproduce in proportion to their fitness, subject to the condition that the total population size remains constant. During reproduction mutations can occur, which change the investment level of the offspring. If we denote the lowest investing strategy in the population by x_i then, since $W(x_i)>W(x_j)$, for all j≠i, strategy x_i will go to fixation. Consequently, the average level of investment in the population will evolve to zero as selection consistently favours lower investing mutants. Evolutionary simulations confirm this result (see figure 1).

Figure 1.

Simulation results for the evolution of cooperation in the public goods game in a single well-mixed population of 100 individuals, with a maximum investment level of V=5. (a) k=90; (b) k=110. All individuals in the population participate in the public goods game. The fitness of each individual is calculated as described in the text. Individuals reproduce in proportion to their fitness, subject to the condition that the total population size remains constant. During reproduction mutations can occur, which change the investment level of the offspring. In both cases cooperative investments evolve to 0. Initial investments were chosen uniformly randomly between 0 and 5. The mutation rate was 0.01, and mutations were randomly picked from a normal distribution with mean 0 and variance 0.01.

Since cooperation cannot evolve in the public goods game in a well-mixed population, it is important to consider the effect of other population structures. In many social situations, individuals do not interact with all members of the population in every generation—rather, in a given generation, individuals only interact socially with a sub-group of the population. Consider now the total population to be composed of m disjoint interaction sub-groups. We assume that each individual in the population obtains a payoff by playing the public goods game with the other individuals in its interaction group. We also assume that individuals compete with all other individuals in the population. Thus, social interactions are local, while competition is global. We implement the assumption of global competition by having individuals reproduce in their group in proportion to their fitness, subject to the condition that the total population size remains constant. To achieve this constraint on the total population size we allow individuals to reproduce in their group (in proportion to their fitness) and then rescale the size of all groups to maintain a constant total population size. During reproduction occasional mutations occur, which change the investment level of the offspring. Finally, a fraction d of the individuals in each group disperses randomly to the other groups in the population. We assume that initially all groups are of equal size, containing n>k individuals, so the public goods game in each group is a social dilemma. We also assume that, if any group consists of only a single individual, then this individual does not play the public goods game, and receives zero payoff.

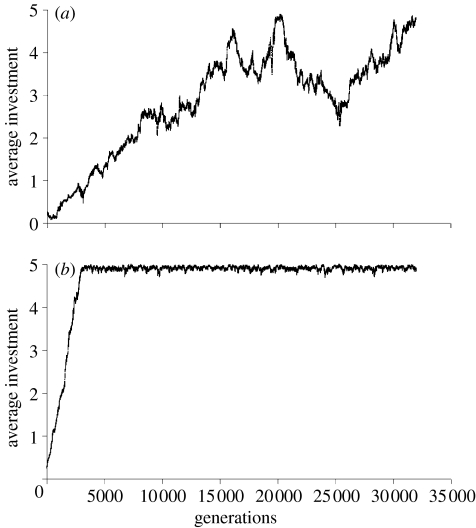
Despite its simple definition, it is not easy to study this group-structured model analytically. Thus, our investigation is based on extensive evolutionary simulations (source code available in the electronic supplementary material). Our simulations show that the evolution of cooperation in such a group-structured population can be dramatically different from that in a well-mixed population and that with such a population structure substantial cooperative investments can readily evolve from low initial levels and be maintained indefinitely. Typical simulation results are shown in figure 2. The following mechanism is responsible for the evolution of cooperation in the group-structured situation. The combination of reproduction within groups and limited random dispersal among groups results in groups of varying size (although the mean group size remains constant at n). For certain parameter values the variation is such that groups with fewer than k individuals form. In such groups, the public goods game is no longer a social dilemma, in that zero investment is no longer the dominant strategy. Although lower investors always have greater fitness than higher investors, in any given group, it is now possible that Simpson's paradox (Sober & Wilson 1999; Hauert et al. 2002) applies—the fitness of higher investors, when averaged over all groups, will be greater than that of lower investors—and higher investors will increase in frequency. Thus, interaction and reproduction within groups, together with limited dispersal among groups, results in a natural mechanism for the evolution of cooperation. The exact range of dispersal values for which cooperation is maintained depends on the parameters in the model. We find that there exists a significant region of dispersal values for which cooperation evolves for a wide variety of parameter choices (see figure 3).

Figure 2.

Simulation results for the evolution of cooperation in a population of 500 individuals, subdivided into 100 interaction groups. (a) k=2; (b) k=3. Each individual in the population obtains a payoff by playing the public goods game with the other individuals in its interaction group, as described in the text. Individuals reproduce in their group in proportion to their fitness, subject to the condition that the total population size remains constant. During reproduction occasional mutations can occur, which change the investment level of the offspring. Finally, a fraction d of the individuals in each group disperses randomly to the other groups in the population (this is achieved by giving each individual a probability d of dispersing to another randomly chosen group). Initially all groups are of equal size, containing five individuals, so the public goods game in each group is a social dilemma. Starting from low initial values, cooperative investments evolve to high values, which are maintained indefinitely. The dispersal rate was set to d=0.1, and initial investments were chosen uniformly randomly between 0 and 0.5. All other parameters as in figure 1.

Figure 3.

Asymptotic level of cooperative investment after 100 000 generations as a function of dispersal rate d, for a population of 500 individuals, subdivided into 100 interaction groups. (a) k=2; (b) k=3. In both cases, there exists a region of dispersal values for which high levels of cooperative investment evolve, and are maintained indefinitely. We note that cooperation breaks down both at high d and at very low (but non-zero) values of d. Results shown are from 10 replicate simulations; initial investments were chosen uniformly randomly between 0 and 0.5. All other parameters as in figure 1.

3. Discussion

The model that we have proposed here for evolution in group-structured populations provides a simple new mechanism for the origin and maintenance of cooperation in public goods situations, which differs in important ways from others that have been proposed. The main established approaches to study the evolution of cooperation in group-structured populations are kin selection in subdivided populations (Maynard Smith 1964; Wade 1985; Queller 1992; Taylor 1992a,b; Wilson et al. 1992; West et al. 2002; Rousset 2004), classical group selection (Maynard Smith 1964; Nunney 1985; Wilson 1987, 1990), trait-group selection (Wilson 1975a, 1979, 1980) and spatial game theory (Nowak & May 1992; Killingback et al. 1999). We will briefly discuss the differences between our model and these approaches.

First, it is clear that, in general, our model is quite different from trait-group models, in which trait-groups of equal size are completely reformed in each generation (Wilson 1975a, 1980). Moreover, it is important to note that trait-group selection does not result in the evolution of cooperation in public goods situations if the groups are formed strictly randomly (Wilson 1975a, 1980). In order for cooperation to evolve in trait-group models it must be assumed that, although the groups are formed anew in each generation, there is some essential source of non-randomness in their composition (Wilson 1975a, 1980). We recover this result in the d=1 limit of our model (which can be regarded as being similar to a trait-group model with randomly formed groups) where cooperation does not evolve (see figure 3).

It is also clear that our model is very different from classical group selection models (Maynard Smith 1964; Nunney 1985; Wilson 1987, 1990), as selection acts purely at the individual level in our model.

Another class of group-structured models that has been considered involves kin selection in a subdivided population (Wade 1982; Queller 1992; Taylor 1992a,b; Wilson et al. 1992; West et al. 2002; Rousset 2004). These models typically assume that the total population is subdivided into local populations, which are distributed over some spatial lattice, with limited dispersal taking place between the different local populations. Whether such an approach allows cooperation to evolve depends critically on the detailed assumptions of the model. In many cases, the negative effect of kin competition exactly cancels out the positive effect of kin selection, hence preventing the evolution of cooperation (Queller 1992; Taylor 1992a,b; Wilson et al. 1992). It is, however, also possible to formulate kin selection models in subdivided populations that do allow the evolution of cooperation (for a good discussion of the factors that facilitate the evolution of cooperation in such models, see West et al. 2002).

Although it is possible for kin selection to facilitate the evolution of cooperation, in situations such as the one we are considering, kin selection alone cannot support cooperation in our model. The mechanism at work in our model is quite different: namely, reproduction in groups, combined with dispersal between groups, results in variations in group size, and for groups of sufficiently small size, the public goods game is no longer a social dilemma. Thus, it is possible, by Simpson's paradox, for high investors, when averaged over all groups, to have greater fitness than low investors. This mechanism is clearly quite distinct from kin selection. This distinction can be explicitly tested by constructing a variant of our model in which the public goods game is replaced by a game such as a continuous version of the Multi-person Prisoner's Dilemma, which is a social dilemma for groups of any size. In such a variant of our model, cooperation never evolves (T. Killingback, J. Bieri & T. Flatt, unpublished work). This is consistent with the fact that the mechanism involving Simpson's paradox cannot operate in the variant model, even though kin selection should favour the evolution of cooperation equally in both cases.

It is also apparent that the mechanism responsible for the evolution of cooperation in our model is quite distinct from that which occurs in spatial game theory models (Nowak & May 1992; Killingback et al. 1999; Hauert & Szabó 2003). Spatial game theory models of the evolution of cooperation depend critically on the assumption that each individual interacts only with its neighbours on a spatial lattice (Nowak & May 1992; Killingback et al. 1999; Hauert & Szabó 2003). In contrast to these models, our group-structured model does not involve any notions of spatial structure—such as spatial neighbours or spatial dimension—all that is required is that the population consists of coherent groups (which can result from various mechanisms, such as social organization).

Finally, we note that direct (Trivers 1971) or indirect (Nowak & Sigmund 1998; Milinski et al. 2002) reciprocity, or punishment (Fehr & Gachter 2002), are not involved in the maintenance of cooperation in our model, since we have not assumed that the individuals playing the game have any capacity for individual recognition or memory. Since we do not assume that individuals can opt out of the public goods game, it is also evident that volunteering (Hauert et al. 2002; Semmann et al. 2003) is not the mechanism at work here (although Simpson's paradox also plays a key role in the maintenance of cooperation through volunteering).

The model we have proposed for evolution in group-structured populations provides a simple and effective mechanism for promoting the evolution of cooperation in social dilemmas such as the Tragedy of the Commons. Biological populations are often structured in such a way that individuals interact socially with other individuals in a sub-group of the whole population, with dispersal occurring between different sub-groups (Wilson 1975b): consequently, the mechanism described here naturally applies to a wide variety of human and animal societies. One potentially interesting class of examples where this mechanism may apply involves the common good achieved by individuals maintaining their habitat in a sanitary state and thereby reducing the prevalence of infectious diseases. In a population subdivided into many groups, each occupying a habitat, the payoff to individuals investing in maintaining sanitary conditions may be higher to those in small groups than to those in larger ones, since many infectious diseases are less likely to be maintained in a small population. Assuming that there is a modest degree of dispersal between individuals in different groups, then our mechanism may apply to such a situation, and would suggest that investment in maintaining sanitary conditions could evolve and be maintained throughout the population.

Our mechanism may also work in conjunction with other mechanisms, such as kin selection, to promote the evolution of cooperation in group-structured populations. In this regard, it is interesting to note that both our mechanism and kin selection facilitate the evolution of cooperation in a similar regime of dispersal values—namely for reasonable low levels of dispersal. Moreover, since our mechanism is based on population dynamics, and does not require any cognitive abilities on the part of the agents involved, it potentially applies to cooperative behaviour at any level of complexity, from humans to micro-organisms.

Supplementary Material

Java source code for simulating the evolutionary public goods game

Annotated version of Java source code, used for evolutionary simulations of the public goods game (PGG).