Skip to main content
PMC home page
Proceedings of the Royal Society B: Biological Sciences logoLink to Proceedings of the Royal Society B: Biological Sciences
. 2005 Jun 21;272(1570):1351–1356. doi: 10.1098/rspb.2005.3081

Changes in maternal investment in eggs can affect population dynamics

TG Benton 1,2,*, SJ Plaistow 1,2, AP Beckerman 1, CT Lapsley 1, S Littlejohns 1
PMCID: PMC1560333  PMID: 16006330

Abstract

The way that mothers provision their offspring can have important consequences for their offspring's performance throughout life. Models suggest that maternally induced variation in life histories may have large population dynamical effects, even perhaps driving cycles such as those seen in forest Lepidoptera. The evidence for large maternal influences on population dynamics is unconvincing, principally because of the difficulty of conducting experiments at both the individual and population level. In the soil mite, Sancassania berlesei, we show that there is a trade-off between a female's fecundity and the per-egg provisioning of protein. The mother's position on this trade-off depends on her current food availability and her age. Populations initiated with 250 eggs of different mean sizes showed significant differences in the population dynamics, converging only after three generations. Differences in the growth, maturation and fecundity of the initial cohort caused differences in the competitive environment for the next generation, which, in turn, created differences in their growth and reproduction. Maternal effects in one generation can therefore lead to population dynamical consequences over many generations. Where animals live in environments that are temporally variable, we conjecture that maternal effects could result in long-term dynamical effects.

Keywords: maternal effects, trade-off, reproductive allocation, population cycles, delayed density dependence, competition

1. Introduction

Differential provisioning of offspring is a widespread phenomenon that has important consequences for offspring fitness (Rossiter 1996; Fox & Savalli 1998; Mousseau & Fox 1998; Einum & Fleming 2000; McIntyre & Gooding 2000). The transmission of maternal quality to offspring probably also has population dynamical consequences, because it leads to a time lag between the environment and the population response. In the presence of environmental fluctuations, the lag created by the delayed life-history effect typically also increases population variability and decreases its predictability (Benton et al. 2001b; Beckerman et al. 2002). In addition, maternal effects can theoretically lead to long-term deterministic population dynamical patterns such as population cycling seen in forest Lepidoptera and many microtine rodents (Ginzburg & Taneyhill 1994; Ginzburg 1998; Inchausti & Ginzburg 1998). Understanding the causes of fluctuations in population size and the interplay between the environment and density dependence is a key goal of current population ecology (Saether 1997; Bjørnstad & Grenfell 2001; Coulson et al. 2001; Clutton-Brock & Coulson 2002; Greenman & Benton 2003) and is crucial for predictive population modelling for management. Understanding the role of maternal effects in creating variation in a population's dynamics is therefore of widespread and general interest.

The influence of maternal effects on population dynamics is difficult to test empirically, as it involves manipulating population processes. Inference has typically been made from information contained in time-series, such as the period and shape (e.g. time reversibility) of population cycles (Ginzburg & Taneyhill 1994; Ginzburg 1998; Inchausti & Ginzburg 1998; Turchin & Hanski 2001; Shaw et al. 2004), or the relationship between population perturbations or size and the life history of cohorts of individuals (Albon et al. 1987; Myers et al. 1998; Erelli & Elkinton 2000; Forchhammer et al. 2001; Beckerman et al. 2002, 2003; Reid et al. 2003). A number of experiments have been conducted to investigate the strength of maternal influence on individual performance in a range of animals (e.g. Erelli & Elkinton 2000; Ergon et al. 2001; Banks & Powell 2004), which have generally found maternal effects to be either absent or weak. The conclusions from these studies are that although maternal effects could drive important features of population dynamics, the maternal effects observed probably have a minor influence on population dynamics.

Previous studies are typically limited because they are correlational in nature, or, if experimental, they have been able to study sufficiently realistic population biology owing to the inherent difficulties of conducting population-level experiments. We use an invertebrate model system to describe simultaneously patterns of reproductive investment and conduct replicated, population-level experiments to show directly, for the first time in any organism, the impact of maternal effects on the population dynamics.

2. Methods

(a) Study organism

Sancassania berlesei mites were collected from agricultural muck heaps in 1998 and kept in cultures in excess of 1×105 individuals. General laboratory methods can be found in (Benton et al. 2001a; Beckerman et al. 2003).

(b) Reproductive allocation experiment

In October 2001, animals were taken from stock culture (high density, low per capita food) and placed into a common garden with greater per capita food than stock. After two weeks, eggs were collected and assigned in batches of 30 to 12 culture tubes. These were randomly assigned to four treatment groups, differing in the per capita food supply (1, 5, 10 or 20 rods of powdered ‘instant’ yeast per day, each approximately 0.13 mg±s.d. 0.03). Upon maturation, adults were allocated in groups of three pairs, to four treatment groups, each with three replicates. The treatment groups differed in per capita food availability from 1 to 4 rods of food per female per day. From maturation, the animals were transferred to a new culture tube daily, and the eggs counted. The eggs from days 2–4, 9–11 and 15–19 of the adult's lives were collected for subsequent assay of protein content.

(c) Protein assay

Eggs were collected in Eppendorf tubes and stored at −20 °C. The tubes were kept on ice during extraction. The eggs were initially washed in 750 μl distilled water and then washed three times by repeated spinning (maximum speed in Heraeus microfuge), removal of supernatant and re-suspension in 500 μl distilled water. The protein was extracted by repeated (five times) homogenization in 0.1% Triton-X-100 and spinning (>0.25 μl per egg). Extracts were boiled for 2 min and spun for 3 min. The supernatant was used as per the method of Sedmak & Grossberg (1977). We added 250 μl of G250 dye to 12.5 μl of protein solution placed in a microtitre plate. The tray was then gently shaken and the absorbance at 620 nm was read using a Versa Max microplate reader with SoftMaxPro software.

(d) Population experiment

In August 2003, eggs were collected from parental cultures that had been fed on ‘high food’ or ‘low food’. To reduce the impact of unwanted maternal effects (which can last for at least three generations; S. J. Plaistow et al., unpublished work), the high-food cultures (n=3) were fed yeast in excess for 60 days (equivalent to about four to six generations).

The low-food cultures were stock cultures (n=2) maintained at population dynamical equilibrium (between 105 and 106 mites). Juveniles were isolated from stock and allowed to mature into adults. Following maturation, approximately 300 adult females (high-food stocks) and 2400 females (low-food stocks) were placed in six culture tubes (high food) or 12 (low food). These females were transferred to clean tubes each day, ensuring that eggs collected all came from the same day of laying. Eggs were assigned to n=5 culture tubes at random, until there were 250 eggs per tube. One culture tube (in the low food=large egg treatment) was discarded as the tube consistently dehydrated, leaving four replicates.

Each day, the numbers of eggs, juveniles and adults were counted under a Leica MZ8 binocular microscope, using a handheld tally counter, a sampling grid scratched on to the plaster base of the tube. All adults were counted; juveniles and eggs were initially counted throughout the tube, but after numbers exceeded 1000, the juveniles and eggs were counted from a randomly selected quarter of the tube. Every day, each tube received two drops of water and a single granule of active dried yeast, sieved for conformance (a sample of which was weighed at 1.08 mg±s.e. 0.03, n=100).

(e) Statistical analysis

Statistical analysis was conducted using Splus (www.insightful.com). Analysis of the assay experiment involved a mixed-effects model fitted using restricted maximum likelihood (REML). REML accounts for the repeated measures of eggs within the lifetime of each culture tube and also that tube is a random factor.

Owing to temporal changes in the autocovariance structure of the time-series, fitting standard autoregressive models to the data is not appropriate (e.g. the generation time is density dependent, so there is no fixed time-delay in the dynamics). Instead, significant divergence between time-series was assessed by estimating the bootstrap confidence intervals for the five replicate cultures at each time point. In cases where the confidence intervals around the means did not overlap, we assumed that the time-series are different.

3. Results

(a) Reproductive allocation

In the soil mite, S. berlesei, there are strong maternal influences on individual performance. For example, juveniles experiencing a low-food environment mature after 14.3±0.3 days if their mothers also came from low-food environments, but after 23.0±0.7 days if their mothers came from high-food environments (Plaistow et al. 2004). To test whether these maternal effects were caused by changes in females’ egg provisioning strategies, we reared groups of mites on different per capita food supplies. During adulthood, we tallied the fecundity (eggs per female per day), and at three stages in the life cycle (young, middle-aged and old) we sampled the eggs and assayed their crude protein.

There is a positive linear relationship between median egg size (measured using an eyepiece graticule) and protein content (F1,34=12.3, p=0.001, R2=24%), so eggs are larger as a result of greater provisioning by mothers. There is a strong negative relationship between the protein allocation per egg and the mother's daily fecundity (figure 1a). The position of females on this trade-off is influenced by their age and their food supply (figure 1b,c; table 1). Well-fed females typically lay more but less well-provisioned eggs than poorly fed females. In addition, well-fed females, towards the end of their life, switch to laying fewer, better provisioned, eggs (figure 1b).

Figure 1.

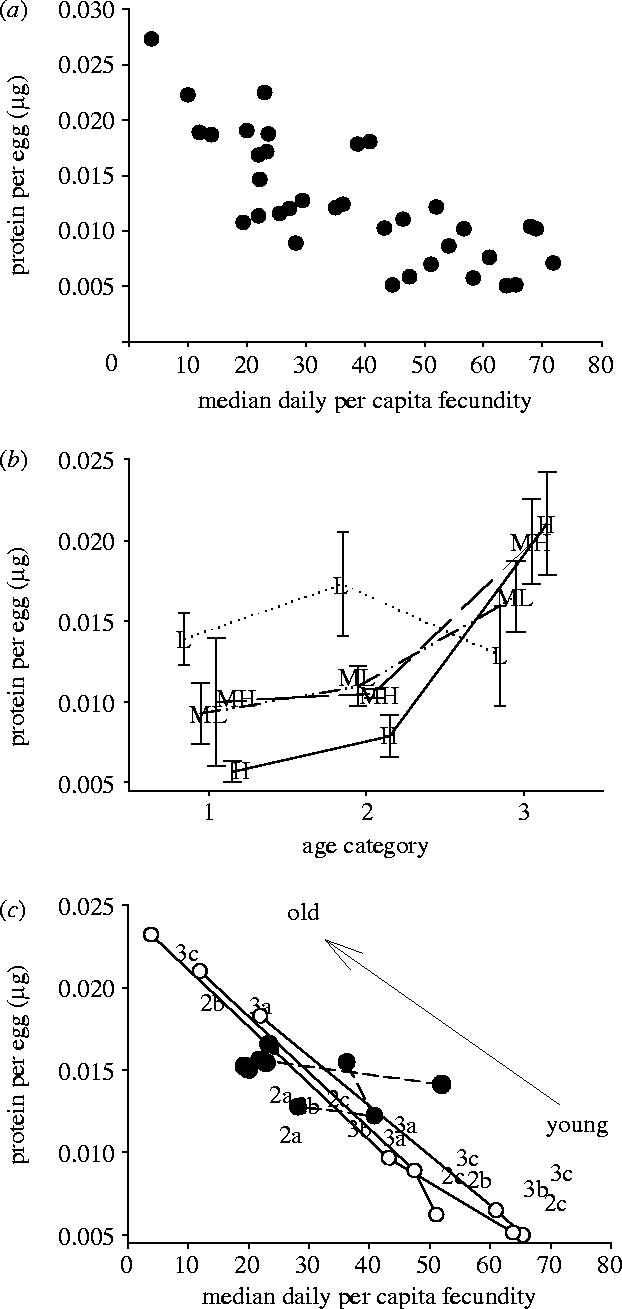
Figure 1

Open in a new tab

The trade-off between per capita protein investment in eggs and fecundity. (a) Data exhibiting the general negative relationship. A simple linear fit to these data gives a significant negative slope of −0.000 226±0.000 033. (b) The influence of food and age on per capita protein investment, points are means±s.e. of the three replicates at each age. Age category is 1=young, 2=middle-aged, 3=old (jitter has been added to aid clarity). Food levels are L=low, ML=medium low, MH=medium high and H=high. (c) Fitted values from the restricted maximum likelihood model (table 1) split by age and food supply. Each culture tube has three points associated with the three ages. Well-fed mothers (white circles) lay many small eggs until old age, when they lay fewer larger eggs. Poorly fed mothers (black circles) show little terminal investment and lay fewer, larger, eggs throughout their life. Medium low-food tubes are labelled as 2a–c, medium–high as 3a–c to aid clarity.

Table 1.

Statistical analysis of the reproductive allocation data.

factor d.f. F p
food 3, 8 1.16 0.38
fecundity 1, 17 29.04 <0.0001
age 1, 17 35.78 <0.0001
fecundity*age 1, 17 4.26 0.0548
food*age 3, 17 3.33 0.0443
Open in a new tab

A linear mixed effects model was fitted to the data to analyse the influence of fecundity, age and food levels on the average protein per egg. The fixed effects were fecundity, age and food, with tube as random effect nested within food. The model including the fecundity*age interaction is a significantly better model (likelihood ratio=4.33, p=0.037) than if it were excluded.

(b) Differences in egg sizes lead to differences in population dynamics

Having established that mothers’ provisioning of eggs varies with variation in maternal resources, we conducted an experiment to evaluate the population dynamical consequences of variation in provisioning. We created variation in egg sizes by collecting eggs from high density, low per capita food cultures, or low density, high per capita food cultures, and confirmed, by measurement, that these eggs were significantly different sizes as expected (low-food culture: 0.198±0.011 mm, high-food culture: 0.183±0.013 mm, t32=3.6, p=0.0011).

There are marked differences in the population trajectories caused by initial differences in the egg sizes, which last far beyond the original generation (figure 2). The largest difference between treatments occurs as the offspring of the original cohort die and the grand-offspring recruit to adulthood (days 50–70; figure 2c). At the end of the experiment, the dynamics approach equilibrium, but the differences in stage structure throughout the experiment mean that the stable stage distribution is approached by markedly different trajectories in phase space (figure 3). At day 92, after about three generations, the populations are close to equilibrium age and stage structure; at this time there were no significant differences in average egg, or adult, sizes between treatments (mixed effects models, tube nested within treatment: eggs F1,8=1.51, p=0.25, males: F1,8=0.95, p=0.39, females: F1,8=0.03, p=0.88, n=5 measurements per tube).

Figure 2.

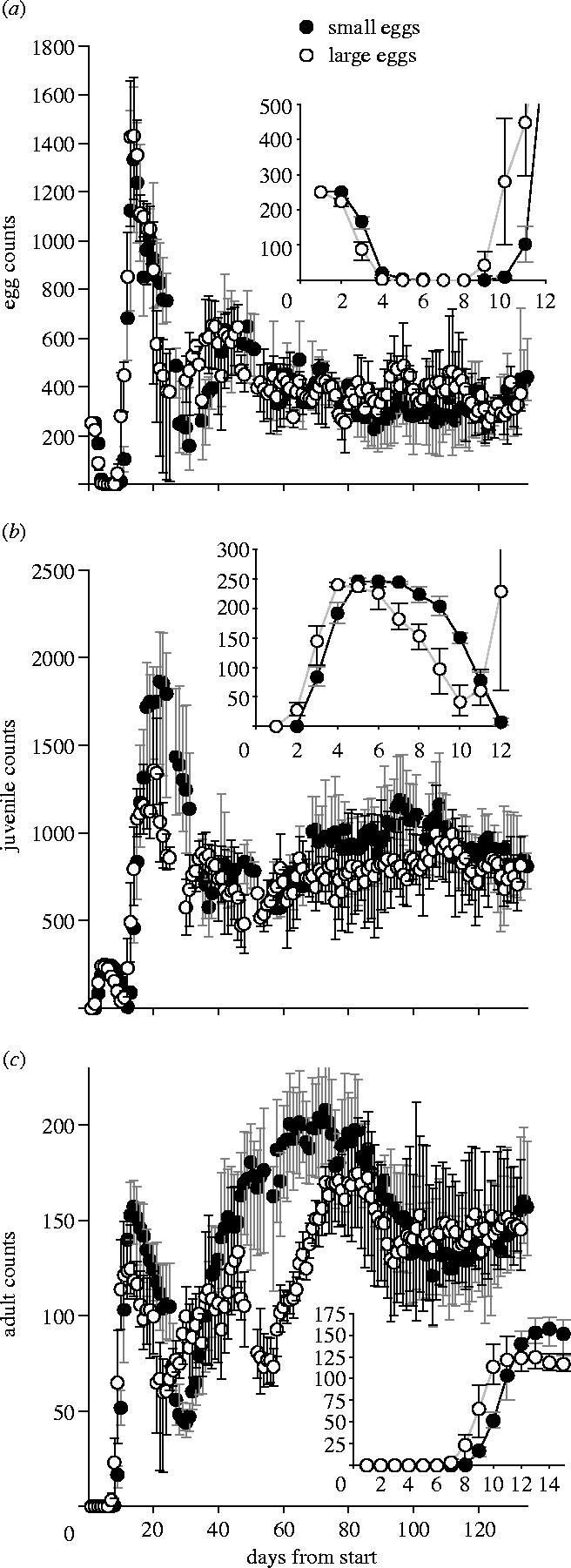
Figure 2

Open in a new tab

Population dynamics’ time-series. The data are the means (with 95% bootstrapped CI) of cultures started from 250 eggs that came from high-food females who laid smaller eggs (filled symbols, n=5 cultures) or low-food females who laid larger eggs (open symbols, n=4 cultures). For each population stage ((a) eggs, (b) juveniles, (c) adults), the inset graph shows the development of the initial F1 cohort.

Figure 3.

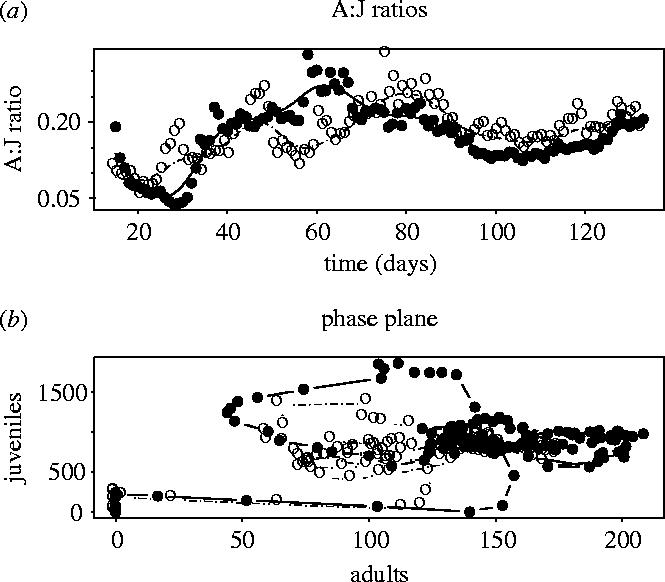
Figure 3

Open in a new tab

Graphical descriptions of the difference in the population time-series shown in figure 2. (a) The average time-series of the adult : juvenile (A:J) ratio, with lines fitted as a 12 d.f. spline, showing how stage structure changes during the experiment. (b) Phase portrait in the adult : juvenile phase plane. The stable age distribution is approached in different ways by the different treatment groups. Each point is the treatment average at each time.

(c) Disentangling the mechanisms giving rise to the dynamics

The biological mechanisms by which the initial differences in egg size are propagated into population trajectories are complex. Large eggs from poorly fed mothers hatched significantly earlier than smaller eggs from well-fed mothers (low food: first egg hatched day 2, median egg hatched day 3; high food: first hatch day 3, median day 4), suggesting that well-provisioned eggs undergo faster development (figure 2a; Einum 2003). As a result, the juveniles grew faster and matured to adulthood earlier (median maturation day for low-food eggs was day 9 cf. day 11; figure 2b,c), but had lower survival (large eggs: peak number of F1 adults 133.4, 95% CI 112.6–155.6, small eggs: peak F1 adults 157.2, 95% CI 133–171). The lower density of F1 adults in the large-egg cultures lead to higher per capita fecundity, resulting in similar total numbers of F2 eggs laid (peak eggs 1333, 95% CI 1034–1581 for small-egg cultures, 1370, 95% CI 1150–1565 for large-egg cultures). Although there were similar numbers of eggs laid, in the large-egg cultures there was a much lower peak juvenile density (mean 1063, 95% CI 836–1337, versus mean 1863, 95% CI 1392–2146 on day 21, figure 2b), suggesting a marked difference in either hatching rate or early juvenile survival. Thus, large eggs hatched first and led to earlier-recruiting, but fewer adults, which had higher fecundity, but whose eggs had a lower hatching success (which is consistent with larger F1 mothers laying smaller eggs that pay a survival penalty in a highly competitive environment; Einum & Fleming 1999; McIntyre & Gooding 2000; Shertzer & Ellner 2002).

The existence of high-density versus low-density cohorts of juveniles in the F2 generation in turn leads to further differences in the dynamics. Increased competition in the high-density cohort leads to lower juvenile growth rates. Juvenile growth rate influences the size and age at which individuals mature. The relationship between size and age at maturity is ‘L’ shaped (Beckerman et al. 2003; Plaistow et al. 2004), with fast growing individuals maturing at the same age (and varying in size) and slow-growing individuals maturing at the minimum size (and varying in age; Plaistow et al. 2004). In the low-density cohorts, individuals receive more food, grow faster and mature simultaneously and at a large size. A cohort of adults maturing simultaneously will tend to die off simultaneously, giving rise to a ‘cohort cycle’. The subsequent death of a cohort of adults reduces competition, allowing juveniles to grow and mature together, thereby creating another cohort of adults. This pattern is visible in the large-egg cultures following the low-density juvenile F2 cohort (figure 2c, F2 cohort, days 25–55 and F3 cohort, days 55–90). Conversely, in the cultures with the high density of F2 juveniles, competition reduces food availability. Consequently, juveniles can only recruit when adult density declines to very low levels. Even then, food remains scarce, so individuals grow slowly and mature at different times, when each reaches the minimum size. This leads to a blurring of the cohort structure and no pronounced cohort cycles (figure 2c); instead there is an oscillation around the equilibrium adult population size which includes individuals from the F2 and F3 generations (figure 2c, days 30–90).

4. Discussion

This study shows that by changing the per capita investment in offspring, maternal effects can give rise to long-lasting differences in the population trajectories. The population dynamical effects arise through two interlinked mechanisms. Firstly, maternal effects may last more than one generation: egg size influences growth rate, which influences age and size at maturity, which influences egg size (S. J. Plaistow et al., unpublished work). Secondly, maternal allocation may indirectly affect growth rates by changing the competitive environment for offspring. This indirect effect arises due to the negative trade-off between the number and quality of offspring. Deciding to lay many eggs may increase the future competition for resources as well as reducing the per capita provisioning of each egg. Changing the juvenile competitive environment changes growth rates and sizes/ages at maturation, which, in turn, affects the allocation decisions the adults make.

The population response to any particular environmental state is probably to be contingent on details of the population structure at the time of the perturbation (Coulson et al. 2001; Clutton-Brock & Coulson 2002). Our results indicate that the differences in initial structure created by differences in egg size lead to different population trajectories for several generations, even though the populations were experiencing the same, constant, environment. Thus, a sequence of identical environmental perturbations applied to populations that have different initial size structures will lead to different population responses, as the effects of each perturbation will be propagated differently over time. This will occur because differences in the population structure create differences in the competition, and therefore per capita food availability. In turn, food availability changes the life history (growth, maturation, reproductive allocation), which further changes the population structure. Such mechanisms may explain why population synchrony is often lower than expected from the synchrony in the environment (Keeling & Grenfell 1999; Benton et al. 2001a). Interestingly, our data suggest that environmental states that give rise to cohorts of large eggs could ‘restart’ the cohort cycles which would otherwise decay away in constant conditions, leading to sustained periodic population fluctuations. Such states could arise through seasonal forcing or occasional catastrophic conditions.

We interpret the reproductive allocation patterns as an adaptive response to different resource levels. In a population setting, females will only be well fed if the population density is low. In such cases, the juvenile environment will tend not to be competitive and the advantage of laying larger eggs is offset by the fitness benefit of laying more eggs. Conversely, low-food environments are representative of high-competition environments, in which case better-provisioned offspring may do better than more poorly provisioned offspring from larger broods. This relationship is reminiscent of r- and K-selected strategies (MacArthur & Wilson 1967), and the plasticity of this response suggests that the population dynamics of this species naturally includes considerable variation in resources. The terminal reproductive investment of well-fed females may be due to their high reproductive output predicting that the juvenile environment will become more competitive, making large egg size a more profitable strategy (Glazier 1992).

Recent population models including maternal effects (Ginzburg & Taneyhill 1994; Inchausti & Ginzburg 1998; Ginzburg 1998) assume that there is a positive relationship between fecundity and maternal quality (defined, for example, as biomass; Ginzburg & Taneyhill 1994), and that high-quality mothers give rise to high-quality offspring. Our data suggest that the first assumption is correct, but the second one may not be: during part of their life, well-fed mothers give rise to eggs with less protein than more poorly fed mothers. Changing this assumption in the models (see Electronic Appendix) shows that maternal effects can still destabilize the population dynamics and lead to population cycles. However, these cycles are alternating high- and low-quality cohorts, and so are much shorter cycles than if offspring and maternal quality are positively associated (Ginzburg & Taneyhill 1994).

The initial cohorts of eggs were derived from stock cultures whose resources had differed for 2 months. It is possible that selection in these initial high- versus low-food cultures, rather than plastic changes in provisioning, led to the initial changes in egg size and dynamics. In this case, had the pre-experimental conditions caused notable genetic divergence between treatments, we might expect that the initial differences in individuals’ sizes between treatments would be maintained during the experiment. After the population dynamics converged, analysis of body sizes showed that size differences were also transient, suggesting that the differences we observed are the result of plasticity rather than selection.

Acknowledgments

NERC provided funding. Tara Marshall and Xavier Lambin provided feedback. Gillian Graham helped refine the idea of a population experiment in her undergraduate project.

Footnotes

†

Present address: Department of Animal & Plant Sciences, University of Sheffield, Western Bank, Sheffield S10 2TN, UK.

‡

Present address: Welcome Centre for Molecular Parasitology, University of Glasgow, Glasgow G11 6NU, UK.

Supplementary Material

rspb20053081s01.pdf (188.4KB, pdf)

References

  1. Albon S.D, Clutton-Brock T.H, Guinness F.E. Early development and population dynamics in red deer. 2. Density-independent effects and cohort variation. J. Anim. Ecol. 1987;56:69–81. [Google Scholar]
  2. Banks P.B, Powell F. Does maternal condition or predation risk influence small mammal population dynamics? Oikos. 2004;106:176–184. [Google Scholar]
  3. Beckerman A, Benton T.G, Ranta E, Kaitala V, Lundberg P. Population dynamic consequences of delayed life-history effects. Trends Ecol. Evol. 2002;17:263–269. [Google Scholar]
  4. Beckerman A.P, Benton T.G, Lapsley C.T, Koesters N. Talking 'bout my generation: environmental variability and cohort effects. Am. Nat. 2003;162:754–767. doi: 10.1086/381056. [DOI] [PubMed] [Google Scholar]
  5. Benton T.G, Lapsley C.T, Beckerman A.P. Population synchrony and environmental variation: an experimental demonstration. Ecol. Lett. 2001a;4:236–243. [Google Scholar]
  6. Benton T.G, Ranta E, Kaitala V, Beckerman A.P. Maternal effects and the stability of population dynamics in noisy environments. J. Anim. Ecol. 2001b;70:590–599. [Google Scholar]
  7. Bjørnstad O.N, Grenfell B.T. Noisy clockwork: time series analysis of population fluctuations in animals. Science. 2001;293:638–643. doi: 10.1126/science.1062226. [DOI] [PubMed] [Google Scholar]
  8. Clutton-Brock T.H, Coulson T. Comparative ungulate dynamics: the devil is in the detail. Phil. Trans. R. Soc. B. 2002;357:1285–1298. doi: 10.1098/rstb.2002.1128. 10.1098/rstb.2002.1128 [DOI] [PMC free article] [PubMed] [Google Scholar]
  9. Coulson T, Catchpole E.A, Albon S.D, Morgan B.J.T, Pemberton J.M, Clutton-Brock T.H, Crawley M.J, Grenfell B.T. Age, sex, density, winter weather, and population crashes in Soay sheep. Science. 2001;292:1528–1531. doi: 10.1126/science.292.5521.1528. [DOI] [PubMed] [Google Scholar]
  10. Einum S. Atlantic salmon growth in strongly food-limited environments: effects of egg size and paternal phenotype? Environ. Biol. Fishes. 2003;67:263–268. [Google Scholar]
  11. Einum S, Fleming I.A. Maternal effects of egg size in brown trout (Salmo trutta): norms of reaction to environmental quality. Proc. R. Soc. B. 1999;266:2095–2100. 10.1098/rspb.1999.0893 [Google Scholar]
  12. Einum S, Fleming I.A. Highly fecund mothers sacrifice offspring survival to maximize fitness. Nature. 2000;405:565–567. doi: 10.1038/35014600. [DOI] [PubMed] [Google Scholar]
  13. Erelli M.C, Elkinton J.S. Maternal effects on gypsy moth (Lepidoptera: Lymantriidae) population dynamics: a field experiment. Environ. Entomol. 2000;29:476–488. [Google Scholar]
  14. Ergon T, Lambin X, Stenseth N.C. Life-history traits of voles in a fluctuating population respond to the immediate environment. Nature. 2001;411:1043–1045. doi: 10.1038/35082553. [DOI] [PubMed] [Google Scholar]
  15. Forchhammer M.C, Clutton-Brock T.H, Lindstrom J, Albon S.D. Climate and population density induce long-term cohort variation in a northern ungulate. J. Anim. Ecol. 2001;70:721–729. [Google Scholar]
  16. Fox C.W, Savalli U.M. Inheritance of environmental variation in body size: superparasitism of seeds affects progeny and grandprogeny body size via a nongenetic maternal effect. Evolution. 1998;52:172–182. doi: 10.1111/j.1558-5646.1998.tb05150.x. [DOI] [PubMed] [Google Scholar]
  17. Ginzburg L.R. Inertial growth—population dynamics based on maternal effects. In: Mousseau T.A, Fox C.W, editors. Maternal effects as adaptations. Oxford University Press; Oxford: 1998. pp. 42–53. [Google Scholar]
  18. Ginzburg L.R, Taneyhill D.E. Population cycles of forest Lepidoptera—a maternal effect hypothesis. J. Anim. Ecol. 1994;63:79–92. [Google Scholar]
  19. Glazier D.S. Effects of food, genotype, and maternal size and age on offspring investment in Daphnia magna. Ecology. 1992;73:910–926. [Google Scholar]
  20. Greenman J.V, Benton T.G. The amplification of environmental noise in population models: causes and consequences. Am. Nat. 2003;161:225–239. doi: 10.1086/345784. [DOI] [PubMed] [Google Scholar]
  21. Inchausti P, Ginzburg L.R. Small mammals cycles in northern Europe: patterns and evidence for a maternal effect hypothesis. J. Anim. Ecol. 1998;67:180–194. [Google Scholar]
  22. Keeling M, Grenfell B. Stochastic dynamics and a power law for measles variability. Phil. Trans. R. Soc. B. 1999;354:769–776. doi: 10.1098/rstb.1999.0429. 10.1098/rstb.1999.0429 [DOI] [PMC free article] [PubMed] [Google Scholar]
  23. MacArthur R.H, Wilson E.O. Princeton University Press; Princeton: 1967. The theory of island biogeography. [Google Scholar]
  24. McIntyre G.S, Gooding R.H. Effects of maternal age on larval competitiveness in house flies. Heredity. 2000;85:480–489. doi: 10.1046/j.1365-2540.2000.00787.x. [DOI] [PubMed] [Google Scholar]
  25. Mousseau T.A, Fox C.W. Oxford University Press; Oxford: 1998. Maternal effects as adaptations. [Google Scholar]
  26. Myers J.H, Boettner G, Elkinton J. Maternal effects in gypsy moth: only sex ratio varies with population density. Ecology. 1998;79:305–314. [Google Scholar]
  27. Plaistow S.J, Lapsley C.T, Beckerman A.P, Benton T.G. Age and size at maturity: sex, environmental variability and developmental thresholds. Proc. R. Soc. B. 2004;271:919–924. doi: 10.1098/rspb.2004.2682. 10.1098/rspb.2004.2682 [DOI] [PMC free article] [PubMed] [Google Scholar]
  28. Reid J.M, Bignal E.M, Bignal S, McCracken D.I, Monaghan P. Environmental variability, life-history covariation and cohort effects in the red-billed chough Pyrrhocorax pyrrhocorax. J. Anim. Ecol. 2003;72:36–46. [Google Scholar]
  29. Rossiter M.C. Incidence and consequences of inherited environmental effects. Ann. Rev. Ecol. Syst. 1996;27:451–476. [Google Scholar]
  30. Saether B.E. Environmental stochasticity and population dynamics of large herbivores: a search for mechanisms. Trends Ecol. Evol. 1997;12:143–149. doi: 10.1016/s0169-5347(96)10068-9. [DOI] [PubMed] [Google Scholar]
  31. Sedmak J.J, Grossberg S.E. A rapid, sensitive and versatile assay for protein using coomassie brilliant blue, G250. Anal. Biochem. 1977;79:544–552. doi: 10.1016/0003-2697(77)90428-6. [DOI] [PubMed] [Google Scholar]
  32. Shaw D.J, Haydon D.T, Cattadori I.M, Hudson P.J, Thirgood S.J. The shape of red grouse cycles. J. Anim. Ecol. 2004;73:767–776. [Google Scholar]
  33. Shertzer K.W, Ellner S.P. Energy storage and the evolution of population dynamics. J. Theor. Biol. 2002;215:183–200. doi: 10.1006/jtbi.2001.2507. [DOI] [PubMed] [Google Scholar]
  34. Turchin P, Hanski I. Contrasting alternative hypotheses about rodent cycles by translating them into parameterized models. Ecol. Lett. 2001;4:267–276. [Google Scholar]

Associated Data

This section collects any data citations, data availability statements, or supplementary materials included in this article.

Supplementary Materials

rspb20053081s01.pdf (188.4KB, pdf)

Articles from Proceedings of the Royal Society B: Biological Sciences are provided here courtesy of The Royal Society

RESOURCES