Experimental evidence rejects pairwise modelling approach to coexistence in plant communities

Abstract

Competition is often invoked as the cause of plant species loss with increasing system productivity. Experimental results for multispecies assemblages are virtually absent and mathematical models are thus used to explore the relationship between competition and coexistence. Modelling approaches to coexistence and diversity in competitive communities commonly employ Lotka–Volterra-type (LV) models with additive pairwise competitive effects.

Using pairwise plant competition experiments, we calibrate the LV system and use it to predict plant biomass and coexistence in six three-species and one seven-species experimental mixture. Our results show that five out of the six three-species sets and the seven-species set deviate significantly from LV model predictions. Fitting an additional non-additive competition coefficient resulted in predictions that more closely matched the experimental results, with stable coexistence suggested in all but one case. These results are discussed with particular reference to the possible underlying mechanisms of coexistence in our experimental community. Modelling the effect of competition intensity on stability indicates that if non-additive effects occur, they will be relevant over a wide range of community sizes. Our findings caution against relying on coexistence predictions based on LV models.

1. Introduction

The relationship between plant competition and species richness has been the subject of many, mainly theoretical, studies (Bengtsson et al. 1994; Chesson 2000; Fargione & Tilman 2002). As biodiversity is an important determinant of ecosystem functioning (Reich et al. 2001), its causes are under intense study (Goldberg & Barton 1992). Competition has been invoked as the driving mechanism behind the loss of biodiversity following experimental fertilization (Gough et al. 2000) and along productivity gradients (Waide et al. 1999; Mittelbach et al. 2001). Experiments on plant competition are plentiful, but few involved more than two species, and hardly any investigated species interactions for one-, two- and three- (or more) species mixtures (Aarssen & Epp 1990; Goldberg & Barton 1992; Gibson et al. 1999). Hence, while there is a general agreement that competition is a real, ubiquitous and important phenomenon in natural communities (Brown et al. 2001), its role in determining species diversity and coexistence remains obscure.

Theoretical studies on plant competition and coexistence have commonly employed Lotka–Volterra-type (LV) competition models (for review see: Tilman 1982; Grover 1997; Chesson 2000) in which competition terms are assumed additive (Case 2000). Either LV models are modelled dynamically (Kokkoris et al. 1999, 2002; Wilson et al. 2003), or different model formulations are used but linearized around an equilibrium point, thereby making competitive effects additive (May 1973; Rozdilsky & Stone 2001). Despite the oversimplified nature of LV models (especially the complete lack of mechanisms, its inability to incorporate two or more resources, the unrealistic symmetry of competition, its assumption of logistic growth; Grover 1997), they are still the most common set of equations used to model competition (for recent examples see Loreau 1998; Kokkoris et al. 1999, 2002; Lehman & Tilman 2000; Rozdilsky & Stone 2001; Byers & Noonburg 2003; Doncaster et al. 2003; Wilson et al. 2003; Loreau 2004). Additive competition effect models led to the formulation of the community matrix approach (May 1976; Case 2000; Dambacher et al. 2003) of community stability on the basis of monoculture and pairwise competition experiments (Wilson & Roxburgh 1992; Roxburgh & Wilson 2000). To date, the ability of LV-based models to encapsulate possibly important non-additive effects in plant communities has not received thorough experimental testing (but see Vandermeer 1969 for protozoa). This is simply because competition experiments with more than two species are very laborious and hence extremely uncommon (Goldberg & Barton 1992). The minimum data needed to test whether LV models can predict coexistence of multiple species are the biomasses of monocultures of all species, all two-species mixtures and multiple-species mixtures.

Here, we report on the results and analysis of a series of three-species and one seven-species competition experiments augmented with all pairwise mixtures and monocultures in an attempt to investigate the ability of LV models to predict the outcome of multispecies competition. We ask if the LV approach fits the data within the accuracy of the data, but we do not attempt to find a better model. Mechanistic ecological explanations for the discrepancy between observed and predicted data are then discussed.

2. Methods

(a) Applying the Lotka–Volterra approach

The growth of species A in a three-species mixture is a function of the direct effect of species B and C on A, subject to the effects that B and C have on one-another (indirect effects: Stone & Roberts 1991; Wootton 1994a,b). In the differential equation notation commonly used to model multispecies interactions (Wilson et al. 2003) this reads

$$\begin{array}{l}\text{d}{w}_{\text{A}\text{.BC}}/\text{d}t=r_{\text{A}}{w}_{\text{A}\text{.BC}}/K_{\text{A}}(K_{\text{A}}-w_{\text{A}\text{.BC}}-{\alpha }_{\text{AB}}{w}_{\text{B}\text{.AC}}-{\alpha }_{\text{AC}}{w}_{\text{C}\text{.AB}})\hfill \\ \text{d}{w}_{\text{B}\text{.AC}}/\text{d}t=r_{\text{B}}{w}_{\text{B}\text{.AC}}/K_{\text{B}}(K_{\text{B}}-w_{\text{B}\text{.AC}}-{\alpha }_{\text{BA}}{w}_{\text{A}\text{.BC}}-{\alpha }_{\text{BC}}{w}_{\text{C}\text{.AB}})\hfill \\ \text{d}{w}_{\text{C}\text{.AB}}/\text{d}t=r_{\text{C}}{w}_{\text{C}\text{.AB}}/K_{\text{C}}(K_{\text{C}}-w_{\text{C}\text{.AB}}-{\alpha }_{\text{CA}}{w}_{\text{A}\text{.BC}}-{\alpha }_{\text{CB}}{w}_{\text{B}\text{.AC}})\hfill \end{array}\},$$ 2.1

where w_X and r_X are the biomass and growth rate of species X, α are influence constants (i.e. competition coefficients) and K are the species' carrying capacities. For example, w_A.BC represents the biomass of species A in competition with B and C, and α_CA is the competitive effect of species A on species C.

At equilibrium, the two-species LV equation system simplifies to (as suggested by Gotelli 1995, p. 115)

$${\alpha }_{\text{AB}}=(K_{\text{A}}-w_{\text{A}\text{.B}})/w_{\text{B}\text{.A}}\text{and}{\alpha }_{\text{BA}}=(K_{\text{B}}-w_{\text{B}\text{.A}})/w_{\text{A}\text{.B}}.$$ 2.2

To investigate non-additivity, we introduced another parameter to the LV equations, which can be interpreted as the degree of deviation from additivity. For species A the parameter is α_A.BC, and the growth equation is (with analogous equations for species B and C)

$$\text{d}{w}_{\text{A}\text{.BC}}/\text{d}t=r_{\text{A}}{w}_{\text{A}\text{.BC}}/K_{\text{A}}[K_{\text{A}}-w_{\text{A}\text{.BC}}-{\alpha }_{\text{A}\text{.BC}}({\alpha }_{\text{AB}}{w}_{\text{B}\text{.AC}}+{\alpha }_{\text{AC}}{w}_{\text{C}\text{.AB}})].$$ 2.3

If the LV approach is valid, we expect α_A.BC to be unity. At equilibrium this simplifies to

$${\alpha }_{\text{A}\text{.BC}}=(K_{\text{A}}-w_{\text{A}\text{.BC}})/({\alpha }_{\text{AB}}{w}_{\text{B}\text{.AC}}+{\alpha }_{\text{AC}}{w}_{\text{C}\text{.AB}}).$$ 2.4

To fit this model to experimental data, the pairwise competition coefficients can be replaced by equation (2.2)

$${\alpha }_{\text{A}\text{.BC}}=(K_{\text{A}}-w_{\text{A}\text{.BC}})/[((K_{\text{A}}-w_{\text{A}\text{.B}})/w_{\text{B}\text{.A}})w_{\text{B}\text{.AC}}+((K_{\text{B}}-w_{\text{B}\text{.A}})/w_{\text{A}\text{.B}})w_{\text{C}\text{.AB}}],$$

or, slightly more concisely,

$${\alpha }_{\text{A}\text{.BC}}=(K_{\text{A}}-w_{\text{A}\text{.BC}})/[w_{\text{B}\text{.AC}}/w_{\text{B}\text{.A}}(K_{\text{A}}-w_{\text{A}\text{.B}})+w_{\text{C}\text{.AB}}/w_{\text{C}\text{.A}}(K_{\text{A}}-w_{\text{A}\text{.C}})].$$ 2.5

This approach can be readily extended to more competing species.

(b) Experimental study

For seven plant species (Agrostis capillaris (Ac), Festuca rubra (Fr), Holcus lanatus (Hl), Hydrocotyle heteromeria (Hh), Prunella vulgaris (Pv), Ranunculus repens (Rr) and Trifolium repens (Tr)), monocultures and all pairwise competition treatments were established in pots with three ramets of each species, and grown for 1 year. A double-density (six ramets) treatment and an additional single-density monoculture (allowed to grow for 2 years) were established to test for equilibrium biomass (i.e. constant final yield; Aarssen 1985). Six three-species and one seven-species mixture were additionally established (with three ramets of each species). At the end of the experiment all above-ground biomass was harvested, sorted, dried and weighed. This experiment is part of a larger study investigating competition and coexistence within a managed lawn community. See Roxburgh & Wilson (2000) for further details.

(c) Statistical analysis

Pairwise competition coefficients were calculated according to equation (2.2), and the mean biomass of the double-density monoculture treatment for each species was used as an estimate of the carrying capacity (K), and the plant biomass (w) was estimated from the experimental pairwise mixtures. Equation (2.5) was fitted using a nonlinear model (function nls in the software package R), with α_A.BC being the parameter to be optimized. Deviance of α_A.BC from unity was tested using a two-sided t-test on parameter estimates and standard error.

For the prediction of biomass in the three-species and seven-species mixtures we additionally calculated plant growth rate r by fitting a logistic growth curve to initial and final biomass data from monocultures, with a weekly step size (using function optim and lsoda; see Electronic Appendix for R-code of this analysis). Predicted final biomass in three-species mixtures was then calculated by simulating for 12 months the equation system (2.1) (for LV predictions) or (2.3) (for non-additive predictions).

(d) Modelling the effect of community size and mean competitive intensity on stability

We analysed competition coefficient matrices for a range of community sizes (2–20 competing species). For each community size, we constructed 10 matrices with the same values for r and K for each species, and off-diagonal competition coefficients randomly sampled from a uniform distribution over an interval from [X−0.1, X+0.1], for varying values of X. (Normally distributed competition coefficients resulted in qualitatively identical results, but led to negative competition coefficients, indicative of positive interactions. As we wanted to analyse purely competitive communities, such as our experimental ones, we used the uniform distribution.) The diagonal competition coefficients were set to 1. If the real part of the maximum eigenvalue of the community matrix (Re(EV)_max) is less than 0, the community is deemed stable (Case 2000; see Appendix A).

4. Results

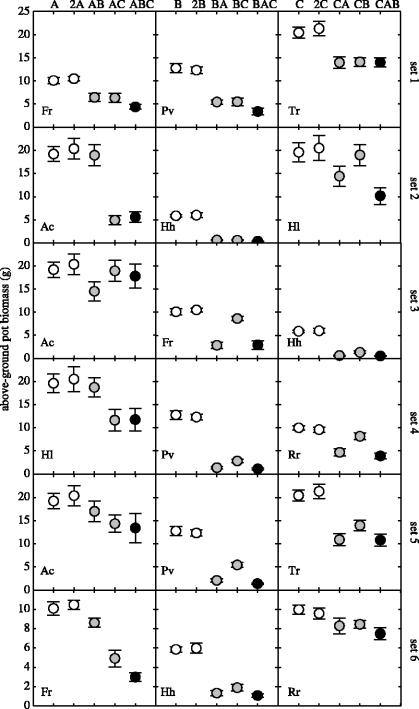
Overall, our experimental results indicate strong competition in almost all species pairs and in three-species mixtures (figure 1). The species Hh and Pv were relatively weak competitors and had only marginal impacts on the other species (sets 2, 3 and 4). In most cases (14 out of 18; figure 1) the biomass in the three-species mixture was lower than in either pairwise pot.

Figure 1.

Above-ground biomass of target species per pot with standard error bars (n=10). Each panel row represents one three-species set. Every panel displays data for single- and double-density monoculture (A and 2A, respectively; white dots), pairwise competition (AB and AC, grey dots) and the three-species mixture (ABC, black dot). The first to third panel of each row refer to species A, B and C, respectively, with the species abbreviation given in the lower left corner (see §2 for species names).

Biomass in single-density monocultures was indistinguishable from that in double-density monocultures (figure 1), indicating that monocultures had reached equilibrium at the time of harvest. The replicate set of single-density monocultures allowed to grow for another year also yielded the same average biomass, supporting the assumption of equilibrium (data not shown). Moreover, pairwise competition coefficients based on equation (2.2) (which assumes equilibrium) were highly consistent with those calculated by fitting two-species LV models to the time-course data for each pairwise experiment (r=0.9958, t_1,47=74.32, p<0.001), further indicating that equilibrium assumptions were not severely violated (data not shown).

Using the above approach, we investigated the appropriateness of LV models to predict final species biomass for six different three-species mixtures. In all six three-species mixtures we found at least one non-additivity coefficient significantly different from unity (table 1). In 13 out of 18 cases the fitted α_A.BC differed significantly from 1, indicating non-additive competition effects (table 1). In those 13 significant cases, competition was less than additive, as shown by the α_A.BC-values being less than 1 (mean for all 18 cases=0.706, s.e.=0.0562). Thus it appears that competition from two simultaneous competitors is weaker than one would expect based on simple extrapolation of the pairwise LV model.

Table 1.

Estimates for non-additivity coefficient α_A.BC.

set	species	αA.BC	s.e.	p
1	Fr	0.581	0.142	0.0080
1	Pv	0.626	0.063	0.0001
1	Tr	0.623	0.073	0.0005
2	Ac	0.903	0.173	0.2947
2	Hh	1.070	0.124	0.2930
2	Hl	0.562	0.112	0.0018
3	Ac	0.285	0.106	<0.0001
3	Fr	0.655	0.062	0.0002
3	Hh	0.762	0.055	0.0009
4	Hl	0.553	0.082	0.0002
4	Pv	0.926	0.059	0.1222
4	Rr	0.936	0.111	0.2898
5	Ac	0.415	0.111	0.0003
5	Pv	0.753	0.061	0.0014
5	Tr	0.881	0.054	0.0274
6	Fr	1.020	0.107	0.4306
6	Hh	0.861	0.051	0.0120
6	Rr	0.259	0.268	0.0111

Estimates are derived from fitting equation (2.5) to three-species competition data, for every species in the six sets. Species are abbreviated by the initials of their scientific name (see §2 for names). Bold printed p-values indicate significant deviations from unity (two-sided t-test, 9 d.f.). For three out of six sets (sets 1, 3 and 5), all three species within the mixture had significant non-additivity coefficients.

This has consequences for the coexistence of the three species. Stability analysis (see Appendix A) shows that none of the six three-species sets is stable according to LV dynamics. Incorporating the non-additivity coefficient α_A.BC led to stable coexistence in five out of six sets. Moreover, in four out of five cases the predicted equilibrium abundances from the non-additive model were very close to the experimental biomasses observed after 12 months growth (table 2).

Table 2.

Stability analysis and predicted/observed equilibrium biomass.

set	Lotka–Volterra model				non-additive model				experimental results
biomass at equilibrium				biomass at equilibrium				biomass after 12 months (mean and s.e.)
A	B	C	Re(EV)max	A	B	C	Re(EV)max	A	B	C
1	−75.82	151.98	−99.29	0.3691	6.69	3.60	13.23	−0.0416	4.38 (0.54)	3.38 (0.69)	14.02 (0.94)
2	3.24	1.34	13.15	0.0062	3.93	−1.05	19.27	0.0161	5.59 (1.13)	0.38 (0.08)	10.16 (1.80)
3	3.66	6.68	1.33	0.0014	17.76	3.85	0.56	−0.0091	17.82 (2.60)	2.91 (0.92)	0.54 (0.09)
4	11.70	−0.10	4.69	0.0006	18.20	0.04	2.16	−0.0004	11.74 (2.48)	1.10 (0.20)	3.88 (0.63)
5	−28.69	29.79	1.86	−0.0028	17.74	0.85	8.92	−0.0104	13.39 (3.19)	1.39 (0.15)	10.80 (1.30)
6	9.05	−3.70	9.87	0.0327	3.25	0.47	9.60	−0.0038	2.98 (0.44)	1.08 (0.16)	7.47 (0.61)

Bold printed equilibrium biomass and maximum eigenvalues (Re(EV)_max) indicate stable and feasible coexistence.

The overall correlation of the LV model predictions to the observed data was very poor (Nash–Sutcliffe efficiency: R²=−88.28, n=18; a negative R² indicates that mean observed biomass across all species and set is a better predictor than model predictions) and improved notably by incorporating the non-additivity coefficient (R²=0.661; i.e. the model is now much better than the grand observed mean).

For the seven-species mixture all seven non-additivity coefficients deviate significantly from unity (α_A.BC±s.e. for seven-species mixtures: Ac 0.74±0.148, Fr 1.96±0.286, Hh 2.51±0.420, Hl 0.72±0.145, Pv 1.15±0.193, Rr 1.95±0.293, Tr 0.73±0.171; p<0.001 in all cases). Here, however, biomass of the focal species is on average lower than expected from the LV model (overall mean for α_A.BC=1.39, s.e.=0.278). The difference between average α_A.BC in three- and seven-species mixtures indicates that not only are competitive effects non-additive, but they can also be greater or less than expected from LV model predictions.

Both approaches predict unfeasible and unstable equilibria for the seven-species mixture (an unfeasible equilibrium occurs when at least one of the species is predicted to have a negative equilibrium biomass). A subset analysis was not possible, because new non-additivity coefficients would have to be fitted on the basis of a six-, five- or four-species mixture, which were not part of the experimental design. Here, both the LV model and its non-additive version failed to estimate the observed species biomasses (LV: R²=−60.27; non-additive: R²=−182.1).

5. Discussion

This first direct experimental test discloses the problems of using LV competition models to predict plant species biomass or coexistence. We do not suggest that our non-additivity coefficient presents a recommended way to incorporate non-additive effects. It is simply a statistical parameter to investigate the phenomenon of non-additivity. Even this rough parameter, however, alters coexistence scenarios and predicted biomass values considerably. Our non-additivity parameter was less than one in the three-species mixtures, indicating less competition than would be expected from LV dynamics, but greater than one in the seven-species mixture. Until further data of this kind become available, it remains unclear if there is a correlation between non-additivity and species richness. Figure 2 illustrates the way our correction factor affects stability. For the three-species mixtures the arrow indicates a reduction of the interspecific competition coefficient values relative to intraspecific, leading the community into an area of stability. The opposite is true for the seven-species community, where the trajectory moves towards greater instability. As the stability isoclines decrease only slowly with the number of species, non-additive phenomena can be expected to be important over a wide richness range, although more intensive modelling is required to test the generality of this result. Further elaborations might include varying the growth rates and carrying capacities of the species and analysing only the feasible subset of solutions (Roberts 1974), exploring the consequences of community assembly (Rummel & Roughgarden 1985), and exploring variations in the topological patterns of coefficients within the competition matrices (Roxburgh & Wilson 2000).

Figure 2.

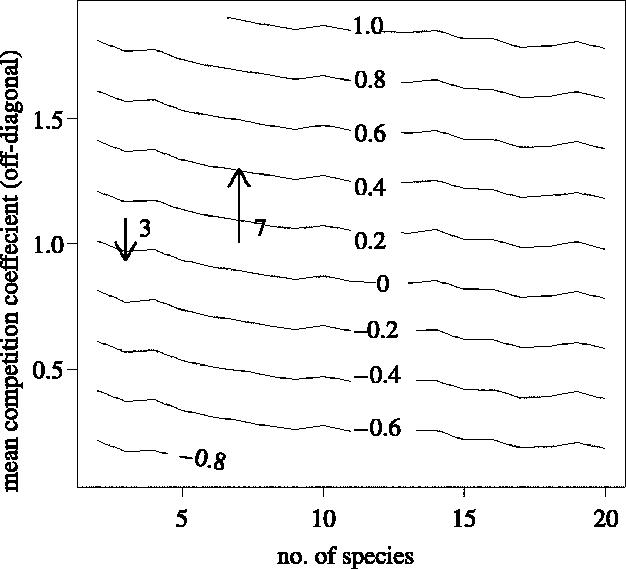
Maximum values of the real parts of the community matrix eigenvalues (Re(EV)_max) as a function of community size and mean competition intensity. Negative values of Re(EV)_max indicate stable communities. Arrows illustrate effect of the non-additivity parameter α_A.BC for the three- and seven-species communities (as indicated by the number next to the arrow), which shifts off-diagonal mean competition coefficients and hence the community matrix eigenvalues.

Ecologically, the failure of the LV model suggests the presence of coexistence-promoting mechanisms that are missing from the LV equations. Although our statistical technique is sufficient to reject the LV formulation for predicting the outcome of competition among more than two species, it cannot provide insight into what the underlying basis for this discrepancy might be. We will now consider some possible explanations.

Case & Bender (1981) suggested two ways in which LV-type models might fail to capture multi-species competitive effects. The first are the presence of ‘higher-order’ interactions, also called ‘interaction modifications’ (Wootton 1994a,b). These are captured in our analysis by the generic term α_A.BC. The second is the possibility of nonlinear single-species terms, whereby monoculture growth is a nonlinear function of density. This was not able to be tested here, simply because we lack the appropriate experimental data at several densities. However, nonlinear single-species growth terms, perhaps in combination with higher-order interactions, provide a potential alternative explanation for our results.

More generally, understanding the ecological basis for the failure of the LV model to predict multi-species competition will be gained only by adopting a more mechanistic approach to species growth and competition, as advocated by Tilman (1987), and many others. In terrestrial plants, this most often takes the form of explicit representations of the resources for which the species are competing, and their dynamics (Tilman 1982; Grover 1997). Mechanistic formulations also allow fluctuation-dependent coexistence-promoting mechanisms to be expressed (Chesson 1994; Roxburgh et al. 2004), or spatial arrangement effects (Stoll & Prati 2001; Hartley & Shorrocks 2002). Adopting a mechanistic approach has the further advantage that the model has greater predictive capability, such that interactions between the plants and the resources become more transparent, and hence more readily interpreted, including departures from additivity.

In the context of our experimental species, previous work within the same community has suggested a number of possibilities for the underlying mechanistic basis of the competitive interactions. Roxburgh et al. (1993) detected consistent vertical stratification patterns within the plant canopy. This analysis showed that Tr occupied the highest strata in the canopy, with Hl, Ac and Hh occurring predominantly in the mid-canopy, and Pv and Fr dominating closest to the ground (Rr, one of the components of the competition experiments analysed here, was not reported in this study). This vertical ranking agrees approximately with the ranking of competitive abilities based on pairwise competition experiments (Roxburgh & Wilson 2000), with the most competitive species occupying the uppermost canopy positions. There are also species differences in the shapes and orientations of the leaf laminae. The forbs Tr and Hh both have horizontal laminae at the top of near-vertical petioles; the grasses Hl, Ac and Fr all tend to hold their laminae vertically, and the forbs Pv and Rr are intermediate, with broad leaves held approximately horizontally within the canopy. Consistent vertical stratification within the plant canopy and a variety of leaf morphologies therefore provides opportunities for differentiating the use of light resource, and hence provides opportunities for above-ground niche differentiation. With respect to light competition and the potential for non-additivity under the LV formulation, Wootton (1994a,b) suggested a multiplicative rather than the LV additive pairwise approach might be more appropriate, on the basis that competition for light might affect population growth rates multiplicatively if each plant captures a fraction, rather than a given amount, of the light intercepted. This hypothesis is consistent with the inability of the LV model to adequately predict the multi-species experimental results reported here, but remains untested.

In other studies of these species, statistical tests have detected the presence of two competitive guilds structuring the community, with Ac, Hl, Tr and Rr belonging to one guild, and Fr, Hh and Pv to the other (Wilson & Roxburgh 1994, 2001). It was shown that members from the two different guilds tended to co-occur at a fine spatial scale, consistent with the assumption that competition is most intense among species within the same guild (Wilson & Roxburgh 1994), an assumption that was later confirmed experimentally (Wilson & Roxburgh 2001). Although not entirely consistent, the members of the first guild tend to be those species that occur at the top of the canopy, and those in the second guild to be lowest in the canopy (the notable exceptions are Rr in the first guild and Hh in the second). However, as noted by Wilson & Roxburgh (2001), coexistence is likely to be determined by many characters of the plants, and many of those characters will interact, hence the actual niche partitioning need not be related to a single morphological trait, such as simple differences in above-ground morphology.

Although we suggest above-ground morphological differentiation as a possible mechanism of coexistence in these species, partitioning of below-ground nutrient and water resources by complementary plant root architecture cannot be discounted. Overall, to gain a more mechanistic understanding of the species interactions in our experimental system requires study of the resources for which the species are competing, along with development of a theoretical framework within which greater mechanistic detail of those interactions is explicitly recognized. The LV model has been criticized for a long time, largely on theoretical grounds, for its inability to capture ‘higher-order interactions’ (Levine 1976; Pomerantz 1981; Billick & Case 1994; Wootton 1994a,b; Beckerman et al. 1997). Despite this, and partly because it yields the same predictions under equilibrium conditions as a single-resource competition model (Tilman 1982), the LV model is still in much use among theoretical ecologists seeking to understand the effect of competition on community structure, coexistence and community stability (Case 2000; Kokkoris et al. 2002; Wilmers et al. 2002). These theoretical studies often contribute significantly to our understanding of the role of biotic interactions in ecosystems (Loreau et al. 2001). It is therefore important to assure their appropriateness by subjecting their predictions to experimental tests.

Appendix A.Stability analysis for three-species mixtures

(a) Lotka–Volterra equations

For a three-species LV system with a vector of carrying capacities K and the matrix containing competition coefficients α the equilibrium biomass (N^*) for the three species can be calculated as (Case 2000)

$${\mathit{N}}^{*}={\mathit{\alpha }}^{-1}\mathit{K}.$$

Only if all ${\mathit{N}}_i^{*}>0$ an equilibrium is feasible.

The stability of the equilibrium solution is found by constructing the community matrix S with diagonal entries defined as (Case 2000)

$$s_{ii}=-r_i/K_i{N}_i^{*},$$

and off-diagonal entries defined as

$$s_{ij}=-r_i/K_i{N}_i^{*}{\alpha }_{ij}.$$

The equilibrium is stable if the real parts of all eigenvalues of S are negative, or equivalently, if the real part of the maximum eigenvalue (Re(EV)_max) is negative.

(b) Non-additive version

The only modification here is that of the competition matrix α. Off-diagonal entries are multiplied with the non-additivity coefficient α_A.BC calculated in table 1. This yields a new competition matrix α′ and new equilibrium biomasses N*′. Also, the off-diagonal elements of the community matrix entries have to be multiplied with α_A.BC, generating a new community matrix S′, which can then be evaluated for stability. The results of the stability analysis are given in the main table 2.

We see that the non-additivity parameter simply alters the intensity of interspecific competition relative to intraspecific competition, thereby allowing for coexistence in some cases (figure 2). The general rule for competitive coexistence is that interspecific competition must be less severe than intraspecific competition (Case 2000, p. 331). If the non-additivity parameter is less than 1, it affects the interspecific competition coefficients in the direction of stability.