Abstract
Aggression is ubiquitous in the animal kingdom, whenever the interests of individuals conflict. In contests between animals, the larger opponent is often victorious. However, counter intuitively, an individual that has little chance of winning (generally smaller individuals) sometimes initiates contests. A number of hypotheses have been put forward to explain this behaviour, including the ‘desperado effect’ according to which, the likely losers initiate aggression due to lack of alternative options. An alternative explanation suggested recently is that likely losers attack due to an error in perception: they mistakenly perceive their chances of winning as being greater than they are. We show that explaining the apparently maladaptive aggression initiated by the likely loser can be explained on purely economic grounds, without requiring either the desperado effect or perception errors. Using a game-theoretical model, we show that if smaller individuals can accurately assess their chance of winning, if this chance is less than, but close to, a half, and if resources are scarce (or the contested resource is of relatively low value), they are predicted to be as aggressive as their larger opponents. In addition, when resources are abundant, and small individuals have some chance of winning, they may be more aggressive than their larger opponents, as it may benefit larger individuals to avoid the costs of fighting and seek alternative uncontested resources.
Keywords: fighting, aggression, Napoleon complex, game theory
1. Introduction
Aggression is ubiquitous in the animal kingdom, occurring whenever the interests of individuals conflict (Huntingford & Turner 1987). It has been shown that differences in resource holding potential (RHP; Parker 1974) play an important role in determining the outcome of contests (Maynard Smith & Parker 1976; Enquist & Leimar 1983; Beaugrand et al. 1996; Petersen & Hardy 1996). Individuals may assess their RHP relative to that of their opponent before making a decision as to how to proceed in a contest, and low RHP individuals may avoid costly competition with superior rivals by assessing their relative inferiority and retreating (Enquist & Leimar 1983). Larger individuals are likely to win an escalated contest, whereas smaller ones are unlikely to obtain the disputed resource in such a contest, and often pay a cost associated with fighting (Parker 1974). Although there are many examples of larger individuals acting aggressively towards smaller ones (e.g. Zack 1975; Brace & Pavey 1978; Dowds & Elwood 1985; Turner & Huntingford 1986; Lindström 1992; Keeley & Grant 1993), there are also cases where aggression is predominantly initiated by the smaller competitors (Dow et al. 1976; Enquist & Jakobsson 1986; Ribowski & Franck 1993; Smith et al. 1994; Morris et al. 1995; Moretz 2003). As one would expect small individuals to be the likely losers, this behaviour may seem irrational, and therefore the evolution of such a ‘Napoleon complex’ (Just & Morris 2003) is intriguing.
There are several possible explanations for why small individuals may initiate fights or act aggressively towards larger individuals. Firstly, small individuals may perceive themselves as being of higher RHP or larger size than their opponent and initiate escalation by mistake (Bradbury & Vehrencamp 1998), or they may not be able to assess relative size prior to an interaction if such information is only obtained through display (Smith et al. 1994). Secondly, small individuals may value the resource item more highly than larger individuals, that is, there is a resource value asymmetry between the contestants, known to influence the outcome of fights (e.g. Davies & Houston 1981; Shutler & Weatherhead 1992; Johnsson & Forser 2002). For instance, in pumpkinseed sunfish Lepomis gibbosus, when probable losers were provided with more food (increasing the expected value of the resource), they became more likely to attack (Dugatkin & Ohlsen 1990). Thirdly, small individuals may attack because this increases the probability that they win the fight. Conflict outcome in favour of the initiator has been observed in a number of species (Jackson 1991; Hack 1997; Taylor et al. 2001). Finally, small individuals may attack because they have few alternative opportunities to obtain resources (the ‘desperado effect’; Grafen 1987). Grafen (1987) pointed out that any convention that leaves a group of individuals unable to gain access to a resource cannot be evolutionarily stable, since members of the excluded group have nothing to lose by ignoring the convention. Thus, if smaller individuals were to always retreat from a resource when they found it contested by a larger individual, they would never be able to obtain such resources, and so aggression by small individuals might be predicted.
Most theoretical models of animal contests, such as the sequential assessment game (Enquist & Leimar 1983; Leimar & Enquist 1984), do not predict which individual should initiate an escalated contest: escalation is assumed to occur simultaneously. Hurd & Enquist (1998) predicted that weaker individuals should attack larger opponents when they have few alternative options, and they are unaware of their opponent's strength. Otherwise, weaker individuals are predicted to retreat, leaving the stronger to claim the resource. However, Hurd & Enquist (1998) assumed that the stronger individual would always be victorious in a contest. Mesterton-Gibbons (1994) investigated the effect of variation in RHP on aggression, and found that under certain limited conditions, a low RHP individual could be expected to become involved in an escalated contest with a higher RHP opponent, but only when the difference in RHP was small. Hurd & Enquist (1998) and Mesterton-Gibbons (1994) predict equal levels of aggression from low and high RHP individuals despite asymmetries in RHP, but do not predict that low RHP individuals should be more aggressive than their opponents of higher RHP.
Although body size has been found to be a good surrogate measure of RHP (e.g. Morris et al. 1995; Jennions & Backwell 1996), the larger individual may not always be successful in a contest. In some cases, smaller individuals may win, as other factors may contribute to fighting ability (e.g. Marden & Waage 1990; Brick 1999). In an investigation of ownership priority as a convention for settling disputes (bourgeois behaviour) in the hawk–dove game, Eshel & Sansone (2001) found that ownership priority is replaced by strength priority (i.e. weak individuals give way to stronger ones) when the availability of territories and the costs of fighting decreases. However, when resources are scarce and non-owners suffer from a high death rate, smaller individuals are predicted to attack opponents that are not much larger than themselves. Where one individual is already resident at a resource, stronger individuals are likely to accumulate as owners (since they have a higher probability of winning a contest), leaving the weaker individuals as floaters. Both respect for ownership and respect for strength would leave these individuals in a desperado position (Grafen 1987).
Investigating the question of why small males initiate escalation in fights in the absence of resource value or ownership asymmetries, Just & Morris (2003) developed a model where individuals estimate their probability of winning a fight, based on the difference in RHP between them. They found that if there is error in this estimation, then likely losers may perceive themselves to be likely winners, and initiate escalation, while likely winners may not always attack first. But is it necessary to invoke perception errors as an explanation for aggression directed from small to large individuals, or is limited resource availability (Grafen 1987) enough to cause small individuals to initiate fights that they are likely to lose? We investigate the aggressive behaviour of individuals differing in RHP when there is no complicating residency asymmetry (for example, contests over food resources rather than territories), and ask whether aggression should be initiated by the small or large contestant, when both competitors are aware of their own and their opponent's fighting ability, but when body size (or RHP) does not perfectly determine fighting outcome.
2. Model
Two individuals that differ in size compete for access to a resource. Each individual can choose one of three behavioural options: it can attack the other (A), display while waiting for its opponent to attack, and then retaliate (W), or retreat from the conflict (R). Throughout, capital letters (A, W, R) refer to the behavioural choice of the larger individual and lower-case letters (a, w, r) to the behavioural choice of the smaller individual. In a bimatrix game, where the payoffs to the opponents differ due to differences in their RHP, and both competitors are aware of their own and their opponent's RHP, mixed strategies cannot be evolutionarily stable (Selten 1980; Crowley 2000), and thus we restrict our analysis to pure strategies.
The smaller individual has a probability p of winning a contest where both individuals choose the same behavioural option (i.e. both decide to attack or wait). We assume that the first to initiate aggression gains an advantage in the fight (e.g. Taylor et al. 2001). Where the smaller individual chooses to attack and the larger individual chooses to wait, the probability of the smaller individual winning increases by α. Likewise, when the smaller individual chooses to wait and the larger individual chooses to attack, the probability that the smaller individual wins the fight decreases by α.
The individuals contest a resource of value V. If one individual chooses to attack and the other to display, the displaying individual will retaliate, and a fight will occur. A fight also occurs if both individuals choose to attack. In either case, both individuals pay a cost cf. If both choose to wait/display, they pay a cost cd. The cost of displaying (cd) is lower than that of fighting (cf). If one individual chooses to attack while the other chooses to retreat (i.e. the smaller individual chooses a and the larger individual chooses R, or the smaller individual chooses r and the larger individual chooses A), the retreating individual pays a cost β, and the attacking individual pays a cost γ. Both costs β and γ are less than the cost paid if a fight occurs (cf). If one individual retreats, the other gains full access to the resource, and can claim the benefits V. If one or both individuals retreat, they have a probability F (F<1) of finding an uncontested resource. This effectively reduces the value of the contested resource: the relative resource value is defined as V−FV.
Table 1 outlines the payoff matrix for the contest for the smaller (a) and larger (b) individuals, for each potential combination of behavioural options. The fitness of an individual is calculated from the total payoff it receives, which depends on the behavioural choice of that individual, and the behavioural choice of the opponent. For example, if both individuals choose to attack (a and A), the payoff to the smaller individual is pV−cf, and the payoff to the larger individual is (1−p)V−cf.
Table 1.
Payoffs to (a) the smaller individual and (b) the larger individual in an interaction, dependent on their respective behaviours.
| behavioural choice of the larger individual | ||||
|---|---|---|---|---|
| A | W | R | ||
| behavioural choice of the smaller individual (a) | a | pV−cf | (p+α)V−cf | V−γ |
| w | (p−α)V−cf | pV−cd | V | |
| r | FV−β | FV | FV | |
| behavioural choice of the smaller individual (b) | a | (1−p)V−cf | (1−(p+α))V−cf | FV−β |
| w | (1−(p−α))V−cf | (1−p)V−cd | FV | |
| r | V−γ | V | FV | |
We derive the best replies to the strategy of the opponent in the following way, and summarize these in table 2: if the larger individual chooses to attack (A), the small individual should reply by attacking (a) if the payoff from doing so is greater than the payoff from either displaying (w) or retreating (r). Thus, a is a best response to A if
| 2.1 |
and
| 2.2 |
Inequality (2.1) is satisfied whenever α>0, that is, whenever there is an advantage to initiating aggression, which is assumed to be true, and inequality (2.2) is satisfied when
| 2.3 |
Displaying (w) is the best response to A when it pays more than a or r, which occurs when inequality (2.1) is not satisfied, and when
| 2.4 |
which is satisfied when
| 2.5 |
Table 2.
Conditions and responses to all opponent strategies.
| opponent strategy | response | conditions | |||||
|---|---|---|---|---|---|---|---|
| A | a | (2.3) | |||||
| w | never | ||||||
| r |
|
||||||
| W | a |
(2.7) and
|
|||||
| w |
|
||||||
| r |
|
||||||
| R | a | never | |||||
| w | always, as γ>0 and F<1 | ||||||
| r | never | ||||||
| a | A |
|
|||||
| W | never | ||||||
| R |
|
||||||
| w | A |
(2.7) and
|
|||||
| W |
(2.9) and
|
||||||
| R |
|
||||||
| r | A | never | |||||
| W | always, as γ>0 and F<1 | ||||||
| R | never |
As α is always positive, inequality (2.1) is always satisfied, and thus w is never a best response to A. Retreating (r) is the best response to A when it pays more than a or w, which occurs when inequalities (2.3) and (2.5) are both not satisfied. By observation, this only requires that inequality (2.3) is not satisfied. The conditions for the best responses to A, W, R, a, w and r are summarized in table 2.
We can now proceed to specify which combinations of pure strategies are candidate evolutionary stable strategies (ESS), and the conditions under which these can occur.
Both attack (A–a). Both individuals should attack when inequalities (2.3) and (2.13) are satisfied; by observation, satisfying equation (2.3) guarantees satisfaction of (2.13).
Both display (W–w). Both individuals should choose to display if inequalities (2.9), (2.10) and (2.16) are satisfied.
Both retreat (R–r). Both individuals retreating can never be an ESS, since retreating is never the best response to a retreating opponent.
Attack–display (A–w) and display–attack (W–a). Attack–display and display–attack can never be ESSs, because waiting is never the best response to an attacking opponent, because the payoff from attacking an attacking opponent is always greater than the payoff from displaying to an attacking opponent (tables 1 and 2).
Retreat–attack (R–a) and attack–retreat (A–r). Retreat–attack and attack–retreat can never be ESSs, since the cost of attacking a retreating opponent means that it is always better to display to it.
Retreat–display (R–w). The larger individual should choose to retreat (R) and the smaller individual should display (w) when inequalities (2.17) and (2.18) are satisfied.
Display–retreat (W–r). The smaller individual should choose to retreat (r) and the larger individual should display (W) when inequalities (2.11) and (2.12) are satisfied.
It is clear by inspection that W–w cannot exist in the same parameter space as W–r or R–w. It is also clear that W–w and A–a can exist as ESSs in the same parameter space, when the conditions for both are met. The parameter space where A–a is an ESS overlaps with that where W–r is an ESS when
which occurs when αV<β (when the additional benefits gained by attacking first are less than the costs associated with being attacked while retreating), and the conditions necessary for both are satisfied. A–a can also exist in the same parameter space as R–w when equations (2.17), (2.18) and (2.3) are all satisfied. R–w parameter space and W–r parameter space overlap when all the conditions for their occurrence are met (equations (2.11), (2.12), (2.17) and (2.18)). By inspection, given that p<0.5, the value of F needed to satisfy equation (2.18) is greater than the value needed to satisfy equation (2.12). Thus, W–r and R–w will overlap when the conditions for R–w are satisfied, but W–r will also exist in parameter space where R–w does not.
3. Results
A ‘Napoleon ESS’, where the model predicts that the smaller individual is more aggressive than the larger one, occurs where the smaller individual displays and the larger individual retreats (R–w). This occurs when the probability of finding an uncontested resource is high (larger values of F) and the probability that the smaller individual wins is low (small p). The Napoleon ESS always exists in the same parameter space as an alternative, mirror image ESS, where the larger individual displays and the smaller individual retreats (figure 1). This ESS is not particularly sensitive to the costs of fighting (cf; figure 2). The alternative ESS (W–r) also exists over a much wider range of the parameter space (figures 1 and 2), and is the predominant solution to the game when RHP accurately determines the outcome of fights (p=0).
Figure 1.
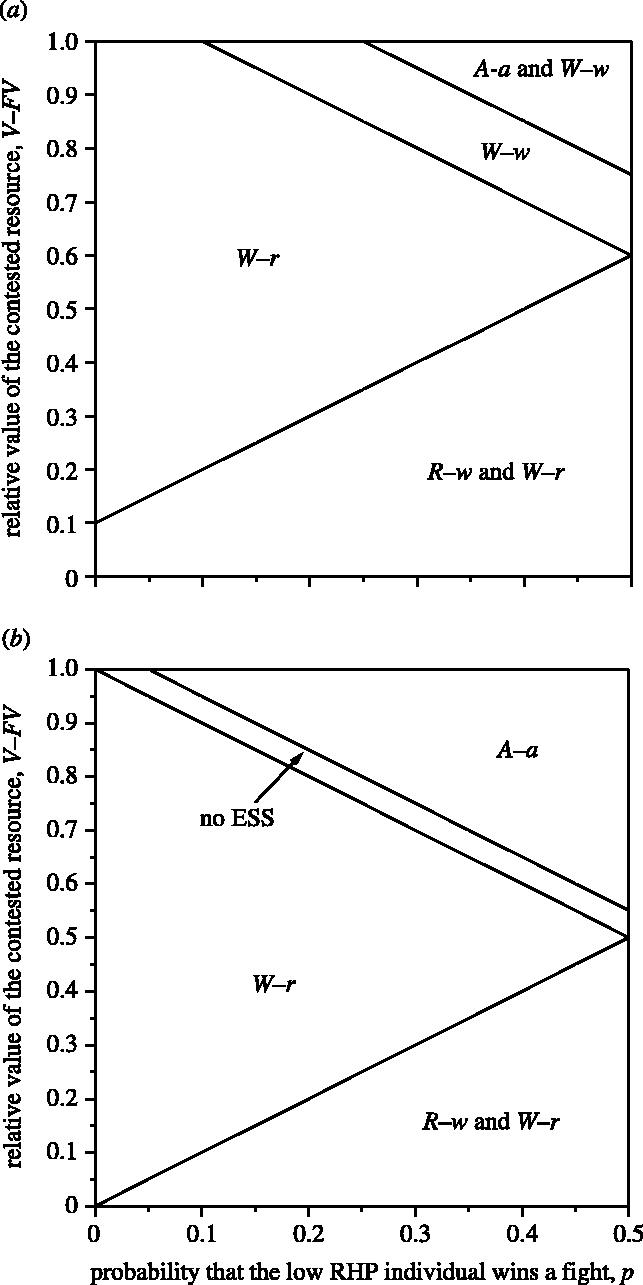
Effect of increasing the probability that the smaller individual wins the fight (p), and relative value of the contested resource (V−FV) on the ESS solutions of the game. Parameter values used: (a) α=0.1, cf=0.3, cd=0.1, β=0.05, V=1, (b) α=0.1, cf=0.1, cd=0.1, β=0.05, V=1.
Figure 2.
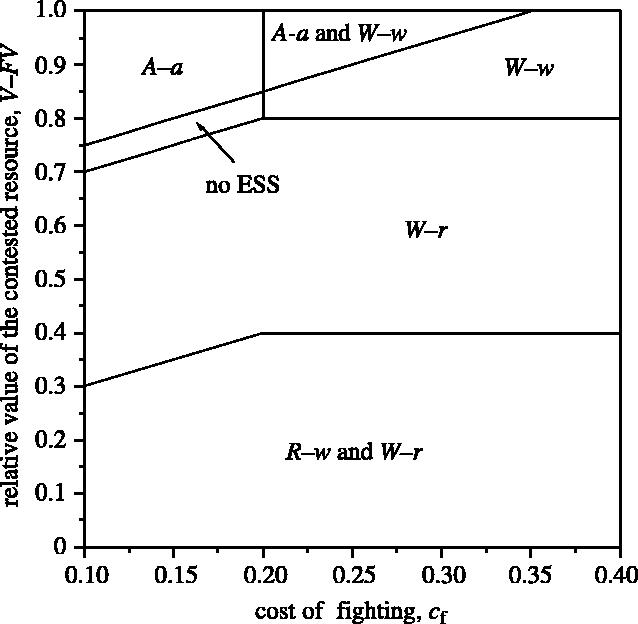
Effect of increasing the cost of fighting (cf) and the relative value of the contested resource (V−FV) on the ESS solutions of the game. Parameter values used: α=0.1, p=0.3, cd=0.1, β=0.05, V=1.
The model predicts equal levels of aggression by both individuals (A–a) when the probability that the smaller individual wins is high (values of p approaching 0.5) and the contested resource is of relatively low value (low V−FV, high values of F; figure 1). This also occurs when the costs associated with fighting are low (low cf; figure 2). This ESS can coexist with an ESS where both individuals choose to display while waiting for their opponent to attack (figure 1a). If, however, , that is, the advantage gained by initiating aggression exceeds the relative difference in the costs of the two fight types (fighting and displaying), the W–w ESS does not exist in the same parameter space as A–a (figure 1b). In this case, an area of parameter space exists where there is no ESS, when β<αV (when the cost of being attacked while retreating is less that the possible gains from attacking first).
4. Discussion
Both attacking (A, a) and displaying (W, w) can be considered to be aggressive behaviours. Displaying represents a low level of aggression, and attacking a high level, as it results in an escalated fight. Only retreating is considered to be a non-aggressive strategy in the current model. Thus, smaller individuals can be said to be aggressive when they select an attack (a) or display (w) strategy. Our model shows that Napoleon ESSs, where the Napoleon strategy is defined as meaning that smaller individuals are more aggressive than their larger opponents, are possible when smaller individuals display and larger ones retreat (R–w). This occurs when the smaller individual has some chance of winning a contest (values of p approaching 0.5), and resources are abundant and of relatively low value (large values of F, resulting in low values for V−FV). Thus, when resources are freely available and contests are not always resolved in the favour of the larger individual, it can benefit the larger individual to retreat without aggression. A possible example of this behaviour may occur in natural populations of snow buntings (Plectrophenax nivalis) where older males retreat in response to aggression over food resources from younger males (Smith & Metcalfe 1997). The Napoleon ESS exists in the same parameter space as an alternative, ‘common sense’, ESS, where smaller individuals retreat when challenged by a larger individual. When there are few alternative options, and the smaller individual has little chance of winning, this common sense solution becomes the only ESS.
The Napoleon ESS appears somewhat similar to the paradoxical solution to the hawk–dove game (Maynard Smith & Parker 1976; Maynard Smith 1982), in that the behaviour in question is seemingly counterintuitive. In the hawk–dove game, the paradoxical solution predicts aggressive territorial intruders and non-aggressive residents, resulting in intruders winning in territorial contests. The Napoleon ESS suggests smaller individuals should act more aggressively. However, in contrast to the Napoleon ESS, which occurs frequently in nature (see §1), paradoxical solutions to animal contests in the context of the hawk–dove game are extremely rare (see e.g. Morrell & Kokko 2005). The Napoleon ESS and its common sense opposite can exist in the same parameter space, with the common sense ESS existing over a wider range of parameters. We do not investigate here which of these is more likely to evolve from an ancestral population, but this represents an interesting avenue for future research. Although here we show that Napoleon behaviour can be explained on economic grounds, such an approach would illustrate whether the mechanisms for conflict initiation by smaller individuals proposed can account for the observations in natural systems.
Although it has been established that smaller individuals may act aggressively towards larger opponents, empirical work is yet to investigate the effect of body size or RHP asymmetries on the propensity of individuals to initiate aggression. However, where differences are large, disadvantaged individuals may perceive their relative inferiority and retreat without conflict (Enquist & Leimar 1983), predicting fights between similar-sized competitors (Jennions & Backwell 1996; Pratt et al. 2003). Thus, one might predict that aggression would be initiated by smaller competitors when the size asymmetry was not too large, as predicted in the current model for the Napoleon ESS.
Smaller individuals attack (a) if resources are scarce and consequently each is of high relative value (low values of F), they have a reasonable chance of winning the fight (p approaching 0.5) and the costs of fighting are low (low cf). Thus, aggression can be expected from smaller individuals when they have few alternative options, and when RHP (or body size) is not a perfect determinant of fight outcome. This reflects the findings of previous models, where aggression was favoured when individuals have similar RHP (Mesterton-Gibbons 1994), and when individuals gain more by fighting than they would by giving up (Hurd & Enquist 1998). Similarly, Eshel & Sansone (2001) predicted that high levels of aggression could occur in smaller individuals, particularly towards those not much larger than themselves (i.e. where they have some chance of winning in a fight), when resources are scarce.
In our model, high intensity aggression (both individuals choose an attacking strategy) is favoured when resources are scarce and valuable, and fights incur low costs relative to the value of the resource. These cases have both been noted previously. In the hawk–dove game (Maynard Smith & Price 1973; Maynard Smith & Parker 1976), low fight costs are more likely to promote aggression, and the desperado effect (Grafen 1987) predicts the undermining of conventional settlement of disputes when resources are scarce and one class of individuals is left with few or no alternative options. Our model suggests that under the conditions that favour aggression (i.e. low fighting costs and scarce, high value resources), smaller individuals are predicted to attack even when they can accurately assess their chances of winning in a fight, so long as body size is not a completely accurate predictor of fight outcome. Smaller individuals do not need to consider themselves to be likely to win in order to act aggressively, as proposed by Just & Morris (2003). Such an outcome has been observed, for instance, in the cichlid fish Aequidens rivulatus, where behavioural signals indicating which individual is likely to win appear to be perceived accurately, and yet escalated fighting is still observed (Maan et al. 2001).
We found no situations where the smaller individual chose an attacking strategy (a) and the larger individual chose to display (W). This occurs because the non-attacking individual can always improve its fitness by retaliating (see §2), as we assume that a fight always occurs if one competitor chooses to escalate. We assume that the costs of fighting are equal for both competitors, but extending the model to incorporate asymmetries in the costs between competitors or fight outcomes may provide a wider range of potential ESSs. For example, smaller individuals may pay higher costs of fighting (Maan et al. 2001), or losing may be more costly than winning (Neat et al. 1998), or display intensity may be an honest signal of RHP and thus more costly to a high RHP individual (Zahavi 1975, 1977). Although for simplicity we assumed that costs (cf, cd, β and γ) are identical for the two competitors, our results are not critically dependent on this assumption. If the cost of fighting for either individual was unilaterally increased, we would expect their, but not their opponent's, enthusiasm for aggression to decrease, leading to a greater area of parameter space where the individuals do not behave identically, but retaining areas of aggression by both.
We assume that the first individual to attack has an advantage, described by α. As this is additive, we implicitly assume that the mechanism providing this advantage is unrelated to RHP. For example, the advantage may be due to the element of surprise, and the use that can be made of that surprise (for example, landing a blow on an unsuspecting opponent) is independent of the qualities that govern victory in more symmetric contests (i.e. is independent of RHP, and equal for both competitors). However, there may be other biological situations where the advantage is more closely related to RHP, and in this case it would be more appropriate to assume a multiplicative rather than additive increment. The consequence for the model would be that the absolute benefits of attacking are greater for the larger individual, and we would therefore expect to see lower levels of aggression by the smaller individual. We would not, however, see a complete lack of aggression by smaller individuals, particularly when resources are scarce and valuable.
Studies of fighting behaviour tend to focus only on the interactions that have occurred, and do not report cases where one individual has retreated without a fight (e.g. Hu & Morse 2004). This makes it difficult to explain why escalated fights occur. However, escalated encounters have been reported when individuals are closely size matched (e.g. Ribowski & Franck 1993; Smith et al. 1994; Morris et al. 1995), suggesting that the smaller individual has some chance of winning in fights (high p, close to 0.5). Where there are large differences in body size, aggression often does not occur, and the smaller individual retreats from the larger (e.g. Smith et al. 1994), presumably because it assesses its chances of winning as being prohibitively low (small values of p).
As escalated fighting is observed much more frequently in experimental systems than in nature (Tinbergen 1968), it has been suggested that the empirical observation that smaller individuals can be aggressive may be an artefact of experimental design. In experiments on fighting, individuals are often placed in artificially symmetrical situations, for example, if residency is confused (Waage 1988), and both contestants consider themselves to own the resource. Escalated fighting occurs in these conditions many times more frequently than when only one of the individuals considers itself owner (reviewed in Kemp & Wiklund 2001). Individuals are also given few alternative options to obtain resources other than fighting. When losers of fights between A. rivulatus were able to withdraw from an experimental arena, conflicts were of shorter duration, and escalated fighting was absent, compared with when no alternative options were present (Maan et al. 2001), suggesting that the desperado effect (Grafen 1987) may be a frequent cause of aggression in experimental situations.
In general, there seems to be little evidence for desperado behaviour in natural populations. In red-winged blackbirds (Agelaius phoeniceus; Shutler & Weatherhead 1992), and Anolis aeneus lizards (Stamps & Krishnan 1995), the costs of fighting to take over a territory appear to be higher than the costs of waiting for a vacancy, and aggressive territorial takeovers rarely occur. Additionally, naturally occurring fights, where individuals are free to choose opponents, tend to be size assortative: individuals avoid contests with opponents who differ greatly in size (Jennions & Backwell 1996; Pratt et al. 2003), but retreating from an opponent occurs relatively rarely in experiments (Smith et al. 1994; Hu & Morse 2004). The desperado effect therefore needs to be ruled out as a cause of aggression in experimental systems before the evolution of aggressive behaviour can be understood (Maan et al. 2001).
Acknowledgments
We would like to thank Stuart Humphries for useful discussions, and Dan Haydon, Neil Metcalfe, Rufus Johnstone and two anonymous referees for insightful comments on the manuscript. L.J.M. was funded by the Natural Environment Research Council.
Footnotes
As this paper exceeds the maximum length normally permitted, the authors have agreed to contribute to production costs.
References
- Beaugrand J.P, Payette D, Goulet C. Conflict outcome in male green swordtail fish dyads (Xiphophorus helleri): interaction of body size, prior dominance/subordination experience, and prior residency. Behaviour. 1996;133:303–319. [Google Scholar]
- Brace R.C, Pavey J. Size-dependent dominance hierarchy in the anemone Actinia equine. Nature. 1978;273:752–753. [Google Scholar]
- Bradbury J.W, Vehrencamp S.L. Sinauer; Sunderland, MA: 1998. Principles of animal communication. [Google Scholar]
- Brick O. A test of the sequential assessment game: the effect of increased cost of sampling. Behav. Ecol. 1999;10:726–732. [Google Scholar]
- Crowley P.H. Hawks, doves, and mixed-symmetry games. J. Theor. Biol. 2000;204:543–563. doi: 10.1006/jtbi.2000.2037. [DOI] [PubMed] [Google Scholar]
- Davies N.B, Houston A.I. Owners and satellites: the economics of territory defence in the pied wagtail, Motacilla alba. J. Anim. Ecol. 1981;50:157–180. [Google Scholar]
- Dow M, Ewing A.W, Sutherland I. Studies on the behavior of cyprinodont fish III. The temporal patterning of aggression in Aphyosemion striatum (Boulenger) Behaviour. 1976;59:252–268. doi: 10.1163/156853976x00398. [DOI] [PubMed] [Google Scholar]
- Dowds B.M, Elwood R.W. Shell wars 2: the influence of relative size on decisions made during hermit crab shell fights. Anim. Behav. 1985;33:649–656. [Google Scholar]
- Dugatkin L.A, Ohlsen S.R. Contrasting asymmetries in value expectation and resource holding power—effects on attack behaviour and dominance in the pumpkinseed sunfish, Lepomis gibbosus. Anim. Behav. 1990;39:802–804. [Google Scholar]
- Enquist M, Jakobsson S. Assessment of fighting ability in the cichlid fish Nannacara anomala. Ethology. 1986;72:143–153. [Google Scholar]
- Enquist M, Leimar O. Evolution of fighting behaviour, decision rules and assessment of relative strength. J. Theor. Biol. 1983;102:387–410. [Google Scholar]
- Eshel I, Sansone E. Multiple asymmetry and concord resolutions of a conflict. J. Theor. Biol. 2001;213:209–222. doi: 10.1006/jtbi.2001.2412. [DOI] [PubMed] [Google Scholar]
- Grafen A. The logic of divisively asymmetric contests—respect for ownership and the desperado effect. Anim. Behav. 1987;35:462–467. [Google Scholar]
- Hack M.A. The energetic costs of fighting in the house cricket, Acheta domesticus L. Behav. Ecol. 1997;8:28–36. [Google Scholar]
- Hu H.H, Morse D.H. The effect of age on encounters between male crab spiders. Behav. Ecol. 2004;15:883–888. [Google Scholar]
- Huntingford F.A, Turner A.K. Chapman & Hall; London: 1987. Animal conflict. [Google Scholar]
- Hurd P.L, Enquist M. Conventional signalling in aggressive interactions: the importance of temporal structure. J. Theor. Biol. 1998;192:197–211. [Google Scholar]
- Jackson W.M. Why do winners keep winning? Behav. Ecol. Sociobiol. 1991;28:271–276. [Google Scholar]
- Jennions M.D, Backwell P.R.Y. Residency and size affect fight duration and outcome in the fiddler crab Uca annulipes. Biol. J. Linn. Soc. 1996;57:293–306. [Google Scholar]
- Johnsson J.I, Forser A. Residence duration influences the outcome of territorial conflicts in brown trout (Salmo trutta) Behav. Ecol. Sociobiol. 2002;51:282–286. [Google Scholar]
- Just W, Morris M.R. The Napoleon complex: why smaller males pick fights. Evol. Ecol. 2003;17:509–522. [Google Scholar]
- Keeley E.R, Grant J.W.A. Asymmetries in the expected value of food do not predict the outcome of contests between convict cichlids. Anim. Behav. 1993;45:1035–1037. [Google Scholar]
- Kemp D.J, Wiklund C. Fighting without weaponry: a review of male–male contest competition in butterflies. Behav. Ecol. Sociobiol. 2001;49:429–442. [Google Scholar]
- Leimar O, Enquist M. The effect of asymmetries in owner–intruder conflicts. J. Theor. Biol. 1984;111:475–491. [Google Scholar]
- Lindström K. The effect of resource holding potential, nest size and information about resource quality in the outcome of intruder–owner conflicts in the sand goby. Behav. Ecol. Sociobiol. 1992;30:53–58. [Google Scholar]
- Maan M.E, Groothuis T.G.G, Wittenberg J. Escalated fighting despite predictors of conflict outcome: solving the paradox in a South American cichlid fish. Anim. Behav. 2001;62:623–634. [Google Scholar]
- Marden J.H, Waage J.K. Escalated damselfly territorial contests are energetic wars of attrition. Anim. Behav. 1990;39:954–959. [Google Scholar]
- Maynard Smith J. Cambridge University Press; Cambridge: 1982. Evolution and the theory of games. [Google Scholar]
- Maynard Smith J, Parker G.A. The logic of asymmetric contests. Anim. Behav. 1976;24:159–175. [Google Scholar]
- Maynard Smith J, Price G.R. The logic of animal conflict. Nature. 1973;246:15–18. [Google Scholar]
- Mesterton-Gibbons M. The hawk–dove game revisited—effects of continuous variation in resource-holding potential on the frequency of escalation. Evol. Ecol. 1994;8:230–247. [Google Scholar]
- Moretz J.A. Aggression and RHP in the northern swordtail fish, Xiphophorus cortezi: the relationship between size and contest dynamics in male–male competition. Ethology. 2003;109:995–1008. [Google Scholar]
- Morrell L.J, Kokko H. Bridging the gap between mechanistic and adaptive explanations of territory formation. Behav. Ecol. Sociobiol. 2005;57:381–390. [Google Scholar]
- Morris M.R, Gass L, Ryan M.J. Assessment and individual recognition of opponents in the pygmy swordtails Xiphophorus nigrensis and Xiphophorus multilineatus. Behav. Ecol. Sociobiol. 1995;37:303–310. [Google Scholar]
- Neat F.C, Taylor A.C, Huntingford F.A. Proximate costs of fighting in male cichlid fish: the role of injuries and energy metabolism. Anim. Behav. 1998;55:875–882. doi: 10.1006/anbe.1997.0668. [DOI] [PubMed] [Google Scholar]
- Parker G.A. Assessment strategy and the evolution of fighting behaviour. J. Theor. Biol. 1974;47:223–243. doi: 10.1016/0022-5193(74)90111-8. [DOI] [PubMed] [Google Scholar]
- Petersen G, Hardy I.C.W. The importance of being larger: parasitoid intruder–owner contests and their implications for clutch size. Anim. Behav. 1996;51:1363–1373. [Google Scholar]
- Pratt A.E, McLain D.K, Lathrop G.R. The assessment game in sand fiddler crab contests for breeding burrows. Anim. Behav. 2003;65:945–955. [Google Scholar]
- Ribowski A, Franck D. Demonstration of strength and concealment of weakness in escalating fights of male swordtails (Xiphophorus helleri) Ethology. 1993;93:265–274. [Google Scholar]
- Selten R. A note of evolutionary stable strategies in animal conflicts. J. Theor. Biol. 1980;84:93–101. doi: 10.1016/s0022-5193(80)81038-1. [DOI] [PubMed] [Google Scholar]
- Shutler D, Weatherhead P.J. Surplus territory contenders in male red-winged blackbirds: where are the desperados? Behav. Ecol. Sociobiol. 1992;31:97–106. [Google Scholar]
- Smith I.P, Huntingford F.A, Atkinson R.J.A, Taylor A.C. Strategic decisions during agnostic behaviour in the velvet swimming crab, Necora puber (L.) Anim. Behav. 1994;47:885–894. [Google Scholar]
- Smith R.D, Metcalfe N.B. Why does dominance decline with age in wintering snow buntings? Anim. Behav. 1997;53:313–322. [Google Scholar]
- Stamps J.A, Krishnan V.V. Territory acquisition in lizards. 3. Competing for space. Anim. Behav. 1995;49:679–693. doi: 10.1006/anbe.1997.0612. [DOI] [PubMed] [Google Scholar]
- Taylor P.W, Hasson O, Clark D.L. Initiation and resolution of jumping spider contests: roles for size, proximity, and early detection of rivals. Behav. Ecol. Sociobiol. 2001;50:403–413. [Google Scholar]
- Tinbergen N. On war and peace in animals and man. Science. 1968;160:1411–1418. doi: 10.1126/science.160.3835.1411. [DOI] [PubMed] [Google Scholar]
- Turner G.F, Huntingford F.A. A problem for game theory analysis: assessment and intention in male mouthbrooder contests. Anim. Behav. 1986;34:961–970. [Google Scholar]
- Waage J.K. Confusion over residency and the escalation of damselfly territorial disputes. Anim. Behav. 1988;36:586–595. [Google Scholar]
- Zack S. A description and analysis of agonistic behaviour patterns in an opisthobranch mollusc, Hermissenda crassicornis. Behaviour. 1975;5:238–267. doi: 10.1163/156853975x00218. [DOI] [PubMed] [Google Scholar]
- Zahavi A. Mate selection: a selection for a handicap. J. Theor. Biol. 1975;53:205–214. doi: 10.1016/0022-5193(75)90111-3. [DOI] [PubMed] [Google Scholar]
- Zahavi A. The cost of honesty (further remarks on the handicap principle) J. Theor. Biol. 1977;67:603–605. doi: 10.1016/0022-5193(77)90061-3. [DOI] [PubMed] [Google Scholar]


