Self-similar patterns of nature: insect diversity at local to global scales

Abstract

The insects are probably the most hyperdiverse and economically important metazoans on the planet, but there is no consensus on the best way to model the dimensions of their diversity at multiple spatial scales, and the huge amount of information involved hinders data synthesis and the revelation of ‘patterns of nature’. Using a sample of more than 600k insect species in the size range 1–100 mm, we analysed insect body sizes and revealed self-similar patterns persisting across spatial scales from several hectares to the World. The same patterns were found in both Northern and Southern Hemispheres. The patterns include: parallel rank-abundance distributions; flatter species–area curves in smaller insects—indicating their wider geographical distribution; the recurrence of the same species-rich family in the same body-size class at all spatial scales—which generates self-similar size-frequency distributions (SFDs)—and the discovery that with decreasing mean body size, local species richness represents an increasing fraction of global species richness. We describe how these ‘rationalizing’ patterns can be translated into methods for monitoring and predicting species diversity and community structure at all spatial scales.

1. Introduction

The task of quantifying insect diversity is frustrated by great uncertainties (Stork 1997). One of the best estimates for the total number of named, living insect species is 720k (May 2000) but for the total number of extant species, a ‘best guess’ is 4 million (May 2000), whereas others (Gaston 1992; Hammond 1995; Godfray et al. 1999) prefer the higher range of 5–10 million species. Estimates up to 30–50 million species (Erwin 1982) are now generally considered to be too high. While the global species richness of insects remains an elusive number, a further layer of confusion is introduced by indications that the global extinction crisis may be spreading to the insects, with evidence at the national scale of regional extinctions in butterflies (Thomas et al. 2004), a major decline in British moth species over the past 35 years (Conrad et al. 2004), and the belief (Samways 2005) that a quarter of all species may become extinct in the next few decades. These changes are widely interpreted as indicators that the natural world is approaching the ‘sixth major extinction’ event in Earth's history (Thomas et al. 2004). If true, the need for ‘fast tracking’ the synthesis of global insect diversity becomes abundantly clear. The purpose of this contribution is to illustrate how simple techniques of data handling can reveal distinctive self-similar and other natural patterns. These can then be subjected to extrapolation and interpolation to estimate community structures and patterns of species richness at different spatial scales.

2. Material and methods

The core species inventory was compiled from McGavin (1992) and to a lesser extent from Arnett (2000). It is a list of 176 insect families including all those that are species-rich, plus the number of species in each family in the UK, North America and the World. Wasp families in the Division Parasitica were excluded. The list of families in McGavin's dataset is in table S1 of the electronic supplementary material. The dataset represents a large proportion of the recorded global species richness of insects. Our sample of ‘World’ has 601 958 species, which is roughly 80% of May's (2000) global estimate (720k) of the ‘number of named and distinct species’. We also used two other datasets—that of Hilbre, a 4.7 ha island in the estuary of the River Dee close to the Wirral (UK), where much recording of insects has been carried out (e.g. Craggs 1982); and at the Monks Wood National Nature Reserve (153 ha) in Huntingdonshire, UK (Steele & Welch 1973). The only difficulty with the latter data is that two groups of small insects (Aphididae and Thripidae) were not included in the recording of species (hence the missing data in figure 2). We compiled a large sample inventory of insects in Australia from various sources (CSIRO 1994; Zborowski & Storey 2003; Gullan & Cranston 2004), consisting of 60 911 species in 350 families. All the analyses were restricted to insect families with geometric mean sizes in the 1–100 mm body length range (or wingspan size range in the Lepidoptera), and the range was divided into 16 logarithmic (base 10) size classes to reveal ‘peak–trough–hillside’ patterns in the size–frequency distributions (SFDs). Families were allocated to size classes based on body sizes determined from McGavin (1992).

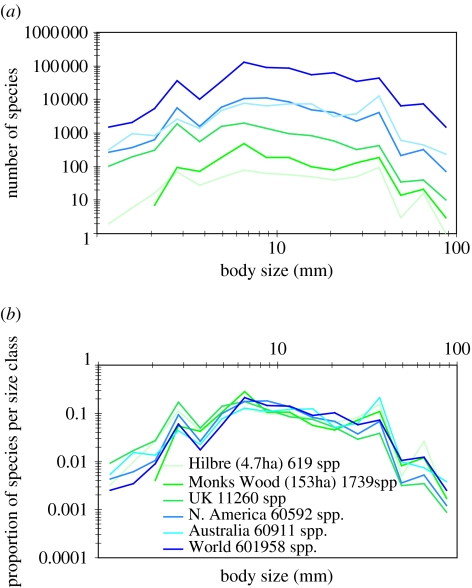
Figure 2.

(a) Size–frequency distributions (SFDs) of insects at six spatial scales, where the insect ‘body length’ axis is divided into 16 logarithmic (base 10) size classes and yields at least four regions of coincident peaks at all spatial scales. Note that North America and Australia are similar in both geographical area and species richness (America: 60 592 spp.; Australia: 60 911 spp.). (b) When the SFD data are expressed on a proportionate basis, the six curves lose their individual identities and become superimposed upon each other.

There is a potential problem with this type of analysis if taxonomic sampling bias is significant. Barlow (1994) suggested that some butterfly families become smaller with increasing latitude, while others are unaffected. May (1978) showed that the mean sizes of insects are generally larger in the tropics, but this did not appear to hold for the Coleoptera or Lepidoptera, and he concluded that systematic differences in body size between tropical and temperate insects may not exist. Hawkins & Lawton (1995) searched for latitudinal gradients in butterfly body sizes and found gradients that were inconsistent. The picture becomes even more unclear because of the systematic tendency for longer insects to be thinner—a tendency that is more pronounced in the tropics (Schoener 1980). In the absence of a convincing and widespread correlation between insect body size and latitude, we decided that the size classes we erected were broad enough to hold groups of families whose individual species are derived from a range of latitudes. The significance of sampling bias could be tested using butterflies—the best known insect taxon, containing six families whose species encompass half the size classes used in our analyses—to compare differences between tropical and temperate fauna. This is easier suggested than done, but here we make the following brief points: (i) even for butterflies, species' lists in tropical countries are growing sufficiently rapidly for comparisons with temperate faunas to be subjected to the same putative bias raised elsewhere. (ii) In the few regions where accumulation curves suggest that butterfly faunas are fully described, the widely held assumption that sampling bias occurs since larger insects are better known than small ones is supported only weakly or not at all. This, we believe, is because a counter trend exists, resulting from a tendency for small insects to be more widespread and for widespread species to be discovered before local ones (Thomas & Clarke 2004). We conclude that the current incomplete global list of insects is unlikely to contain sufficient bias towards larger bodied species to obviate the other patterns we describe, although we acknowledge that other undetected biases may exist, a problem that afflicts all analyses of such datasets.

3. Results

(a) Rank abundance distributions of species per family

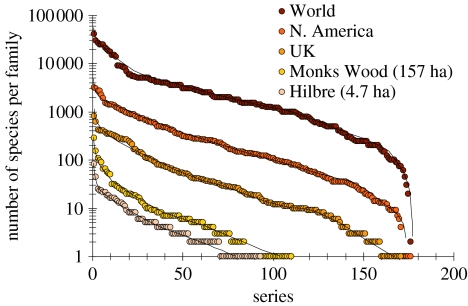
Figure 1 illustrates a simple synthesis of the data, with rank-abundance distributions plotted for five spatial scales ranging from a few hectares (Hilbre) to the World. The first datapoint in the ‘World’ curve represents the 41 000 species in the family Curculionidae, and the final point—number 176 (the Pediculidae)—marks the two species known worldwide.

Figure 1.

The species richness of insects in 176 families including all species-rich families, at five spatial scales from 4.7 ha to the World, drawn as rank-abundance distributions.

All the distributions have similar shapes, at least in the intermediate rank regions of slow decline, suggesting that insects may share the same or similar patterns of decreasing species richness per family, irrespective of spatial scale. There are some minor differences, as in the initial steeper rate of decline for Hilbre and Monks Wood, which is consistent with the repeatedly reported (e.g. May 1975) observation that as species richness decreases, the distribution of relative species abundance becomes steeper. In contrast, the upper three distributions (UK, North America and World) can be almost perfectly superimposed upon each other when expressed on a proportionate basis (figure not shown).

To investigate this further, we compared the observed probability distribution function of species per family at sub-World scales with those expected by taking a random sub-sample of the same total number of species from the World dataset. The expected probability distribution function was based on averaging the distributions of 1000 random sub-samples. The maximum difference between observed and expected cumulative distribution functions was less than 0.09 in all comparisons except for UK versus World (figure S1 of the electronic supplementary material). This analysis suggests that the similar distributions of species per family at different spatial scales can be explained solely as the statistical outcome of random sampling from the species pool—which is to say that differences in rank-abundance curves probably do not require any biological interpretation.

(b) Size–frequency distributions

The orders of insects yield SFDs that tend to be unimodal, and peak at an intermediate body size for the order (Siemann et al. 1996). This is believed to be the result of diversification around some ancestral body-size range, which represents an adaptive peak in phenotype. But, if we increase resolution by dropping down to the taxonomic level of family, we find that the prediction of a unimodal SFD is confounded by four size classes where distinct peaks represent elevated species richness (figure 2). These peaks are coincident in specific size classes, at all spatial scales. The first is in the size class 2.4–3.2 mm, with the other size classes peaking at 5.6–7.5, 32–42 and 75–100 mm. Self-similarity becomes apparent when the y-axis is converted to ‘proportion of species per size class’ and the six SFDs become virtually superimposed upon each other.

Given the number of peaks and troughs within each SFD, the extent to which they co-occur in specific size classes at all spatial scales is unlikely due to chance alone. Specifically, exact chi-square tests of association of ‘peaks, troughs and hillsides’ were found to be statistically significant for all pairwise combinations of spatial scales (all p values<0.035; StatExact v. 4 for Windows, 1998; table S2 of the electronic supplementary material).

These patterns raise two questions. First—which species-rich families are responsible for the peaks? The first peak is dominated by Scolytidae (9k species globally) and Chironomidae (5k); the second by a group of three species-rich families—Curculionidae (41k), Staphylinidae (27k) and Chrysomelidae (30k); the third by Noctuidae (25k); and the fourth by Nymphalidae (3.5k). As for the second question—whether a single family is responsible for coincident peaks at all spatial scales—it is clearly demonstrated that either one family, or a group of up to three families dominate species richness in specific size classes, and they do so at all spatial scales (table 1). The Noctuidae, e.g. are the most species-rich family in the 32–42 mm size class at all geographical scales from the 5 ha island (Hilbre) to the World. This phenomenon is also revealed by a number of other families, e.g. Carabidae (10–13 mm) and Geometridae (24–32 mm).

Table 1.

The dominant (i.e. most species-rich) family or families in size classes across six regions. Where several families are grouped, they are similarly species rich. Note the two families (Sphecidae and Oecophoridae) that are species-rich only in Australia, these are shown in bold.

size class (mm)	Hilbre	Monks Wood	United Kingdom	North America	Australia	World
4.2–5.6	Muscidae	Muscidae	Muscidae	Muscidae	Miridae	Muscidae
Miridae	Miridae	Miridae	Miridae	Sphecidae	Miridae
5.6–7.5	Staphylinidae	Staphylinidae	Staphylinidae	Staphylinidae	Curculionidae	Curculionidae
Curculionidae	Curculionidae	Curculionidae	Curculionidae	Staphylinidae	Chrysomelidae
Chrysomelidae	Chrysomelidae	Chrysomelidae	Chrysomelidae	Chrysomelidae	Staphylinidae
7.5–10	Cicadellidae	Cicadellidae	Cicadellidae	Cicadellidae	Cicadellidae	Cicadellidae
10–13	Carabidae	Carabidae	Carabidae	Carabidae	Carabidae	Carabidae
13–18	Tortricidae	Tortricidae	Tortricidae	Scarabaeidae	Scarabaeidae	Scarabaeidae
			Tortricidae	Tortricidae
18–24	Pyralidae	Pyralidae	Tipulidae	Tipulidae	Cerambycidae	Cerambycidae
Tipulidae	Cerambycidae	Pyralidae	Pyralidae	Tipulidae	Pyralidae
Cerambycidae	Tipulidae	Cerambycidae	Cerambycidae	Pyralidae	Tipulidae
24–32	Geometridae	Geometridae	Geometridae	Geometridae	Geometridae	Geometridae
32–42	Noctuidae	Noctuidae	Noctuidae	Noctuidae	Noctuidae	Noctuidae
				Oecophoridae

It is fortuitous that the continental areas of North America and Australia have similar geographical areas, and that their species inventories are similarly large (approx. 61k species in each region). This permits sensible comparison of large species inventories separated by almost maximum possible geographic distance. The SFDs for North America and Australia are fundamentally similar, notwithstanding the elevated peak in species richness in the Australian SFD at a body length of around 30 mm. This is due to a large number of moth species, mainly oecophorids. The evolutionary radiation of Oecophoridae in Australia has apparently developed in parallel with evolutionary diversification in the genus Eucalyptus (more than 700 species) on which oecophorids are largely dependent, resulting in a very large number of species. Unfortunately, estimates of species richness of Oecophoridae in Australia range from 2000 to 5500, with many endemic species, so in figure 2 we have used the conservative figure of 2500 species.

Although there is only a limited amount of useful data from the Southern Hemisphere, it appears that similar SFD patterns do persist in both northern and southern hemispheres—even when challenged by vast numbers of biologically distinct or endemic species. Comparison of the number of species in each size class in North America, with the equivalent size classes for Australia, indicates that they are significantly correlated (R²=0.84).

(c) Absolute abundance, body size and dispersal

The abundance of individuals in any species-population tends to be inversely proportional to individual body size (Damuth 1981; Schmid et al. 2000; Finlay 2002; Fenchel & Finlay 2004). This is true for the insects also (Morse et al. 1985), insofar as species of thrips and aphids have the potential to produce vast populations, in contrast to much larger species. Neutral models (e.g. Hubbell 2001) predict that the probability of dispersal is proportional to absolute population size, implying that smaller species—those with greater absolute abundance—are more widely dispersed. We obtained evidence for this, insofar as increasing absolute dispersal rate is reflected in higher local : global species ratios.

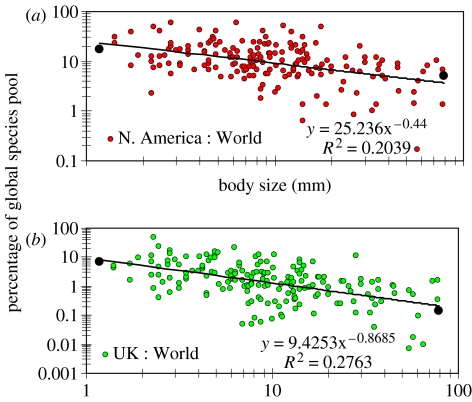
Each part of figure 3 has 176 datapoints—each one representing a family. Each datapoint also represents a ratio—of species richness in a particular family in North America, to species richness in the same family at the global scale (figure 3a). In the same way, figure 3b illustrates the UK : World ratio per family. In each part of figure 3 are two black symbols close to the extremes of body-size range. The large symbol on the right represents a family of large-bodied insects—the Papilionidae. There is one recorded species in the UK and 700 species in the World, so the plotted species ratio (UK : World) is 0.14%. The enlarged symbol at the small-body-size end of the distribution represents the Thripidae. With 105 species in the UK and 1500 species worldwide, Thripidae in the UK represent 7% of Thripidae species worldwide. If we consider a bigger area—North America, we find that Thripidae there represent 18% of the global species pool. In both cases (figure 3a,b) we observe a trend which, we suggest, indicates greater dispersal in small insects, driven by their larger absolute abundances. With decreasing mean body size, local species richness represents an increasing fraction of global species richness. Exactly the same phenomenon has recently been documented for a wide size range of aquatic organisms, ranging from microscopic chrysomonads (where local species richness may represent more than 80% of global species richness) to fishes (Hillebrand & Azovsky 2001; Finlay & Fenchel 2004).

Figure 3.

With decreasing mean body size, local species richness (e.g. UK or North America) represents an increasing fraction of global species richness. Each symbol represents one insect family. The black symbols represent the example data for Papilionidae and Thripidae referred to in the text. (a) North America : World, y=25.24x^−0.44; R²=0.204. (b) UK : World, y=9.425x^−0.869; R²=0.276.

Alternative explanations include the possibility that smaller insects have a greater capacity for passive dispersal because of transport in air currents, but this explanation probably excludes a large proportion of the insects, e.g. those that are soil-dwelling. In addition, the same problem will apply to any other subjectively selected dispersal mechanism. The patterns in figure 3 could also be artefacts—e.g. small insects may be more thoroughly surveyed and described in the UK and North America than in the rest of the World. This would elevate the UK : World and North America : World ratios in the size range of small insects and produce a kink in the distributions, but the evidence does not support this, for we find seamless linear trends across the whole size range. However, we cannot deny that biased insect data exist, and this will remain so long as the global inventory remains incomplete—a problem that is shared, to a greater or lesser extent, by all studies of macro-ecological patterns.

The size dependence of local : global species ratios seems to exist independently of the taxonomic identity of the organisms concerned, suggesting that the common factor underlying all patterns is body size. Body size is inversely correlated with population size, which, we suggest, determines the absolute dispersal rate (Finlay 2002; Fenchel & Finlay 2004); and evidence supporting the idea that insect abundance is inversely related to body size over three orders of magnitude has been published (Morse et al. 1985). Therefore, to some extent, a neutral model of community structure (Bell 2001; Hubbell 2001) can probably be supported; dispersal can be modelled by a random walk, and species that are abundant or rare in one area tend to be similarly abundant or rare in other parts.

(d) Species-area curves

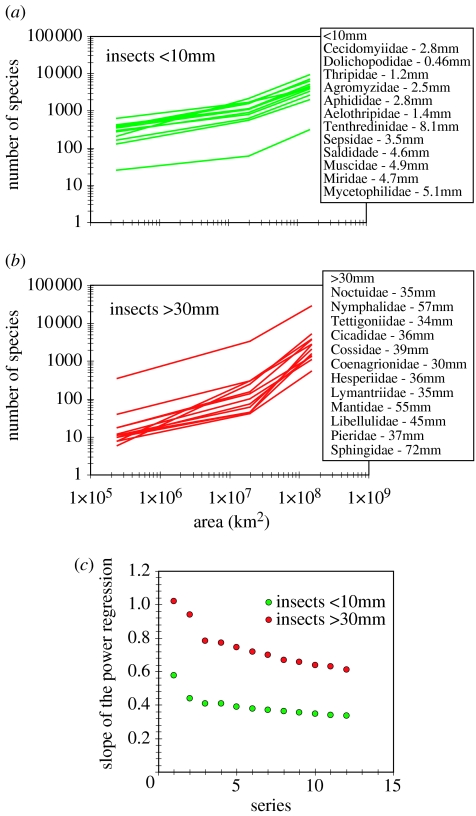
Within any taxonomic group living in a specific area, a species-area curve can usually be described by the power function S∝A^z, where S is number of species and A is area. However, when we plot species richness at progressively larger geographical areas up to the global scale, we incorporate additional and qualitatively novel biogeographic zones, and the species-area curve bends sharply upwards as it embraces, e.g. the insects that are confined to tropical areas. Figure 4 shows species-area curves for 12 families with mean body sizes greater than 30 mm, and 12 families less than 10 mm. The curves for all insects greater than 30 mm share a similar pattern, they are approximately parallel, and they fulfil the prediction of simultaneously bending upwards with increasing area in the order UK–North America–World. In contrast, insects less than 10 mm all share a pattern of flatter species-area curves, indicating wider geographical distribution, with the implication that some small species occur in both UK and North America and some also occur elsewhere. The two sets of species-area curves are distinctly different, as demonstrated by the large divergence in the slopes of the power regressions (figure 4).

Figure 4.

Species–area curves for large and small insects. (a) Insects <10 mm, (b) insects >30 mm; (c) the large divergence in slopes of the regressions.

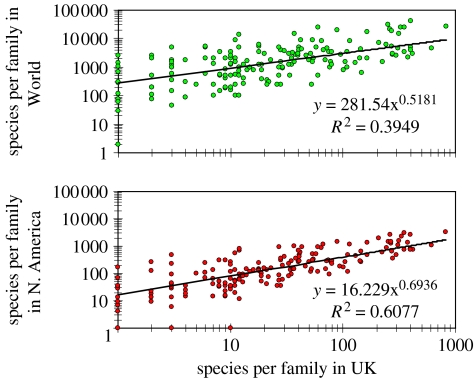
(e) Correlated local and global species richness

Finally, within any insect family, local and global species richness tend to be correlated (figure 5) because of the ‘stream of migrants’ (Bell 2001). For example, families that are species poor in the UK also tend to be species poor in North America and the World; and families that are species rich in the UK tend to be species-rich globally.

Figure 5.

Families that are species poor tend to be species poor everywhere, and those that are species rich tend to be species rich everywhere.

4. Discussion

We have shown that the global diversity of insects is supported by a framework of self-similar patterns that emerge with some force, are relevant in both Northern and Southern Hemispheres, and across spatial scales from a few hectares to global. All of these suggest that some fundamental characteristics of dispersal, speciation and evolutionary divergence are common to most, if not all insects. Further, we suggest that extrapolation and interpolation of macroecological patterns may provide a practical advance towards understanding and quantifying the dimensions of insect diversity in other regions or geographical scales (Blackburn & Gaston 2002), notwithstanding the existence of regionally restricted phenomena such as the oecophorid radiation in Australia and the small number of species inventories tested to date. We are aware of the likelihood that stochastic events and environmental drivers may destabilize or change SFD patterns, especially at the smaller spatial scales, and where ecosystems are homogeneous, barren or degraded. However, it is precisely these responses that could be developed into tools for monitoring change over time in insect communities. Our discoveries may contribute to the following areas of insect conservation.

Among the most fundamental questions in conservation are: how many species exist, what are their rates of decline and what is driving the observed declines. For insects—the most diverse metazoans—the revealed patterns support some assumed relationships that underpin the two most widely accepted approaches to estimate global species richness (Ødegaard 2000). Figure 2 confirms the existence and describes the shape of the presumed curve spanning the body-size range where the positive correlation between species richness and body size described in small organisms (Finlay & Fenchel 2004) switches to the inverse relationship for larger organisms (May 1988), within a transition zone where body length is around 7 mm. Clearly, increased precision in describing the area under this curve increases the accuracy of the approach. Independently of this, our identification of the same dominant (species-rich) family in a particular body-size class at all spatial scales (table 1) lends increased confidence to the many estimates of insect species richness based on extrapolation from subsets of the World's fauna. This has potential for extrapolating to other regional scales, but it remains to be seen if the pattern breaks down in particular biogeographic regions, rare habitat types, or in oceanic islands separated by great distance from continental land masses.

Recent analyses showing that extinction rates in UK butterflies have been similar to those of other insects (Thomas & Clarke 2004) and greater than those of birds and plants in recent decades, have been extrapolated to suggest that similar patterns, including the anthropogenic drivers of change (Parmesan et al. 1999; Warren et al. 2001), might apply worldwide (Thomas et al. 2004). This huge extrapolation, and others based on a few reliable databases describing regional changes in well-studied taxa (e.g. Ceballos & Ehrlich 2002), are strengthened considerably by our demonstration of self-similar SFDs.

(a) Practical applications

The degree of vertical displacement of self-similar SFDs in figure 2 is a function of area sampled, and this facilitates interpolation to further SFDs for defined areas. For example, although an agreed insect inventory for Europe does not exist, it can reasonably be predicted that the SFD for Europe will be approximately parallel to the UK distribution, but displaced slightly upwards. Similarly, the nested series of self-similar SFDs occurring in the sequence: UK, Monks Wood, Hilbre, indicates that it is feasible to interpolate to other natural areas, such as small nature reserves of a few hectares or less, and this can be tested by building new local inventories.

We may also extrapolate with confidence outside of the nested series of datasets because the non-nested SFDs for North America, UK and Australia are qualitatively similar, although further work is required to test whether isolated islands of relatively recent origin, e.g. display similar or distorted patterns. If the patterns are self-similar, it should be possible to safely predict patterns of species richness for specific areas within continental areas.

At the local level—e.g. a water meadow, nature reserve or farmland ecosystem, the SFD approach could be used to monitor change over time, while simultaneously continuing to monitor the butterflies and other ‘flagship’ insects that are already responding to global climate change in the northern hemisphere, with migration to higher latitudes and altitudes (Parmesan et al. 1999; Samways 2005). Thus, the diversity of insects—encapsulated within the ‘peaks and troughs’ of a SFD—could potentially provide an indicator of ‘ecosystem health’ that augments more specific approaches such as monitoring the abundance and spatial distribution of butterfly species (Thomas 2005). The low resolution self-similar plots in figure 2b will of course provide more useful detail by simply increasing the number of size classes.

We have discovered the recurrence of the same species-rich family (or families) in the same body-size class at all spatial scales, and it is this natural phenomenon which generates the self-similar SFDs. The patterns appear robust, but further rigorous testing is needed, as well as new sampling from a variety of ecosystem types worldwide. Datasets do not need to be very large to produce recognizable and reproducible patterns—the data for Hilbre consists of only 619 species; that for Monks Wood contains 1739 species. A cooperative venture involving partners worldwide, building new local species inventories while also resurrecting the older, half-forgotten inventories, would surely make giant strides towards revealing, testing and exploring more fully, the significance of these ‘patterns of nature’.

Finally, a further challenge will be to determine whether self-similar patterns lie hidden within other species-rich animal taxa. We suspect they do, and when they are revealed, they too will provide useful tools for characterizing and monitoring biodiversity (Nee & Lawton 1996) across spatial scales.

Supplementary Material

Click here for additional data file