Abstract
Based on 69 scanned Chinese male subjects and 25 Caucasian male subjects, the present study showed that the volume height index (VHI) is the most important visual cue to male body attractiveness of young Chinese viewers among the many body parameters examined in the study. VHI alone can explain ca. 73% of the variance of male body attractiveness ratings. The effect of VHI can be fitted with two half bell-shaped exponential curves with an optimal VHI at 17.6 lm−2 and 18.0 lm−2 for female raters and male raters, respectively. In addition to VHI, other body parameters or ratios can have small, but significant effects on male body attractiveness. Body proportions associated with fitness will enhance male body attractiveness. It was also found that there is an optimal waist-to-hip ratio (WHR) at 0.8 and deviations from this optimal WHR reduce male body attractiveness.
Keywords: male body attractiveness, volume height index, body mass index, body proportion, waist-to-hip ratio
1. Introduction
The notion that male body attractiveness is a reliable indicator of male qualities was proposed by Wallace as an alternative to Darwin’s good taste explanation (Cronin 1991). This alternative explanation assumes that: (i) a reliable connection exists between body attractiveness and male quality; (ii) male attractiveness is an indicator of some components of fitness such as health and vigour; and (iii) females detect and use this indicator for choosing a mate (Singh 1995b; Shackelford et al. 2000).
In contrast to the considerable investigations of the female attractiveness (Singh 1993a, b, 1994a, b, 1995a; Furnham et al. 1997, 1998; Tovée et al. 1998, 1999, 2002; Tovée & Cornelissen 1999, 2001; Fan et al. 2004), less work is reported on the body attractiveness of males.
The waist-to-hip ratio (WHR) is considered as an important measure of the health of both men and women as it is related to the distribution of fat between the upper and lower body and the relative amount of intra- versus extra-abdominal fat. The degree of obesity is positively correlated with WHR in both men and women (Hartz et al. 1984; Jones et al. 1986; Shimokata et al. 1989). The typical range of WHR for Caucasian men had been reported to be 0.80–0.95 (Jones et al. 1986; Marti et al. 1991).
Singh (1995b) investigated the role of WHR in the male body attractiveness as viewed by females. In his study, 87 women volunteers (68 white and 19 hispanic) aged between 18 and 22 years ranked 12 line drawing stimuli of male figures representing four levels of WHR and three levels of body weight. The results showed that the stimulus whose WHR is 0.9 was overwhelmingly ranked as the most attractive out of all stimuli. This finding was also confirmed with German men in a separate study using the same line drawing stimuli.
Maisey et al. (1999) considered two new body parameters in addition to WHR, namely waist–to-chest ratio (WCR) and the body mass index (BMI). In their study, 30 female undergraduates (average age: 20.6 years, s.d. 1.4) rated colour pictures of 50 men in front view. Multiple-polynomial regression was used to identify the parameters that were the best predictors of male attractiveness. WCR was found to be the principal determinant of attractiveness and accounted for 56% of the variance, whereas BMI accounted for only 12.7% of additional variance. WHR was not a significant predictor of attractiveness in the model. Their finding means that women prefer men whose torso has an ‘inverted triangle’ shape (i.e. a narrow waist and a broad chest and shoulders). This is a shape consistent with physical strength and muscle development in the upper body. The relatively less importance of BMI in male attractiveness is in sharp contrast to the significance of BMI in determining female attractiveness (Tov ée et al. 1998; Tovée & Cornelissen 1999; Fan et al. 2004).
In the present study, three-dimensional (3D) male body images were viewed and rated in terms of body attractiveness, and the relationship between the male body attractiveness and the body measurements and ratios are investigated.
2. Samples and methods
The subjects were 25 Caucasian males and 69 Chinese males with BMIs ranging from 17.4 to 30.7 kg m−2 (the corresponding volume height index (VHI) ranged from 15.2 lm−2 to 44.2 lm−2). They were scanned using a [TC]2 body scanner (Davis 2001) to obtain 3D body measurements, which were then used to create 3D wire-frame male body images and short film clips by Maya software for viewing and rating the attractiveness. Each film clip was standardized in the same way as reported in a previous paper (Fan et al. 2004). The body image rotates 360° during viewing. The descriptive statistics of the important biometric measures of the 94 male subjects are listed in table 1.
Table 1.
Descriptive statistics of important biometric measures of male subjects.
| minimum | maximum | mean | s.d. | |
| BMI (kgm−2) | 17.40 | 30.73 | 21.4578 | 2.51129 |
| VHI (lm−2) | 15.18 | 44.19 | 20.0083 | 4.27646 |
| WCR | 0.72 | 1.01 | 0.8216 | 0.04740 |
| WHR | 0.72 | 1.01 | 0.8323 | 0.05522 |
| SHC | 0.62 | 0.78 | 0.6946 | 0.03774 |
| abs(WHR–0.8) | 0.00 | 0.21 | 0.0486 | 0.04139 |
| height (cm) | 160.00 | 192.85 | 174.8630 | 6.73167 |
| chin height (cm) | 134.01 | 167.14 | 149.8487 | 6.93226 |
| chest girth (cm) | 84.73 | 139.07 | 100.0350 | 9.99057 |
| chest height (cm) | 117.71 | 144.39 | 129.4790 | 5.96096 |
| waist girth (cm) | 64.43 | 132.64 | 82.4835 | 12.22553 |
| waist height (cm) | 30.74 | 65.11 | 39.9323 | 6.10893 |
| hip girth (cm) | 85.97 | 142.13 | 98.7292 | 9.18260 |
| hip height (cm) | 70.46 | 108.81 | 82.6332 | 6.11101 |
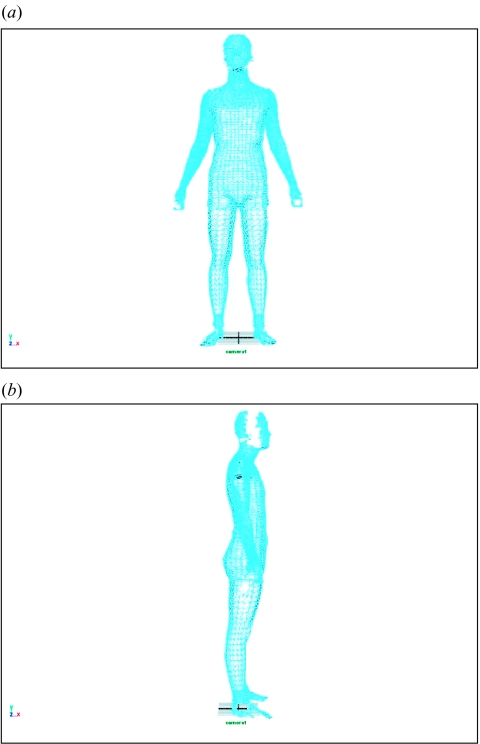
An example of the 3D male image is shown in figure 1. Forty-three young Hong Kong Chinese (20 male, aged 20–35 years; 23 female, aged 20–30 years) were invited to rate the scanned male images in terms of body attractiveness on a nine-point Likert scale (1 the least attractive, 9 the most attractive). The meaning of ‘attractiveness’ was defined and explained to the rater as ‘beauty of body’.
Figure 1.
(a) An example of a film clip in front view. (b) An example of a film clip in side view.
In this paper, VHI is defined as the total body volume divided by the stature height in litres per square metre. The volumes of the male images were calculated using Rapidform Basis (INUS 2004). The VHI defined in the present paper is linearly related to the VHI* defined in our previous paper (Fan et al. 2004), which is the volume excluding the head and feet divided by the chin height (viz. the height from chin to feet):
| (2.1) |
Here, both VHI and VHI* are in litres per square metre. All data on scanned male subjects were analysed by using SPSS.
3. Results analysis
3.1 Comparison of attractiveness ratings by male and female raters
For the male raters, the s.d. of ratings ranged from 0.88 to 1.68; and for the female raters, the s.d. ranged from 1.01 to 1.76. The average attractiveness ratings (AR) by female raters were compared with those by male raters using the Wilcoxon signed-rank method. The results (z=−4.653 (based on negative ranks); asymp. sig. (two-tailed)=0.000 < 0.05) indicated some statistical differences between the ARs by female and male raters. Detailed comparison of ARs by male raters (ARMs) and ARs by female raters (ARFs) revealed that ARMs are slightly greater (4.3% on average) than those of ARFs, although they are strongly related (r2=0.872, p < 0.01). This means that although there is very good agreement between the two genders on male body attractiveness as predicted by the mate-selection theory (Tovée & Cornelissen 2001), females tend to have slightly higher expectations of male body attractiveness than males themselves do. By one-sample Kolmogorov–Smirnov testing, we obtained the following result: for female raters, Kolmogorov–Smirnov Z=1.187 with asymp. sig. (two-tailed)=0.120; for male raters, Kolmogorov–Smirnov Z=1.253 with asymp. sig. (two-tailed)=0.087. ARF/ARM is of normal distribution, which means that raters agree that image A is more or less attractive than image B.
3.2 Effects of waist-to-chest ratio, body mass index and waist-to-hip ratio
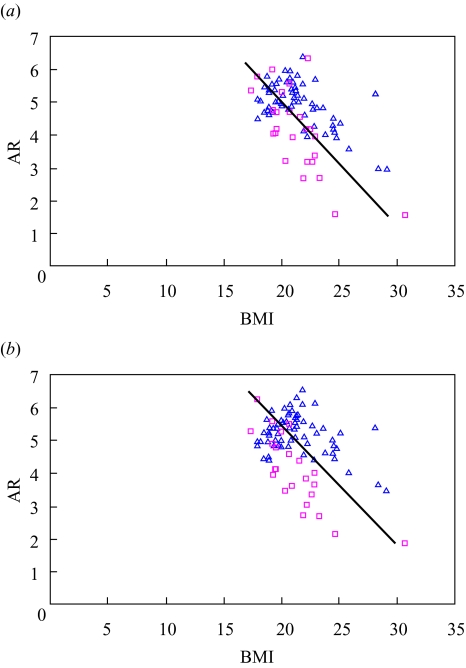
Maisey et al. (1999) identified WCR, BMI and WHR as main factors of male body attractiveness. The effects of these parameters on ARs are further examined in this study. Figure 2a,b plots the ARs of female raters and male raters versus BMI, respectively. The similarity between figure 2(a) and 2(b) is owing to the high correlation between the male and female assessments of the same male body. It can be seen that there tends to be a weak negative correlation between ARs and BMI. The r2 for ARFs versus BMI is 0.299 and that for ARMs versus BMI is 0.161.
Figure 2.
(a) Plot of BMI versus male AR by female raters. (b) Plot of BMI versus male AR by male raters.
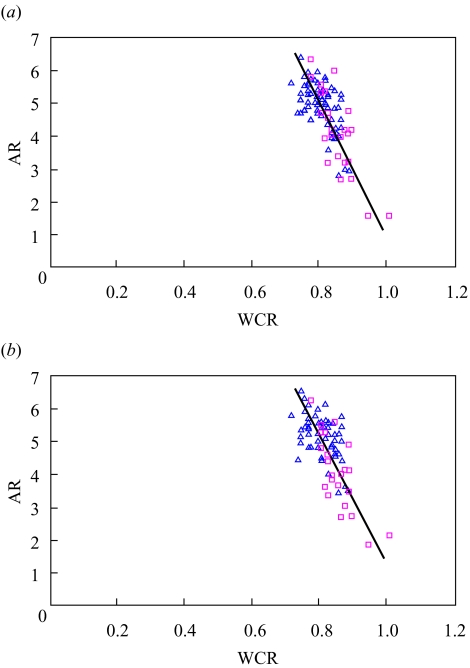
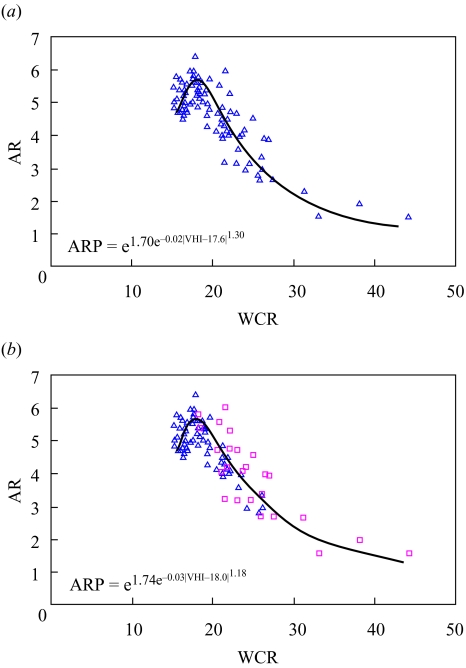
Figure 3a,b plots the male ARs of male raters and female raters versus WCR, respectively. There is a significant difference between WCR and BMI (Z=−8.329 with asymp. sig. (two-tailed)0.000 based on Wilcoxon signed ranks test). WCR has a stronger negative correlation with ARs than BMI (r2=0.545 and r2=0.507, respectively). Male bodies having lower WCR values tend to have higher AR within the range of male bodies investigated, which is in agreement with the findings of Maisey et al. (1999).
Figure 3.
(a) Plot of WCR versus male AR by female raters. (b) Plot of WCR versus male AR by male raters.
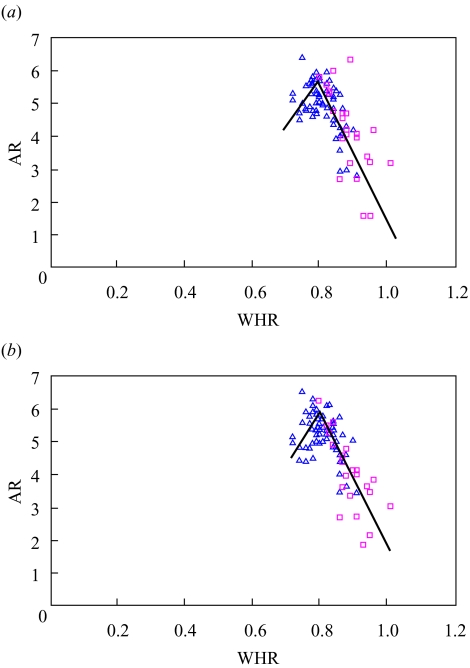
Figure 4a,b plots the male ARs of male raters and female raters versus WHR, respectively. There is also a moderate linear relationship between WCR and male AR (r2=0.450 and r2=0.487, respectively). It is obvious from figure 4a,b that there is an optimal WHR for male body attractiveness. The optimal WHR for the range of male bodies investigated is ca. 0.8.
Figure 4.
(a) Plot of WHR versus male AR by female raters. (b) Plot of WHR versus male AR by male raters.
Following Maisey et al.’s (1999) approach, we applied multiple linear regression with a stepwise variable selection method with ARMs or ARFs as the dependent variable and WCR, BMI and WHR as potential independent variables. The analysis showed that, for both ARFs and ARMs, WCR was chosen as the most important factor and accounted for 53.6% and 49.6% of the variance, respectively. BMI was chosen as the second significant factor for ARs by female raters and accounted for only 4.3% of additional variance; WHR was chosen as the second significant factor for ARs by male raters and accounted for only 3.5% of additional variance. These results are in good agreement with what was reported by Maisey et al. (1999), whose results were only for the male attractiveness assessed by male raters.
3.3 Interrelationships between key biometric ratios and male attractiveness
With reference to Singh (1995b)’s work, there could be an optimum WHR and the deviation from the optimum WHR is related to male attractiveness. To find out the value of the optimum WHR, we first assumed it to be a value between 0.65 and 0.90 and compute AWHR=abs(WHR-i), where i=0.65, 0.70, 0.75, 0.79, 0.80, 0.81, 0.82, 0.85, 0.90, respectively. We then calculate the Pearson correlation between the respective AWHRs and ARF and ARM. It was found that the correlation between abs(WHR-i) and ARF or ARM was the highest when i=0.80, which is −0.704 and −0.767, respectively. It was believed that the optimum WHR for our group of male samples is 0.80.
Key biometric ratios such BMI (body mass index). VHI (volume height index), WCR (waist–chest ratio), AWHR, SHC (stomach–chin height ratio) were included for Pearson correlation analysis. The results are listed in table 2.
Table 2.
Correlation between key biometric ratios and male AR. (**Pearson correlation is significant at the 0.01 level (two-tailed); *Pearson correlation is significant at the 0.05 level (two-tailed).)
| ARF | ARM | BMI | VHI | WCR | WHR | SHC | Abs(WHR−0.8) | |
| ARF | 1 | 0.934** | −0.547** | −0.796** | −0.738** | −0.671** | 0.090 | −0.704** |
| — | 0.000 | 0.000 | 0.000 | 0.000 | 0.000 | 0.389 | 0.000 | |
| ARM | 0.934** | 1 | −0.402** | −0.754** | −0.712** | −0.698** | 0.061 | −0.767** |
| 0.000 | — | 0.000 | 0.000 | 0.000 | 0.000 | 0.563 | 0.000 | |
| BMI | −0.547** | −0.402** | 1 | 0.650** | 0.506** | 0.466** | −0.204 | 0.324** |
| 0.000 | 0.000 | — | 0.000 | 0.000 | 0.000 | 0.052 | 0.002 | |
| VHI | −0.796** | −0.754** | 0.650** | 1 | 0.735** | 0.746** | −0.219* | 0.680** |
| 0.000 | 0.000 | 0.000 | — | 0.000 | 0.000 | −0.036 | 0.000 | |
| WCR | −0.738** | −0.712** | 0.506** | 0.735** | 1 | 0.790** | −0.418** | 0.600** |
| 0.000 | 0.000 | 0.000 | 0.000 | — | 0.000 | 0.000 | 0.000 | |
| WHR | −0.671** | −0.698** | 0.466** | 0.746** | 0.790** | 1 | −0.406** | 0.778** |
| 0.000 | 0.000 | 0.000 | 0.000 | 0.000 | — | 0.000 | 0.000 | |
| SHC | 0.090 | 0.061 | −0.204 | −0.219* | −0.418** | −0.406** | 1 | −0.030 |
| 0.389 | 0.563 | 0.052 | 0.036 | 0.000 | 0.000 | — | 0.771 | |
| abs(WHR–0.8) | −0.704** | −0.767** | 0.324** | 0.680** | 0.600** | 0.778** | −0.030 | 1 |
| 0.000 | 0.000 | 0.002 | 0.000 | 0.000 | 0.000 | 0.771 | — |
It is clear that VHI has the strongest correlation with male body attractiveness. The second parameter is WCR, followed by abs(WHR − 0.8), WHR and then BMI. This means that VHI is a more important factor than WCR and BMI, and the deviation of WHR from its optimal value affects the male body attractiveness, rather than WHR itself.
3.4 Volume height index versus male AR
Figure 5a,b shows the plots of the male ARs versus VHI. As can be observed, there is an optimal VHI for male attractiveness. The effect of VHI on male body attractiveness is not linear. The relationship between VHI and ARFs or ARMs can be best fitted with a bell-shaped exponential curve:
| (3.1) |
Clearly, we can see an optimal value of VHI for ARF at ca. 17.6 l m−2 and for ARM at 18.0 l m−2, respectively. As VHI deviates from the optimal value, AR reduces. When VHI is far from the optimal value, AR will approach the minimum rating. This trend can be explained by the response compression theory in psychophysics.
Figure 5.
(a) Plot of VHI versus male AR by female raters. (b) Plot of VHI versus male AR by male raters.
From the plots shown in figures 2–5, it can also be seen that there are various degrees of differences between Chinese and Caucasian male images in terms of the effect of BMI, WCR, WHR or VHI on ARF/ARM. The difference is more pronounced for the effect of BMI, but much less for the effect of WCR, WHR or VHI. This may be caused by the racial differences in mass–volume relationship and body proportions other than WCR, WHR and VHI.
3.5 Relationship between AR and other male body physical parameters by the principal component analysis method
To find out how other body proportions, in addition to VHI, affect the perception of male body attractiveness, 18 key body ratios were considered. They include the ratios of vertical measurements such as the ratio of waist height over chin height, the ratios of horizontal measurements such as WHR, the ratios of width over depth such as ratio of waist width over waist depth, etc.
Principal component analysis (PCA) in SPSS was first applied to examine collinearity and extract key components. The results shown in table 3 indicate that five independent components can be extracted with eigenvalues greater than 1.0. Table 4 shows the correlation between the five principal components and the body ratios. Component 1 (C1) is related to the majority of the ratios and is hence a measure of the appropriateness of the main body proportions. Component 2 (C2) is mainly related to the ratios of horizontal dimensions (such as chest girth, waist girth and hip girth) over waist measurement. Component 3 (C3) is mainly related to the proportions in the vertical direction. Component 4 (C4) is mainly related to the relative position of the waist in vertical and horizontal directions. Component 5 (C5) is mainly related to the proportions of the lower torso, especially abdomen and hips. The effects of these five independent components on male body attractiveness were analysed using the stepwise variable selection method in multiple linear regression. In this analysis, the actual ARFs or ARMs were considered as the dependent variable, and ARs predicted by equation (3.1), and the five components as potential independent variables. The components selected with significance level of p<0.05 (t=2.04) are C1, C2 and C5. Tables 5 and 6 list the prediction models derived from the multiple linear regression. Taking into account the effects of these three parameters for the male body, ARs can be predicted using the following equations:
| (3.2) |
| (3.3) |
where ARFP* and ARMP* are the ARs by female raters and male raters predicted by VHI alone using equation (3.1). The component scores C1, C2 and C5 are component scores which can be calculated from
| (3.4) |
where is the score of the jth component for case k, is the standardized value (standardized to a mean of 0 and s.d. 1) of the ith body ratio for case k, and Wji is the component score coefficient for the jth component and the ith variable. The values of Wji are listed in table 7.
Table 3.
Total variance explained. (Extraction method: PCA.)
| initial eigenvalues |
extraction sums of squared loadings |
rotation sums of squared loadings |
|||||||
| component | total | percentage of variance | cumulative (%) | total | percentage of variance | cumulative (%) | total | percentage of variance | cumulative (%) |
| 1 | 6.729 | 37.384 | 37.384 | 6.729 | 37.384 | 37.384 | 5.020 | 27.887 | 27.887 |
| 2 | 2.925 | 16.252 | 53.636 | 2.925 | 16.252 | 53.636 | 2.546 | 14.144 | 42.031 |
| 3 | 1.736 | 9.642 | 63.279 | 1.736 | 9.642 | 63.279 | 2.439 | 13.549 | 55.580 |
| 4 | 1.226 | 6.811 | 70.090 | 1.226 | 6.811 | 70.090 | 1.951 | 10.837 | 66.417 |
| 5 | 1.111 | 6.170 | 76.260 | 1.111 | 6.170 | 76.260 | 1.772 | 9.843 | 76.260 |
| 6 | 0.901 | 5.004 | 81.264 | — | — | — | — | — | — |
Table 4.
Varimax rotated component matrix.(Extraction method: PCA. Rotation method: varimax with Kaiser normalization. Rotation converged in seven iterations.)
| component |
|||||
| 1 | 2 | 3 | 4 | 5 | |
| ratio of chest height over chin height | — | — | 0.700 | — | — |
| ratio of waist height over chin height | — | — | 0.608 | 0.513 | |
| ratio of hip height over chin height | 0.497 | — | 0.630 | — | — |
| ratio of crotch height over chin height | — | — | 0.878 | — | — |
| ratio of knee height over chin height | — | — | 0.700 | — | — |
| ratio of waist girth over chin height | 0.869 | — | — | — | — |
| ratio of hip girth over chin height | 0.825 | — | — | — | — |
| ratio of chest girth over chin height | 0.852 | — | — | — | — |
| ratio of waist girth over hip girth | 0.687 | 0.430 | — | −0.416 | — |
| ratio of waist girth over chest girth | 0.627 | 0.548 | — | — | — |
| ratio of waist girth over abdomen girth | — | 0.557 | — | — | — |
| ratio of waist width over shoulder width | — | 0.865 | — | — | — |
| ratio of waist width over waist depth | −0.792 | — | — | — | — |
| ratio of chest width over chest depth | — | −0.712 | — | — | — |
| ratio of hip width over hip depth | −0.616 | — | — | — | −0.474 |
| ratio of waist girth over stomach girth | — | — | — | — | 0.876 |
| ratio of stomach height over chin height | — | — | — | 0.851 | — |
| abs(WHR–0.8) | 0.664 | — | — | — | — |
Table 5.
Models of ARF based on components extracted by PCA.(Dependent variable: AR by female viewers.)
| unstandardized coefficients |
standardized coefficients |
|||||
| model | B | s.e. | β | t | adjusted r2 | |
| 1 | (constant) | −0.409 | 0.331 | — | −1.234 | 0.728 |
| ARFP | 1.072 | 0.069 | 0.855 | 15.624 | ||
| 2 | (constant) | 0.555 | 0.502 | — | 1.105 | 0.743 |
| ARFP | 0.869 | 0.105 | 0.693 | 8.285 | ||
| C1 | −0.199 | 0.079 | −0.210 | −2.506 | ||
| 3 | (constant) | 1.315 | 0.636 | — | 2.067 | 0.750 |
| ARFP | 0.710 | 0.133 | 0.566 | 5.329 | ||
| C1 | −0.291 | 0.092 | −0.307 | −3.162 | ||
| C2 | −0.121 | 0.064 | −0.128 | −1.901 | ||
| 4 | (constant) | 2.008 | 0.684 | — | 2.937 | 0.763 |
| ARFP | 0.564 | 0.143 | 0.449 | 3.934 | ||
| C1 | −0.377 | 0.096 | −0.397 | −3.903 | ||
| C2 | −0.164 | 0.065 | −0.174 | −2.542 | ||
| C5 | 0.127 | 0.053 | 0.135 | 2.395 | ||
Table 6.
Models of ARM based on components extracted by PCA.(Dependent variable: AR by male viewers.)
| unstandardized coefficients |
standardized coefficients |
|||||
| model | B | s.e. | β | t | ajusted r2 | |
| 1 | (constant) | 0.138 | 0.295 | — | 0.468 | 0.746 |
| ARMP | 0.984 | 0.060 | 0.865 | 16.364 | ||
| 2 | (constant) | 0.262 | 0.297 | — | 0.882 | 0.754 |
| ARMP | 0.958 | 0.061 | 0.842 | 15.812 | ||
| C5 | 0.096 | 0.049 | 0.105 | 1.976 | ||
| 3 | (constant) | 0.523 | 0.319 | — | 1.640 | 0.762 |
| ARMP | 0.904 | 0.065 | 0.795 | 13.885 | ||
| C5 | 0.105 | 0.048 | 0.115 | 2.183 | ||
| C2 | −0.104 | 0.051 | −0.114 | −2.036 | ||
| 4 | (constant) | 1.494 | 0.538 | — | 2.775 | 0.772 |
| ARMP | 0.703 | 0.111 | 0.618 | 6.328 | ||
| C5 | 0.141 | 0.050 | 0.154 | 2.829 | ||
| C2 | −0.167 | 0.058 | −0.182 | −2.900 | ||
| C1 | −0.178 | 0.081 | −0.193 | −2.214 | ||
Table 7.
Component score coefficient matrix.(Extraction method: PCA. Rotation method: varimax with Kaiser normalization. Component scores.)
| component | |||||
| 1 | 2 | 3 | 4 | 5 | |
| ratio of chest height over chin height | 0.014 | −0.077 | 0.269 | 0.059 | 0.052 |
| ratio of waist height over chin height | −0.025 | 0.139 | 0.044 | 0.271 | 0.256 |
| ratio of hip height over chin height | 0.106 | 0.031 | 0.224 | 0.058 | 0.156 |
| ratio of crotch height over chin height | −0.087 | −0.001 | 0.417 | −0.165 | −0.039 |
| ratio of knee height over chin height | −0.009 | −0.040 | 0.336 | −0.130 | −0.128 |
| ratio of waist girth over chin height | 0.173 | −0.004 | −0.011 | −0.012 | 0.007 |
| ratio of hip girth over chin height | 0.223 | −0.059 | −0.110 | 0.131 | 0.019 |
| ratio of chest girth over chin height | 0.237 | −0.138 | −0.033 | 0.076 | −0.110 |
| ratio of waist girth over hip girth | 0.063 | 0.059 | 0.122 | −0.202 | −0.013 |
| ratio of waist girth over chest girth | 0.019 | 0.189 | 0.040 | −0.176 | 0.173 |
| ratio of waist girth over abdomen girth | −0.094 | 0.261 | 0.038 | −0.118 | −0.042 |
| ratio of waist width over shoulder width | −0.171 | 0.513 | −0.020 | −0.031 | 0.170 |
| ratio of waist width over waist depth | −0.259 | 0.232 | 0.041 | −0.008 | 0.031 |
| ratio of chest width over chest depth | 0.080 | −0.410 | 0.131 | −0.345 | 0.138 |
| ratio of hip width over hip depth | −0.222 | 0.084 | 0.045 | −0.175 | −0.263 |
| ratio of waist girth over stomach girth | −0.024 | 0.037 | −0.037 | −0.138 | 0.534 |
| ratio of stomach height over chin height | 0.077 | −0.009 | −0.081 | 0.538 | −0.150 |
| abs(WHR–0.8) | 0.117 | 0.027 | 0.080 | 0.072 | −0.188 |
The negative effect of C1 can be understood as a smaller C1 is associated with better ratios of horizontal measurements over vertical height and relatively flat body shape, which is associated with fitness. In other words, an obese male with larger waist girth, hip girth or chest girth over chin height has poor body attractiveness. Also because C1 is positively related to abs(WHR–0.8), greater deviations of WHR from the ideal value of 0.8 will increase C1 and hence reduce the body attractiveness.
The negative effect of C2 can be interpreted that the smaller the waist girth in relation to chest, abdomen and hip girth, the more attractive the body shape tends to be. The positive effect of C5 can be explained by the fact that a smaller hips width over depth (i.e. greater prominence of hips) and a greater ratio of waist girth over stomach girth (i.e. smaller stomach) will enhance male body attractiveness.
4. Conclusions
In this paper, male body attractiveness by young viewers was investigated based on 69 Chinese male subjects and 25 Caucasian male subjects. We first analysed the relationship between male attractiveness and the three body parameters as proposed by Maisey et al. (1999), WCR, BMI and WHR. The results showed that for both female and male observers, WCR was the most important factor of male attractiveness among the three. This finding is to some extent consistent with that of Maisey et al. (1999). Further analysis of the relationship between male ARs and body ratios showed the following.
Among the body ratios considered, VHI is the most important visual cue to male body attractiveness, which explains ca. 73% of the variance. The effect of VHI can be fitted with two half bell-shaped exponential curves with an optimal VHI for female raters and male raters at 17.6 lm−2 and 18.0 lm−2, respectively. VHIs of either greater or less than the optimal value will reduce male body attractiveness. As VHI deviates from the optimal value, the rate of reduction in AR increases and then reduces. When VHI is far from the optimal value, AR will approach a minimum rating and changes in VHI cause little further reduction in AR.
Apart from VHI, other body parameters or ratios representing the appropriateness of body proportions, can have small, but significant effects on male body attractiveness. These parameters include the relative positions of body parts in the vertical direction, the ratios of horizontal dimensions, and the shape and appearance of important parts, i.e. the waist, chest and hip.
The ideal WHR for male body attractiveness is 0.80. This is smaller than the ideal value of 0.9 reported by Singh (1995b). The difference may be caused by the different racial backgrounds of the viewers.
Acknowledgments
This work is supported by the ASD and CRG fund of the Hong Kong Polytechnic University. The authors thank W. Zhang for facilitating the use of [TC]2 BMS system, A. Chan for assistance in organizing the rating of male images and Dr K. F. Choi for advice on PCA analysis.
References
- Cronin H. The ant and the peacock. Cambridge University Press; New York: 1991. [Google Scholar]
- Davis K. [TC]2’s body scanner. 2001 See http://www.tc2.com/RD/RDBody.htm.
- Fan J.T., Liu F., Wu J., Dai W. Visual perception of female physical attractiveness. Proc. R. Soc. B. 2004;271:347–352. doi: 10.1098/rspb.2003.2613. doi:10.1098/rspb.2003.2613 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Furnham A., Tan T., McManus C. Waist-to-hip ratio and preferences for body shape: a replication and extension. Personality Individual Differences. 1997;22:539–549. [Google Scholar]
- Furnham A., Dias M., McClelland A. The role of body weight, waist-to-hip ratio, and breast size in judgments of female attractiveness. Sex Roles. 1998;39:311–326. [Google Scholar]
- Hartz A.J., Rupley D.C., Rimm A.A. The association of girth measurement with disease in 32856 women. Am. J. Epidemiol. 1984;119:71–80. doi: 10.1093/oxfordjournals.aje.a113727. [DOI] [PubMed] [Google Scholar]
- INUS Technology Inc. Rapidform Basis. 2004 See http://www.rapidform.com/product/product10_1.htm.
- Jones P.R.M., Hunt M.J., Brown T.P., Norgan N.G. Waist–hip circumference ratio and its relation to age and overweight in British men. Hum. Nutr. Clin. Nutr. 1986;40C:239–247. [PubMed] [Google Scholar]
- Maisey D.S., Vale E.L.E., Cornelissen P.L., Tovée M.J. Characteristics of male attractiveness for women. Lancet. 1999;353:1500. doi: 10.1016/s0140-6736(99)00438-9. [DOI] [PubMed] [Google Scholar]
- Marti B., Tuomilehto J., Saloman V., Kartovaara L., Korhonen H.J., Pietinen P. Body fat distribution in the Finnish population: environmental determinants and predictive power for cardiovascular risk factor levels. J. Epidemiol. Community Hlth. 1991;45:131–137. doi: 10.1136/jech.45.2.131. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Shackelford T.K., Weekes-Shackelford V.A., LeBlanc G.J., Bleske A.L., Euler H.A., Hoier S. Female coital orgasm and male attractiveness. Hum. Nature Interdisciplin. Biosocial Perspect. 2000;11:299–306. doi: 10.1007/s12110-000-1015-1. [DOI] [PubMed] [Google Scholar]
- Shimokata H., Tobin J.D., Muller D.C., Elahi D., Coon P.J., Andres R. Studies in the distribution of body fat: in effects of age, sex and obesity. J. Gerontol. Med. Sci. 1989;44:M66–M73. doi: 10.1093/geronj/44.2.m66. [DOI] [PubMed] [Google Scholar]
- Singh D. Adaptive significationce of female physical attractiveness: role of the waist-to-hip ratio. J. Personality. Social. Psychol. 1993a;65:293–307. doi: 10.1037//0022-3514.65.2.293. [DOI] [PubMed] [Google Scholar]
- Singh D. Body shape and women’s attractiveness: the critical role of waist-to-hip ratio. Hum. Nature. 1993b;4:297–321. doi: 10.1007/BF02692203. [DOI] [PubMed] [Google Scholar]
- Singh D. Is thin really beautiful and good? Relationship between waist-to-hip ratio (WHR) and female attractiveness. Pers. Indiv. Diff. 1994a;16:465–481. [Google Scholar]
- Singh D. Ideal female body shape: the role of body weight and waist-to-hip ratio (WHR) ratio. Int. J. Eat. Disord. 1994b;16:283–288. doi: 10.1002/1098-108x(199411)16:3<283::aid-eat2260160309>3.0.co;2-q. [DOI] [PubMed] [Google Scholar]
- Singh D. Female health, attractiveness and desirability for relationships: role of breast asymmetry and waist-to-hip ratio. Ethol. Sociobiol. 1995a;16:465–481. doi: 10.1037//0022-3514.69.6.1089. [DOI] [PubMed] [Google Scholar]
- Singh D. Female judgment of male attractiveness and desirability for relationships: role of waist-to-hip ratio and financial status. J. Personality Social Psychol. 1995b;69:1089–1101. doi: 10.1037//0022-3514.69.6.1089. [DOI] [PubMed] [Google Scholar]
- Tovée M.J., Cornelissen P.L. The mystery of female beauty. Nature. 1999;399:215–216. doi: 10.1038/20345. [DOI] [PubMed] [Google Scholar]
- Tovée M.J., Cornelissen P.L. Female and male perceptions of female physical attractiveness in front-view and profile. Br. J. Psychol. 2001;92:391–402. [PubMed] [Google Scholar]
- Tovée M.J., Reinhardt S., Emery J.L., Cornelissen P.L. Optimal BMI and maximum sexual attractiveness. Lancet. 1998;352:548. doi: 10.1016/s0140-6736(05)79257-6. [DOI] [PubMed] [Google Scholar]
- Tovée M.J., Maisey D.S., Emery J.L., Cornelissen P.L. Visual cues to female physical attractiveness. Proc. R. Soc. B. 1999;266:211–218. doi: 10.1098/rspb.1999.0624. doi:10.1098/rspb.1999.0624 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Tovée M.J., Hancock P.J.B., Mahmoodi S., Singleton B.R.R., Cornelissen P.L. Human female attractiveness: waveform analysis of body shape. Proc. R. Soc. B. 2002;269:2205–2213. doi: 10.1098/rspb.2002.2133. doi:10.1098/rspb.2002.2133 [DOI] [PMC free article] [PubMed] [Google Scholar]