Embryo development and ageing in birds and mammals

Abstract

The rate of ageing is a genetically influenced feature of an individual's life history that responds to selection on lifespan. Various costs presumably constrain the evolution of prolonged life, but these have not been well characterized and their general nature is unclear. The analyses presented here demonstrate a correlation among birds and mammals between rates of embryonic growth and ageing-related mortality, which are quantified by the exponents of fitted power functions. This relationship suggests that rapid early development leads to accelerated ageing, presumably by influencing some aspect of the quality of the adult individual. Although the mechanisms linking embryo growth rate and ageing are not known, a simple model of life-history optimization shows that the benefits of longer life can be balanced by connected costs of extended development.

1. Introduction

Individual vertebrates age at different rates (Finch 1990), but the biological basis of this variation is poorly understood. Lifespan is influenced by genetic factors (Curtsinger et al. 1995; Zwaan 1999; Finch & Ruvkun 2001), and artificial selection on laboratory populations of organisms has both extended and reduced lifespan (Rose 1991; Partridge & Barton 1993; Stearns et al. 1998; Stearns et al. 2000). As an evolved life-history trait, rate of ageing is correlated with the level of extrinsic mortality experienced by a population (Ricklefs 1998), which governs the proportion of individuals surviving to a particular age and hence the strength of selection to extend lifespan beyond that age (Williams 1957; Hamilton 1966; Abrams 1993).

Connections between early development and ageing, which might constrain the evolution of lifespan, have received considerable attention recently. These include phenotypic effects of poor nutrition or other stress during the growth period (Metcalfe & Monaghan 2003), pleiotropic interactions (Williams 1957; Rose 1991) between development of immune function and adverse effects of inflammation later in life (Finch & Crimmins 2004), and postulated accumulation of defects during embryo development (Gavrilov & Gavrilova 2001). Comparative analyses have related lifespan to rate of early development (Promislow 1991; Ricklefs 1993; Ricklefs & Scheuerlein 2001), but the mechanisms responsible for these connections are unknown.

Most ideas about the causes of ageing focus on the accumulation of genetic and other damage. This process presumably starts at the beginning of life and individuals are, therefore, born with defects that influence their potential lifespans (Gavrilov & Gavrilova 2001). Underscoring this point, stress during early development (foetal programming; Schwartz & Morrison 2005), including nutritional deficiency and other traumas, can reduce longevity. Considerable evidence points to reactive oxygen species as a primary cause of damage (Beckman & Ames 1998; Barja 2004). However, organisms vary in the rate at which they accumulate such damage and species with similar rates of metabolism have quite different lifespans (Austad & Fischer 1991).

As pointed out by Kirkwood & Holliday (1979), organisms can invest, at a cost measured in units of evolutionary fitness, in mechanisms that prevent or repair damage and thereby extend lifespan. The optimal balance between lifespan and other fitness-generating activities presumably depends on the potential longevity afforded by extrinsic causes of mortality (Williams 1957; Hamilton 1966; Partridge & Barton 1993).

Despite the evidence for connections between development and ageing, biologists have yet to understand their functional relationship. Here, I demonstrate a link between rate of increase in ageing-related mortality and embryo growth rate in birds and mammals and show how balancing selection on embryo growth and adult ageing could optimize both within this constraint. These analyses are consistent with a functional relationship between the beginning and the end of an individual's life.

2. Materials and methods

(a) Embryo growth

Details concerning the sources of data for avian embryonic growth may be found in Ricklefs (1987). Foetal growth data for a variety of placental mammals were obtained from literature sources whose details are provided in the electronic supplementary material. Increase in embryo mass (w, grams) with age (x, days) can be described by a power function,

$$w=a{x}^b,$$ 2.1

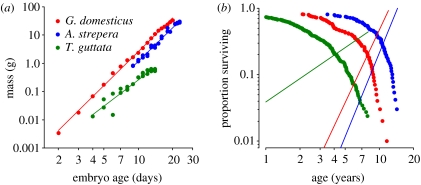
where b (dimensionless) defines the rate of increase in the growth rate with respect to time, and a (dimension: mass×time^−b) is a scaling constant that influences growth rate equally throughout the embryo stage (Huggett & Widdas 1951; figure 1a). Growth curves were fit by nonlinear least-squares regression (SAS procedure NLIN) of the equation w=a(x−t₀)^b, where t₀ is a lag in the onset of embryo growth. For avian embryos, t₀ generally did not differ significantly from zero, except in the case of the exceptionally long development periods of pelagic seabirds (Ricklefs 1987). Owing to delayed implantation, mammal embryos typically exhibited significant positive values of t₀ (Huggett & Widdas 1951).

Figure 1.

Power functions fitted to the relationship of embryo mass and adult survival to time. (a) Embryo growth curves for zebra finch, Taeniopygia guttata (b=1.98), Rhode Island Red chicken, Gallus domesticus (3.25) and gadwall, Anas strepera (4.47). (b) Survival curves for zebra finch (β=1.17) to red jungle fowl females, Gallus gallus (3.63) and gadwall (4.17). The straight lines are the ageing-related components of hazard functions for power (Weibull) models fitted to the survival curves.

(b) Ageing-related mortality

Age-specific rates of mortality were obtained by analysis of ages at death in zoo populations of birds and mammals and in a limited number of wild populations (Ricklefs 1998, 2000; Ricklefs & Scheuerlein 2001). Data within ranges of age exhibiting high juvenile mortality were excluded from this analysis. Thus, age-specific mortality refers only to adult individuals from the age of the minimum age-specific mortality rate (x_m), although age 0 was nonetheless defined by birth and survival at age x_m was estimated by extrapolating the minimum exponential mortality rate from age 0. In parallel to the description of embryo growth (equation (2.1)), one can represent the increase in mortality rate with age by a power relationship of the form αx^β (the Weibull ageing function; Gavrilov & Gavrilova 1991) to which a constant term (m₀) can be added to represent extrinsic non-ageing-related mortality, to produce the Weibull-plus-constant function,

$$m_x=m_0+a{x}^{\beta }.$$ 2.2

Here, age (x) is expressed in years. The initial mortality rate m₀ is assumed, as a simplification, to be constant throughout the adult lifespan. As expected, this component of mortality differs markedly between wild and captive populations of the same species (Ricklefs 2000; Ricklefs & Scheuerlein 2002). The ageing-related component (αx^β) characterizes increasing mortality, presumably caused by intrinsic factors (e.g. cancer, cardiovascular disease; Horiuchi & Wilmoth 1997) or associated with weakening physiological performance (Ricklefs & Scheuerlein 2002). Units of m are yr⁻¹. The Weibull-plus-constant function is preferable on biological grounds to the Gompertz equation, m_x=m₀e^γx (or its variations, such as the Gompertz–Makeham equation), which is commonly used to describe human demographic ageing (Finch 1990; Gavrilov & Gavrilova 1991). Comparisons have shown that a decrease in the initial mortality rate, for example, by bringing animals into captivity or by domestication, does not change the parameters of the ageing component of mortality in the Weibull-plus-constant function, as required by the Gompertz relationship (Ricklefs 2000; Ricklefs & Scheuerlein 2002). Parameters α and β were estimated for ageing-related mortality by fitting the Weibull-plus-constant survival function (Ricklefs 1998),

$$l_x=\text{exp}\left(-m_{0}x-\frac{\alpha x^{\beta +1}}{\beta +1}\right),$$ 2.3

by nonlinear least-squares regression to the probability of survival with age (l_x) of individuals in wild or captive populations (figure 1b) and, in a few cases, to increasing mortality with age (equation (2.2); see Ricklefs 1998).

I used stepwise regression to determine the relationship between either log α or β representing ageing-related mortality (dependent variable) and parameters loga and b representing embryo growth, as well as adult body mass, egg (bird) or neonate (mammal) mass and incubation (bird) or gestation (mammal) period obtained from a variety of literature sources.

(c) Life-history modelling

The life-history consequences of coupled variation in the embryo growth parameter b and the ageing parameter β were modelled as the product of survival to the end of the embryo development period and the average lifespan of the individual. This product is approximately proportional to individual fitness, all other things being equal. The maximum value of the product defines the optimum development/ageing phenotype. The model isolates the coupled effects of selection on embryo growth and ageing. If such selection also affected survival from hatching to maturity or adult fecundity, the model would be more complex, but these variables are assumed here to be independent of changes in embryo growth and ageing. The point of this model is to demonstrate that the coupled change in embryo growth and ageing has opposite effects on fitness through embryo and adult survival. The optimum point on the slow–fast continuum of correlated rates of embryo growth and ageing is determined by time-dependent mortality rates during the embryo and adult periods. Survival to birth or hatching (s_n), assuming constant time-dependent mortality (m) during the embryo growth period (x_n), is given by the exponential function s_n=exp (−mx_n). The embryo period is related to the embryo growth parameters by rearranging equation (2.1) to x_n=(w_n/a)^1/b, where w_n is the neonatal weight. Average lifespan was calculated as Σxl_x/Σl_x, where l_x is the survivorship to age x (equation (2.3)), iterated over 0.1 year intervals.

3. Results

(a) Neonate size, incubation period, and embryonic growth

Values of b averaged 3.36 (s.d.=0.94, range 1.67–5.16, n=22) for birds and 3.69 (s.d.=1.30, range 1.65–5.60, n=20) for mammals. Embryo growth in birds is constrained by neonatal size and development period. Rearranging equation (2.1) to b=(ln w_n−ln a)/ln x_n shows the constrained relationship between b and ln a for particular values of w_n and x_n. Accordingly, if a remained constant, b would increase with increasing w_n and decrease with increasing x_n, although not linearly. Because the size of the avian chick at hatching is proportional to the size of the egg, one can substitute egg mass for neonate mass in this equation. In a multiple linear regression based on the avian data used in this analysis, the growth parameter b was related to the logarithm of egg mass (F_1,23=150.2, p<0.0001, slope=0.75±0.06), the logarithm of the incubation period (F_1,23=93.3, p<0.0001, slope=−2.02±0.21) and ln a (F_1,23=678, p<0.0001, slope=−0.253±0.010; model F_3,23=567, p<0.0001, R²=0.987). For mammals, b was related to the logarithm of neonate mass (F_1,19=5.6, p=0.029, slope =0.36±0.15), the logarithm of the gestation period minus t₀ (F_1,19=15.9, p=0.0008, slope=−1.70±0.43) and ln a (F_1,19=408, p<0.0001, slope =−0.522±0.023; model F_3,19=147, p<0.0001, R² =0.959). Thus, the acceleration of embryonic growth varies in direct relation to neonate mass, albeit weakly in mammals, and inversely with the development period. This explains why a tiny bird such as the zebra finch (Taeniopygia guttata; adult mass=11 g) has a value of embryo b (1.98) as low as that of the much larger black-footed albatross (Phoebastria nigripes; 3090 g, b=2.32).

(b) Growth and ageing

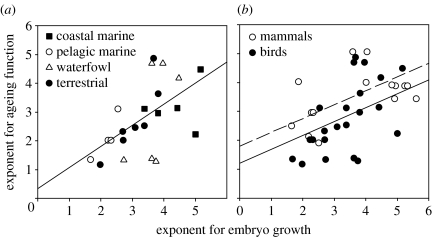
Values of β averaged 2.77 (s.d.=1.21, range 1.17–4.87, n=22) for birds and 3.56 (s.d.=0.83, range 1.90–5.06, n=20) for mammals. The potential relationships of the ageing parameters logα and β to embryo growth parameters a and b, as well as adult body mass, egg or neonate mass and incubation or gestation period, were assessed by stepwise multiple regression with the ageing parameters as the dependent variables. The most prominent result was the relationship of ageing β to embryo b in both birds and mammals, which was independent of variation in adult and neonate size and of variation in the length of the embryo growth period. The slope of the relationship between β and b for birds (figure 2a) was 0.74±0.24 (F_1,21=9.7, p=0.005, R²=0.32). Among mammals, ageing β also was related only to embryo b (F_1,18=6.7, p=0.019, slope=0.33±0.13, R²=0.27). When birds and mammals were considered together (figure 2b), the slopes did not differ significantly, and the common slope was 0.48±0.13 (F_1,40=16.9, p=0.0002); intercepts (1.79 for mammals and 1.20 for birds) differed marginally by 0.59±0.28 (F_1,40=4.4, p=0.043; overall R²=0.35). Ageing logα was weakly negatively related only to embryo b and only among birds (F_1,20=5.0, p=0.036, slope=−1.51±0.67, R² =0.20).

Figure 2.

Relationship between the exponents of power functions for embryo growth, b, and ageing-related mortality, β. (a) Birds (F_1,21=9.7, p=0.005, slope=0.74±0.24, R²=0.32). Several groups of birds are distinguished to show that the relationship is generalized across taxa. (b) Birds and mammals together. Among mammals, ageing β was uniquely related only to embryo b (F_1,18=6.7, p=0.019, slope=0.33±0.13, R²=0.27). The slopes for birds and mammals did not differ significantly and their common slope was 0.48±0.13 (F_1,40=16.9, p=0.0002); intercepts (1.79 for mammals and 1.20 for birds) differed by 0.59±0.28 (F_1,40=4.4, p=0.043; overall R² =0.35). Lines represent least-squares regressions.

(c) Life-history optimization

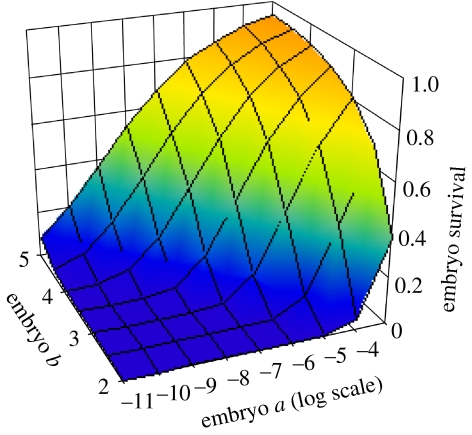
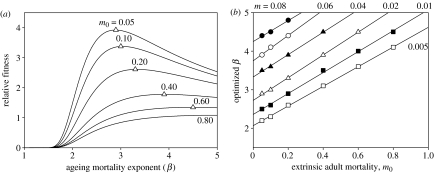
The positive relationship between rates of acceleration of embryo growth and ageing-related mortality constrains the evolution of the life history because their separate influences on fitness are of opposite sign and the two therefore potentially balance each other. Prolonging the reproductive lifespan increases fitness, but the related slowing of embryo growth imposes a cost in terms of the survival of the egg to hatching or of the mother to parturition (figure 3). When embryo b and ageing β are functionally connected, opposing selection on fecundity (embryo survival) and lifespan result in an optimized value of β (figure 4), which varies in direct relation to both extrinsic adult mortality (m₀) and embryo mortality (m). The lowest values of β are predicted for species with (i) low extrinsic adult mortality, which affords the potential of long lifespan and favours delayed senescence, and (ii) low embryo mortality rate, which reduces the cost of extending the embryonic growth period. Lower β is also favoured by small neonate size, which reduces the embryo development period.

Figure 3.

The proportion of embryos surviving to birth as a function of embryo growth parameters a and b, illustrated for m=0.01 d⁻¹ and w_n=10 g.

Figure 4.

Optimization of embryo growth balances embryo and adult survival. (a) Relative fitness (the product of embryo survival (s_n, fecundity) and average adult lifespan) as a function of ageing mortality exponent (β) for m=0.02 d⁻¹, neonate mass=10 g, and the scaling constants a (embryo growth) and α (ageing-related mortality) equal to 0.0001. I set b=β/0.8 (see figure 2a). For this figure, embryo survival was calculated as in figure 3b. Average lifespan was calculated as Σxl_x/Σl_x, where l_x is survivorship to age x, iterated over 0.1 year intervals. The open triangles represent the maximum relative fitness and optimized β for each value of extrinsic mortality, m₀, and correspond to the same symbols for a single line in figure part (b). (b) Optimum β as a function of extrinsic adult mortality and nest mortality rate (m) having descending values as in (a).

4. Discussion

Comparative analyses suggest that embryo b and ageing β are functionally connected, in which case this relationship would constrain the evolutionary response of both embryo development and ageing. For example, if a remained constant, both larger neonatal size and shorter gestation period would require more rapid acceleration of embryo growth rate, which would lead to more rapid acceleration of the ageing-related mortality rate. Extending lifespan by reducing β would require a decrease in b, hence a smaller neonate or longer prenatal development period, both of which have associated costs. Many long-lived birds with low extrinsic adult mortality, particularly pelagic seabirds, have exceptionally long incubation periods for the size of their eggs (Rahn & Ar 1974). Compensating benefits gained from this prominent life-history trait have not been demonstrated (Ricklefs 1984), but it is plausible that slow embryonic development, with a low acceleration of embryo growth rate, is an adaptation to delay ageing.

Embryo growth and ageing are presumably linked through the accumulation of damage by the neonate or the early development of mechanisms to prevent or repair such damage. The only quantitative model of ageing-related mortality that provides as an output the Weibull parameter β comes from reliability theory (Armitage & Doll 1961; Gavrilov & Gavrilova 2001). When the failure (=death) of a system (=organism) follows upon the failure of n independent parts, the rate of system failure initially increases as the n−1 power of time. However, this model does not explain the connection between embryo b and ageing β, in the sense that hastening embryo development need not increase the number of parts that must fail before an individual crosses the threshold to death. Indeed, one might expect the reverse, namely that more rapid growth might result in building less redundancy into a system, lowering β and increasing ageing-related mortality earlier in life.

A consequence of accelerated growth (increased b) is that cell cycles are abbreviated and less time might be available for quality control, for example, through mechanisms of DNA proof-reading and repair (Sancar 1995; Wood 1996; Mol et al. 1999; Park & Gerson 2005). To link the failure of parts (e.g. cells) to a Weibull (power) function, either the rate of failure or the impact of failure on mortality must increase as a power function. Moreover, according to the analyses presented here, the power of the relationship would vary in parallel with the acceleration of embryo growth. Suppose that defective parts have a constant time-dependent rate of failure, so that the number of failures (F_x) increases linearly with time x at rate f as in a Poisson process, hence F_x∝fx. If mortality rate were proportional to some power (k) of the number of failed parts, mortality would be proportional to (fx)^k, which is a power function of time. If k were directly related to the acceleration of growth of the embryo, the exponent β describing the rate of increase in mortality rate with age would vary in direct relation to embryo b. Thus, a simple model inversely relating quality control to relative growth rate, and further relating the rate of increase in mortality to the failure of defective parts, would make a direct connection between embryonic (and plausibly postnatal) growth and ageing. The mechanisms represented by such a model remain to be specified.

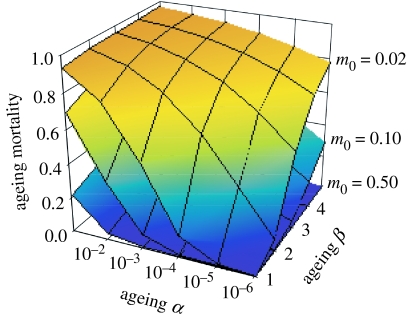
If the rate of increase in ageing related mortality (β) were determined during the embryonic growth period, the mortality rate of an individual at a particular age would be directly proportional to the scaling factor (α). To compensate an increase in the exponent β, the only option available is to decrease α, which would require the deployment of anti-ageing mechanisms by the individual, potentially throughout its lifetime. The strength of selection required to reduce α is directly related to the proportion (P_S) of total mortality that is ageing-related (Ricklefs 1998), $P_{\text{s}}=\int \alpha x^{\beta }{l}_x\text{d}x(x=0\text{to}\infty )$ (figure 5). In natural populations, estimated P_S varies from less than 1% of total mortality in species with high extrinsic mortality rates to more than 80% in species, such as pelagic seabirds and large mammals, which have low extrinsic mortality. Such high proportions of ageing-related mortality indicate limits to the evolutionary response in the rate of senescence at very high longevities (Ricklefs 1998). Indeed, such longevity evidently could be achieved only with low values of ageing β and, consequently, with low values of embryo b, which are permitted by the long incubation or gestation periods of these species.

Figure 5.

The proportion of a population's mortality due to ageing-related causes (P_S) indicates the strength of selection to extend lifespan by delaying senescence. P_S increases with lower extrinsic mortality (and longer lifespan). The combination of a steep increase in mortality at higher values of α and β with high P_S in long-lived birds (low m₀, uppermost surface) suggests that further reduction of ageing-related mortality is strongly constrained.

Empirical evidence indicates a strong relationship between the acceleration of embryo growth rate and the acceleration of ageing-related mortality in birds and mammals. This relationship suggests that more rapid embryo growth produces lower-quality adult individuals, at least with respect to the acceleration of mortality rate late in life and consequently reduced longevity. Related to this point, comparative data indicate that resistance to some types of parasites—an indicator of immune system function—increases with the length of the embryo development period (Ricklefs 1992). If ageing-related mortality depended upon the failure of parts of the organism, either the number of failures would have to increase as a power function of age or the impact on mortality would have to increase as a power function of the number of failures, to produce a Weibull model of ageing-related mortality. In either case, the value of this exponent is influenced by the rate of acceleration of embryo growth through unknown mechanisms that influence the quality of the individual throughout its life. If this functional connection between embryo growth and ageing involved mechanisms for detecting and correcting errors in the replication of DNA or damage incurred in other macromolecules, the effectiveness of these quality control mechanisms would appear to decrease with increasing embryo growth rate. Regardless of the mechanisms involved, the balancing fitness consequences of patterns of embryo growth and ageing-related mortality suggest an optimum rate of acceleration of both functions that varies according to the time-dependent mortality suffered during the embryo and adult stages of the life cycle.

Supplementary Material

Sources of data and estimated parameters for birds and mammals

Literature sources of embryo growth data for birds and mammals, and tables listing the parameters of power functions fitted to the relationships between embryo mass and age and between adult mortality rate and age.