Evolutionary patterns in early tetrapods. I. Rapid initial diversification followed by decrease in rates of character change

Abstract

Although numerous studies have examined morphological diversification during major radiations of marine taxa, much less attention has been paid to terrestrial radiations. Here, we examine rates of character change over phylogeny and over time for Palaeozoic limbed tetrapods. Palaeozoic tetrapods show significant decreases in rates of character change whether the rate is measured per sampled cladistic branch or per million years along phylogeny. Given changes per branch, rates decrease significantly from the Devonian through the Pennsylvanian, but not from the Pennsylvanian through the Permian. Given changes per million years, rates decrease significantly over each boundary, although the decrease is least significant over the Pennsylvanian–Permian boundary. Decreasing rates per million years through the Permian might be an artefact of the method being able to ascribe longer durations to Permian branches than to Carboniferous ones; however, it is difficult to ascribe the general pattern of decreasing rates of change over time to sampling biases or methodological biases. Thus, the results implicate biological explanations for this pattern.

1. Introduction

Morphological diversification studies are fundamental to investigations of macroevolutionary patterns and their underlying causes. Large-scale analyses of morphological changes and diversification rates of terrestrial organisms lag considerably behind analyses of comparable magnitude in marine organisms. Conspicuously absent from the literature is a study of disparity and diversification rates over time for Palaeozoic tetrapods. The early diversification of tetrapods from the Late Devonian onwards coincides with the emergence of a new body plan and the colonization of terrestrial habitats. Moreover, Palaeozoic tetrapods underwent two subsequent episodes of major diversification: a stem-lissamphibian radiation within the aquatic habitats occupied by basal tetrapods and a stem-amniote radiation marking the first expansion into fully terrestrial habitats. Thus, we have reasons to expect high rates of morphological change not only early in tetrapod evolution, but also later in the clade's history (Simpson 1944; Valentine 1978, 1980; Valentine & Erwin 1987; Gould 1989).

There are further theoretical and practical reasons for investigating rates of morphological change among Palaeozoic tetrapods. First, empirical evidence suggests that the morphological variation among limb characters was unusually high very early in tetrapod history (Coates & Clack 1990; Coates et al. 2002; Shubin et al. 2004). Second, although numerous studies of morphological diversification (i.e. rates of change and ranges of morphological variation) have focused on clade origins (e.g. Foote 1997), the association between terrestrialization and morphological diversification has been explored in detail only for arthropods (Stockmeyer Lofgren et al. 2003). Many hypotheses predict that the colonization of new ecospace induces high rates of morphological change followed by reduced rates of change as ecological partitioning becomes more specific (e.g. Valentine 1980). Third, Ruta & Coates's (2006) phylogenetic analyses of Palaeozoic tetrapods provide both the morphological data and the phylogenetic framework for assessing rates of character change (e.g. Briggs et al. 1992; Anstey & Pachut 1995; Wagner 1995a,b, 1997; Sidor & Hopson 1998; Smith & Lieberman 1999; Cotton 2001). The latter issue is especially important because it allows us to test the statistical null hypothesis of consistent rates across phylogeny, and thus to assess whether over-attention to particular novelties (e.g. early tetrapod limb features) exaggerates depictions of overall morphological change (e.g. Wills et al. 1994).

Finally, two aspects of tetrapod diversification allow us to contrast predictions of intrinsic constraint (i.e. developmental or genetic) or ecological restriction (i.e. filling of general ecospace) models for reducing rates of morphological change. First, Ruta & Coates's (2006) results suggest that post-Devonian tetrapods evolved from a single Late Devonian/Early Carboniferous taxon. This phylogenetic ‘bottleneck’ (sensu Jablonski 2002) yields a second radiation into similar (i.e. semi-aquatic) ecospace; thus, if ecology alone is responsible for rates of morphological change, then we expect Early Carboniferous rates to mimic Devonian rates; however, if intrinsic constraints accumulated in the interim, then we do not. Second, the diversification of stem- and basal crown-amniotes in the Late Carboniferous represents a second invasion into a new ecospace (i.e. fully terrestrial environments). If ecological restrictions affect rates of morphological change more strongly than intrinsic constraints do, then we expect to see additional high rates of change of tetrapod diversification. Conversely, if intrinsic constraints accumulated prior to this radiation, then we might see increased disparity due to diversification, but no increase in rates.

2. Material and methods

(a) Tetrapod data

Our analyses use a dataset of 339 skeletal characters coded for 95 species of Palaeozoic tetrapods (19 stem-tetrapods; 51 stem-amniotes; 3 crown-amniotes; 22 stem-lissamphibians). They are grouped by age as follows: 6 Middle–Late Devonian; 19 Lower Carboniferous; 31 Upper Carboniferous; 6 Upper Carboniferous and/or Lower Permian (this category includes taxa with either extended stratigraphic range or uncertain stratigraphic position); 30 Lower Permian; 3 Upper Permian.

The present study excludes early fish-like members of the tetrapod total group (see Ruta et al. 2003; Laurin & Anderson 2004) because they are ecologically and structurally different from limbed members which form the focus of our investigation. We also exclude Stereospondyli, a large monophyletic radiation of (mostly) Upper Permian and Lower Triassic temnospondyls (Schoch & Milner 2000; Stayton & Ruta 2006). Again, because our primary focus is on contrasting the initial diversification of Palaeozoic tetrapod diversity with subsequent diversifications, exclusion of stereospondyls should not affect our major conclusions. If their eventual inclusion did affect our results then this probably would reflect unusual features about stereospondyl evolution rather than about Palaeozoic tetrapod evolution.

(b) Rates of character change

We assess frequencies of change using a model tree (Ruta & Coates 2006), obtained from a maximum parsimony analysis in PAUP^* v. 4.0b10 (Swofford 2002). We treated all characters as equally informative and assumed unordered evolution among states. The analysis produced 324 shortest trees (1584 steps; Consistency Index=0.22; Retention Index=0.67; Rescaled Consistency Index=0.15). We present results based exclusively upon the first tree output by PAUP^*, but identical results are obtained when other trees are used. Minimum steps parsimony assumes that character change is equally probable on all tree branches (Edwards & Cavalli-Sforza 1964). This will bias our results only if there is a temporal trend towards declining preservation rates, such that (say) Pennsylvanian and Permian branches span more evolution than do Devonian and Mississippian branches: if so, then the null hypothesis is that there should be a higher probability of change on Pennsylvanian and Permian branches than on Devonian and Mississippian branches rather than equal probabilities as assumed by parsimony. Fortunately, studies (e.g. Smith et al. 1992; Sidor & Hopson 1998) show that parsimony reconstructions of changes along tree branches correlate well with the minimum temporal durations of those branches. Thus, if poor sampling results in phylogenetic branches spanning long time periods then those branches should show many changes, which, in turn, biases our results either towards the null hypothesis or suggesting high rates of change late in the clade's history, well after the initial invasion of new ecospace. Parsimony still will miss multiple changes of individual characters along long branches. However, we compensate for this in part by examining both rates of change per branch and rates of change per time (see below).

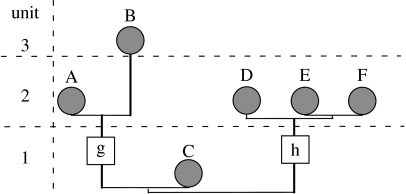
We describe rates of change using the patristic dissimilarity of each branch (Wagner 1997). This is simply the phenetic dissimilarity between the reconstructed ancestor and either an observed taxon or another reconstructed ancestor. Thus, ‘rate’ here reflects (in part) the frequency of character change per branch. We use this as one approximation of rates of change. When examining the correlation between time and rate, we assigned changes to the latest possible chronostratigraphic unit. For observed taxa, this is the first occurrence. For reconstructed ancestors (e.g. g and h; figure 1), this is the unit in which the first sampled descendant appears (e.g. unit 2 in both cases), as the ancestral morphotype must have evolved by then. (Note figure 1 illustrates g and h in unit 1 simply because of space restrictions.)

Figure 1.

Example phylogeny. A–F represent observed taxa, g and h represent reconstructed ancestral morphotypes. ‘Unit’ refers to chronostratigraphic units.

Of course, the duration over which morphological changes accumulated affects expected amounts of change given either continuous change (i.e. where change is per unit time) or punctuated change (i.e. where the number of events inducing change is affected by branch duration). Therefore, we also estimate rates of change per million years (Myr) by estimating the temporal duration of each branch and then dividing each patristic dissimilarity by that estimated duration. This is straightforward for taxa that appear in a younger chronostratigraphic unit than their closest relative (e.g. taxon B in figure 1); here, a range extension (sensu Smith 1988) provides a duration over which taxon B could accumulate apomorphies. Here, we would posit that B appeared in the middle of unit 3 and assume that it accumulated apomorphies from the middle of chronostratigraphic unit 2 to that time. We based durations on Gradstein et al. (2004).

Duration estimation becomes less straightforward in cases where the model phylogeny does not specify a minimum divergence time. For example, the model phylogeny in figure 1 does not necessitate branch lengths for taxa A, D, E, F or the common ancestor of E+F. Here, we assigned branch lengths within the range of possible divergence times that were proportional to the frequencies of character change. For example, taxon A and branch g accumulate changes over units 1 and 2. Suppose that taxon A had 10% of its characters change and branch g had 5% of its characters change; to maximize fit to the null hypothesis, we assumed that branch g accumulated changes over $5/(5+10)=33\%$ of the elapsed time, and that taxon A accumulated changes over 67% of the elapsed time between the occurrences of taxon A and taxon C (the immediate outgroup). In the cases where whole clades appear at once (e.g. clade DEF), then this averaging would apply to two nodes (branch h and the unlabelled E+F node) as well as taxa D–F. Thus, appearances of whole clades in one time interval did not induce infinite rates. Alternative approaches to approximating branch durations in these cases, such as ignoring apomorphies and dividing durations equally among the branches in question, generated very similar results and the same conclusions.

Unlike the distribution of traits on a phylogeny, the changes along branches need not reflect any temporal or serial autocorrelation (Felsenstein 1985). Correlation between rates and either time or adjacent branches will exist only if rates do typify periods of time (and thus portions of the phylogeny) and if particular rates typify particular intervals of time. The null hypothesis predicts that rates are uniform through time and thus through phylogeny. We used Kendall's rank correlation test to this hypothesis. Using rank orders avoids an assumption about a particular distribution (e.g. Gaussian) for the raw data and simply assesses whether relatively large and small changes are concentrated at opposite ends of the time-scale. Kendall's test also is well suited for binned data (such as numerous taxa in one chronostratigraphic unit), unlike other rank correlation tests such as Spearman's. We examine the correlation both for the entire time series, and also across pairs of periods (i.e. Devonian through Mississippian, Mississippian through Pennsylvanian and Pennsylvanian through Permian). The latter breakdown allows us to recognize particular intervals over which rate shifts might have occurred: for example, high rates in the Devonian followed by indistinguishable but lower rates in the Mississippian–Permian. A significant negative correlation is predicted by the hypotheses positing elevated rates of early change. Failure to reject the null hypothesis is consistent with the idea that a small number of novel characters exaggerates apparent morphological diversification among early tetrapods.

3. Results

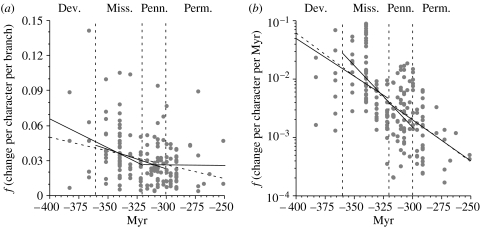
The model phylogeny implies significantly higher frequencies of character change early in tetrapod history than in the late Palaeozoic (figure 2; table 1). This is true given either the estimated change per branch (figure 2a) or estimated change per million years (figure 2b). Whereas rates per million years show significant decreases over each pair of adjacent periods, rates per branch show no significant change from the Pennsylvanian through the Permian (table 1), and simple regression suggests a levelling of rates over that time. Moreover, the correlation between time and change per million years is the weakest for those two intervals.

Figure 2.

Rates of character change (Δ). (a) Frequencies of character change per cladistic branch. Grey circles give only those branches that did not necessarily diverge prior to that interval; the corresponding τ and p are given in table 1. (b) Approximated frequencies of character change per million years (Myr) per branch. These are given on a log scale due to the apparent exponential decrease in rates. Partitions separate Devonian, Mississippian, Pennsylvanian and Permian branches. Dashed regression lines give the relationship between time and change for the entire study; solid lines give relationships between time and change over adjacent intervals. Time-scale from Gradstein et al. (2004).

Table 1.

Correlation between time and rate of change along tetrapod phylogeny using either raw patristic dissimilarity or estimated rates per million years (i.e. patristic dissimilarity divided by estimated branch duration). (τ gives Kendall's rank correlation coefficient.)

	rate per branch		rate per million years
	τ	p	τ	p
Devonian to Permian	−0.176	4×10−4	−0.427	1×10−17
Devonian to Mississippian	−0.210	0.005	−0.327	1×10−5
Mississippian to Pennsylvanian	−0.171	0.005	−0.385	3×10−10
Pennsylvanian to Permian	−0.033	0.627	−0.215	0.002

4. Discussion

Our estimates of rates of change per million years suggest that rates were approximately 10 times lower in the Permian than in the Devonian. If one reconstructed the phylogeny with basal branches 10 times longer than our analyses reconstruct them, then the difference between the Devonian and Permian would dissipate. Thus, one might wonder if sampling might account for this pattern. We consider this unlikely for the following reasons. Terrestrial ecosystems prior to the Middle Devonian are unlikely to be conducive to the existence of even amphibious vertebrates. Moreover, the earliest known closest relatives of tetrapods among ‘osteolepiform fishes’ do not appear until the late Early Devonian, i.e. about 20 Myr prior to the earliest limbed tetrapods (Ahlberg & Johanson 1998; Zhu & Ahlberg 2004). Because ‘osteolepiforms’ are paraphyletic relative to tetrapods, positing an earlier origin of the latter necessarily implies earlier origins for numerous other taxa in the marine realm. Finally, asserting additional tens of millions of years over which Devonian taxa might have evolved does nothing to explain the significant decrease in rates after the Devonian.

One might worry that including only the best-known Devonian tetrapods distorts apparent rates. However, fragmentary remains allow only a few characters to be compared, and the few differences used to recognize distinct taxa yield large pairwise dissimilarities. Thus, their inclusion probably would exaggerate the patterns illustrated here. Ultimately, one must invoke a ‘special pleading’ model in which the fossil record somehow selectively preserved outliers of Devonian tetrapod morphospace to attribute either the rate or disparity pattern to geological rather than biological factors.

Two fairly different rate metrics lead to almost identical conclusions. Alternative approaches (not discussed here) also yield almost identical conclusions. Thus, it is difficult to dismiss decreasing rates over time to methodological artefacts. The one finding that one might call into question is that rates of change per million years decreased from the Pennsylvanian through the Permian. Reconstructed lineage durations over which change might have occurred are not randomly distributed through time, with the longest durations occurring among Permian taxa (τ=0.392; p=10⁻¹⁴); however, the late appearing taxa necessarily are the ones with the longest possible range extensions, and error in phylogenetic reconstruction tends to exaggerate reconstructed ranges (Wagner 2000). Moreover, because we used parsimony optimizations, we could not account for multiple changes per individual character (Felsenstein 1973). Thus, this particular method probably is biased towards underestimating rates among the latest appearing taxa.

In the absence of geological or methodological explanations, we require biological explanations. Workers have offered two general non-exclusive hypotheses for accelerated morphological evolution: (i) reduced intrinsic constraints (e.g. developmental or phylogenetic) and (ii) reduced ecological restrictions (Valentine 1969, 1980). The initial burst of morphological diversification could easily represent ‘relaxation’ in both types of constraints. The decrease of rates in Mississippian suggests increased intrinsic constraints: if there was a phylogenetic bottleneck, then ecospace should have been nearly as available as it was in the Devonian. Moreover, the Pennsylvanian–Permian radiation of amniotes into fully terrestrial habitats does not induce an increase in rates, as the ecological restrictions hypothesis predicts. Note that there are many possible mechanisms for intrinsic constraints (see Wagner 2001), and future work might be able to test these models against one another. Moreover, even if invasion of new ecospace did not accelerate rates of character evolution among early amniotes, we cannot dismiss the idea that increasing ecological restrictions played a role in tetrapod evolution. In particular, the continued decrease of rates from the Mississippian into the Pennsylvanian is consistent with ecological restrictions playing some role in slowing rates of character change.

5. Conclusions

Tetrapods display decreasing rates of anatomical change over time. Although this pattern has been documented for marine taxa, this is the first time that it has been documented for terrestrial vertebrates. It is difficult to contrive a scenario in which the rate pattern is an artefact of poor sampling of the earliest tetrapods. The observed patterns are consistent with ideas about low intrinsic/extrinsic constraints early in the history of a major clade. Future studies on increasing constraints on particular aspects of the skeletal system might generate testable predictions for developmental and functional biologists to test.