Abstract
Residual force enhancement has been observed consistently in skeletal muscles following active stretching. However, its underlying mechanism(s) remain elusive, and it cannot be explained readily within the framework of the cross-bridge theory. Traditionally, residual force enhancement has been attributed to the development of sarcomere length non-uniformities. However, recent evidence suggests that this might not be the case. Rather, it appears that residual force enhancement has an active and a passive component. The active component is tentatively associated with changes in the cross-bridge kinetics that might be reflected in decreased detachment rates following active muscle stretching, while the passive component possibly originates from a structural protein, such as titin, whose stiffness might be regulated by calcium.
Background
When an actively contracting muscle is stretched, force increases quickly for the first part of the stretch and then increases more slowly (or might remain constant or even decrease) for the remainder of the stretch. This has been referred to as the force enhancement during stretch and has been observed for a long time (e.g. Fenn & Marsh, 1935; Hill, 1938). Force enhancement during stretch is well explained by the cross-bridge theory of muscle contraction (e.g. A. F. Huxley, 1957; H. E. Huxley, 1969; Huxley & Simmons, 1971), and the earliest description of the cross-bridge model (Huxley, 1957) was specifically designed to accommodate and predict the dynamic changes of muscle force during active shortening and stretching.
In addition to the force enhancement during stretch, the steady-state isometric force after stretch remains higher than the corresponding force obtained at the same length for a purely isometric contraction. This has been referred to as the steady-state or residual force enhancement after stretch, which is the focus of this review (Fig. 1).
Figure 1.
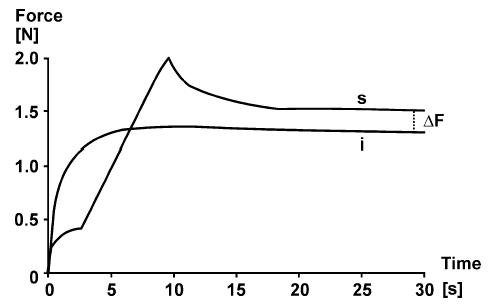
Force–time plots of an isometric contraction (i) of dogfish muscle at 34 mm length, and an isometric stretch–isometric contraction (s) in which the muscle is stretched from 29 to 34 mm (about 15% stretch) at time 3–9 s. Note that the steady-state isometric force following stretch is greater than the corresponding force of the purely isometric contraction at the same length. This so-called ‘steady-state’ or ‘residual force enhancement’ is indicated as ΔF. Muscle temperature 0°C, stimulation frequency 5 Hz. Adapted from Abbott & Aubert (1952) with permission.
In contrast to the force enhancement during stretch, the residual force enhancement after stretch is not explained by the classic cross-bridge theory, as the steady-state isometric force of a muscle should be independent of its contractile history (e.g. Huxley, 1957; Huxley & Simmons, 1971), and for a given amount of activation, should only depend on its length, or more precisely, the overlap between actin and myosin filaments (e.g. Gordon et al. 1966).
Abbott & Aubert (1952) were the first to systematically describe residual force enhancement in whole muscle preparations from frog and toadfish more than half a century ago. They found that force enhancement occurred at all lengths tested, including the ascending, plateau and descending limb portions of the force–length relationship, and that force enhancement increased systematically with the magnitude of stretch. Sugi (1972) studied residual force enhancement in small fibre bundles of frog semitendinosus, and found, in agreement with Abbott & Aubert's (1952) work in whole muscle preparations, that force enhancement depended on the magnitude of stretch. The first systematic studies on force enhancement in single fibre preparations were made by Edman et al. (1978, 1982) who initially reported that force enhancement exceeded the isometric forces at the plateau of the force–length relationship (1978), but then changed their interpretation (1982) in view of evidence from force transients that were followed for up to 6 s following the end of active stretching. Edman et al. (1982) also observed that shortening a fibre prior to stretch gave essentially the same dynamic force transients during stretch and the same residual force enhancement after stretch as a fibre that was stretched without prior shortening, thereby eliminating the idea that a passive structure might engage upon activation and contribute to the observed residual force enhancement. However, more recent experiments in whole muscle and single fibre preparations demonstrated that forces in the enhanced state could exceed the steady-state isometric forces at the plateau of the force–length relationship (Peterson et al. 2004; Schachar et al. 2004) and that there is a passive component that contributes to the residual force enhancement, at least at long muscle and fibre lengths (Herzog & Leonard, 2002; Rassier et al. 2003). These recent studies therefore questioned previous observations and earlier proposals as to the possible mechanism underlying residual force enhancement.
Basic questions
Abbott & Aubert's (1952) work was followed by many similar experiments and, independent of the muscles used or the structural level of investigation, most studies, with very few exceptions (e.g. Brown & Loeb, 2000), concur that there is a residual force enhancement following muscle stretching. The detailed properties of this force enhancement are controversial, and the mechanisms remain a matter of debate. From the research conducted in this area, three questions seem most fundamental to understanding the nature of force enhancement: (i) Is force enhancement caused by the development of sarcomere length non-uniformities during muscle stretch? (ii) Is force enhancement associated with the molecular mechanism of contraction, and thus, should it be reflected in the cross-bridge thinking? (iii) Can force enhancement be explained by the engagement of a passive structural element, independent of cross-bridge action and structural non-uniformities? These three questions will be discussed in the following.
Is force enhancement caused by sarcomere length non-uniformities?
Residual force enhancement following active muscle stretching (Abbott & Aubert, 1952) had been observed prior to the formulation of the sliding filament (Huxley & Niedergerke, 1954; Huxley & Hanson, 1954) and the cross-bridge model (Huxley, 1957), but was not considered in the formulation of these theories. Therefore, a mechanism that was not associated with the cross-bridge kinetics would be convenient and would not challenge existing ideas on the mechanism of muscle contraction. Within this framework, the idea that residual force enhancement was caused by sarcomere length non-uniformities caused by active stretch of muscle, an idea previously used to explain force creep at long muscle length (Hill, 1953), gathered immediate support. Specifically, Morgan (1990, 1994) proposed that upon stretch on the ‘unstable’ descending limb of the force–length relationship (Hill, 1953), a small number of weak sarcomeres would be stretched beyond myofilament overlap, and would be held at long lengths by passive forces exclusively, while most of the remaining sarcomeres would hardly be stretched at all, and therefore would exhibit the isometric forces essentially present prior to stretch, rather than the expected lower forces had all sarcomeres been stretched uniformly (Fig. 2). Theoretical models of unstable sarcomeres arranged in series (resembling a myofibril) could produce the observed residual force enhancement and other phenomena of actively stretched muscles (Morgan, 1990; Zahalak, 1997; Denoth et al. 2002), and experimental observations of sarcomere length non-uniformities following stretch of isolated muscles gave further support to this theory (Talbot & Morgan, 1996).
Figure 2. Schematic illustration of force enhancement following stretch according to the sarcomere length non-uniformity theory.
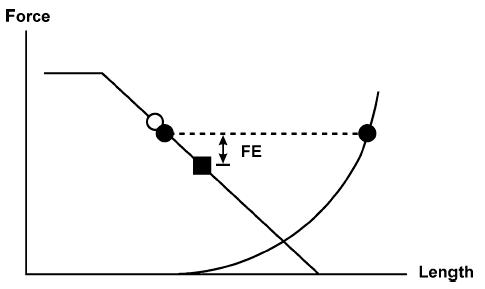
A muscle is stretched on the descending limb of the force–length relationship from an initial mean sarcomere length (○) to a final mean sarcomere length (▪). During stretching, it is assumed that a few sarcomeres are stretched much more than average (• right) while most sarcomeres are stretched less than average (• left). The sarcomeres that are stretched less than average are stronger than the average sarcomere would be because of the slope of the force–length relationship. The sarcomeres that are stretched more than average become weaker initially, lose overlap between actin and myosin, and are ‘caught’ by passive elements. They elongate until the passive force is at equilibrium with the force of the ‘short’, actively force-producing sarcomeres. This force equilibrium (dashed line connecting the filled circles) is greater than the expected force at the final average sarcomere length. If we assume now that in a purely isometric contraction, sarcomere lengths are ‘uniform’, then this mechanism could potentially account for the experimentally observed force enhancement (FE).
The sarcomere length non-uniformity theory allows for precise predictions. Among them, two seem crucial: (i) if residual force enhancement is caused by the instability of sarcomeres and the associated development of sarcomere length non-uniformities, force enhancement should not occur on the ascending limb of the force–length relationship, as the positive force–length slope of that portion of the relationship conveys an inherent (mathematical) stability to the lengths of serially arranged sarcomeres, and (ii) under no circumstances should the steady-state force following active stretch exceed the purely isometric forces observed at the plateau of the force–length relationship (Morgan, 1994; Morgan et al. 2000).
There is an abundance of experimental findings demonstrating residual force enhancement on the ascending limb of the force–length relationship in whole muscle preparations (Abbott & Aubert, 1952; Cook & McDonagh, 1995; De Ruiter et al. 2000; Herzog & Leonard, 2002). However, results from whole muscle experiments might be criticized as a whole muscle could show increasing forces with increasing length (i.e. ascending limb behaviour), but because of fibre length non-uniformities, a small percentage of fibres could be on the descending limb of the force–length relationship producing the observed force enhancement. Research on frog single fibres or fibre bundles provides evidence of small but consistent residual force enhancement on the ascending part of the force–length relationship (Sugi, 1972; Peterson et al. 2004) when stretch conditions are optimized (Fig. 3).
Figure 3. Mean ascending limb portion of the force–length relationship (○, continuous line) and steady-state forces (mean ± s.d.) following 10% stretching (□) of single fibres (n = 10) from lumbrical muscles of frog (Rana pipiens).
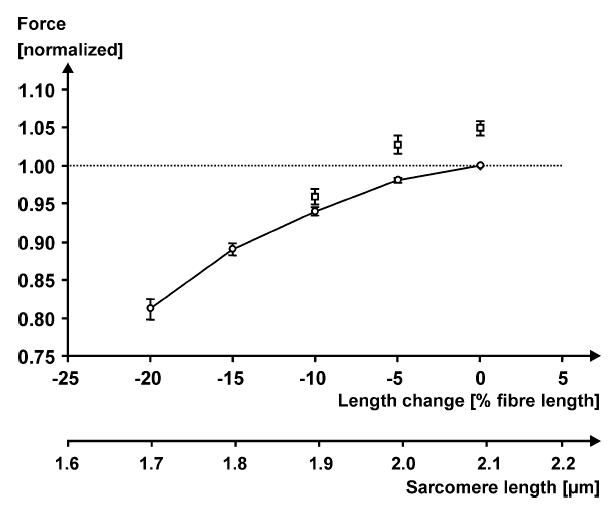
Forces are normalized with respect to the maximal isometric force on the plateau of the force–length relationship (dotted horizontal line) for comparison across fibres. Fibre lengths were normalized relative to the optimal fibre length (0%), i.e. the length at which the purely isometric force was greatest. Note that there is a small but consistent amount of force enhancement on the ascending part of the force–length relationship, and that forces in the enhanced state are greater than the isometric force at optimal length for some of the experimental conditions. Temperature 8°C, stimulation frequencies for the 10 fibres ranged from 23 to 26 Hz (Peterson et al. 2004).
Similarly, residual force enhancement above the purely isometric steady-state forces obtained on the plateau of the force–length relationship have been obtained in whole muscle (Abbott & Aubert, 1952; De Ruiter et al. 2000; Lee & Herzog, 2002; Schachar et al. 2004) and single fibre preparations (Rassier et al. 2003; Peterson et al. 2004) (Figs 3 and 4). In summary, it appears that crucial predictions of the sarcomere length non-uniformity theory are violated, suggesting that sarcomere length non-uniformity is probably not the single cause for force enhancement, although it cannot be excluded that it might contribute to the experimentally observed force enhancement under some conditions. This conclusion is further supported by studies in which force enhancement was observed despite careful enforcement of sarcomere length uniformity in single fibre preparations (Edman et al. 1982).
Figure 4.
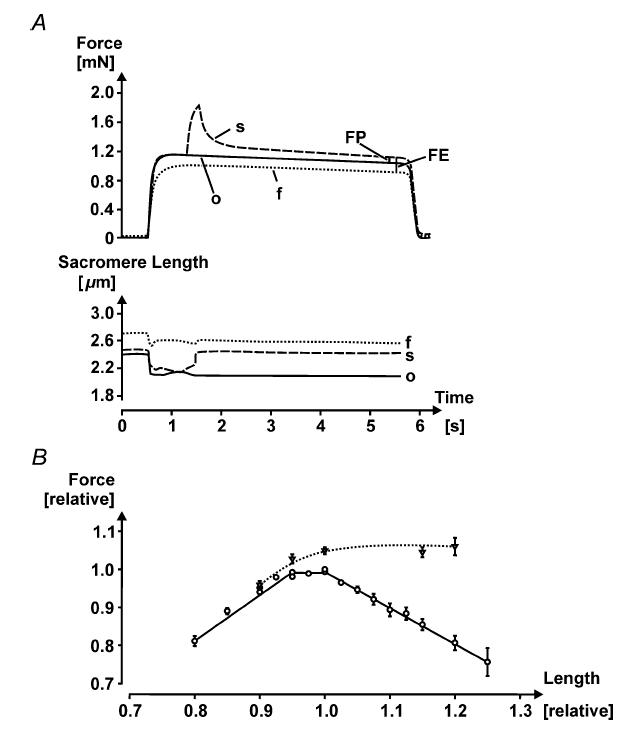
A, force–time and corresponding sarcomere length–time plots for an isometric contraction performed at optimal sarcomere length (○), an isometric contraction performed at the final length (f, about 2.6 µm), and an isometric stretch–isometric contraction (s). The stretch was made from optimal to the final sarcomere length (stretch magnitude of about 16%). Note the force enhancement, evaluated by the difference in isometric force at the final length and the steady-state isometric force following stretch at the same length (FE), and the enhanced force above the isometric plateau force (FP), demonstrating that force enhancement can exceed the isometric forces at optimal sarcomere length. Note also that the sarcomere length following stretch (s) is not identical to the final sarcomere length (f), despite the fibre length being the same, possibly because force in the enhanced state (s) is greater than the corresponding purely isometric force (f). Finally, note that ‘isometric’ refers to the idea that fibre length is held constant therefore sarcomere length can change as shown in (A) upon contraction and force rise. Single fibre from lumbrical muscle of frog, Rana pipiens, temperature 12°C, stimulation frequency 45 Hz. B, normalized force–length relationship of single fibres from frog, Rana pipiens (circles, continuous line), and the corresponding isometric, steady-state forces following 10% stretching (triangles, dotted line). Note that there is a small but consistent force enhancement on the ascending part of the force–length relationship (n = 10 fibres from lumbrical muscles) on the plateau (n = 16; 10 lumbrical fibres and six fibres from tibialis anterior), and on the descending limb of the force–length relationship (n = 22 fibres from lumbrical muscles).
Is force enhancement caused by cross-bridge action?
There are two conceptual ways in which force enhancement could be caused by cross-bridge action: either by a stretch-induced increase in the proportion of attached cross-bridges or by an increase in the average force per cross-bridge. Edman et al. (1982), working with single fibres from frog tibialis anterior, argued that force enhancement was not a property of the cross-bridges because if it was, it should occur at all muscle lengths, whereas they did not observe it on the ascending limb of the force–length relationship. Furthermore, they found that forces in the enhanced state never exceeded the purely isometric forces at the plateau of the force–length relationship, thereby eliminating the idea that there was a recruitment of additional contractile material that caused force enhancement. These two observations were supported by others who did not find force enhancement on the ascending or plateau regions of the force–length relationship in cat caudofemoralis and soleus muscles (e.g. Brown & Loeb, 2000; Morgan et al. 2000).
However, as discussed above, there is strong evidence that, for specific experimental conditions, force enhancement occurs consistently on the ascending part of the force–length relationship, and forces following active muscle stretch can exceed the purely isometric forces at the plateau of the force–length relationship. Therefore, it seems possible that force enhancement is associated with the recruitment of additional contractile material.
One way to determine if force enhancement is associated with an increase in the proportion of attached cross-bridges is to measure stiffness in the enhanced state and the corresponding reference configuration, while carefully accounting for stiffness originating from sources other than the cross-bridges. Herzog & Leonard (2000) measured stiffness in the cat soleus following 2–8 mm stretches (i.e. about 2–8% of total muscle length) and compared the values obtained when residual force enhancement was achieved to the corresponding values of the isometric reference contractions. They found an average increase in stiffness in the enhanced state of about 6% indicating that the residual force enhancement might be partly caused by an increase in the proportion of attached cross-bridges. However, Sugi & Tsuchiya (1988) did not find such an increase in stiffness in single frog fibres in the force-enhanced compared to the isometric reference state, thereby leaving this topic unresolved.
Further support that force enhancement may be associated with cross-bridge function comes from studies showing that force enhancement increases with decreasing temperature (Sugi, 1972) and increasing the proportion of weakly to strongly bound cross-bridges through 2,3-butanedione monoxime (BDM) (Rassier & Herzog, 2004a). These results suggest that force enhancement might be accomplished by a stretch-induced facilitation of a transition of weakly to strongly bound cross-bridges. Furthermore, the rate of force relaxation has been found to decrease with increasing force enhancement (Rassier & Herzog, 2005), suggesting a direct link between force enhancement and the kinetics of cross-bridge action. Force enhancement, in conjunction with an increase in stiffness and a decrease in the rate of force relaxation, could be explained within the framework of the cross-bridge theory by a stretch-induced decrease in the cross-bridge detachment rate.
Finally, it has been observed in skinned mammalian muscle fibres that force is increased following quick stretches (< 1 ms) of small amplitude (about 0.2% of fibre length) after an initial drop in force. This so-called ‘stretch activation’ phenomenon is well correlated with the isoforms of the myosin heavy chains (Galler et al. 1994; Andruchov et al. 2004), and although it is observed shortly after stretch (for fast myosin isoforms typically less than 100 ms) and not at steady state, these results provide evidence that stretch activation is directly associated with the cross-bridge kinetics and could play a role in the residual force enhancement discussed here. However, the detailed relationship that may exist between stretch activation and steady-state force enhancement needs systematic investigation before this issue can be resolved satisfactorily. In summary, observations of steady-state forces following stretch that exceed the purely isometric forces at the plateau of the force–length relationship, indications of increased stiffness in the force-enhanced compared to the isometric reference state, evidence of stretch activation, and predictable changes in residual force enhancement in preparations in which the cross-bridge kinetics have been manipulated, all suggest that the residual force enhancement is directly associated with cross-bridge actions.
Is force enhancement caused by a passive structural element?
Residual force enhancement increases with increasing stretch magnitudes, at least within a certain range (e.g.Abbott & Aubert, 1952; Edman et al. 1978, 1982) but has been reported to be nearly independent of stretch speed (Edman et al. 1982; De Ruiter et al. 2000) for many experimental conditions. These results provided the basis for suggesting that residual force enhancement might be caused by the recruitment of a passive elastic element in parallel with the contractile system at the onset of activation (Edman et al. 1978,1982; Noble, 1992; Edman & Tsuchiya, 1996; De Ruiter et al. 2000). If so, it was argued that shortening of an activated muscle prior to stretching would decrease force enhancement in a shortening magnitude-dependent manner. Edman et al. (1982) reported that shortening preceding a given stretch gave essentially the same residual force enhancement in isolated frog fibres as when the stretch was not preceded by shortening, thus apparently disproving this idea (Fig. 5A). In contrast, Herzog & Leonard (2000) in cat soleus, and Rassier & Herzog (2004b) in single frog fibres found that shortening preceding stretch reduced the residual force enhancement in a dose-dependent manner (Fig. 5B).
Figure 5.
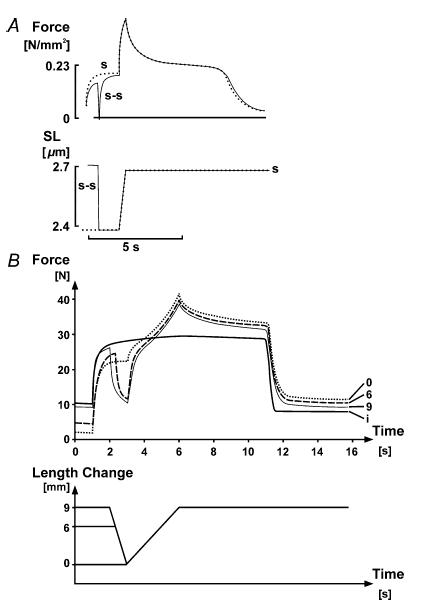
A, force–time and sarcomere length–time plots of an isometric–stretch–isometric contraction (s), and an isometric–shortening–stretch–isometric contraction (s-s). The stretch and shortening–magnitudes were similar and corresponded to about 14% of the optimal fibre length. Note that the (s) and (s-s) contractions virtually overlap during the stretch and the isometric phase following stretch, thereby indicating that shortening preceding stretch did not affect the stretch-induced force enhancement, and that there is no engagement of a passive force at the instant of activation. Single frog fibre at 3.8°C. Adapted from Edman et al. (1982) with permission. B, force–time and muscle length–time plots of an isometric reference contraction (i) and three identical 9 mm amplitude stretch contractions preceded by 0, 6 and 9 mm of shortening, respectively (0, 6 and 9, respectively). Optimal length for this muscle was about 100 mm, therefore the stretch amplitude was about 9% of muscle length and the shortening magnitudes were approximately 6 and 9% of optimal muscle length. Note that the total and the passive force enhancement decrease in a dose-dependent manner with the magnitude of shortening preceding stretch, thereby indicating that shortening reduces the stretch-induced total and passive force enhancement, and that the engagement of a passive elastic element upon muscle activation is a real possibility for contributing to force enhancement. Whole cat soleus at 35°C, stimulation frequency 30 Hz.
Furthermore, Herzog & Leonard (2002) demonstrated that when cat soleus is stretched on the descending limb of the force–length relationship, it produces a greater passive force (after the muscle has been deactivated) than a muscle activated purely isometrically at the corresponding length or a muscle stretched passively to that same length (Fig. 6), thereby providing evidence that there might be a recruitment of some passive force during active stretch of muscles. Inspection of previously published stretch records from different laboratories using different preparations revealed that this passive force enhancement had been present many times (e.g. Edman et al. 1982, their Fig. 6; Josephson & Stokes, 1999, their Fig. 1; Morgan et al. 2000, their Fig. 5B) before it was first noticed and analysed (Herzog & Leonard, 2002).
Figure 6. Force–time plots of an isometric reference contraction (i), and isometric stretch–isometric test contractions performed passively (p) and actively (a).
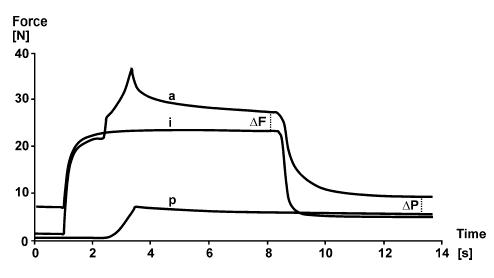
The active and passive stretches were identical (9 mm or about 9% of optimal muscle length). Note the force enhancement following active stretch (ΔF), and the increased passive force (ΔP) following deactivation of the actively stretched muscle compared to the passively stretched muscle and the isometric reference contraction at the corresponding length. Whole cat soleus, temperature 35°C, stimulation frequency 30 Hz.
The idea of a passive element contributing to the total force enhancement is strengthened by studies on single frog fibres by Bagni et al. (2002, 2004) who demonstrated experimentally that there is a non-cross-bridge-dependent stiffness (i.e. the stiffness changes were largely independent of force) that contributed to the forces during and after stretch. They showed that this increased stiffness arises from an elastic element and is affected by calcium concentration. It appears that the passive component of the residual force enhancement (Herzog & Leonard, 2002) and the passive stretch-induced increase in stiffness (Bagni et al. 2002,2004) might be caused by the same structure. Since the passive stiffness increase is calcium dependent, and the passive force enhancement is only observed after active, but not passive, stretching, it appears that there might be a passive structural component whose stiffness changes in a stretch- and calcium-dependent manner. Given its properties, the molecular spring titin seems a possible candidate for the passive force enhancement.
Titin is a structural protein spanning the half-sarcomere, and it provides much of the passive force in isolated myofibrils (Horowits et al. 1989). It acts as a molecular spring whose characteristic length and stiffness change with the unfolding of molecular bonds in the so-called immunoglobulin domain (Kellermayer et al. 1997; Rief et al. 1997; Marszalek et al. 1999). Recent observations suggest that titin's stiffness changes with calcium concentration either by changing its interaction with actin or by affecting its characteristic length (Tatsumi et al. 2001; Yamasaki et al. 2001; Labeit et al. 2003).
In summary, there is good evidence that part of the residual force enhancement originates from a passive structural component. However, this passive component only takes effect at long muscle length (Herzog & Leonard, 2002), and is always smaller than the total residual force enhancement; therefore it cannot be the sole mechanism.
Concluding remarks
Residual force enhancement appears to be a property of all muscles and preparations ranging from single myofibrils to whole muscles. Force enhancement increases with increasing stretch magnitude, is associated with a passive component at long muscle length, and might be associated with an increase in non-cross-bridge-derived stiffness, although this is a point of controversy. Force enhancement is observed at all muscle lengths (if appropriate stretch conditions are imposed) and force in the enhanced state can exceed the peak isometric forces at the plateau of the force–length relationship.
Based on these observations, we suggest that force enhancement has an active and a passive component. The active component appears to be associated with actin–myosin interactions rather than the development of sarcomere length non-uniformities. The passive component appears to be ‘engaged’ at activation and to depend on calcium concentration. The molecular spring titin seems a prime candidate for contributing to the passive force enhancement at long muscle length.
References
- Abbott BC, Aubert XM. The force exerted by active striated muscle during and after change of length. J Physiol. 1952;117:77–86. [PMC free article] [PubMed] [Google Scholar]
- Andruchov O, Andruchova O, Wang Y, Galler S. Kinetic properties of myosin heavy chain isoforms in mouse skeletal muscle: comparison with rat, rabbit, and human and correlation with amino acid sequence. Am J Physiol Cell Physiol. 2004;287:C1725–C1732. doi: 10.1152/ajpcell.00255.2004. [DOI] [PubMed] [Google Scholar]
- Bagni MA, Cecchi G, Colombini B, Colomo F. A non-cross-bridge stiffness in activated frog muscle fibers. Biophys J. 2002;82:3118–3127. doi: 10.1016/S0006-3495(02)75653-1. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Bagni MA, Colombini B, Geiger P, Berlinguer PR, Cecchi G. Non-cross-bridge calcium-dependent stiffness in frog muscle fibers. Am J Physiol Cell Physiol. 2004;286:C1353–C1357. doi: 10.1152/ajpcell.00493.2003. [DOI] [PubMed] [Google Scholar]
- Brown IE, Loeb GE. Measured and modeled properties of mammalian skeletal muscle: III. The effects of stimulus frequency on stretch-induced force enhancement and shortening-induced force depression. J Muscle Res Cell Motil. 2000;21:21–31. doi: 10.1023/a:1005619014170. [DOI] [PubMed] [Google Scholar]
- Cook CS, McDonagh MJN. Force responses to controlled stretches of electrically stimulated human muscle–tendon complex. Exp Physiol. 1995;80:477–490. doi: 10.1113/expphysiol.1995.sp003862. [DOI] [PubMed] [Google Scholar]
- Denoth J, Stussi E, Csucs G, Danuser G. Single muscle fibre contraction is dictated by inter-sarcomere dynamics. J Theor Biol. 2002;216:101–122. doi: 10.1006/jtbi.2001.2519. [DOI] [PubMed] [Google Scholar]
- De Ruiter CJ, Didden WJM, Jones DA, De Haan A. The force-velocity relationship of human adductor pollicis muscle during stretch and the effects of fatigue. J Physiol. 2000;526:671–681. doi: 10.1111/j.1469-7793.2000.00671.x. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Edman KAP, Elzinga G, Noble MIM. Enhancement of mechanical performance by stretch during tetanic contractions of vertebrate skeletal muscle fibres. J Physiol. 1978;281:139–155. doi: 10.1113/jphysiol.1978.sp012413. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Edman KAP, Elzinga G, Noble MIM. Residual force enhancement after stretch of contracting frog single muscle fibers. J Gen Physiol. 1982;80:769–784. doi: 10.1085/jgp.80.5.769. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Edman KAP, Tsuchiya T. Strain of passive elements during force enhancement by stretch in frog muscle fibres. J Physiol. 1996;490:191–205. doi: 10.1113/jphysiol.1996.sp021135. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Fenn WO, Marsh BO. Muscular force at different speeds of shortening. J Physiol. 1935;85:277–297. doi: 10.1113/jphysiol.1935.sp003318. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Galler S, Schmitt TL, Pette D. Stretch activation, unloaded shortening velocity, and myosin heavy chain isoforms of rat skeletal muscle fibres. J Physiol. 1994;478:513–521. doi: 10.1113/jphysiol.1994.sp020270. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Gordon AM, Huxley AF, Julian FJ. The variation in isometric tension with sarcomere length in vertebrate muscle fibres. J Physiol. 1966;184:170–192. doi: 10.1113/jphysiol.1966.sp007909. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Herzog W, Leonard TR. The history dependence of force production in mammalian skeletal muscle following stretch-shortening and shortening-stretch cycles. J Biomech. 2000;33:531–542. doi: 10.1016/s0021-9290(99)00221-3. [DOI] [PubMed] [Google Scholar]
- Herzog W, Leonard TR. Force enhancement following stretching of skeletal muscle: a new mechanism. J Exp Biol. 2002;205:1275–1283. doi: 10.1242/jeb.205.9.1275. [DOI] [PubMed] [Google Scholar]
- Hill AV. The heat of shortening and the dynamic constants of muscle. Proc R Soc Lond B Biol Sci. 1938;126:136–195. doi: 10.1098/rspb.1949.0019. [DOI] [PubMed] [Google Scholar]
- Hill AV. The mechanics of active muscle. Proc R Soc Lond B Biol Sci. 1953;141:104–117. doi: 10.1098/rspb.1953.0027. [DOI] [PubMed] [Google Scholar]
- Horowits R, Maruyama K, Podolsky RJ. Elastic behaviour of connectin filaments during thick filament movement in activated skeletal muscle. J Cell Biol. 1989;109:2169–2176. doi: 10.1083/jcb.109.5.2169. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Huxley AF. Muscle structure and theories of contraction. Prog Biophys Biophys Chem. 1957;7:255–318. [PubMed] [Google Scholar]
- Huxley HE. The mechanism of muscular contraction. Science. 1969;164:1356–1366. [PubMed] [Google Scholar]
- Huxley HE, Hanson J. Changes in cross-striations of muscle during contraction and stretch and their structural implications. Nature. 1954;173:973–976. doi: 10.1038/173973a0. [DOI] [PubMed] [Google Scholar]
- Huxley AF, Niedergerke R. Structural changes in muscle during contraction. Interference microscopy of living muscle fibres. Nature. 1954;173:971–973. doi: 10.1038/173971a0. [DOI] [PubMed] [Google Scholar]
- Huxley AF, Simmons RM. Proposed mechanism of force generation in striated muscle. Nature. 1971;233:533–538. doi: 10.1038/233533a0. [DOI] [PubMed] [Google Scholar]
- Josephson RK, Stokes DR. Work-dependent deactivation of a crustacean muscle. J Exp Biol. 1999;202:2551–2565. doi: 10.1242/jeb.202.18.2551. [DOI] [PubMed] [Google Scholar]
- Kellermayer MSZ, Smith SB, Granzier HLM, Bustamante C. Folding-unfolding transitions in single titin molecules characterized with laser tweezers. Science. 1997;276:1112–1116. doi: 10.1126/science.276.5315.1112. [DOI] [PubMed] [Google Scholar]
- Labeit D, Watanabe K, Witt C, Fujita H, Wu Y, Lahmers S, Funck T, Labeit S, Granzier HL. Calcium-dependent molecular spring elements in the giant protein titin. Proc Natl Acad Sci U S A. 2003;100:13716–13721. doi: 10.1073/pnas.2235652100. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Lee HD, Herzog W. Force enhancement following muscle stretch of electrically and voluntarily activated human adductor pollicis. J Physiol. 2002;545:321–330. doi: 10.1113/jphysiol.2002.018010. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Marszalek PE, Lu H, Li H, Carrion-Vazquez M, Oberhauser AF, Schulten K, Fernandez JM. Mechanical unfolding intermediates in titin molecules. Nature. 1999;402:100–103. doi: 10.1038/47083. [DOI] [PubMed] [Google Scholar]
- Morgan DL. New insights into the behavior of muscle during active lengthening. Biophys J. 1990;57:209–221. doi: 10.1016/S0006-3495(90)82524-8. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Morgan DL. An explanation for residual increased tension in striated muscle after stretch during contraction. Exp Physiol. 1994;79:831–838. doi: 10.1113/expphysiol.1994.sp003811. [DOI] [PubMed] [Google Scholar]
- Morgan DL, Whitehead NP, Wise AK, Gregory JE, Proske U. Tension changes in the cat soleus muscle following slow stretch or shortening of the contracting muscle. J Physiol. 2000;522:503–513. doi: 10.1111/j.1469-7793.2000.t01-2-00503.x. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Noble MIM. Enhancement of mechanical performance of striated muscle by stretch during contraction. Exp Physiol. 1992;77:539–552. doi: 10.1113/expphysiol.1992.sp003618. [DOI] [PubMed] [Google Scholar]
- Peterson D, Rassier D, Herzog W. Force enhancement in single skeletal muscle fibres on the ascending limb of the force-length relationship. J Exp Biol. 2004;207:2787–2791. doi: 10.1242/jeb.01095. [DOI] [PubMed] [Google Scholar]
- Rassier DE, Herzog W. Active force inhibition and stretch induced force enhancement in frog muscle treated with BDM. J Appl Physiol. 2004a;97:1395–1400. doi: 10.1152/japplphysiol.00377.2004. [DOI] [PubMed] [Google Scholar]
- Rassier DE, Herzog W. Effects of shortening on the stretch-induced force enhancement in single skeletal muscle fibers. J Biomech. 2004b;37:1305–1312. doi: 10.1016/j.jbiomech.2003.12.033. [DOI] [PubMed] [Google Scholar]
- Rassier D, Herzog W. Force enhancement and relaxation rates after stretch of activated muscle fibers. Proc R Soc Lond B Biol Sci. 2005;272:475–480. doi: 10.1098/rspb.2004.2967. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Rassier DE, Herzog W, Wakeling J, Syme D. Stretch-induced, steady-state force enhancement in single skeletal muscle fibers exceeds the isometric force at optimal fibre length. J Biomech. 2003;36:1309–1316. doi: 10.1016/s0021-9290(03)00155-6. [DOI] [PubMed] [Google Scholar]
- Rief M, Gautel M, Oesterhelt F, Fernandez JM, Gaub HE. Reversible unfolding of individual titin immunoglobulin domains by AFM. Science. 1997;276:1109–1112. doi: 10.1126/science.276.5315.1109. [DOI] [PubMed] [Google Scholar]
- Schachar R, Herzog W, Leonard TR. The effects of muscle stretching and shortening on isometric forces on the descending limb of the force-length relationship. J Biomech. 2004;37:917–926. doi: 10.1016/j.jbiomech.2003.10.006. [DOI] [PubMed] [Google Scholar]
- Sugi H. Tension changes during and after stretch in frog muscle fibres. J Physiol. 1972;225:237–253. doi: 10.1113/jphysiol.1972.sp009935. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Sugi H, Tsuchiya T. Stiffness changes during enhancement and deficit of isometric force by slow length changes in frog skeletal muscle fibres. J Physiol. 1988;407:215–229. doi: 10.1113/jphysiol.1988.sp017411. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Talbot JA, Morgan DL. Quantitative analysis of sarcomere non-uniformities in active muscle following a stretch. J Muscle Res Cell Motil. 1996;17:261–268. doi: 10.1007/BF00124247. [DOI] [PubMed] [Google Scholar]
- Tatsumi R, Maeda K, Hattori A, Takahashi K. Calcium binding to an elastic portion of connectin/titin filaments. J Muscle Res Cell Motil. 2001;22:149–162. doi: 10.1023/a:1010349416723. [DOI] [PubMed] [Google Scholar]
- Yamasaki R, Berri M, Wu Y, Trombitás K, McNabb, Kellermayer M, Witt C, Labeit D, Labeit S, Greaser ML, Granzier HLM. Titin–actin interaction in mouse myocardium: passive tension modulation and its regulation by calcium/S100A1. Biophys J. 2001;81:2297–2313. doi: 10.1016/S0006-3495(01)75876-6. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Zahalak GI. Can muscle fibers be stable on the descending limbs of their sarcomere length-tension relations? J Biomech. 1997;30:1179–1182. doi: 10.1016/s0021-9290(97)00079-1. [DOI] [PubMed] [Google Scholar]


