Abstract
Analysis of the complete genome sequence of Saccharomyces cerevisiae confirms and extends earlier evidence that a majority of yeast genes are not essential, at least under laboratory conditions. Many fail to yield a discernible mutant phenotype even when disrupted. Genes not subject to natural selection would accumulate inactivating mutations, so these “cryptic” genes must have functions that are overlooked by the standard methods of yeast genetics. Two explanations seem possible: (i) They have important functions only in environments not yet duplicated in the laboratory and would have conditional phenotypes if tested appropriately. (ii) They make small, but significant, contributions to fitness even under routine growth conditions, but the effects are not large enough to be detected by conventional methods. We have tested the second “marginal benefit” hypothesis by measuring the fitnesses of a random collection of disruption mutants in direct competition with their wild-type progenitor. A substantial majority of mutant strains that lack obvious defects nevertheless are at a significant selective disadvantage just growing on rich medium under normal conditions. This result has important implications for efforts to understand the functions of novel genes revealed by sequencing projects.
Keywords: evolution, gene function, yeast genome
One of the most provocative discoveries of the yeast genome sequencing project is that many genes have no evident function. As pointed out by Dujon (1) this conclusion emerged early, with the publication of the first complete chromosome sequence, and, it has changed little as the balance of the sequencing was completed (2). About 6,000 genes have been identified by sequence analysis, but fewer than 2,000 have experimentally characterized functions. About an equal number reveal probable homology to genes with known functions or have recognizable sequence motifs. Thus, more than one-third of all genes recognized in the yeast sequence have neither established nor inferred function, and the figure is closer to one-half if weak or limited homologies and isolated sequence motifs are discounted. Even targeted disruption of previously unknown genes usually fails to produce recognizable phenotypes (2).
This result was both anticipated and confirmed by more traditional experimental strategies. Goebl and Petes (3) showed that about 80% of random insertions produce no evident phenotype. Because about 75% of the yeast genome is transcribed, this result suggested that about 60% of the expressed genes are not essential. This finding was substantiated by Burns et al. (4) who investigated insertions specifically into vegetatively expressed sequences, which they estimated to constitute over 80% of the genome excluding rDNA. Even under a range of stressful growth conditions, they could detect mutant phenotypes for only about 50% of such disruptions.
Analyses in other eukaryotes are less complete, but it is clear that genes without obvious mutant phenotypes are common (5). For example, among 483 putative genes detected in a 2.2-megabase block of the Caenorhabditis elegans genome, only 20 (4.1%) were known from conventional genetic analyses, and only about one-third were homologous to known genes in other organisms (6). In Drosophila, an analysis of several chromosomal regions that have been subjected to saturation mutagenesis suggests that there are about 3,600 lethal loci in a genome of about 12,000 genes (5). Even the very biased set of genes for which targeted disruptions have been produced in mice yields only about 25% embryonic lethals (7).
These “cryptic” genes must have some function. The neutral theory of molecular evolution (8) predicts that genes not subject to natural selection will accumulate inactivating mutations, including stop codons, and the rapid accumulation of synonymous relative to nonsynonymous substitutions substantiates that expectation (9, 10). Probably the most obvious (and widely accepted) explanation is that many genes have important functions in environments not yet tested in the laboratory or in response to stresses not normally encountered there. In other words, mutants will have conditional phenotypes if the right conditions are found. This “contingent function” hypothesis is implicit in important proposals for further analysis of the yeast genome (11–13). However, there is an alternative perfectly compatible with well-established evolutionary theory; many genes may never be essential but instead make small contributions to the efficiency and/or reliability of routine processes under ordinary conditions. We call this alternative the “marginal benefit” hypothesis.
Genes with functions that are largely “redundant” (14) are encompassed by the marginal benefit hypothesis, but that is not the only possibility. Some genes may exist only to “fine-tune” functions carried out principally by other genes; others may be involved in processes that intrinsically are nonessential or not even very important. One interesting feature of the yeast genome data hints at these latter possibilities. Genes previously detected by conventional genetic criteria are much more likely to have known homologs in other species (over 75%) than are genes detected only by sequence analysis (under 50%). That is, genes without experimentally verified functions in yeast are more likely to have escaped detection also in other species (1). That most nonessential genes are expressed during routine vegetative growth (4) also is consistent with marginal benefit. One might expect genes with strictly contingent functions to be induced by relevant environmental conditions.
The marginal benefit model is consistent with theoretical treatments of “nearly neutral” mutations (8, 9, 15). However, that theory has been applied primarily to allelic sequence variation assumed to have a minor effect on gene function. As far as we know, marginal benefit has not been explicitly formulated or experimentally verified as an explanation for the very existence of genes in which even null mutations produce no evident phenotypes. The selective advantage required to maintain a functional gene is surprisingly small. The nearly neutral theory predicts that selection will prevail over mutation and drift roughly when the coefficient of selection (s, defined as the fractional difference in relative fitness) is larger than the reciprocal of the effective population size (i.e., when Nes > 1). Effective population sizes for yeast are very large, as confirmed by the existence of highly significant codon usage bias (9, 10, 16), so useful genes might increase fitness by much less than 1 percent.
Effects of that magnitude certainly would escape detection in conventional genetic analysis, but methods are available that exploit the large population sizes and short generation times of microbes to measure small fitness differences (17–20). The basic experimental design is very simple: appropriate strains are grown together for multiple generations and the frequencies of the genotypes in the population are determined periodically. If one is less fit, it declines in frequency. A particularly informative analysis focuses on the ratio of one genotype to another, typically mutant/wild type (21). It can be shown that the change in ratio with time is given by the equation ln(Rt) = ln(R0) − st (where R0 is the initial genotype ratio, Rt is the ratio after t generations, and s is the selection coefficient). By using this approach, we show here that a majority of a random set of disruption mutations that lack obvious phenotypes have significantly reduced fitness.
MATERIALS AND METHODS
Strains and Mutations.
Saccharomyces cerevisiae haploid strain FY10 (leu2Δ1, ura3–52) was the parent for all mutant lines. We generated insertion mutations in FY10 by transformation with a genomic library previously mutagenized with a mini-Tn3∷LEU2 transposon also containing lacZ coding sequences (4). This procedure inserts the LEU2 and lacZ markers by homologous recombination but essentially at random throughout the yeast genome. LEU2 is the conditional marker used to recover insertion mutants, but preliminary experiments showed that it confers a significant selective advantage even in rich medium, so the “wild type” for all competition experiments was a LEU2 (+) derivative of FY10 designated FY10(leu+). It was constructed by repairing the leu2 deficiency in FY10 by homologous recombination (22) with the 1.6-kb HpaI–AccI restriction fragment of LEU2 from pRS415 (Stratagene). Because our experimental design involved sensitive measurements of small fitness effects, we took precautions to minimize the possibility that strains differed at sites other than the disrupted gene. We used a haploid parental stock that could not be segregating unknown polymorphisms and all strains, including FY10(leu+), derived from the same single colony grown for a minimum number of generations before transformation. Because FY10 is haploid, only disruptions of nonessential genes were recovered, typically about 80% of all insertions (3, 4). Among these disruptions, we chose a subset that expressed lacZ during vegetative growth as determined by β-galactosidase staining of nitrocellulose lifts from patch plates (23). The lacZ gene in the disruption construct lacks a promoter and initiator ATG codon, so these strains have in-frame insertions disrupting routinely expressed sequences that we assume to be a random sample of such genes in the genome. Because most genes are vegetatively expressed at levels detected by this method (4), this criterion does not introduce a major bias into the sample of genes analyzed. The lacZ marker also was used to score genotype frequencies during the actual competitions. Finally, we performed Southern blot analyses with a LEU2 probe to identify and eliminate from further consideration stocks with more than one chromosomal insertion or with an insertion in a 2-μ plasmid.
Fitness Measurements.
To measure the fitness of each mutant stock relative to FY10(leu+) (our “wild type”), a mixed population was established by inoculating 2 ml of yeast extract/peptone/dextrose (YPD) (rich medium) with 32 μl each from overnight cultures of the two strains, both grown in YPD. All populations were maintained at 30°C in 16 × 150-mm tubes shaken at 200 rpm. The competition cultures were back-diluted 32-fold into 2 ml of fresh YPD daily, so they went through five generations each day to return to saturation. Periodically, a sample was plated onto YPD to score relative genotype frequencies. These plates were incubated for 2 days, then stained for β-galactosidase activity (24) to determine the frequency of each strain in the population.
Data Analysis.
Sample size at each time point ranged from 500 to 1,000 colonies. We calculated a selection coefficient for each mutant relative to the control strain by fitting the equation ln(Rt) = ln(R0) − st to the frequency data (18, 21). Data were analyzed and graphs were prepared by using Kalediagraph software for the Apple Macintosh.
RESULTS
We selected at random 34 disruption strains that produced visible colonies on standard YPD medium and that stained for β-galactosidase activity. Because the parent strain is haploid, these strains presumably carry insertions in expressed but nonessential genes. Each strain was tested in a battery of conditional selection procedures (4) as follows: YPD supplemented with (i) 0.9 M NaCl, (ii) 10 mM EGTA, (iii) 8 mM caffeine, or (iv) 10 μg/ml benomyl; incubation at (v) 25°C or (vi) 37°C; (vii) growth on minimal medium (with necessary supplements for the known markers in FY10); and (viii) growth with 3% glycerol as primary carbon source. One strain grew slowly on minimal medium, five produced small colonies even on YPD and failed to grow on glycerol (typical petite phenotypes), and one produced small colonies on glycerol. All others (82%) produced apparently normal colonies under all conditions.
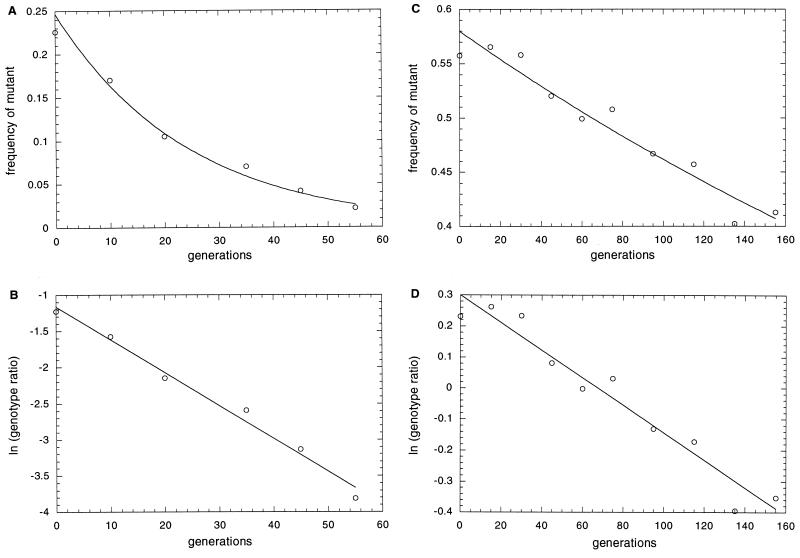
We measured the fitness of the same 34 strains by coculturing each with wild type in rich medium for 75–150 generations and periodically determining the relative frequencies of the two genotypes. Fig. 1 illustrates typical results. One mutant, TD64, declined almost to extinction within 60 generations (Fig. 1A). As expected, a logarithmic plot of genotype ratios (Fig. 1B) gives an excellent fit to a straight line whose slope provides an estimate of the selection coefficient (s = 0.045 ± 0.007). (Positive values of s indicates selective disadvantage.) Even a selective disadvantage of less than 0.5% (s < 0.005) can be detected with confidence in some cases (Fig. 1 C and D). The frequency of TD63 declines only from about 56% to about 41% even after more than 150 generations and the estimated selection coefficient is only 0.004 ± 0.001, but the difference from zero is highly significant (P < 0.001).
Figure 1.
Representative competition experiments. (A and C) The decline in frequency of the disruption mutants TD64 and TD63, respectively, as a function of generations in culture. Note the different scales. (B and D) The natural log of the ratio of the disruption strain to the wild-type strain for the same data, each with a line that is the least-squares fit with a slope that provides an estimate of the selection coefficient (s) reported in Table 1.
Similar analyses of all 34 strains are summarized in Table 1. Twenty five strains (74%) showed significant fitness defects ranging from 0.3% to about 23% (s = 0.003 to 0.228). The apparent petite mutants cluster near the top, with competitive defects ranging from about 9% to 23%. Interestingly, three strains with defects in the same range (TD88, TD87, and TD10) had no visible phenotype under any of the tested conditions. In all, 19 of 27 strains (70%) that had no apparent phenotype by conventional criteria did have significant fitness defects revealed in the competition experiments. Seven of the 34 strains did not change significantly in frequency relative to wild type, and two increased modestly.
Table 1.
Competition of disruption strains against wild type
| Strain | s | R | n | P |
|---|---|---|---|---|
| TD84* | 0.228 ± 0.046 | 0.992 | 5 | <0.001 |
| TD24* | 0.220 ± 0.061 | 0.992 | 4 | <0.001 |
| TD88 | 0.216 ± 0.157 | 0.870 | 5 | <0.05 |
| TD83* | 0.215 ± 0.075 | 0.977 | 5 | <0.001 |
| TD30* | 0.159 ± 0.022 | 0.994 | 4 | <0.001 |
| TD87 | 0.104 ± 0.025 | 0.994 | 4 | <0.001 |
| TD66* | 0.098 ± 0.018 | 0.993 | 5 | <0.001 |
| TD92† | 0.094 ± 0.014 | 0.996 | 5 | <0.001 |
| TD10 | 0.090 ± 0.018 | 0.988 | 6 | <0.001 |
| TD37 | 0.058 ± 0.008 | 0.992 | 7 | <0.001 |
| TD61 | 0.045 ± 0.010 | 0.981 | 7 | <0.001 |
| TD64 | 0.045 ± 0.007 | 0.993 | 6 | <0.001 |
| TD2 | 0.013 ± 0.003 | 0.938 | 8 | <0.001 |
| TD8 | 0.013 ± 0.004 | 0.928 | 12 | <0.001 |
| TD19 | 0.012 ± 0.001 | 0.996 | 9 | <0.001 |
| TD23 | 0.011 ± 0.003 | 0.923 | 8 | <0.01 |
| TD48 | 0.010 ± 0.003 | 0.982 | 5 | <0.001 |
| TD27 | 0.006 ± 0.003 | 0.922 | 7 | <0.01 |
| TD79 | 0.006 ± 0.004 | 0.889 | 6 | <0.01 |
| TD52 | 0.006 ± 0.004 | 0.847 | 7 | <0.01 |
| TD21 | 0.005 ± 0.001 | 0.968 | 8 | <0.001 |
| TD80 | 0.005 ± 0.003 | 0.871 | 7 | <0.01 |
| TD63 | 0.004 ± 0.001 | 0.972 | 10 | <0.001 |
| TD4 | 0.003 ± 0.002 | 0.679 | 13 | <0.01 |
| TD53 | 0.003 ± 0.003 | 0.804 | 7 | <0.02 |
| TD90 | 0.005 ± 0.006 | 0.586 | 8 | NS |
| TD9 | 0.005 ± 0.004 | 0.468 | 11 | NS |
| TD16 | 0.003 ± 0.004 | 0.241 | 12 | NS |
| TD50 | 0.001 ± 0.001 | 0.494 | 10 | NS |
| TD60 | 0.001 ± 0.002 | 0.508 | 9 | NS |
| TD1‡ | −0.001 ± 0.005 | 0.401 | 5 | NS |
| TD6 | −0.002 ± 0.004 | 0.344 | 8 | NS |
| TD7 | −0.005 ± 0.004 | 0.641 | 12 | <0.05 |
| TD5 | −0.007 ± 0.004 | 0.800 | 10 | <0.01 |
Selection coefficients (s) were measured as in Fig. 1. Strains are arranged in descending order of the estimated selection coefficient. The final three columns report the correlation coefficient (R), the number of time points at which genotype frequencies were measured (n), and the statistical significance (P) for a test of the null hypothesis that the slope is not different from zero. NS, not significant.
Produces small colonies on rich medium, fails to grow with glycerol as primary carbon source (petite).
Produces small colonies on rich medium, marginal growth with glycerol as primary carbon source.
Produces small colonies on minimal medium.
DISCUSSION
Seven of 34 strains (21%) with disruptions in nonessential genes exhibited conditional phenotypes, and most of those produced small colonies even on standard medium (i.e., they would have been recognized as mutant in a conventional analysis). In contrast, and consistent with the marginal benefit model, at least 19 of 27 strains (70%) that have no conventional phenotype do have significantly reduced fitness. The fitness defects relative to the parental stock ranged from about 0.3% to almost 22%, but most were below 5% and several below 1%. It is important to keep in mind that gene disruptions of the type analyzed here are expected to abolish, or nearly so, the normal function of the target gene (4). Thus, the modest selective disadvantages observed cannot be attributed to “weak” alleles of genes that could be mutated in some other way to produce clear phenotypes. That is, the maximum contribution of many of these genes may be a small improvement in fitness.
Although loss of function of the disrupted gene is the most obvious explanation for the decreased fitness of mutants, we cannot rigorously exclude some alternatives: (i) The transformation process used to generate disruptions might create mutations at sites other than the main insertion. (ii) Some insertions may produce lacZ fusion proteins that actively interfere with growth (i.e., they produce antimorphs). (iii) The insertions may cause delays in replication, problems with segregation, or some other effect related neither to loss of gene function nor to production of an abnormal protein. (iv) Insertions may in some way interfere with the function of genes other than the disruption target. We think these alternatives are unlikely to be general explanations for effects of the consistency and magnitude seen. Moreover, the first alternative just changes the locus of the selective effect, and the second requires that a protein performing no useful function under the conditions tested can, in altered form, be harmful under those same conditions. In addition, the seven strains that test as “neutral” serve as negative controls; they eliminate any general effect of replicating extra DNA, producing β-galactosidase, etc. Thus, any alternative explanation must invoke effects that are specific to the site of insertion. On the whole, the parsimonious explanation is that the fitness defects in most cases result from loss of function at the insertion site, and hence, that many genes have modest consequences for fitness without being essential.
As this manuscript was being prepared, Smith et al. (25) reported results of an analysis of 255 putative genes on yeast chromosome V using a “genetic footprinting” method that detects loss of random TY1 insertions from a mass population. Although focused on identifying contingent functions of novel genes, their results are entirely consistent with ours, providing additional strong support for the marginal benefit model. Just 20% of the loci examined were essential or had major defects (obvious phenotypes) on rich medium. In contrast, about 39% had more subtle “growth” defects (in effect, selection coefficients ranging from about 0.05 to 0.25). A few loci had conditional phenotypes under a range of selections similar to those we used, but most of these mutants also were at a disadvantage even on rich medium. In all, they were unable to detect any phenotype for 98 of the 255 loci (38.4%). Adjusting our data for the fact that essential or nearly essential loci were eliminated at the outset, our more sensitive procedure leaves only about 20% of the tested loci without a detected contribution to fitness, even in rich medium and absent “stressful” conditions.
What about that 20%? The theory of nearly neutral replacement (8, 15) indicates that a selection coefficient approximately the inverse of the effective population size is sufficient to prevent loss of function by mutation and drift. Because effective population sizes for yeast are very large (10, 16), fitness contributions much smaller than those we measured would be important. Thus, the marginal benefit hypothesis still might apply even to strains for which we failed to detect reduced fitness. Alternatively, these loci could be disrupted in genes with strictly conditional phenotypes. Indeed, one of our “neutral” strains (TD1) does have a conditional phenotype (small colonies on minimal medium). Finally, it is conceivable that some novel genes are “selfish” (selected to propagate themselves but making no contribution to fitness of the “host”).
Although the marginal benefit hypothesis provides a sufficient explanation for the evolution and maintenance of nonessential genes, we cannot be sure it is a complete explanation. Marginal benefit and contingent functions are not mutually exclusive. Thus, some mutations that cause small growth defect under standard conditions on rich medium may have much stronger phenotypes under other conditions. Indeed, we found this outcome to be true for the leu2 mutation present as a marker in the FY10 stock with which we started all strain constructions. In a preliminary series of experiments (data not shown), we used FY10 as the “wild type” in competitions with disruption mutants and the frequency of the disruption genotype was followed by using the LEU2 marker. This design rested on the assumption that the leu2 deficiency in FY10 would be selectively neutral on rich medium (YPD). However, we tested this assumption in competitions between FY10 and the “repaired” derivative, FY10(leu+), and found that the latter consistently had a selective advantage of about 2.5% (s = 0.0233 ± 0.0021, 0.0266 ± 0.0066, and 0.0267 ± 0.0116 in three replicates).
Smith et al. (25) report similar observations on several genes with known conditional phenotypes. Thus, the ability to produce a strong phenotype under specific conditions does not necessarily mean that the “real” function of the gene is to cope with those conditions. Which phenotype is more significant for the evolution and selective maintenance of genes that have both marginal and contingent functions must depend on the relative frequencies of relevant environmental conditions. In the simplest case, in which individuals carrying a particular mutation encounter different environments essentially at random, the costs/benefits attributable to effects in each environment should be proportional to the product of the selection coefficient in that environment and its frequency. In other words, a gene that improves fitness by 1% under “normal” conditions should be as “valuable” as one that is essential under unusual conditions experienced, at any given time, by 1% of the population.
Effects of the magnitude reported here (or smaller) will be difficult to document in organisms for which large-scale competition experiments are not feasible (26). Measurements of fluctuating asymmetry provide an interesting alternative that may be feasible at least for genes involved in development (27). Nevertheless, our results strongly suggest that the marginal benefit hypothesis can account for the evolution and selective maintenance of many genes that cannot be mutated to produce any obvious phenotypes and that genome-level analyses need to address this possibility.
Acknowledgments
We thank Deepika DeSylva for the DNA used to make disruptions, Dawn Blomquist and Lance McQuillan for assistance with some competition experiments, and Greg Hermann, Denichiro Ostuga, and Amy Roeder for technical advice. Jon Seger made useful suggestions on a draft of the manuscript. This research was support in part by grants to W.J.D. from the University of Utah Research Committee and to J.W.T. from the Bioscience Undergraduate Research Program.
ABBREVIATION
- YPD
yeast extract/peptone/dextrose
References
- 1.Dujon B. Trends Genet. 1996;12:263–270. doi: 10.1016/0168-9525(96)10027-5. [DOI] [PubMed] [Google Scholar]
- 2.Oliver S G, van der Art Q J M, Agostone-Carbone M L, Aigle M, Alberghina L, Alexandraki D, Antoine G, Anwar R, Ballesta J P G, Benit P, et al. Nature (London) 1992;357:38–46. doi: 10.1038/357038a0. [DOI] [PubMed] [Google Scholar]
- 3.Goebl M G, Petes T D. Cell. 1986;46:983–992. doi: 10.1016/0092-8674(86)90697-5. [DOI] [PubMed] [Google Scholar]
- 4.Burns N, Grimwade B, Ross-MacDonald P B, Choi E-Y, Finberg K, Roeder G S, Snyder M. Genes Dev. 1994;8:1087–1105. doi: 10.1101/gad.8.9.1087. [DOI] [PubMed] [Google Scholar]
- 5.Miklos G L G, Rubin G M. Cell. 1996;86:521–529. doi: 10.1016/s0092-8674(00)80126-9. [DOI] [PubMed] [Google Scholar]
- 6.Wilson R, Ainscough R, Anderson K, Baynes C, Berks M, Bonfield J, Burton J, Connell M, Copsey T, Cooper J, et al. Nature (London) 1994;368:32–38. doi: 10.1038/368032a0. [DOI] [PubMed] [Google Scholar]
- 7.Brandon E P, Idzerda R L, McKnight G S. Curr Biol. 1995;5:1–27. doi: 10.1016/s0960-9822(95)00177-1. [DOI] [PubMed] [Google Scholar]
- 8.Kimura M. The Neutral Theory of Molecular Evolution. Cambridge, U.K.: Cambridge Univ. Press; 1983. [Google Scholar]
- 9.Ohta T. BioEssays. 1996;18:673–677. doi: 10.1002/bies.950180811. [DOI] [PubMed] [Google Scholar]
- 10.Kreitman M. BioEssays. 1996;18:678–683. doi: 10.1002/bies.950180812. [DOI] [PubMed] [Google Scholar]
- 11.Smith V, Botstein D, Brown P O. Proc Natl Acad Sci USA. 1995;92:6479–6483. doi: 10.1073/pnas.92.14.6479. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 12.Johnston M. Trends Genet. 1996;12:242–243. doi: 10.1016/0168-9525(96)30064-4. [DOI] [PubMed] [Google Scholar]
- 13.Oliver S G. Trends Genet. 1996;12:241–242. doi: 10.1016/0168-9525(96)30053-x. [DOI] [PubMed] [Google Scholar]
- 14.Brookfield J F Y. Curr Biol. 1997;7:R366–R368. doi: 10.1016/s0960-9822(06)00176-x. [DOI] [PubMed] [Google Scholar]
- 15.Ohta T. Nature (London) 1974;252:351–354. doi: 10.1038/252351a0. [DOI] [PubMed] [Google Scholar]
- 16.Sharp P M. In: Evolution and Animal Breeding. Hill W G, Mackey T F C, editors. Wallingford, U.K.: CAB International; 1989. pp. 23–32. [Google Scholar]
- 17.Paquin C, Adams J. Nature (London) 1983;302:495–500. doi: 10.1038/302495a0. [DOI] [PubMed] [Google Scholar]
- 18.Dykhuizen D E. Annu Rev Ecol Syst. 1990;21:378–398. [Google Scholar]
- 19.Lenski R E, Rose M R, Simpson S C, Tadler S C. Am Nat. 1991;138:1315–1341. [Google Scholar]
- 20.Elena S F, Cooper V S, Lenski R E. Science. 1996;272:1802–1804. doi: 10.1126/science.272.5269.1802. [DOI] [PubMed] [Google Scholar]
- 21.Lenski R E. In: Assessing Ecological Risks of Biotechnology. Ginzburg L R, editor. Boston: Butterworth–Heinemann; 1991. pp. 173–192. [Google Scholar]
- 22.Kaiser C, Michaelis S, Mitchell A. Methods in Yeast Genetics. Plainview, NY: Cold Spring Harbor Lab. Press; 1994. [Google Scholar]
- 23.Breeden L, Nasmyth K. Cold Spring Harbor Symp Quant Biol. 1985;50:643–650. doi: 10.1101/sqb.1985.050.01.078. [DOI] [PubMed] [Google Scholar]
- 24.Duttweiller H M. Trends Genet. 1996;12:340–341. doi: 10.1016/s0168-9525(96)80008-4. [DOI] [PubMed] [Google Scholar]
- 25.Smith V, Chow K N, Lashkari D, Botstein D, Brown P O. Science. 1996;274:2069–2074. doi: 10.1126/science.274.5295.2069. [DOI] [PubMed] [Google Scholar]
- 26.Brookfield J. Curr Biol. 1992;2:553–554. doi: 10.1016/0960-9822(92)90036-a. [DOI] [PubMed] [Google Scholar]
- 27.Batterham P, Davies A G, Game A Y, McKenzie J A. BioEssays. 1996;18:841–845. doi: 10.1002/bies.950181011. [DOI] [PubMed] [Google Scholar]