Abstract
We investigated the extent to which subjects’ ability to perceive the fine spatial structure of a stimulus depends on its temporal properties (namely the frequency at which it vibrates). Subjects were presented with static or vibrating gratings that varied in spatial period (1–8 mm) and vibratory frequency (5–80 Hz) and judged the orientation of the gratings, presented either parallel or perpendicular to the long axis of the finger. We found that the grating orientation threshold (GOT)—the spatial period at which subjects can reliably discriminate the orientation of the grating—increased as the vibratory frequency of the gratings increased. As the spatial modulation of SA1 and RA afferent fibers has been found to be independent of vibratory frequency, the frequency dependence of spatial acuity cannot be attributed to changes in the quality of the peripheral signal. Furthermore, we found GOTs to be relatively independent of stimulus amplitude, so the low spatial acuity at high flutter frequencies does not appear to be due to an inadequacy in the strength of the afferent response at those frequencies. We hypothesized that the RA signal, the strength of which increases with vibratory frequency, interfered with the spatially modulated signal conveyed by SA1 fibers. Consistent with this hypothesis, we found that adapting RA afferent fibers improved spatial acuity, as gauged by GOTs, at the high flutter frequencies.
INTRODUCTION
The study of tactile spatial acuity has a long and rich history, dating back to Ernst Weber’s seminal book De Tactu (Weber 1996). For more than a century, the standard test of tactile acuity was the two-point limen, i.e., the smallest separation of two punctate indentations such that two distinct points of contact are perceived. This so-called “compass” test was found to be inherently flawed and has largely been replaced by the grating orientation discrimination (OD) task (see Craig and Johnson 2000; Johnson et al. 1994 for a review). In the OD task, subjects are presented with a square-wave grating with bars oriented either parallel or perpendicular to the long axis of the finger. The subject’s task is to identify the orientation of the grating, which can only be done when the ridges and grooves of the grating are sufficiently wide (Craig and Rollman 1999; Johnson and Phillips 1981). The grating orientation threshold (GOT) is the bar width at which subjects can reliably discriminate the orientation of the grating. OD has been found to yield reproducible thresholds that are highly correlated with the density of innervation of the mechanoreceptors thought to convey information about fine spatial structure (Johnson and Phillips 1981), namely Meissner and Merkel receptors. Fibers innervating Meissner corpuscles, referred to as rapidly adapting (RA) fibers, respond vigorously at the onset and offset of the deformation of the skin and are otherwise silent. In contrast, Merkel receptors exhibit a sustained response to an indentation, and the associated fibers are referred to as slowly adapting (SA1) fibers (Johnson and Hsiao 1992).
The RA and SA1 channels, each referring to a population of mechanoreceptive afferent fibers and their central connections, have been implicated in the perception of the fine spatial features of a tactile stimulus. Results from paired neurophysiological and psychophysical studies suggest that the SA1 system mediates the finest discriminations of which humans are capable (see Johnson and Hsiao 1992 for a review). However, the contribution of the RA system to spatial perception remains to be elucidated. The ability of this sensory channel to convey fine spatial information has been investigated using the Optacon, a reading aid for the blind (Bliss et al. 1970). The Optacon, which consists of an array of pins vibrating at 230 Hz, has been found to stimulate RA fibers (as well as PC fibers, which innervate Pacinian corpuscles) but not SA1 fibers (Gardner and Palmer 1989). Because PC fibers convey little fine spatial information (Johnson and Lamb 1981), the perception of the spatial features of tactile patterns generated using the Optacon is likely mediated by the RA system. Psychophysical studies have shown that subjects are capable of discriminating Optacon-generated spatial patterns, although the spatial resolution of the RA signal seems to be coarser than that of its SA1 counterpart (Johnson and Hsiao 1992). The question remains how much the RA system contributes to the perception of more naturalistic spatial stimuli, such as patterns scanned across the skin, which evoke activity in both RA and SA1 systems.
In the present study, we attempt to address this question by exploiting another way in which the RA and SA1 channels differ, namely in their sensitivity to vibratory stimuli (see Bolanowski et al. 1988 for a review). RA fibers are more sensitive to mechanical oscillations than their SA1 counterparts across much of the frequency spectrum (Freeman and Johnson 1982a; Johansson et al. 1982). Thus the information about the spatial features of a pattern vibrating at a frequency at the high end of the flutter range (i.e., at 40 and 80 Hz) might be conveyed by RA fibers, whereas spatial information about a static pattern or one vibrating at a low frequency might be conveyed by SA1 fibers. Although RA fibers have lower thresholds and exhibit higher firing rates at higher frequencies than SA1 fibers, the spatial modulation of SA1 responses is higher than that of RA responses at all vibratory frequencies (≤80 Hz at least) (Bensmaia et al. 2005a).
The fourth population of myelinated mechanoreceptive afferent fibers, SA type II fibers, which innervate Ruffini end organs in human skin (but are thought to be absent in macaques) is unlikely to contribute to OD because the resolution of the spatial signal they carry is low (Phillips et al. 1992) and they respond primarily to skin stretch (Johansson 1978), which is minimized in the absence of lateral movement between the stimulus and the skin.
Previous studies have shown that the depth or the force with which a grating is indented into the skin has little effect on OD performance. Only over a range of light forces, between 0 and 25 g, has performance been shown to improve (Goldreich and Kanics 2003; Vega-Bermudez and Johnson 2004). Increasing the indentation into the skin up to several millimeters results in increases in perceived magnitude (Greenspan et al. 1984) but no further improvement in OD performance (Gibson and Craig 2005; Johnson and Phillips 1981). The relative independence of OD performance from depth of indentation suggests that only at the lightest forces or shallowest indentations is the strength of the neural signal likely to limit the perception of fine spatial detail. We thus expect that information about the fine spatial structure of static as well as vibrating stimuli should be conveyed at the periphery by SA1 fibers provided these stimuli are sufficiently intense. As the spatial modulation of the SA1 response is nearly frequency-independent, increasing slightly from 0 (static) to 80 Hz (Bensmaia et al. 2005a), we predict that subjects’ ability to resolve the fine spatial structure of tactile stimuli should either be the same regardless of the frequency of the stimulus or improve slightly at the higher frequencies. Similarly, the spatial modulation in the RA response also improves slightly with vibratory frequency. If spatial sensitivity is dependent on this channel, then, again, performance should remain the same or improve slightly with increasing frequency, insofar as spatial modulation is the key factor.
To examine the roles of the RA and SA1 channels on spatial acuity, GOTs were measured using static gratings and gratings that vibrated at frequencies ranging from 5 to 80 Hz. Four experiments were conducted. In experiment 1, we determined suitable levels of intensity at different frequencies using a magnitude estimation procedure. In experiment 2, grating orientation thresholds were measured at six different frequencies. Thresholds were also measured using a standard, nonvibratory technique involving hand-held gratings (Johnson-Van Boven-Phillips domes, Stoelting, Wood Dale, IL). These domes are hemispheric in shape with equal-width grooves and ridges cut into them. In experiment 3, the effect of intensity on GOTs was measured. Finally, in an attempt to further differentiate the contributions of the RA and SA 1 channels, we examined the effect of vibratory adaptation on GOTs in experiment 4.
METHODS
Stimuli
Stimuli were generated and delivered by means of a dense tactile array, consisting of 400 independently controlled probes arrayed in a 20 × 20 matrix. The probes, spaced at 0.5 mm, center to center, cover a 1 × 1 cm area (Pawluk et al. 1998). The subject’s distal index fingerpad was pressed up against the probe array with a force of 100 g using a counter-weight. The stimuli were square-wave gratings, which consisted of alternating ridges and grooves of equal widths. The spatial periods of the gratings (1 ridge + 1 groove) were 1, 2, 4, 6, and 8 mm unless otherwise specified; the groove and ridge widths were thus 0.5, 1, 2, 3 and 4 mm (Bar width is used to quantify the spatial dimensions of the gratings in accordance with the psychophysical literature). Gratings were either static (0 Hz) or vibrated at 5, 10, 20, 40, or 80 Hz. The ridges of the static gratings were indented into the skin (while the grooves remained at the baseline indentation) for 1 s with on and off ramps lasting 50 ms (in addition to the 1-s “hold”). For the vibratory gratings, the grating ridges vibrated about the baseline indentation for 1 s at the specified amplitude and frequency and also included 50-ms-long on and off ramps. Stimulus amplitudes varied from experiment to experiment and are described in the following text.
Magnitude estimation of intensity
EXPERIMENT 1
On each trial, the subject was presented with a static or vibrating grating that varied in bar width, amplitude, and vibratory frequency. The ridges and grooves of the gratings were oriented perpendicular to the axis of the finger. The subject’s task was to produce a number proportional to the perceived intensity of the stimulus. No constraints were placed on the range of numbers the subject used. The ranges of amplitudes varied from frequency to frequency and are shown in Fig. 1. The maximum intensity at each frequency was constrained by the limitations of the stimulator. Six ratings were obtained from each subject for each frequency, amplitude and bar width. The first set of ratings was for practice and was not included in the analysis.
Fig. 1.
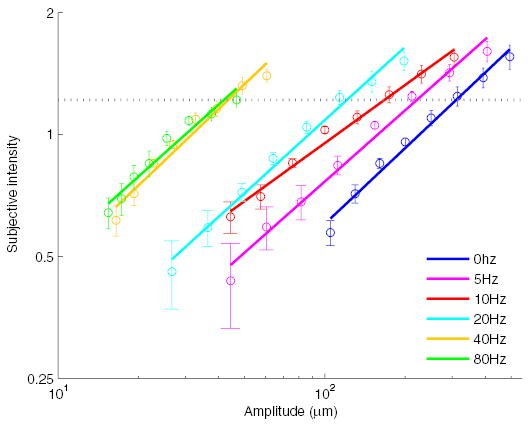
Experiment 1: mean subjective intensity of gratings as a function of vibratory frequency and amplitude. Bar width had a negligible effect on subjective intensity; ratings are therefore averaged across spatial periods at each frequency and amplitude. The horizontal line denotes the subjective intensity of the most intense 80-Hz stimulus achievable with the stimulator. In the orientation discrimination (OD) experiment, grating amplitudes were matched to that subjective level.
Grating orientation discrimination
EXPERIMENT 2
On each trial, the subject was presented with a grating at one of two orientations: parallel to the long axis of the finger (vertical) or perpendicular to it (horizontal). The subject’s task was to indicate the orientation of the grating by pressing a button; no feedback was provided. A 4.5-s interval followed the subject’s response to prevent masking effects (Craig 1983, 1985). Stimulus amplitudes were set based on the ratings, averaged across subjects, obtained in experiment 1 (see gray line in Fig. 1). Specifically, all stimuli were equated in subjective intensity with the most intense 80-Hz stimulus that the stimulator could accurately produce. On each experimental run, 240 gratings were presented in pseudorandom order (6 frequencies ×5 bar widths ×2 orientations ×4 repetitions); there were six runs per subject, the first of which was for practice and was not included in the analysis. Subjects were allowed a 10- to 15-min break in between runs.
The OD task was also performed using manually presented Johnson-Van Boven-Phillips (JVP) domes (Johnson et al. 1997) to compare GOTs obtained using the 400-probe stimulator to those obtained using traditional, hand-held stimuli. The bar widths of the domes were 0.5, 0.75, 1, 1.2, 1.5, and 2 mm; each stimulus was presented 20 times.
EXPERIMENT 3
The trial structure in experiment 3 was identical to that in experiment 2. These two experiments differed only in the stimuli that were presented. In experiment 2, all of the gratings with a given bar width were presented at the same spatial offset, beginning with a full ridge on the left (for horizontal gratings) or at the bottom (for vertical gratings). In experiments 3 and 4, the spatial offset of the gratings was varied randomly from trial to trial to reduce the possibility that subjects might use local cues to identify orientation. The gratings, all with bar widths of 1.5 mm, were presented at three intensities per vibratory frequency (0, 5, 10, 20, 40, and 80 Hz). The highest of the three amplitudes at each frequency was the same as that used in experiment 2. The other two sets of amplitudes used in experiment 3 were 5 and 10 dB below the maximum amplitude (the manipulation involved a decrement rather than an increment in stimulus amplitude to remain within the optimal operational range of the stimulator). The objective of the experiment was to ascertain the effect of stimulus intensity on grating orientation discrimination.
EXPERIMENT 4
In experiment 4, an adaptation procedure was used. During the 2 min before the beginning of the OD task and during the first 4 s of each inter-trial interval (which lasted 4.5 s), an intense vibratory stimulus was presented, at 60 Hz on some runs and 250 Hz on others. The conditioning stimuli were chosen such that their adapting effects targeted one of two populations of mechanoreceptive fibers, namely RA or PC fibers. Selective adaptation was achieved by setting the stimulus amplitudes and frequencies such that one or the other population of fibers was preferentially stimulated. The rationale behind the adapting conditions and the parameters of the adaptors are discussed in the following text. In the control condition for this set of measurements, subjects sat for 2 min before the first trial of the OD task without a stimulus. Thus the adaptation and control conditions were identical in every respect other than the presence of the adaptor. As in experiment 3, the gratings were equated in subjective intensity with the most intense 80-Hz stimulus.
Subjects
Five subjects participated in experiment 1, all males aged 21–31 yr old. Five subjects participated in experiment 2, all males aged 21–31 yr old, two of whom had also participated in experiment 1. In experiments 3 and 4, the subjects were four males and two females, ranging in age from 19 to 31 yr. All subjects were paid for their participation. One subject, the first author, participated in all four experiments. Consent was obtained from each subject. The Human Subjects Institutional Review Board of Johns Hopkins University approved the experiments.
Analysis
The GOT is the bar width at which subjects can discriminate the orientation of a grating at a criterion performance level (here, 75% correct). The GOT at a given vibratory frequency was obtained by first fitting a psychometric function to the proportion correct, p(C), as a function of bar width, w. The function was of the form (Gibson and Craig 2005)
| (1) |
where α and β were free parameters. The resulting sigmoid ranges from 0.5, which corresponds to chance performance in a two-alternative forced choice task, to 1 (perfect performance). Another method by which the thresholds can be recovered is through linear interpolation between the points adjacent to 75% correct performance. Both methods yield similar threshold estimates.
RESULTS
The objective of the magnitude estimation experiment (experiment 1) was to characterize the relationship between subjective intensity and stimulus amplitude at each vibratory frequency. From the resulting psychometric functions, we wished to derive a set of grating amplitudes such that stimuli were matched in subjective intensity across vibratory frequencies. Bar width had a negligible effect on subjective intensity so magnitude estimates were averaged across bar widths to obtain the functions shown in Fig. 1. As had been found in previous studies (Hollins and Roy 1996; Stevens 1968; Verrillo et al. 1969), subjectively matched amplitudes decreased with stimulus frequency: in other words, the perceived intensity of a stimulus increased with frequency when its amplitude was held constant.
In experiment 2, we estimated subjects’ grating orientation thresholds as a function of vibratory frequency. Figure 2 shows p(C), averaged across subjects, as a function of w, along with the fitted functions at each vibratory frequency. From Eq. 1, we computed, for each subject individually, the bar width that would have yielded 75% correct judgments (similar GOTs would be obtained if we had used linear interpolation to compute them). Figure 3 shows GOTs, averaged across subjects, as a function of vibratory frequency. Note the GOT for static gratings obtained with the probes was comparable to that obtained with the JVP domes (see the gray line in Fig. 3), demonstrating that the inherent pixelation of the stimulator did not degrade performance.
Fig. 2.
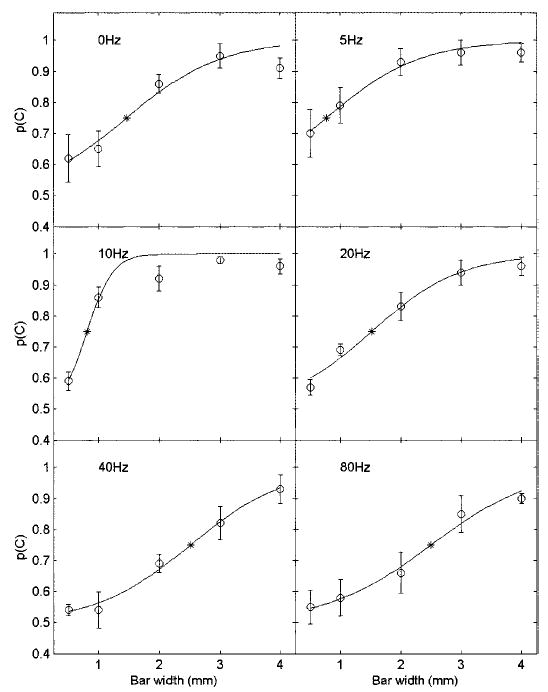
Psychometric functions relating bar width to percent correct, averaged across subjects, at each vibratory frequency.○, mean and SE. Each data point is based on 40 observations for each of 5 subjects. —, fitted function at each frequency (see Eq. 1). *, the GOT computed for that frequency using the function obtained from the p(C) averaged across subjects.
Fig. 3.
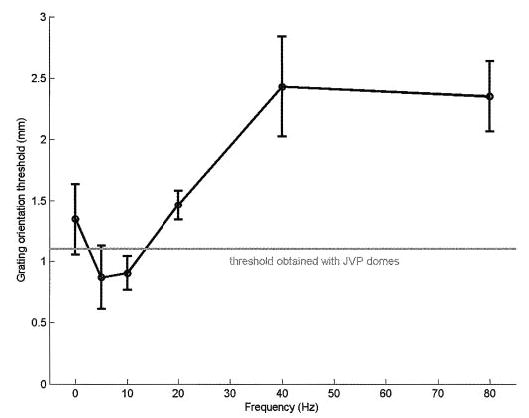
Grating orientation thresholds as a function of vibratory frequency (0 Hz corresponds to the static gratings). The gray line denotes the thresholds obtained using the hand-held JVP domes. GOTs were lower for gratings vibrating at 5 Hz than for static gratings; thresholds then triple from 5 to 80 Hz.
As mentioned in the preceding text, in neurophysiological recordings, we found that the spatial modulation in SA1 and RA responses to gratings, identical to those used in the present psychophysical experiments, was largely independent of vibratory frequency (Bensmaia et al. 2005a). As the limits of human spatial acuity have been found to be determined by the limited spatial resolution of the sensory periphery, we expected the GOTs to be approximately the same at all frequencies. In fact, however, there was a substantial decline in spatial acuity as the frequency increased: thresholds at 40 and 80 Hz were 2.4 and 2.35 mm, respectively, whereas the threshold at 5 Hz was 0.87 mm. A 6 × 5 ANOVA revealed that the decrease in GOT with vibratory frequency was significant after controlling for differences across subjects [F(5,20) = 12.1, P < 0.001].
One possible reason for the decline in GOT with increasing frequency is the way in which the stimulus amplitude was set, i.e., by matching subjective intensity across vibratory frequencies. The subjective intensity of a stimulus has been shown to be a complex function of the activity it elicits in mechanoreceptive channels (Hollins and Roy 1996). One possibility is that high-frequency stimuli were not as efficacious in stimulating the population of afferent fibers that mediate OD as were the low-frequency stimuli. The observed effect of vibratory frequency on spatial acuity may then be an effect of stimulus intensity within the relevant neural channel. To examine this possibility, we tested the effect of varying amplitude on GOT. Specifically, we presented gratings at three amplitudes at each vibratory frequency, all with a bar width of 1.5 mm (see METHODS, experiment 3). If performance was found to be strongly dependent on grating amplitude, particularly at high vibratory frequencies, then the decrement in spatial acuity with frequency could be ascribed to differences in intensity.
Figure 4 shows the effects of stimulus amplitude on OD performance: performance exhibits a slight tendency to improve as the stimulus amplitude increases. Note, however, that the –10-dB stimulus still yielded near ceiling performance at 5 and 10 Hz. An ANOVA with frequency, amplitude, and subject as factors confirmed that the main effect of amplitude on performance was significant, albeit small [F(2,10) = 4.89, P < 0.05]. The effect of amplitude was large for static gratings (0 Hz) and gratings vibrating at 40 Hz (14.4 and 12.5% improvement, respectively) but was small or nil for all other gratings (< 6%). For instance, increasing the stimulus amplitude more than threefold had no effect on performance for gratings vibrating at 20 or 80 Hz. Note that GOTs at 20 and 80 Hz are (on average) 70 and 160% higher, respectively, than those measured at 5 Hz. Furthermore, the relative insensitivity of OD performance to stimulus intensity cannot be attributed to ceiling or floor effects because this insensitivity is observed at all performance levels: at 5 and 10 Hz, where performance is nearly perfect; at 80 Hz where p(C) is just above chance; and at 20 Hz where performance is intermediate. Thus the large decrement in spatial acuity with vibratory frequency cannot be explained solely in terms of differences in stimulus intensity.
Fig. 4.
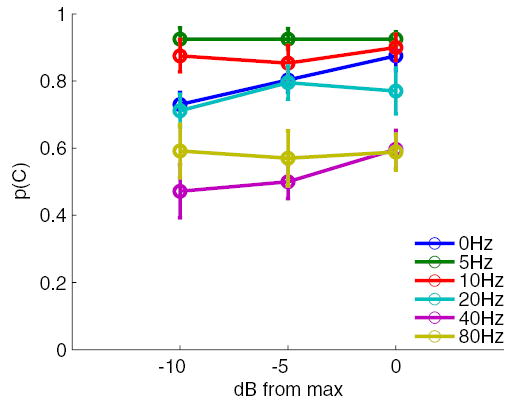
Effect of grating amplitude on grating orientation discrimination. The bar width of the gratings was 1.5 mm. There was a slight tendency for performance to improve as the amplitude of the gratings increased. However, the effect of amplitude was not strong enough to mediate the observed effects of vibratory frequency.
Thus the low spatial acuity at high vibratory frequencies does not appear to be due either to changes in the effective stimulus intensity or to a decrement in spatial modulation in the signal conveyed by peripheral afferent fibers (Bensmaia et al. 2005a). Another possibility is that the signal conveyed by RA or PC afferent fibers interferes centrally with the spatially modulated signal conveyed by SA1 fibers. Indeed, RA fibers, which are less spatially modulated than their SA1 counterparts, produce a threefold stronger response to a grating vibrating at 80 Hz than to one vibrating at 5 Hz (Bensmaia et al. 2005a). PC fibers, which convey virtually no information about the fine spatial structure of the stimulus (Johnson and Lamb 1981), are nearly silent at 5 Hz but are highly responsive to stimuli vibrating at 80 Hz. Signals emanating from one or both of these two populations of afferent fibers may effectively introduce noise to the highly modulated spatial signal conveyed by SA1 fibers, particularly in response to gratings vibrating at the high flutter frequencies.
We can explore the RA interference hypothesis by computing the magnitude of the RA response relative to its SA1 counterpart at each vibratory frequency (using data from Bensmaia et al. 2005a) and comparing it to the GOT at that frequency. If OD is mediated by SA1 fibers and the RA signal interferes with the peripheral spatial signal, then thresholds should be higher when the RA signal is high relative to the SA1 signal and vice versa. Figure 5 shows the ratio of the RA to the SA1 response,1 along with psychophysical GOTs, as a function of vibratory frequency. The two functions are strikingly similar: when the SA1 signal is weak and the RA signal is strong, the GOT is high; the highest spatial acuity is observed when the SA1 signal is strongest relative to its RA counterpart, namely at 5 Hz. Note that the signal ratio and GOT curves diverge maximally for static gratings. One possible explanation for this divergence may be found in the temporal structure of the response. First, the strongest portion of the SA1 response coincides with an even stronger RA response, namely at the onset of the stimulus. Furthermore, the off response produced by RA fibers may serve to mask the signal conveyed by SA1 fibers, which is substantially weaker after a one-second indentation, than it is at the onset of the stimulus. Nevertheless, the relative strength of the SA1 and RA signals is a good predictor of spatial acuity (the correlation between the 2 curves in Fig. 5 is 0.94). Because PC sensitivity increases across the range of frequencies used in the present study, one would expect a qualitatively similar result if we examined the magnitude of the PC response relative to its SA1 counterpart. In other words, the ratio of the PC to the SA1 response is likely to be highly correlated with GOTs. We also examined the possibility that GOTs might be correlated with SA1 or RA spatial modulation or possibly with SA1 and RA spike rates. No substantial correlation was found between any of these other measures and the GOTs (see DISCUSSION).
Fig. 5.
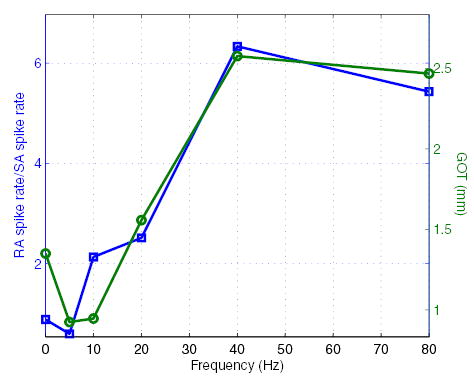
Ratio of the spike rate evoked in RA fibers by gratings at each vibratory frequency to that evoked in SA1 fibers by the same stimuli (□) (data from Bensmaia et al. 2005a) along with GOTs (○) from Fig. 3. The strength of the RA response relative to its SA1 counterpart is much greater at 40 and 80 Hz than it is for static gratings or for gratings that vibrate at a low frequency. There is a striking resemblance between the signal intensity ratio and GOTs.
In experiment 4, we tested the interference hypothesis by examining the effect of adaptation on OD using two adaptors. One adaptor was set to 60 Hz and 40 μm (zero-to-peak) and targeted primarily RA fibers; the other was set to 250 Hz and 10 μm and targeted PC fibers. We sought to reduce the response of one or the other population of fibers without causing substantial adaptation upstream along the sensory pathway (see DISCUSSION). The idea behind experiment 4 was to assess the extent to which desensitization of RA and PC afferent fibers affected OD performance. Sixty-hertz adaptors have been shown to produce a substantial decrement in RA sensitivity (Bensmaia et al. 2005b); similarly, the peak of PC sensitivity is ~250–300 Hz (Freeman and Johnson 1982a), and substantial PC adaptation has been obtained with conditioning stimuli at 200 and 300 Hz (Bensmaia et al. 2005b). From the interference hypothesis, we predicted that the reduction in RA sensitivity caused by the 60-Hz adaptor would result in a decrement in the RA response evoked by the gratings, particularly those vibrating at 40 and 80 Hz. If the RA signal interferes with the SA1 signal and this interference is responsible for the decrement in spatial acuity observed at the high flutter frequencies, then OD performance at those frequencies should improve when the finger is adapted at 60 Hz. Similarly, if the PC signal interferes with the spatial signal conveyed by SA1 fibers, then adapting at 250 Hz should yield an improvement in performance.
Figure 6A shows the effects of the two adaptors on GOTs. Vibratory adaptation had no effect on GOT for either static gratings or gratings vibrating at low frequencies (<40 Hz). On the other hand, in both adapting conditions, there appears to be an improvement in spatial acuity for gratings vibrating at 40 and 80 Hz. At 80 Hz, where the effect of adaptation on spatial acuity was strongest, the mean GOTs decreased from 2.9 to 2.2 mm after adaptation at 60 Hz; after adaptation at 250 Hz, thresholds measured using 80-Hz gratings decreased by 0.4 mm. An ANOVA was performed to assess the reliability of the effect of adaptation on GOT. The main effect of adaptation was not significant for either adaptation condition [F(1,60) = 0.15 and 0.56, P > 0.5 for both conditions]. However, there was a significant frequency × adaptation condition interaction for the 60-Hz adaptor F(5,60) = 3.33, P < 0.01] but not for the 250-Hz adaptor [F(5,60) = 2.01, P >0.05], suggesting that the effect of RA adaptation on spatial acuity at the high vibratory frequencies was statistically reliable. Post hoc inference testing revealed that the improvement in acuity at 80 Hz was significant with the 60-Hz adaptor [t(10) = 4.25, P < 0.01] but not the 250-Hz adaptor t(10) = 2.07, P > 0.05].
Fig. 6.
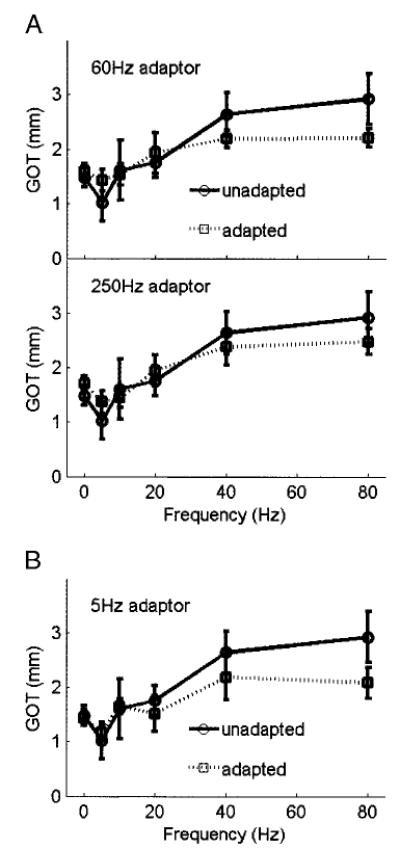
A: effect of vibratory adaptation at 60 and 250 Hz on OD. The 60-Hz adaptor produces a significant improvement in performance at the high frequencies while having little effect at the low frequencies. The 250-Hz adaptor produces a similar but nonsignificant effect. These results suggest that the RA signal interferes with the spatially modulated signal conveyed by SA1 fibers. B: effect of vibratory adaptation at 5 Hz on OD. The effect of the 5-Hz adaptor is almost identical to that of the 60-Hz adaptor, suggesting that the improvement in OD after vibratory adaptation is due to the effect of the adaptor on RA rather than PC fibers.
The possibility remains that the effect of the 60-Hz adaptor on OD was in part or in whole due to a decrement in PC sensitivity. Indeed, a 60-Hz, 40-μm stimulus evokes a robust response in these fibers (Bolanowski and Zwislocki 1984; Freeman and Johnson 1982a; Johansson et al. 1982) and may therefore have also adapted them. To test this hypothesis, we implemented a third adaptation condition, in which the conditioning stimulus was set to 5 Hz and 200 μm. Given the insensitivity of PC fibers to 5-Hz vibrations (Johansson et al. 1982), a conditioning stimulus at that frequency is unlikely to affect PC fibers. On the other hand, RA fibers produce a strong response to 5-Hz vibrations (Freeman and Johnson 1982b; Johansson et al. 1982) and are thus liable to be adapted by these vibrations if they are of sufficient intensity. If a substantial improvement in OD was observed after 5-Hz adaptation, then the effect of adaptation on GOTs is likely due to a desensitization of RA fibers (the 5-Hz stimulus may have desensitized SA1 fibers as well, but this effect was likely negligible, see DISCUSSION). As shown in Fig. 6B, the decrement in GOT at 40 and 80 Hz produced by the 5-Hz adaptor was almost identical to that produced by its 60-Hz counterpart: the main effect of adaptation was not significant [F(5,60) = 1.74, P > 0.15], whereas the frequency × condition interaction was significant [F (5,60) = 2.76, P < 0.05]. Again, post hoc inference testing revealed that the improvement in spatial acuity at 80 Hz was statistically significant [t(10) = 3.56, P < 0.01].
Thus vibratory adaptation targeting RA afferent fibers caused a significant improvement in OD for gratings vibrating at the high flutter frequencies while having little to no effect on OD for static gratings or gratings vibrating at low flutter frequencies. On the other hand, vibratory adaptation targeting PC fibers appears to have little effect on OD performance.
DISCUSSION
One of the central findings in the present study is that spatial acuity decreases with vibratory frequency (Fig. 3). The observed effect of frequency on GOT is not due to differences in effective intensity, as manipulating the stimulus amplitude had little effect on OD (Fig. 4). The decline in spatial acuity at higher vibratory frequencies is puzzling given that the spatial modulation of SA1 and RA afferent fibers changes little (or even increases) with increasing vibratory frequency (Bensmaia et al. 2005a). It is possible that GOTs depend on the strength of the SA1 or RA signals. The correlations between the spike rate evoked in SA1 and RA fibers and GOTs is –0.37 and 0.54, respectively; thresholds tend to be lower (and spatial acuity higher) when the spike rate evoked in SA1 fibers is high and higher when the RA spike rate is high. However, these response measures do not individually account for the effect of vibratory frequency on spatial acuity. Thus the observed decrement in spatial acuity with vibratory frequency cannot be directly attributed to changes in the spatial information available at the periphery.
Vibratory adaptation
In experiment 4, an intense suprathreshold vibratory stimulus was presented to desensitize one of two populations of mechanoreceptive fibers. Two of the vibratory stimuli (at 5 and 60 Hz) targeted RA fibers, one (at 250 Hz) targeted PC fibers. The frequencies, amplitudes, and durations of the adapting stimuli were chosen based on a study investigating the effects of vibratory adaptation on afferent sensitivity (Bensmaia et al. 2005b; Leung et al. 2005). Based on these neurophysiological recordings, we estimate that the absolute threshold of RA fibers increased, on average, by a factor of 2.75 after stimulation with a 60-Hz, 40-μm stimulus. In contrast, the estimated relative shifts in SA1 and PC thresholds produced by this adapting stimulus were 0 and 15%, respectively. Thus at the periphery, the effect of the 60-Hz adaptor was likely restricted to RA fibers. Similarly, we estimate that PC thresholds increased on average by a factor of 2.5 after stimulation with a 300-Hz, 10-μm stimulus; as PC sensitivity is similar at 250 and 300 Hz, the threshold shift produced in the present experiment is probably comparable to that observed in these neurophysiological recordings (we used a 250-Hz stimulus rather than a 300-Hz stimulus because the stimulator cannot deliver well-controlled vibrations at the higher frequency). No neurophysiological data exist to estimate the effect of a 5-Hz, 200-μm adaptor on afferent thresholds; at this frequency, however, PC adaptation is likely to be virtually nil as PC fibers are highly insensitive to 5-Hz vibrations (Johansson et al. 1982). The 5-Hz adaptor may also have desensitized SA1 fibers, but this effect was likely negligible given the similarity between the effects of the 5- and 60-Hz adaptors.
As the central component of vibratory adaptation is thought to involve short-term temporal dynamics in networks of neurons in SI cortex (Lee and Whitsel 1992; Lee et al. 1992), it is difficult to predict the effect of central adaptation on spatial acuity. We thus wished to minimize the effects of the adapting stimulus on central neurons. Afferent adaptation has been found to operate on an exponential time scale with mean time constants ranging from 10 to 40 s depending on the afferent type (Leung et al. 2005). In contrast, central adaptation seems to operate on a longer time scale as evidenced by the fact that the time course of psychophysical adaptation is longer than that of afferent adaptation. The duration of the initial conditioning stimulus was chosen to be long enough to fully adapt peripheral fibers but short enough to minimally affect central neurons.
RA interference hypothesis
The respective contributions of SA1 and RA fibers to fine spatial perception have been a matter of debate for decades (see Johnson and Hsiao 1992 for a review). The consensus is that although the SA1 signal is more sensitive to the fine spatial structure of a tactile stimulus, the RA signal also conveys some spatial information, as has been shown in studies involving the Optacon (Craig 1976). Here we investigate the extent to which RA fibers contribute to fine spatial perception when both SA1 and RA signals are present. To that end, in this and the companion study (Bensmaia et al. 2005a), we manipulated the strength of the SA1 response relative to its RA counterpart and measured the effect of this manipulation on spatial sensitivity. We conclude that fine spatial perception relies on SA1 fibers and that the RA signal interferes with its SA1 counterpart when the system is probed at the limits of its spatial resolution. We base this conclusion on the following findings.
First, SA1 afferent fibers are more spatially modulated than their RA counterparts at all frequencies and amplitudes tested in the present study (Bensmaia et al. 2005a). It is thus likely that SA1 fibers convey the spatial signal that mediates OD performance at threshold, as has been argued previously (Phillips and Johnson 1981).
Second, GOTs were found to increase with vibratory frequency. This phenomenon cannot be explained in terms of the spatial modulation of the peripheral responses as modulation is relatively independent of vibratory frequency, nor can it be explained in terms of the strength of the responses in each population of afferent fibers (Bensmaia et al. 2005a). However, GOT is highly correlated with the ratio of the strength of the RA signal to that of the SA1 signal. Thus the resolution of the sensory representation of a tactile stimulus is proportional to the strength of the SA1 signal and inversely proportional to that of the RA signal. Conceptually, the SA1 response constitutes the spatial signal and the RA response, the noise. Sensitivity to the fine spatial features of a tactile stimulus, as gauged by the GOT, increases as the signal to noise ratio (SNR) increases. Of course, the limit on spatial acuity is set by the innervation density of Merkel receptors; this maximum spatial resolution, which we may be measuring with the gratings vibrating at 5 Hz, is only modulated by the SNR.
Third, OD performance improves when the strength of the RA response is reduced by presenting a strong adapting stimulus targeting this population of fibers (see Fig. 6, A, top and B) as predicted from the interference hypothesis. In contrast, adapting PC fibers does not have a significant effect on spatial acuity (Fig. 6A, bottom).
That the RA signal interferes with the SA1 signal in a spatial acuity task, and thus reduces rather than improves the resolution of the spatial signal, may be interpreted as evidence that the role of the RA channel is not spatial in nature. Although RA responses to gratings and other spatial patterns are spatially modulated (Bensmaia et al. 2005a), the spatial information conveyed by RA fibers may not be functionally significant in naturalistic situations. However, in the absence of a robust SA1 signal, e.g., when spatial patterns are presented with the Optacon, the RA fibers can convey spatial information, albeit at a lower resolution than their SA1 counterparts.
Mechanisms of RA interference
The increase in GOT with vibratory frequency cannot be explained in terms of peripheral responses alone. Indeed, the spatial modulation of SA1 and RA responses is nearly independent of vibratory frequency across the range of frequencies tested (Bensmaia et al. 2005a). Furthermore, the relationship between the strength of the SA1 or RA response and spatial acuity is weak. Here, we provide evidence that the effect of vibratory frequency on spatial acuity is due to the interference of one peripheral signal with another, a phenomenon that must occur centrally, where these two signals converge onto a single brain structure. The nature of this interference remains to be elucidated. There are at least two possibilities. On the one hand, information from SA1 and RA afferent fibers may converge onto single neurons in the primary somatosensory cortex (SI). According to this scenario, then, the responses of individual cortical neurons receiving both SA1 and RA input will be spatially modulated to the extent that their input is dominated by SA1 signals as SA1 fibers convey a more spatially defined signal than their RA counterparts. In this case, the interference occurs at the single-neuron level.
Another possibility is that SA1 and RA signals do not appreciably converge in cortex but rather stimulate distinct populations of cortical neurons. In this case, the responses of the individual neurons that carry the finest spatial information (and receive their input from SA1 fibers) are not perturbed. The RA signal interferes with its SA1 counterpart because it produces an increase in cortical activity without a commensurate increase in the availability of spatial information. In this case, there is no net decrement in spatial information at the cortical level but rather an increase in activity irrelevant to the spatial task.
Neurons in SI have been traditionally classified as slowly adapting if they produce a sustained response to a step indentation and as rapidly adapting if they only produce a response at the onset and offset of the indentation (Hyvärinen and Poranen 1978; Mountcastle and Powell 1959; Paul et al. 1972; Powell and Mountcastle 1959; Sur et al. 1984). The SA/RA distinction in SI has been interpreted as evidence that information originating from SA1 and RA fibers remains segregated as it ascends the perceptual pathway. In other words, individual SI neurons are thought to receive signals from one type of afferent fiber. The apparent segregation of SA and RA pathways seems to rule out the hypothesis that the interference is operating at the single-neuron level; rather, it suggests that an increase in the peripheral RA response causes an increase in the activity of a population of neurons distinct from that carrying the signal that underlies the finest spatial discriminations of which humans are capable.
Two caveats are in order. First, even in SI, the distinction between SA and RA neurons is not absolute: many RA cortical neurons exhibit a weak sustained response throughout step indentations and many SA cortical neurons produce off responses (Sur et al. 1984). In fact, the responses of neurons in areas 3b and 1 to precisely controlled step indentations (delivered by means of the 400-probe stimulator described above) fall along a continuum between slowly and rapidly adapting (P. Denchev and A. Sripati, personal communication). To the extent that SA1 and RA signals converge onto single SI neurons, neuron-level interference could underlie the decrement in spatial acuity at the high flutter frequencies. Second, the interference may take place upstream from or along a pathway parallel to SI, for instance in the second somatosensory cortex (SII), which has been implicated in spatial acuity tasks: SII lesions have been shown to produce severe deficits in a task analogous to OD (the horizontal/vertical task in Murray and Mishkin 1984). Importantly, there is much greater convergence of the SA and RA submodalities in SII as evidenced by the fact that the responses of SII neurons to step indentations fall along a continuum between slowly and rapidly adapting (Burton and Sinclair 1990).
The two hypotheses of interference, neuron-level versus population-level interference, can be tested by examining the responses of cortical neurons to static and vibrating gratings. The neuron-level interference hypothesis predicts that individual neurons should exhibit more spatially modulated responses to static gratings and gratings vibrating at low frequencies than to gratings vibrating at the high flutter frequencies. In contrast, the population-level interference hypothesis predicts that the activity of a subpopulation of cortical neurons (namely those that receive RA input) should be higher when stimulated by high-frequency than by low-frequency gratings, whereas the spatial modulation of the responses of the most spatially modulated neurons (namely those that receive SA1 input) should be relatively insensitive to the stimulus frequency.
Conclusions
A major finding in the present study is that the ability to distinguish the fine spatial features of a stimulus decreases substantially as the frequency at which it vibrates increases (Fig. 3). The frequency dependence of spatial acuity is not due to changes in the quality of the peripheral neural signal as the spatial modulation of SA1 and RA responses to vibrating gratings has been found to be approximately constant across frequencies (Bensmaia et al. 2005a). Furthermore, as spatial acuity is relatively independent of stimulus amplitude (Fig. 4), the effect of vibratory frequency on acuity cannot be attributed to differences in the effective stimulus intensity. However, spatial sensitivity increases as the strength of the SA1 response increases and decreases as the strength of the RA response increases (Fig. 5). In fact, reducing the strength of the RA response results in an improvement in spatial acuity (Fig. 6). We hypothesize that the RA signal, which increases over the range of frequencies tested, interferes centrally with the spatially modulated signal conveyed by SA1 fibers. We propose that this interference effect underlies the observed frequency dependence of the spatial acuity.
Acknowledgments
We thank A. Watson and L. Carey for participating in the data collection and J. Yau and M. Hollins for careful reading of the manuscript.
Footnotes
Bensmaia et al. (2005a) presented stimuli lasting between 100 and 500 ms while the duration of the stimuli presented in the present study was 1 s. These differences in stimulus duration were taken into account in computing the relative strength of the SA1 and RA responses. Specifically, for static stimuli, RA afferents respond only to the onset and offset of the stimulus while SA1 afferents respond throughout the indentation. Thus the relative strength of the SA1 and RA response evoked by a 1-s stimulus is underestimated if it is computed on the basis of firing rates evoked by a 100-ms stimulus. For instance, RA fibers produce as many spikes in response to the 100-ms stimulus as they do to 1-s stimulus, so the estimated firing rate (spikes/unit time) will decrease in proportion to the stimulus duration. To take into account stimulus duration, then, we verified that a 100- and a 500-ms ramp-and-hold stimulus evoke the same total number of spikes in an RA afferent and computed the RA response to the static gratings accordingly. Furthermore, we compared the SA1 response evoked by a 100-ms stimulus to that evoked by a 500-ms stimulus (from Bensmaia et al. 2005a) and, on the basis of this comparison, estimated the SA1 response to a 1-s stimulus.
GRANTS
This work was supported by National Institutes of Health Grants NS-18787, NS-38034, and DC-00095.
References
- Bensmaia SJ, Craig JC, Yoshioka T, Johnson KO. SA1 and RA responses to static and vibrating gratings. J Neurophysiol. 2005a;95:1771–1782. doi: 10.1152/jn.00877.2005. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Bensmaia SJ, Leung YY, Hsiao SS, Johnson KO. Vibratory adaptation of cutaneous mechanoreceptive afferents. J Neurophysiol. 2005b;95:3023–3036. doi: 10.1152/jn.00002.2005. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Bliss JC, Katcher MH, Rogers CH, Shepard RP. Optical-to-tactile image conversion for the blind. IEEE Trans Man-Machine Sys. 1970;11:58–65. [Google Scholar]
- Bolanowski SJ, Gescheider GA, Verrillo RT, Checkosky CM. Four channels mediate the mechanical aspects of touch. J Acoust Soc Am. 1988;84:1680–1694. doi: 10.1121/1.397184. [DOI] [PubMed] [Google Scholar]
- Bolanowski SJ, Zwislocki JJ. Intensity and frequency characteristics of Pacinian corpuscles. I. Action potentials. J Neurophysiol. 1984;51:793–811. doi: 10.1152/jn.1984.51.4.793. [DOI] [PubMed] [Google Scholar]
- Burton H, Sinclair RJ. Second somatosensory cortical area in macaque monkeys. I. Neuronal responses to controlled, punctate indentations of glabrous skin on the hand. Brain Res. 1990;520:262–271. doi: 10.1016/0006-8993(90)91714-r. [DOI] [PubMed] [Google Scholar]
- Craig JC. Vibrotactile letter recognition: the effects of a masking stimulus. Percept Psychophys. 1976;20:317–326. [Google Scholar]
- Craig JC. Some factors affecting tactile pattern recognition. Int J Neurosci. 1983;19:47–57. doi: 10.3109/00207458309148645. [DOI] [PubMed] [Google Scholar]
- Craig JC. Tactile pattern perception and its perturbations. J Acoust Soc Am. 1985;77:238–246. doi: 10.1121/1.392265. [DOI] [PubMed] [Google Scholar]
- Craig JC, Johnson KO. The two-point threshold: not a measure of tactile spatial resolution. Curr Dir Psychol Sci. 2000;9:29–32. [Google Scholar]
- Craig JC, Rollman GB. Somesthesis. Annu Rev Psychol. 1999;50:305–331. doi: 10.1146/annurev.psych.50.1.305. [DOI] [PubMed] [Google Scholar]
- Freeman AW, Johnson KO. A model accounting for effects of vibratory amplitude on responses of cutaneous mechanoreceptors in macaque monkey. J Physiol. 1982a;323:43–64. doi: 10.1113/jphysiol.1982.sp014060. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Freeman AW, Johnson KO. Cutaneous mechanoreceptors in macaque monkey: temporal discharge patterns evoked by vibration, and a receptor model. J Physiol. 1982b;323:21–41. doi: 10.1113/jphysiol.1982.sp014059. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Gardner EP, Palmer CI. Simulation of motion on the skin. I. Receptive fields and temporal frequency coding by cutaneous mechanoreceptors of Optacon pulses delivered to the hand. J Neurophysiol. 1989;62:1410–1436. doi: 10.1152/jn.1989.62.6.1410. [DOI] [PubMed] [Google Scholar]
- Gibson GO, Craig JC. Tactile spatial sensitivity and anisotropy. Percept Psychophys. 2005;67:1061–1079. doi: 10.3758/bf03193632. [DOI] [PubMed] [Google Scholar]
- Goldreich D, Kanics IM. Tactile acuity is enhanced in blindness. J Neurosci. 2003;23:3439–3445. doi: 10.1523/JNEUROSCI.23-08-03439.2003. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Greenspan JD, Kenshalo DR, Sr, Henderson R. The influence of rate of skin indentation on threshold and suprathreshold tactile sensations. Somatosens Res. 1984;1:379–393. doi: 10.3109/07367228409144556. [DOI] [PubMed] [Google Scholar]
- Hollins M, Roy EA. Perceived intensity of vibrotactile stimuli: the role of mechanoreceptive channels. Somatosens Mot Res. 1996;13:273–286. doi: 10.3109/08990229609052583. [DOI] [PubMed] [Google Scholar]
- Hyvärinen J, Poranen A. Receptive field integration and submodality convergence in the hand area of the post-central gyrus of the alert monkey. J Physiol. 1978;283:539–556. doi: 10.1113/jphysiol.1978.sp012518. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Johansson RS. Tactile sensibility in the human hand: receptive field characteristics of mechanoreceptive units in the glabrous skin area. J Physiol. 1978;281:101–123. doi: 10.1113/jphysiol.1978.sp012411. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Johansson RS, Landstrom U, Lundstrom R. Responses of mechano-receptive afferent units in the glabrous skin of the human hand to sinusoidal skin displacements. Brain Res. 1982;244:17–25. doi: 10.1016/0006-8993(82)90899-x. [DOI] [PubMed] [Google Scholar]
- Johnson KO, Hsiao SS. Neural mechanisms of tactual form and texture perception. Annu Rev Neurosci. 1992;15:227–250. doi: 10.1146/annurev.ne.15.030192.001303. [DOI] [PubMed] [Google Scholar]
- Johnson KO, Lamb GD. Neural mechanisms of spatial tactile discrimination: neural patterns evoked by Braille-like dot patterns in the monkey. J Physiol. 1981;310:117–144. doi: 10.1113/jphysiol.1981.sp013540. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Johnson KO, Phillips JR. Tactile spatial resolution: I. Two-point discrimination, gap detection, grating resolution, and letter recognition. J Neurophysiol. 1981;46:1177–1191. doi: 10.1152/jn.1981.46.6.1177. [DOI] [PubMed] [Google Scholar]
- Johnson KO, Van Boven RW, Hsiao SS. The perception of two points is not the spatial resolution threshold. In: Boivie J, Hansson P, Lindblom U, editors. Touch, Temperature, and Pain in Health and Disease: Mechanisms and Assessments. Seattle: IASP; 1994. pp. 389–404. [Google Scholar]
- Johnson KO, Van Boven RW, Phillips JR. Operation Manual: J.V.P. Domes For Cutaneous Spatial Resolution Measurement. Wood Dale, IL: Stoelting; 1997. pp. 1–6. Catalog 18020. [Google Scholar]
- Lee CJ, Whitsel BL. Mechanisms underlying somatosensory cortical dynamics. I. In vivo studies. Cereb Cortex. 1992;2:81–106. doi: 10.1093/cercor/2.2.81. [DOI] [PubMed] [Google Scholar]
- Lee CJ, Whitsel BL, Tommerdahl M. Mechanisms underlying somatosensory cortical dynamics. II. In vitro studies. Cereb Cortex. 1992;2:107–133. doi: 10.1093/cercor/2.2.107. [DOI] [PubMed] [Google Scholar]
- Leung YY, Bensmaia SJ, Hsiao SS, Johnson KO. Time course of vibratory adaptation and recovery in cutaneous mechanoreceptive afferents. J Neurophysiol. 2005;94:3037–3045. doi: 10.1152/jn.00001.2005. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Mountcastle VB, Powell TPS. Neural mechanisms subserving cutaneous sensibility, with special reference to the role of afferent inhibition in sensory perception and discrimination. Bull Johns Hopkins Hosp. 1959;105:201–232. [PubMed] [Google Scholar]
- Murray EA, Mishkin M. Relative contributions of SII and area 5 to tactile discrimination in monkeys. Behav Brain Res. 1984;11:67–85. doi: 10.1016/0166-4328(84)90009-3. [DOI] [PubMed] [Google Scholar]
- Paul RL, Merzenich MM, Goodman H. Representation of slowly and rapidly adapting cutaneous mechanoreceptors of the hand in Brodmann’s areas 3 and 1 of Macaca mulatta. Brain Res. 1972;36:229–249. doi: 10.1016/0006-8993(72)90732-9. [DOI] [PubMed] [Google Scholar]
- Pawluk DTV, van Buskirk CP, Killebrew JH, Hsiao SS, Johnson KO. Control and pattern specification for a high density tactile display. In: Furness RJ, editor. Proc of the ASME Dynamic Systems and Control Division, ASME International Mechanical Engineering Congress and Exposition. Vol. 64. Anaheim, CA: DSC; 1998. pp. 97–102. [Google Scholar]
- Phillips JR, Johansson RS, Johnson KO. Responses of human mechanoreceptive afferents to embossed dot arrays scanned across fingerpad skin. J Neurosci. 1992;12:827–839. doi: 10.1523/JNEUROSCI.12-03-00827.1992. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Phillips JR, Johnson KO. Tactile spatial resolution. II. Neural representation of bars, edges, and gratings in monkey primary afferents. J Neurophysiol. 1981;46:1192–1203. doi: 10.1152/jn.1981.46.6.1192. [DOI] [PubMed] [Google Scholar]
- Powell TPS, Mountcastle VB. Some aspects of the functional organization of the cortex of the postcentral gyrus of the monkey: a correlation of findings obtained in a single unit analysis with cytoarchitecture. Bull Johns Hopkins Hosp. 1959;105:133–162. [PubMed] [Google Scholar]
- Stevens SS. Tactile vibration: change of exponent with frequency. Percept Psychophys. 1968;3:223–228. [Google Scholar]
- Sur M, Wall JT, Kaas JH. Modular distribution of neurons with slowly adapting and rapidly adapting responses in area 3b of somatosensory cortex in monkeys. J Neurophysiol. 1984;51:724–744. doi: 10.1152/jn.1984.51.4.724. [DOI] [PubMed] [Google Scholar]
- Vega-Bermudez F, Johnson KO. Skin conformance accounts, in part, for differences in tactile spatial acuity in young subjects but not for the decline in spatial acuity with aging. Percept Psychophys. 2004;66:60–67. doi: 10.3758/bf03194861. [DOI] [PubMed] [Google Scholar]
- Verrillo RT, Fraioli AJ, Smith RL. Sensation magnitude of vibrotactile stimuli. Percept Psychophys. 1969;6:366–372. [Google Scholar]
- Weber EH. De subtilitate tactus (De tactu) In: Ross HE, translator; Ross HE, Murray DJ, editors. E. H. Weber on the Tactile Senses. 2nd ed. Hove, UK: Erlbaum Taylor and Francis; 1996. pp. 19–134. Original work published 1834. [Google Scholar]


