Nitric Oxide Red Blood Cell Membrane Permeability at high and low Oxygen Tension

Abstract

Red blood cell (RBC) encapsulated hemoglobin in the blood scavenges nitric oxide (NO) much more slowly than cell-free hemoglobin would. Part of this reduced NO scavenging has been attributed to an intrinsic membrane barrier to diffusion of NO through the RBC membrane. Published values for the permeability of RBCs to NO vary over several orders of magnitude. Recently, the rate that RBCs scavenge NO has been shown to depend on the hematocrit (percentage volume of RBCs) and oxygen tension. The difference in rate constants was hypothesized to be due to oxygen modulation of the RBC membrane permeability, but also could have been due to the difference in bimolecular rate constants for the reaction of NO and oxygenated vs deoxygenated hemoglobin.

Here we model NO scavenging by RBCs under previously published experimental conditions. A finite-element based computer program model is constrained by published values for the reaction rates of NO with oxygenated and deoxygenated hemoglobin as well as RBC NO scavenging rates. We find that the permeability of RBCs to NO under oxygenated conditions is between 4,400 μm/s and 5,100 μm/s while the permeability under deoxygenated conditions is greater than 64,000 μm/s. The permeability changes by a factor of 10 or more upon oxygenation of anoxic RBCs. These results may have important implications with respect to NO import or export in physiology.

Introduction

Nitric Oxide (NO) is produced in the blood vessel endothelium by nitric oxide synthase, leading to vasodilation through activation of soluble guanyl cyclase present in the smooth muscle cells [1–5]. Hemoglobin reacts rapidly with NO, making it difficult to understand how endothelial-derived NO can reach the smooth muscle cells with so much Hb present in blood [6]. Several mechanisms act to reduce NO scavenging by red blood cells (RBCs) compared to cell-free hemoglobin, thus enabling NO to act as the endothelium-derived relaxation factor [7–16]. These mechanisms include (1) a RBC free zone created adjacent to the endothelium due to the velocity gradient in laminar flow [8, 10, 11], (2) an unstirred layer surrounding the RBCs that results in NO uptake being rate-limited by diffusion of NO to the RBC [7, 14], and (3) an intrinsic, physical RBC membrane barrier to NO diffusion [12, 13, 15–18]. The relative importance of each of these factors is the subject of much debate [12, 14, 16, 19].

Liao and coworkers tested the contribution of the unstirred layer versus a physical membrane barrier to diffusion by conducting experiments where limited amounts of NO were slowly released by an NO-donor with a half life on the order of tens of minutes in the presence of both cell free Hb and RBCs [12]. In these competition experiments, the relative rate of NO uptake by RBCs to NO consumption by cell free Hb was calculated by examining the distribution of reacted Hb in the two fractions (cell free Hb and RBC encapsulated Hb). They argued that because the release of NO was slow and the concentration of the NO-donor was homogeneous, the NO concentration would also be homogeneous and thus limit the formation of an unstirred layer. Liao and co-workers found that in these experiments the rate of NO uptake by RBCs was still several hundred times slower than the reaction with free Hb, implying that a physical membrane barrier is the primary cause of the slow RBC uptake of NO [12]. Others have since argued that even in these competition experiments, one still has an unstirred layer, so that these experiments do not rule out its role in slow uptake [14, 20]. One way to think of this is that even though the NO release from the donor is slow and the NO scavenging is fast, there is always some steady state concentration of NO in solution during the competition experiments. This NO will not be distributed homogeneously due to continuous scavenging by the RBCs, thereby creating an unstirred layer. In addition, whereas cell-free Hb in the competition experiments reacts with released NO at the same rate everywhere in solution, NO released far from RBCs is taken up slower than NO released nearby RBCs. Thus, diffusion of NO still limits the rate of NO uptake in competition experiments, but perhaps not as much as in stopped-flow experiments. Further evidence for a role of the RBC membrane has been provided whereby chemical or physical modification of the RBC membrane resulted in a significant change in the RBC NO uptake rates [15, 16].

Recently, we performed competition experiments under oxygenated and deoxygenated conditions at 15% and 50% hematocrit (Hcts) [19]. Our results are summarized in Table 1. We found that under oxygenated conditions the rate that cell free Hb scavenges NO, k_f, divided by the rate that RBCs scavenge NO, k_r, is about 410 at 15% Hct (consistent with previous results [12] and 140 at 50% Hct. Note that these rates are normalized by the concentration of Hb in each fraction (RBC or cell-free Hb) [19]. The Hct dependence indicates that external diffusion of NO to the RBC plays a significant role in limiting the rate that oxygenated RBCs scavenge NO. Surprisingly, we found that under deoxygenated conditions, k_f/ k_r was about 50 at both Hcts tested [19]. As pointed out by Tsoukias and Popel, the difference in k_f/ k_r may be partially due to the difference in bimolecular rates for the reactions of deoxyHb and OxyHb with NO [20]. The rate for the deoxyHb reaction, k′, is thought to be 3–6 × 10⁷ M⁻¹s⁻¹ and that for the OxyHb, k_ox, reaction is thought to be 4–8 × 10⁷ M⁻¹s⁻¹ [21–27]. The slower the bimolecular rate constant, the smaller the expected difference in k_f/k_r, since any diffusional factors would be less significant if the reaction itself were slower. This hypothesis is supported computationally [20] and by the case of hemoglobin ligands that combine with slower bimolecular rate constants, carbon monoxide and isocyanide [22]. The slower bimolecular rate may also explain, at least partly, why no hematocrit difference was seen for deoxygenated cells. However, since the difference in bimolecular rates is only about 1.5 (a result determined in this work based on analysis of data collected when NO is added to mixtures of oxy and deoxyHb [27]) it may not completely account for the differences between oxygenated and deoxygenated cells. Thus, we proposed that permeability of the RBC membrane limits NO consumption to some degree in a way that is modulated by oxygenation.

Table 1.

Value of k_f/k_r obtained from competition experiments [19].

	15% Hct	50% Hct
Deoxygenated	51 ± 19*	53± 32
Oxygenated	410 ± 93	140 ± 44

^*Values of k_f/k_r are shown as the average ± one standard deviation.

Literature values of the RBC NO permeability, P_m, range from 4 × 10⁻⁴ m s⁻¹ to 0.9 m s⁻¹ (= 400 μm/s to 900,000 μm/s) [13, 20, 28, 29]. In order to determine if measured differences in NO scavenging rates are due to oxygen associated changes in P_m, or simply due to differences in bimolecular rates of the Hb/NO reactions, in this work, we model NO competition experiments and compare the results to experimental data (Table 1) to obtain values for the NO RBC permeability under oxygenated conditions, P_m^oxy, and under deoxygenated conditions, P_m^deoxy.

Experimental Procedures

NO competition simulation development

In order to properly approximate the true geometry of the RBC we modeled it as a biconcave disk using the software package Comsol Multiphysics (Comsol, Inc., Burlington, MA) which entails a partial differential equation-based finite element modeling environment. A finite element model of a single-cell competition experiment was constructed from a biconcave disk RBC mesh and its surrounding cylindrical “plasma” space, separated by a thin-film approximated membrane, under Comsol Multiphysics’s time-dependent diffusion mode solver (Figure 1). For the biconcave disk cell, a cross section was constructed of third degree Bezier curves in the XY plane with control points shown in Figure 2. The cross section was then revolved about the Y axis to produce a biconcave disk. The dimensions of the disk were chosen according to average human red blood cell morphology [30], and the cylinder’s dimensions were adjusted to the desired hematocrit such that the cylinder walls remained an equal distance from the biconcave disk’s radial and facial boundaries.

Figure 1.

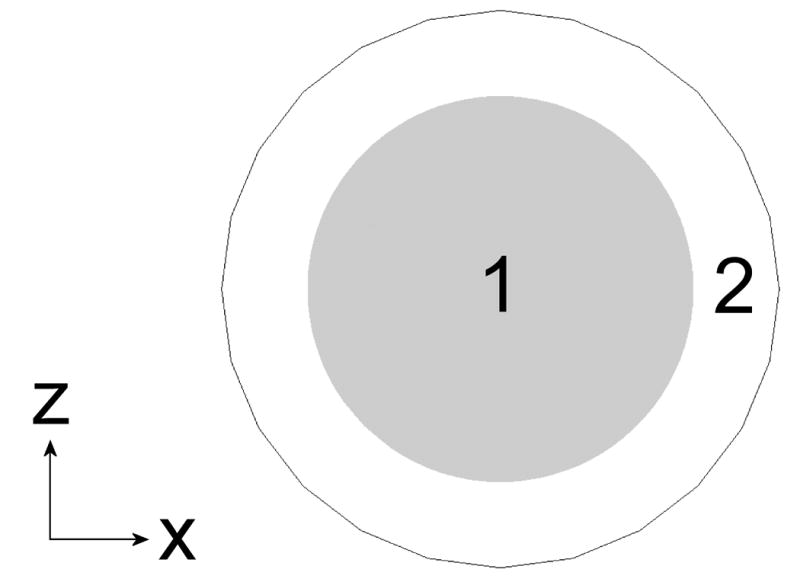
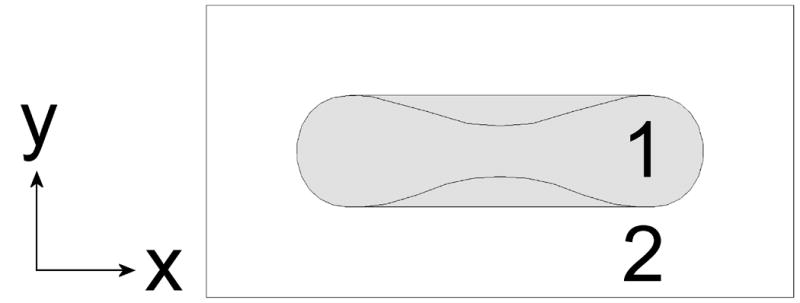
NO competition scenario with biconcave disk (15% HCT shown). To adjust hematocrit, the cylinder width and height are increased by the same distance. A top (A) and side (B) view are shown. Region 1 is the RBC and region 2 is the extracellular space. The NO donor is homogenously distributed in region 2 which also contains cell-free Hb.

Figure 2.

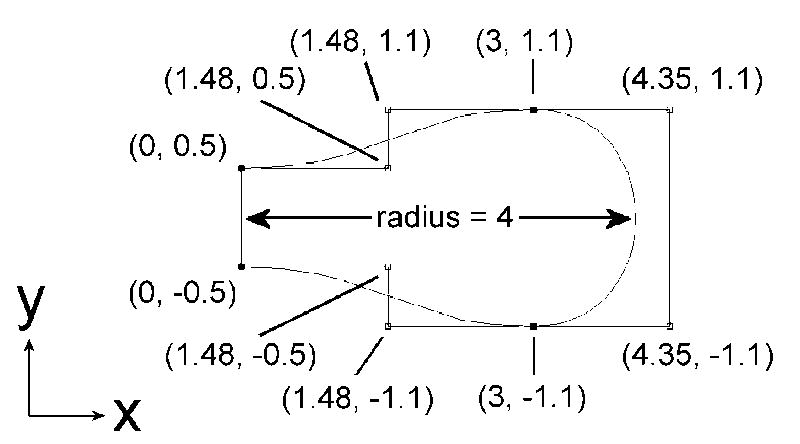
Construction of the biconcave disk model using third degree Bezier curves, with control points shown. A cell radius of 4 μm was chosen to reflect average physiological RBC width.

Governing Equations

The diffusion of both NO and Hb were governed by the equation

$$\frac{\partial C}{\partial t}+\nabla \cdot (-D\nabla C)=R,$$ (1)

where C is the concentration of the species, D is its diffusion rate, and R is its net reaction rate. The NO donor concentration was assumed to be homogeneous in the extracellular compartment. For Hb and intracellular NO,

$$R=k_{Hb}[\text{NO}][\text{Hb}],$$ (2)

where k_Hb is equal to either k′ or k_ox depending on whether the Hb is deoxygenated or oxygenated. In the extracellular space, the overall reaction rate for NO is given by

$$R=2\times k_{donor}[\text{NO donor}]-k_{Hb}[\text{NO}][\text{Hb}],$$ (3)

where k_donor is the rate constant that NO is released by the donor.

Boundary conditions

At the outer boundary of the plasma space,

$$\mathbf{n}\cdot (-D\nabla C)=0,$$ (4)

where n is the unit normal vector. At the RBC membrane,

$$-\mathbf{n}\cdot (-D\nabla C)={\text{P}}_{\text{m}}(C_o-C_i),$$ (5)

where C_o is the concentration at the outer surface of the RBC and C_i is the concentration inside the RBC, and the permeability of Hb through the membrane is zero.

Simulation parameters

The geometries were meshed by Comsol Multiphysics using the “Coarse” predefined meshing parameters. Finer mesh presets were tested and found to produce solutions insignificantly different (<1%) at the expense of significantly increased computing time. A slow-release NO donor was applied homogenously throughout the plasma space, and the entire model was allowed to react with the initial conditions shown in Table 2 for 10 simulation-seconds to correspond with the first 10 seconds of a real experimental reaction, after which the average concentration of NO metabolites (iron nitrosyl Hb (HbNO) for deoxygenated conditions or Methemoglobin (MetHb) for oxygenated conditions) were calculated for both cell and plasma fractions to correspond with experimental cell and plasma fraction measurements. The relative rate, k_f/k_r was then calculated using these simulated data as previously described [19] using equation 6 where [Product] is [HbNO] under deoxygenated conditions and [MetHb] under oxygenation.

Table 2.

Competition simulation parameters

Parameter	Value	Units
RBC volume	90	μm3
RBC diameter	8	μm
Initial [RBC Hb]	20300	μM (in heme)
Hct	0.01–60	%
Initial [NO donor]	10	μM
kHb (kox or k′)	20–100	μM−1s−1
kdonor	3.2 × 10−5	s−1
NO diffusion rate in cell	880–1600	μm2/s
NO diffusion rate in plasma	3300	μm2/s
Hb diffusion rate in cell	0.27	μm2/s
Hb diffusion rate in plasma	1	μm2/s
Initial [cell free Hb]	2–200	μM
RBC membrane permeability Pm	1000–400000	μm/s
Initial [NO]	0	μm
Initial [HbNO] or [MetHb]	0	μm

$$\frac{{[\text{Product}]}_f}{{[\text{Product}]}_r}=\frac{k_f{[Hb]}_f}{k_r{[Hb]}_r}$$ (6)

The time dependent solver was run using a relative tolerance of 0.01 and an absolute tolerance of 0.001. Various reaction durations were tested, and the resultant relative rate remained constant provided pseudo-first order conditions with respect to the concentrations of Hb and NO were maintained; the relative rate’s time independence is both expected and observed [19]. A range of parameters (Table 2) was used, with the results compiled by Matlab (Mathworks Inc, Natick MA). Mass conservation of donor, NO, hemoglobin, and their products was observed in all simulations.

Simulation verification

To verify the finite element method, the spherical competition model and conditions described by Tsoukias and Popel [20] were recreated in Comsol Multiphysics and allowed to run for 10 simulation seconds. The finite element solution agrees well with the previously published analytical solution, with an average deviation of 7.8% and a maximum deviation of 13% from the analytical result.

Ratio of rate constants for OxyHb and DeoxyHb reactions, k_ox/k′

Most of the data used to calculate k_ox/k′ was published by our lab previously [27]. Briefly, NO buffer was added to either Hb or whole blood at various oxygen saturations and the HbNO yield (amount of HbNO formed/amount of NO added) was determined using electron paramagnetic resonance (EPR). To insure that previously published results were not compromised due to a bolus effect (where concentrated NO in saturated buffer exhausts local heme sites before it can be properly mixed so that secondary chemistry can occur [31–33]) we performed additional experiments using an NO donor, ProliNO (Cayman Chemical (Ann Arbor, MI)). A stock solution of ProliNO was first prepared in pH 10, 0.05 M Trizma base buffer (Sigma Chemicals, St. Louis, MO) and a couple of microliters of the stock was diluted into 800 μL of Hb at a concentration of 3.4 mM in pH 7.4, 0.1 M sodium phosphate buffer to give a final concentration of ProliNO of 50 μM. The HbNO yield was the determined as described previously [27]. No significant difference was observed when NO was added to our concentrated Hb samples either as a bolus or using the NO donor.

The HbNO yield was plotted against Hb oxygen saturation, Y. The HbNO yield (assuming no competing reactions such as those involving nitrosation) is predicted to be

$$\text{HbNO\hspace{0.17em}yield\hspace{0.17em}}=\frac{[\text{Fe}(\text{II})\text{NO}]}{{[\text{NO}]}_0}=\frac{1-Y}{1-Y+rY},$$ (6)

where r = k_ox/k′. The value of k_ox/k′ was determined by fitting the HbNO yield data to Eq. 6.

Results

In order to determine the membrane permeability of RBCs we conducted simulations of competition experiments performed at different oxygen saturations and Hcts (previously published data summarized in Table 1). Figure 3A plots the calculated values of k_f/k_r vs the membrane permeability and bimolecular reaction rate k_ox for oxygenated conditions with 15% Hct. For a given membrane permeability, raising k_ox results in a larger calculated value of k_f/k_r. This is expected as for a very slow bimolecular reaction rate between NO and Hb, one expects diffusion of NO to the RBC to be unimportant whereas for a very fast bimolecular reaction rate one expects that only NO that is produced in the immediate vicinity of a RBC will be able to diffuse to it before being scavenged by extracellular Hb. For a given bimolecular rate constant, as the membrane permeability increases, the calculated value of k_f/k_r decreases. This is expected as when the RBC permeability is very low, almost all of the NO would be scavenged by cell-free Hb, and k_f/k_r would be very large. Figure 3B plots the calculated values of k_f/k_r vs the membrane permeability and bimolecular reaction rate k_ox for oxygenated conditions with 50% Hct. The Hct dependence of k_f/k_r is attributed to the time it takes the NO to diffuse to the RBC [19]. At a higher Hct, the NO has less far (on the average) to diffuse to the RBC, so k_f/k_r is lower. Thus, as expected, we find lower values of k_f/k_r at 50% Hct compared to 15% Hct. Experimentally, we found that k_f/k_r ≈150 ± 50 at 50% Hct and k_f/k_r ≈400 ± 100 at 15% Hct [19]. The experimental values, together with our calculations summarized in Figures 3A and 3B, place a limit on allowable values for P_m^oxy and k_ox. Given that the bimolecular reaction rate and the membrane permeability should be independent of Hct, we can combine these limitations as illustrated in Figure 3C. The black shaded region indicates values of P_m^oxy and k_ox that are consistent with all the data collected under oxygenated conditions.

Figure 3.

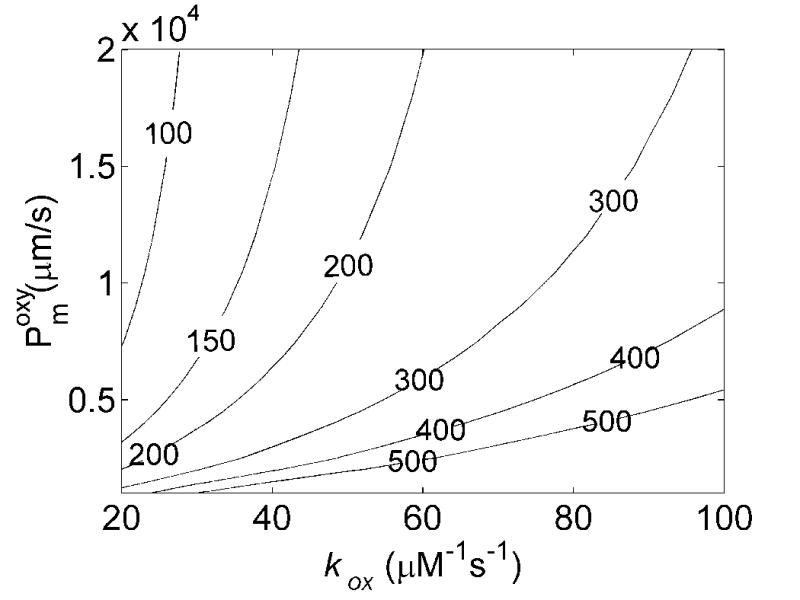
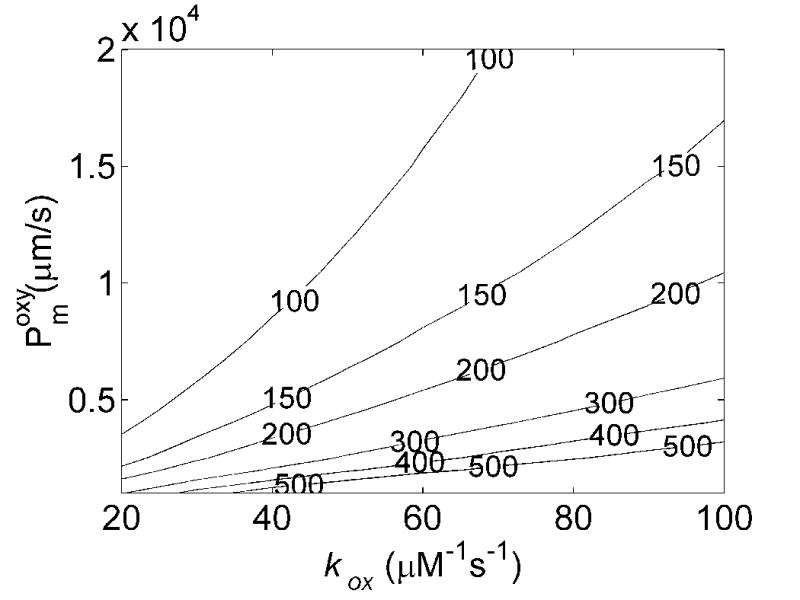
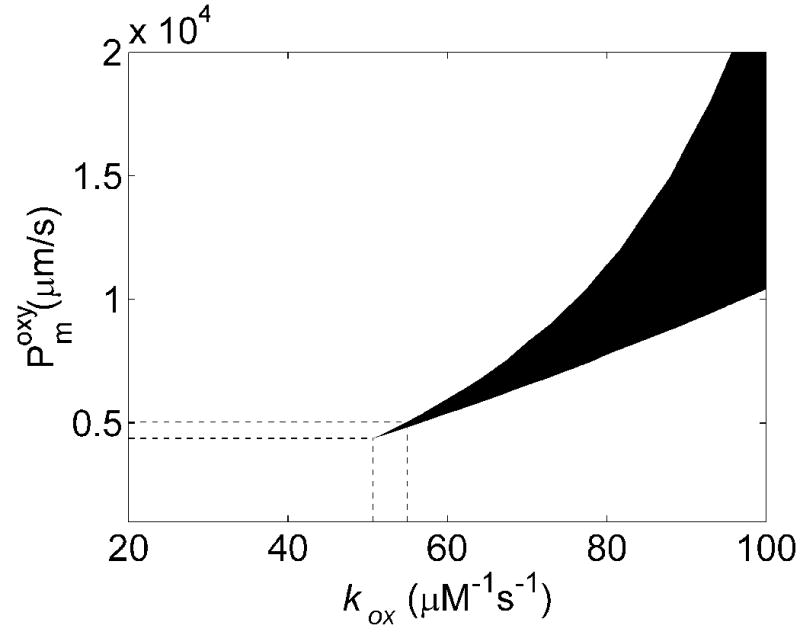
Effects of membrane NO permeability, P_m^oxy, and bimolecular reaction rate, k_ox, on k_f/k_r. A) Contour plot of k_f/k_r vs the bimolecular reaction rate and RBC membrane permeability with 15% hematocrit, 20 μM cell-free Hb and B) 50% hematocrit, 200 μM cell-free Hb. The numbers on the contours correspond to the values of k_f/k_r. Concentrations of cell-free Hb were chosen to be representative of those used experimentally [19]. Small variations in the amount of cell free Hb used were found to have little effect on the calculated values of k_f/k_r, consistent with experimental results [12]. C) Allowable values of P_m and k_ox are shown. Incorporation of experimentally measured values of k_f/k_r (Table 1) constrain allowable calculated values of k_f/k_r from 3A and 3B giving the black shaded region. The minimum value of k_ox allowable is 50.6 μM⁻¹s⁻¹ and the minimum value for P_m is 4400 μm/s. Additional constraint is obtained incorporating the maximum value of the bimolecular rate of the reaction of deoxyHb and NO, k′, and r = k_ox/k′ (Figure 5). These provide upper limits for P_m and k_ox (shown with the lines extending from horizontal and vertical axes) given by k_ox = 55.0 μM⁻¹s⁻¹ and P_m = 5100 μm/s.

The simulated values of k_f/k_r under deoxygenated conditions as functions of membrane permeability and k′ at 15% and 50% hematocrit are shown in Figures 4A and 4B. Similar trends in the dependence of k_f/k_r on P_m and k′ are seen as those observed for oxygenated conditions. Figure 4C summarizes the allowed P_m^deoxy and k′ when restricted to regions where k_f/k_r ≈ 50 ± 25 at 50% Hct and k_f/k_r ≈ 50 ± 40 at 15% Hct. Here it was found that any membrane permeability was consistent with the data that was above a minimal value. The maximal value of P_m^deoxy shown in the plot is that corresponding to the largest one quoted in the literature corresponding to the permeability of a lipid bilayer [13, 20, 28, 29]. Thus, values shown in the gray shaded region in Figure 4 were consistent with the data collected at 50% Hct. Those consistent with data collected at 15% Hct are shown in the black shaded region. In order to achieve a given value of k_f/k_r as k′ increases P_m^deoxy must also increase so that rapid diffusion through the RBC membrane can make up for rapid scavenging by cell-free Hb. Thus, it is seen that the minimal value for P_m^deoxy increases as k′ increases. The results for low Hct constrained the possible values of P_m^deoxy and k′ more than those at high Hct. Here, even when the permeability is essentially infinite, the data cannot be fit using a value of k′ above 31.6 μM⁻¹s⁻¹.

Figure 4.

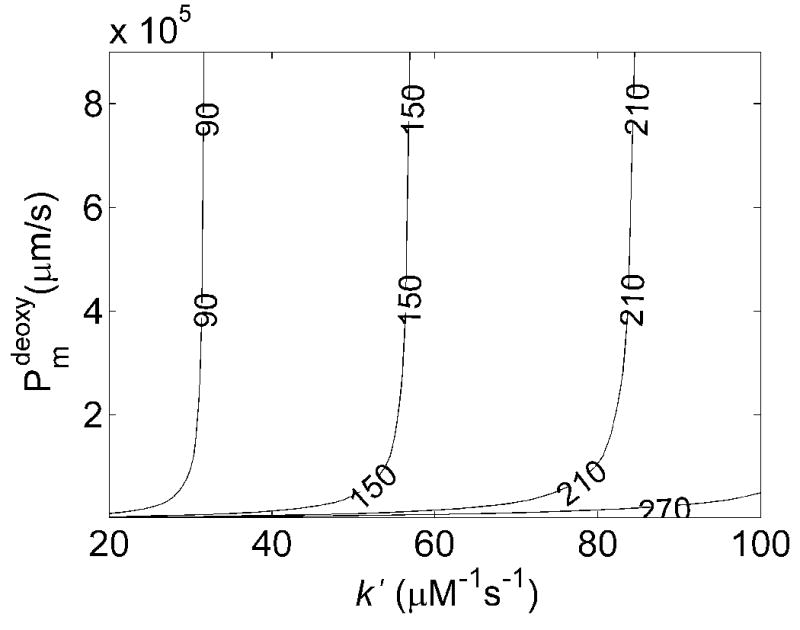
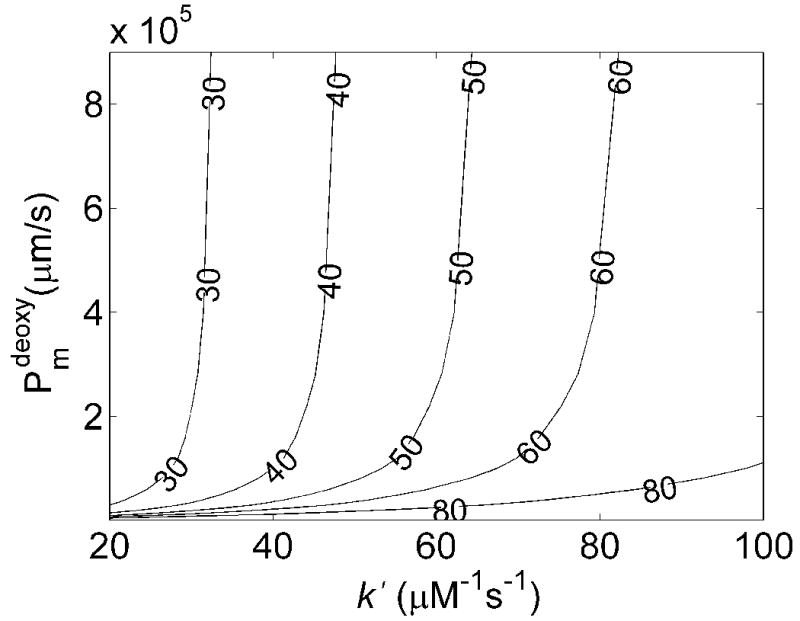
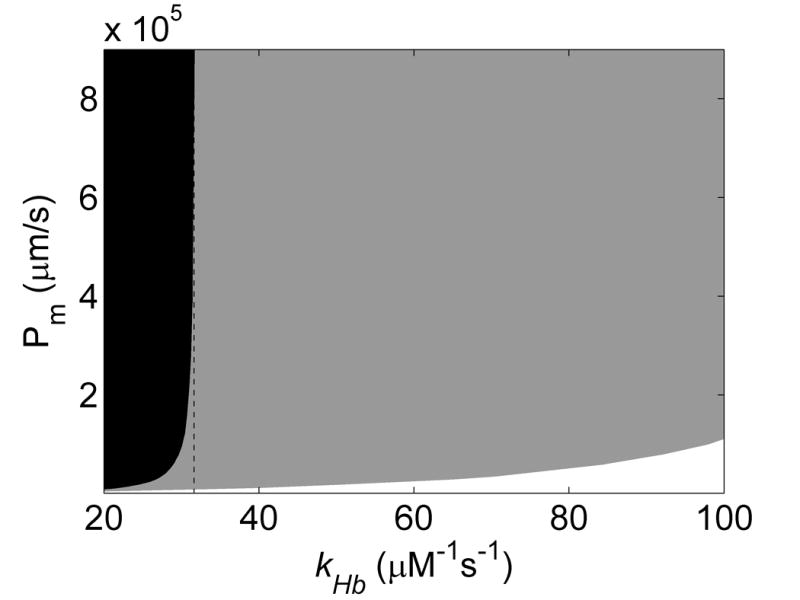
Effects of membrane NO permeability, P_m^deoxy, and bimolecular reaction rate, k′, on k_f/k_r. A) Contour plot of k_f/k_r vs the bimolecular reaction rate and RBC membrane permeability with 15% hematocrit, 20 μM cell-free Hb and B) 50% hematocrit, 200 μM cell-free Hb. C) Contour data from 15% (black) and 50% (gray) Hct were overlaid, showing allowed regions where P_m^deoxy and k′ satisfy experimental observations (black) for NO competition performed under deoxygenated conditions (Table 1). The maximum k′ is 31.6 μM⁻¹s⁻¹. The minimum value of P_m^deoxy of 64,000 μm/s is obtained at the lowest value of k′ allowable given the constraints on r and k_ox, P_m^deoxy = 29.1 μM⁻¹s⁻¹.

Consideration of the ratio r = k_ox/k′ further constrains allowable values for k_ox, k′, P_m^oxy, and P_m^deoxy. In order to obtain the ratio of k_ox to k′, NO was added to partially oxygenated Hb or blood and the amount of HbNO was measured to calculate the HbNO yield [27]. The HbNO yield was then plotted against the Hb oxygen saturation (Figure 5). At 100% oxygen saturation the HbNO yield is zero since all of the Hb is converted to MetHb via the reaction governed by k_ox. At partial oxygen saturations some HbNO is made and the amount depends on the ratio k_ox/k′ according to Eq. 6. The value of r can be obtained from the data shown in Figure 5 by performing a least square fit. The result is that r = 1.56 ± 0.18 (P ≤ 0.05). Given that we have a maximum value for k′ based on Figure 4, our value of r provides a maximum value of k_ox = 55.0 μM⁻¹s⁻¹. This information is incorporated into Figure 3C to provide a maximum possible value for P_m^oxy = 5100 μm/s. Similarly, the minimum value of P_m^oxy from Figure 3C gives and minimum value of k_ox which (incorporating r) gives a minimum value of k′ and thereby (using Figure 4) a minimum value of P_m^deoxy = 64,000 μm/s. The results of our calculations and comparison to experiments are summarized in Table 3. One sees that P_m^deoxy is at least five times greater than P_m^oxy.

Figure 5.

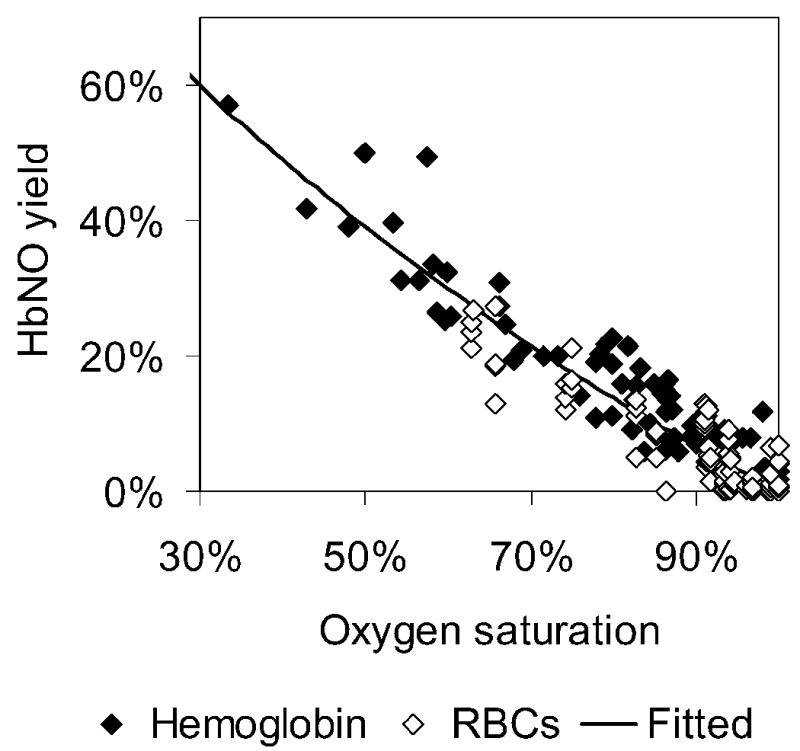
HbNO yield at different Hb oxygen saturations. Data are shown for Hb and RBCs. Most of the data shown were published previously [27] where NO was added using buffer but some additional data on Hb were collected for this figure using an NO donor ProliNO. The solid line represent the best fit of Eq. 6 to the data and corresponds to a value of r = 1.56. A 95% confidence level gives r = 1.56 ± 0.18.

Table 3.

Summary of simulation results

	Minimum	Maximum	Units
k′	29.1	31.6	μM−1s−1
kox	50.6	55.0	μM−1s−1
r	1.62	1.74
Pmoxy	4400	5100	μm/s
Pmdeoxy	64000	900000	μm/s

Discussion

Data collected on the relative rate of NO scavenging at different Hcts and oxygenation states constrains possible values of P_m^oxy and P_m^deoxy, as well as the values for the bimolecular rate constants for Hb reactions with NO, k_ox and k′. We find that k′ must be within the range of 29.1–31.6 × 10⁷ M⁻¹s⁻¹ and k_ox must be within the range of 5.16–5.50 × 10⁷ M⁻¹s⁻¹, consistent with previously published values [21–27]. In addition, we show that k_ox is 1.4 to 1.7 times greater than k′. We find that P_m^deoxy must be greater than 64,000 μm/s and P_m^oxy must be between 4,400 μm/s and 5,100 μm/s so that P_m^deoxy must be at least five times greater than P_m^oxy.

Previous measurements on anaerobic red cells found that pre-treatment of hypoxic RBCs with NO increases the NO uptake rate. [18]. The authors suggested that the accelerated NO uptake was due to formation of HbNO [18]. One may suggest that our previously published experimental results [19] showing faster NO uptake by deoxygenated RBCs is due to formation of HbNO. However, this suggestion is not supported by the fact that our measurements of k_f/k_r did not increase in time as more HbNO formed [19]. Liao and coworkers proposed a mechanism for the modulation of NO uptake by erythrocytes in which HbNO binds Band 3 tetramers in the cytoskeleton and thereby displaces ankyrin [18]. Band 3 is a membrane protein that, through interaction with ankyrin, binds to spectrin which (along with actin and protein 4.1) makes up the fibrous protein skeleton of the membrane [34]. The cytoplasmic N-terminus of band 3 binds to the 2,3-disphosphoglycerate binding site of deoxygenated Hb with high enough affinity so that a significant fraction of band 3 molecules may be bound to deoxyHb under physiological conditions [35, 36]. Our work suggests that the binding of deoxygenated Hb to Band 3 may be primarily responsible for disruption to the cytoskeleton barrier and changes in RBC NO permeability.

Our result is surprising when one considers that NO is readily soluble in lipids and the RBC membrane has a lot of surface area that is likely to be free of proteins. Thus, it is difficult to see how Hb binding to the RBC cytoskeleton can affect NO permeability. Another possibility is that NO reacts with oxygen in the lipid bilayer of the RBC membrane and this leads to an apparent decrease in the RBC NO uptake rate under oxygenated conditions. The reaction of NO and O₂ is often thought to be of little significance when Hb is around but it may be more important when the concentration of NO is low and it has been demonstrated that the reaction of NO with O₂ is accelerated in the hydrophobic interior of membranes [37, 38]. In our simulation we have ignored the NO/O₂ reaction as the NO and oxygen would have to be concentrated to an extraordinary result to compete with Hb reactions. Although our work suggests that there is an oxygen-linked regulation of RBC permeability, other mechanisms, such as the NO/oxygen reaction may be at work. Alternatively, the RBC membrane may (somehow) accelerate the decay rate of the NO donor under anaerobic conditions.

At this time it is difficult to evaluate the implications of our results in vivo. Our experiments showed that even when the Hb was not 100% deoxygenated, RBC NO scavenging increased, implying potential physiological relevance to hypoxic conditions [19]. This could be important in hemolytic anemias such as sickle cell disease where increased NO scavenging under hypoxia would be detrimental. It is also interesting to speculate about whether or not our results concerning NO uptake of oxygenated vs deoxygenated cells may be extrapolated to export by RBCs of NO or some other nitrogen oxides. However, the competition experiments modeled here do not include the impact of a cell-free zone, which is likely to be a major factor in controlling NO scavenging in vivo. Further work is required to test the relative importance of the intrinsic rates of RBC NO scavenging, and their modulation by oxygen, under physiological conditions.