Coronary tortuosity: a long and winding road

Abstract

Coronary tortuosity is a phenomenon often encountered by cardiologists performing coronary angiography. The aetiology and clinical importance of coronary tortuosity are still unclear. Coronary tortuosity without fixed atherosclerotic stenosis in patients with angina pectoris and an abnormal exercise stress test has never been described in the literature.

This article describes three cases of patients with anginal complaints, an abnormal exercise stress test and coronary angiography without the presence of a fixed atherosclerotic lesion.

It is hypothesised that coronary tortuosity leads to flow alteration resulting in a reduction in coronary pressure distal to the tortuous segment of the coronary artery, subsequently leading to ischaemia. Future studies will be necessary to elucidate the actual mechanism of coronary tortuosity and its clinical significance. (Neth Heart J 2007;15:191-5.)

Coronary tortuosity, an anatomical variant, is a phenomenon often encountered during coronary angiography.^1-3 Unfortunately, the aetiology and clinical importance of coronary tortuosity are unclear.

In general, atherosclerotic arteries tend to be more tortuous than others. Apart from the pulsatile arterial movement, the coronary vascular bed has repetitive flexion and relaxation during each cardiac cycle.¹

The combination of coronary tortuosity without fixed atherosclerotic stenosis in patients with anginal complaints and an abnormal exercise stress test has never been described in the literature. In this article three cases are reported of patients with anginal complaints, an abnormal exercise stress test and coronary tortuosity without fixed atherosclerotic lesions. A hypothesis about the possible mechanism is put forward. The sparse literature on arterial tortuosity and especially coronary tortuosity is reviewed.

Case 1

A 48-year-old man was referred to the hospital because of exercise-induced chest pain, typically disappearing at rest and after nitrates. The patient had no relevant clinical history.

Physical examination showed no abnormalities (RR 125/65 mmHg). Laboratory findings and echocardiography were normal. An ECG showed no abnormalities. Thallium-201 and Persantine myocardial perfusion single photon emission computed tomography (SPECT) showed a reversible defect in the anterior wall, apex and distal inferior wall. The stress test provoked chest pain. The patient was treated with atenolol, acetylsalicylic acid and atorvastatin.

Coronary angiography showed tortuosity of the left anterior descending artery and the circumflex artery without a fixed coronary stenosis (figure 1).

Figure 1.

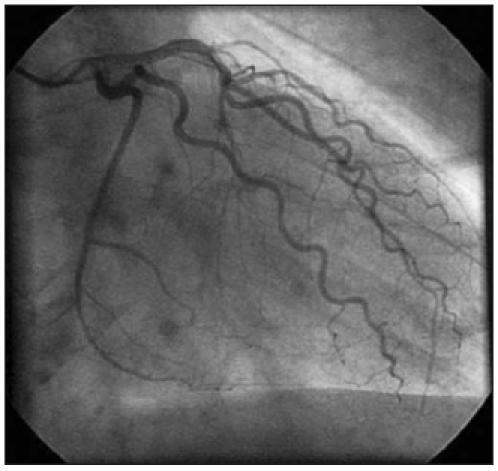
Coronary angiography performed in patient 1 showed tortuosity of the left anterior descending artery and the circumflex artery without a fixed coronary stenosis.

Case 2

A 34-year-old woman was referred to our hospital because of typical exercise-induced chest pain. She had undergone renal transplantations 20 and 14 years previously, because of renal dysplasia after vesicoureteral reflux. Physical examination showed no abnormalities (RR 120/80 mmHg). Laboratory findings showed a slightly abnormal renal function test (creatinine 140 μmol/l). An ECG showed no abnormalities. Technetium-99m tetrofosmin rest/stress myocardial SPECT during adenosine-induced coronary vasodilation showed a reversible defect in the anterior wall and apex. She was treated with atenolol, acetylsalicylic acid, lisinopril and atorvastatin. Coronary angiography showed tortuosity of all coronary arteries without a fixed coronary stenosis (figure 2).

Figure 2.

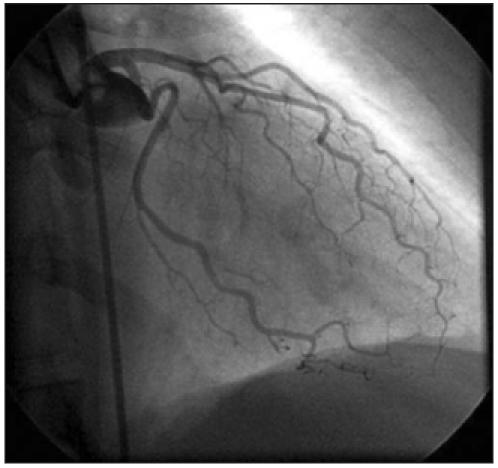
Coronary angiography performed in patient 2 showed tortuosity of the left anterior descending artery and the circumflex artery without a fixed coronary stenosis.

Case 3

A 51-year-old man was referred because of exerciseinduced chest pain, typically disappearing at rest and after nitrates. Clinical history 20 years previously revealed a non-seminomatous testicular tumour with thoracoabdominal metastases treated with surgical resection and chemotherapy using cisplatin, bleomycin and etoposide. Complete remission was achieved. Three years later a benign mature teratoma located in the posterior mediastinal space was surgically removed.

Physical examination showed no abnormalities (RR 138/82 mmHg). Laboratory findings were normal. An ECG showed signs of left ventricular hypertrophy. Echocardiography, however, showed no abnormalities. SPECT during adenosine-induced coronary vasodilation showed a reversible defect in the anterobasal wall and apex. The patient was treated with metoprolol, carbasalate calcium and simvastatin. Coronary angiography showed tortuosity of all coronary arteries. No fixed stenotic lesions were seen.

Discussion

Arterial tortuosity has been described in several vascular systems and organs.^2-13 More specifically, coronary tortuosity has also been described.^1-3 A clear uniform definition of coronary tortuosity has not yet been established. The aetiology of coronary tortuosity is still unclear. Traction and pressure in the lumen are two forces that tend to lengthen a vessel. These two forces together are opposed by a retractive force. Normally, the retractive force is equal and opposite to the sum of the traction and pressure forces resulting in a stable length of the vessel.¹⁴ The retractive force is generated almost entirely by elastin. Degeneration of elastin in the arterial wall leads to aneurysmal dilatation and development of arterial tortuosity.¹⁴ In general, tortuosity of arteries is caused by age-dependent or pathological changes of the elastic material in the vessels.4,11,14,15 An example of the latter can be found in the arterial tortuosity syndrome. This is a rare autosomal recessive connective tissue disorder associated with generalised tortuosity and elongation of all major arteries and involvement of the skin and joints. Arterial changes are especially found in the aorta and coronary arteries.¹⁶ The underlying gene defect has not yet been identified. The aetiology of tortuous variants in other cases is considered mainly acquired and linked with atheroma, atherosclerosis, ageing and hypertension.6,7,11,17 Coronary tortuosity may be more pronounced during systole and may be less clear in large hearts.^1-3 Of the three coronary arteries the circumflex artery is most often affected, especially when associated with hypertension.² Tortuosity is more often seen in atherosclerotic arteries than in other arteries.⁵ It was shown in tortuous nonstenotic femoral arteries that the severity of abnormal blood flow dynamics may affect progression of atherosclerosis.^18,19The causative effects of wall shear stress on atherogenesis have received much attention and are still being debated. Mechanical forces related to the dynamics of blood flow in arteries have been proposed as factors that lead to the development of atherosclerosis.^20,22-24

In tortuous arteries, like the carotid arteries, abnormalities such as kinking, coiling and tortuosity were associated with haemodynamic changes in the vascular bed distal to the abnormalities.⁷ The role of carotid artery tortuosity in relation to neurological symptomatology is, however, also under debate.

There are no literature references to tortuosity of the coronary arteries in normal hearts in relation to anginal complaints and abnormal exercise stress tests.

We put forward the hypothesis that coronary tortuosity leads to flow alteration resulting in a reduction in coronary perfusion pressure distal to the coiling of the coronary artery ultimately leading to ischaemia.

Fluid mechanical theories give a plausible explanation for the pressure reduction, caused by energy loss in the distal bed. Winding arteries cause higher energy loss than straight ones; therefore, perfusion pressure will also be reduced. There are two causes for energy loss leading to pressure reduction. One is friction through shear stress and the other is the centrifugal effect. This may be explained as follows:

The energy loss in a straight tube will be determined by the friction loss (ΔE_fr). This can be calculated with Poiseuille’s law:

ΔE_fr = 32 η l v/d²

(ΔE_fr = energy loss by friction; η = absolute viscosity of blood; l = length artery; v= velocity; d = diameter artery)

Bends give extra energy loss (figure 3), almost entirely caused by eddies, which originate because the flow has to separate from the wall due to a sharp bend (separation). Because of the increase in centrifugal overpressure (P_co) on section AB in the outside bend and the decrease in underpressure (P_ci) on section CD in the inside bend, areas will be built up where the flow may separate from the wall; this is accompanied by eddies and extra energy losses (ΔE_sep).²⁵ The fastest particles are pressed outwards by the centrifugal effect, the original symmetrical velocity profile will be asymmetrical and a secondary transverse flow is generated perpendicular to the main flow. Gijsen et al. showed in their report about non-Newtonian influence measured and calculated patterns of main and transverse flow in a 90° bend of an artery during systole and diastole.²⁶

Figure 3.

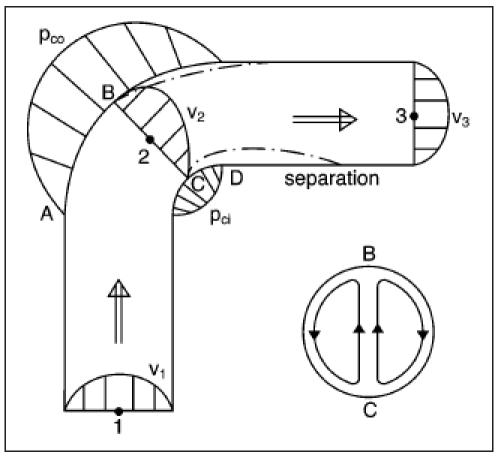
The energy loss in a straight tube will be determined by the friction loss (ΔE_fr). This can be calculated with Poiseuille’s law. Bends give extra energy loss almost entirely caused by eddies, which originate because the flow has to separate from the wall due to a sharp bend (separation). Because of the increase in centrifugal overpressure (P_co) on section AB in the outside bend and the decrease in the underpressure (Pci) on section CD in the inside bend, areas will be built up where the flow may separate from the wall; this is accompanied by eddies and extra energy losses (ΔE_sep).²⁵ The fastest particles are pressed outwards by the centrifugal effect, the original symmetrical velocity profile will be asymmetrical and perpendicular to the main flow a secondary transverse flow is generated.

Nippert concluded from model measurements that at turbulent water flow in tubes only small losses were caused by the transverse flow. Sharp bends, however, will generate high-energy losses caused by separation.²⁷ He showed a relation between R (radius)/D (width tube) and ΔE_sep/ΔE_fr for turbulent flow in a 90° bend of a rectangular wooden tube (figure 4). The friction loss ΔE_fr is nearly constant for different R/D values and is almost equal to E_fr in straight arteries.

Figure 4.

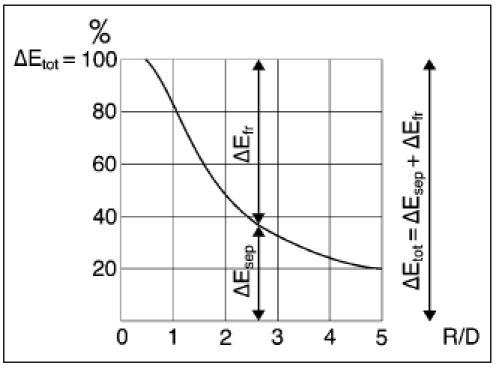
Nippert concluded from model measurements that sharp bends will generate high energy losses caused by separation.²⁷He showed a relation between R (=radius)/D (=width tube) and ΔE_sep/ΔE_frfor turbulent flow in a 90° bend of a rectangular wooden tube. The loss by separation ΔE_sep is at R/D = 2 equal to the friction loss ΔE_fr ; so the total energy loss in the bend is twice as high as in a straight tube. At R/D = 1 the total energy loss is even five times higher (ΔE_sep = appr. 4 ΔE_fr).

The loss by separation ΔE_sep is at R/D = 2 equal to the friction loss ΔE_fr ; so the total energy loss in the bend is twice as high as in a straight tube. At R/D = 1 the total energy loss is even five times higher (ΔE_sep = approx. 4 ΔE_fr).

In figure 5, two 90° bends with R₁/D=3 and R₂/D=1 are drawn. From figure 4 we may conclude that the total energy loss at R₂ is much higher than at R₁.

Figure 5.

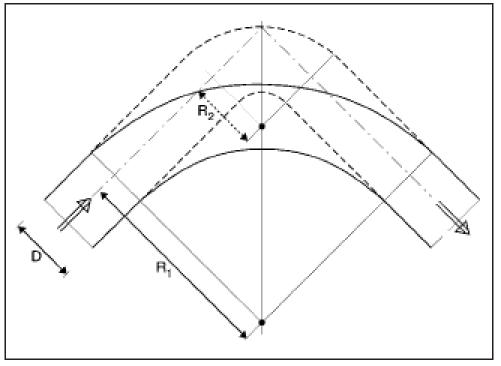
Two 90° bends with R₁/D=3 and R₂/D=1 are drawn. From figure 4 we may conclude that the total energy loss at R₂is much higher than at R₁.

The pressures, velocities and energy losses are related in the energy equation. The basic assumption is that the sum of potential energy (pressure P), kinetic energy (local velocity v) and energy losses (shear stress: ΔE_fr, separation: ΔE_sep) is constant:

P₁ + 1/2 ρ v₁² = P₂ + 1/2 ρ v₂² + ΔE_1-2fr = P₃ + 1/2 ρ v₃² + ΔE_1-3fr + ΔE_sep

(ρ=density)

The energy equation with regard to the main flow in the bend is presented in figures 3 and figure 6. While v₂ is higher than v₁ and ΔE_1-2fr is relatively small, P₂ is smaller than P₁. After the bend the velocity v² decreases to v₃ (=v₁): the pressure increases but will be lower than p₁ because of the energy loss by the eddies of the separation.

Figure 6.

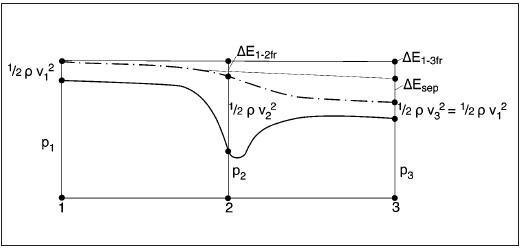
The pressures, velocities and energy losses are related in the energy equation. The basic assumption is that the sum of potential energy (pressure P), kinetic energy (local velocity v) and energy losses (shear stress: ΔE_fr, separation: ΔE_sep) is constant. While v₂ is higher than v₁ and ΔE_1-2fr is relatively small, P₂ is smaller than P₁ (see also figure 3). After the bend the velocity v2 decreases to v₃ (=v₁): the pressure increases but will be lower than p1 because of the energy loss by the eddies of the separation.

Of course, model research with turbulent flow in rectangular tubes is hardly comparable with laminar blood flow. But in the coronary arteries, the cardiac cycle with its high flow fluctuations will cause turbulence, certainly directly after a flow peak. Caro et al. indicate that separation in the main arteries occurs in inside bends with a small radius.²⁸ The mechanism of higher losses in the bends at lower values of R/D will quite likely be present in arteries.

We have to prove that these losses are significant and cause an insufficient perfusion pressure. The sharper and the more numerous the bends are, the higher the energy loss and with that the pressure loss. Conceivably, coronary tortuosity leads to flow alteration resulting in a reduction in coronary perfusion distal to the curves of the coronary artery, possibly leading to ischaemia.

To prove our hypothesis that this reduction in coronary perfusion between the beginning and the end of a tortuous coronary artery can lead to ischaemia, a reduction in pressure has to be determined. A theoretical calculation is hardly possible because of the complexity of the blood flow. Research, for instance with coronary flow reserve, seems to be the best option. However, the present wires tend to stretch the bends and introduce the risks of an invasive procedure. Because of the diffuse nature of coronary tortuosity, imaging of the ischaemic area with SPECT will be difficult. A possible option will be positron emission tomography (PET) scanning. However, PET scanning will not be able to differentiate between ischaemia caused by coronary tortuosity or a capillary-cellular block as is suggested in syndrome X.^29,30 Model research seems another option to prove the hypothesis and to find a relation between pressure reduction and coronary tortuosity, i.e. radius, angle and number of bends. Nevertheless, model research hardly corresponds with real coronary physiology.

We are aware that there might be a compensatory mechanism of the tortuous coronary system which will compensate for the theoretical decrease in perfusion pressure in coronary tortuosity. If such a mechanism exists without signs of ischaemia, abnormal stress tests in these patients might be falsely positive.

If research develops in this field, one should define its population e.g. coronary tortuosity. We define coronary tortuosity as two or more segments of the coronary arteries with three or more curvatures ≤120° measured during diastole.

In order to select and classify patients with coronary tortuosity, a coronary tortuosity classification based on the above-mentioned hypothesis has to be designed. A classification with visible indications for the size of the pressure reduction is desirable. Nippert concluded that the bend radius has a direct relation with the pressure reduction, but it is difficult to measure this parameter in the coronary circulation. The bend angle is more easily measurable, but it is a less accurate indication. Future research should result in a practical basis for a coronary tortuosity classification.

Conclusion

This report describes three cases of patients with a history of anginal complaints, an abnormal stress test and coronary tortuosity without haemodynamically significant stenosis. This association has not been reported before. One explanation could be coincidence: coronary tortuosity happens to be present in a false-positive exercise test. Our hypothesis, however, that coronary tortuosity leads to flow alteration resulting in a reduction in coronary pressure distal to the tortuous segment of the coronary artery leading to ischaemia is a plausible explanation. We propose that the sharper and more numerous the coronary bends the higher the energy loss and subsequently pressure loss. Further studies will be necessary to elucidate the actual mechanism and determine the clinical significance of coronary tortuosity.