Abstract
While the number of variant Creutzfeldt–Jakob disease (vCJD) cases continues to decline, concern has been raised that transmission could occur directly from one person to another through routes including the transfer of blood and shared use of surgical instruments. Here we firstly present data on the surgical procedures undertaken on vCJD patients prior to onset of clinical symptoms, which supports the hypothesis that cases via this route are possible. We then apply a mathematical framework to assess the potential for self-sustaining epidemics via surgical procedures. Data from hospital episode statistics on the rates of high- and medium-risk procedures in the UK were used to estimate model parameters, and sensitivity to other unknown parameters about surgically transmitted vCJD was assessed. Our results demonstrate that a key uncertainty determining the scale of an epidemic and whether it is self-sustaining is the number of times a single instrument is re-used, alongside the infectivity of contaminated instruments and the effectiveness of cleaning. A survey into the frequency of re-use of surgical instruments would help reduce these uncertainties.
Keywords: variant Creutzfeldt–Jakob disease, self-sustaining epidemic, mathematical model, epidemiology
1. Introduction
To date it is believed that the majority of clinical cases of variant Creutzfeldt–Jakob disease (vCJD) in the UK have been caused by consumption of Bovine spongiform encephalopathy (BSE)-infected beef (Bruce et al. 1997; Hill et al. 1997; Scott et al. 1999). Despite high estimates of the number of BSE-infected cattle that entered the food supply (in the order of 3–4 million animals (Donnelly et al. 2002)), the number of cases of vCJD has remained low with 161 cases to the end of 2005, and the annual incidence steadily decreasing since 2000. Estimates for the total scale of the epidemic through this route have reduced over time and now lie in the low hundreds (Clarke & Ghani 2005).
A survey of appendix and tonsil tissues (Hilton et al. 2004) estimated a much higher prevalence of infection in the population than suggested by the clinical cases. This finding is best explained by the hypothesis that a large proportion (84.4%) of infections are sub-clinical (i.e. will never go on to develop symptoms within their lifetime; Clarke & Ghani 2005). Evidence for a sub-clinical vCJD state has been found in animal studies (Hill et al. 2000; Hill & Collinge 2003; Bishop et al. 2006). Infection is detectable throughout the CNS and lymphatic system in patients with clinical vCJD (Wadsworth et al. 2001). In addition, the abnormal form of the prion protein (PrPSc) has been detected in the spleen of a patient who died from other causes (Peden et al. 2004) and in the appendix of a vCJD case removed 3 years prior to their death (Hilton et al. 1998). Given that infectious PrPSc is detectable in patients showing no clinical symptoms, it is possible that it could be transmitted through surgical procedures. Surgical instruments are decontaminated routinely before use on another patient. However, research suggests that PrP binds strongly to stainless steel and that current sterilisation procedures are unlikely to be effective because of the high temperatures required to deactivate PrPSc (Flechsig et al. 2001; Yan et al. 2004). Furthermore, a survey of decontamination practices has shown that past practices fell short of the expected standard in many hospitals (Estates 2000). Although steps have been taken to improve the situation (Estates 2001), it is likely that the reality of decontamination is still less than perfect and hence that residual infectivity will remain.
In this paper, we firstly present results from an ongoing case-control study of risk factors for vCJD in relation to previous surgical procedures. We then extend the mathematical framework developed to explore the potential for self-sustaining epidemics via blood transfusions (Clarke et al. submitted) to one appropriate for high- and medium-risk surgical procedures in the UK. Data from hospital episode statistics (HES; www.hesonline.nhs.uk) are used to parameterize the rate and age distribution of high- and medium-risk procedures, and the model is used to assess the current risks and uncertainties associated with an epidemic transmitted by this route.
2. Surgical procedures undertaken on variant Creutzfeldt–Jakob disease cases
Data on previous surgical procedures are routinely collected for all vCJD cases at initial interview with a close relative of the patient (Ward et al. 2006). Furthermore, after death of the patient, medical records are obtained from their GP. According to these medical records, in total 130 patients have undergone a surgical procedure, with 119 patients having undergone a total of 335 surgical procedures prior to the onset of clinical symptoms. Since individual-level data on surgical procedures in the general population are not available, it is difficult to determine whether this rate is higher than average. However, no evidence has been found in the ongoing case-control study that the rate of surgical procedures is higher among cases than in age- and sex-matched controls (Ward et al. 2006).
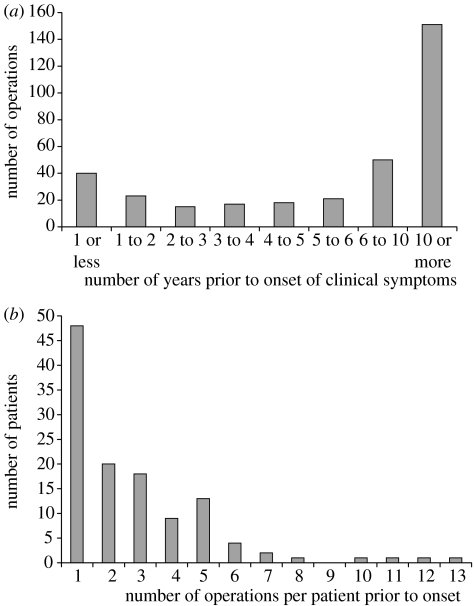
Figure 1a shows the distribution of when surgical procedures were undertaken in relation to the onset of clinical symptoms. While a large proportion (45%) of procedures were undertaken over 10 years prior to onset (when the patients, if infected, could be expected to have low levels of infectivity and hence pose little risk for onward transmission), a substantial number of procedures were undertaken close to clinical onset. A minority of patients underwent multiple procedures (figure 1b). Overall the majority of procedures were classified as medium-risk for vCJD transmission, with only 19 classified as high-risk. For details of the classification of procedures see §3 below. These data therefore suggest that, while the overall rate of high-risk procedures is low, there remains an important potential risk of transmission via surgical instruments.
Figure 1.
The number of operations performed prior to the onset of clinical symptoms on 130 vCJD cases. (a) By the number of years prior to onset of clinical symptoms in the case; (b) frequency of multiple operations.
3. Current rates of operations and classifications in relation to the risk of variant Creutzfeldt–Jakob disease transmission
The distribution of infective prion protein PrPSc is uneven across the human body so that surgical operations on different body parts pose different risks for transmission. A classification of tissues according to the level of infectivity (into high, medium and none; WHO 2003) was translated into a classification of surgical procedures according to OPCS-4 codes (HSMO 1990; see tables 1 and 2).
Table 1.
List of high-infectivity tissues and corresponding OPCS-4 operation codes.
| tissue | corresponding OPCS-4 codes |
|---|---|
| brain | AA: tissue of brain |
| AB: ventricle of brain and subarachnoid space | |
| optic nerve | AC: cranial nerves |
| dura mater | AD: meninges of brain |
| spinal cord | AE: spinal cord and other contents of spinal canal (only A44–A48) |
| pituitary gland | BA: pituitary and pineal glands (without B06) |
| retina | CH: retina and other parts of eye (only C82–C84) |
Table 2.
List of medium-infectivity tissues and corresponding OPCS-4 operation codes.
| tissue | corresponding OPCS-4 code |
|---|---|
| peripheral nerves | AF: peripheral nerves |
| thymus & adrenall | BC: other endocrine glands |
| cornea | CE: conjunctiva and cornea (only C45–C51) |
| lung | EF: lung and mediastinum (only E53–E59) |
| gingival tissue | F20: operations on gingiva |
| tonsil | F34, F36: excision of & other operations on tonsil |
| salivary gland | FE: salivary apparatus (only F44–F48) |
| oesophagus | GA: oesophagus including hiatus hernia |
| stomach | GB: stomach pylorus & gen uppr gastr'inst'l tract endoscop |
| duodenum | GC: duodenum |
| jejunum | GD: jejunum |
| ileum | GE: ileum |
| large intestine | H: lower digestive tract |
| liver | JA: liver |
| pancreas | JD: pancreas |
| spleen | JE: spleen |
| blood vessels | L: arteries and veins |
| kidney | MA: kidney |
| lymph nodes | TG: lymphatic and other soft tissue (only T85–T88) |
| bone marrow | W34: graft of bone marrow |
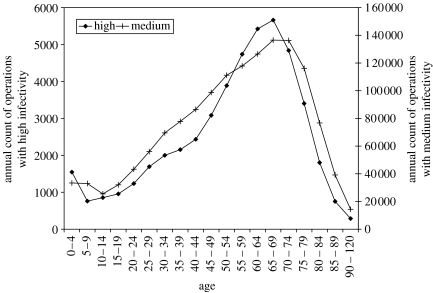
The total number of operations performed in NHS hospitals in England from 1990 to 2004 stratified by the age of the patient at operation were obtained from HES (see figure 2). These numbers were scaled up by a factor of 1.2 to account for the difference in population size between England and Great Britain and by a further factor of 1.15 to account for 15% of operations that are undertaken in the private sector (Economics & Division 2001).
Figure 2.
Age-distribution of the annual number of operations performed in England stratified by the high- and medium-risk classification.
4. Model structure
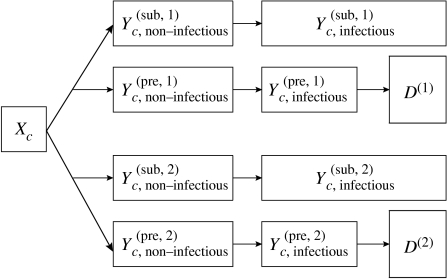
The model used here builds on previous models used to describe vCJD transmission (Ghani et al. 2003; Clarke & Ghani 2005). The population is described in a deterministic compartmental model, stratified by birth cohort c. A flowchart for one cohort of the model is depicted in figure 3. The population is subdivided into susceptibles Xc, primary infected (by beef consumption) and secondary infected (by surgery) . Infection can either be pre-clinical, with individuals going on to develop clinical disease at the end of the incubation period, or sub-clinical, meaning that individuals never develop clinical disease. The first part of the incubation period is assumed to be non-infectious, whereas the later stages of the incubation period are infectious at a constant level. It shall be assumed that the distribution of the non-infectious period for those with sub-clinical infection is identical to that for the pre-clinically infected.
Figure 3.
Flowchart of cohort c of the population: susceptibles Xc and primary/secondary infected . During the first part of the incubation period, pre- as well as sub-clinical individuals are non-infectious, in the later stages of incubation, they are infectious. D(1/2) are the cumulative deaths from primary/secondary infection.
Previous studies have found that the incubation period distribution for pre-clinical vCJD infections is well approximated by a Γ-distribution. To model Γ-distributed incubation periods, the infected population is partitioned into F consecutive compartments with exponentially distributed waiting times in each. More precisely, a pre-clinically infected individual must pass through each of the F stages before dying, becoming infectious after fmin stages. Similarly, a sub-clinically infected individual must pass through fmin stages before becoming infectious. The purpose of the F stages is not to mimic an underlying aetiological process, but to ensure the incubation period is Γ-distributed. Also, the waiting time in the non-infectious and infectious parts of the incubation periods follow Γ-distributions, with means summing to the mean of the total incubation period.
For pre-clinical infection, the onset of clinical symptoms, which inevitably leads to death, occurs upon leaving the last incubation stage. The time span from onset of symptoms to death (approx. 1 year) is short compared to the incubation period (approx. 11 years), and any surgical operations undertaken on a patient known to have vCJD would follow the strictest standards for hygiene, minimizing any transmission after the onset of symptoms. Therefore, patients are removed from the model with the onset of symptoms, and death is effectively assumed to coincide with the onset of clinical disease. In contrast, the sub-clinically infected never leave the last incubation stage, and finally die from causes unrelated to vCJD.
For birth cohort c, which is composed of all individuals born in year c, the differential equations governing the dynamics of the susceptible population are
| (4.1) |
with the delta-distribution δ(·) (defined as δ(x)=0 if x≠0 with a singularity at x=0, and ). Age a=t−c, and for t<c, Xc(t)=0. Bc is the number of births into cohort c (for simplicity assumed to happen at the beginning of year c), and μa is an age-dependent death rate of causes other than vCJD. λc,1 and λc,2 are the time- and age-dependent rates of infection for primary and secondary infection, respectively.
For the primary and secondary infection, we have
| (4.2) |
| (4.3) |
| (4.4) |
| (4.5) |
| (4.6) |
with f=1 … F−1 and i=1 for primary and i=2 for secondary infection, where ωi is the probability of sub-clinical infection, and γi is the rate of progression through the incubation stages.
The cumulative number of deaths from primary and secondary infection, respectively, is given by
| (4.7) |
The parameters λc,1, ω1, γ1 and F are fixed at the maximum-likelihood values obtained by fitting a survival model to the clinical cases of vCJD observed to the end of 2005 assuming that all but one (suspected to have been infected through a blood transfusion) occurred through consumption of infected beef (Ghani et al. 2003; Clarke & Ghani 2005). At the beginning of the epidemic (taken to be in 1980), the whole population is in the susceptible classes, subdivided by birth cohorts. The population size, age distribution and survival distribution are estimated from census data (Ghani et al. 2003; Clarke & Ghani 2005), details can be found in appendix A. In the absence of data, we make the conservative assumption that survival is not affected by having had a surgical procedure.
4.1 Transmission via surgery
To incorporate surgical transmission, additionally we need to track clean and contaminated surgical instruments, which act as vectors for transmission. We assume that surgical instruments are kept in sets, and that the number of sets of surgical instruments n is constant over time. The proportion of sets that are contaminated is denoted by x(t) and the proportion of clean sets by 1−x(t). With this, the rate of infection for secondary transmission, λc,2, is given by
| (4.8) |
where βhi is the transmission coefficient for transmission from surgical instruments to humans, τs is the rate of surgical procedures per person, Ψs(a) is the age distribution of surgical procedures (i.e. the probability that the patient is age a conditioned on the event of surgery), Nc(t) is the number of people alive in cohort c at time t and is the population size at time t. The factor N(t)/Nc(t) is used to adjust the age group specific procedure rate Ψs(a) in equation (4.8) for application to a specific birth cohort (note that generally, age groups are wide and made up of several yearly birth cohorts), under the assumption that events occurring in an age group are allocated to each birth cohort in direct proportion to its surviving population.
Clean instruments are contaminated with a contamination rate χ, which clearly depends on the frequency of operations and the prevalence of infection in the human population. As the rate of operations per set of instruments is τsN(t)/n, we have
| (4.9) |
with the transmission coefficient from humans to instruments βih and the number of infectious people in cohort c given by
| (4.10) |
This assumes that both sub- and pre-clinically infected patients are on average infectious for the last proportion of their incubation period given by ρ=(F−fmin)/F.
The number of operations for which contaminated instruments stay infectious is assumed to follow an exponential distribution with average d. Instruments are replaced at a rate of 1/d per operation, therefore the rate at which contaminated instruments are replaced by clean instruments is given by
| (4.11) |
With this, the proportion of contaminated instruments is given by
| (4.12) |
The frequency of operations and age profiles Ψs(a) were fixed at the values presented in figure 2 with τs=1.13×10−3 operations per person per year for high-risk procedures, and τs=3.41×10−2 operations per person per year for medium-risk procedures. The total number of sets in use n was parameterized via the frequency with which a set of instruments is used, ν and the total number of operation per year, τsN, as ν=τsN/n.
4.2 Calculation of the basic reproductive number R0
If we assume that the total number of infecteds remains small compared to the population size, we can follow the approach by Diekmann et al. (1990) to obtain an analytical expression for the basic reproduction number R0:
| (4.13) |
with
| (4.14), (4.15) |
| (4.15) |
and
| (4.16) |
Here, A is the maximal age that can be reached in the model population, Na is the number of people of age a and S(a′|a) is the survival probability to age a′ conditioned on survival to a. The conditional survival probability is related to the annual death rate at age a, μa, by S(a+1|a)=1−μa. A derivation of this equation can be found in appendix B.
4.3 Numerical simulations
Extensive sampling of parameter space was undertaken using Latin Hypercube sampling, varying most of the input parameters for which no data was available, as detailed in table 3. Scenarios were accepted if they were consistent at the 95% level with having observed no deaths from surgical routes to the end of 2005 (i.e. less than 3.3 expected deaths via this route). About 62% of scenarios for high-infectivity procedures and 8% of scenarios for medium-infectivity procedures were accepted. Simulations were run in batches of 1000 and 10 000 for high- and medium-infectivity procedures, respectively, and the results shown in figure 6 represent a typical batch.
Table 3.
List of key model parameters for which no data was available, and range considered.
| parameter and description | range | |
|---|---|---|
| ω2 | probability of sub-clinical infection | 0–1 |
| γ2 | annual rate of progression through the incubation stages for secondary infection (fixed at the primary infection value) | 0.877 |
| ρpre | proportion of incubation period that is non-infectious | 0–1 |
| βihβhi | combined transmission parameter | 0–1 |
| d | average number of operations for which an instrument stays infectious after initial contamination | 1–100 |
| ν | annual frequency with which a set of instruments is used | 1–50 |
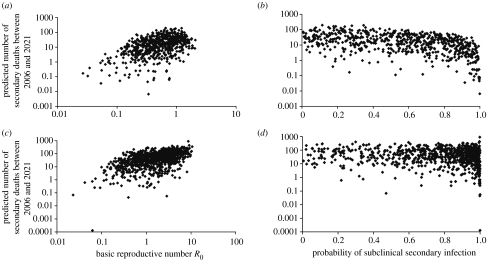
Figure 6.
Predicted number of deaths from secondary infection between 2006 and 2021, dependent on the basic reproductive number R0 and the probability of sub-clinical infection ω2 for high-risk procedures (a and b) and medium-risk procedures (c and d).
5. The potential for a self-sustaining epidemic
Inspection of the model equations and simulations reveals three factors influencing the potential for a self-sustaining epidemic of vCJD via surgical instruments: the infectivity of contaminated instruments, βihβhi, the average number of times an instrument is used (and the subsequent decay in infectivity which may be enhanced by cleaning), d, and the number of operations undergone in different age-groups, Ψ(a). Similarly to the transmission via blood transfusion (Clarke et al. submitted), the overall frequency of surgical procedures τs rather than the age profile Ψs(a) appears to dominate the potential for a self-sustaining epidemic.
The infectivity of contaminated instruments is unknown but in the worst-case scenario can be no greater than one, which occurs if use of a contaminated instrument always results in infection. Thus, for a given frequency of operations, the major unknown determining the potential for a self-sustaining epidemic is the average number of times an instrument is used. It is difficult to put an upper bound on this value; the results presented here consider values up to 100 but it could plausibly be higher. It is not known whether infectivity declines over time or remains constant after initial infection (e.g. if protein is not removed during the first cleaning cycle, does it become more strongly baked onto the instrument?). Here, constant infectivity is assumed, and therefore our results correspond to worst-case scenarios.
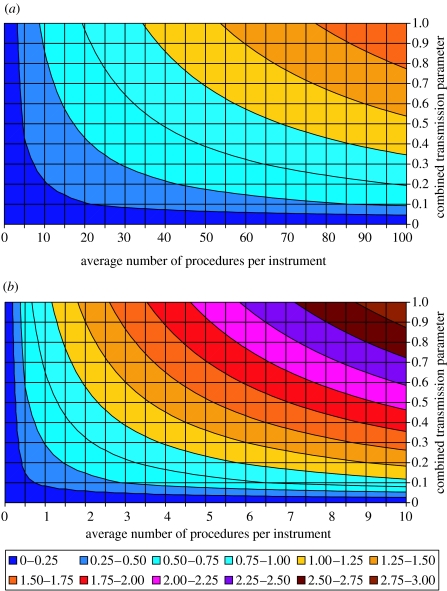
Under the assumption that the cases observed to date have not been infected via surgery, our scenarios give values of the reproductive number R0, ranging from well below 1 (the value required for a self-sustaining epidemic) to approximately 2 for high-risk procedures, and over 10 for medium-risk procedures. Figure 4 shows the relationship between the average number of procedures in which an instrument is used, d, the product of the probability of transmission from an infected person to the instrument, βih, and from a contaminated instrument to a susceptible person, βih (which we term the combined transmission parameter) and the reproductive number for high- and medium-risk surgical procedures, R0. Self-sustaining epidemics can occur via this route at plausible values for d. For example, for high-risk procedures the average number of procedures per instrument need only be greater than 35 for high values of the combined transmission parameter while for lower values an instrument may need to be used up to 100 times.
Figure 4.
The figure shows how the basic reproductive number R0 given in equation (4.13) depends on the combined transmission parameter, βihβhi and the average number of procedures an instrument is used in before it is discarded, d. (a) For high-risk procedures and (b) for medium-risk procedures. For this figure we assume that pre- and sub-clinically infected individuals are infectious throughout their incubation period and that 40% of secondary infections are sub-clinical.
The main difference between the high- and medium-risk procedures stems from the difference in the frequency of operations, with medium-risk procedures occurring approximately 30 times more frequently than high-risk procedures, and in the plausible values for the combined transmission parameter (although it is not possible at this stage to attempt to quantify how much lower this would be). Thus if medium-risk procedures still result in a considerable infection risk, instruments used only 4–8 times could plausibly result in a self-sustaining epidemic.
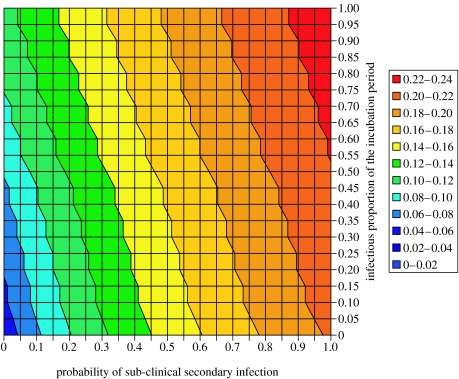
A final uncertainty in the potential for self-sustaining transmission is the probability of sub-clinical infection and how infectious these and asymptomatic individuals are. For simplicity, the model assumes that both pre- and sub-clinically infecteds have a constant infectivity in the later stages of the incubation period. Figure 5 shows the relationship between the infectious proportion of the incubation period ρ, the probability of sub-clinical infection ω2 and the reproductive number R0 for high-risk operations. Higher values of R0 are obtained if the probability of sub-clinical infection is high; such scenarios result in infected individuals living longer and hence being more likely to transmit infection. In contrast, asymptomatic pre-clinical infections play a relatively minor role in onward transmission under such scenarios.
Figure 5.
Basic reproductive number R0 dependent on the probability of sub-clinical infection ω2 and the infectious proportion of the incubation period ρpre for high-risk operations. Parameter values used were the combined transmission parameter βihβhi=1, the average number of re-uses per instrument d=1 and the rate of progression through the incubation stages γ2=0.877 per year.
6. Potential scale of an epidemic
Figure 6 shows the relationship between the reproductive number, the probability sub-clinical infection and the potential numbers of cases between 2006 and 2021. Data shown correspond to results obtained from 1000 and 10 000 different scenarios for high-risk and medium-risk operations, respectively, where those scenarios predicting more than 3.3 deaths to the end of 2005 were discarded as they are not compatible with having observed no cases via this route. This left 621 scenarios for high-infectivity procedures and 828 scenarios for medium-infectivity procedures.
In this range of scenarios cases in the next 15 years range up to approximately 200 for high-infectivity procedures and up to 1000 for medium-infectivity procedures. While the higher scenarios assume an perhaps unrealistically high probability of sub-clinical infection, the scenarios were only run using values of d, the average number of times an instrument is re-used, up to 100. Thus it is possible to generate a much larger range of epidemics if this constraint is relaxed and hence at present it is not possible to provide any guidance on future case numbers through this route.
7. Discussion
Given the frequency of high- and medium-risk surgical procedures undertaken in the UK, a range of plausible scenarios suggest that surgical procedures could provide a potential route for a self-sustaining epidemic of vCJD. The main factors driving such an epidemic are the intrinsic ability of this route to transmit infection (both from infected individual to the instrument and from a contaminated instrument to an individual undergoing surgery with this instrument) and the average number of times a single instrument is re-used. Experimental studies have demonstrated that current sterilization procedures undertaken in hospitals are insufficient to totally remove all infectious PrPSc (Yan et al. 2004; Jackson et al. 2005). However, translating such studies into measurable levels of infectivity in terms of human infectious doses will remain difficult. Thus it will be difficult to assess the potential impact of reducing infectivity on the scale of an epidemic. Our results demonstrate that the level of infectivity for different procedures is particularly important because it appears twice in the expression for the reproductive number: firstly, as the infectivity from infected person to instrument (which is difficult to reduce) and secondly, from contaminated instrument to susceptible individual (which can be reduced through improved cleaning).
The other unknown factor driving the potential for an epidemic is the average number of times an instrument is re-used. Currently, there are no data available to guide our choice for this parameter. Of course, if instruments are used only once then this effectively removes all potential for onwards transmission. Such a policy was introduced in Great Britain for tonsillectomies in 2001, but was later stopped in England because of concerns over the safety of the single-use instruments. However, as tonsillectomies form only a very small percentage of the total operations performed each year, such a policy is unlikely to have an impact at the population level. A first step to reducing the current uncertainty in the potential for self-sustaining transmission via surgery would be to survey the frequency with which different instruments are used, particularly those used on high-infectivity procedures. Also, tracking of surgical instruments should be improved, so that, at the very least, instruments are not re-used once the infection status of a patient is known.
The results presented here are based on a number of simplifying assumptions which are made in the absence of data but which may also be important in determining the potential for onward transmission: first, we have assumed that the risk of becoming infected during a surgical procedure depends only on the overall prevalence of infection on surgical instruments. Cohorting of instruments clearly occurs (e.g. an instrument used for eye surgery will subsequently likely be used again for eye surgery). This type of cohorting can increase the risk of a self-sustaining epidemic within the very high-risk procedures but is likely to reduce the overall spread of infection (Anderson & May 1991).
Second, we have not incorporated multiple operations per patient, which again could reduce the overall spread of infection, but increase the risk for patients that frequently undergo surgical procedures.
Third, we have neglected the effect of reduced survival after surgery due to the lack of quantitative data. Survival is however likely to be substantially reduced, particularly for some procedures of the high-risk group such as neurosurgery. Incorporating this would lead to a reduction in transmission.
And finally, we have assumed that no cases so far have been infected via surgery. If there was even one case infected via surgery, this would increase our estimates substantially.
Despite these simplifications, our results clearly demonstrate that surgical procedures provide a potential route for self-sustaining vCJD transmission from human-to-human. The further strong association between surgical procedures and blood transfusion (with approximately half of all transfusions occurring during surgery, Wells et al. (2002)) will increase the probability of such an event. Data on these overlaps as well as some simple measures of surgical instrument use are therefore a high priority so that models can be further refined to guide potential public health interventions.
Acknowledgments
We are grateful to James Ironside and David Hilton for providing data on the survey of lymphoreticular tissues. We also thank Simon Cousens and Peter Smith for helpful comments. This work was supported by the Department of Health. The views expressed in this publication are those of the authors and not necessarily those of the Department of Health.
Appendix A. Survival probability
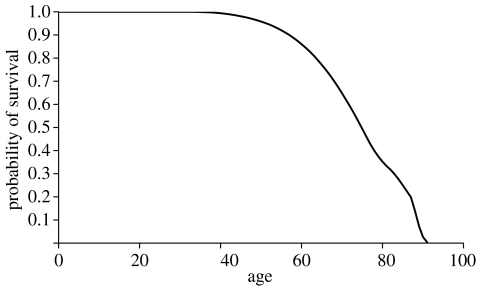
The annual numbers of births Bc and the age-dependent death-rate μa have been estimated from census data (see Ghani et al. (2003); Clarke & Ghani (2005)). A plot of the probability of survival S(a) is shown in figure 7. The conditional survival probabilities used in appendix B are given as S(a|a′)=S(a)/S(a′), whereas the death rate at age a can be obtained as .
Figure 7.
Probability of survival dependent on age.
Appendix B. Calculation of the basic reproduction number R0
It is assumed that any potential vCJD epidemic is much smaller than the population size, and then the method from Diekmann et al. (1990) can be used for calculating R0.
R0 is given as the leading eigenvalue of the next generation matrix
| (A1) |
where Nπhi is the expected number of humans infected by one infected set of surgical instruments, whereas nπih is the expected number of sets of surgical instruments that are infected by one human.
The calculation of Nπhi is fairly easy: once a set is infected, it has on average d operations left before it is cleaned/discarded, and thus the expected number of humans infected by it is
| (A2) |
The expected number of sets that are infected by one human depends on the age a at which the human himself became infected, and thus we have
| (A3) |
where nπia is the expected number of sets that are infected by one human who became infected at age a. This is given by the infectivity Π(t;i, a) of humans (infected at age a) towards instruments, at time t after infection as
| (A4) |
We have
| (A5) |
where
| (A6) |
is the rate of infection of sets of instruments by one infected person infected at age a, time t after infection. is the cumulative distribution function of the non-infectious period after infection, which is a Γ-distribution, and it ensures that individuals can only transmit disease after having passed the non-infectious period.
P(t;a) is the survival probability of an infected person from age a to a+t, given as
where u=⌊t⌋. Here, is the cumulative distribution function of the incubation period distribution, and it determines the additional risk of dying from disease for the pre-clinically infected.
Thus,
| (A7) |
| (A8) |
| (A9) |
Integration by parts gives
| (A10) |
with m(u, a), kr(u, a) and lr(u, a) from equations (4.14), (4.15) and (4.16), respectively. For R0 we have
| (A11) |
This yields the basic reproduction number given in equation (4.13).
References
- Anderson R.M, May R.M. Oxford University Press; Oxford, UK: 1991. Infectious diseases of humans. [Google Scholar]
- Bishop M.T, et al. Predicting susceptibility and incubation time of human-to-human transmission of vCJD. Lancet Neurol. 2006;5:393–398. doi: 10.1016/S1474-4422(06)70413-6. doi:10.1016/S1474-4422(06)70413-6 [DOI] [PubMed] [Google Scholar]
- Bruce M.E, et al. Transmissions to mice indicate that ‘new variant’ CJD is caused by the BSE agent. Nature. 1997;389:498–501. doi: 10.1038/39057. doi:10.1038/39057 [DOI] [PubMed] [Google Scholar]
- Clarke P, Ghani A.C. Projections of the future course of the primary vCJD epidemic in the UK: inclusion of subclinical infection and the possibility of wider genetic susceptibility. J. R. Soc. Interface. 2005;2:9–31. doi: 10.1098/rsif.2004.0017. doi:10.1098/rsif.2004.0017 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Clarke, P., Will, R. G. & Ghani, A. C. Submitted. Is there the potential for a self-sustaining epidemic of variant creutzfeldt-jakob disease via blood transfusion in the UK? [DOI] [PMC free article] [PubMed]
- Diekmann O, Heesterbeek J.A.P, Metz J.A.J. On the definition and the computation of the basic reproduction ratio R0 in models for infectious diseases in heterogeneous populations. J. Math. Biol. 1990;28:365–382. doi: 10.1007/BF00178324. doi:10.1007/BF00178324 [DOI] [PubMed] [Google Scholar]
- Donnelly C.A, Ferguson N.M, Ghani A.C, Anderson R.M. Implications of BSE infection screening data for the scale of the British BSE epidemic and current European infection levels. Proc. R. Soc. B. 2002;269:2179–2190. doi: 10.1098/rspb.2002.2156. doi:10.1098/rspb.2002.2156 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Economics and Operational Research Division 2001 Risk assessment for transmission of vCJD via surgical instruments: a modelling approach and numerical scenarios. Technical report, Department of Health.
- Flechsig E, Hegyi I, Enari M, Schwarz P, Collinge J, Weissmann C. Transmission of scrapie by steel-surface-bound prions. Mol. Med. 2001;7:679–684. [PMC free article] [PubMed] [Google Scholar]
- Ghani A.C, Donnelly C.A, Ferguson N.M, Anderson R.M. Updated projections of future vCJD deaths in the UK. BMC Infect. Dis. 2003:30. doi: 10.1186/1471-2334-3-4. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Hill A.F, Collinge J. Subclinical prion infection. Trends Microbiol. 2003;11:578–584. doi: 10.1016/j.tim.2003.10.007. doi:10.1016/j.tim.2003.10.007 [DOI] [PubMed] [Google Scholar]
- Hill A.F, Desbruslais M, Joiner S, Sidle K.C.L, Gowland I, Collinge J, Doey L.J, Lantos P. The same prion strain causes vCJD and BSE. Nature. 1997;389:448–450. doi: 10.1038/38925. doi:10.1038/38925 [DOI] [PubMed] [Google Scholar]
- Hill A.F, Joiner S, Linehan J, Desbruslais M, Lantos P.L, Collinge J. Species-barrier-independent prion replication in apparently resistant species. Proc. Natl Acad. Sci. USA. 2000;97:10 248–10 253. doi: 10.1073/pnas.97.18.10248. doi:10.1073/pnas.97.18.10248 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Hilton D.A, Fathers E, Edwards P, Ironside J.W, Zajicek J. Prion immunoreactivity in appendix before clinical onset of variant Creutzfeldt–Jakob disease. The Lancet. 1998;352:703–704. doi: 10.1016/S0140-6736(98)24035-9. doi:10.1016/S0140-6736(98)24035-9 [DOI] [PubMed] [Google Scholar]
- Hilton D.A, Ghani A.C, Conyers L, Edwards P, McCardle L, Ritchie D, Penney M, Hegazy D, Ironside J.W. Prevalence of lymphoreticular prion protein accumulation in UK tissue samples. J. Pathol. 2004;203:733–739. doi: 10.1002/path.1580. doi:10.1002/path.1580 [DOI] [PubMed] [Google Scholar]
- HSMO 1990 Tabular list of the classification of surgical operations and procedures, vol. 1. TSO.
- Jackson G.S, et al. An enzyme-detergent method for effective prion decontamination of surgical steel. J. Gen. Virol. 2005;86:869–878. doi: 10.1099/vir.0.80484-0. doi:10.1099/vir.0.80484-0 [DOI] [PubMed] [Google Scholar]
- NHS Estates 2000 Decontamination review: report on a survey of current decontamination practices in healthcare premises in England. Technical report, Department of Health.
- NHS Estates 2001 A review of the decontamination of surgical instruments in the NHS in England. Technical report, Department of Health.
- Peden A.H, Head M.W, Ritchie D.L, Bell J.E, Ironside J.W. Preclinical vCJD after blood transfusion in a PRNP codon 129 heterozygous patient. Lancet. 2004;364:527–529. doi: 10.1016/S0140-6736(04)16811-6. doi:10.1016/S0140-6736(04)16811-6 [DOI] [PubMed] [Google Scholar]
- Scott M.R, Will R, Ironside J, Nguyen H.-O.B, Tremblay P, DeArmond S.J, Prusiner S.B. Compelling transgenetic evidence for transmission of bovine spongiform encephalopathy prions to humans. Proc. Natl Acad. Sci. USA. 1999;96:15 137–15 142. doi: 10.1073/pnas.96.26.15137. doi:10.1073/pnas.96.26.15137 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Wadsworth J.D, Joiner S, Hill A.F, Campbell T.A, Desbruslais M, Luthert P.J, Collinge J. Tissue distribution of protease resistant prion protein in variant Creutzfeldt–Jakob disease using a highly sensitive immunoblotting assay. Lancet. 2001;358:171–180. doi: 10.1016/s0140-6736(01)05403-4. doi:10.1016/S0140-6736(01)05403-4 [DOI] [PubMed] [Google Scholar]
- Ward H.J.T, et al. Risk factors for variant Creutzfeldt–Jakob disease: a case-control study. Ann. Neurol. 2006;59:111–120. doi: 10.1002/ana.20708. doi:10.1002/ana.20708 [DOI] [PubMed] [Google Scholar]
- Wells A.W, Mounter P.J, Chapman C.E, Stainsby D, Wallis J.P. Where does blood go? Prospective observational study of red cell transfusion in north England. Br. Med. J. 2002;325:803. doi: 10.1136/bmj.325.7368.803. [DOI] [PMC free article] [PubMed] [Google Scholar]
- WHO 2003 WHO guidelines on transmissible spongiform encephalopathies in relation to biological and pharmaceutical products. Technical report, WHO, Geneva.
- Yan Z, Stitz L, Heeg P, Pfaff E, Roth K. Infectivity of prion protein bound to stainless steel wires: a model for testing decontamination procedures for transmissible spongiform encephalopathies. Infect. Control Hosp. Epidemiol. 2004;25:280–283. doi: 10.1086/502392. doi:10.1086/502392 [DOI] [PubMed] [Google Scholar]