Abstract
When blindfolded subjects match the position of their forearms in the vertical plane they rely on signals coming from the periphery as well as from the central motor command. The command signal provides a positional cue from the accompanying effort sensation required to hold the arm against gravity. Here we have asked, does a centrally generated effort signal contribute to position sense in the horizontal plane, where gravity cannot play a role? Blindfolded subjects were required to match forearm position for the unloaded arm and when flexors or extensors were bearing 10%, 25% or 40% of maximum loads. Before each match the reference arm was conditioned by contracting elbow muscles while the arm was held flexed or extended. For the unloaded arm conditioning led to a consistent pattern of errors which was attributed to signals from flexor and extensor muscle spindles. When elbow muscles were loaded the errors from conditioning converged, presumably because the spindles had become coactivated through the fusimotor system during the load-bearing contraction. However, this convergence was seen only when subjects supported a static load. When they moved the load differences in errors from conditioning persisted. Muscle vibration during load bearing or moving a load did not alter the distribution of errors. It is concluded that for position sense of an unloaded arm in the horizontal plane the brain relies on signals from muscle spindles. When the arm is loaded, an additional signal of central origin contributes, but only if the load is moved.
At any particular moment we know quite precisely where our limbs are, even if we are not looking at them. The present-day view is that signals arising in limb muscles are primarily responsible for our limb position sense. That has not always been so. During the first half of the nineteenth century the prevailing view was that the act of willing the limbs to move provided us with information about their location (Muller, 1837; Von Helmholtz, 1867).
The modern view has its roots in the influential writings of Sherrington (1900) who stated that, ‘the perceived results of volition are the outward ends obtained, and not the inward action of neuromuscular machinery’. The experimental basis for that view was provided by the observations of Goodwin et al. (1972) who demonstrated that muscle vibration, a stimulus known to excite the afferents of muscle spindles, led subjects to report illusions of movement and displacement of their vibrated limb. So the majority view, at present, is that muscle spindles provide us with our kinaesthetic sense, the sense of position and movement of the limbs.
However, there were persistent reports in the literature which suggested that things were not as simple as that. It was pointed out by Lackner & DiZio (2000) that we move about in a gravitational field, often unaware of the forces acting. Any change in the gravitational field can lead to postural and proprioceptive illusions. In support of these ideas are reports of kinaesthetic disturbances in high-gravity (Lackner & Graybiel, 1981) and low-gravity environments (Young et al. 1993).
More recently it has also been reported that subjects make errors in matching the position of their forearms after exercise of one arm (Saxton et al. 1995; Brockett et al. 1997). Errors were correlated with the size of the exercise-induced fall in force, except when the limb was supported (Walsh et al. 2004; Allen & Proske, 2006). It was concluded that in a task involving unsupported forearm position matching, a source of positional information came from the amount of effort required to support the limb.
In another recent report, when the forearms were placed on supports, so that a sense of effort could not play a role, it was shown that position sense in the vertical plane was largely provided by muscle spindles. When the arm was not supported, or held unsupported with extra weights added, positional information seemed to be coming from both spindles and the sense of effort (Winter et al. 2005). The findings implied that when we maintain the position of our limb against the force of gravity, we are accessing information from two sources, one peripheral and the other central in origin.
In support of such a view, we have recently shown that after a period of eccentric exercise of one arm, which significantly reduced maximum voluntary contraction (MVC) force in elbow flexors, there was an increase in position errors in a matching task in the vertical plane, confirming our earlier work. More importantly for the present study, the fall in force was not accompanied by significant matching errors in a position matching task in the horizontal plane, where little or no effort was required to maintain the test position (Walsh et al. 2006). The results suggested that altering the effort : force relationship by fatiguing muscles had no effect on position sense in the horizontal plane. It left open the question of matching accuracy when the arm was bearing a load.
In the earlier experiments using position matching in the vertical plane, we had introduced a method of conditioning muscles that led to systematic changes in limb position errors (Gregory et al. 1988; Winter et al. 2005). We submitted these observations in support of muscles spindles providing a position signal in the relaxed arm.
In this new series we wanted to determine whether muscle spindles also provided the positional signal for passive movements in the horizontal plane and what were the changes, if any, in position sense when the arm was loaded. The central hypothesis was that matching forearm position in the horizontal plane involved signals of both peripheral and central origin, but that a centrally generated effort signal, in its simplest form, would not be able to provide positional information.
Methods
A total of 34 subjects (16 males, 18 females) participated in the two experiments (some subjects participated in both experiments), 11 in the static load experiment (including 40% load), 28 in the moving load experiment (including 40% Load). Of the 34 subjects, 17 participated in a supplementary series testing the effects of muscle vibration during static loading or moving a load. Subjects gave their informed, written consent prior to undertaking the experiments, which were all approved by the Monash University Committee for Human Experimentation, and the ethical aspects of the experiments conformed with the Declaration of Helsinki.
Each subject attended several test sessions involving forearm matching trials. A series of control trials was carried out to help familiarize subjects with the equipment and procedures. In the control trials, subjects were asked to participate further only if they achieved acceptable levels of reliability in their matching performance, which was set as a standard deviation of matching errors of less than 5 deg.
Testing apparatus
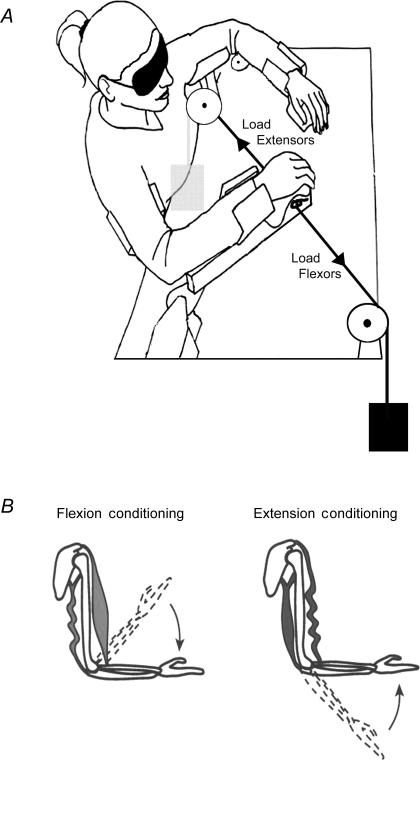
The task involved matching the positions of the forearms in the horizontal plane using a custom built device. The subject was blindfolded and sat at a table, with each forearm and upper arm supported by a cradle, hinged at a point coaxial with the elbow joint (Fig. 1). The arms were moved across a surface subdivided into angular positions in degrees, the locations of the arms being indicated by a pointer mounted below each hand. It allowed the experimenter to determine position of the cradle supporting the arm with a resolution of 0.5 deg. The use of bearings at both elbow pivots meant that horizontal movement was almost frictionless and required little or no effort to maintain a given elbow angle. The angles were recorded as the included angle between the forearm and the upper arm. When the forearm was extended at 90 deg to the trunk, this corresponded to 130 deg. Stops were attached to the apparatus so that the subject could move no further into extension. They could move into flexion to an angle of 50 deg, giving a total movement range of 80 deg. Position errors were calculated by taking the difference between reference angle and matching angle. Thus, a positive error meant that the matching arm had adopted a more extended position than the reference arm.
Figure 1. Experimental set-up and muscle conditioning.
A, experimental set-up. Subjects sat with their forearms supported by cradles which were hinged at a point coaxial with the elbow joint. The arms could be moved in the horizontal plane across a surface that was subdivided into angular positions in degrees, the locations of the arms being indicated by a pointer below each hand. The horizontal movement was almost frictionless and in the unloaded condition required no effort to maintain a given elbow angle. To load the arm, a pulley system with a cable was connected to the apparatus, allowing weights to be attached (Load Flexors and Load Extensors). B, muscle conditioning. A simplified diagram of an arm with one elbow flexor and one extensor. Contracting the muscles while holding the arm flexed and then moving it to an intermediate test angle leaves flexors taut and extensors slack. Similarly, contracting the arm while it is extended and then moving it to the test angle leaves extensors taut and flexors slack. The conditioning positions have been indicated by the dashed outlines, the arrows indicating the direction of movement to the test angle.
In these experiments we chose to use test angles in the mid-range, approximately 75–85 deg where passive resistance from extensors and flexors was minimal. For the first series of experiments, the relaxed reference arm was moved to the test position by the experimenter. The precise value of the test angle depended on the accuracy of placement by the experimenter. In practice, angles in the range of 70–90 deg were achieved. In previous experiments of this kind, we had selected three different test angles (Walsh et al. 2004). However, for the kinds of measurements we were making, we found a single test range adequate. In none of the trials was there any evidence of learning during successive matches (see, for example, Fig. 2).
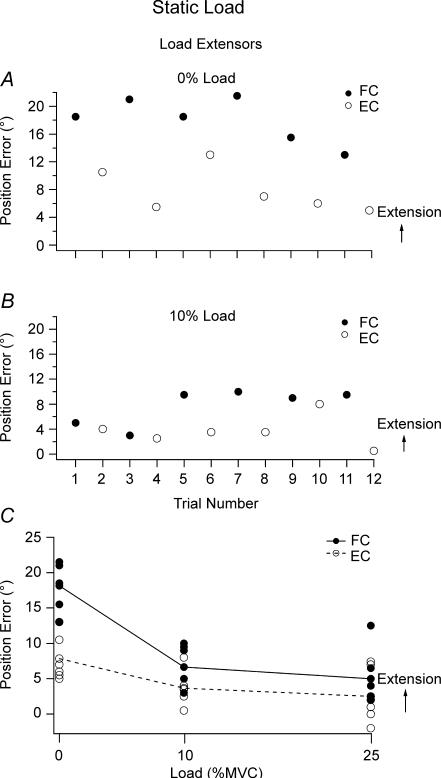
Figure 2. Effect of loading extensors.
Data for single subject. Matching errors for 6 matching trials with the reference arm flexion conditioned (•, FC) or extension conditioned (^, EC). When the matching arm adopted a more extended position this was assigned a positive value, if it was more flexed it was negative. A, no load; B, 10% MVC load on the extensor muscles; C, pooled errors for a single subject following flexion conditioning (•, continuous line, FC) and extension conditioning (^, dashed line, EC) for the relaxed arm (0% load), and extensor loads representing 10% and 25% MVC.
In a second series of experiments the subjects moved their reference arm themselves until they were told to stop. At that point they were asked to match position.
To ensure that subjects complied with the instructions and kept their reference elbow flexors and extensors relaxed during the matching trials, they were provided with audio feedback of electromyographic (EMG) activity recorded from the surface of biceps brachii and triceps brachii muscles. EMG recordings were also made during load bearing to establish agonist : antagonist activation patterns. Recordings were made with Ag–AgCl electrodes with an adhesive base and solid gel contact point (3M Health Care, London, Ontario, Canada). The EMG signals were amplified using a BIO Amp (ADInstruments, Castle Hill, NSW, Australia) acquired with a MacLab 4/s running Chart software (ADInstruments), and displayed on a Macintosh computer as well as being fed through a loud speaker. Recordings showed that when subjects were provided with feedback and instructions, once the reference arm had been conditioned, elbow muscles remained electrically silent during the matching procedure, unless they were supporting a load.
Strength testing
Before each experiment subjects had the maximum strength of their reference arm flexors and extensors measured by means of a spring balance attached to the cradle just below the subject's wrist. With the reference arm placed at 80 deg the subject was asked to either push out or pull in as hard as possible for 3 s while being given verbal encouragement. Maximum voluntary contraction (MVC) force was used to calculate the load on the arm for the subsequent experiments (Fig. 1).
Experiment 1. Position sense while supporting a static load
Eleven subjects undertook this experiment, which was carried out over two separate sessions in which either flexors or extensors were loaded. Subjects carried out three sets of 12 trials. Each trial began with elbows fully extended followed by either flexion or extension conditioning, which was alternated between trials (Winter et al. 2005). For flexion conditioning, the reference arm was moved into flexion by the experimenter to an included elbow angle of 50 deg and the elbow flexors were contracted isometrically. Once the subject had relaxed, the experimenter moved the relaxed arm to the test angle at which point the load was applied (Fig. 1B). In extension conditioning the subjects left their reference arm in its extended position (130 deg included elbow angle) and pushed out against the stop to contract elbow extensors. Again the relaxed arm was then moved to the test angle and the load applied. The three sets of trials differed in the size of the load applied to the reference arm, 0% (no load), 10% or 25% of MVC force. After each set of trials the subject was given a few minutes' rest with the blindfold removed. This was done to allow them to maintain alertness. The order of the three sets of trials was randomised between subjects. Data for two subjects were excluded because they did not meet the required matching consistency (s.d. < 5 deg). This left a total of nine subjects in each group.
Experiment 2. Position sense after moving the loaded arm
This experiment was carried out by 14 subjects. For 11 subjects measurements were made with a load on flexors, for 10 subjects with a load on extensors, with the two sets of measurements performed on separate days. There were seven subjects common to the two parts of the experiment. Each trial began with flexion or extension conditioning, as before. Once the subject had relaxed, following conditioning, the arm was loaded. Subjects were asked to move the loaded arm slowly and as smoothly as possible, in either the direction of extension or flexion, depending on the form of conditioning, until it had reached the test angle. At that point they were told to match positions of the two arms. As in the previous series, loads of 0% (no load), 10% and 25% of MVC loads were used. The data from one subject was excluded from the experiment involving loading of flexors (s.d. > 5 deg). This left 10 subjects for analysis in both parts of the experiment.
Experiment 3. Muscle vibration
Vibrating the muscle while it is supporting a static load
Eleven subjects took part in this experiment. Here both arms carried out a conditioning isometric contraction of elbow flexors in a flexed position before the reference arm was moved to the test position. This starting position was chosen as it was found to give more accurate matches than a starting position with the arms extended.
In the vibration trials, position matching was carried out while elbow flexors of the reference arm were vibrated at 80 Hz and approximately 1 mm amplitude. A frequency of 80 Hz was chosen rather than the 100 Hz originally used by Goodwin et al. (1972), because since then it has been shown that 80 Hz is the optimal frequency for stimulating primary endings of human spindles (Roll et al. 1989). The vibrator was strapped to the belly of biceps brachii so that it was slightly pressed into the muscle. It consisted of a plastic cylinder, 3 cm diameter, housing a weight mounted eccentrically on the shaft of a small electric motor. Vibration started ∼0.5 s after the reference arm had been positioned by the experimenter at the test angle and it was turned off once the subject had indicated that a match had been achieved.
Following the conditioning contraction, the reference arm was moved to the test angle. Matching was performed under four different conditions: no load (0% MVC), no load with vibration, loaded (25% MVC) and loaded with vibration. The load and vibration were applied after the arm had been placed at the test position, and only when the subject had fully supported the load was the vibrator turned on. Subjects were instructed not to resist any perceived movement of their arm during vibration, but to simply indicate the position of the vibrated arm with their matching arm. The four different conditions were given in random order, with a 2–3 min rest between each set of 12 trials. Two sets of 12 trials (6 trials per condition) were carried out for each subject.
Vibration during the movement
Eight subjects participated in this supplementary series. Position sense was tested after the loaded elbow flexors (25%MVC) had been vibrated at 80 Hz while subjects moved the arm to the test position. As soon as the test angle had been reached the vibrator was turned off and subjects were asked to match the positions of their forearms, as before. The data from two subjects were excluded (s.d. > 5 deg), leaving six subjects for data analysis.
Statistical analysis
Position matching errors were calculated as angle (reference arm) – angle (indicator arm). A positive error meant that the matching arm had adopted a more extended position. Data were analysed using the software Igor Pro v.4 (Wavemetrics, Lake Oswego, OR, USA) running on a PC. Statistical analysis used SPSS version 12.0.1 (Systat Software Inc., Point Richmond, CA, USA).
Analysis used a two-way ANOVA with repeated measures to test for differences in position errors between the paired conditions of flexion conditioning and extension conditioning of the reference arm for three levels of load (0%, 10%, 25% MVC) and for the different starting positions of the matching arm (extension, flexion). For the vibration experiment a two-way repeated measures ANOVA tested for differences in position errors with vibration for the unloaded and loaded reference arm. Significance was accorded a P-value < 0.05. Values are given as means ± standard error of the mean (s.e.m.).
Results
Experiment 1. Position sense while supporting a static load
Muscle conditioning
We had previously shown in experiments involving forearm position matching in the vertical plane that a conditioning voluntary contraction of elbow muscles produced errors consistent with a position signal arising in muscle spindles of the conditioned muscles. Here we wanted to confirm that a similar pattern of errors could be demonstrated for position matching in the horizontal plane. When the reference arm was unloaded, conditioning in the flexed position led the matching arm to adopt a more extended position than after its conditioning in the extended position (Fig. 2A). When data from the nine subjects was pooled mean errors after flexion conditioning were 9.3 ± 2 deg, after extension conditioning they were 3.8 ± 1.6 deg (Fig. 3A). This difference was significant.
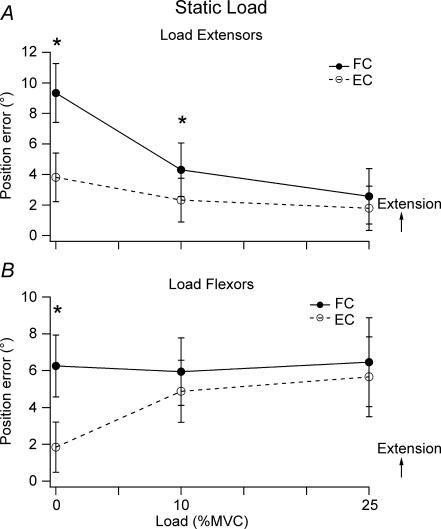
Figure 3. Effect of holding a static load.
A, pooled data for 9 subjects undertaking loading of the extensors showing mean position error (±s.e.m.) for 0%, 10% and 25% loading of the extensor muscles. Flexion conditioning (•, continuous line), extension conditioning (^, dashed line). B, pooled data for 9 subjects undertaking loading of the flexors showing mean position error (±s.e.m.) for 0%, 10% and 25% loading conditions. Flexion conditioning (•, continuous line), extension conditioning (^, dashed line).
Loading extensors
When the reference arm was required to support a load with its extensor muscles, that is, the load was pulling the arm in the direction of flexion, there were two effects. As the reference arm was loaded, the difference in errors from muscle conditioning became less (Fig. 2B and C) and this effect was significant when the data was pooled, using a two-way repeated measures ANOVA F1,8= 27.79, P < 0.01(Fig. 3A). Secondly, matching errors lay more in the direction of flexion, closer to the reference angle (Figs 2B and C and 3A). Effects of the two forms of conditioning were significantly different for the unloaded reference arm (one-way repeated measures ANOVA F1,8= 44.80, P < 0.01) and with 10% load (one-way repeated measures ANOVA F1,8= 11.88, P < 0.01) but not a 25% load.
The errors following conditioning were analysed separately and it was shown that when the reference arm was flexion conditioned and loaded, the matching arm adopted a significantly more flexed position (2.6 ± 1.8 deg) than when not loaded (9.3 ± 1.9 deg, one-way repeated measures ANOVA (F2,7= 10.28, P < 0.01). When the arm was extension conditioned, loading had no significant effect (Fig. 3A).
The observations up to this point support the view that with a 25% MVC load, conditioning elbow muscles no longer produced any significant differences in position errors. Matching performance appeared to be becoming more accurate, with values for mean matching errors, relative to the reference position, being small (2.0 ± 1.5 deg). In an attempt to bring out further emerging trends in the data, for three subjects the load on the flexors was increased to 40% MVC. This did not result in any further changes in matching errors.
Loading flexors
When subjects were asked to support a load pulling the arm in the direction of extension and requiring activity in flexor muscles, the pattern of matching errors was similar to that for loading extensors, although bias of the errors was in the opposite direction (Fig. 3B). The difference in positional errors observed after conditioning in unloaded matching was again significant (one-way repeated measures ANOVA F1,8= 15.50, P < 0.05). This difference was less when the arm was loaded (2-way repeated measures ANOVA, F1,8= 12.729, P < 0.01). The pooled data showed that already by 10% load the effects of the two forms of conditioning were no longer significantly different from each other.
The errors following flexion or extension conditioning were analysed separately and it was shown that loading flexors had no effect on matching errors when the arm had been flexion conditioned (No load 6.3 ± 1.7 deg, load 6.5 ± 2.4 deg). When the arm had been extension conditioned, after loading the errors lay more in the direction of extension (1.8 ± 1.4 deg, no load; 5.7 ± 2.2 deg, load, Fig. 3B). This trend was significant (F2,7= 36.70, P < 0.05).
Experiment 2. Moving the loaded arm
Moving the loaded extensors
When subjects were asked to move their unloaded arm themselves to the target, they were able to do so quite accurately. Movement speed was kept slow, at about 3 deg s−1, and they were told to match position of the two arms as soon as the reference angle had been reached.
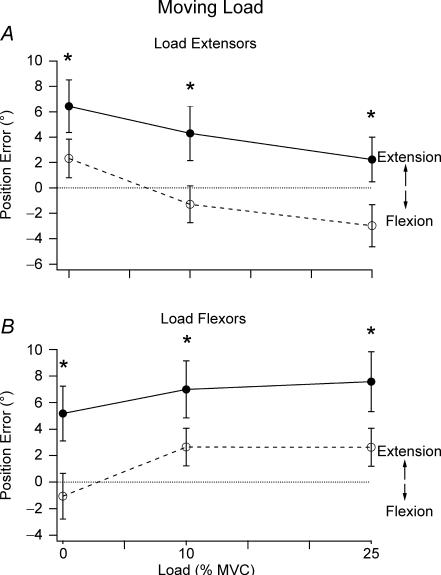
The pattern of position errors when the subject moved the unloaded arm were about the same as when the unloaded arm had been moved by the experimenter. However, the pattern differed when subjects moved their loaded arm themselves (Fig. 4A). The most striking feature was that differences in position errors produced by conditioning of the relaxed arm persisted for the 10% and 25% MVC loads (main effect of condition F1,9= 20.776, P < 0.05 and no interaction between load and condition). That is, where the load was static, conditioning dependent errors converged as the load was increased (Fig. 3). However, when the load was moved, they did not converge (Fig. 4A). In addition, when the load was moved, there was a trend for errors to lie progressively in the direction of flexion as the load was increased (main effect of loading, F2,8= 11.121, P < 0.05).
Figure 4. Effect of moving a loaded arm.
A, pooled data for 10 subjects, showing mean position error (±s.e.m.) for 0%, 10% and 25% loading of the extensor muscles in a matching task where subjects moved the load to the target themselves. Flexion conditioning, •, continuous line; extension conditioning, ^, dashed line. Asterisks indicate significant differences between the two forms of conditioning. Dotted line indicates zero error. B, pooled data for 10 subjects where they moved the load themselves, showing mean position error (±s.e.m.) for 0%, 10% and 25% loading of the flexor muscles. Flexion conditioning, •, continuous line; extension conditioning, ^, dashed line. Asterisks indicate significant differences between the two forms of conditioning. Dotted line indicates zero error.
Moving loaded flexors
When subjects moved their unloaded reference arm to the test position, errors were dependent on the form of conditioning, as before. For this group of subjects the differences in the effects of conditioning in the unloaded condition were a little larger than when the experimenter moved the arm (Fig. 4B). Again, as for loading the extensors, the difference in errors from conditioning did not converge with increasing load when the arm moved the load in the direction of extension, requiring flexor activity (main effect of condition F1,9= 18.351, P < 0.05, and no interaction between load and condition). With increasing load, position errors tended to lie progressively further in the direction of extension (main effect of loading F2,8= 5.026, P < 0.05).
In a supplementary experiment carried out on six subjects, this trend continued up to 40% MVC, but it was not significant.
Experiment 3. Muscle vibration
Vibrating the muscle while it is supporting a static load
Our working hypothesis for the effects of loading the arm was that under load muscle spindles became coactivated through the fusimotor system. It raised the question of whether fusimotor coactivated spindles could still generate illusions of limb position during vibration.
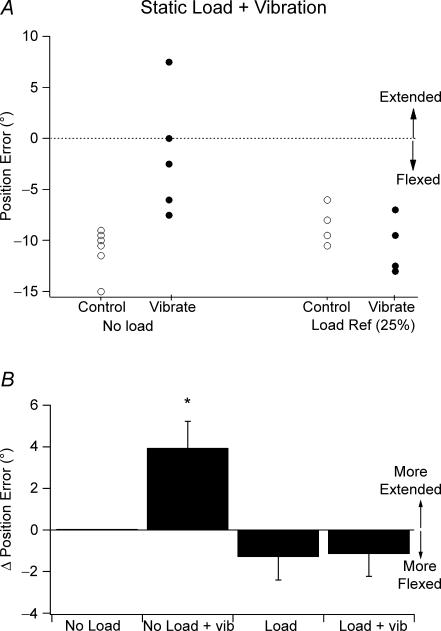
Representative data of position errors during vibration for one subject is shown in Fig. 5A. This particular subject matched the two arms with the indicator arm adopting a position more flexed than the reference (Fig. 5A). When the six trials were repeated, but during vibration of the reference arm, the subject matched with errors in the direction of extension relative to the positions adopted before vibration. The result supported the idea that the vibration-evoked spindle activity made the subject perceive their elbow flexors to be more stretched than was actually the case, leading to errors in the direction of extension. When the experiment was repeated but with the reference flexors supporting a 25% load, the distribution of errors did not change during vibration when compared with control values (Fig. 5A).
Figure 5. Effect of vibration.
A, data for a single subject of the effect of vibration of elbow flexors on position sense when the reference arm was unloaded (left-hand panel) and when the flexors were supporting a load (25% MVC, right-hand panel). Control, ^; vibration, •. Dotted line indicates zero error. B, position errors (means ±s.e.m.) for 11 subjects expressed relative to the unloaded, non-vibrated condition (No Load). Errors were large during vibration of the unloaded muscle (No Load + vib) and small during vibration of the loaded muscle (Load + vib). Asterisk indicates significant difference.
Pooled data from 11 subjects are shown in Fig. 5B. In the figure values are shown relative to the position errors for the unloaded, non-vibrated arm, given as zero. Analysis showed that there was a significant interaction between load and vibration (two-way repeated measures ANOVA, F1,10= 8.78, P < 0.05). Further analysis showed that in the unloaded muscle, errors during vibration were significantly different from errors without (change of 3.9 ± 1.3 deg; 2 × 2 × 6 repeated measures ANOVA, F1,10= 5.292, P < 0.05). By contrast errors during vibration of the loaded muscle were not significantly different from errors without vibration (change of −1.3 ± 1.1 deg (load) versus change of −1.2 ± 1.1 deg (load + vibrate)).
Vibration during the movement
In this experiment position errors were measured during vibration of elbow flexors of the moving arm while they were supporting a 25% MVC load. The result was the same as for supporting a static load. Errors after vibration of biceps during loaded movements from a flexed starting point were no different from errors with the same movement but without vibration.
Discussion
The main purpose of these experiments was to explore the origin of position sense in a passive limb and when limb muscles were contracting in the horizontal plane, that is, under conditions where the role of gravity was minimal.
Experiment 1. Position sense while supporting a static load
The unloaded arm
In our earlier vertical matching experiments, the influence of gravity could be removed by supporting the arm (Winter et al. 2005), or by counterweighting it (Walsh et al. 2006) so that no effort was required to maintain its position. Under these conditions position matching ability was poor with errors of 10 deg or more and the distribution of matching errors was not systematically disturbed by fatigue (Walsh et al. 2004; Walsh et al. 2006). The observations suggested that the remnant position sense present when the arm was supported arose largely from the signals of muscle spindles. Evidence for this was based on the thixotropic properties of the intrafusal fibres of muscle spindles producing systematic changes in position errors (Gregory et al. 1988).
Here we have studied position matching ability in the horizontal plane with a relaxed arm. We predicted that with the arm unloaded, position matching accuracy would resemble that in the vertical plane where the arms were supported. That prediction was fulfilled. Flexion or extension conditioning of the reference arm led to systematic position matching errors (Figs 2–4). It did not appear to matter whether the experimenter placed the arm at the test angle or the subject moved it themselves. The simplest interpretation of these errors is that in a relaxed arm, for matching in the horizontal plane, the kinaesthetic signal is coming predominantly from muscle spindles, perhaps with some contribution from skin (Collins et al. 2005) and joint afferents (Ferrell et al. 1987). However, skin and joint afferents would not be expected to show conditioning-dependent changes in responses.
When the reference arm was contracted in a flexed position and then extended to the test angle, intrafusal fibres of elbow flexor spindles would be expected to be taut and resting discharge levels high (Fig. 1). At the same time the extension movement would shorten elbow extensors, they would fall slack and their spindle signal would be low. This would be indicated by the matching arm adopting a more extended position, representing a long flexor muscle and short extensor muscle (Gregory et al. 1988). Trends in the opposite direction would be expected when the conditioning contraction was with the arm extended (Fig. 1). For a more detailed account of intrafusal thixotropy see Proske et al. (1993).
Loading arm muscles
An immediate effect observed with loading the arm, be it with forces requiring activity in flexors or extensors, was to reduce the matching errors due to muscle conditioning (Figs 2–4). The most likely explanation was that the voluntary activity required to support the load led to fusimotor: skeletomotor coactivation (Vallbo, 1974). Any fusimotor activity will take up the slack in intrafusal fibres and resting discharge levels would be expected to rise towards the condition where no slack was present.
Our working hypothesis was that position sense in the passive muscle is derived from the balance of activity coming from spindles in flexors and extensors (Gilhodes et al. 1986; Ribot-Ciscar & Roll, 1998). First, considering the situation with a load on extensor muscles, this would not be expected to change the state of flexor muscles since these were not involved in supporting the load. In practice, EMG recordings of flexor and extensor activity showed that, on average, for a 25% MVC extensor load, a small amount of flexor activity could be detected (∼20% of the extensor activity). A similarly small amount of cocontraction could be detected with flexor loads. For extensor muscles, in the unloaded condition, only if there was slack present after conditioning (flexion conditioning) would loading them have any effect. As they were loaded, slack would be removed by coactivation and therefore position errors would lie progressively more in the direction of flexion, 6 deg in Fig. 3A. Loading extensors should have little or no effect after extension conditioning as now there was no slack to remove.
For three subjects we increased the load from 25% to 40% MVC. There were no further changes in the distribution of the errors. That is, beyond a certain point (> 10% MVC), conditioning-dependent errors in position sense were gone and increasing the load did not introduce new errors. It led to the important conclusion that the larger perceived effort accompanying support of the heavier loads did not introduce additional errors as it did when matching a loaded arm in the vertical plane (Winter et al. 2005).
Similar arguments can be applied when the flexors were supporting the load, but leading to errors with a mirror-image pattern of distribution from that seen with extensors (Fig. 3B).
Two conclusions can be drawn from the observations on static loads. One, the combination of conditioning elbow muscles and loading them provides a pattern of position errors which supports the view that muscle spindles are producing a position signal and that the central nervous system is always listening to the signals coming from the antagonist pair to determine arm position. Secondly, when the signal from one muscle group is dominating, for example from elbow flexors during flexion conditioning, loading the flexors produces little change in position errors (Fig. 3B, filled symbols). That is, in a muscle in which the spindle signal is large, as a result of conditioning, contracting the muscle, which would be expected to coactivate fusimotor neurons, does not appear to lead to any additional errors. Errors change with load only when the spindle signal is low before loading.
How then does the brain know where the arm is when it is supporting the heavier loads? If loading increased the extent of spindle coactivation, this would lead to a large increase in spindle firing rate as a result of the fusimotor activity. Therefore, if the raw spindle signal was used to indicate position, position errors under load should have continued to increase, which they did not. Perhaps the spindle signal undergoes some kind of central processing, where the reafference (spindle signal from fusimotor coactivation) is subtracted from the total spindle signal to derive the exafferent component, that is, the length-related component of the signal (Von Holst & Mittelstaedt, 1950; McCloskey et al. 1983). If so, some indication of such processing might have been expected, a change in the distribution or regularity of the errors, reflecting the additional processing involved. No such signs were apparent.
Experiment 2. Position sense after moving the loaded arm
Since our earlier experiments on position sense in the vertical plane had suggested the operation of an effort signal during load bearing (Winter et al. 2005), we sought evidence for a similar effect in the horizontal plane. Since it was not obvious how an effort signal, on its own, could provide positional information in the horizontal plane, we considered that such a signal might be obtained from moving a load.
When subjects moved the loaded arm to the target position, differences in errors from conditioning no longer converged. When it was a load on the extensors (Fig. 4A) errors lay in the direction of flexion, and when the load was on the flexors (Fig. 4B) errors lay in the direction of extension. Our current working hypothesis is that under load additional position errors are generated by a centrally derived effort signal. This signal is not present with static loads and only manifests itself when the loaded arm is moved. In other words, for position matching in the horizontal plane under load, the brain determines arm position not from the effort required to support the load but from the effort to move the load from one position to another. Such a signal would act in concert with any available spindle signal.
In addition, in the experiments where the load was moved to the target, vibration during the movement did not introduce new errors. It suggests that if there was a peripheral signal contributing to the errors from moving the load, this signal was not disturbed by vibration.
Is there any evidence of a signal of central origin, concerned with position sense?
Recently, Gandevia et al. (2006) reported in an experiment on position sense in the horizontal plane at the wrist that when all afferent and efferent nerves to the forearm were blocked, a large effort signal was unmasked, giving subjects the impression that when they willed their paralysed hand to move it did appear to move by 20 deg or more. The observations indicated that a large position signal is available, derived from the motor command itself, which has both magnitude and direction. We propose that such a signal can contribute to forearm position sense in the horizontal plane when the arm is bearing a load but only if the load is moved by the subject.
There is another, rather different interpretation of the movement data. It has recently been shown that when a torque motor applies a force to the tip of a finger, an attempt by the subject to reproduce the level of force using the finger of their other hand leads to a significant overestimation (Shergill et al. 2003). It was proposed that attenuation of the perceived self-generated force was the result of a predictive process in which the sensory consequences of a movement are anticipated. Something similar could be going on in our experiments (see Davidson et al. 2005). When the subject moved the loaded arm to the target position, it is conceivable that there was some attenuation of the sensation accompanying the movement, leading to an overshoot of the target position.
Experiment 3. Muscle vibration
Muscle vibration
In experiments on position matching in the vertical plane the data had suggested that during voluntary contractions of 5–15% of maximum, positional information came from the spindles that had not yet been coactivated and this was supplemented by an additional signal, an effort signal, of central origin (Winter et al. 2005). It was hypothesized that as soon as all spindles were activated through the fusimotor system they were no longer directly involved in signalling positional information. If that was so, then vibrating a muscle which was contracting sufficiently forcefully to coactivate all spindles should not lead to any illusions of position. We therefore measured position matching errors during vibration of the loaded arm.
It was first shown by Goodwin et al. (1972) that vibrating a muscle produces illusions of limb movement accompanied by smaller, but significant positional errors. If fusimotor activated spindles no longer contributed to position sense, vibration of a contracting muscle should not produce any illusions of altered position. That prediction was fulfilled (Fig. 5). Muscle vibration of the loaded muscle at 80 Hz produced no additional errors above those seen with the contracting muscle in the absence of vibration (Fig. 5). A similar result was reported by Goodwin et al. (1972, p. 720) who found that voluntary contractions of 6 kg (∼30% MVC) abolished the vibration illusions. The absence of a vibration illusion with a loaded muscle suggests that position sense is no longer derived from a spindle signal alone, at least not the spindle signal evoked by vibration, and may involve signals of central origin.
Kinaesthetic illusions evoked by vibration of a contracting muscle have been studied before by McCloskey (1973). He reported illusions of changed position produced by 100 Hz vibration, which were greater with load for 10 of the 15 subjects studied. For the other five, illusions were not significantly different or smaller than from vibrating the non-contracting muscle. When vibration frequency was reduced from 100 Hz to 20–48 Hz all 15 subjects showed smaller positional illusions during vibration of the contracting muscle. So it seems that the size of vibration illusions in a contracting muscle is frequency dependent. In any case, in our experiments vibrating a contracting muscle at 80 Hz did not produce significant position illusions in the horizontal plane.
Conclusions
We submit these findings as representing a number of new observations for the subject of proprioception. First, the evidence supports a role for muscle spindles providing a position signal for movements of the unloaded arm in the horizontal plane. These findings therefore fall in line with similar observations made on position sense in the vertical plane (Walsh et al. 2004; Winter et al. 2005). Secondly, loading the arm progressively removes conditioning-dependent position errors, presumably because of spindle coactivation through the fusimotor system. Again, this is consistent with observations in the vertical plane. Thirdly, there is no evidence of a change in the position signal or its variability during the transition from the unloaded condition to one where elbow muscles are supporting the load, provided the spindles are not slack. We therefore hypothesize that unlike for position matching in the vertical plane, any centrally derived signal associated with effort sensations during support of a static load in the horizontal plane does not provide additional positional information.
However the situation changes when the load is moved by the subject. Now the subject moves the matching arm further than expected. The new errors are in the direction of extension when moving loaded flexors and in the direction of flexion when moving loaded extensors. We conclude that when the brain generates a command for movement of load-bearing elbow muscles in the horizontal plane, it uses this information to determine forearm position, in addition to any previously available signal from muscle spindles.
Acknowledgments
This work was done with support from the National Health and Medical Research Council of Australia. We would also like to thank Lee Walsh for his assistance with some of the experiments and helpful comments on the manuscript.
References
- Allen TJ, Proske U. Effect of muscle fatigue on the sense of limb position and movement. Exp Brain Res. 2006;170:30–38. doi: 10.1007/s00221-005-0174-z. [DOI] [PubMed] [Google Scholar]
- Brockett C, Warren N, Gregory JE, Morgan DL, Proske U. A comparison of the effects of concentric versus eccentric exercise on force and position sense at the human elbow joint. Brain Res. 1997;771:251–258. doi: 10.1016/s0006-8993(97)00808-1. [DOI] [PubMed] [Google Scholar]
- Collins DF, Refshauge KM, Todd G, Gandevia SC. Cutaneous receptors contribute to kinesthesia at the index finger, elbow and knee. J Neurophysiol. 2005;94:1699–1706. doi: 10.1152/jn.00191.2005. [DOI] [PubMed] [Google Scholar]
- Davidson PR, Wolpert DM, Scott SH, Flanagan JR. Common encoding of novel dynamic loads applied to the hand and arm. J Neurosci. 2005;25:5425–5429. doi: 10.1523/JNEUROSCI.0429-05.2005. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Ferrell WR, Gandevia SC, McCloskey DI. The role of joint receptors in human kinaesthesia when intramuscular receptors cannot contribute. J Physiol. 1987;386:63–71. doi: 10.1113/jphysiol.1987.sp016522. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Gandevia SC, Smith J, Crawford M, Proske U, Taylor JL. Motor commands contribute to human position sense. J Physiol. 2006;571:703–710. doi: 10.1113/jphysiol.2005.103093. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Gilhodes JC, Roll JP, Tardy-Gervet MF. Perceptual and motor effects of agonist-antagonist muscle vibration in man. Exp Brain Res. 1986;61:395–402. doi: 10.1007/BF00239528. [DOI] [PubMed] [Google Scholar]
- Goodwin GM, McCloskey DI, Matthews PB. The contribution of muscle afferents to kinaesthesia shown by vibration induced illusions of movement and by the effects of paralysing joint afferents. Brain. 1972;95:705–748. doi: 10.1093/brain/95.4.705. [DOI] [PubMed] [Google Scholar]
- Gregory JE, Morgan DL, Proske U. Responses of muscle spindles depend on their history of activation and movement. Prog Brain Res. 1988;74:85–90. doi: 10.1016/s0079-6123(08)63002-2. [DOI] [PubMed] [Google Scholar]
- Lackner JR, DiZio PA. Aspects of body self-calibration. Trends Cogn Sci. 2000;4:279–288. doi: 10.1016/s1364-6613(00)01493-5. [DOI] [PubMed] [Google Scholar]
- Lackner JR, Graybiel A. Illusions of postural, visual, and aircraft motion elicited by deep knee bends in the increased gravitoinertial force phase of parabolic flight. Exp Brain Res. 1981;44:312–316. doi: 10.1007/BF00236568. [DOI] [PubMed] [Google Scholar]
- McCloskey DI. Differences between the senses of movement and position shown by the effects of loading and vibration of muscles in man. Brain Res. 1973;63:119–131. doi: 10.1016/0006-8993(73)90521-0. [DOI] [PubMed] [Google Scholar]
- McCloskey DI, Gandevia S, Potter EK, Colebatch JG. Muscle sense and effort: motor commands and judgements about muscular contractions. In: Desmedt JE, editor. Motor Control Mechanisms in Health and Disease. New York: Raven Press; 1983. pp. 151–167. [PubMed] [Google Scholar]
- Muller J. The sense of feeling. In: Handwerker HO, Brune K, editors. Classical German Contributions to Pain Research. Hassfurt, Germany: Tagblatt-Druckerei KG; 1837. 1987 translated by Dr Biederman-Thorson. [Google Scholar]
- Proske U, Morgan DL, Gregory JE. Thixotropy in skeletal muscle and in muscle spindles: a review. Prog Neurobiol. 1993;41:705–721. doi: 10.1016/0301-0082(93)90032-n. [DOI] [PubMed] [Google Scholar]
- Ribot-Ciscar E, Roll J. Ago-antagonist muscle spindle inputs contribute together to joint movement coding in man. Brain Res. 1998;791:167–176. doi: 10.1016/s0006-8993(98)00092-4. [DOI] [PubMed] [Google Scholar]
- Roll JP, Vedel JP, Ribot E. Alteration of prioprioceptive messages induced by tendon vibration in man: a microneurographic study. Exp Brain Res. 1989;76:213–222. doi: 10.1007/BF00253639. [DOI] [PubMed] [Google Scholar]
- Saxton JM, Clarkson PM, James R, Miles M, Westerfer M, Clark S, Donnelly AE. Neuromuscular dysfunction following eccentric exercise. Med Sci Sports Exerc. 1995;27:1185–1193. [PubMed] [Google Scholar]
- Shergill SS, Bays PM, Frith CD, Wolpert DM. Two eyes for an eye: the neuroscience of force escalation. Science. 2003;301:187. doi: 10.1126/science.1085327. [DOI] [PubMed] [Google Scholar]
- Sherrington CS. The muscular sense. In: Schafer EA, editor. Textbook of Physiology. Edinburgh: Pentland; 1900. pp. 1002–1025. [Google Scholar]
- Vallbo A. Human muscle spindle discharge during isometric voluntary contractions. Amplitude relations between spindle frequency and torque. Acta Physiol Scand. 1974;90:319–336. doi: 10.1111/j.1748-1716.1974.tb05594.x. [DOI] [PubMed] [Google Scholar]
- Von Helmholtz H. Treatise on Physiological Optics. 1925. Optical Society of America, Menasha, WI, USA: trans/ed Southall JPC; 1867. [Google Scholar]
- Von Holst E, Mittelstaedt H. Das Reafferenzprinzip (Wechselwirkungen zwischen Zentralnervensystem und Peripherie) Naturwissenschaften. 1950;37:464–476. [Google Scholar]
- Walsh LD, Allen TJ, Gandevia SC, Proske U. Effect of eccentric exercise on position sense at the human forearm in different postures. J Appl Physiol. 2006;100:1109–1116. doi: 10.1152/japplphysiol.01303.2005. [DOI] [PubMed] [Google Scholar]
- Walsh LD, Hesse CW, Morgan DL, Proske U. Human forearm position sense after fatigue of elbow flexor muscles. J Physiol. 2004;558:705–715. doi: 10.1113/jphysiol.2004.062703. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Winter J, Allen T, Proske U. Muscle spindle signals combine with the sense of effort to indicate limb position. J Physiol. 2005;568:1035–1046. doi: 10.1113/jphysiol.2005.092619. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Young LR, Oman CM, Merfeld C, Watt D, Roy S, DeLuca C, Balkwill D, Christie J, Groleau N, Jackson DK. Spatial orientation and posture during and following weightlessness: human experiments in Spacelab Life Sciences 1. J Vest Res. 1993;3:231–239. [PubMed] [Google Scholar]