Abstract
Alternative male mating strategies within populations are thought to be evolutionarily stable because different behaviors allow each male type to successfully gain access to females. Although alternative male strategies are widespread among animals, quantitative evidence for the success of discrete male strategies is available for only a few systems. We use nuclear microsatellites to estimate the paternity rates of three male lizard strategies previously modeled as a rock-paper-scissors game. Each strategy has strengths that allow it to outcompete one morph, and weaknesses that leave it vulnerable to the strategy of another. Blue-throated males mate-guard their females and avoid cuckoldry by yellow-throated “sneaker” males, but mate-guarding is ineffective against aggressive orange-throated neighbors. The ultradominant orange-throated males are highly polygynous and maintain large territories; they overpower blue-throated neighbors and cosire offspring with their females, but are often cuckolded by yellow-throated males. Finally, yellow-throated sneaker males sire offspring via secretive copulations and often share paternity of offspring within a female's clutch. Sneaker males sire more offspring posthumously, indicating that sperm competition may be an important component of their strategy.
Alternative reproductive strategies often involve complex behaviors and the evolution of strategy-specific morphology and physiology (1–5). Identifying how these polymorphisms are maintained within populations is crucial to our understanding of the evolution of mating strategies. Although genetically based alternative male strategies are widespread (2–7), evidence that they differ in their relative fitness when in direct competition with one another has been lacking in most species (refs. 5–7, but see ref. 8). Three throat-color and behavioral morphotypes are present in one population of side-blotched lizards (Uta stansburiana): aggressive and territorial orange-throated males, mate-guarding and territorial blue-throated males, and nonterritorial yellow-throated males that mimic females and sneak copulations in territories of the other two male morphs (6). It has been proposed that the evolution of these genetically based morphs is driven by frequency-dependent reproductive success and that alternative strategies coexist in nature because no male type has higher overall fitness in the long term (6, 9).
In a territorial species such as the side-blotched lizard, the success of one morph in competition with others must arise from direct interactions among neighbors. We can predict the fitness outcome of dyadic interactions among neighboring morphs from the behavioral strategies of the rock-paper-scissors game (6): ultradominant orange-throated males should outcompete blue-throated mate-guarders, mate-guarding blue-throated males should outcompete the “sneaker” yellow-throated males, and yellow-throated males should outcompete the aggressive orange-throated males. Testing these predictions, and quantifying the reproductive success of males adopting each tactic, may clarify the mechanisms underlying positive selection for the maintenance of these morphs and allow us to characterize the selection rules that govern evolution of mating system polymorphisms.
Materials and Methods
Estimating Reproductive Success of Males.
Paternity was estimated by using nine microsatellite loci (Table 1) cloned from one individual from the Los Baños Grandes population, Merced County, California. Loci were cloned from genomic DNA by standard cloning methods (10). A genomic library was developed by using size-selected fragments cloned into M13 vector and transformed into E. coli, followed by screening with a radiolabeled oligonucleotide with motif (CA)n. Plaques with inserts containing microsatellites were sequenced for identification of primer sites in the flanking regions.
Table 1.
Forward (F) and reverse (R) primers for amplification of microsatellites in Uta stansburiana
| Primer | Primer sequence (5′ to 3′) | Repeat | Ta, °C | Alleles | Ho |
|---|---|---|---|---|---|
| 10,000 M's-F | AAC-CAT-ACA-GCA-TAG-CAG-TGA-T | (CA)12 | 54 | 11 | 0.30 |
| 10,000 M's-R | AAG-CAT-CAT-GTT-TAC-CAG-ATG-C | ||||
| BRtt-F | CAT-TTC-ATG-CAC-TCT-TGA-TGC-AC | (CA)10 | 51 | 7 | 0.73 |
| BRtt-R | GGG-CAC-AGA-AGA-TGT-TAT-AGA-C | ||||
| IGs-F | GAG-CTA-GGC-AAT-ATG-TAC-TTA-ATG | (CA)14 | 54 | 12 | 0.77 |
| IGs-R | TGT-ACC-ATC-CTG-CAA-CGT-TGT-T | ||||
| MCC-F | TCA-GCT-GTA-ACA-CCC-AGA-AAC | (CA)6A(CA)6 | 53 | 8 | 0.20 |
| MCC-R | TTA-ACT-GCC-AGA-AAA-GGA-CCG | ||||
| NGff-F | GGG-AAT-CAG-GCA-GCA-CAC-AAT | (CA)10 | 58 | 6 | 0.38 |
| NGff-R | TTG-TCA-GCA-AAC-TCC-AGC-GG | ||||
| PLkn-F | GTA-CCT-TGT-GAC-TGC-AGT-GCT | (CA)17 | 58 | 12 | 0.76 |
| PLkn-R | TTG-AGA-CAC-AGG-AGG-CAG-AAG | ||||
| SMcL-F | TAA-TCT-TTG-CAT-ACT-GAG-AT | (CA)15 | 51 | 12 | 0.81 |
| SMcL-R | CCT-AGC-ACA-TCT-CTA-GTA-AG | ||||
| SPhill-F | GAT-CAT-ATA-CTG-GTT-TAA-GAC-A | (CA)9 | 54.5 | 15 | 0.81 |
| SPhill-R | TCA-CAC-ATC-GAC-TCC-AAA-GTC-AG | ||||
| SVeg-F | TGG-TTG-CAA-TGA-GCA-TAT-CAG | (CA)3CG(CA)6 | 51 | 2 | 0.12 |
| SVeg-R | TCA-TTT-CAC-CAT-GCT-AGC-AA |
Also listed are the core repeat (from cloned individual), PCR amplification annealing temperature (Ta), total number of alleles, and observed heterozygosity at each locus.
In this population all males mature and adopt a strategy one year after birth. Mating occurs in three distinct bouts and females normally lay three clutches during the course of the reproductive season. We captured gravid females in the field and harvested and incubated their eggs in the laboratory; thus, maternal identity was known for all hatchlings. All individuals present on the site during the 1992 breeding season (96 females, 131 putative sires, and 458 offspring) were nondestructively sampled by removal of one toe clip per individual. Genomic DNA was extracted from toes by overnight incubation at 55°C in 500 μl 5% Chelex (Bio-Rad) and 2 μl Proteinase K solution (20 mg/ml). Loci were amplified from this template via PCR and length polymorphisms among individuals were assessed with an automated DNA sequencer (Applied Biosystems 377). All individuals were genotyped at all nine loci and these data were used to estimate paternity for every hatchling born during the 1992 season.
For each hatchling, paternity was assigned by comparing likelihood scores among potential fathers with the computer programs KINSHIP V. 1.1.2 (11, 12) and CERVUS (13). In kinship, the male with the highest likelihood is accepted as sire if his likelihood score is significantly different from that expected for a nonrelated male (P < 0.05); in cervus, a male is assigned as sire only if his likelihood score falls within an 80% confidence interval derived from a simulation based on observed population allele frequencies. The kinship analysis yields a higher number of assignments; however, it may inappropriately assign paternity when more than one male meets the significance criterion. This situation will be exacerbated if the adult population includes a large number of siblings and half-siblings. The program cervus is more strict, allowing us to assess our confidence in assignment, but also reducing the number of assigned fathers (and hence sample sizes and statistical power) because some “most likely” sires do not fall within the simulated confidence intervals. We estimated paternity under both assumptions and compared the results. If paternity assignments from both methods are similar, and differ only in the number of males assigned as sires, then this corroborates our results overall.
We also estimated the mean relatedness, R, among hatchlings within each female's clutch (14). Relatedness within clutches gives us an indication of the degree of multiple paternity that can be compared with the results from the sire assignments. By using the simulation function of kinship, we estimated the expected frequency distribution of relatedness coefficients for 10,000 pairs of full siblings (r = 0.5) and half siblings (r = 0.25), given the allele frequencies in our population (14). We then compared the observed levels of relatedness within clutches to these simulated distributions. If multiple paternity exists in this population, the observed mean levels of relatedness should fall between the means for the simulated distributions.
Paternity tests were designed to take into account patterns of male movement. Males were only considered as possible sires if their territories fell within the boundaries of male seasonal movement at this site. Because of the expectation that males in the immediate vicinity of a female's territory were more likely to be the sire of her offspring, we subdivided the entire study site into 11 “lizard neighborhoods”—semiisolated rock outcroppings separated by unsuitable habitat. We searched for sires first within the female's immediate neighborhood and assigned the male with the highest significant likelihood value under the criteria of each program. We then performed a paternity analysis including all males in the female's neighborhood and all adjacent neighborhoods (an “extended neighborhood”). Males from this larger area were assigned as fathers only if their likelihood values were higher than those for males in the female's immediate neighborhood. This hierarchical approach to assigning paternity insures that the occasional male traversing neighborhood boundaries will be considered as a potential father. The “extended neighborhood” is biologically relevant as it encompasses the largest area within which males have been observed moving across the outcrop during the course of the season (15), but male territories are typically stable within extended neighborhoods.
The total reproductive success of each male was estimated as the number of offspring sired during the entire reproductive season. We quantified the average reproductive contribution of males of each morph, and the rates with which males share paternity within a female's clutch with males of other morphs. Heritability was estimated by comparing breeding coloration of sons at maturity with that of their male parents by using linear regression. Narrow-sense heritability estimates assume throat color is a quantitative character where we score yellow as 1, blue as 2, and orange as 3.
Territorial Behavior of Males.
Territory sizes for every male were estimated in the field during the first bout of the breeding season. Multiple passes were made over the field site for visual determination of male lizard locations. All observations of exact locations were mapped and used in reconstruction of territory size. Territories were estimated as the area of the minimum convex polygon that circumscribes all observed locations for each male (16).
Nearest Neighbor and Shared Paternity Analyses.
To estimate spatial associations among morphs, the centroids of all male territories on the field site were converted to Gabriel-connected maps (17) and the morphs of all connected neighbors were determined by using the computer program macturf (18). Observed frequencies of pairwise morph territorial associations (O vs. O, B vs. O, O vs. Y, etc.) were tested against a random expectation that was generated by permuting the morphotype of males in territories throughout the field site. The total numbers of males of each morph in the population remained constant in the permutations.
We also estimated the observed rates of shared paternity between pairs of morphs from the kinship analysis and compared these to values derived from randomizations of the paternity data. Expected values for shared paternity were generated by randomizing the morphs of sires within a female's clutch. The overall frequency of paternity for each male morph in the population (i.e., overall morph fitness) was maintained constant in the permutations. In both randomization tests significance values for each pairwise comparison were derived from 1,000 permutations, with cells deviating significantly from random if observed values fell within the 5% tails of the randomized distributions.
Results
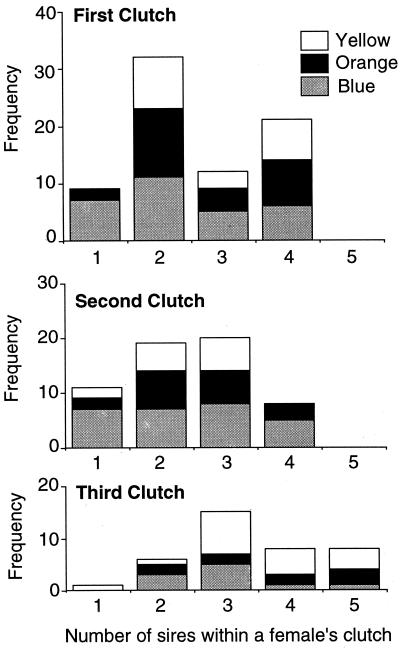
We genotyped all adults alive during the 1992 breeding season and successfully assigned paternity to 71% of their progeny by using kinship, and 48% by using cervus (Table 2). Regardless of the paternity method used, we observed very high multiple paternity rates in this population of lizards: 83% (kinship) or 62% (cervus) of clutches produced during the entire reproductive season were sired by more than one male. Differences in rates of multiple paternity between the two analyses are mostly due to the smaller number of sires assigned per clutch by cervus (especially in the second and third reproductive bouts; Table 2). If we look just at clutches from the first reproductive bout (for which we have the highest sample sizes), 73% (kinship) or 68% (cervus) of clutches included progeny from multiple fathers (Fig. 1). These are high rates of multiple paternity compared with those reported for other amniotes (19–21). As predicted, the number of assigned fathers is lower using the stricter assignment criteria of cervus; however, the patterns obtained from the two methodologies are similar (Table 2).
Table 2.
Paternity assignment results for hatchlings using kinship and cervus
| Program | No. hatchlings assigned sires | No. of clutches | No. progeny/clutch | No. hatchlings in multiply-sired clutches | No. of multiply-sired clutches | No. progeny/multiply-sired clutch |
|---|---|---|---|---|---|---|
| kinship | ||||||
| First bout | 152 | 57 | 2.67 ± 0.20 | 139 | 44 | 3.16 ± 0.20 |
| Second bout | 103 | 44 | 2.34 ± 0.20 | 88 | 29 | 3.03 ± 0.21 |
| Third bout | 72 | 22 | 3.27 ± 0.27 | 66 | 16 | 4.12 ± 0.3 |
| cervus | ||||||
| First bout | 118 | 50 | 2.36 ± 0.18 | 105 | 37 | 2.84 ± 0.19 |
| Second bout | 67 | 31 | 2.09 ± 0.21 | 55 | 20 | 2.75 ± 0.23 |
| Third bout | 36 | 16 | 2.33 ± 0.38 | 29 | 9 | 3.22 ± 0.46 |
For each reproductive bout we estimated the number of hatchlings assigned sires, the number of clutches, and the mean (± SE) number of progeny/clutch that were assigned sires. We estimated the same statistics for clutches that were sired multiply (by more than one male).
Figure 1.
Frequency of singly- and multiply-sired clutches and the contribution of the three alternative male mating strategies to those clutches during three reproductive bouts of the 1992 breeding season (data from KINSHIP analysis). The height of each bar reflects the relative frequency of orange-, blue-, and yellow-throated males that sired progeny in singly- or multiply-sired clutches.
The degree of relatedness within clutches of individual females supports our finding of multiple paternity. Simulated full sibling and half sibling distributions are normal with mean relatedness of Rfull = 0.495 (SD = 0.196) and Rhalf = 0.246 (SD = 0.201), respectively. Mean relatedness within our genotyped clutches is Robs = 0.382 (SD = 0.222), indicating a mixture of full siblings and half siblings in clutches in this population.
Recovery of mature male offspring of known parentage in 1993 was relatively low because of high prematurational mortality (22); nonetheless, we recaptured some hatchlings as adults the following year. A significant sire–offspring regression (P = 0.02, n = 11) for our throat-color scores confirms that this color polymorphism and their correlated behaviors have a genetic basis (narrow-sense heritability, h2n = 0.87; ref. 6).
In 1992, we observed no significant difference in total number of offspring produced by each male type (kinship: B = 2.49 ± 0.45, n = 51; O = 2.82 ± 0.90, n = 29; Y = 2.63 ± 0.47, n = 46; P = 0.92; cervus: B = 0.86 ± 0.19, n = 51; O = 1.26 ± 0.28, n = 29; Y = 1.11 ± 0.24, n = 46; P = 0.51). Likewise, the average number of sired offspring that survive to the following reproductive season (1993) does not vary significantly by morph (kinship: B = 0.36 ± 0.09, n = 51; O = 0.42 ± 0.14, n = 29; Y = 0.44 ± 0.13, n = 46; P > 0.80; cervus: B = 0.22 ± 0.06, n = 51; O = 0.40 ± 0.14, n = 29; Y = 0.27 ± 0.10, n = 46; P > 0.50).
The territorial behavior and spatial distribution of males describes the ecological opportunity for male–male competition among morphs. Orange-throated males have significantly larger territories than blue- and yellow-throated morphs (mean territory size ± SE: O = 39.5 ± 8.8 m2, n = 29; B = 22.8 ± 4.7 m2, n = 50; Y = 20.5 ± 4.8 m (2,) n = 46; Fisher's Least Significant Difference (LSD) posthoc comparisons: O vs. Y, P = 0.03; O vs. B, P = 0.05; B vs. Y, P > 0.75) and this presumably affords them access to a larger number of females. The spatial associations among morphs during the first bout of the reproductive season deviate significantly from random in two cases (Table 3): blue-throated males are less likely to be neighbored by yellow-throated sneakers and yellow-throated males are more likely to be neighbors with other yellow-throated males.
Table 3.
Spatial associations and shared paternity associations among males of different mating strategies during the first reproductive bout of the season
| O vs. O | O vs. B | O vs. Y | B vs. B | B vs. Y | Y vs. Y | |
|---|---|---|---|---|---|---|
| Spatial associations | ||||||
| Observed | 15 | 31 | 36 | 35 | 45 | 32 |
| Expected | 11.7 | 38.2 | 35.1 | 29.5 | 55.1 | 24.6 |
| P-value | >0.05 | >0.05 | >0.05 | >0.05 | 0.044* | 0.021* |
| Shared paternity associations | ||||||
| Observed | 7 | 20 | 24 | 9 | 12 | 16 |
| Expected | 9.6 | 19.5 | 18.0 | 7.1 | 20.8 | 12.9 |
| P-value | >0.05 | >0.05 | 0.046* | >0.05 | 0.014* | >0.05 |
In both tests expected values are estimated from 1,000 randomizations of the data; significant deviations from random (P < 0.05) are indicated by an asterisk. Shared paternity associations are estimated from the kinship paternity data.
Our paternity data reveal the fitness outcome of male–male competition among neighbors. Blue-throated males defend smaller territories and assiduously mate-guard their females. We would predict that this behavioral strategy results in a high proportion of singly-sired clutches. By using the paternity results from kinship, we find that blue-throated males fathered 66% of all singly-sired clutches. In the first reproductive bout alone, 78% of singly-sired clutches were fathered by a blue-throated male (Fig. 1). Blue-throated males are significantly more likely to exclusively sire all offspring in a clutch (overall χ2 = 8.61, df = 1, P < 0.001; pairwise comparisons: B vs. O, χ2 = 5.50, df = 1, P < 0.01; B vs. Y, χ2 = 6.95, df = 1, P < 0.01; O vs. Y, χ2 = 0.40, P > 0.05). The paternity data from cervus result in sample sizes that are not sufficiently large for pairwise comparisons or analyses of reproductive bouts separately; however, the pattern over the entire breeding season is complementary. The contribution of males of the three morphs to singly- and multiply-sired clutches varies significantly over the whole reproductive season (overall χ2 = 7.54, df = 1, P < 0.01); in this case, yellow-throated males are significantly more likely than the other morphs to sire offspring in multiply-sired clutches (93% of yellow-sired offspring belong to multiply-sired clutches, compared with 52% and 57% for blue- and orange-throated males, respectively).
The analyses above compare the relative contribution of each morph to singly- and multiply-sired clutches; a second way to assess degree of multiple paternity is to count the number of males with which each sire shares paternity within a female's clutch. We would predict that yellow-throated males have a higher number of cosires than males of the other two morphs. In fact, yellow-throated males share paternity of a female's clutch with other males significantly more often than other morphs—this pattern is apparent in both paternity datasets, although this result is not statistically significant in the cervus analysis (ANOVA of mean shared paternity among morphs: F2,168 = 3.586, P = 0.03, mean ± SE: B = 1.470 ± 0.132, O = 1.811 ± 0.148, Y = 1.981 ± 0.144 for kinship, first reproductive bout; F1,109 = 1.99, P = 0.14, mean ± SE: B = 2.16 ± 0.15, O = 2.14 ± 0.15, Y = 2.58 ± 0.18 for cervus, all three reproductive bouts combined).
Pairwise comparisons of cosiring by the three male morphs in the first reproductive bout using our larger paternity dataset (kinship) indicates that the observed incidence of shared paternity among morphs deviates significantly from random in two cases: shared paternity within a female's clutch occurs at a higher rate between orange- and yellow-throated males and at a lower rate between blue- and yellow-throated males (Table 3).
Estimates of population-wide shared paternity rates among morphs can be difficult to interpret because they do not take into account the spatial associations of the morphs that may influence a male's opportunity for shared paternity. Thus, we combined our data on spatial arrangement of territories and degree of shared paternity among morphs to analyze the advantage of each morph when the other two morphs are in close proximity (Table 4). We use the kinship paternity data from the first reproductive bout of the season, and the cervus paternity data from all reproductive bouts combined, to compare the frequency with which morphs share paternity within a female's clutch (i.e., cosire with another morph) relative to the frequency with which the same morphs share space (i.e., nearest-neighbor associations; Table 4). Blue-throated males share paternity with yellow-throated males at rates lower than expected from the association of their territories. In contrast, blue-throated males share paternity with orange-throated males at rates higher than would be expected from the association of their territories (Table 4, kinship: χ2 = 4.26, df = 1, P = 0.03; cervus: χ2 = 4.43, df = 1, P = 0.03). Orange-throated males share paternity with the other two morphs at rates not significantly different from expected given their territorial associations (Table 4, kinship: χ2 = 0.01, df = 1, P = 0.93; cervus: χ2 = 1.67, df = 1, P = 0.19).
Table 4.
Relationship between shared paternity and territorial associations for dyadic interactions between morphs in the rock-paper-scissors system
|
kinship
|
cervus
|
|||
|---|---|---|---|---|
| Yellow | Orange | Yellow | Orange | |
| Association of blue-throated territories | ||||
| Observed | 45 | 31 | 45 | 31 |
| Expected | 40.1 | 35.9 | 40.7 | 35.3 |
| Association of blue-throated cosirings | ||||
| Observed | 12 | 20 | 7 | 14 |
| Expected | 16.9 | 15.1 | 11.3 | 9.7 |
|
kinship
|
cervus
|
|||
|---|---|---|---|---|
| Yellow | Blue | Yellow | Blue | |
| Association of orange-throated territories | ||||
| Observed | 36 | 31 | 36 | 31 |
| Expected | 36.2 | 30.8 | 38.5 | 28.5 |
| Association of orange-throated cosirings | ||||
| Observed | 24 | 20 | 14 | 6 |
| Expected | 23.8 | 20.2 | 11.5 | 8.5 |
Expected values are estimated from row and column totals in the 2 × 2 contingency table. Results from kinship and cervus analyses are identical: Blue-throated males share paternity less with yellow-throated males than would be expected from their spatial associations; shared paternity between orange-throated males and the other two morphs is not significantly different than would be predicted from their spatial associations.
Combining our paternity data with demographic data on individual survival indicates that many offspring were a result of fertilizations that occurred after their sire was already dead. Posthumous sirings are assumed only when males are known to have produced at least one offspring during the season (and thus are known to have copulated at least once) but disappeared after the first breeding period. Individuals not sighted in two months of daily censuses were assumed to have died; to discount emigration we also surveyed a 600 m-wide perimeter area around our site. We estimate from the kinship paternity data that 32% of the offspring in the third reproductive bout (23 of 72 offspring) were the unambiguous product of fertilizations that occurred long after the father's death (Table 5). The mean number of offspring posthumously sired by each morph was not significantly different during the second reproductive bout. However, by the third reproductive bout, yellow-throated males show a significantly higher number of posthumous fertilizations (Table 5; P < 0.02, adjusted for multiple comparisons by Sequential Bonferroni). Likewise, the number of posthumous fertilizations by males increases later in the season if we use the sires assigned by cervus in the second and third reproductive bouts combined (Table 5; P < 0.02). Both orange- and yellow-throated males produce more posthumous offspring later in the season, and yellow-throated males show significantly higher mean rates of posthumous fertilizations than blue-throated males (Table 5).
Table 5.
Posthumous fertilizations by males of different morphotypes estimated from the kinship and cervus data
| Mean number of posthumous progeny/male | SE | Total number of progeny for each morph | ||
|---|---|---|---|---|
| kinship | ||||
| Clutch 1 | ||||
| Orange | (0.833) | 0.401 | 5 | ANOVA, P = 0.43 |
| Blue | (1.056) | 0.206 | 19 | |
| Yellow | (1.538) | 0.475 | 20 | |
| Clutch 2 | ||||
| Orange | 1.000 | 0.447 | 6 | ANOVA, P = 0.56 |
| Blue | 0.611 | 0.143 | 11 | |
| Yellow | 0.846 | 0.274 | 11 | |
| Clutch 3 | ||||
| Orange | 0.333 | 0.211 | 2 | ANOVA, P < 0.02 |
| Blue | 0.222 | 0.129 | 4 | |
| Yellow | 1.308 | 0.414 | 17 | |
| cervus | ||||
| Clutch 1 | ||||
| Orange | (1.20) | 0.58 | 6 | ANOVA, P = 0.72 |
| Blue | (0.80) | 0.22 | 20 | |
| Yellow | (0.73) | 0.26 | 16 | |
| Clutch 2/3 | ||||
| Orange | 0.60 | 0.40 | 3 | ANOVA, P < 0.02 |
| Blue | 0.04 | 0.04 | 1 | |
| Yellow | 0.59 | 0.20 | 13 |
We estimated the mean number of progeny sired by dead males in the second and third reproductive bouts of the season and compared those to the mean number of progeny sired by males in the first reproductive bout (in parentheses) when all males were still alive. Yellow-throated males sire significantly more posthumous offspring at the end of the reproductive season [kinship: Fisher's Least Significant Difference (LSD) post-hoc comparisons O vs. Y, P = 0.01; O vs. B, P = 0.68; B vs. Y, P = 0.008; cervus: Fisher's LSD post-hoc comparisons O vs. Y, P = 0.87; O vs. B, P = 0.24; B vs. Y, P = 0.03].
Discussion
The high heritability estimates for throat color based on our paternity analysis are similar to those estimated from behavioral assessment of putative sires (h2n = 0.96; ref. 6) and indicate that the three behavioral and morphological variants in this system are genetically-based. It is important to distinguish between genetically-based systems and those that are facultative or condition-dependent (23, 24) because the conditions favoring their evolution and maintenance are different. In a facultative system, sneakers should be maintained in a population provided that in some situations the benefit of sneaking outweighs the cost. On the other hand, genetically-based alternative mating tactics will be maintained only as an evolutionary stable state (ESS; ref. 9) when male strategies have equal fitness or when there is frequency-dependent selection for different genotypes (1, 6).
Orange-throated males were the commonest morph from 1991–1993; thus, we would predict from the frequency-dependent nature of the rock-paper-scissors model that yellow-throated males would have high reproductive success in this interval, until they become the commonest morph in 1994. In 1992, the average number of sired offspring and the number that survive to the following reproductive season (1993) do not vary significantly by morph, suggesting that increased fitness of yellow-throated males was spread over more than one reproductive season. The rock-paper-scissors model predicts that the fitness advantage of any rare morph is accrued over 2–3 years of the cycle. Testing long-term predictions of equal male fitness for the morphs over the entire cycle will require at least 5 years of paternity data on the rock-paper-scissors cycle.
Although the frequencies of males adopting each strategy in the rock-paper-scissors game change during a five year cycle (6), the processes of male–male competition that drive the cycle should remain constant and arise from interactions among neighboring males. Our data on territorial behavior and relative reproductive success of the three male strategies support predictions of the rock-paper-scissors model for the dyadic interactions among morphs (6). Nearest-neighbor analyses indicate that yellow-throated “sneaker” males associate with the blue-throated territorial males less frequently than expected by chance (Table 3), possibly because mate-guarding by blue-throated males deters yellow-throated sneakers. Orange-throated males associate with yellow-throated males at rates not significantly different from expected if morphs were randomly distributed on the site (Table 3). Finally, yellow-throated males are more likely to have another yellow-throated male as a nearest neighbor suggesting that they are clumped in space, which is not surprising given that this morph does not defend territories.
Pairwise comparisons of shared paternity within a female's clutch indicate that yellow- and blue-throated males share paternity at rates lower than expected (Table 3), suggesting that blue-throated males successfully curb the sneaking behaviors of the yellow-throated males. In contrast, orange-throated males share paternity with yellow-throated males at rates significantly higher than expected by chance (Table 3), confirming their vulnerability to the yellow-throated sneaker strategy. The rock-paper-scissors model predicts this pattern; the behavioral strategy of yellow-throated sneaker males should allow them to identify orange-throated males and specifically target females on their territories for secretive copulations.
Blue-throated males infrequently share paternity with yellow-throated males but commonly share paternity with orange-throated males, despite the fact that during this season blue-throated territories were more often neighbors to yellow-throated home ranges than orange-throated territories (Table 4). Thus, blue-throated males successfully deter neighboring yellow-throated males from copulating with their females, but they cannot exclude aggressive orange-throated neighbors, and consequently more frequently share paternity with the ultradominant orange-throated morph. In contrast, there are no significant differences in the rates of territorial and paternity associations between orange-throated males and males of the other two morphs (Table 4). Orange-throated males are unable to exclude yellow-throated sneakers from their territories, resulting in high rates of shared paternity between these two morphs (Table 4). Sneaker males capitalize on the times when orange-throated males leave females unattended and copulate with females on the orange-throated male's territory. Concurrently, orange-throated males successfully invade the territories of blue-throated neighbors and copulate with their females (digital videos typifying pairwise behavioral interactions among morphs can be viewed at http://www.biology.ucsc.edu/∼barrylab), resulting in rates of paternity that would be expected from their territorial associations.
Our results on the distribution of posthumous fertilizations suggest that yellow-throated sneaker males also exploit sperm storage as an important component of their reproductive strategy (Table 5). Sperm storage has been demonstrated in many lizards (25, 26); however, the potential fitness consequences of sperm competition have been significantly underestimated in natural populations. Yellow-throated males derive a significantly higher mean fitness from posthumous fertilizations later in the season compared with other morphs (Table 5); this difference cannot be attributed to increased mortality because orange-throated males are the shortest-lived morph in this population (6). The mechanisms underlying these differences in posthumous siring are unknown but could be the result of cryptic female choice (27, 28) or perhaps a higher quantity or quality of sperm of yellow-throated males. Our paternity analysis suggests that two-month-old sperm of yellow-throated males transferred to females on the first clutch competes successfully (mean fitness = 1.31 ± 0.414, n = 13) with fresh sperm of surviving males that potentially inseminated females during the third mating bout (mean fitness = 0.92 ± 0.201, n = 54).
Male fitness can be partitioned into distinct episodes involving different processes of sexual and natural selection. First, a male must successfully breed with females to sire young; in the rock-paper-scissors system this is a direct result of behavioral strategies that each morph adopts in competing with its neighbors. Second, a male's offspring must survive and reproduce the following year to contribute to his fitness. The outcome of this second component depends on numerous factors including differential hatchling survival for each male morph, differential hatchling survival in each reproductive bout, or the contribution of maternal effects such as differential allocation by females to their clutches. In this study, we used the number of hatchlings sired by each male type as an index of male fitness because we detected no significant biases in hatchling mortality or maternal allocation that would confound our estimates. Despite significant hatchling mortality during the season, we did not observe a morph difference in the survival of progeny to maturity (ANOVA, F2,310 = 1.11, P = 0.33), and this holds true for all reproductive bouts (ANOVA, interaction between morph and clutch: F4,310 = 1.04, P = 0.39). We also did not find significant differences in maternal allocation to progeny (assessed by egg size) as a function of the morph that sired those progeny (ANOVA, F2,312 = 0.68, P = 0.51). Thus, although survival of hatchlings to maturity and possible maternal contributions to hatchling survival may have effects on the fitness of morphs (29), in this year these effects did not seem to alter the outcome of male competition and sperm competition that are intimately related to the reproductive success of male strategies.
We have confirmed that the frequency-dependent selection of the rock-paper-scissors game can arise from local interactions among pairs of males adopting different mating strategies. During the 1992 breeding season, each morph successfully used a different tactic to exploit weaknesses of another strategy and a morph's success depended on the close proximity of a vulnerable alternative strategy. Dominant orange-throated territorial males successfully maintain larger territories with many females, but they are unable to thwart the sneaking tactics of yellow-throated males. Blue-throated males rely on mate-guarding a limited number of females to curb cuckoldry by yellow-throated female mimics, but mate-guarding is not successful against aggressive orange-throated males. Finally, the sneaking tactics of yellow-throated males is most effective against polygynous orange-throated males, and sneaker males also augment fitness with higher levels of posthumous fertilizations later in the season. Frequency-dependent selection arising from local competition can promote conditions that favor each morph, and thus preserve all three strategies of the rock-paper-scissors cycle in the long term.
Acknowledgments
We thank L. Morrison, M. Curran, L. Waits, and R. Ward for their help in developing Uta microsatellite loci; K. Allred for collection of samples; W. B. Dean and L. Barcellos for assistance in the laboratory; and T. Comendant, H. W. Greene, W. R. Rice, E. Svensson, M. Wainstein, T. Burke, R. Calsbeek, D. B. Wake, and two anonymous reviewers for comments on previous versions of the manuscript. We thank D. DeNardo and the 1992 Los Baños field crew for their expert lizard-noosing of all oranges, blues, and yellows. This work was supported by National Science Foundation grants (to B.S.) and a National Science Foundation Minority Postdoctoral Fellowship (to K.Z.).
Footnotes
This paper was submitted directly (Track II) to the PNAS office.
Article published online before print: Proc. Natl. Acad. Sci. USA, 10.1073/pnas.011544998.
Article and publication date are at www.pnas.org/cgi/doi/10.1073/pnas.011544998
References
- 1.Gross M R. Philos Trans R Soc London B. 1991;332:59–66. [Google Scholar]
- 2.Lank D B, Smith C M, Hanotte O, Burke T, Cooke F. Nature (London) 1995;378:59–62. [Google Scholar]
- 3.Shuster M, Wade M J. Nature (London) 1992;350:606–661. [Google Scholar]
- 4.Moore M C, Thompson C W. In: Progress in Clinical and Biological Research, Proceedings of the Eleventh International Symposium on Comparative Endocrinology. Epple A, Scanes C G, Stetson M H, editors. New York: Wiley–Liss; 1990. pp. 685–690. [Google Scholar]
- 5.Thompson C W, Moore I T, Moore M C. Behav Ecol Sociobiol. 1993;33:137–146. [Google Scholar]
- 6.Sinervo B, Lively C M. Nature (London) 1996;380:240–243. [Google Scholar]
- 7.Gross M R. In: Fish Reproduction: Strategies and Tactics. Wooton R, Potts G, editors. London: Academic; 1984. pp. 55–75. [Google Scholar]
- 8.Phillip D P, Gross M R. Mol Ecol. 1994;3:563–569. [Google Scholar]
- 9.Maynard Smith J. Evolution and the Theory of Games. Cambridge, U. K.: Cambridge Univ. Press; 1982. [Google Scholar]
- 10.Maniatis T E, Frisch E F, Sambrook J. Molecular Cloning: A Laboratory Manual. Plainview, NY: Cold Spring Harbor Lab. Press; 1982. [Google Scholar]
- 11.Goodnight K F, Quellar D C, Poznansky T. KINSHIP V. 1.1.2, Goodnight Software. distributed by the author; 1996. [Google Scholar]
- 12.Goodnight K F, Queller D C. Mol Ecol. 1999;8:1231–1234. [Google Scholar]
- 13.Marshall T C, Slate J, Kruuk L E B, Pemberton J M. Mol Ecol. 1998;7:639–655. doi: 10.1046/j.1365-294x.1998.00374.x. [DOI] [PubMed] [Google Scholar]
- 14.Queller D C, Goodnight K F. Evolution. 1989;43:258–275. doi: 10.1111/j.1558-5646.1989.tb04226.x. [DOI] [PubMed] [Google Scholar]
- 15.DeNardo D F, Sinervo B. Horm Behav. 1994;28:53–65. doi: 10.1006/hbeh.1994.1005. [DOI] [PubMed] [Google Scholar]
- 16.Tinkle D W, McGregor D, Dana S. Ecology. 1962;43:223–229. [Google Scholar]
- 17.Gabriel K R, Sokal R R. Syst Zool. 1969;18:259–270. [Google Scholar]
- 18.Sinervo B. MACTURF. distributed by the author; 1996. [Google Scholar]
- 19.Gullberg A, Olsson M, Tegelstrom H. Mol Ecol. 1997;6:105–112. [Google Scholar]
- 20.Murie J O. Can J Zool. 1995;73:1819–1826. [Google Scholar]
- 21.Höggren M, Tegelstrom H. Copeia. 1995;1995:271–277. [Google Scholar]
- 22.Sinervo B, DeNardo D F. Evolution. 1996;50:1299–1313. doi: 10.1111/j.1558-5646.1996.tb02370.x. [DOI] [PubMed] [Google Scholar]
- 23.DeWoody J A, Fletcher D E, Wilkins S D, Nelson W S, Avise J C. Evolution. 1998;52:1802–1810. doi: 10.1111/j.1558-5646.1998.tb02257.x. [DOI] [PubMed] [Google Scholar]
- 24.Jones A G, Ostlund-Nilsson S, Avise J C. Evolution. 1998;52:848–858. doi: 10.1111/j.1558-5646.1998.tb03709.x. [DOI] [PubMed] [Google Scholar]
- 25.Villaverde G A, Zucker N. The Southwestern Naturalist. 1998;43:92–95. [Google Scholar]
- 26.Birkhead T R, Møller A P. Biol J Linn Soc. 1993;50:295–311. [Google Scholar]
- 27.Eberhard W G. Female Control: Sexual Selection by Cryptic Female Choice. Princeton: Princeton Univ. Press; 1996. [Google Scholar]
- 28.Olsson M, Shine R, Madsen T, Gullberg A, Tegelström H. Nature (London) 1996;383:585. doi: 10.1111/j.1558-5646.1996.tb02372.x. [DOI] [PubMed] [Google Scholar]
- 29.Sinervo B, Svensson E, Comendant T. Nature (London) 2000;406:985–988. doi: 10.1038/35023149. [DOI] [PubMed] [Google Scholar]