A Polygenic Hypothesis for Sex Determination in the European Sea Bass Dicentrarchus labrax

Abstract

Polygenic sex determination, although suspected in several species, is thought to be evolutionarily unstable and has been proven in very few cases. In the European sea bass, temperature is known to influence the sex ratio. We set up a factorial mating, producing 5.893 individuals from 253 full-sib families, all reared in a single batch to avoid any between-families environmental effects. The proportion of females in the offspring was 18.3%, with a large variation between families. Interpreting sex as a threshold trait, the heritability estimate was 0.62 ± 0.12. The observed distribution of family sex ratios was in accordance with a polygenic model or with a four-sex-factors system with environmental variance and could not be explained by any genetic model without environmental variance. We showed that there was a positive genetic correlation between weight and sex (r_A = 0.50 ± 0.09), apart from the phenotypic sex dimorphism in favor of females. This supports the hypothesis that a minimum size is required for sea bass juveniles to differentiate as females. An evolution of sex ratio by frequency-dependent selection is expected during the domestication process of Dicentrarchus labrax populations, raising concern about the release of such fish in the wild.

IN gonochoric species with genetically determined sex, a one-to-one sex ratio is known to be optimal in an infinite population of diploid individuals with random mating and Mendelian segregation (Fisher 1930; Charnov 1975). The observation of skewed sex ratios may imply, among other things, non-Mendelian segregation as in Drosophila (Vaz and Carvalho 2004), nonrandom mating (Hamilton 1967), or environmental sex determination (ESD; Bull 1985). In the latter case, the sex of an individual is not fixed at conception, but is influenced by environmental conditions during its early life. ESD is expected to be favored when the offspring lives in patchy environments, which may confer advantages to being male or female, and neither the offspring nor the parent have control and/or predictive ability on the type of patch in which the offspring will live (Charnov and Bull 1977). Temperature (e.g., Bull and Vogt 1979; Baroiller and D'Cotta 2001) seems to be the main environmental factor implied, but density (Ellenby 1954) and social status (Francis and Barlow 1993) have been shown to be possible sex-determining environmental factors. In species with ESD, in many cases there is also a genetic variation (Bull et al. 1982; Conover and Heins 1987a; Janzen 1992; Baroiller and D'Cotta 2001), which in some cases has been described as polygenic (Bull et al. 1982; Janzen 1992). Polygenic sex determination, however, is considered to be evolutionarily unstable (Rice 1986) and its maintenance is still poorly understood. It is thought by some authors to be the ancestral type of sex determinism in fish (Kirpichnikov 1981), but organisms where it is accepted that sex has a polygenic component are indeed very few: the parasitic wasp Nasonia vitripennis (Orzack and Gladstone 1994), the turtles Graptemys ouachitensis (Bull et al. 1982) and Chelydra serpentina (Janzen 1992), and probably the swordtail fish Xiphophorus helleri (Kosswig 1964).

The European sea bass (Dicentrarchus labrax) is a gonochoristic teleost fish distributed in the northeastern Atlantic, the Mediterranean, and the Black Sea (Pickett and Pawson 1994). They live in shallow, coastal waters, estuaries, lagoons, and harbors, moving to deeper waters (up to 100 m deep) as they grow. Although they can live in waters <5°, they seek temperatures >10°, and even 15° in their first year (Kelley 1988). They spawn in open waters from late winter to early spring, depending on the latitude. The eggs hatch in 4–9 days, and the young fish move inshore in their first month toward the warmest waters, especially in estuaries (Pickett and Pawson 1994). Sex remains undifferentiated for a long period: differentiation occurs between 128 and 250 days postfertilization (dpf; Saillant et al. 2003a). Records of sea bass sex ratio in wild populations are scarce. They show balanced sex ratios (Saillant et al. 2003a) and an excess of males (B. Menu, personal communication) or of females (Arias 1980), but as a whole do not contradict the hypothesis of balanced sex ratios in the wild. The sea bass is an important species in Mediterranean aquaculture, and it appears that, in all aquaculture populations, sex ratios are strongly biased toward males (75–95%, e.g., Blazquez et al. 1998; Saillant et al. 2002, 2003a), which is a problem for farmers as males mature earlier and grow less than females. Temperature has been shown to have a major effect on sex determination in sea bass (Blazquez et al. 1998; Pavlidis et al. 2000; Saillant et al. 2002; Mylonas et al. 2005). The effect of temperature is not fully understood, as two studies show an increased proportion of males with cold temperature (15°: Blazquez et al. 1998; 13°: Saillant et al. 2002) while the other two studies show an increased proportion of females at 13° and 15° (Pavlidis et al. 2000; Mylonas et al. 2005). The current hypothesis is that low temperatures early in development (<100 dpf) may favor female sex differentiation, but that long-lasting low temperatures, through a negative effect on growth, may preclude female differentiation and result in an increased proportion of males (Piferrer et al. 2005). Thus, for productivity reasons, the excess of males observed in culture would be due to the use of temperatures higher than in nature. From the genetic point of view, in addition to the environmental effect on sex, simple female homogamety can be excluded, as the sex ratios of normal diploid and gynogenetic offspring are equivalent (Felip et al. 2002; Peruzzi et al. 2004). The sex ratio of the offspring from masculinized females is not female biased and would rule out both XX–XY (female homogamety) and ZW–ZZ (male homogamety) systems (Blazquez et al. 1999). In this latter study, however, the possible male bias induced by high rearing temperatures (22.5°), and the impossibility of ascertaining the genetic sex of the sex-reversed parents, makes the demonstration a little weak. Therefore, male homogamety with environmentally male-biased sex ratios would still be a possibility. Additionally, parental influence on the sex ratio of progeny has also been demonstrated, in very limited experimental settings, however (Saillant et al. 2002; Gorshkov et al. 2003), showing that there is a genetic component of the progeny sex ratio. Although it is clear that the sex of sea bass is determined both by genetic factors and by the environment (mostly temperature), the sex determination system of this species remains basically unknown (Piferrer et al. 2005).

In this study, our aim was to describe the genetic component influencing sex ratio in sea bass, using a large number of families in classical aquaculture conditions, and to determine which genetic models could describe it best. We described sex using a threshold model with an underlying variable (sex tendency), as this type of model integrates both genetic and environmental effects, which are known to influence sex ratio in the sea bass.

MATERIALS AND METHODS

A partly factorial mating design:

The brood fish used were from a group of 33 males and 51 females of wild Atlantic origin, collected in 2000 on the coasts of Brittany, France. Each brood fish was individually tagged and fin clipped for DNA extraction. The sperm of males was cryopreserved in 250 μl straws (Fauvel et al. 1998). In January 2001, 51 females were injected with 10 μg/kg luteinizing hormone-releasing hormone (Sigma, D-TRP6-LHRH), and eggs were stripped 72 hr later. Twenty-three females gave a sufficient quantity of good quality oocytes. From these spawns, we produced a mating design combining 33 males and 23 females in three full factorial sets of 11♂ × 9♀, 11♂ × 7♀, and 11♂ × 7♀, for a total of 253 families. All full-sib families were fertilized individually and then eggs were grouped by female for incubation (48 hr at 13°), after which 2 ml of viable eggs per female (∼2.000 eggs/female) were collected to create one batch containing all families. Standard rearing conditions were used, with temperature gradually increasing from 13° to 18° in the first 64 days. Temperature was then kept at 18° until 238 days (mean length of fish was 117 mm, and mean weight was 23.6 g) and then lowered to 14° to slow down growth until the time scheduled for tagging. Although late low temperatures are suspected of masculinizing the progeny (Piferrer et al. 2005), this does not apply at 238 days, as it was shown before that lowering the temperature from 20° to 13° at 149 days (mean length was 81 mm) had no impact on the sex ratio (Saillant et al. 2002).

Recording of traits and parentage assignment:

At 370 days, the fish had reached a mean weight of 35 g. Seven thousand of them were randomly selected, on which individual weight and length were measured. Each fish was individually tagged and fin clipped for DNA extraction. The fish were then sent to four different sites (1.750/site), where they were reared until reaching ∼400 g mean weight. This rearing in different sites was designed for estimating genetic parameters and genotype–environment interactions for growth and quality traits, in parallel with this study. Still, it was not expected to have any impact on the sex ratio, as the differentiation period is over well before 370 days. At 400 g, the remaining fish (5.988) were slaughtered and sex was recorded by visual observation of the gonads after dissection; 5.960 had an identifiable sex phenotype. In all sites, the difference between males and females was straightforward (female gonads were orange, and male gonads pink/white), and only 28 fish in total could not be determined with certainty. Parentage assignment was done by Landcatch Natural Selection (Alloa, UK) using six microsatellite markers on both parents and offspring. Of the 5.960 offspring with a sex phenotype, 5.896 (98.9%) could be assigned to a single parental pair.

Statistical methods:

Sire 23 (set 3) gave only 3 offspring, probably due to bad sperm quality. It was removed from the analysis, as it created a major disequilibrium in the data, thus reducing the number of families studied from 253 to 246. Thereafter, the base data set comprised 5.893 offspring from 246 families. Apart from sire 23, 245 of 246 possible families had offspring. No offspring were found in the sire 9 × dam 2 family (set 1), probably due to a bad quality straw of cryopreserved sperm, as both male 9 and female 2 gave satisfactory results in all other crosses. To avoid computational problems, these missing data were replaced by simulated data corresponding to the expected numbers of male and female offspring in this family (19 males and three females, which are the average numbers of males and females per family produced by male 9 and female 2).

In a first analysis, the number of females was calculated in each paternal or maternal half-sib family and compared to the expected number of females with a uniform proportion corresponding to the observed proportion of females in the whole sample, using χ² tests to test for the existence of significant genetic variation in progeny sex ratio. In a second step, the family sex ratios in each of the three full-factorial sets were analyzed by logistic regression using SAS proc Logistic, where the proportion of females was explained by a sire and dam effect. The model fit was tested using the Hosmer and Lemeshow test (Hosmer and Lemeshow 1989). In a third step, sex was considered as a threshold trait with a polygenic basis (Bulmer and Bull 1982). Sex was analyzed using a single-trait model including additive random effects for sire and dam and a residual error. Restricted maximum-likelihood estimates of variance components for the random effects in the model were obtained on the underlying liability scale using the ASREML software (Gilmour et al. 2002). Both sire and dam heritabilities were estimated, using standard formulae (Becker 1984). Genetic correlations between sex and growth were estimated with a trivariate (sex, weight, and length at 370 days) animal model, with sex coded on the observed scale (0 or 1), using the VCE5.0 software (Kovac and Groeneveld 2003). This model included an animal additive genetic effect for all traits and for length and weight, a fixed effect of sex, which was necessary due to sex dimorphism on length and weight (females are larger than males). We used the observed scale since it produces unbiased genetic correlations as long as the threshold trait does not have both low heritability and low incidence (Olausson and Ronningen 1975). We also estimated the heritability of sex dimorphism for growth using classic formulas of (co)variance for a difference (Chapuis et al. 1996).

Then, we used the estimates generated to simulate samples of 5.893 fish from 23 dams and 32 males in three sets of 9 × 11, 7 × 10, and 7 × 11, using a heritability of sex of 0.62 on the underlying scale, and a mean proportion of females of 18.3%. We also generated simulated samples of the same size using a threshold model where the underlying sex tendency m would be determined, instead of polygenes, by one or two biallelic loci with the effect size f, such that genotype aa had a genetic effect of −f on m, genotype Aa had a genetic effect of 0, and genotype AA had a genetic effect of +f. The allelic frequencies were 0.5 for each allele, except for the one locus case where allelic frequencies 0.2, 0.4, 0.5, 0.6, and 0.8 were tested. The effect size f was tuned so that the proportion of genetic variance over phenotypic variance was 0.62, and a random residual effect representing 38% of the total phenotypic variance was added. This model is an extension of the two-factor model with environmental variance, adapted to populations with skewed sex ratios (Bull 1983). Purely genetic threshold models were also tested, either polygenic with h² = 1 or with one to five biallelic loci, but no residual environmental variance. For each model, we generated 10.000 samples and compared the simulated distribution of full- and half-sib family sex ratios with the observed one, to test for the coherence of the model with the observed data set.

RESULTS

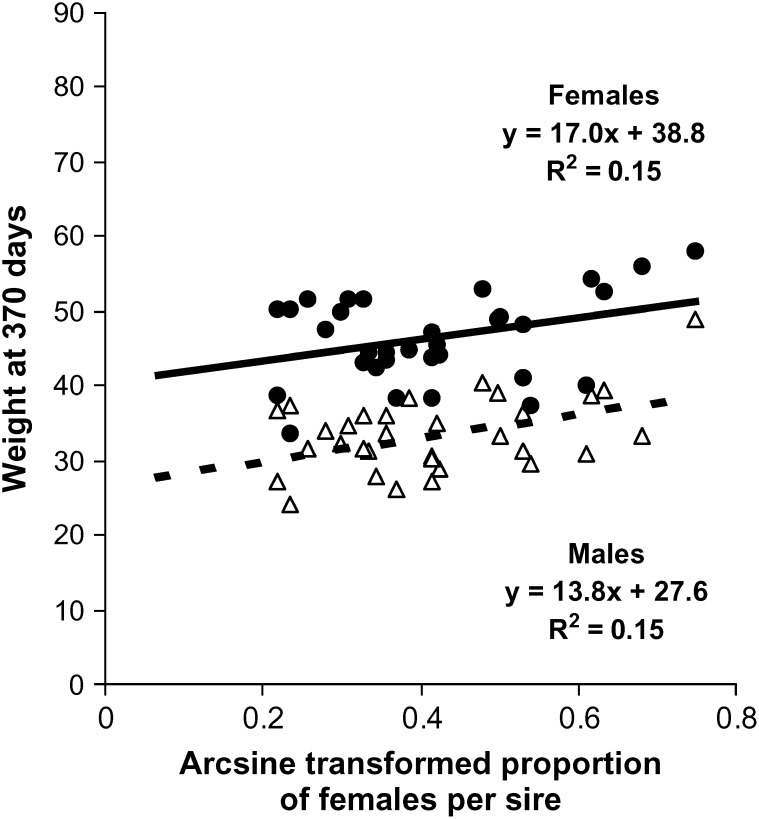
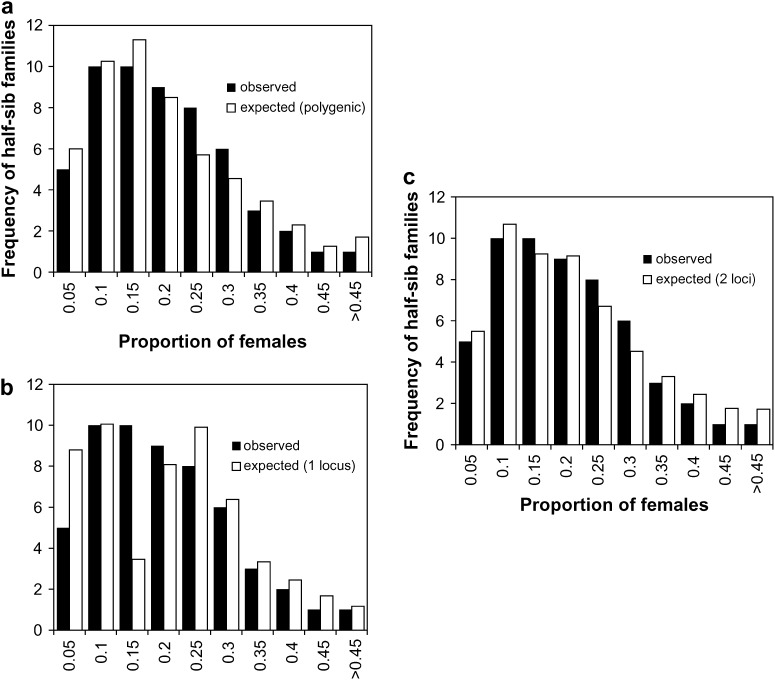
The overall proportion of females in the population was 18.3%, and there were no sex-ratio differences among fish from each of the four growing sites (17.4–19.4%, χ² = 4.62, 3 d.f., P > 0.20). Family sex ratios are given in appendixes a–c. Comparison of observed values to expected values under the hypothesis of equal sex ratio in all families shows a strong inequality of contributions between half-sib families (χ² = 350.6, 31 d.f., P < 0.0001 for paternal half-sib families and χ² = 327.6, 22 d.f., P < 0.0001 for maternal half-sib families). Proportions of females ranged from 4.7 to 46.3% in paternal half-sib families and from 0.5 to 40.3% in maternal half-sib families. The logistic regression analysis showed that both sire and dam had a highly significant effect on the progeny sex ratio (P < 0.0001) and that this model without interaction was enough to explain the observed data set, the Hosmer and Lemeshow χ² tests being far from significance (χ² = 6.04, 8 d.f., P > 0.6 in set 1, χ² = 1.94, 8 d.f., P > 0.9 in set 2, and χ² = 6.04, 8 d.f., P > 0.6 in set 3). The estimated heritability of sex on the underlying scale was 0.52 ± 0.13 (sire heritability), 0.72 ± 0.20 (dam heritability), or 0.62 ± 0.12 (sire and dam heritability). The maternal-effect ratio (nongenetic maternal variance/phenotypic variance) that would explain the difference between sire and dam heritability is m² = 0.05 ± 0.06, which is clearly nonsignificant. The heritability of growth sex dimorphism was low (0.15 for weight and 0.09 for length at 370 days). The estimated genetic correlation was 0.50 ± 0.09 between sex and weight and 0.48 ± 0.09 between sex and length. An illustration of this can be seen in Figure 1, which shows that, apart from the fixed effect of sex on weight (females being on average 40.8% heavier than males at 370 days), larger fish tended to be found in the families with the highest proportion of females. The distribution of proportions of females among the 55 half-sib families scored (32 paternal half-sib families, 23 maternal half-sib families) is plotted in Figure 2 and compared with simulated distributions in the same experimental setting. Detailed information about model fit can be found in Table 1. Additive polygenes with h² = 0.62 could explain the data with excellent fit. For a single locus with environmental variance, the best fit was observed with allelic frequencies of 0.6 for the male-orienting allele and 0.4 for the female-orienting allele, but this could not account for the observed half-sib data. On the contrary, a two-locus system with environmental variance could fit both half-sib and full-sib observed data. No purely genetic model (without environmental variance) could explain the observed data, whatever the number of loci implied (from monogenic to polygenic).

APPENDIX A.

Males and females (M:F) in 99 European sea bass families: set 1 (11 sires × 9 dams)

	Dams									Mean proportion of females	Total no. of offspring
Sires	D34	D35	D36	D37	D38	D39	D40	D41	D42
S01	19:2	21:4	20:5	20:12	20:11	19:12	30:9	19:8	30:5	0.256	266
S02	17:0	13:0	16:0	14:3	35:4	26:6	33:3	33:5	21:0	0.092	229
S03	16:1	22:0	25:1	14:3	25:1	36:2	30:9	26:8	31:2	0.107	252
S04	7:3	15:0	12:3	10:7	27:9	17:12	36:11	17:7	18:3	0.257	214
S05	14:4	21:2	19:5	5:5	11:7	15:6	20:4	27:7	13:3	0.229	188
S06	16:0	9:0	21:2	10:2	18:3	33:4	22:4	27:8	18:1	0.121	198
S07	14:8	18:3	24:10	12:8	22:8	12:17	29:15	15:10	7:3	0.349	235
S08	9:1	11:1	11:2	6:0	22:0	18:0	31:0	6:2	7:0	0.047	127
S09	16:2	a	14:0	21:1	37:4	24:3	22:4	15:5	17:0	0.103	185
S10	3:7	14:3	8:3	4:12	14:7	12:14	18:16	15:12	13:13	0.463	188
S11	11:8	24:0	20:5	20:21	17:11	19:16	28:13	22:14	30:6	0.330	285
Mean proportion of females	0.202	0.072	0.159	0.352	0.208	0.285	0.227	0.279	0.149
Total no. of offspring	178	181	226	210	313	323	387	308	241		2367

^aNo offspring were observed in this family.

APPENDIX B.

Males and females (M:F) in 77 European sea bass families: set 2 (11 sires × 7 dams)

	Dams							Mean proportion of females	Total no. of offspring
Sires	D43	D44	D45	D46	D47	D48	D49
S12	29:4	17:3	15:0	20:1	19:0	27:0	12:0	0.054	147
S13	15:8	34:8	30:4	47:2	29:4	35:1	11:6	0.141	234
S14	11:17	27:2	12:0	28:0	26:4	26:2	7:3	0.170	165
S15	18:3	28:3	18:1	19:0	24:0	18:0	16:0	0.047	148
S16	9:16	21:11	26:4	28:3	38:12	20:4	13:6	0.265	211
S17	25:18	34:15	27:1	37:1	39:6	34:1	25:2	0.166	265
S18	11:10	29:5	31:1	26:1	41:1	35:2	9:1	0.103	203
S19	11:10	22:2	13:1	21:0	26:3	27:1	18:2	0.121	157
S20	19:10	24:9	14:1	28:1	29:1	31:1	14:1	0.131	183
S21	18:10	11:5	11:2	25:1	18:1	26:3	14:2	0.163	147
S22	16:17	11:15	24:8	22:1	14:8	16:3	11:5	0.333	171
Mean proportion of females	0.403	0.232	0.094	0.035	0.117	0.058	0.157
Total no. of offspring	305	336	244	312	343	313	178		2031

APPENDIX C.

Males and females (M:F) in 70 European sea bass families: set 3 (10 sires × 7 dams)

	Dams							Mean proportion of females	Total no. of offspring
Sires	D50	D51	D52	D53	D54	D55	D56
S24	3:1	4:0	5:0	5:0	5:1	7:0	13:2	0.087	46
S25	13:3	24:9	12:2	29:0	24:9	18:1	43:20	0.213	207
S26	34:9	9:5	10:1	32:0	12:2	11:0	15:7	0.163	147
S27	22:4	17:6	9:0	27:0	22:2	6:0	21:4	0.114	140
S28	44:4	19:2	8:0	23:0	24:4	9:0	18:2	0.076	157
S29	13:8	9:9	4:3	10:1	6:4	5:1	6:9	0.398	88
S30	37:10	21:9	17:2	33:0	38:7	16:0	20:7	0.161	217
S31	33:6	20:9	14:1	25:0	18:11	14:1	19:15	0.231	186
S32	29:3	31:4	16:0	18:0	28:2	6:0	30:0	0.054	167
S33	26:0	27:3	9:0	12:0	22:1	11:0	24:5	0.064	140
Mean proportion of females	0.159	0.236	0.080	0.005	0.178	0.028	0.254
Total no. of offspring	302	237	113	215	242	106	280		1495

Figure 1.—

Relationship between the arcsine-transformed proportion of females in sire half-sib families of European sea bass and mean weight at 370 days of male and female offspring in the same families.

Figure 2.—

Observed frequencies of females in 55 half-sib families of European sea bass and expected frequencies under a threshold model with 18.3% females in the offspring and 38% environmental variance, where the genetic component of the underlying variable is (a) polygenic; (b) one biallelic locus with p = 0.6 for the masculinizing allele, q = 0.4 for the feminizing allele; and (c) two biallelic loci with p = q = 0.5.

TABLE 1.

Comparison of family sex-ratio distributions with simulated distributions from various sex-determination models

	Sex-ratio distribution of half-sib families (d.f. = 6)		Sex-ratio distribution of full-sib families (d.f. = 11)
Model	χ2	P-value	χ2	P-value
Polygenic, h2 = 0.62	2.07	>0.9	10.9	>0.4
One locus with environmental variance
p = 0.2	58.1	<0.001	171	<0.001
p = 0.4	59.6	<0.001	18.4	>0.05
p = 0.5	22.9	<0.001	6.2	>0.8
p = 0.6	14.8	<0.05	10.2	>0.5
p = 0.8	18.2	<0.01	28.1	<0.01
Two loci with environmental variance, p = 0.5	1.42	>0.9	12.6	>0.3
One locus without environmental variance	383	<0.001	865	<0.001
Two loci without environmental variance	23.9	<0.001	139	<0.001
Three loci without environmental variance	12.8	<0.05	63.3	<0.001
Five loci without environmental variance	6.33	>0.4	38.2	<0.001
Polygenic without environmental variance (h2 = 1)	8.04	>0.2	40.1	<0.001

P-value < 0.05 shows incompatibility between simulated and observed data. p, frequency of the male-orienting allele.

DISCUSSION

The genetic influence on sex in sea bass:

Our results are fully in accordance with a polygenic model, as described by Bulmer and Bull (1982), where the sex of an offspring is determined by the fact that an underlying sex tendency (determined by both polygenes and environmental effects) is greater or less than a threshold value. In our experiment, both sires and dams have an effect on the sex ratio of their progeny, and the effects are similar in size, pleading for an additive genetic effect on sex ratio. The heritability is high (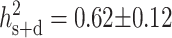) and clearly different from zero. It may not be biased by nonadditive genetic factors, as the mating design is factorial and all fish were in the same environment at the time of sex determination. Moreover, we verified that each dam (χ² = 73, 66 d.f., P > 0.2) and each sire (χ² = 104, 93 d.f., P > 0.2) contributed the same proportion of offspring in all sites and that the same sex ratios were observed in all sites, so even unexpected late actions of the environment on the sex ratio would not bias the between-family data. The “classical” vertebrate chromosomal sex determination model is expected to give 50% males and 50% females and clearly cannot explain the data obtained, even with eventual sex reversal of genetic females to males due to temperature. This holds as long as sex reversal is homogeneous among families (i.e., not genetically determined). If sex reversal was not homogeneous among families, this would imply the existence of a secondary genetic component of sex ratio, in addition to the chromosomal system. Pure ESD can also be excluded, as it is not supposed to yield any between-families differences in sex ratios. This was already suggested by previous results (Blazquez et al. 1999; Saillant et al. 2002; Gorshkov et al. 2003; Peruzzi et al. 2004). As it is clear that environment can influence sex ratio in sea bass (Piferrer et al. 2005), the genetic component that we evidence can be considered either as a genetic variation of the primary sex ratio or as a genetic sensitivity to the environmental effects. As we tested only one environmental condition, however, we cannot distinguish between both. The observed occurrence of intratesticular oocytes in many (up to 62%) young sea bass males (Gorshkov et al. 1999; Saillant et al. 2003a) could be in favor of a polygenic primary sex ratio, with sex reversal occurring as a consequence of environmental effects. However, the existence of genetic-by-environment interactions, which would favor the second hypothesis, has already been evidenced (Saillant et al. 2002). Nevertheless, we show that, whatever its true nature, there is a genetic effect that leads to a continuous distribution of family sex ratios in this species, at least in one (masculinizing) environmental condition. We also show that, in addition to the global effect of temperature, which certainly skewed sex ratio toward males in this experiment, it is necessary to include environmental variance within this global environment to explain the observed distribution of sex ratio, meaning that a purely genetic model where individual sex would be uniformly influenced by the environment could not explain our data. Even with environmental variance allowed, a two-factor system can be excluded, but a four-factor system (two biallelic loci) can explain the observed data. Similarly, in the apple snail, it was concluded that a continuous variation in family sex ratios was most likely due to at least four sex factors (Yusa 2007). Unlike what was seen in the silverside fish Menidia menidia (Conover and Heins 1987b), we could not observe a multimodal distribution of family sex ratios, which was considered an indication of the existence of only a few sex factors in this species. Indeed, as pointed out by Bull (1983), it is extremely difficult to ascertain the polygenic nature of a sex-determining system when compared to a system with only a few factors and some environmental variance. Nevertheless, both systems are expected to behave in a very similar manner and may be described by the Bulmer and Bull threshold model, provided that sex factors have individually weak effects (Bull 1983). Finally, we showed that there was considerable genetic variation for sex ratio in a given environment, as half-sib family sex ratios range from 0.5 to 46.3% of females (the proportions range from 0 to 75% in full-sib families, but their small size—on average 24 individuals—makes it less significant). We can compare this range of variation in female proportions to that produced by temperature treatments: 0–27% (Blazquez et al. 1998), 18–66% (Koumoundouros et al. 2002), 24–74% (Pavlidis et al. 2000), and 11–32% (Saillant et al. 2002). This shows that, in this species, the genetic and environmental components of sex determinism are of comparable magnitude, which confirms that there are no fundamental barriers between ESD and genotypic sex determination, as proposed by Bull (1983).

The genetic relationship between size and sex:

An interesting feature of our results is the relatively strong genetic correlation between sex and size (r_A = 0.50 ± 0.09), which means that some of the genes acting on sex determination and growth are the same, or at least are strongly linked in our sample. This is in accordance with previous experimental evidence showing that females are larger at the time of sex differentiation (Blazquez et al. 1999; Saillant et al. 2001). Still, we have shown that, once corrected for this sex dimorphism, there was still a size advantage in families with high proportions of females (Figure 1). This strengthens a lot the connection that was established between growth and sex. Indeed, as sea bass is a group spawner, it is clear that the size of females should have a strong impact on their fitness, through their absolute fertility, whereas it may be less important for males. Therefore, the idea that a minimum size is needed at the time of sex differentiation to be able to differentiate as female is plausible and strengthened by the fact that the size advantage of females is never as large as at the time of sex differentiation (+41% weight at 1 year and +20% at 2 years in this study; +67% at 10 months and +25% from 2 years in Saillant et al. 2001). This type of determinism is observed in nematodes (Ellenby 1954) and eels (Roncarati et al. 1997), where high density (hence limited resources for growth) favors male differentiation. However, density had no effect on sex ratio in sea bass (Saillant et al. 2003b), but in this latter case the densities and rearing conditions were chosen to avoid any impact on growth, to not confound the effects of growth and density per se.

Evolutionary consequences of polygenic sex in sea bass:

Our heritability estimates for sex ratio are high (0.62), as in turtles, where high estimates (in the range of 0.5–0.8) have also been found (Bull et al. 1982; Janzen 1992). However, the estimates in turtles were likely inflated by maternal and/or dominance effects, due to the use of full-sib designs, which is not the case in our experiment. Moreover, in turtles, the impact of high-heritability estimates was considerably lowered in natural conditions by the high variance between nest temperature, which, combined with the very narrow temperature range for complete sex change, reduces a lot the potential for selection on sensitivity to ESD (Bull et al. 1982). In our case, although temperature has a large effect on sex ratio, it cannot produce 100% female progeny, and there is a wide spectrum (13°–25°) having an impact on sex determination. Still, the temperature of the coastal waters during the first year of life of the sea bass may be quite variable and thus reduce the effectiveness of natural selection on polygenic sex ratio in this species. As our experimental growing conditions are different from natural ones [fish are 13.5 cm at 1 year vs. 7–10 cm in the wild in the Atlantic (Pickett and Pawson 1994)], and with the suspected genotype–environment interactions for sex ratio in sea bass (Saillant et al. 2002), variation that may be hidden in wild (low temperature) conditions may be expressed in experimental or farm (warm) conditions. This may be even accentuated by the growth–sex genetic relationship, as growth also has a high heritability in this experiment (0.54 ± 0.08 for weight) and is expected to have a higher heritability in fast-growing conditions than in slow-growing conditions.

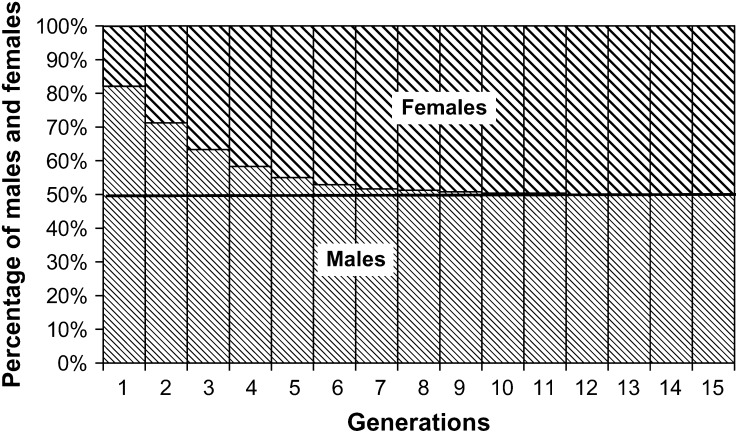
Considering the high heritability of sex ratio observed in our conditions, we could calculate the effects of frequency-dependent selection on sex using the Bulmer and Bull (1982) model (Figure 3). It shows that equilibrium sex ratio should be reached in seven to eight generations. This shift toward females, which are more interesting for aquaculture, could even be accelerated through artificial selection of female-producing families. If artificial selection on growth is also practiced, as is the case in several hatcheries, the shift in sex ratios in aquaculture populations should be even faster and may lead to predominantly female populations. As aquaculture of sea bass expands in the Mediterranean area, the impact of aquaculture escapees, with modified sex determinism, will have to be carefully evaluated, as sex ratio is doubtlessly a major determinant of fitness and may therefore have an important impact on the fitness of natural populations of this species (Lynch and O'Hely 2001).

Figure 3.—

Expected evolution of sea bass sex ratio along generations of random mating in constant environmental conditions, using the frequency-dependent model of Bulmer and Bull (1982), with heritability 0.62 and initial sex ratio 18.3% females (this study's estimates).

Conclusion:

We have demonstrated that sex determinism in the sea bass is not monogenic and is sensitive to within-tank variations in the environment and that the genetic component is essentially additive, is linked to the growth capacity of the fish, is of the same magnitude as the environmental component controlled by temperature, and can be precisely described using a polygenic threshold model with h² = 0.62 on the underlying scale.

Selective breeding experiments are under way to explore the effective response to sex-ratio selection and the correlated sex-ratio response to selection for growth. They will also provide material for QTL search in the coming years, hopefully allowing us by that time to determine more precisely if sex determinism in this species is effectively polygenic or only oligogenic.