Skip to main content
PMC home page
NIHPA Author Manuscripts logoLink to NIHPA Author Manuscripts
. Author manuscript; available in PMC: 2008 Nov 1.
Published in final edited form as: Invest Ophthalmol Vis Sci. 2007 Nov;48(11):5030–5037. doi: 10.1167/iovs.07-0443

Regulation of Corneal Fibroblast Morphology and Collagen Reorganization by Extracellular Matrix Mechanical Properties

Dimitris Karamichos 1, Neema Lakshman 1, W Matthew Petroll 1
PMCID: PMC2080831  NIHMSID: NIHMS33314  PMID: 17962454

Abstract

Purpose

To investigate how extracellular matrix mechanical properties influence cell and matrix patterning in three-dimensional culture.

Methods

Human corneal fibroblasts were seeded within 30 × 10 mm collagen matrices that were unconstrained (UN), fully constrained (CO) along the long axis by attaching the construct to two immobilized plastic bars, or partially constrained (PC) by allowing linear elastic displacement of one bar. After 24 hours, constructs were labeled with phalloidin and were imaged using fluorescent and reflected light (for collagen) confocal microscopy. Cell morphology and local collagen fibril density and alignment were measured using digital image processing.

Results

Corneal fibroblasts in UN matrices were less elongated (UN < PC < CO; P < 0.05) than those in constrained matrices. Cells were aligned parallel to the long axis in the anisotropic region of constrained matrices but were randomly aligned in unconstrained (isotropic) matrices (UN < PC = CO; P < 0.05). Both the local collagen density and the degree of cell/collagen coalignment were higher in constrained matrices (UN < PC < CO; P < 0.05). In regions of higher cell density, additional bands of aligned collagen were often observed between individual cells.

Conclusions

These data suggest that cell spreading, alignment, and contractile force generation are directly influenced by the mechanical properties of the surrounding extracellular matrix (ECM). Corneal fibroblasts generally align and compact collagen parallel to the axis of greatest ECM stiffness. Mechanical cross-talk between adjacent cells leads to enhancement of matrix reorganization, and results in additional, more complex matrix patterning.

Cell-induced matrix reorganization and patterning are fundamental processes in developmental morphogenesis, corneal tissue engineering, and wound healing. During embryonic development, physical forces exerted by corneal fibroblasts organize extracellular matrix into a unique pattern that provides structural support while it maintains transparency.1–5 Similarly, one of the challenges in corneal tissue engineering is the development of an organized stroma with mechanical and optical properties that mimic the native tissue.6–10 Finally, after injury or refractive surgery, wound contraction and matrix remodeling by corneal fibroblasts play a central role in determining corneal clarity, mechanical integrity, and refractive properties.11–13 Despite the importance of cell-induced matrix patterning in corneal stromal biology, few studies have directly investigated the factors that regulate this process.

One potential player in the regulation of cell-induced extracellular matrix (ECM) patterning is the mechanical state of the ECM itself. It is well established that mechanical stimuli play key roles in regulating growth and function in a variety of cell types.14–19 The formation of focal contacts and stress fibers by fibroblasts are tension-dependent processes,20–22 and these structures tend to align along the tensile axis under anisotropic conditions.23–26 Alignment and global force generation parallel to the axis of greatest mechanical resistance by dermal fibroblasts, myoblasts, and bone marrow stem cells have also been documented in three-dimensional (3-D) culture models.27–31 However, the effect of ECM anisotropy on corneal fibroblast behavior has not been assessed. Furthermore, these previous studies were performed using high cell densities, which does not allow detailed investigation of the underlying pattern of matrix reorganization at the cellular and subcellular levels. Thus, the effects of matrix anisotropy on the local pattern of cell-induced ECM remodeling (i.e., compaction and alignment of collagen fibrils) have not been directly assessed.

Confocal microscopy allows quantitative assessment of local changes in ECM organization within 3-D collagen matrices.32–37 In the present study, we applied this technique to directly investigate the role of ECM mechanical anisotropy on corneal morphology and matrix patterning using an in vitro 3-D culture model. Human corneal fibroblasts were cultured at low density within rectangular collagen constructs with varying degrees of mechanical constraint along the long axis. Overall, significant differences in cell alignment, morphology, and matrix reorganization were observed between constrained (anisotropic) and unconstrained (isotropic) matrices. Cells were aligned nearly parallel to the long axis of the construct in constrained matrices, whereas cells in unconstrained matrices showed no preferential orientation. At the ends of cells, local collagen density and degree of cell/collagen coalignment were higher in constrained matrices. Taken together, these data demonstrate for the first time that under anisotropic conditions, isolated corneal fibroblasts tend to align and compact collagen parallel to the axis of greatest effective stiffness. This effect was observed several millimeters away from the location of the physical constraints, indicating that collagen ECM is an effective conduit for long-range transmission of mechanical forces. Interestingly, in regions of higher cell density within constrained matrices, additional bands of compacted and aligned collagen spanning between adjacent cells were often observed, suggesting mechanical coupling which leads to local amplification of matrix patterning.

Materials and Methods

Cell Culture

Experiments were performed using a previously characterized telomerase-infected, human corneal fibroblast (HTK)-extended lifespan cell line.38 HTK cells were cultured in serum-containing (S+) medium consisting of Dulbecco modified Eagle medium (DMEM; Gibco Invitrogen Cell Culture, Carlsbad, CA) supplemented with 1% penicillin, 1% streptomycin, and 1% fungizone (BioWhittaker Inc., Walkersville, MD) and 10% fetal bovine serum (Sigma Chemical, St. Louis, MO).

Collagen Matrices and Constraints

Rectangular collagen constructs were prepared as described previously.31,30 Briefly, 2 mL type I bovine dermal collagen (3 mg/mL; PureCol [Inamed Corp., Fremont, CA]) was added to 0.2 mL of 10× MEM. After dropwise neutralization with 1 M sodium hydroxide, a suspension of 60,000 HTK cells in 0.2 mL DMEM was added to the collagen mixture. The solution containing the cells and the collagen was poured into a 30 × 10 mm well and was allowed to set for 1 hour at 37°C. Three different models were used (Fig. 1).

Figure 1.

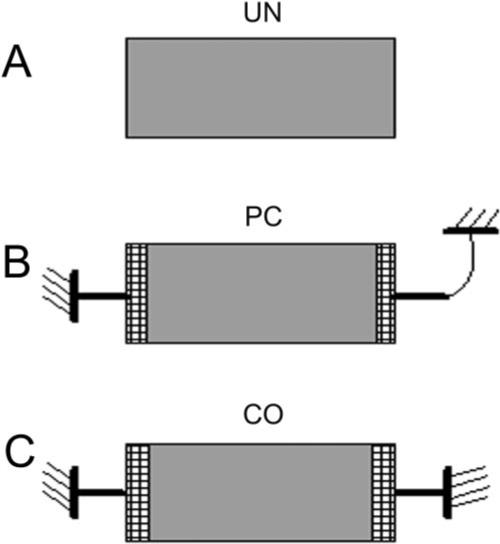
Figure 1

Open in a new tab

Schematic of the three models used. (A) UN model. (B) PC model. (C) CO model.

Unconstrained (UN)

After 1-hour incubation, constructs were released from the bottom of the well and allowed to float in S+ medium (Fig. 1A).

Partially Constrained (PC) along the Long Axis

The two short ends of the construct were attached to plastic bars precoated with collagen (these bars are placed into the well before pouring in the collagen/cell solution). After 1-hour incubation, constructs were released from the bottom. One plastic bar was held in a fixed position, and the other was attached to a flexible copper–beryllium beam that allowed linear elastic displacement (Fig. 1B).

Fully Constrained (CO) along the Long Axis

The two short ends of the construct were attached to plastic bars. After 1 hour of incubation, constructs were released from the bottom. Both bars were held in a fixed position (Fig. 1C).

Experiments were performed with and without cells and were repeated twice (n = 3 for each condition).

F-Actin Staining

After 24-hour incubation, constructs were immediately fixed in 4% formaldehyde for 1 hour and permeabilized with 0.5% Triton X-100 in phosphate buffer for 3 minutes. Cells were then incubated in phalloidin (Alexa Fluor 488, 1:50; Molecular Probes, Eugene, OR) for 60 minutes and washed in phosphate-buffered saline (3 times, 5 minutes each).

With the use of finite element analysis, Eastwood et al.30 have established that the principal mechanical stress that develops during cell-induced matrix contraction is aligned parallel to the long axis of PC or CO rectangular collagen constructs in the central region (Fig. 2, A-zone). This anisotropic behavior is caused by uniaxial constraint along the long axis. They also established the existence of stress-shielded zones adjacent to the plastic bars at the ends of the constructs (Fig. 2, D-zones). In contrast to the A-zone, the mechanical properties of the D-zones remained isotropic during contraction because of stress shielding by the bars. The relative sizes of these two zones are approximately 70% for the A-zone and 15% for each of the two D-zones. In the present study, a region at the center of the A-zone and one near the end of one D-zone were cut out and processed for each construct evaluated. Images were collected from the central area of each region (not at the edges).

Figure 2.

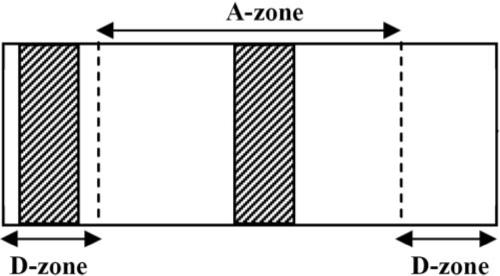
Figure 2

Open in a new tab

Regions selected for imaging and further analysis were obtained from the A- and D-zones of the constructs. Shaded areas represent collagen blocks processed for confocal microscopy. Images were collected from the central area of each region (not at the edges).

Laser Confocal Microscopy

After labeling with phalloidin, fluorescent (for F-actin) and reflected light (for collagen fibrils) 3-D optical section images were acquired simultaneously using laser confocal microscopy (SP2; Leica, Heidelberg, Germany). A HeNe laser (633 nm) was used for reflected light, and an agon laser (488 nm) was used for fluorescent imaging of F-actin. Stacks of optical sections (z-series) were acquired by changing the position of the focal plane in 0.5-μm steps using a 63× water immersion objective (1.2 NA, 220 μm free working distance) or 2-μm steps using a 20× dry objective (0.7 NA, 590 μm free working distance). The same procedure was followed for acellular constructs.

Image Processing and Analysis

F-actin and reflected light images from 63× images were overlaid to show the pattern of cell-induced matrix reorganization. Maximum intensity projections were also generated along the z-axis. The axis of cell alignment and the projected cell length were quantified from 20× maximum intensity projections of F-actin using image analysis software (Metamorph; Molecular Devices, Sunnyvale, CA). Cell length was measured as the span of the longest chord through the cell, as previously described.32,39 This is a measure of cell elongation and polarization along a single axis. Twenty-seven cells were analyzed for each of the six conditions studied (A- and D-zones for UN, PC, and CO constructs).

Fourier transform (FT) analysis was used to assess local collagen fibril alignment. This approach is based on the fact that the relative strength of different angle bands within the FT spectrum is an indicator of the relative number and magnitude of fibers oriented at 90° to that angle in the original image. An “orientation index” (OI) was used to quantify the degree of orientation of the collagen fibrils at each angle (θ). The OI has a value of 100% for fibers oriented parallel to θ, –100% for an orientation perpendicular to θ, and 0% for a completely random distribution.24,32

Collagen Density

The percentage of reflected light confocal images occupied by collagen fibrils was used to quantify collagen fibril density, as previously described.32,39 Images were thresholded manually to segment the collagen fibrils and were binarized. The number of segmented pixels was then measured from the binary image and was expressed as a percentage of the total number of pixels in the original image. All analyses were performed by a masked observer to eliminate subjectivity.

Statistical Analyses

Statistical analyses were performed (SigmaStat, version 3.11; Systat Software Inc., Point Richmond, CA). One- or two-way ANOVA was used to compare group means, and differences were considered significant if P < 0.05.

Results

Cell Morphology

Human corneal fibroblasts seeded within all three models were incubated in S+ media. Cells in the UN model had either a stellate or a bipolar morphology with numerous pseudopodial processes (Fig. 3A). In general, cells seeded within PC and CO models (Figs. 3B, 3C) appeared longer, more polarized, and had fewer pseudopodial processes. Cells in PC and CO matrices were aligned parallel to the x-y plane, whereas cells in UN matrices often had processes that were oriented slightly more obliquely.

Figure 3.

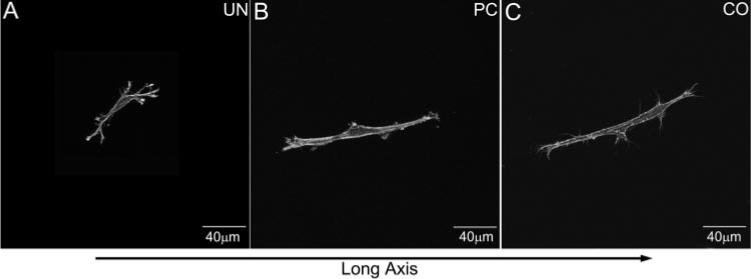
Figure 3

Open in a new tab

Maximum intensity projections demonstrating typical cell morphologies within the A-zone of the three models (UN, PC, CO). The long axis of the construct is horizontal. Note the numerous branching cell processes within the UN model; cells within the PC and CO models are bipolar.

Consistent with our qualitative observations, quantitative analysis demonstrated a projected cell length increase within the A-zone that correlated with the increase in constraint (CO > PC > UN; P < 0.05), indicating increased cell elongation and polarization under these conditions (Fig. 4). Cells within the D-zone were less elongated than those within the A-zone for both the PC and the CO models (P < 0.05).

Figure 4.

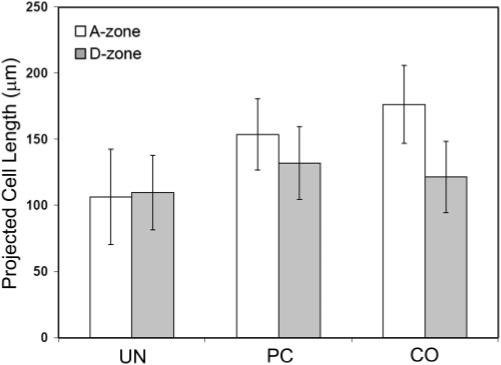
Figure 4

Open in a new tab

Quantitative analysis of projected cell lengths for the three models studied.

Cell Alignment

Cells were aligned nearly parallel to the long axis of the construct in the A-zone of CO and PC matrices, whereas cells in UN matrices appeared randomly oriented (Fig. 5). Quantitative analysis showed significant up-regulation of cell alignment with the long axis of the construct for both the PC and CO models compared with the UN model (Fig. 6). There was no difference between the PC and CO models.

Figure 5.

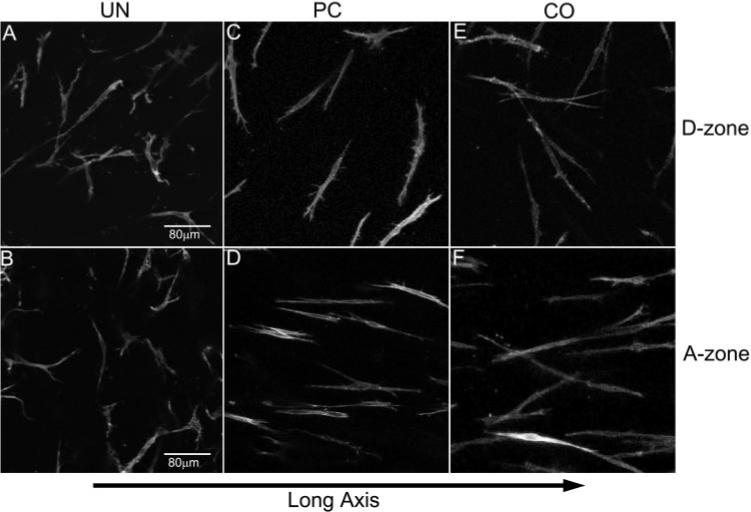
Figure 5

Open in a new tab

Maximum intensity projections showing cell orientation for the three models (UN, PC, CO) within the two different zones (A-zone and D-zone). The long axis of the construct is horizontal. (A, C, E) Randomly oriented cells within the D-zone for all three models. (B, D, F) Cells within the A-zone. Note that cells in the UN model are randomly oriented (B), whereas cells within the PC and CO models (D, F) are aligned parallel to the long axis of the construct.

Figure 6.

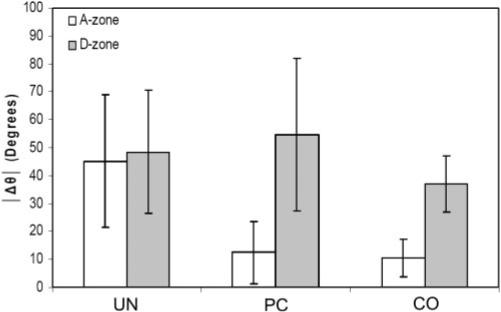
Figure 6

Open in a new tab

Quantitative analysis of cellular alignment for the three models studied. Δθ is the difference between the long axis of the cell and the long axis of the construct (45° = random orientation; 0° = aligned with the long axis).

In contrast, cells in the isotropic D-zone appeared randomly oriented in all three models (Fig. 5), and quantitative analysis showed a significant decrease in cell alignment compared with the A zone in the CO and PC models (Fig. 6). Previous studies have shown a similar pattern of cellular orientation in the PC model using much higher densities30,31,40; however, the UN and CO models have received little attention.

Cell-Induced Matrix Reorganization

Color overlay images revealed differences in matrix reorganization at the ends of cells because of changes in the mechanical environment (Fig. 7). A striking increase in ECM reorganization was observed in the A-zone of PC and CO matrices (Figs. 7D and 7F, Movie 1; movies are online at http://www.iovs.org/cgi/content/full/48/11/5030/DC1) compared with UN matrices (Fig. 7B, Movie 2). Differences were also observed within the D-zone of the different constructs. To quantify the cell-induced changes in the local collagen fibril orientation, FT analysis was used. For each model, 10 to 12 cells were analyzed within each zone. Image subregions at the ends of pseudopodia were used for analysis. Acellular constructs were also analyzed to investigate the potential influence of the construct geometry on the collagen organization. The magnitude of the orientation index (OI) was used to quantify the degree of collagen alignment parallel to the pseudopodial axis (OI = 100% total orientation parallel to a given θ, OI = –100% perpendicular orientation to the given θ, and OI = 0% completely random orientation).

Figure 7.

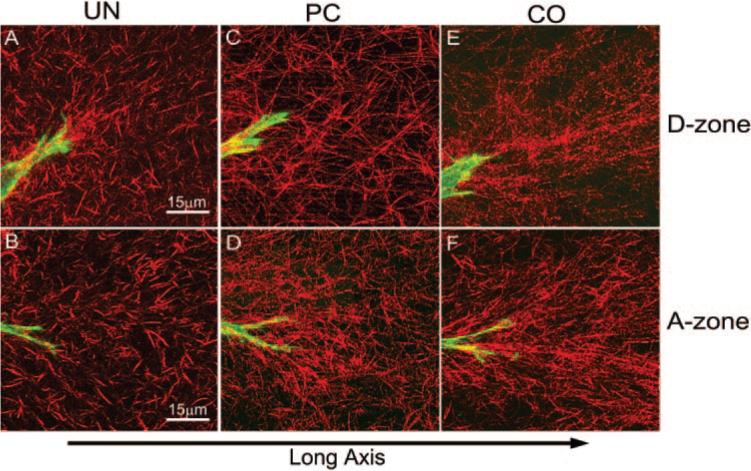
Figure 7

Open in a new tab

Representative images of cell-induced matrix reorganization at the ends of cells. F-actin is shown in green, and collagen is shown in red. (A, C, E) Cell-collagen interactions in the D-zone for UN, PC, and CO, respectively. (B, D, F) Cell–collagen interactions in the A-zone for UN, PC, and CO, respectively. The long axis of the construct is horizontal.

In acellular constructs, no significant differences in the OI were identified between the different constructs and the two (A and D) zones (Fig. 8A). Average OI values were close to zero, indicating that collagen orientation was random when cells were absent. As expected, there was a significant increase in OI in cellular constructs (Fig. 8B). Within the A-zone, we found that increased ECM stiffness resulted in higher coalignment between the cell processes and the collagen fibrils with which they interact (CO > PC > UN; Fig. 8B). Interestingly, OI values within the D-zone were similar to those within the A-zone in the CO and PC matrices. The distribution of tension in the D-zone is isotropic (because of stress shielding), suggesting that coalignment of cells and ECM may be dependent on ECM stiffness and not on ECM anisotropy. Surprisingly, in the UN model, the mean OI was higher in the D-zone than in the A-zone, suggesting increased stiffness in this region, perhaps because of an “edge” effect at the corners of the construct.

Figure 8.

Figure 8

Open in a new tab

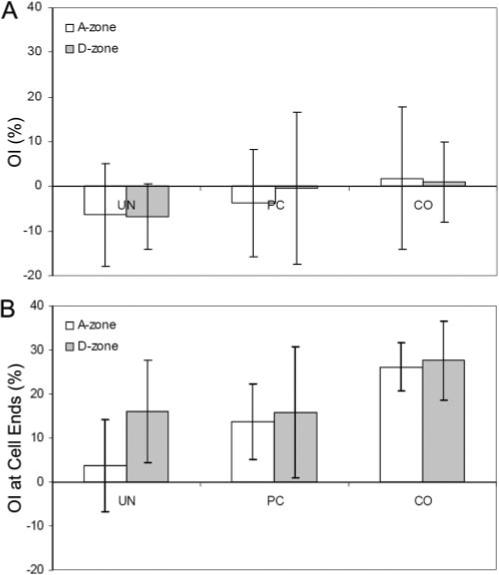
OI results from FT analysis are shown for (A) acellular constructs and (B) cellular constructs. OI values are shown at the cell angle.

Finally, we assessed the relative collagen fibril density in the image subregions at the ends of cells by calculating the area occupied by collagen fibrils in the confocal images. Collagen density in the acellular constructs was generally similar, with the CO having the lowest density (Fig. 9A). As expected, there was a significant increase in collagen density in the cellular constructs for all three models (Fig. 9B). Collagen density at the cell ends within the A-zone was significantly higher when the ECM constraint was increased (CO > PC > UN). In the D-zone, UN values were significantly lower than PC and CO values, but there was no significant difference between PC and CO models.

Figure 9.

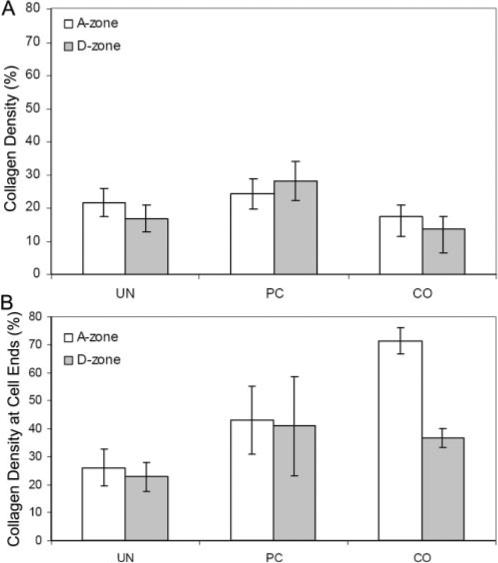
Figure 9

Open in a new tab

Summary of local collagen density measurements for UN, PC, and CO models. (A) Collagen density for acellular constructs. (B) Collagen density for cellular constructs.

To further quantify the differences between the acellular and cellular constructs, we normalized the data by subtracting the mean acellular density values for each model. Figure 10 shows more clearly the increase in collagen density as ECM constraint increases, particularly within the A-zone. Taken together, these data suggest that collagen compaction is influenced by both ECM stiffness and ECM anisotropy in this model.

Figure 10.

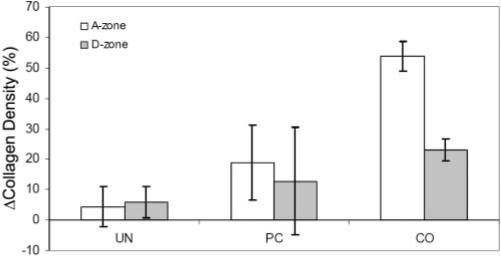
Figure 10

Open in a new tab

Normalized collagen density measurements for UN, PC, and CO models.

Cell–Cell Interactions

Isolated cells were used for our quantitative analyses. However, regions in which cells were in closer proximity were also observed. Interestingly, enhancement of cell-induced collagen reorganization was seen between these cells. For cells aligned in parallel (i.e., end to end), a linear region of compacted collagen was generally observed between the ends of the cells (Fig. 11A, Movie 3). When cells were oriented obliquely to one another, enhancement of collagen reorganization often originated from the side of a pseudopodial process (Fig. 11B, arrow). This sometimes resulted in the formation of compacted bands of collagen at oblique angles from the long axis of the cell (Fig. 11B, arrowhead).

Figure 11.

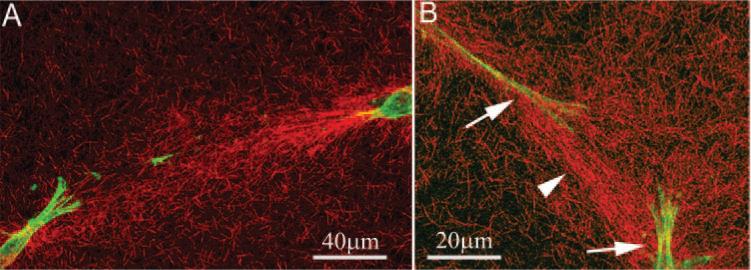
Figure 11

Open in a new tab

Interactions between parallel (A) cells within the A-zone and obliquely (B) aligned cells within the D-zone. (A) Band of highly compacted and aligned collagen between the ends of the two cells. (B) When cells were not aligned in parallel, straps of collagen were often observed originating from the sides of the pseudopodial processes (arrows), resulting in the formation of collagen bands at oblique angles from the long axis of the cells (arrowhead).

Discussion

ECM reorganization by corneal fibroblasts plays a critical role in corneal wound healing and developmental morphogenesis and in stromal tissue engineering. This study is the first, to our knowledge, to directly assess corneal fibroblast responses to ECM anisotropy. Cells showed no preferential alignment in either the A- or the D-zone of the UN construct, which is unconstrained in all directions and should therefore have relatively isotropic mechanical properties. Cells were also randomly oriented within the D-zones of the PC and CO matrices, which are also isotropic because of stress shielding by the plastic bars.30 In contrast, corneal fibroblasts showed alignment nearly parallel to the long axis in the A-zone of the PC and CO matrices. In this region, the effective stiffness in the x-y plane is greater along the long axis than it is along the short axis.15,30 Thus, corneal fibroblasts respond to ECM anisotropy by aligning along the axis of highest mechanical resistance. Similar differences in cell alignment between the A- and D-zones have been shown in PC matrices for human dermal fibroblasts and bone marrow stem cells.15,29,31,40 However, in these studies, cells were plated at a much higher density (1,000,000 cells/mL vs. 24,000 cells/mL). In pilot experiments, when corneal fibroblasts were plated at higher density, a similar pattern of alignment was observed. However, collagen fibrils could not be resolved using reflected light imaging, presumably because of ECM compaction beyond the resolution of the microscope. Given that our goal was to correlate the observed changes in cell alignment with local ECM reorganization, lower cell densities were used for all quantitative analysis in this study.

We used a previously described orientation index to quantify the alignment of collagen fibrils at the ends of cells.32 Within the A-zone, we found that increased ECM stiffness resulted in higher coalignment between the cell processes and the collagen fibrils with which they interact (CO > PC > UN). Collagen density at the ends of cells within the A-zone also increased in parallel with ECM stiffness (CO > PC > UN), confirming greater cell-induced compaction of the ECM. Grinnell et al.22,41 have previously reported that global contraction of attached collagen matrices (CO matrices) is Rho kinase dependent, whereas contraction of free-floating matrices (UN matrices) is not. More recently, we have demonstrated that Rho kinase also plays a central role in regulating local matrix reorganization (i.e., compaction and alignment of collagen fibrils) in attached matrices.32 Taken together, these data suggest that cell alignment and matrix reorganization parallel to the axis of greatest ECM stiffness in CO and PC matrices is also Rho kinase dependent. Cells in the UN matrices were less elongated and had fewer stress fibers and more pseudopodial processes than cells in the PC and CO matrices. Thus, it appears that the lower stiffness in the UN model resulted in a more random pattern of cell spreading, smaller forces, and a reduction in local matrix reorganization and patterning.

Interestingly, the OI values within the D-zone were similar to those within the A-zone in the CO and PC matrices. The distribution of tension in the D-zone is isotropic (because of stress shielding), thus our results suggest that coalignment of cells and ECM may be more dependent on ECM stiffness than it is on ECM anisotropy. In contrast, ECM density was significantly reduced in the D-zone of CO matrices as compared to the A-zone. In the A-zone, collagen can more easily be compacted across the short axis of the construct because it is unconstrained. However, in the D-zone there is resistance to collagen displacement in both directions (long and short axes); thus, overall compaction of collagen is reduced. This is consistent with the “waist” that develops in the A-zone of PC constructs when seeded with a high density of cells.29,31,40,42 Overall, the anisotropic mechanical environment allows for enhancement of collagen compaction along a particular axis through the path of least resistance.

Previous studies have established that cell spreading is enhanced on more rigid 2-D substrates.43 In this study, quantitative analysis demonstrated a projected cell length increase that correlated with the increase in internal stress (higher constraint), indicating a similar enhancement of cell spreading in our 3-D culture model. Because fibroblasts have been shown to spread and migrate preferentially along aligned collagen fibrils,44 there may also be a positive feedback mechanism in CO matrices that leads to enhanced elongation of corneal fibroblasts as the collagen ECM at the ends of the cells becomes more aligned.

Despite the relatively low cell density used in this study, corneal fibroblasts were still able to sense and respond to the differing constraints applied at the ends of the construct. Sawhney et al.45 showed enhanced compression of collagen perpendicular to the axis between two explants of fibroblasts within collagen matrices, leading to alignment of the fibers into a strap. It was proposed that this amplification resulted from the meshlike geometry of the collagen matrix and that analogous amplified movements might have driven morphologic changes in other biological meshes, both outside and inside the cell. Taken together with the data shown in this study, we suggest that the fibrillar structure of 3-D collagen ECMs is a good conduit for cellular force transmission.

Enhancement of cell-induced collagen reorganization was often observed between cells in regions of higher cell density. For cells aligned in parallel, this enhancement occurred between the ends of the two cells. However, when cells were not aligned in parallel, compacted bands of collagen often originated from the base of a pseudopodial process and extended at oblique angles from the cells. In previous studies using non-confocal imaging, we have demonstrated that extension of pseudopodial processes can produce ECM tension in front of the cell (because of pulling in of the collagen fibrils) and ECM compression at the base of pseudopodia (because of cellular contraction).46,47 The two patterns of ECM organization observed in this study are entirely consistent with those observations. Overall, our data demonstrate that isolated corneal fibroblasts generally align and compact collagen parallel to the axis of greatest stiffness within anisotropic matrices but that mechanical cross-talk between cells at higher density can result in additional, more complex matrix patterning.

Acknowledgments

The authors thank Dwight Cavanagh and Fred Grinnell for their helpful comments and suggestions.

Supported in part by National Institutes of Health Grant EY 13322, Infrastructure Grant EY 16664, and by an unrestricted grant and a Lew R. Wasserman Merit Award (WMP) from Research to Prevent Blindness.

Footnotes

Disclosure: D. Karamichos, None; N. Lakshman, None; W.M. Petroll, None

The publication costs of this article were defrayed in part by page charge payment. This article must therefore be marked “advertisement” in accordance with 18 U.S.C. §1734 solely to indicate this fact.

References

  • 1.Bard JBL, Hay ED. The behavior of fibroblasts from the developing avian cornea: morphology and movement in situ and in vitro. J Cell Biol. 1975;67:400–418. doi: 10.1083/jcb.67.2.400. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 2.Cintron C, Covington H, Kublin CL. Morphogenesis of rabbit corneal stroma. Invest Ophthalmol Vis Sci. 1983;24:543–556. [PubMed] [Google Scholar]
  • 3.Hay ED. The mesenchymal cell, its role in the embryo, and the remarkable signaling mechanisms that create it. Dev Dyn. 2005;233:706–720. doi: 10.1002/dvdy.20345. [DOI] [PubMed] [Google Scholar]
  • 4.Maurice DM. The structure and transparency of the cornea. J Physiol. 1957;136:263–286. doi: 10.1113/jphysiol.1957.sp005758. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 5.Trelstad RL, Coulombre AJ. Morphogenesis of the collagenous stroma in the chick cornea. J Cell Biol. 1971;50:840–858. doi: 10.1083/jcb.50.3.840. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 6.Doillon DJ, Watsky MA, Hakim M, et al. A collagen-based scaffold for a tissue engineered human cornea: physical and physiological properties. Int J Artif Organs. 2003;26:764–773. doi: 10.1177/039139880302600810. [DOI] [PubMed] [Google Scholar]
  • 7.Griffith M, Osborne R, Munger R, et al. Functional human corneal equivalents constructed from cell lines. Science. 1999;286:2169–2172. doi: 10.1126/science.286.5447.2169. [DOI] [PubMed] [Google Scholar]
  • 8.Alaminos M, Sanchez-Quevedo MDC, Munoz-Avila JI, et al. Construction of a complete rabbit cornea substitute using a fibrinagarose scaffold. Invest Ophthalmol Vis Sci. 2006;47:3311–3317. doi: 10.1167/iovs.05-1647. [DOI] [PubMed] [Google Scholar]
  • 9.Han B, Schwab IR, Madsen TK, Isseroff RR. A fibrin-based bioengineered ocular surface with human corneal epithelial stem cells. Cornea. 2002;21:505–510. doi: 10.1097/00003226-200207000-00013. [DOI] [PubMed] [Google Scholar]
  • 10.Talbot M, Carrier P, Glasson CJ, et al. Autologous transplantation of rabbit limbal epithelia cultured on fibrin gels for ocular surface reconstruction. Mol Vis. 2006;12:65–75. [PubMed] [Google Scholar]
  • 11.Petroll WM, New K, Sachdev M, Cavanagh HD, Jester JV. Radial keratotomy, III: relationship between wound gape and corneal curvature in primate eyes. Invest Ophthalmol Vis Sci. 1992;33:3283–3291. [PubMed] [Google Scholar]
  • 12.Jester JV, Petroll WM, Cavanagh HD. Corneal stromal wound healing in refractive surgery: the role of the myofibroblast. Prog Retinal Eye Res. 1999;18:311–356. doi: 10.1016/s1350-9462(98)00021-4. [DOI] [PubMed] [Google Scholar]
  • 13.Moller-Pedersen T, Vogel MD, Li H, Petroll WM, Cavanagh HD, Jester JV. Quantification of stromal thinning, epithelial thickness, and corneal haze following photorefractive keratectomy using in vivo confocal microscopy. Ophthalmology. 1997;104:360–368. doi: 10.1016/s0161-6420(97)30307-8. [DOI] [PubMed] [Google Scholar]
  • 14.Brown TD. Techniques for mechanical stimulation of cells in vitro: a review. J Biomech. 2000;33:3–14. doi: 10.1016/s0021-9290(99)00177-3. [DOI] [PubMed] [Google Scholar]
  • 15.Karamichos D, Brown RA, Mudera V. Complex dependence of substrate stiffness and serum concentration on cell-force generation. J Biomed Mater Res A. 2006;78:407–415. doi: 10.1002/jbm.a.30814. [DOI] [PubMed] [Google Scholar]
  • 16.Liu M, Tanswell AK, Post M. Mechanical force-induced signal transduction in lung cells. Am J Physiol. 1999;277:L667–L683. doi: 10.1152/ajplung.1999.277.4.L667. [DOI] [PubMed] [Google Scholar]
  • 17.Sadoshima J, Izumo S. The cellular and molecular response of cardiac myocytes to mechanical stress. Annu Rev Physiol. 1997;59:551–571. doi: 10.1146/annurev.physiol.59.1.551. [DOI] [PubMed] [Google Scholar]
  • 18.Shyy JY-J, Chien S. Role of integrins in endothelial mechanosensing of shear stress. Circ Res. 2002;91:769–775. doi: 10.1161/01.res.0000038487.19924.18. [DOI] [PubMed] [Google Scholar]
  • 19.Tummina SJ, Mitton KP, Arora J, Zelenka P, Epstein DL, Russell P. Mechanical stretch alters the actin cytoskeletal network and signal transduction in human trabecular meshwork cells. Invest Ophthalmol Vis Sci. 1998;39:1361–1371. [PubMed] [Google Scholar]
  • 20.Burridge K, Chrzanowska-Wodnicka C. Focal adhesions, contractility, and signaling. Ann Rev Cell Dev Biol. 1996;12:463–519. doi: 10.1146/annurev.cellbio.12.1.463. [DOI] [PubMed] [Google Scholar]
  • 21.Riveline D, Zamir E, Balaban NQ, et al. Focal contacts as mechanosensors: externally applied local mechanical force induces growth of focal contacts by an mDia1-dependent and ROCK-independent mechanism. J Cell Biol. 2001;153:1175–1185. doi: 10.1083/jcb.153.6.1175. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 22.Tamariz E, Grinnel F. Modulation of fibroblast morphology and adhesion during collagen matrix remodeling. Mol Biol Cell. 2002;13:3915–3929. doi: 10.1091/mbc.E02-05-0291. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 23.Kolodney MS, Wysolmerski RB. Isometric contraction by fibroblasts and endothelial cells in tissue culture: a quantitative study. J Cell Biol. 1992;117:73–82. doi: 10.1083/jcb.117.1.73. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 24.Petroll WM, Cavanagh HD, Barry-Lane P, Andrews P, Jester JV. Quantitative analysis of stress fiber orientation during corneal wound contraction. J Cell Sci. 1993;104:353–363. doi: 10.1242/jcs.104.2.353. [DOI] [PubMed] [Google Scholar]
  • 25.Takakuda K, Miyairi H. Tensile behavior of fibroblasts cultured in collagen gel. Biomaterials. 1996;17:1393–1397. doi: 10.1016/0142-9612(96)87280-2. [DOI] [PubMed] [Google Scholar]
  • 26.Wakatsuki T, Elson EL. Reciprocal interactions between cells and extracellular matrix during remodeling of tissue constructs. Biophys Chem. 2003;100:593–605. doi: 10.1016/s0301-4622(02)00308-3. [DOI] [PubMed] [Google Scholar]
  • 27.Huang D, Chang TR, Aggarwal A, Lee RC, Ehrlich HP. Mechanisms and dynamics of mechanical strengthening in ligament-equivalent fibroblast populated collagen matrices. Ann Biomed Eng. 1993;21:289–305. doi: 10.1007/BF02368184. [DOI] [PubMed] [Google Scholar]
  • 28.Cheema U, Yang SY, Mudera V, Goldspink GG, Brown RA. 3-D in vitro model of early skeletal muscle development. Cell Motil Cytoskeleton. 2003;54:226–236. doi: 10.1002/cm.10095. [DOI] [PubMed] [Google Scholar]
  • 29.Eastwood M, McGrouther DA, Brown RA. A culture force monitor for measurement of contraction forces generated in human dermal fibroblast cultures: evidence for cell matrix mechanical signalling. Biochim Biophys Acta. 1994;1201:186–192. doi: 10.1016/0304-4165(94)90040-x. [DOI] [PubMed] [Google Scholar]
  • 30.Eastwood M, Mudera VC, McGrouther DA, Brown RA. Effect of precise mechanical loading on fibroblast populated collagen lattices: morphological changes. Cell Motil Cytoskeleton. 1998;40:13–21. doi: 10.1002/(SICI)1097-0169(1998)40:1<13::AID-CM2>3.0.CO;2-G. [DOI] [PubMed] [Google Scholar]
  • 31.Mudera VC, Pleass R, Eastwood M, et al. Molecular responses of human dermal fibroblasts to dual cues: contact guidance and mechanical load. Cell Motil Cytoskeleton. 2000;45:1–9. doi: 10.1002/(SICI)1097-0169(200001)45:1<1::AID-CM1>3.0.CO;2-J. [DOI] [PubMed] [Google Scholar]
  • 32.Kim A, Lakshman N, Petroll WM. Quantitative assessment of local collagen matrix remodeling in 3-D culture: the role of Rho kinase. Exp Cell Res. 2006;312:3683–3692. doi: 10.1016/j.yexcr.2006.08.009. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 33.Petroll WM. Differential interference contrast and confocal reflection imaging of collagen organization in 3-dimensional matrices. Scanning. 2006;28:305–310. doi: 10.1002/sca.4950280602. [DOI] [PubMed] [Google Scholar]
  • 34.Petroll WM, Cavanagh HD, Jester JV. Dynamic three-dimensional visualization of collagen matrix remodeling and cytoskeletal organization in living corneal fibroblasts. Scanning. 2004;26:1–10. doi: 10.1002/sca.4950260102. [DOI] [PubMed] [Google Scholar]
  • 35.Friedl P, Noble PB, Zanker KS. T lymphocyte locomotion in a three-dimensional collagen matrix. J Immunol. 1995;154:4973–4985. [PubMed] [Google Scholar]
  • 36.Friedl P, Maaser K, Klein CE, Niggemann B, Krohne G, Zanker KS. Migration of highly aggressive MV3 melanoma cells in 3-dimensional collagen lattices results in local matrix reorganization and shedding of alpha2 and beta1 integrins and CD44. Cancer Res. 1997;57:2061–2070. [PubMed] [Google Scholar]
  • 37.Hegerfeldt Y, Tusch M, Brocker E- B, Friedl P. Collective cell movement in primary melanoma explants: plasticity of cell-cell interaction, b1-integrin function, and migration strategies. Cancer Res. 2002;62:2125–2130. [PubMed] [Google Scholar]
  • 38.Jester JV, Huang J, Fisher S, et al. Myofibroblast differentiation of normal human keratocytes and hTERT, extended-life, human corneal fibroblasts. Invest Ophthalmol Vis Sci. 2003;44:1850–1858. doi: 10.1167/iovs.02-0973. [DOI] [PubMed] [Google Scholar]
  • 39.Lakshman N, Kim A, Bayless KJ, Davis GE, Petroll WM. Rho plays a central role in regulating local cell-matrix mechanical interactions in 3D culture. Cell Motil Cytoskeleton. 2007;64:434–445. doi: 10.1002/cm.20194. [DOI] [PubMed] [Google Scholar]
  • 40.Brown RA, Prajapati R, McGrouther DA, Yannas IV, Eastwood M. Tensional homeostasis in dermal fibroblasts: mechanical responses to mechanical loading in three-dimensional substrates. J Cell Physiol. 1998;175:323–332. doi: 10.1002/(SICI)1097-4652(199806)175:3<323::AID-JCP10>3.0.CO;2-6. [DOI] [PubMed] [Google Scholar]
  • 41.Grinnell F. Fibroblast-collagen matrix contraction: growth-factor signalling and mechanical loading. Trends Cell Biol. 2000;10:362–365. doi: 10.1016/s0962-8924(00)01802-x. [DOI] [PubMed] [Google Scholar]
  • 42.Eastwood M, Porter RA, Kahn U, McGrouther DA, Brown RA. Quantitative analysis of collagen gel contractile forces generated by dermal fibroblasts and the relationship to cell morphology. J Cell Physiol. 1996;166:33–42. doi: 10.1002/(SICI)1097-4652(199601)166:1<33::AID-JCP4>3.0.CO;2-H. [DOI] [PubMed] [Google Scholar]
  • 43.Lo CM, Wang HB, Dembo M, Wang YL. Cell movement is guided by the rigidity of the substrate. Biophys J. 2000;79:144–152. doi: 10.1016/S0006-3495(00)76279-5. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 44.Poole K, Khairy K, Friedrichs J, et al. Molecular-scale topographic cues induce the orientation and directional movement of fibroblasts on two-dimensional collagen surfaces. J Mol Biol. 2005;349:380–386. doi: 10.1016/j.jmb.2005.03.064. [DOI] [PubMed] [Google Scholar]
  • 45.Sawhney RK, Howard J. Slow local movements of collagen fibers by fibroblasts drive the rapid global self-organization of collagen gels. J Cell Biol. 2002;157:1083–1091. doi: 10.1083/jcb.200203069. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 46.Petroll WM, Ma L. Direct, dynamic assessment of cell-matrix interactions inside fibrillar collagen lattices. Cell Motil Cytoskeleton. 2003;55:254–264. doi: 10.1002/cm.10126. [DOI] [PubMed] [Google Scholar]
  • 47.Petroll WM, Ma L, Jester JV. Direct correlation of collagen matrix deformation with focal adhesion dynamics in living corneal fibroblasts. J Cell Sci. 2003;116:1481–1491. doi: 10.1242/jcs.00357. [DOI] [PubMed] [Google Scholar]

RESOURCES