Simulation of Ca-Calmodulin-Dependent Protein Kinase II on Rabbit Ventricular Myocyte Ion Currents and Action Potentials

Abstract

Ca-calmodulin-dependent protein kinase II (CaMKII) was recently shown to alter Na⁺ channel gating and recapitulate a human Na⁺ channel genetic mutation that causes an unusual combined arrhythmogenic phenotype in patients: simultaneous long QT syndrome and Brugada syndrome. CaMKII is upregulated in heart failure where arrhythmias are common, and CaMKII inhibition can reduce arrhythmias. Thus, CaMKII-dependent channel modulation may contribute to acquired arrhythmic disease. We developed a Markovian Na⁺ channel model including CaMKII-dependent changes, and incorporated it into a comprehensive myocyte action potential (AP) model with Na⁺ and Ca²⁺ transport. CaMKII shifts Na⁺ current (I_Na) availability to more negative voltage, enhances intermediate inactivation, and slows recovery from inactivation (all loss-of-function effects), but also enhances late noninactivating I_Na (gain of function). At slow heart rates, with long diastolic time for I_Na recovery, late I_Na is the predominant effect, leading to AP prolongation (long QT syndrome). At fast heart rates, where recovery time is limited and APs are shorter, there is little effect on AP duration, but reduced availability decreases I_Na, AP upstroke velocity, and conduction (Brugada syndrome). CaMKII also increases cardiac Ca²⁺ and K⁺ currents (I_Ca and I_to), complicating CaMKII-dependent AP changes. Incorporating I_Ca and I_to effects individually prolongs and shortens AP duration. Combining I_Na, I_Ca, and I_to effects results in shortening of AP duration with CaMKII. With transmural heterogeneity of I_to and I_to downregulation in heart failure, CaMKII may accentuate dispersion of repolarization. This provides a useful initial framework to consider pathways by which CaMKII may contribute to arrhythmogenesis.

INTRODUCTION

Cardiac sodium (Na⁺) channels control cardiac excitability and the velocity of impulse propagation by initiating the action potential (AP). Different disorders in heart excitability have been related to derangement of the cardiac Na⁺ channel (1). A number of inherited diseases associated with mutations in SCN5A, the gene encoding the α-subunit of the cardiac Na⁺ channel, have been discovered and linked to long QT type 3 (LQT3) and Brugada (BrS) syndromes, conduction diseases, and structural defects. Notably, mutations showing overlapping phenotypes have been characterized. A single human mutation (1795InsD) in SCN5A is linked to simultaneous LQT3 and BrS features. This 1795InsD mutation in humans has been studied in expressed Na⁺ channels (2). The mutant Na⁺ current (I_Na) exhibits increased intermediate inactivation and slowed recovery from inactivation, and the mutant Na⁺ channel availability is shifted to more negative potentials, all of which would reduce I_Na (loss of function) and could explain Brugada-like symptoms in patients at faster heart rates. In addition, this 1795InsD mutation causes persistent I_Na, which fails to completely inactivate during long-duration APs. The persistent I_Na would produce a gain of function, and that could contribute to AP prolongation and LQT syndrome. This would be especially pronounced at slow heart rates where I_Na recovery from inactivation may be more complete (even with the mutation) and where the longer intrinsic AP duration (APD) would increase the impact of the late I_Na.

Na⁺ channel gating may also be altered in acquired diseases, e.g., drug-induced LQT syndrome, cardiac ischemia, and heart failure (HF). In HF, altered Na⁺ channel regulation could cause widespread acquired Na⁺ channel dysfunction and arrhythmogenesis. For example, Ca-calmodulin-dependent protein kinase II (CaMKII) is upregulated and also more active in HF (3). Wagner et al. (4) recently showed that CaMKII alters cardiac myocyte Na⁺ channel gating in a manner very similar to the combined LQT/Brugada genetic phenotype mentioned above (1795InsD). Adenovirus-mediated (acute) and transgenic (chronic) overexpression of the cytosolic δ isoform (CaMKIIδc) shifted voltage dependence of Na⁺ channel availability to more negative membrane potentials, enhanced intermediate inactivation, and slowed recovery from inactivation (and these effects were prevented by CaMKII inhibitors). In addition, CaMKIIδc markedly increased persistent (late) inward I_Na and intracellular [Na⁺] ([Na⁺]_i). From these alterations, one might expect less Na⁺ channel availability at fast heart rates but more inward I_Na during long APs at slow heart rates.

The increased levels of CaMKII in HF may target several myocyte proteins (5). CaMKII phosphorylates Ca²⁺ transport proteins such as phospholamban (6), ryanodine receptors (7–9), and L-type Ca²⁺ channels (8,10,11). In addition, CaMKII can also modulate K⁺ channels (12–15). CaMKII is known to mediate Ca²⁺-dependent I_Ca facilitation (8,10,11), and Kohlhaas et al. (8) showed that CaMKII overexpression in rabbit myocytes increases peak I_Ca and slows inactivation (possibly related to I_Ca facilitation). CaMKII can also modulate transient outward K⁺ current (I_to). Wagner et al. (15) showed that upon CaMKII overexpression, the expression levels of K_v1.4 channels (responsible for the slow component of I_to) are enhanced, as is the current recovery from inactivation. Given the multiple CaMKII targets, it is difficult a priori to discern the relative effects of CaMKII modulation of I_Na, I_Ca, and I_to on ventricular myocyte APs. In this study, we simulate the CaMKII-induced alterations of I_Na, I_Ca, and I_to (individually and collectively) on the rabbit ventricular AP.

Mathematical models have been widely used to simulate the voltage-dependent gating of ion channels. Composite models of ion channels and excitation-contraction coupling (ECC) allow investigation of the effects of altered Na⁺ channel kinetic properties on myocyte electrical activity (16–18). Clancy and Rudy (16) showed that a theoretical AP model could simulate the 1795InsD mutant current behavior by including an extended formulation of their Markovian Na⁺ channel structure that incorporates a bursting mode. Here, we used a variation of this Markovian Na⁺ channel model to simulate the CaMKIIδc effects on I_Na reported by Wagner et al. (4) and we incorporate this into a comprehensive AP and ECC model of rabbit ventricular myocytes (19). We also incorporated CaMKII-dependent effects on I_Ca and I_to in rabbit ventricular myocytes to distinguish the independent and combined effects of these CaMKII targets on APD.

METHODS

Na⁺ current model simulation

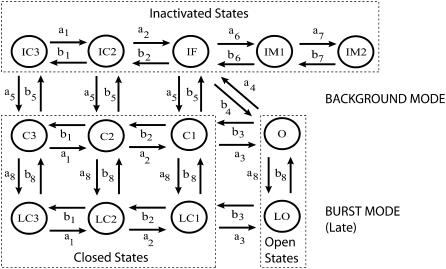
The Markov model structure (Fig. 1) implemented in this study is based on that proposed by Clancy and Rudy (16,17). It contains two modes of gating, a background mode and a burst mode. The background mode reflects the normal sequence of activation and inactivation that most channels undergo upon depolarization and contributes predominantly to the large transient component of I_Na. The burst mode reflects a small population of channels that transiently fail to inactivate during the AP plateau and results in a sustained inward current. The background mode includes the nine states in the upper two rows, consisting of three closed states (C3, C2, and C1), a conducting open state (O), a fast inactivation state (IF), and two intermediate inactivation states (IM1 and IM2) that are required to reproduce the complex fast and slow recovery features of I_Na inactivation. The lower four states in Fig. 1 correspond to the burst or late mode (prefix L), in which inactivation does not occur. Voltage (V_m)-independent transition rates between upper and lower states represent a probability of transition between the two modes of gating.

FIGURE 1.

Markov model of the cardiac Na⁺ channel. The channel model contains background (upper nine states) and burst (lower four states) gating modes. The burst mode reflects a population of channels that transiently fail to inactivate and accounts for a sustained inward current (or late current).

Table 1 shows the expressions of the transition rates between the Markov model states. The corresponding parameters were identified by a fitting procedure to simulate the electrophysiological characterization of cardiac I_Na in βGal and in CaMKIIδc-overexpressing rabbit myocytes reported by Wagner et al. (4). The following voltage-clamp protocols were simulated for parameter identification: activation, steady-state inactivation, intermediate inactivation, recovery from inactivation, and long-depolarization voltage step to assess late I_Na. The model results for each protocol were compared with experimental data. The transition rate parameters that maximally influence each voltage-clamp protocol were identified with an automatic procedure. Namely, the ratio a₈/b₈ was modified to reproduce the experimental data accounting for late I_Na; a₅/b₅ was tuned to correctly simulate the channel availability curve; a₆, b₆ and a₇, b₇ were adjusted to represent the observed characteristics of intermediate inactivation and recovery from inactivation. The Nelder-Mead simplex direct algorithm was used to find the parameter values minimizing the sum of the least-square errors between model predictions and experimental data for each protocol. The values proposed by Clancy and Rudy (16) were chosen as initial guesses in the minimization procedure to identify the transition rate parameters that allowed the best fitting of our βGal data. The identified βGal set in Table 2 was subsequently used as an initial guess to identify the CaMKIIδc channel parameters. The parameters that influence more than one protocol were then manually tuned to optimize fitting to all the experimental data. A full list of the parameters is given in Table 2. Matlab 7 and Simulink (The MathWorks, Natick, MA) were used for all numerical computations.

TABLE 1.

Transition rate expressions (ms⁻¹)

Transition rates
a1 = (P1a1/(P2a1 exp(−Vm/17) + 0.20 exp(−Vm/150)))
a2 = (P1a1/(P2a1 exp(−Vm/15) + 0.23 exp(−Vm/150)))
a3 = (P1a1/(P2a1 exp(−Vm/12) + 0.25 exp(−Vm/150)))
b1 = P1b1 exp(−Vm/P2b1)
b2 = P1b2 exp(− (Vm − P2b2)/(P2b1))
b3 = (P1b3 exp(− (Vm − P2b3)/(P2b1)))
a5 = P1a5 exp(−Vm/P2a5)
b5 = (P1b5 + P2b5Vm)
a4 = (P1a4 exp(Vm/P2a4))
b4 = (a3a4a5)/(b3b5)
a6 = a4/P1a6
b6 = P1b6 exp(−Vm/P2b6)
a7 = (P1a7 exp(Vm/P2a7))
b7 = P1b7 exp(−Vm/P2b7)
a8 = P1a8
b8 = P1b8

TABLE 2.

Na⁺ channel model parameters for βGal and CaMKII channels

Parameters	βGal	CaMKII
P1a1	3.802
P2a1	0.1027
P1a4	9.178
P2a4	25
P1a5	3.7933e−7
P2a5	7.7
P1b1	0.1917
P2b1	20.3
P1b2	0.2
P2b2	5
P1b3	0.22
P2b3	10
P1b5	0.0042	0.0067
P2b5	2e−6
P1a6	100
P1b6	8.8061e−7	7.0449e−7
P2b6	11.3944
P1a7	0.3543e−3	0.6377e−3
P2a7	23.2696
P1b7	0.2868e−3
P2b7	35.9898
P1a8	4.7e−7	11.75e−7
P1b8	9.5e−4

L-type Ca²⁺ current simulation

The L-type Ca²⁺ current formulation of Shannon et al. (19) was modified to reproduce the effects of CaMKII (8) by increasing the channel maximal conductance by 10% and mimicking Ca²⁺-dependent facilitation with an additional term that makes the Ca²⁺-dependent inactivation less complete.

In βGal-overexpressing cardiomyocytes, Ca²⁺-dependent inactivation (local K_d(Ca) = 7 μM) is modeled by using the variable f_Ca, in which dynamic changes are described as

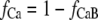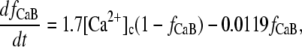where [Ca²⁺]_c is the [Ca²⁺] in the actual compartment (either junctional or subsarcolemmal space, in mM).

To account for CaMKII overexpression, the Ca²⁺-dependent inactivation variable becomes

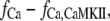where

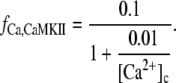Transient outward K⁺ current simulation

The formulations of the slow and fast component of I_to were adapted from Bassani et al. (20) to account for the increased expression levels of K_v1.4 channels and for the enhanced recovery from inactivation in CaMKII-overexpressing rabbit myocytes (15). To this purpose, maximal conductance of the slow component G_to,slow was increased by 50% in the CaMKII model and the time constants of I_to,slow inactivation were modified as follows:

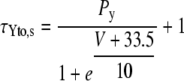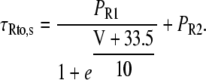Values of P_y, P_R1, and P_R2 are (in ms) 182, 8085, and 131, respectively, for βGal and 15, 3600, and 500, respectively, for CaMKII-overexpressing cardiomyocytes.

Action potential simulation

The ventricular AP was simulated using the Chicago model of rabbit ventricular myocyte (19), which includes both a cleft space (between sarcoplasmic reticulum (SR) and sarcolemmal membrane) and a separate subsarcolemmal compartment (where sarcolemmal ion channels and transporters sense higher [Ca²⁺]_i than in the bulk cytosol). The model was implemented in Matlab 7 (The MathWorks, Natick, MA). The model was adjusted to correctly describe the ratio between slow and fast components of I_to to reproduce the experimental APD adaptation to the pacing rate observed in rabbit myocytes (21). For this purpose, the conductances of I_to,fast and I_to,slow were set to 0.02 and 0.06 mS/μF, respectively.

The I_Na model was replaced with the above Markov model. Maximal conductance G_Na at 37°C was calculated as 23 mS/μF for both βGal and CaMKII (Q₁₀ = 1.5 for Na⁺ channel conductance (22)). The kinetic rates were normalized to 37°C with a Q₁₀ of 2.1 (22). I_Ca was similarly scaled up to 37°C, using our previous measurements in rabbit ventricular myocytes at 25°C and 35°C (23). Total I_to density was lower in rabbit ventricular myocytes expressing βGal after 24–36 h in culture (15), compared to freshly isolated myocytes (20). To better reflect the physiological situation for AP simulations, we used fresh myocyte I_to amplitudes (I_to,fast and I_to,slow) for control and relative changes caused by CaMKII versus βGal overexpression to simulate the CaMKII case. Pacing was simulated by a current pulse train (pulses of 5 ms in duration) of 9.5 A/F in amplitude with different frequencies (0.1, 0.25, 0.5, 1, 2, and 3 Hz). A variable order solver based on the numerical differentiation formulas was used to numerically solve the model equations (ode15s) (24). The stimulation was maintained until a steady AP was reached (typically ∼100 s).

RESULTS

Na⁺ current simulation

Steady-state inactivation and activation

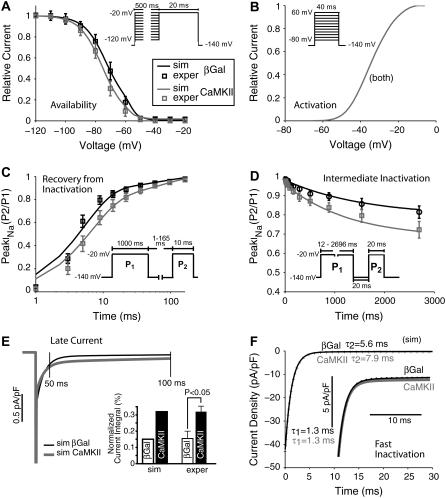
Fig. 2 shows experimental data (7) and simulations, which confirm the adequacy of the Na⁺ channel Markovian model. Table 3 shows sources of experimental data used for validation of the simulations here. To assess the voltage dependence of steady-state inactivation (or availability), I_Na was measured during test pulses to −20 mV (20 ms) after 500-ms prepulses from −140 mV to various V_m (with 10-mV increments). As in the experimental data, CaMKIIδc overexpression shifts inactivation to more negative potentials with respect to βGal (Fig. 2 A).

FIGURE 2.

Experimental (squares) and simulated (solid lines) voltage-clamp protocols for βGal- (black) and CaMKII-modulated (gray) Na⁺ channels (data at 140 mM external Na⁺ concentration). (A) Steady-state inactivation. CaMKIIδc overexpression shifts the availability curve toward negative potentials. (B) Activation. Na⁺ channel activation is not affected by CaMKIIδc overexpression (traces are superimposed). (C and D) CaMKIIδc slows the recovery from inactivation (C) and enhances the intermediate inactivation (D). (E) Late I_Na is enhanced by CaMKIIδc overexpression. Simulated traces are shown at left, and bar graphs of simulated and experimental normalized current integrals at right. (F) Fast and slow time constants of I_Na decay. Acute CaMKIIδc overexpression slows the late component of fast I_Na inactivation. Experimental data are from Wagner et al. (15).

TABLE 3.

CaMKII overexpression data

Figure	Measure	Source	Experiment
Fig. 2	INa	Wagner et al. (4)	Rabbit myocyte, 22°C, ruptured patch clamp
Fig. 4	[Na+]i	Wagner et al. (4)	Rabbit myocyte, 22°C, ruptured patch clamp
Fig. 5	ICa	Kohlhaas et al. (8)	Rabbit myocyte, 22°C, ruptured patch clamp
Fig. 6	Ito	Wagner et al. (15)	Rabbit myocyte, 22°C, ruptured patch clamp
Fig. 8	AP	Wagner et al. (15)	Rabbit myocyte, 22°C, ruptured patch clamp

Steady-state activation was not altered by CaMKIIδc in the experimental data (4). This was assessed by 40-ms depolarizations from a holding potential of −140 mV to −80/+60 mV (with 10-mV increments). Relative conductance was taken by dividing peak I_Na by the driving force (V_m − E_Na). The resulting conductance was normalized to the maximal chord conductance. As in the experimental data, simulated CaMKIIδc overexpression does not affect I_Na activation with respect to βGal (Fig. 2 B; note that βGal and CaMKIIδc traces are superimposed).

Recovery from inactivation and intermediate inactivation

Recovery from inactivation was investigated using a 1000-ms depolarization to −20 mV, which initiates fast and intermediate inactivation (P₁), followed by a recovery interval of variable duration and a subsequent test pulse (P₂). CaMKIIδc slows recovery from inactivation in our simulations, as well as in the experimental results (Fig. 2 C). Accumulation of intermediate inactivation was assessed using depolarizations of variable duration (P₁) followed by a 20-ms recovery period at −140 mV (which allows channel recovery from fast inactivation). Na⁺ channels that have undergone intermediate inactivation would still be largely unavailable, causing a reduction in I_Na at the subsequent test pulse P₂ to −20 mV. In the computer simulations, as in the experimental recordings, intermediate inactivation is enhanced by CaMKIIδc (Fig. 2 D).

Late current

CaMKIIδc enhances late I_Na. Currents were elicited by 1000-ms depolarizations to −20 mV (from −140 mV), and normalized to peak I_Na. The current integral was calculated between 50 and 500 ms (Fig. 2 E) and plotted relative to the I_Na integral if no inactivation had occurred (i.e., peak current × 450 ms). As shown in Fig. 2 E, compared to control, simulated CaMKIIδc overexpression resulted in a larger late I_Na. These results fit the experimental data (4) shown for comparison in Fig. 2 E.

Fast inactivation

CaMKIIδc slows fast Na⁺ channel inactivation in rabbit CaMKIIδc myocytes compared to βGal (Fig. 2 F). The Na⁺ current decay (first 50 ms) was fitted with a double exponential function. Fits (dotted lines) to the simulated traces (solid lines) and corresponding parameters τ₁ and τ₂ (ms) are shown in black (βGal) and gray (CaMKIIδc). The deceleration of current decay was most prominent in the late component (at right in Fig. 2 F), so that τ₂ was greater compared to control (7.8 vs. 5.6 ms), whereas τ₁ appeared to be unchanged (1.3 ms), in agreement with Wagner et al. (4).

CaMKII-dependent Na⁺ current alterations on AP and [Na⁺]_i

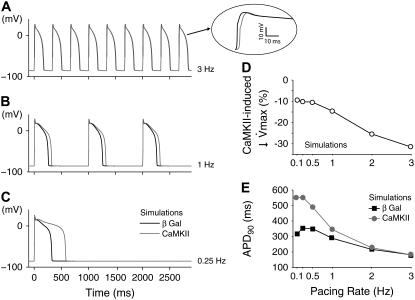
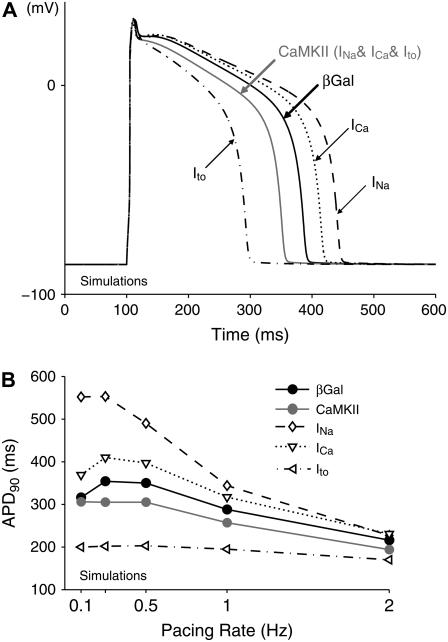
The simulations of the effect of the CaMKII-dependent Na⁺ channel kinetic alterations on ventricular AP are shown in Fig. 3 for three pacing rates (3, 1, and 0.25 Hz). Na⁺ channel modulation did not change overall AP morphology with respect to βGal. The βGal AP (black line) exhibits a characteristic AP shape and its duration (185, 288, and 354 ms) shows the typical rabbit APD dependence on pacing frequency (Fig. 3 E, black symbols). CaMKII APs (gray line) exhibit distinctive rate-dependent durations (Fig. 3 E, gray symbols). At fast rates (3 Hz), the CaMKII AP is grossly superimposable on the control AP (Fig. 3 A, APD₉₀ = 188 ms). However, the short recovery interval limits Na⁺ channel recovery in the CaMKII case, resulting in reduced I_Na amplitude, slight decrease in AP peak, and reduced AP upstroke velocity (maximum dV_m/dt; see Fig. 3 A, inset). At low frequencies, AP upstroke velocity is reduced by ∼10% by the CaMKII effect, but this decrease becomes more prominent at higher frequencies (Fig. 3 D). This Na⁺ channel loss of function would reduce AP rate of rise and conduction velocity, especially at fast heart rates. At lower rates (Fig. 3, B and C), APD is significantly prolonged, especially at the slowest rates (APD₉₀ = 344 ms at 1 Hz and APD₉₀ = 553 ms at 0.25 Hz). At these low frequencies, CaMKII-dependent impaired fast inactivation and enhanced late I_Na outweigh the slowed recovery from inactivation (because the long diastolic interval allows more complete Na⁺ channel recovery). Thus, CaMKIIδc-induced AP prolongation is especially apparent at slow heart rates, and is due to the presence of a larger inward late I_Na during phases 2 and 3 of the AP.

FIGURE 3.

CaMKII effects on Na⁺ channel gating affect the AP in a rate-dependent manner. (A–C) Simulations show that at slower heart rates the enhanced late I_Na prolongs the AP (B and C); this effect is completely blunted at faster rates (A), where the reduced channel availability slows down the AP upstroke (A, inset). (D) The upstroke velocity (dV_m/dt) is reduced upon CaMKII overexpression in a rate-dependent fashion: the faster the pacing rate, the more significant the decrease in maximum dV_m/dt. (E) Quantitative summary of the APD₉₀ dependence on the pacing rate.

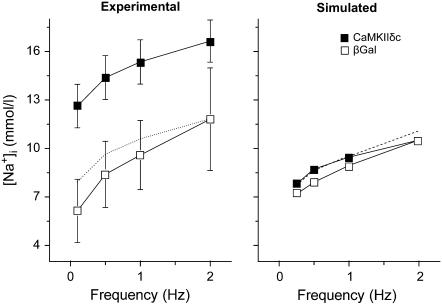
Intracellular [Na⁺] is slightly increased at low pacing frequencies in CaMKII simulations as a result of the enhanced late I_Na during the AP plateau (Fig. 4 right). However, at faster rates, where APD is short and late I_Na is very small, [Na⁺]_i does not change significantly between βGal and CaMKII. The experimental findings of Wagner et al. (4), on the other hand, show a markedly higher [Na⁺]_i in CaMKII-overexpressing cardiac myocytes at all frequencies (Fig. 4 left). However, if we normalize the measured [Na⁺]_i to the value at 2 Hz (Fig. 4 left, dotted line), we again see that the increase in [Na⁺]_i is larger at lower frequency. The higher overall [Na⁺]_i seen in the experimental data suggests that our simulation fails to account for an overall gain in [Na⁺]_i in the myocytes with more active CaMKII. Possibly, CaMKII modulates background Na⁺ influx or efflux by another mechanism.

FIGURE 4.

Experimental (left) and simulated (right) CaMKII effects on [Na⁺]_i induced by I_Na alterations. (Left) The dotted line shows the shifted experimental CaMKII data to equalize [Na⁺]_i at 2 Hz. (Right) The dashed line represents the [Na⁺]_i at different frequencies when I_Na, I_Ca, and I_to alterations due to CaMKII are incorporated into the model.

Ca²⁺ current simulation

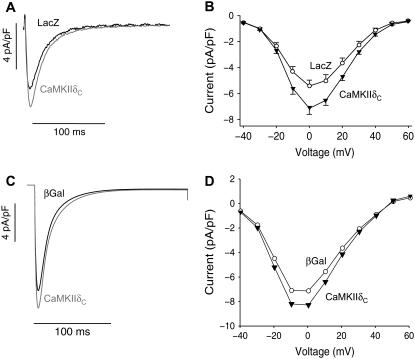
In both acute and chronic CaMKIIδc overexpression, L-type Ca²⁺ current was significantly increased in amplitude and exhibited some slowing of inactivation (7,8). Data from rabbit myocytes acutely overexpressing CaMKIIδc versus LacZ rabbit myocytes are shown in Fig. 5, A and B. The simulated I_Ca under voltage clamp produces an increase in I_Ca amplitude, slowing of inactivation, and unaltered voltage dependence (Fig. 5, C and D) similar to the experimental data. The larger I_Ca amplitude and slower inactivation would be expected to increase APD.

FIGURE 5.

CaMKII effects on I_Ca. (Left) Experimental (A) and simulated (C) traces recorded upon a depolarization pulse. (Right) Experimental (B) and simulated (D) I/V relations. I_Ca is significantly increased in CaMKIIδc compared to control (LacZ). Inactivation is slowed by CaMKIIδc. Experimental data (upper panels) are from Kohlhaas et al. (8).

K⁺ current simulation

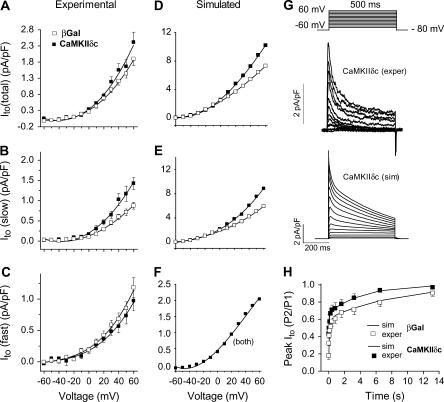
Acute CaMKIIδc overexpression in rabbit ventricular myocytes increases I_to amplitude and speeds recovery from inactivation, and these effects are primarily attributable to alterations in the slowly recovering fraction of I_to (I_to,slow versus I_to,fast (15)). Fig. 6 shows the agreement between experimental and simulated effects of CaMKIIδc overexpression on I_to. Representative traces are shown for comparison between the recordings and the simulated currents upon voltage-clamp stimulation (Fig. 6 G). The V_m dependence of I_to (Fig. 6, left and middle columns) shows an increase in total I_to in both the experiments (Fig. 6 A) and simulations (Fig. 6 D). I_to,slow amplitude was also larger in both experiments and simulations (Fig. 6, B and E), whereas no significant differences between experiments and simulations are evident for I_to,fast (Fig. 6, C and F). I_to,total recovery from inactivation was faster in CaMKIIδc-overexpressing rabbit myocytes (Fig. 6 H, symbols) as well as in silico (Fig. 6 H, lines). The combined CaMKII-dependent increase of I_to amplitude and faster I_to,slow recovery would tend to shorten APD with CaMKII activation (i.e., opposite to that caused by the I_Na and I_Ca effects discussed above).

FIGURE 6.

CaMKII effects on I_to. Experimental (left) and simulated (middle) I/V relations for total (A and D), slow (B and E), and fast (C and F) I_to. CaMKIIδc-mediated increase of the total current (solid symbols) is mainly due to CaMKIIδc effects on the slow component. (G) Representative current traces during voltage-clamp activation protocol for experimental (upper) and simulated (lower) CaMKII-mediated I_to. (H) Recovery from inactivation was investigated using a 500-ms depolarization pulse (from −80 mV holding potential to +50 mV) followed by recovery intervals of increasing duration and a subsequent test pulse. Recovery from inactivation was significantly increased by CaMKIIδc. Symbols represent experimental data, and lines indicate simulation results for βGal (black) and CaMKII (gray). Data are from Wagner et al. (15).

Impact of Na⁺, Ca²⁺, and K⁺ current alterations on AP and [Na⁺]_i

In simulations (compared with experiments), it is possible to test the impact of each individual CaMKIIδc current effect on the rabbit ventricular AP, individually or collectively. Fig. 7 A shows the control (βGal) AP morphology and duration (black solid line) at 1 Hz and Fig. 7 B shows APD dependence on pacing rate (Fig. 7 B, black circles). As in Fig. 3, the effects of CaMKII on I_Na alone tend to prolong the AP (Fig. 7 A, dashed line) especially at slow heart rates, but less so at 2 Hz pacing (Fig. 7 B, diamonds). The CaMKII-induced gain of function of I_Ca also prolongs APD at 1 Hz (Fig. 7 A, dotted line) and other frequencies, though less so than the I_Na change (Fig. 7 B). CaMKII-dependent I_to modulation alone markedly shortens the AP, and flattens the frequency dependence of APD (Fig. 7 A and B, dash-dotted curve).

FIGURE 7.

Simulated APs at 1-Hz pacing rate (A) and APD-frequency relations (B) in βGal and CaMKIIδc-overexpressing cardiac myocytes. The separate effects of each CaMKII-induced current alteration, as well as their combined impact, have been considered. CaMKII alteration of I_Na prolongs the AP at slow heart rate, with no effects at a 2-Hz pacing. The APD is reduced by I_to and increased by I_Ca at all frequencies. The predicted overall effect of CaMKII is AP shortening.

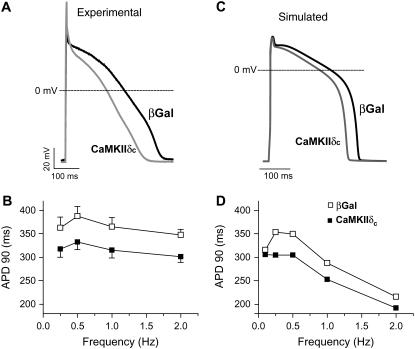
When all three CaMKII target channels are considered (I_Na, I_Ca, and I_to), the net effect is that APD is reduced compared to βGal (Fig. 7, A and B, gray line and solid circles). The large effect of I_to enhancement to shorten APD appears to be dominant over the smaller effects on I_Na and I_Ca which prolong APD. However, the individual ion channel effects on APD modulation seem roughly additive. Fig. 8 shows experimental APD results in rabbit ventricular myocytes overexpressing CaMKIIδc: at left are the results from the study of Wagner et al. (15) and at right are those from the simulations presented in this study. CaMKIIδc overexpression shortens the rabbit ventricular APD. Over the full range of experimental measurements (0.25–2 Hz), APD was reduced by 40–50 ms in the CaMKII case compared to βGal (Fig. 8, B and D). At 0.1 Hz, the model predicts APs of similar duration for βGal and CaMKII (Fig. 8 D). It is possible that at extremely low frequencies the more prominent late I_Na counteracts the enhancement of I_to with almost no changes in the APD.

FIGURE 8.

Experimental (A and B) and simulated (C and D) APs and APD-frequency relations in βGal and CaMKIIδc-overexpressing cardiac myocytes. Experimental data are from Wagner et al. (15). CaMKII shortens the AP in the entire range of pacing rates. At 0.1 Hz, where no experimental data are available, the difference between βGal and CaMKIIδc APs is negligible.

[Na⁺]_i was also assessed during AP simulations. When I_Ca and I_to effects were added to the I_Na effect, there was slightly more CaMKII-induced rise in [Na⁺]_i at higher frequency (Fig. 4, right, dashed line). This might be due to higher Na⁺ influx via Na⁺/Ca²⁺ exchange in this situation. However, the rise in [Na⁺]_i still does not reach the CaMKII-dependent [Na⁺]_i rise seen in the experimental data (Fig. 4, left).

APD sensitivity to the late Na⁺ current

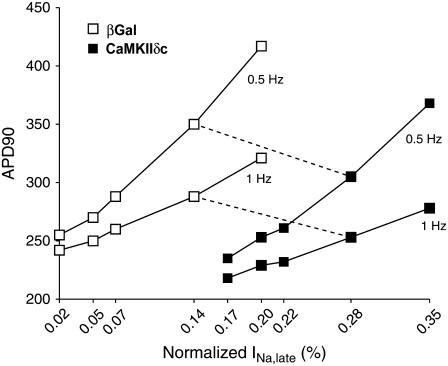
We investigated the impact of different amounts of late I_Na on the APD. To this purpose, the value of the transition rate between background and bursting modes of I_Na (a₈) was varied. For βGal, a range of late I_Na integrals (normalized to peak I_Na if no inactivation had occurred) from 0.02 to 0.2% was explored. Accordingly, late currents ranging from 0.17% to 0.35% were reproduced for CaMKIIδc (to keep the 0.15% differential amount of late current according to experimental data). The results of this sensitivity analysis (Fig. 9) show that the noninactivating Na⁺ current has a significant effect on the AP, and this effect is more pronounced at slower pacing rates (compare 0.5 and 1 Hz). Moreover, such analysis qualitatively confirms our results, since in the entire range explored, CaMKII shortens the AP with respect to βGal, independent of the actual amount of sustained I_Na.

FIGURE 9.

APD₉₀ sensitivity to the late Na⁺ current amplitude. APs were simulated by varying the amount of late Na⁺ current in a range between 0.02% and 0.2% of the peak current in βGal (open symbols) and between 0.17% and 0.35% in CaMKII (solid symbols), thus keeping constant the differential current amplitude. APD changes significantly with the late current amplitude, and the sensitivity increases with the lowering of the pacing frequency (compare 0.5 and 1 Hz). Note that the results of AP shortening upon CaMKII overexpression are confirmed for any value of late Na⁺ current amplitudes in the explored range.

APD changes with different I_to levels

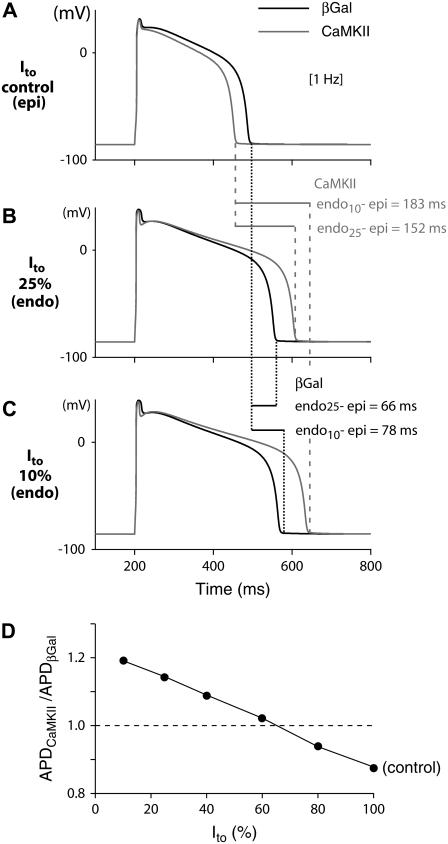
Differences in the expression pattern of the channels responsible for I_to,fast and I_to,slow in different regions of the heart produce marked differences in current density and contribute to regional variations in AP duration (25). In addition, downregulation of I_to is a central and consistent electrophysiological change in cardiac diseases (e.g., HF) (26). Thus, different levels of I_to density (both fast and slow) were simulated (Fig. 10). The simulation of CaMKII has different impacts depending on I_to density. That is, when I_to is fully expressed (I_to 100% (Fig. 8 C and Fig. 10, A and D)), CaMKII shortens the AP, whereas in cells expressing 75% or 90% I_to reduction, the effect of CaMKII-induced I_to enhancement is limited (Fig. 10, B and C). In conditions where I_to expression is <60% (Fig. 10 D), net AP prolongation occurs due to the I_Na and I_Ca effects (which then outweigh the I_to effect).

FIGURE 10.

Simulated APs at 1 Hz in βGal and CaMKIIδc-overexpressing cardiac myocytes in the presence of (A) 100%, (B) 25%, and (C) 10% I_to. (D) Ratio of CaMKII to βGal APDs for different levels of I_to. When I_to is fully expressed (A, epi), CaMKII shortens the AP, whereas the AP is prolonged due to I_to downregulation (current expression <60% (D), e.g., endo (B and C)). This could amplify transmural dispersion of repolarization (endo-epi: 66 and 78 ms for βGal versus 152 and 183 ms for CaMKII), which may predispose cells to reentry phenomena.

DISCUSSION

In this study, we used an in silico approach to investigate CaMKIIδc effects on cardiac myocyte ionic currents and APs in a comprehensive computer model of ECC (19). A motivating factor was our recent report (4) that CaMKII associates with and phosphorylates the cardiac Na⁺ channel and produces I_Na gating changes that strikingly resemble those in a human genetic mutation in Nav1.5 (1795InsD) that is linked with simultaneous arrhythmogenic LQT3 and Brugada syndrome (2). In addition, CaMKII expression is upregulated and more active in HF (3,27,28) and arrhythmias are a major cause of death in HF. There are also reports of enhanced late I_Na in HF (29). Thus, increased CaMKII-dependent I_Na modulation in HF may be an acquired arrhythmogenic Na⁺ channel defect that could affect millions of people with HF (as compared with the relatively small number of individuals affected by the mechanistically informative genetic Na⁺ channel mutations). Along these lines, overexpression of CaMKIIδc in transgenic mice leads to a lethal phenotype characterized by HF (30) and increased propensity to ventricular arrhythmias (4). Transgenic or pharmacologic CaMKII inhibition has also been shown to be protective against maladaptive remodeling from chronic β-adrenergic receptor stimulation, myocardial infarction (31), and arrhythmias (32). Thus, CaMKII may be a viable therapeutic target in the treatment of common forms of cardiac disease, and understanding how CaMKII alters ionic currents and APs is important to better understand arrhythmias that occur in conditions of CaMKII upregulation.

We focused initially on CaMKII-dependent modulation of I_Na (4), but we have extended the rabbit ventricular myocyte model to incorporate other channels modulated by CaMKII in heart, I_Ca (8) and I_to (15). This sort of computational approach has proven valuable to integrate information obtained at the molecular level to the whole cell and to the clinical phenotype in a number of inherited (16–18,33) and acquired (34–36) cardiac diseases.

Na⁺ channel model

A Markov model of I_Na was identified to simulate I_Na measured using whole-cell patch clamp in βGal versus CaMKIIδc-overexpressing rabbit cardiomyocytes (4). This model structure has been used successfully to simulate several I_Na features (16–18,33). Clancy and Rudy (16) used a nine-state model (only the background mode) for the wild-type Na⁺ channel. To account for long-lasting bursts experimentally observed in mutant Na⁺ channels they introduced an additional mode of gating (burst mode) with a second (long-lasting and noninactivating) open state (17). Thus, the burst layer, thought to be responsible for a whole-cell persistent current, was incorporated into both our βGal and CaMKIIδc Na⁺ channel models. The resulting model satisfactorily reproduces the characteristics of CaMKIIδc-modulated I_Na in rabbit ventricular myocytes (4) and seems suitable to analyze the effects of CaMKIIδc at the AP level.

Impact of Na⁺ current alterations on AP and [Na⁺]_i

CaMKIIδc enhances intermediate inactivation and reduces availability, at the same time impairing fast inactivation and enhancing persistent I_Na. These divergent alterations of I_Na function cause a paradoxical phenotypic overlap of LQT3 (where I_Na inactivation is slowed or incomplete) and Brugada syndrome (where available I_Na is reduced), both thought to underlie arrhythmias. Indeed, the altered Na⁺ channel phenotype caused by CaMKIIδc is very similar both qualitatively and quantitatively to that caused by 1795InsD mutation in human Nav1.5, which is linked with simultaneous LQT3 and Brugada syndrome features (2).

At faster heart rates, CaMKII causes Na⁺ channel accumulation in intermediate inactivation, with slow recovery therefrom, and reduces steady-state availability. The result is a loss of I_Na function, reduced rate of AP rise, and, consequently, slowed propagation velocity, consistent with a BrS phenotype at fast heart rate. At slow heart rates where the diastolic interval is longer, there is little loss of Na⁺ channel availability (longer recovery time), and the intrinsically longer APD is further prolonged by sustained or late I_Na. This results in accentuated APD prolongation at slow heart rates (Fig. 3, B, C, and E) and an LQT3 phenotype.

It is possible that increased CaMKIIδc activity in HF may alter Na⁺ channel gating, contributing to arrhythmogenesis. Indeed, noninactivating late I_Na is increased in human HF and failing canine hearts (29), and this could contribute to the prolonged APD characteristic of HF.

A higher sustained I_Na in HF due to CaMKIIδc could contribute to the elevated [Na⁺]_i observed in HF (37). However, the simulations here show that the I_Na gating changes that prolong APD are insufficient to explain the elevated [Na⁺]_i in either HF or CaMKII-overexpressing rabbit myocytes. Despa et al. (38) found that the elevated [Na⁺]_i in HF was due to increased tetrodotoxin-sensitive diastolic influx. The fact that CaMKII overexpression produced a [Na⁺]_i increase (4) similar to that seen in HF (38), where CaMKII is upregulated (3), suggests an untested hypothesis that CaMKII might enhance diastolic tetrodotoxin-sensitive Na⁺ influx in both situations.

The slight additional rise in [Na⁺]_i at higher frequency when I_Ca and I_to effects of CaMKII were considered (versus I_Na alone), could be due to the close interplay between Na⁺ and Ca²⁺ regulation in myocytes. The higher I_Ca and SR Ca²⁺ release would increase Na⁺ influx via a Na⁺/Ca²⁺ exchanger (which is the largest normal pathway for Na⁺ influx in myocytes).

CaMKII has multiple targets that may alter AP

Although Na⁺ channel alterations can result in gain or loss of function, depending on the pacing frequency, CaMKII modulation of I_Ca and I_to produced only gain of function at all pacing rates. Enhanced I_Ca prolonged APD, whereas I_to gain of function dramatically shortened APD (Fig. 7). When considering the effects of CaMKII on I_Na, I_Ca, and I_to together, APD was shortened, indicating the stronger impact of the enhanced repolarizing I_to (compared to the combined depolarizing contributions of I_Ca and I_Na). It is noteworthy that this balance is shifted toward a net repolarizing current in a wider range of late I_Na, as demonstrated by the sensitivity analysis in Fig. 9.

We also included CaMKII-dependent alterations to increase the [Ca²⁺]_i affinity of the SR Ca-ATPase (simulating phospholamban phosphorylation) and sensitization of the SR Ca²⁺ release channel (not shown) using parameters similar to those used by Shannon et al. (39). Although this altered Ca²⁺ transients and APD characteristics slightly, the APD shifts reported here were not qualitatively altered. Thus, these effects do not modify our main conclusions about I_Na, I_Ca, and I_to effects.

The CaMKII-induced enhancement of I_to would tend to prevent the LQT3 effect of late I_Na and I_Ca enhancement, and it would not ameliorate the BrS phenotype at fast heart rates. Indeed, the greater I_to might slightly further snub the latter part of the AP rise and the AP peak, accentuating the BrS phenotype. On the other hand, the concerted effect of CaMKII on I_to (with I_Na and I_Ca) may be an orchestrated response to limit APD prolongation when CaMKII is physiologically activated. In pathophysiological conditions like HF, this situation may differ. In HF there is typically a strong downregulation of I_to (26), and this would limit the ability of enhanced I_to to offset the APD prolongation resulting from I_Na and I_Ca effects (Fig. 10). The lower amplitude of SR Ca²⁺ release in HF would also limit the extent of Ca-dependent inactivation of I_Ca, which would also tend to prolong APD. These effects could make the HF patient (in whom APD is already long) more prone to this sort of acquired LQT3 and its arrhythmogenic consequences.

The AP-ECC model implemented here (19) is for a generic rabbit ventricular myocyte, without distinction for regional differences in channel density within the normal ventricular wall (40,41). However, in regions where I_to is poorly expressed (e.g., endocardium), the effects of I_Na and I_Ca might be predominant, thus potentially leading to a LQT3-like phenotype at slow heart rates (Fig. 10, B and C) and BrS phenotype at high frequencies. In regions where I_to is maximally expressed (e.g., epicardium), CaMKII activation or upregulation may lead to a markedly short AP, thus resembling an acquired form of short QT syndrome. In fact, although prolonged QT intervals are intuitively associated with the risk of life-threatening events, the notion of a short QT syndrome being arrhythmogenic is relatively new (42).

Heterogeneity of transmural ventricular repolarization in the heart has been linked to a variety of arrhythmic manifestations (43). If CaMKII prolongs APDs in the endocardium (Fig. 10, B and C) and shortens APDs in the epicardium (Fig. 10 A), this could amplify transmural dispersion of repolarization (endo-epi 66 and 78 ms for control versus 152 and 183 ms with CaMKII (Fig. 10)), thus predisposing to the development of reentrant arrhythmias. Thus, there are several potential pathways by which cardiac CaMKII activation may be arrhythmogenic, and further experimental and in silico work will be needed to clarify which pathways are most important.

Species differences and time domain of CaMKII overexpression

In striking contrast to the AP shortening shown in rabbits, we have shown that chronic CaMKII overexpression in mice significantly prolongs APD in transgenic versus wild-type mice (7,15). Mouse electrophysiology differs significantly from that of rabbit, but this difference may be simply explicable. In the mouse, with chronic overexpression of CaMKII, I_Na and I_Ca are enhanced, as in rabbit, but I_to is decreased. The result is that all three current changes considered here will tend to prolong APD in mouse. Thus, it is not surprising that CaMKII prolongs the APD in the transgenic CaMKIIδc mouse (and differs from rabbit). In rabbit myocytes, acute CaMKII overexpression increased I_to mainly by increasing I_to recovery from inactivation for both I_to,fast and I_to,slow (and this is an acute regulatory effect sensitive to CaMKII inhibitors), but there is also a minor increase in I_to,slow functional expression. In transgenic mice chronically overexpressing CaMKIIδc, the same acceleration of I_to recovery from inactivation occurs (acutely blockable by CaMKII inhibition), but down-regulation of I_to,fast (the predominant repolarizing current in mouse) and Kv4.2/3 expression causes the dominant effect to be reduced functional I_to (15). This raises the notion that CaMKII can have both acute regulatory effects on ion channels (via CaMKII-dependent phosphorylation), but can also alter the expression levels (via CaMKII effects to alter protein transcription, translation, or trafficking (44)). There may also be time-dependent differences in this regulation, since 24-h overexpression of CaMKII was sufficient to demonstrate upregulation of I_to,slow (and Kv1.4), whereas the downregulation of I_to,fast was only evident during chronic transgenic overexpression of CaMKII. Eventually, it would be valuable to incorporate these longer-term aspects of channel modulation into dynamic computational models.

CaMKII modeling in cardiac myocytes

In our model, we simply turned on the CaMKII-modified version of the modulated currents, without considering either dynamic or local changes of its activity or association with target ion channels. Recently, Hund and Rudy (45) proposed a novel theoretical model of the canine ventricular epicardial AP and Ca²⁺ cycling, including a CaMKII regulatory pathway. They investigated aspects of Ca²⁺ transient and APD rate dependence, and concluded that CaMKII is an important determinant of the rate dependence of Ca²⁺ transient but not of APD (although neither I_Na or I_to were considered as CaMKII targets). They also showed that elevated CaMKII activity increased the cell propensity to Ca²⁺ transient and APD alternans (36).

Saucerman and Bers (2007) integrated mathematical descriptions of calmodulin-dependent regulation of CaMKII and calcineurin with the Chicago ECC model in the rabbit ventricular myocyte (46). They showed how CaMKII is especially well activated in regions where local [Ca²⁺]_i is transiently high, especially in the junctional cleft near I_Ca and SR Ca release sites. The subsarcolemmal region, where most other ion channels (e.g., I_Na and I_to) are localized, also exhibits higher [Ca²⁺]_i than bulk cytosolic [Ca²⁺]_i (47) and would thus also be expected to exhibit more dynamic CaMKII activation.

In conclusion, we present a computational modeling approach to study how CaMKII-dependent changes in ionic currents (I_Na, I_Ca, and I_to) influence APD at different heart rates. This may provide novel mechanistic insights into cellular bases of acquired arrhythmogenesis in HF and other pathophysiological settings. It may also help to identify or tailor novel potential therapeutic targets.