MAPPING ELASTICITY IN HUMAN LENSES USING BUBBLE-BASED ACOUSTIC RADIATION FORCE

Abstract

This study uses acoustic radiation pressure to displace a femtosecond laser-produced bubble in human lens tissue. Bubble displacement is monitored with low-amplitude, high-resolution ultrasound. Displacements are compensated by bubble size determined from ultrasonic backscatter. The Young’s modulus is proportional to the inverse of the compensated displacement with the constant of proportionality determined from similar measurements in a controlled gelatin sample. Multiple measurements were obtained on 12 human lens specimens grouped into two age categories, middle-age (about 40 years old) and old-age (63-70 years old). There were 3 lenses from 2 donors in the middle-age group and 9 lenses from 5 donors in the old-age group. At each radial position, the median value was computed for all measurements within each group. For middle-age lenses, Young’s modulus ranged from 5.2 kPa in the center to 1.1 kPa on the periphery. For old-age lenses, Young’s modulus ranged from 10.6 kPa in the center to 1.4 kPa on the periphery. These values are the same order of magnitude as previous measurements using other techniques. The age related change in elasticity distribution is also similar to a previous study. Radially varying elasticity may provide insight into the mechanics of accommodation.

Lens accommodation is not fully understood because proper tools for measuring its relevant properties are not fully developed. Early measurements of lens elastic moduli were limited to averages over large volumes (Burd, et al., 2006; Fisher, 1971). These techniques assumed major regions, such as the nucleus and cortex, were homogeneous. More recent techniques have greater spatial resolution and have demonstrated elastic heterogeneity (Heys, et al., 2004).

Our laboratory is developing a potential in-vivo elasticity measurement method based on acoustic radiation force applied to laser-produced bubbles. This technique was originally demonstrated with measurements on controlled gelatin specimens (Erpelding, et al., 2005). It was further improved by switching the pushing pulse from a tone burst to a chirp signal (Erpelding, et al., 2007a). More recently, we used this technique to measure radially varying elasticity in porcine lenses (Erpelding, et al., 2007b). In the current study, we present the first measurements using this technique on human lenses.

Fresh lenses were obtained from human donors at autopsy. Specimens were embedded in collagen gelatin with ultrasound access from the top coupled by water and laser access from the bottom. An ultrafast femtosecond laser created targeted microbubbles in the tissue at various radial distances from the lens center with 1 mm spacing. A two-element confocal ultrasound transducer applied acoustic radiation force to the bubble with the 1.5 MHz outer element while monitoring bubble displacement within the lens using pulse-echoes with the 7.44 MHz inner element. A correlation-based algorithm was applied to pulse-echo data to determine bubble displacements using the final position as reference. Several displacement measurements were made on each bubble until it dissipated. Often measurements could be made at several circumferential positions in the same specimen. The same technique and equipment were used for the porcine study reported earlier (Erpelding, et al., 2007b).

Laser-produced bubbles in tissue vary in size. Also, gas and/or vapor in a bubble diffuse into surrounding tissue so the bubble gradually shrinks. Acoustic radiation force is proportional to bubble cross-sectional area, so size must be measured simultaneously with each displacement measurement. Fortunately, ultrasonic integrated backscatter (O’Donnell, et al., 1979; Thomas, et al., 1986) (the amount of signal reflected from a bubble) is proportional to bubble size, so it can be used to compensate displacement. Young’s modulus is proportional to the inverse of this compensated displacement.

In the porcine study we did not determine the constant of proportionality and so calculated relative stiffness coefficients. For the current study, the proportionality constant was determined from similar measurements on controlled gelatin specimens. Young’s modulus of gelatin was independently measured with a displacement piston and electronic force scale. Size-compensated bubble displacement was also measured in the same gelatin. Typical bubbles in gelatin were about an order of magnitude larger than bubbles in tissue, but compensation should account for this difference.

Human specimens were divided into two groups. The old-age group consisted of 9 lenses from 5 donors ranging in age from 63 to 70 years. The middle-age group consisted of 3 lenses from 2 donors ranging in age from 40 to 41 years. Often, more than one measurement could be made for a given radial position in the same lens. Occasionally, a bubble could not be produced at a given location. This happens more often in the periphery. For the old-age group, the number of independent measurements at a given radial position ranged from 12 to 25. For the middle-age group, it ranged from 4 to 8.

Fig. 1 shows bubble-size compensated displacement for all data points. While there is not enough data in the middle-age group to statistically prove they are a different population from the old-age group, this figure qualitatively suggests that possibility. Although it shows some overlap between populations, at all positions several values from the middle-age group are greater than the maximum from the old-age group. Also several values from the old-age group are lower than the minimum from the middle-age group. More measurements are needed to quantitatively verify this trend.

Fig. 1.

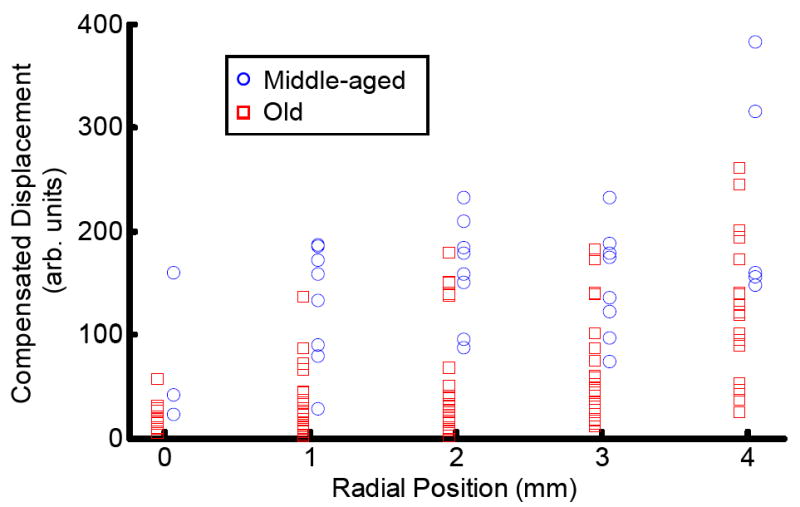
Bubble displacement as a function of radial position in human lenses. Displacement has been compensated for bubble size by dividing displacement by the square root of the integrated ultrasonic backscatter signal. For radial position, the origin is the lens’ center. Data from the subset of old human lenses are represented by red squares. Data from middle-aged human lenses are represented by blue circles. Both data sets have been slightly radially offset on the graph to better distinguish between them. True radial positions are the closest integer millimeter.

Even though we used the same equipment and technique, we notice greater measurement variation for uncompensated displacements in human compared to porcine lenses. A quick reanalysis of porcine results indicates that their Young’s modulus is about the same order of magnitude as older human lenses. We cannot account for this difference in variation and do not understand it at this time.

Young’s modulus as a function of radial position for both human age groups is shown in Fig. 2. These curves display the median value of the data with error bars indicating the average difference from the median above and below. This unusual analysis was used because variations in human data were large with an asymmetric probability distribution, particularly for the old-age group. Acoustic radiation force should only produce a positive displacement where the bubble only moves in the wave propagation direction. Under ideal conditions, negative displacements from traveling waves would violate the laws of physics. However, measurement variations were large so, constrained by the physics, they were more likely to favor greater displacement rather than less. This situation is particularly relevant to older (stiffer) lenses that result in small displacements compared to the variation. If mean is used to estimate the maximum likelihood of an asymmetric distribution then a few large displacements as well as the distribution’s asymmetry will skew the estimate. In such cases, the median is a better estimate. In fact, a median different than the mean indicates asymmetry in the distribution. For these results, the medians are indeed significantly different than the means.

Fig. 2.

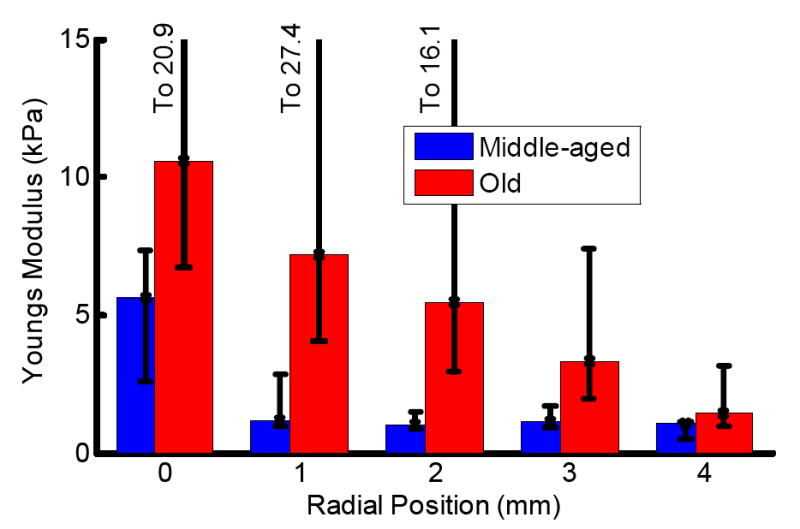
Young’s modulus as a function of radial position in human lenses. Bar graph values represent the median of the distributions. Error bars indicate positive and negative average deviations from the median. Red bars show results from old human lenses. Blue bars show results from middle-aged human lenses. Small compensated displacements create large variations in high modulus tissues.

Our results show the same general trend in age related changes of human lens as a previous study from another laboratory using a different measurement technique (Heys, et al., 2004): the lens center stiffens at a faster rate than the periphery. Even with this general agreement, our results for the old-age group are a factor of 5 times softer. They report a Young’s modulus of 54 kPa at lens’ center to 7.4 kPa at the periphery for a 64 year-old human donor. Note that they report elasticity as shear modulus. For nearly incompressible materials (Poisson’s ratio = 0.5) Young’s modulus is approximately three times the shear modulus. For the same old-age group, we measure values of 10.6 kPa at center to 1.4 kPa at periphery. This is a comparison between nearly direct mechanical based methods with comparable resolutions.

In contrast, middle-aged humans (when effects of presbyopia initially become apparent) have a flatter elasticity distribution as well as a much lower average. Because of lower mortality, lens research tissue from middle-aged (as well as young) humans is rarer, leading to a small sampling size. More specimens are required to confirm initial results. Also, more measurements at more independent sites within a single specimen could improve measurement accuracy, although they would not help with specimen-to-specimen variations. Even so, our elasticity measurements for this age group ranged from 5.2 kPa in the center to 1.1 kPa at the periphery. There is no representative data set from a 40 year-old in the previous study (Heys, et al., 2004), but they do list average elasticity in the nucleus and cortex regions of 1.4 and 0.83 kPa respectively. Component measurements used in the average should be higher at the exact center and lower at the periphery. For the middle-aged group, our results compare favorably with this previous study.

Another study (Fisher, 1971) of experiments conducted in the early 1970’s, used a less direct measure of elasticity with much poorer resolution. They only measured and reported elasticity averaged over the nucleus and cortex regions. This study also explicitly assumed the regions were homogeneous in elasticity. Their results indicated that, while both regions increased in elasticity with age, they either increased at the same rate or the cortex increased at a slightly greater rate. Greater resolution of the two newer methods (ours and Heys, et. al.), the more direct nature of the measurements, as well as an assumption of non-homogeneous elasticity raises questions about these results.

Radially varying elasticity may provide insight into the mechanics of presbyopia. Even though the ciliary muscle acts on the lens in the radial direction, geometric symmetry of the lens dictates that radial deformation is zero at the axis of symmetry (the lens center) and minimal in most of the optical path near the center. Polar deformation is not limited by symmetry so it has the greatest influence on curvature changes of the lens surfaces, and therefore the greatest influence on accommodation. Polar deformation is influenced by average stiffness along the polar direction, so is affected by radial variations in elasticity.

If lens elasticity was homogeneous, then volume conservation (lens tissue has a Poisson’s ratio close to 0.5) dictates that radially stretching the lens would flatten it in the center. However a stiff center would resist flattening so more deformation would occur at the periphery outside the optical path. A soft center would have the opposite affect, encouraging greater curvature change in the optical path that would produce a greater change in optical power.

Unlike other published mechanical measurements, the bubble-based acoustic radiation force technique can potentially be used in-vivo. Both laser and ultrasound systems are non-contact, and corresponding beams can propagate through the cornea, the anterior chamber, and into the lens. Such in-vivo measurements of elasticity are highly desirable to guide and fine-tune any laser surgical procedure addressing presbyopia. The results of this study are an important step toward that goal.