Problems in obtaining precise and accurate Sr isotope analysis from geological materials using laser ablation MC-ICPMS

Abstract

This paper reviews the problems encountered in eleven studies of Sr isotope analysis using laser ablation multicollector inductively coupled plasma mass spectrometry (LA-MC-ICPMS) in the period 1995–2006. This technique has been shown to have great potential, but the accuracy and precision are limited by: (1) large instrumental mass discrimination, (2) laser-induced isotopic and elemental fractionations and (3) molecular interferences. The most important isobaric interferences are Kr and Rb, whereas Ca dimer/argides and doubly charged rare earth elements (REE) are limited to sample materials which contain substantial amounts of these elements. With modern laser (193 nm) and MC-ICPMS equipment, minerals with >500 ppm Sr content can be analysed with a precision of better than 100 ppm and a spatial resolution (spot size) of approximately 100 μm. The LA MC-ICPMS analysis of ⁸⁷Sr/⁸⁶Sr of both carbonate material and plagioclase is successful in all reported studies, although the higher ⁸⁴Sr/⁸⁶Sr ratios do suggest in some cases an influence of Ca dimer and/or argides. High Rb/Sr (>0.01) materials have been successfully analysed by carefully measuring the ⁸⁵Rb/⁸⁷Rb in standard material and by applying the standard-sample bracketing method for accurate Rb corrections. However, published LA-MC-ICPMS data on clinopyroxene, apatite and sphene records differences when compared with ⁸⁷Sr/⁸⁶Sr measured by thermal ionisation mass spectrometry (TIMS) and solution MC-ICPMS. This suggests that further studies are required to ensure that the most optimal correction methods are applied for all isobaric interferences.

Introduction

The radioactive beta (β⁻) decay of ⁸⁷Rb to ⁸⁷Sr is an important isotope system that has been widely applied for geochronological purposes. More importantly the isotopic system is extensively used to constrain the rates and fluxes involved in a wide range of geological processes operating from within the hydrosphere of the Earth to the deep mantle. In addition, recently Sr isotopes have become widely applied as provenance tracers in many different scientific disciplines (e.g. biology, nutrition, medical, forensic and art history) [1–7]. Sr isotope ratios have always been difficult to analyse to high precision (better than 20 ppm), because there are large differences in the abundances of the isotopes ⁸⁴Sr, ⁸⁶Sr, ⁸⁷Sr and ⁸⁸Sr. In many materials the low abundance of Rb and their relatively young age leads to small isotopic variations (e.g. 0.05% in mantle rocks and biogenic carbonates). This range is considerably smaller than the combined U-Th-Pb isotope system for example. Recent technical improvements in thermal ionisation mass spectrometry (TIMS) design, most notably in terms of Faraday collectors and amplifier electronics, now allow analytical precisions better than 0.0005% (5 ppm, 1 SE). Despite this significant improvement in precision, the classical TIMS technique requires time-consuming liquid chromatographic techniques to remove matrix and interfering elements (⁸⁷Rb), inhibiting any possibility for an “online” in situ analytical technique.

The arrival of the first commercial multicollector inductively coupled plasma mass spectrometry (MC-ICPMS) instruments in the first half of the 1990s, coupled with laser ablation (LA) facilities promised an enormous new potential for in situ analytical techniques in petrology, marine sciences and many other applications (e.g. see [8, 9]). The study of small-scale variations of Sr isotopes in geological materials has provided very important constraints on the rates of volcanic processes and the fluxes that operate in diverse geological environments (e.g. see [2, 10]). However, these studies are extremely time consuming, typically averaging more than 10 hours per sample because they require careful microdrilling techniques, low-blank liquid chromatographic separation techniques and TIMS analyses. The MC-ICPMS coupled with a laser ablation system should be able to perform a Sr isotope analyses within minutes, while importantly maintaining the spatial resolution and avoiding the extensive wet chemistry and warm up times on the TIMS instrument.

Despite the great potential of LA-MC-ICPMS analyses for Sr isotopes and its availability for more than a decade, the technique has not become routine. Eight out of eleven papers published in the period 1995–2006 that reported in situ analyses of Sr isotopes by LA-MC-ICPMS are mainly focussed on technique development [11–21]. There is clearly a huge potential and a demand for the application of in situ Sr isotope analysis. Therefore the goals of this paper are to: (1) describe the different approaches that have been followed to date, (2) summarize the problems encountered, (3) discuss the solutions attempted to solve the encountered problems and (4) discuss studies which are needed in the near future to improve the LA-MC-ICPMS technique so that “routine” Sr isotope analysis becomes possible.

Description of instrument setups

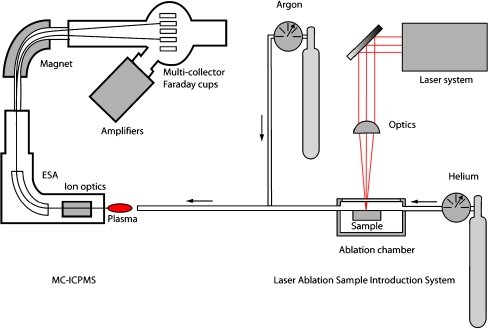
All LA-MC-ICPMS instrument setups have the same basic geometry of LA system, pulsed laser focussed onto ablation chamber, sample transfer-inlet system, differential vacuum pumping system, mass-energy filter and signal detection (Fig. 1). It is beyond the scope of this review to discuss the actual performance of the different mass spectrometers, so we concentrate upon the sample handling and data interpretation aspects of the LA-MC-ICPMS technique.

Fig. 1.

Typical LA-MC-ICPMS setup with He as carrier gas. ESA electrostatic filter analyser

Laser ablation systems

Laser ablation systems have been tremendously improved in the last decade (see [22] for a review). This development has mainly focussed on how to obtain maximum sensitivity in both sample ablation and transfer of the ablated sample to the ion optics of the ICP-MS and a minimized fractionation of elemental ratios, for example Th/U (e.g. see [23, 24]). The early studies of Christensen et al. [11] and Davidson et al. [12] used a pulsed 266-nm quadrupled Nd:YAG laser, whereas more recent studies have tended to use shorter wavelengths, e.g. pulsed 213-nm and Excimer 193-nm lasers (Table 1). Owing to the more efficient absorption of shorter wavelength light by most materials, the reported interelement fractionation is less with lower wavelength lasers (e.g. see [25, 26]). Consequently, the application of lower wavelength lasers tends to produce more accurate Sr isotope results. However, it is difficult to judge from the published papers which laser system has the highest sensitivity, as this is highly dependent on the repetition rate of the laser, the pit size or raster technique used and the energy density of the laser system. Moreover, ICP-MS inlet design and instrument sensitivity have improved by several orders of magnitude in the last decade, so direct comparison between the sensitivity of systems of different age is perhaps pointless.

Table 1.

Instrument setups and materials analysed in eleven publications on Sr isotope analyses by LA-MC-ICPMS

Reference	Laser type	Pit sizes (μm)	Materials (Sr concentration)	Instrument	Sensitivity/blank levels
[11]	Nd:YAG, 266 nm, 8 Hz, 20–30 mJ pulse−1, carrier gas Ar	Spot 20–40 and 150–300	Carbonate (2,000 ppm)	VG P54	LA 2,000 ppm, sample material gives >3 V total Sr
			Feldspar (2,000 ppm)		No blank reported
[12]	Nd:YAG, 266 nm, 5–20 Hz, 1.2 mJ, carrier gas not specified	Spot 100–300, raster	Plagioclase (1,200–2,100 ppm)	IsoProbe	No blank reported
[13]	Nd:YAG, 266 nm, 10 Hz, 0.66 mJ, carrier gas not specified	Spot 70, raster	Carbonate, fresh water otolith (ca. 500 ppm)	VG P54	No blank reported
[14]	Nd:YAG, 266 nm and 213 nm, 20 Hz, 4 mJ, carrier gas Ar	Spot 10–200	Plagioclase, apatite sphene, clinopyroxene otolith	VG Axiom	ca. 25 V ppm−188Sr (solution work); blank 0.5–3 mV 85Rb and 88Sr
[15]	Excimer, 193 nm, 1–2 Hz, 4–5 J cm−2, carrier gas He	Spot 150–330	Apatite (>3,000 ppm)	IsoProbe	Not reported
			Carbonate (>3,000 ppm)
[16]	Excimer, 193 nm, (see [15])	Spot 330	Clinopyroxene (100–400 ppm), plagioclase, carbonate	IsoProbe	Not reported
[17]	Nd:YAG, 213 nm, 10 Hz, 7–10 J cm−2, carrier gas He	Spot 80, raster 160 × 500	Carbonate (1,000 ppm)	ThermoFinnigan Neptune	88Sr blank <5 mV
			Plagioclase (900 ppm)
			Clinopyroxene (50 ppm)
			Basaltic groundmass (400 ppm)
[18]	Excimer, 193 nm, 5 Hz, 10 J cm−2, carrier gas He	Spot 10–350, raster	Carbonate otolith	Nu Plasma	Not reported
[19]	Nd:YAG, 213 nm	Raster 60–500, 80 deep	Carbonate, fresh water otolith (ca. 300–800 ppm)	ThermoFinnigan Neptune	Not reported
[20]	Excimer, 193 nm, 5 Hz, 50 mJ, carrier gas He	Raster, 80 wide	Carbonate, otoliths	ThermoFinnigan Neptune	Not reported
[21]	Nd:YAG, 213 nm, 20 Hz	Spot 120, raster	Melt inclusions	ThermoFinnigan Neptune	ca. 40 V ppm−188Sr (solution work)
					Blank not reported

He (0.5–1.0 L min⁻¹) is used as the transport gas through the ablation cell in nearly all studies (see Table 1) following the publication of [27], which demonstrated that He gives a higher sensitivity and less inter-element fractionation (Rb/Sr) compared with other potential carrier gases (e.g. Ar). The He flow is mixed with Ar flow before it enters the plasma in a ratio between 0.5 and 1.0 (see Fig. 1).

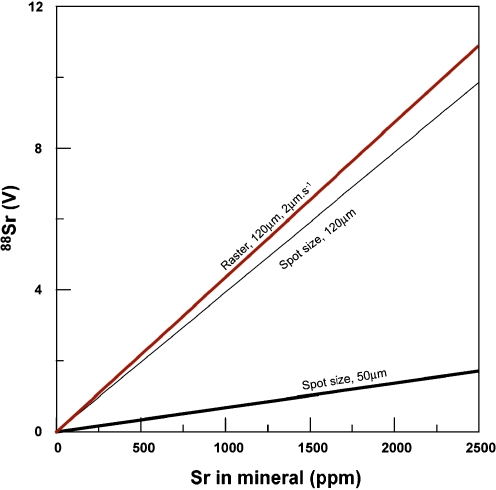
With laser ablation analyses there are essentially two possible ablation methods: spot analysis and rastering. A spot analysis leaves the laser beam in the same position where the laser beam progressively ablates material from deeper levels of the ablation pit. There are two advantages of spot analysis: first, the optimal spatial resolution is obtained, typically between 100–300 μm for Sr isotopes; second, this approach limits the influence of surface contamination. A significant disadvantage of the single spot analysis is that on many laser ablation systems the laser will go out of focus if the depth to diameter ratio of the ablation pits is larger than one [28]. This will result in lower beam intensities due to lower ablation rates coupled with less efficient sample fragmentation that also reduces ionisation efficiency in the plasma. Furthermore, if the depth to diameter ratio becomes larger than 6, significant fractionation occurs between elements [28], which could make the ⁸⁷Rb correction on ⁸⁷Sr less accurate. For a 50-μm spot analyses the maximum ablation time is 1 min (assuming 1 μm s⁻¹ ablation rate, see Fig. 2).

Fig. 2.

Signal intensity obtained by ablating different spot sizes (50 and 120 μm) and rastering (120-μm spot, moving at 5 μm s⁻¹) as a function of Sr abundance in a mineral (modified from Fig. 2 in [12]). The ablation rate is 1 μm s⁻¹, and the typical efficiency is 0.05% (e.g. 1 in 2,000 ions that get ablated are counted by the detector). This diagram illustrates that with a 50-μm spot size, only the minerals with >2,000 ppm can be analysed with sufficient precision. Rastering results in slightly better ⁸⁸Sr signals, but its greatest advantage is that the beam does not run out of focus during the analysis, and the ⁸⁸Sr ion current does not decay as with a spot analysis

The rastering technique involves the movement of the sample with a low speed (typically 1–5 μm s⁻¹, e.g. see [18]). The advantage is that the ion beam size is more stable over time, and the ⁸⁸Sr signal is larger (see Fig. 2). Disadvantages are that the surface contamination of the sample could be a problem and the advantage of high spatial resolution of LA is diminished. The surface contamination problem can be overcome by “pre-cleaning” the ablating area with the same raster area but with a lower ablation rate.

MC-ICPMS instruments

Five different MC-ICPMS instruments have been used to obtain Sr isotopes LA-MC-ICPMS (see Table 1). Although different in configuration, the type of MC-ICPMS instrument does not seem to be very important. All MC-ICPMS instruments can collect the Sr isotope masses and the additional masses required to perform the isobaric corrections on the Sr isotope masses. There are probably differences in the sensitivity, whereby the older generation instruments (P54, Isoprobe and Axiom) have a lower sensitivity than more recently designed instruments (ThermoFinnigan Neptune and Nu Plasma instruments). The collision cell arrangement is not beneficial for Sr isotope analysis (maybe for Fe interferences, see below), nor is the high-resolution capability of the Nu Plasma HR, Nu Plasma 1700 and the ThermoFinnigan Neptune instruments because isobaric interferences, such as ⁸⁷Rb on ⁸⁷Sr, can only be resolved with a mass resolution of >10,000, significantly beyond the capabilities of all MC-ICP-MS instruments.

Materials ablated

Table 1 lists the different types of geologically relevant materials which have been analysed. Except for the melt inclusion work reported by Jackson and Hart [21], most of the tested materials are characterized by low Rb/Sr (<0.002) ratios. The majority of studied materials are marine carbonate and magmatic plagioclase. Most studies have used present-day marine carbonate as an “in-house” standard, assuming that it has the ⁸⁷Sr/⁸⁶Sr composition of present-day seawater: 0.709172 [29]. Marine carbonate has in the order of 2,000 ppm Sr. Plagioclase is another suitable material, since it has also low Rb/Sr ratios and high Sr contents (600–1,800 ppm). Although these minerals have low Rb/Sr ratios, the Ca/Sr ratios are high, which could result in potential interferences from Ca (see below).

Other materials that have been ablated and analysed successfully are clinopyroxene, magmatic carbonates (Sr-rich >3,000 ppm and Rb-poor <1 ppm, [15]), apatite and melt inclusions from relatively Sr-rich alkaline melts. These materials generate several types of isobaric interferences which will be discussed below.

The highest Rb/Sr ratios materials were analysed by Jackson and Hart [21]. In their study they analysed basaltic melt inclusions with Rb/Sr ratios of up to 0.14, although the reported external reproducibility of the ⁸⁷Sr/⁸⁶Sr is about 5 times worse than that of a melt inclusion with a Rb/Sr of 0.04.

Factors influencing the data quality of Sr isotope analysis by LA-MC-ICPMS

The following factors influence the quality of data which can be obtained by LA-MC-ICPMS: (1) counting statistics, (2) blank levels, (3) instrumental mass discrimination and laser-induced elemental and isotopic fractionation and (4) molecular interferences.

Counting statistics

The precision which can be obtained by Sr isotope analyses by LA-MC-ICPMS depends upon the number of ions counted. The number of ions that can be collected by the Faraday cups depends on (1) the amount of Sr in the sample (see Fig. 2), (2) ablation spot size, ablation rate (energy density and pulse rate), (3) the laser ablation efficiency (e.g. the particle size distribution of ablated material), (4) transport from sample chamber to plasma and (5) tuning conditions of the plasma and mass spectrometer.

The amount of Sr brought to the plasma is unclear in most publications. Davidson [12] reported that approximately 10 ng of Sr is needed for a 3-V beam of ⁸⁸Sr. The theoretical best possible precision for such beam intensity is then 23 ppm.

Background (blank levels)

The instrumental background levels for ⁸⁸Sr and ⁸⁵Rb that have been reported are 0.5–5.0 mV ([14, 17], see Table 1). Since running samples spiked with ⁸⁷Rb and ⁸⁴Sr resulted in non-natural background isotopic composition of these isotopes, Waight et al. [14] concluded that the Sr and Rb background is an accumulation of material from samples within the introduction system. It is important to note that these backgrounds are significantly higher than those reported for Pb and Hf isotopes, and that careful monitoring of the background is clearly vital to obtain accurate results. In nearly all studies the blank is measured by collecting data with conditions as during the analysis, except for not firing the laser. Only in two studies [13, 21] are baselines measured “off-peak” as with a standard TIMS analysis.

Instrumental mass discrimination and laser-induced elemental and isotopic fractionation

Both the laser system and the MC-ICPMS induce isotopic fractionations. The isotope fractionation in the MC-ICPMS is generally referred to as instrument mass discrimination. In this case the measured elemental or isotope ratio are different from the “true” ratio due to differences in ionisation potentials, space charge effects, effects of the matrix of the sample, preferential transmission of one type of ion, and reactions in the ICP (e.g. oxide formation). The instrument mass discrimination is generally corrected during solution MC-ICPMS analyses by using a stable isotope pair, which can be corrected by an exponentional law (similar to TIMS mass fractionation correction), or when there is no stable isotope pair available (e.g. Pb isotopes), corrected using a standard-sample bracketing method [30].

In addition to the instrument mass discrimination, it is now well established that during the ablation process of a sample, both isotopic and elemental fractionations are induced. The best studied elemental fractionations have been reported for U/Pb, for which an increase of the U/Pb ratio by a factor 2 during a single ablation has been reported (e.g. see [24, 31]). Laser-induced isotopic fractionation has also been reported, e.g. for Fe isotopes [32].

Both the laser-induced isotopic fractionation and the instrument mass discrimination can be corrected for during Sr isotope analyses by using the stable ⁸⁶Sr/⁸⁸Sr ratio and an exponential correction [30]. All 11 LA ablation studies have done so, except one [13] in which no mass bias correction was applied to the data.

Elemental fractionation induced by the laser is another important factor. The Sr isotope ratios can be internally normalized to ⁸⁶Sr/⁸⁸Sr, but correcting for the ⁸⁷Rb interference on the ⁸⁷Sr (see below) is complicated by the fractionation of the Rb/Sr ratio. In seven of the published studies it is assumed that the laser-induced isotope fractionation and instrument mass discrimination are the same for both Sr and Rb. This is possibly not the case, as suggested by the study of Jackson and Hart [21] who employed a standard-sample bracketing method to establish the total Rb laser-induced fractionation and instrument mass discrimination, in order to cope with large Rb corrections (see below).

Similarly, most authors make the Kr corrections on the Sr masses by assuming that the laser-induced isotope fractionation and instrument discrimination are the same for Sr and Kr. For a good Kr correction, the mass bias has to be established in an independent way. We will use the term mass bias to describe both the laser-induced isotope fractionation and the instrument mass discrimination.

Molecular interferences

Measurement and correction of the interferences of elements and molecules on the Sr masses (Table 2) is perhaps the most challenging aspect of Sr isotope analyses by MC-ICPMS. This is especially true when comparing LA analysis with solution work, where some of the interfering elements (e.g. Ca, Rb and the REE) can be removed by chromatographic purification of the sample or “burnt off” by heating at temperatures below that required for Sr ionization on a TIMS instrument. Unfortunately, for LA-MC-ICPMS Sr isotope analysis, large corrections for interfering elements need to be made. Since these interferences can be more than half of the signal intensity on the Sr isotope mass, these corrections are large and the correction procedures need to be extremely rigorous if accurate and precise Sr isotope ratios are to be obtained.

Table 2.

Sr isotope masses and possible interferences in the mass region 82–89

Source of interference		Mass
		82	83	84	85	86	87	88	89
Sr				84Sr		86Sr	87Sr	88Sr
Kr		82Kr	83Kr	84Kr		86Kr
Rb					85Rb		87Rb
REE	Y								89Y
	Yb2+			168Yb2+	170Yb2+	172Yb2+	174Yb2+	176Yb2+
	Er2+		166Er2+	168Er2+	170Er2+
	Lu2+							176Lu2+
	Hf2+						174Hf2+	176Hf2+
Fe/Zn/Ga oxides	54Fe					54Fe16O2	54Fe16O17O	54Fe16O18O
								54Fe17O2
	56Fe							56Fe16O2
	66Zn		66Zn17O
	67Zn		67Zn16O
	68Zn			68Zn16O	68Zn17O	68Zn18O
	70Zn					70Zn16O	70Zn17O	70Zn18O
	69Ga					69Ga17O	69Ga18O
	71Ga						71Ga16O	71Ga17O
Ca dimers			40Ca43Ca	40Ca44Ca	42Ca43Ca	40Ca46Ca		40Ca48Ca
						42Ca44Ca		42Ca46Ca
						43Ca2	43Ca44Ca	44Ca2
Ca argides			43Ca40Ar	48Ca36Ar
				46Ca38Ar		48Ca38Ar
				44Ca40Ar		46Ca40Ar		48Ca40Ar
Ca-P							40Ca31P16O

There are many potential interfering elements and molecules on the Sr isotope spectrum, most of which have been extensively discussed by Ramos et al. [17]. This study concluded that careful monitoring and correcting for the interferences can produce Sr isotope data with a precision suitable for use in most geological applications. In the next section we discuss the main interferences and how they can compromise Sr isotope data, leading to errors in excess of those that can be tolerated for everyday applications.

Rubidium

Rb is a well-known interference on ⁸⁷Sr during TIMS analyses (e.g. see [33]). The presence of a significant Rb signal can hamper TIMS and solution- and LA-MC-ICPMS analyses of Sr isotopes. Whereas with TIMS and solution MC-ICPMS work, the Rb can be separated from the Sr by chromatographic techniques, this is obviously not possible during LA analysis.

All LA-MC-ICPMS studies (see Table 1) use the peak stripping method for the Rb correction, by measuring the ⁸⁵Rb signal on mass 85 and subtracting the ⁸⁷Rb signal from ⁸⁷Sr using the ⁸⁵Rb/⁸⁷Rb value of 2.58745 [33]. An additional problem with LA-MC-ICPMS analysis is the unknown mass bias of Rb during the analysis. Most studies (see Table 2) assume that the mass bias of Rb and Sr (derived from the ⁸⁸Sr/⁸⁶Sr ratio) is constant and correct the Rb interference using the Sr mass bias. However, Jackson and Hart [21] deployed a different strategy to correct for high Rb/Sr alkaline melt inclusions. The mass bias of Rb was measured by sample-standard bracketing natural basalt glasses with sample material. The measured ⁸⁵Rb/⁸⁷Rb was than used to correct the mass bias of the melt inclusions measured between successive glass standards. By determining a more accurate Rb mass bias they were probably in a better position to measure high Rb/Sr material, although the reproducibility of their isotopic data deteriorated with increasing Rb/Sr. A similar approach was followed by McCulloch et al. [20].

Unfortunately, Jackson and Hart [21] did not report measurement of standards of known Rb and Sr isotopic composition, so it is difficult to fully assess the accuracy of their method. There is no consensus as to how high the Rb/Sr ratio of materials can be analysed to still produce a reliable LA analysis. Ramos et al. [17] proposed that the materials should have Rb/Sr < 0.002, whereas Jackson and Hart [21] and Davidson et al. [12] report successful corrections of materials with Rb/Sr of 0.14 and 0.2, respectively.

Krypton

The noble gas krypton interferes with masses ⁸⁴Sr and ⁸⁶Sr and is therefore an important interference that needs to be corrected. The source of the krypton is the argon (and helium) gas used to transport the sample into the plasma, and to generate the argon plasma (see Fig. 1). The amount of krypton in the argon gas is supplier dependent. Studies that report the magnitude of the Kr interference indicates a total Kr contribution between 20 mV [18] and 40–50 mV ([14, 17, 21]). Woodhead et al. [18] observed that Ar derived from compressed gas cylinders gives a more stable Kr signal than those from liquid Ar supplies. They further noted that the Kr abundances in the Ar supply vary largely between different batches. It is important to note that Jackson and Hart [21] suggest that there are also isobaric interferences of unknown origin on the ⁸³Kr peak, making a good Kr correction extremely difficult (see Table 2). In addition, as with Rb it is impossible to monitor directly the mass bias of the Kr isotope ratios during an analysis if there are isobaric interferences on ⁸²Kr or ⁸³Kr, so sample-standard bracketing may be required.

Four different correction methods have been deployed to correct for the Kr interference:

1. “Gas blank” or “on peak zero” [11, 12, 14–20]. This method measures the Kr levels as a blank measurement, with the He flow going through the sample chamber, but without ablating the sample (laser shutter closed, or no laser firing). The advantage of this method is that there is no mass bias correction involved. The gas blank also corrects for minor amounts of Sr and Rb on masses 84, 85, 86, 87 and 88 which are thought to be derived from the sample introduction system (see above).

2. Peak stripping. In this case the ⁸³Kr (or ⁸²Kr) signal is used to calculate the ⁸⁴Kr and ⁸⁶Kr corrections on mass ⁸⁴Sr and ⁸⁶Sr, respectively. Christensen et al. [11] used this method to correct for the ⁸⁶Sr/⁸⁸Sr and ⁸⁷Sr/⁸⁶Sr ratios. However, they do not report how they performed the mass bias correction on this ratio, but instead used a ⁸⁶Kr/⁸³Kr ratio of 1.53, which is significantly higher than the natural ratio of 1.5 [34]. To date there is no report in the literature of a method to calculate the mass bias of Kr from the ⁸²Kr/⁸³Kr ratio, which should be possible provided there are no isobaric interferences on ⁸²Kr or ⁸³Kr.

3. Waight et al. [14] used the on-peak-zeros to correct for the Kr interference, and subsequently correct any remaining Kr interference by the peak stripping technique by monitoring the ⁸³Kr signal.

4. Jackson and Hart [21] monitored both the ⁸²Kr and the ⁸³Kr and observed deviations of up to a factor of 2 in the ⁸³Kr/⁸²Kr (ca. 1). Therefore, these authors deployed another technique to correct for Kr on the Sr masses which uses the most abundant Kr isotope at mass 84 (abundance 57%) instead of ^82,83Kr. This mass has a major ⁸⁴Sr “interference” that can reach 35–75% of the signal. Correction is made by subtracting ⁸⁴Kr from the 84 signal until the internationally accepted ratio of 0.00675476 is obtained for the ⁸⁴Sr/⁸⁸Sr ratio [34]. Iterations are necessary for the mass bias correction (e.g. substitute the Kr number in the ⁸⁶Sr/⁸⁸Sr ratio, and repeat the calculations until there is no change in the calculated isotope ratios). This technique will only work if there are no other interferences involved on masses 84, 86 and 88. A significant disadvantage of this Kr correction technique is that the ⁸⁴Sr/⁸⁶Sr ratio cannot be used to check if the interference corrections on the ⁸⁷Sr/⁸⁶Sr ratio were successful by providing an independent check that the ⁸⁴Sr/⁸⁶Sr obtained from the analysis is the same as the internationally accepted value of 0.056500.

In summary: there are several ways to correct for Kr on masses 86 and 84. The most widely applied technique is the on peak zero, which seems to be successful for low Rb/Sr (<0.1) ratios. The method published by Jackson and Hart [21] is the only one reporting Sr isotope analyses on high Rb/Sr (>0.1) samples.

Calcium dimers and argides

When ablating sample material with high Ca/Sr ratios (e.g. marine carbonate, ca. 500 and plagioclase, ca. 50–200) calcium dimer and calcium argides can be formed. Ca dimers have been reported to be interfering molecules during SIMS analyses [35]. Waight et al. [14] and Bizarro et al. [15] were the first to suggest that Ca argides (e.g. ⁴⁴Ca⁴⁰Ar) could interfere with the Sr isotope masses for materials with high Ca/Sr ratios. The published studies on Sr isotopes are confusing regarding Ca dimer and argides. Ramos et al. [17] conducted solution work using NIST SRM-987 doped with Ca in such a way that the Ca/Sr ratios varied between 50 and 550. They did not observe a change of the ⁸⁷Sr/⁸⁶Sr outside the reported error. In addition, Jackson and Hart [21] did not find a change in the ⁸⁷Sr/⁸⁶Sr ratio outside analytical error in alkali basalts with Ca/Sr ratios of ca. 150. In contrast, Woodhead et al. [18] reported Ca argide and Ca dimer signals of approximately 100 mV for all Sr masses during the ablation of carbonates. This study also reports that the influence on the accuracy of the ⁸⁷Sr/⁸⁶Sr ratio is beyond the levels of within-run precision. In addition, Woodhead et al. [18] also reported that the ⁸⁴Sr/⁸⁶Sr ratio can be significantly modified by Ca dimer and argides when ablating carbonates, increasing the ratio from 0.0565 to 0.0575. They successfully corrected the interferences on mass 84, 86 and 88 by monitoring the ⁴²Ca⁴⁰Ar/⁴²Ca⁴⁰Ca peak on mass 82. Peak stripping resulted in the correct ⁸⁴Sr/⁸⁶Sr ratio, because the relative isotopic abundances of these argide and dimer molecules are very similar. Therefore, it is not necessary to know the correct argide/dimer ratio. It is important to note that Jackson and Hart [21] reported significant variations in the ⁸²Kr/⁸³Kr which they attributed to interferences. Ca argides and dimer appear a probable source of such interferences.

REE

The rare earth elements (REE) can interfere on the Sr isotope masses and on interfering element masses as doubly charged ions (see Table 2). The formation of doubly charged REE in an Ar plasma depends on the operating conditions (e.g. RF power, gas flows), but is generally in the order of 1–2% (e.g. see [17]). The REE interferences of course depend on the material analysed. For recent marine carbonates with low absolute REE abundances (<10 ppm total REE) the effect can be neglected, but for clinopyroxene, with significant REE abundances (Dy ca. 3 ppm, Yb ca. 2 ppm), it could be a potential problem (e.g. see [17]).

Waight et al. [14] and Ramos et al. [17] were the first to study the interferences of Er and Yb in detail. For Sr isotope analyses ¹⁶⁸Er²⁺ and ¹⁷⁰Er²⁺ are relevant, because these ions interfere with ⁸⁴Sr and ⁸⁵Rb, respectively (Table 2). With an increasing Er interference on mass 85 the ⁸⁷Sr/⁸⁶Sr ratio will decrease due to an incorrect Rb correction [17]. The net effect is that the amount of ⁸⁵Rb on mass 85 is overestimated, and too much ⁸⁷Rb will be subtracted from the 87 ion current, resulting in a low ⁸⁷Sr/⁸⁶Sr ratio. In a similar way, an increasing interference of Er will result in a higher ⁸⁴Sr/⁸⁶Sr ratio, because the Kr correction on the 86 mass is not correct. The signal on mass 84 is assumed to be ⁸⁴Sr, and therefore the measured ⁸⁴Sr/⁸⁶Sr ratios will be too high due to the presence of ¹⁶⁸Er²⁺.

Another potential interfering REE on the Sr isotope masses is Yb (see Table 2). Yb has five isotopes which appear on masses 84, 85, 86, 87 and 88 as doubly charged ions. The ⁸⁷Sr/⁸⁶Sr and ⁸⁶Sr/⁸⁸Sr ratios will increase with increasing Yb content of the ablated material, whereas the ⁸⁴Sr/⁸⁶Sr ratio will decrease [17]. The correction for these interfering doubly charged REE can be done by monitoring half masses. Erbium can be accounted for by measuring ¹⁶⁷Er²⁺ at mass 83.5 during an analysis. Peak stripping with mass ¹⁶⁸Er²⁺ (mass 84) can then be done, using a natural Er solution prior to the Sr LA measurement to establish the Er mass bias. The mass bias can also be established by measuring the ¹⁷¹Yb²⁺/¹⁷³Yb²⁺ ratio on masses 85.5 and 86.5 and assume that the mass bias for Yb is equal to that of Er [17]. The Yb corrections can be deployed by using the ¹⁷¹Yb²⁺ abundance and mass bias from the ¹⁷¹Yb/¹⁷³Yb ratio. Ramos et al. [17] also examined if Hf (e.g. ¹⁷⁶Hf²⁺) interferes with the Sr isotope masses, but did not find a significant contribution.

Zn, Ga and Fe

Schmidberger et al. [16] did not observe correlations between the FeO content and Sr isotope ratios of ablated clinopyroxenes. Iron can potentially generate two isobaric interferences (⁵⁴Fe³²O₂ = 86, ⁵⁶Fe³²O₂ = 88), which are according to Schmidberger et al. [16] eliminated by the collision cell in the Isoprobe instrument. Ramos et al. [17] only reported a limited effect of Fe oxide on the Sr isotope ratios, and did not correct for Fe oxide interferences.

Ga and Zn oxides (e.g. ⁷¹Ga¹⁶O⁺ and ⁶⁸Zn¹⁶O⁺) could also interfere with the Sr isotope masses. However, Ramos et al. [17] did not find significant deviations in solution work with Sr/Ga (10–20) and Sr/Zn (1–5) ratios typical of geological materials, and decided not to correct for these potential interferences during laser ablation work.

Calcium phosphates

Due to the large interference of ⁴⁰Ca³¹P¹⁶O on ⁸⁷Sr during LA-MC-ICPMS (see Table 2), it has become apparent that in situ analysis of biogenic phosphates and apatite is extremely challenging and currently does not yield accurate results (e.g. see [36, 37]).

Proposed solutions to correct for isobaric interferences

From the above discussions of the interferences on the strontium masses (see Table 2) it is clear that the order in which the interference corrections are applied is very important. Nearly all interference corrections interact with other corrections. For example the Rb correction is important for the ⁸⁷Sr, but is also influenced by the Yb correction. In some cases iterations of calculations are necessary to minimize the errors on the calculations. In Fig. 3. the order of corrections is given for the eleven published Sr LA-MC-ICPMS procedures. The correction routines can be divided in two groups: (I) Kr (gas blank), Rb, Sr mass bias correction and (II) the Jackson and Hart [21] method with Kr correction using ⁸⁴Kr and Rb mass bias correction by sample standard bracketing.

Fig. 3.

Order of interference corrections in the eleven publications concerning Sr isotope analysis by LA-MC-ICPMS. **Not mentioned in publication, but inferred from published isotope ratios. See text for discussion

The group I correction method in its most simple form has been deployed by Christensen et al. [11] and involves Kr correction by ⁸³Kr peak stripping, ⁸⁵Rb peak stripping and subsequently normalization to ⁸⁶Sr/⁸⁸Sr ratio of 0.1194 (see Fig. 3). The more elaborate correction methods of group I [17, 18] include corrections for the tail, REE [17] and Ca argide/dimers [18]. The group I data reduction method does work for the relatively simple matrix of carbonate material and plagioclase. The group II method from Jackson and Hart [21] is unique, and has as the major advantage that the Rb correction is done very precisely, resulting in the possibility to analyse higher Rb/Sr materials (see above), but more standards should be analysed to fully validate this approach.

Precision and accuracy of Sr isotopes by laser ablation

From the above discussion it is obvious that LA-MC-ICPMS will probably never obtain the 5- to 10-ppm precision and accuracy which are possible with state-of-the-art TIMS (e.g. see [38]). Therefore, LA-MC-ICPMS will not replace microdrilling of sample material, dissolution and chromatographic processing in a clean laboratory environment and measurement by TIMS when high-precision analysis are required in sample materials with limited variation in Sr isotopes (see [10]). However, the microdrilling/TIMS technique is very time consuming, and requires a significant skill from the operator. Measurement of Sr isotopes by LA-MC-ICPMS, on the other hand, has great potential if the precision and accuracy required is in the order of 50 ppm, with the additional advantage of reduced analysis time (average of <5 min, including wash out and standard analysis, compared with >100 min for the TIMS analysis).

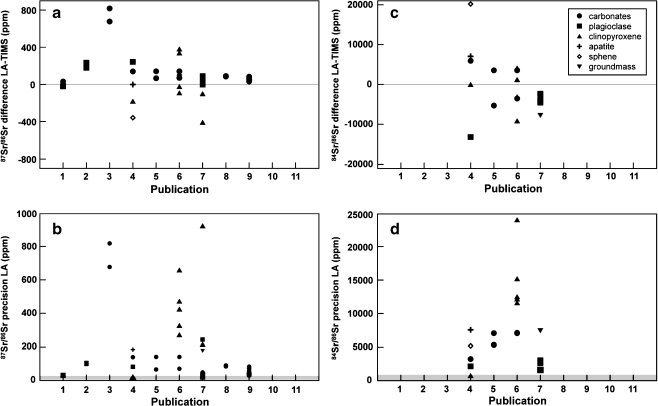
In Fig. 4 the precision and accuracy are reported for the eleven Sr isotope studies by LA-MC-ICPMS for which data were available. The precision is controlled by the counting statistics that are mainly controlled by the concentration of Sr in the ablated material. From Fig. 4 it is obvious that due to their high Sr contents, calcium carbonate and plagioclase provide the best precision. The precision for carbonate material is better than 150 ppm for six laboratories that have published data on marine carbonate. The precision for other materials can be significantly worse, for example for many clinopyroxenes the precision is between 300 and 1,000 ppm. Plagioclase also gives good results with a precision better than 250 ppm in four studies.

Fig. 4.

Difference between laser ablation and TIMS in ppm (⁸⁷Sr/⁸⁶Sr_LA−⁸⁷Sr/⁸⁶Sr_TIMS)/⁸⁷Sr/⁸⁶Sr_TIMS×10⁶) for ⁸⁷Sr/⁸⁶Sr (a) and ⁸⁴Sr/⁸⁶Sr (c) and the precision (2sd/average)×10⁶ for laser ablation analysis (in ppm) for ⁸⁷Sr/⁸⁶Sr (b) and ⁸⁴Sr/⁸⁶Sr (d). Publications 1–11=[11–21], respectively. Typical TIMS precision (in ppm) is represented by the grey shaded area (e.g. see [38])

The accuracy of the data is more important than the precision. The accuracy, expressed in ppm deviations of ⁸⁷Sr/⁸⁶Sr from reported values, is given in Fig. 4. The accuracy is calculated for samples where LA data and chromatographically cleaned samples measured by TIMS or solution MC-ICPMS were given. Some interesting conclusions can be made:

1. Carbonate material gives the most accurate results: data are within 150 ppm of the solution MC-ICPMS and TIMS values. The good results for carbonate material are to be expected, since corrections for the REE and Rb are minimal in this material. The good accuracy also suggests that the Ca dimer and argides are probably not a significant problem for carbonate material, since a correction for these interferences was actually performed in only one study [18]. However, this is a question that needs to be explored in greater detail in future studies. Another observation is that all the LA-MC-ICPMS carbonate data are shifted towards higher ⁸⁷Sr/⁸⁶Sr compared with TIMS and solution MC-ICPMS data (Fig. 4a).

2. Plagioclase tends to result in ⁸⁷Sr/⁸⁶Sr ratios that are higher than TIMS or solution MC-ICP-MS values. The plagioclase samples of Ramos et al. [17] and Christensen et al. [11] are closest to the TIMS/solution-MC-ICPMS value, whereas those of Waight et al. [14] and Davidson [12] are 150–250 ppm too high. The most likely cause for this discrepancy is the significant Rb correction for this material (especially those of Davidson [12], since these samples are artificially enriched in Rb).

3. In contrast, clinopyroxene results tend to be lower than the published TIMS/solution MC-ICPMS results. This is true in the work reported by Waight et al. [14] and Ramos et al. [17], but the clinopyroxene ⁸⁷Sr/⁸⁶Sr accuracy published by Schmidberger et al. [16] is highly variable (accuracy varies between −408 and 382 ppm). The large range in the accuracy of clinopyroxene is probably partly caused by the significantly lower Sr content of this material compared with plagioclase and carbonate material (see Fig. 4b–d). The variation in precision is up to 900 ppm, a range that is also observed in the accuracy (ca. 800 ppm). In addition, clinopyroxene needs significant corrections for Rb and the REE, hampering both the precision and accuracy.

4. Sr isotopes by laser ablation analysis of other geological materials also looks promising (e.g. groundmass), whereas apatite and sphene require such large corrections that accurate results will be difficult to obtain.

Unfortunately, not all studies published so far have reported the ⁸⁴Sr/⁸⁶Sr ratio. From the five studies who did measure this ratio, the results from Woodhead et al. [18] are only presented in a figure. A summary of the data from the other four studies is shown in Fig. 4c and d. As can be expected from the significant Kr corrections, there are large deviations from the “true” value of 0.0565 (e.g. see [38]). Results from clinopyroxene display a large variation of ca. 14,000 ppm, but the same is true for carbonate material (ca. 12,000 ppm). Sphene again has the largest deviation from the true ⁸⁴Sr/⁸⁶Sr value, suggesting problems with corrections of Ca dimer and/or argides. We recommend that ⁸⁴Sr/⁸⁶Sr ratios are routinely reported as an indicator for the quality of the Sr isotope analysis and that greater effort is made to account for Ca dimer and argides.

Future directions

All published studies so far have demonstrated the potential of Sr isotopes by LA-MC-ICPMS. As discussed above the precision and accuracy of Sr by LA-MC-ICPMS is clearly limited by the corrections involved with this technique. Some of these are technique dependent (such as the Kr corrections), whereas others depend on the sample material analysed (e.g. Rb, REE and Ca dimer). Some of the potential developments for this technique are evaluated below:

1. Laser systems. In the past decade there has been a tremendous effort to improve laser design and shorter wavelength lasers are now being used [25]. The net effect of using shorter wavelength lasers is the more efficient volatilization of the sample which produces smaller aerosol particles in the plasma that are more efficiently ionized (e.g. see [26]). This development was partly driven by the effects of trace-element fractionation during laser ablation of materials where the measurement of elements with different volatility was hampered if large aerosol particles were produced, for example U/Pb dating of zircons [24]. Since corrections for isobaric interferences on Sr isotope masses are so large (compared with TIMS) the assumption that Rb instrumental discrimination and laser-induced elemental fractionation are the same as for Sr is probably not true. Lasers with low wavelength (e.g. 193 nm) will reduce this elemental and isotopic fractionation. However, the mass-bias contributions by instrumental mass discrimination and laser-induced elemental fractionation need to be determined independently.

2. Careful determination of the Rb instrumental discrimination by introducing a Rb standard aerosol through a desolvating nebulizer to correct for instrumental discrimination may help. However, probably the best solution would be that followed by Jackson and Hart [21] by measuring Rb-bearing standards with the laser in a standard-sample bracketing method.

3. The Kr interference can be reduced by using clean sources of (liquid) Ar. It could be useful to experiment with Kr removal techniques, in a similar manner as suggested by Zuzel et al. [39].

4. The interferences of the REE are probably only important for materials that contain significant amounts of REE. Experimenting with optimal plasma conditions, which reduce the creation of doubly charged REE, is important.

5. The situation with Ca argides and dimer is unclear. More experimental work is needed, especially by ablating Ca-rich materials, which do not contain Sr.

Conclusions

The eleven publications reporting on strontium isotope analyses by LA-MC-ICPMS all conclude that it is a very powerful technique which is feasible, although it does have significant limitations, depending mainly on the material ablated. So far low Rb/Sr ratios have proved essential for a good analysis. Successful corrections for Rb have been reported for values up to 0.05. This suggests that carbonate and plagioclase represent reliable materials for analysis, but the analysis of high Rb/Sr minerals such as mica and biotite are not currently possible and will remain so unless there is a significant improvement in the way that the Rb correction on ⁸⁷Sr is performed. Higher Rb/Sr materials have been ablated using a standard-sample bracketing technique for correct Rb corrections with, to date, limited success.

Correction of Kr interferences on Sr masses was reported not to be a major problem in the eleven studies published to date; however, the accuracy and precision of the data reported so far do not fully substantiate this conclusion. Different strategies are followed to correct for Kr interference, in which gas blank subtraction seems to be the most preferable. Further work is required to determine the instrumental mass discrimination of Kr to ensure the correct ⁸⁷Sr/⁸⁶Sr and ⁸⁴Sr/⁸⁶Sr ratios are obtained.

Doubly charged REE interferences are only a problem in materials where significant REE contents are present, such as clinopyroxene. However, successful correction is possible by collecting data at half masses.

The influence of Ca dimer and Ca argides, is unclear, and needs more investigation. It appears that the effect is limited on the ⁸⁷Sr/⁸⁶Sr ratio, but for ⁸⁴Sr/⁸⁶Sr corrections are necessary. Given that monitoring ⁸⁴Sr/⁸⁶Sr is a good way to establish the veracity of the data, these corrections should be performed routinely.