Evolution of frequency-dependent mate choice: keeping up with fashion trends

Abstract

The diversity of sexual traits favoured by females is enormous and, curiously, includes preferences for males with rare or novel phenotypes. We modelled the evolution of a preference for rarity that yielded two surprising results. First, a Fisherian ‘sexy son’ effect can boost female preferences to a frequency well above that predicted by mutation–selection balance, even if there are significant mortality costs for females. Preferences do not reach fixation, however, as they are subject to frequency-dependent selection: if choosy females are too common, then rare genotypes in one generation become common, and thus unattractive, in the offspring generation. Nevertheless, even at relatively low frequency, preferences maintain polymorphism in male traits. The second unexpected result is that the preferences can evolve to much higher frequencies if choice is hindered, such that females cannot always express their preferences. Our results emphasize the need to consider feedback where preferences determine the dynamics of male genotypes and vice versa. They also highlight the similarity between the arbitrariness of behavioural norms in models of social evolution with punishment (the so-called ‘folk theorem’) and the diversity of sexual traits that can be preferred simply because deviating from the norm produces unattractive offspring and is, in this sense, ‘punished’.

1. Introduction

The evolution of female choice for male traits that signal only breeding value for fitness still raises challenges for sexual selection theory (Kokko et al. 2006). Mating preferences for these additive genetic benefits are self-defeating unless countered by other forces: if many females express the same preference, then preferred genes are rapidly driven towards fixation. As additive genetic variation among males declines, the benefits of choosiness vanish (the lek paradox: Kirkpatrick & Ryan 1991; Blows et al. 2004; Tomkins et al. 2004), and there is selection against costly choice (Lande 1981). There are, however, also cases where mate choice favours genes with non-additive effects on offspring fitness (Jennions & Petrie 1997; Neff & Pitcher 2005). For example, numerous studies have demonstrated mate choice for inbreeding avoidance (Amos et al. 2001; Tregenza & Wedell 2002; Lehmann et al. 2007), others show that choice acts to optimize offspring heterozygosity (e.g. Penn & Potts 1999; Reusch et al. 2001) and a few indicate that genetically incompatible mates are avoided due to strong fitness effects associated with deleterious maternal–paternal genetic combinations at specific loci (Zeh & Zeh 2003). Different females preferring different traits reduce directional selection on specific male genotypes and might partly explain the ongoing heritability of sexually selected traits (for a critique see Blows & Hoffmann 2005).

Surprisingly, in a few species, females prefer males with rare (or novel) rather than specific phenotypes (guppies, Poecilia reticulata: Hughes et al. 1999, Eakley & Houde 2004; fruitflies, Drosophila sp.: Singh & Sisodia 2000). This is potentially a mating preference for genetic benefits that does not readily fit into the familiar scenario described previously. It makes it implausible that female choice drives preferred genes to fixation even if the preferred genes have an additive effect on fitness. This is because rare genotypes do not remain rare in the presence of a preference for rarity. Surprisingly, the evolution of a female preference for rarity has not been formally modelled, despite the ‘rare male advantage’ featuring prominently in several widely read early reviews of mate choice evolution (e.g. Partridge & Halliday 1984).

For mate choice for additive genetic benefits to evolve, both female mating preferences and male traits have to persist at equilibrium. A key criterion for all models is the maintenance of genetic variation in male traits (Cameron et al. 2003; Kokko et al. 2006). With a female preference for rarity, the explanation is seemingly straightforward. Rarer male types have greater fitness due to their elevated mating success that leads to negative frequency-dependent selection. Frequency-dependent selection can readily maintain polymorphism in populations in a wide range of circumstances (Sinervo & Calsbeek 2006). Indeed, it is often invoked in studies of alternative mating strategies, such as female mimicry in isopods (Shuster & Wade 1991) and territoriality versus satellite behaviour in lizards (Sinervo & Lively 1996). At equilibrium, however, the frequency of each mating strategy is such that fitness is identical. When the male trait that increases fitness is rarity, this implies that, all else being equal, at equilibrium each male type will be equally common. If true, rarity would vanish. This then begs a question: can a female preference for rarity persist if rarity does not? The answer would seem to be no, but brief reflection on Fisher's original argument for selection maintaining equal sex allocation reminds us that fluctuations from equal proportions of male types could suffice to maintain the female preference.

A mating preference for rarity could, of course, be directly beneficial. For example, rare male phenotypes might be less susceptible to diseases (Lively & Dybdahl 2000), which, if carried, could be transmitted during mating, or less likely to attract predators that form a search image for common phenotypes (reviewed in Merilaita 2006; see also Olendorf et al. 2006), increasing predation risk for females in mating pairs (Pocklington & Dill 1995). There is also good evidence that rarity can signal non-additive genetic benefits. Guppies live in tropical streams and small populations become partially isolated as streams recede into pools in summer. This elevates the risk of inbreeding that, in turn, reduces fitness (van Oosterhout et al. 2003), but a female preference for rare male phenotypes increases the likelihood of outbreeding as male coloration is highly heritable due to strong Y-linkage (Brooks & Endler 2001). A rare colour pattern is therefore indicative of a recent, unrelated immigrant (Kelley et al. 1999). Even if these benefits exist, a preference for rarity, as with any established mating preference, could subsequently confer additive genetic benefits that might sustain it if the advantages it originally conferred disappear. For example, what will happen to a preference for rarity in guppies in larger rivers where inbreeding is unlikely?

Genetic benefits of choice are the outcome of multiple life-history traits that can covary positively or negatively (Kokko et al. 2006) and often have sex-specific effects (Fedorka & Mousseau 2004). By definition, the benefit that really matters is that net offspring fitness, averaged across offspring, is elevated and, as a result, the frequency of preference genes increases in the next generation. The two composite traits most often considered are offspring viability and sons' attractiveness (or, if multiple mating is taken into account, net fertilization success). Male attractiveness is a special trait in this context, because its value depends on the strength, direction and ubiquity of female preferences. If enhanced male attractiveness is the only benefit of choice, preference evolution is difficult, because the preference has to reach a threshold frequency in the population before a Fisherian process can ‘take off’ (Kokko et al. 2002).

A moment of introspection suggests, however, that preferences for rare male traits will evolve in rather unusual ways, whether or not rarity is linked to viability. If rarity confers a viability benefit to offspring (e.g. because predators lack a search image for uncommon prey; Merilaita 2006), it is conceivable, but not certain, that a preference for rare males will evolve. The mating preference could cancel the viability benefit if it causes previously rare (preferred) phenotypes to become common in the next generation; thus, it matters how long rare types remain rare in the population. Explaining the evolution of a mating preference when rarity only affects attractiveness is even more challenging (Cameron et al. 2003). The greater attractiveness females confer to their sons by mating with rare males is again dependent on how often rare genotypes are preferred (i.e. still rare) in the next generation. Preference genes should spread if they sufficiently often end up in the bodies of ‘sexy sons’ (the ‘sons effect’ sensu Parker 2006). Whether this will occur in the context of rarity is a non-trivial question, because rarity is an elusive trait. Rare genotypes are destined to become common if they are preferred, and any ‘sexiness’ benefit based on rarity is ephemeral. So a key question is whether preference alleles are still found relatively more often in sexy sons when the preferred male type keeps changing. Our model will address this question, assuming that viability benefits of being rare are either absent or present.

2. The model

Here, we use individual-based simulation models to investigate how female mating preferences for rare male traits evolve. We determine how this process is influenced by the presence or absence of a relationship between rarity and survival. We then consider the effect of temporal (and potentially frequency dependent) variation in male survival prospects. Finally, we ask what happens when females are less often able to convert mating preferences into actual mate choice. Our model is loosely based on findings in guppies, in which females prefer rare or novel male colour patterns (Farr 1977; Hughes et al. 1999; Eakley & Houde 2004) and for whom there is also recent evidence that males with rare colour patterns survive better, possibly as a result of predator search images for common types (Olendorf et al. 2006). The details of our model are provided in the electronic supplementary material. Here, we simply outline the main assumptions.

For simplicity (see chapter 2 in Kokko 2007 for justification), we assume a haploid species with a gene having k alleles that determine male phenotype but is not expressed in females (e.g. male colour morphs). This gene also potentially influences male viability (e.g. colour affects visibility to predators). There is also a choice gene such that females either have the preference allele or mate randomly. When k=1, all males are identical, so choosy and randomly mating females mate in an identical fashion. We use this state to determine the frequency of a costly preference (see below) under mutation–selection balance. Subsequently, when k>1, we use this frequency of the preference allele as a benchmark: when exceeded it indicates the cases where the preference allele is favoured. In all the cases, having a preference carries a cost, so that a fraction c of females with the preference allele die before they can breed.

We begin with a situation where there is no differential viability selection on males, thereby excluding frequency-dependent predation and similar processes (Nosil 2006; hereafter ‘no viability selection’). We then add two kinds of temporally varying viability selection on males. First, we examine frequency-independent temporal variation in viability selection, so that in each generation we randomly make several genotypes (we use k/2 for simplicity) suffer an extra mortality risk d_I (0<d_I<1, subscript I denoting independence of frequency); hereafter ‘frequency-independent viability selection’. This process introduces stochasticity in a biologically relevant fashion. Second, we examine ‘frequency-dependent viability selection’, where the extra mortality risk d_D (subscript D denoting dependency on frequency) affects only the most common genotype, as has been recently reported from experimental work on male coloration in guppies (Olendorf et al. 2006).

We investigate two types of preference that both involve each female independently sampling n males from the population to estimate phenotypic frequencies. If there is unhindered choice, females mate exclusively with either the rarest male type in their sample (hereafter ‘strict preference for rarity’) or any male type whose frequency lies below a threshold in their sample, say 5 or 10% (hereafter ‘threshold preference for rarity’). If two or more types are equally rare or both lie below the threshold, choice among these males is random. If no mate type is sufficiently rare, then females with a threshold preference mate randomly. Finally, we consider the effect of female choice being hindered (e.g. due to male interference or environmental constraints). Females with the preference allele mate with their preferred male types with probability y, otherwise they mate randomly with probability 1−y. If y=1, females always mate with their preferred male type. For each generation, there is a non-directional mutation rate μ between preference and random mating. In our simulations, we consider the cases with small values of mutation rate μ and cost c to increase the realism of the model.

In each population, the preference allele is originally either absent or fixed. Using these two starting points allows us to determine the influence of initial mate choice after T generations (T≥2500). If there is none, then the two population types will converge on a similar outcome. In each generation, N newborn offspring are randomly picked to form the next generation, thereafter viability selection is applied. We ran 50 replicate populations for each scenario and recorded the frequency of the preference allele after T generations. Instead of presenting test statistics, we used a high number of replicates, so that the 95% CIs for evolved frequencies become narrow enough to allow accurate assessment of the changes in preference frequency arising under different conditions (Colegrave & Ruxton 2003).

3. Results

(a) Simple mutation–selection balance

In an infinite population, a viability cost c that is only expressed in females and an unbiased mutation rate μ between preference and random mating translate to a mutation–selection balance equilibrium for the preference allele of (Falconer & Mackay 1996)

$$x=\frac{\mu }{2\mu +\frac{1}{2}c}.$$ (3.1)

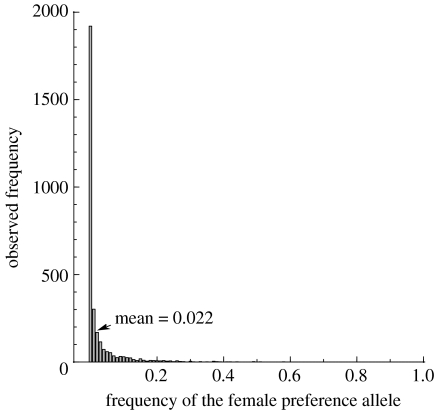
However, in a finite population, the frequency of the preference allele, x, is more often closer to either 0 or 1 due to drift. This is particularly relevant when x is small, thus the mutation–selection balance case for the preference allele was analysed numerically by running a single population with k=1 (i.e. no indirect selection on preferences) for 1.5 million generations. We started with a frequency of 0 and then sampled it every 500 generations. When c=0.01, the mean is 0.022 (histogram in figure 1). The means from this and similar analyses run with different values of c generate baseline frequencies against which preference evolution in various scenarios can be compared (see legend to figure 1).

Figure 1.

Mutation–selection balance histogram of preference frequencies when k=1, μ=0.0002 and cost c=0.01, obtained sampling a simulated population every 500 generations for 1.5 million generations. The mean of the distribution is 0.022. Repeating this procedure for other cost values gives the mean values 0.50 (for c=0), 0.46 (c=0.0001) and 0.35 (for c=0.001).

(b) Multiple male types

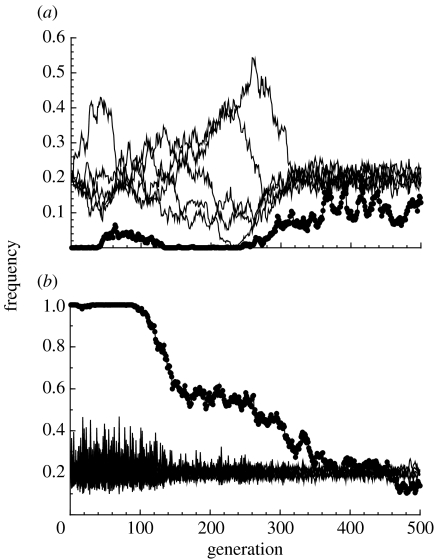
Figure 2 provides an illustrative example of preference evolution in the absence of viability selection on males. There are two populations, each with five male types, which differ only in whether the preference allele is initially fixed (figure 2a) or absent (figure 2b). The frequency of the preference allele stabilizes around the same equilibrium value in both populations in less than 500 generations. When the mating preference reaches even modest frequencies, it has dramatic effects on the relative frequency of male types. The initial random drift (‘red noise’; see Ripa & Lundberg 1996) of male types when the preference is uncommon is replaced by tight regulation (figure 2a, when T>300). Rare male types increase in frequency, common ones decline and, most importantly, polymorphism is maintained indefinitely. This regulation is overly strong in populations where the preference is initially fixed, so that the rarest male type is invariably the most common in the next generation (figure 2b, when T<100). This ‘spiky’ pattern of changes in the proportion of male types (‘blue noise’; see Ripa & Lundberg 1996) eventually dampens as the preference reaches equilibrium.

Figure 2.

Example trajectories of the frequencies of the female preference (dots) and male types (lines) when preferences are initially (a) absent or (b) fixed. The examples use strict preferences with no temporal variation in viability selection. Parameters used are N=1000, n=50, c=0.01, k=5, y=1 and μ=0.0002. The dynamics of male genotypes are characterized by drift when female preferences are absent and much tighter regulation when a fraction of females prefer rare males. Where preferences are fixed (i.e. up to generation 100 in (b)), the rarest male types are the most common in the next generation, leading to sharp fluctuations in male frequencies.

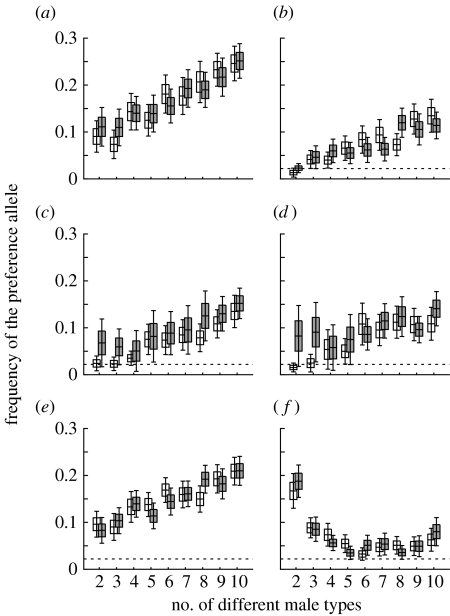
The preference frequency can be trusted to have reached values close to the true equilibrium within T generations, because the 95% CIs for the mean at generation T (T=2500 or 5000) always overlap between populations where the preference allele was initially either fixed or absent. This implies convergence such that the true mean is very unlikely to lie outside the values spanned by the two alternative CIs. As expected, based solely on varying c in equation (3.1), a costly preference reaches a lower equilibrium frequency than a cost-free one (compare figure 3a with b). The value of the equilibrium frequency is, however, far more closely associated with frequency-dependent indirect selection than the position of the mutation–selection balance equilibrium. The mutation–selection balance (calculated when k=1) predicts a value of 0.5 for a cost-free preference (no selection) and a far lower value of 0.022 for a costly preference (c=0.01, figure 1). In contrast, when indirect selection affects preference evolution (k≥2), equilibrium values are relatively insensitive to the direct costs of choice, instead they clearly increase with the number of male types (figure 3a,b). This implies that female preferences, when rare, yield indirect benefits that select for the preference, but when they become ‘too common’, they are selected against both directly (if there are costs) and indirectly. The fact that the equilibrium values are relatively insensitive to the direct costs suggests that the latter factor, frequency-dependent indirect selection, can be strong.

Figure 3.

Evolution of fully expressed (y=1) female preferences, with different numbers of male types k in the population. Data are presented as mean±s.e. (boxes) and ±95% CI for the mean (whiskers) for the frequency of the female preference allele after T generations. The expected frequency under mutation–selection balance is indicated by a dashed horizontal line (not visible in (a), where it is 0.5). It was numerically derived (figure 1). Populations started with either the preference allele absent (open boxes) or fixed (shaded boxes). (a) Strict preference for rarity with cost c=0 and no temporal variation in viability. (b) Strict preference for rarity with c=0.01 and no temporal variation in viability. (c) The same as for (b), but females have a threshold preference for rarity, mating with any male whose type frequency in her sample of n is below 5%. (d) The same as for (c), but the threshold frequency is 10%. (e) The same as for (b), but with frequency-independent viability selection using d_I=0.5. (f) The same as for (b), but with frequency-dependent viability selection, d_D=0.5. In all the examples, n=50, N=1000, μ=0.0002 and T=2500, except in (c) and (d) where T=5000 due to slower convergence between populations with different initial frequencies.

A strong signature of a frequency-dependent indirect fitness component can also explain why a preference is selected for at low frequencies and subsequently maintained at an equilibrium frequency that, in some cases, remains below the value predicted in the absence of indirect selection (figure 3a: no value reaches the theoretical prediction 0.5). This occurs when the case with no indirect selection also has no direct selection on the preference (true in figure 3a because c=0). The comparison performed is thus one of frequency-dependent indirect selection versus random drift, which explains the high frequency 0.5 predicted by the latter. When indirect selection is operating (k≥2), the evolutionary dynamics turns from favouring a preference (low x) to selecting against it (high x) much before the drift frequency x=0.5 is reached, thus explaining the low values of equilibria in figure 3a. This finding is general, however, only in the sense that frequency-dependent indirect selection can produce equilibria that are either above or below the value predicted in the absence of indirect selection, not in the sense that indirect selection would always decrease preference frequencies when considering cost-free cases (see below and figure 4 for counterexamples).

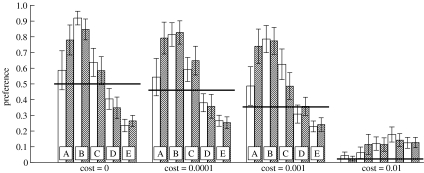
Figure 4.

The effect of changes in the fraction y of females that fully express their mating preferences on the frequency of the preference after 7500 generations. The mean and 95% CI are based on 50 replicates per scenario, and populations started with the preference allele either absent (open bars) or fixed (shaded bars). The black horizontal lines give the expected frequency under mutation–selection balance for a given cost c of the female preference. Cost is indicated on the x axis. The effect of a greater ability of females to express preferences can be seen by noting the trend from (A) y=0.1, (B) y=1/3, (C) y=0.5, (D) y=2/3 to (E) y=1. For the sake of visual clarity, these labels are omitted in the case where the cost c=0.01. Other parameter values are k=10, n=50, N=1000 and μ=0.0002.

For a costly preference, there is no discernible difference between the cases where choice involves a strict preference for the rarest male type sampled, or a rarity threshold (compare figure 3b with c,d), except that the equilibrium frequency is approached more slowly in the latter case (figure 3b: T=2500; figure 3c,d: T=5000). The more male types there are, the more clearly the estimated true means for preference frequencies exceed the mutation–selection baseline value of 0.022 (figure 1). Temporal variation in frequency-independent viability selection on males boosts female preferences to still higher frequencies (compare figure 3b–d with e). The exception to the rule that preferences evolve to higher frequencies when there are many male types (figure 3a–e) arises when there is frequency-dependent viability selection that only acts against the most common male type. This case yields a different outcome, where the equilibrium frequency of the preference allele is the largest with only two male types (figure 3f). The equilibrium frequencies with many male types are lower than those in the other scenarios (compare figure 3f with b–e). When only the most common phenotypes suffer a viability cost in populations with more than two male types, they form a smaller proportion of the population. Consequently, rare male phenotypes, that more often carry preference alleles, form a relatively greater proportion of the population, decreasing the skew in mating success. These findings remained robust when other types of viability correlations were examined, e.g. viabilities that covary linearly with their relative frequency (not shown).

There is a strong frequency-dependent selection on both males (figure 2) and females (figure 3). The frequency of a costly preference allele approaches a parameter-dependent equilibrium that clearly deviates from the one predicted by mutation–selection balance (figure 3b–f). The strength of frequency-dependent selection is also evident, in that populations approach the frequency-dependent equilibrium much faster when female preferences have an effect on offspring genotypes (k≥2) than when they do not (k=1): repeating calculations in figure 3 with k=1 (not shown) does not yield convergence by generation T, which is why longer time spans were used for figure 1. Frequency dependence means that both randomly mating and choosy females are selected against when they become too common. As indicated previously, the equilibrium is far more sensitive to this frequency-dependent selection than the null expectation of the mutation–selection balance. Despite the latter being 0.5 for a cost-free preference and only 0.022 for a costly one (c=0.01), the equilibria are very similar in our examples (compare figure 3a with b–f; the same trend emerges for parameter values other than those used in figure 3).

Finally, if female choice is constrained so that females sometimes fail to express their mating preferences (i.e. y<1), then, at least for moderate costly preferences, the equilibrium frequency is considerably higher than it is when preferences are always expressed (figure 4, compare A–D with E). The low values of y, meaning that many females end up mating randomly despite having preferences, can make preferences reach values close to fixation (figure 4, cases A–C for c≤0.001). However, it is notable that when choice is greatly hindered (e.g. y=0.1), it takes far longer for the preference to reach its equilibrium frequency (figure 4, case As have not converged by generation 7500 when c≤0.001). This is because selection cannot be strong when most choosy females cannot express preferences, and their actual mating behaviour is therefore indistinguishable from that of randomly mating females.

4. Discussion

Our model tracking the evolution of a female preference for rare male phenotypes yields two main findings. First, when females fully express a costly mating preference for rare males, the preference will initially spread or decline until halted by frequency-dependent selection. Although the equilibrium frequencies of female preferences are not high, they are sufficient to tightly maintain the polymorphism in male traits. Second, if females are usually prevented from expressing the preference, it can evolve to far higher frequencies and approach fixation. Neither finding was an immediately obvious and predictable outcome of the model, so we consider each in turn before reaching some general conclusions.

(a) Why is indirect selection on the mating preference frequency dependent?

Why are fully expressed mating preferences selected for when uncommon and against when common? If the preference is overly abundant, it causes a rare male phenotype to become the most common one in the next generation. Therefore, choosy females will more often produce sons with common phenotypes. This transmits preference alleles into evolutionary oblivion because choosy females' sons are strongly selected against: they are only accepted as mates by randomly mating females, who are themselves under-represented. This frequency-dependent selection makes it highly unlikely that a mating preference for rarity can ever reach fixation. On the other hand, if the mating preference is rare, it can spread owing to indirect selection due to the sons effect (Parker 2006). Males with rare phenotypes have a mating advantage that creates indirect benefits for choosy females, as long as choosiness is not so common that the superior mating success of rare males becomes strong enough to make their offspring common and thus unattractive. A preference for a trait as exotic as ‘rarity’ can therefore initially spread until it is expressed by a sizeable fraction of the female population. This explanation highlights the general importance of any feedback loop where female preferences influence the dynamics of male genotypes and vice versa (Lehmann et al. 2007).

Ultimately, a mating preference for rarity is maintained by negative, indirect frequency-dependent selection balanced between there being sufficient numbers of choosy females so that sons with rare phenotypes are sexy, but not so many that rare male phenotypes become common. At equilibrium, all male phenotypes are, on average, equally common. Strictly speaking, at such an equilibrium, females no longer gain indirect benefits by preferring rarer males, but in a real population this will rarely happen. To understand why mating preferences are nevertheless maintained, one needs to consider only Fisher's analogous argument for equal investment in the sexes. With equal investment, the fitness returns from both the sexes are the same if they are equally costly to produce. In a large population, it is then inconsequential whether a parent produces only sons or only daughters, but as soon as production of one sex becomes more common, it immediately pays more to produce the other sex. Likewise, any deviation from precisely equal proportions of each male phenotype—which is likely to occur in every generation of a finite population—means that some phenotypes will be rarer than others. Females with a mating preference for rarity then gain the indirect benefit of producing sexy sons. This indirect selection is typically stronger when there are more male types, because the potential rare male mating advantage is greater. For example, if k=3, the rarest male type that gains all the matings with choosy females must form less than 33% of the population; if k=5, this decreases to less than 20%. In other words, a rare type at less than 20% of the population is ‘sexier’ than a rare type at less than 33% of the population because fewer males share in matings with the choosy females.

Although in many Fisherian models the sexy son effect arises because the female preference covaries with a male trait (linkage disequilibrium), the fact that in our case the preferred trait is not a temporally stable phenotypic trait is not a problem. A consistent definition of the sexy son effect (Kokko et al. 2006) only requires that there is indirect selection that arises owing to a statistical association of the female preference with attractiveness of her sons. In our context, this association arises because preferences result in offspring whose genotypes are sufficiently often rare. As in models of culturally transmitted Fisherian mating advantages (Ihara et al. 2003; McNamara et al. 2003), the statistical association does not necessarily equal the linkage disequilibrium of classical Fisherian models (e.g. Lande 1981).

It is noteworthy that, despite incurring costs, mating preferences are maintained well above the level predicted by the mutation–selection balance. In classical Fisherian models, an additional mortality of 1% for choosy females (c=0.01), when combined with choice depleting variation in the preferred male trait, results in the preference being lost (Lande 1981; Pomiankowski et al. 1991). In contrast, when there is a mating preference for rarity, frequency-dependent selection on males can counter relatively high direct costs of choosiness because the preferred trait never becomes fixed, which readily maintains choosiness in the population through indirect benefits when it is rare. Although the proportion of choosy females was generally fairly low in our models, their mere existence has the major evolutionary impact on maintaining male trait polymorphism.

(b) Why do costly preferences persist at higher frequencies if mate choice is hindered?

If females are prevented from expressing a mating preference, one might predict that choosiness will reach a lower equilibrium frequency than it would if fully expressed. The logic behind this statement is that the benefits of the preferences are reduced while the costs remain the same (e.g. Lehmann et al. 2007). Somewhat surprisingly, this is not the case when females have a preference for rarity. Instead, we find the exact opposite: a higher frequency of costly preferences persists when females mate with their preferred type less often, even though we assume that the cost of the preference is expressed regardless of mating outcome. Again, this reflects feedback between the mating behaviour of females and the dynamics of the male types. The sexy son effect is knocked back every time a rare phenotype becomes common, and this happens much less often when some females fail to express the preference: it then takes longer for the rare phenotype to become common.

There are many scenarios in which constraints on female choice seriously hamper the evolution of preferences (e.g. Greenfield & Rodriguez 2004; Björklund 2006). By creating a counterexample where an imprecisely expressed preference evolves far more easily than the one which is fully realized, our model emphasizes that the inaccurate expression of preferences can be a double-edged sword. The expected benefit is obviously diminished if females do not always successfully mate with their preferred type, but, on the other hand, this also maintains more variation in male traits. Our study shows that it is not trivial to state where the balance of these two factors lies, as it can vary from case to case.

(c) Are there any general lessons to be drawn?

Our model considered a rather special kind of preference, and it is instructive to compare it with its counterpoint: preferences for common genotypes rather than rare ones. McLain (2005) showed that a preference for common genotypes can spread when commonness is indicative of current fitness. In McLain's (2005) model, abundance correlates with future viability, but it is initially independent of attractiveness. At first glance, it is surprising that preferences for rarity and commonness can both evolve, yet this simply reflects the fact that sexy son effects favour whatever trait is currently preferred. The only fundamental difference between preferences that do or do not confer indirect viability benefits is that the former evolve more readily (Kokko et al. 2002): they do not require strong pre-existing female preferences to kick-start self-reinforcing preference evolution. Preferences that only confer a mating advantage to offspring have to exceed a threshold before they spread (Pomiankowski et al. 1991), so they are expected to be far less often observed in nature.

There is an interesting parallel to the ‘folk theorem’ (so named as there is no acknowledged discoverer) in game theory, whose applicability to the studies of social behaviour and the evolution of punishment has recently been emphasized (Boyd 2006). It states that any behavioural rule can be stable if individuals evolve to punish those who deviate from the rule. If we replace ‘behavioural rule’ with ‘male phenotype’, ‘punishment’ with ‘mating preference’ and ‘being punished’ with ‘giving birth to unsuccessful offspring’, we can recast mate choice as a situation where males are subject to female-imposed rules as to what phenotypes they should express. The folk theorem means that an almost infinite variety of pointless rules of etiquette can arise in human societies if they are suitably policed. Knowing this makes it easier to appreciate how such an amazing diversity of female preferences has evolved.

The question we are ultimately left with is why some mating preferences, such as ones for rarity or commonness, as with some cultural rules, arise far less often than others? One answer is that, as mentioned previously, ‘sensible’ preferences that bring about viability benefits evolve more easily than those that do not. Another is that species extinction rates could partly depend on the population-level consequences of adaptive preferences at the level of the individual (e.g. Kokko & Brooks 2003; Rankin & López-Sepulcre 2005; Dieckmann & Metz 2006; Rankin et al. in press). For example, in mosquitofish, Gambusia holbrooki, an increase in the frequency of a rare, melanic male type leads to elevated female mortality and reduced temporal variation in female numbers (Horth & Travis 2002). A mating preference for rare, melanic males in this species would therefore have effects on both population density and stability.

Supplementary Material

Electronic appendix

The electronic supplementary material consists of the details of the individual-based model