Use of perceptual weights to test a model of loudness summation

Abstract

Leibold et al. [J. Acoust. Soc. Am. 121, 2822–2831 (2007)] examined the perceptual weight subjects assigned to individual components of a multitone complex while performing a loudness-matching task. Weights agreed with the Moore et al. loudness model [J. Audio Eng. Soc. 45, 224–237 (1997)], except when components were widely spaced in frequency. In an effort to account for the data, the just-noticeable-difference (jnd) for intensity discrimination was measured for each component and compared to the weight for that component. The model predicts greater improvement in intensity discrimination with increasing bandwidth than was observed in the data. Jnds were not correlated with weights in the widest frequency-spacing condition.

1. Introduction

Complex sounds, such as speech, are made up of multiple components that vary in frequency and in level. If we hope to develop a complete understanding of loudness perception, we must determine how these individual components contribute to the overall loudness of the complex sound. For example, do individual components contribute equally or do some components influence our perception of loudness more than others?

We recently examined the contribution of individual components to judgments of overall loudness in the context of a band-widening experiment by estimating the perceptual weights subjects assign to the individual components of a multi-tone complex during a two-interval, loudness-matching task (Leibold et al., 2007). Stimuli were five-tone complexes centered on 1000 Hz, with six different logarithmic frequency spacing conditions, ranging from a frequency ratio of 1.012 to 1.586. The perceptual weights were in good agreement with the predictions of a model of loudness summation (Moore et al., 1997), except at the widest bandwidth. The model proposed by Moore et al. (1997) accounts for loudness summation by representing sounds as excitation patterns that are summed and then converted to specific loudness, as proposed in earlier models (e.g., Zwicker and Scharf, 1965). We will briefly review the original data and present new data on discrimination of the level of the individual components obtained from the same listeners. We will compare the new data to predictions obtained from the model and to predicted and obtained perceptual weights.¹

2. Method

2.1 Subjects

The subjects were six adults (18–40 years), including author LL. All subjects had normal hearing sensitivity, with thresholds for 200-ms tones in quiet of 20 dB SPL or less at octave frequencies from 250–8000 Hz in both ears, measured using a two-interval, forced-choice (2IFC) adaptive procedure. All subjects participated in the experiments reported by Leibold et al. (2007). Data for a seventh subject who participated in the earlier experiments were excluded from the analyses reported here because the intensity discrimination thresholds were abnormally high and variable.

2.2 Stimuli and equipment

The stimuli were five-tone complexes centered on 1000 Hz. All stimuli were presented for 300 ms. Six different frequency spacing conditions were examined, corresponding to bandwidths from 46 to 2119 Hz. The overall level of the complex was 60 dB SPL (53 dB SPL/component). Stimuli were digitally generated and presented monaurally over headphones as described in greater detail by Leibold et al. (2007).

2.3 Intensity discrimination procedure

Following collection of data for loudness matching, perceptual weights, and masked thresholds in the three experiments described by Leibold et al., data were collected for intensity discrimination at each of the component frequencies in the presence of the five-tone complex for each of the six spacing conditions. The pedestal level of the five-tone complex was 60 dB SPL (53 dB SPL/component). Thresholds were estimated using a 2IFC, adaptive procedure that adjusted the level of an increment tone added in quadrature phase to one of the 5 tones to estimate the 71%-point on the psychometric function (Levitt, 1971). Two 100-block trials were run for each component in each frequency-spacing condition. This procedure was identical to the procedure used by Leibold et al. (2007) to estimate masked thresholds except that fewer threshold estimates were obtained in this case and the complex, or masker, contained 5 tones rather than 4, where one of the 5 tones was identical in frequency to the increment tone, or signal. For reasons described below, we report the data in terms of the threshold level of the increment tone. Paraphrasing an explanation of this terminology given by Viemeister and Bacon (1988), the threshold level of the increment can be interpreted as the SPL of a waveform that, when added to the 5-tone complex, produces a change in level that is detectable on 71% of the trials.

3. Results and Discussion

3.1 Overview of results reported by Leibold et al

Leibold et al. (2007) obtained loudness matching data consistent with the classic experiment by Zwicker et al. (1957) demonstrating that the loudness of the multitone complex was independent of bandwidth when components were within a critical band, but that the loudness of the complex increased as bandwidth increased beyond the critical band. The Moore et al. (1997) loudness model predicts a pattern of results consistent with the observed data and, also similar to the data, predicts no decrease in loudness growth with increasing bandwidth at the larger bandwidths. That is, the model assumes partial masking across several critical bands before conversion to loudness, with reduction in partial masking leading to increased loudness.

Leibold et al. examined mean perceptual weight as a function of component position for each bandwidth. The range of weights increased with increasing frequency spacing, with more weight assigned to the lowest and highest frequency components. Estimates of perceptual weight provided by the loudness model were largely in agreement with observed weights, except for the widest spacing condition. The discrepancy between the data and model in that condition suggests that central processes contribute to how subjects assign loudness judgments when components are widely spaced.

To determine the contribution of partial masking to loudness, Leibold et al. obtained mean masked thresholds for each component in the presence of the other four components for each spacing condition. Thresholds varied little across components for the two narrowest conditions, but the range of thresholds increased with increasing frequency spacing. Masked thresholds predicted by the loudness model were in good agreement with the data at all but the widest spacing condition, where the model predicted less masking than was observed.

Leibold et al. observed a strong correlation between masked threshold and weight for the two narrowest bandwidth conditions (46- and 92-Hz) and for the two intermediate conditions (231- and 456-Hz) for both the data and the model. In contrast, the correlation was not significant between weights and masked thresholds for the widest two conditions (956- and 2119-Hz).

3.2 Intensity discrimination for individual components

For the widest bandwidth condition, Leibold et al. observed a lack of agreement between the observed and predicted perceptual weight associated with individual components, between observed and predicted thresholds for individual components, and between thresholds and weights. A potential explanation for the discrepancy between the observed data and the model is that all components were too far above threshold in this condition for differences in threshold to have an effect on the weight associated with individual components or on the contribution of those components to loudness. The purpose of measuring the just-noticeable-difference (jnd) for intensity discrimination for each component was to obtain a threshold measure at the level of the components used in the loudness judgment task.

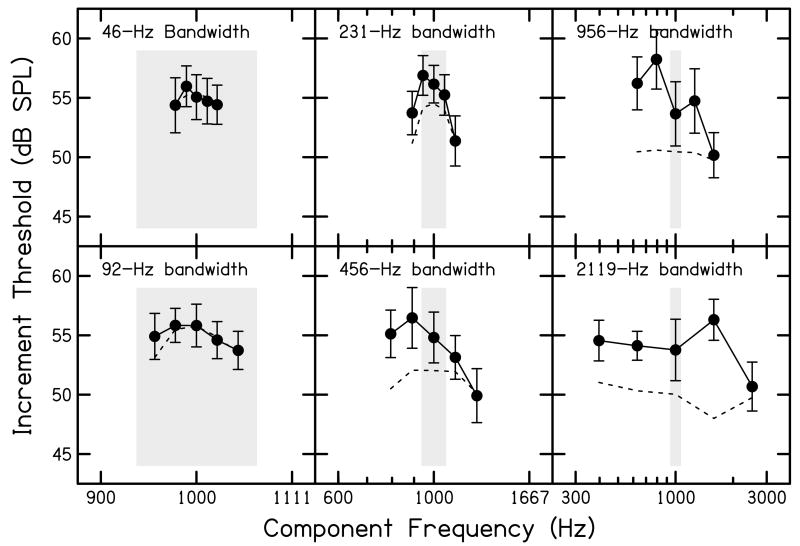
The filled circles in Figure 1 show the mean threshold as a function of component position for each bandwidth, reported as the level of the added tone producing the intensity increment. Error bars are 1 S.E around the mean across subjects. The data are reported as increment thresholds because conversion to other standard measures of intensity discrimination, 10 log (ΔI/I) or (10 log (1+ΔI/I)), would require an assumption regarding the level of the standard or pedestal. Should we assume that the subjects are detecting an increment in a single component or an increment in the total complex? At the two narrowest bandwidths, where all components fall within a critical band, it is reasonable to assume that the standard is 60 dB SPL, the level of the total complex, but the appropriate assumption regarding the level of the standard becomes less clear as the bandwidth increases. By reporting increment thresholds, the need to assume a level of the standard is avoided and the data are presented in units comparable to the masked thresholds reported by Leibold et al. (2007). There was little overall improvement in the average increment threshold across the five components as bandwidth increased. This suggests that the subjects were unable to process the components individually in the broad bandwidth conditions, but yet performance varied as a function of component position even at the narrowest bandwidth. At broader bandwidths, performance was better for the highest than for the lowest frequency component.

Figure 1.

Average increment thresholds for individual components across subjects for each frequency-spacing condition.

Estimates of increment threshold provided by the loudness model are shown by the dashed lines in each panel. These were obtained by treating the five-tone complex as a masker and the increment tone as a signal and assuming that the partial loudness of the signal at threshold is 8 phons. This higher criterion was chosen because it resulted in equal increment thresholds for the model and the data across the five components in the narrowest bandwidth condition. The model predicts differences in increment thresholds as a function of component position that parallel the data for the three narrowest bandwidths. The model deviates from the data in predicting an orderly improvement in performance with increasing bandwidth and comparable performance for the highest and lowest frequency components.

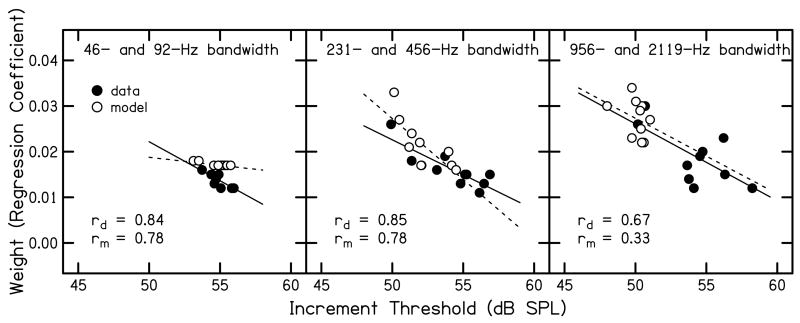
The relation between increment threshold and weight is shown in Figure 2. A strong correlation was found for the two narrowest bandwidth conditions (46- and 92-Hz) and for the two intermediate conditions (231- and 456-Hz). The results are similar for the model and the data. These results are comparable to the strong correlations observed for the two narrowest and two intermediate conditions in the previous study (Leibold et al., 2007). In contrast, the correlation was not significant between weights and increment thresholds for the widest two conditions (956- and 2119-Hz). Note also that Leibold et al. reported no significant correlation between weights and masked thresholds at the widest bandwidth.

Figure 2.

Average weight as a function of increment threshold for individual components across six subjects.

The results in Figure 1 indicate that intensity discrimination for high and low frequency components differs by more than the model would predict at broader bandwidths. There may be a connection between this pattern of results and the increased perceptual weight associated with higher frequency components at broader bandwidths in the loudness matching task used by Leibold et al.. The connection is not apparent, however, in Figure 2.

The subjects’ task in this experiment, detection of an increment in one tone of a multitone complex, is comparable to profile analysis (e.g., Green, 1988), but without an overall rove in level between observation intervals. Profile-analysis data generally indicate better performance for detection of an increment in the center tone of the complex than for detection of an increment in the highest or lowest tone (Green, 1988; Green and Mason, 1985). There is also an improvement with increasing bandwidth (Green et al., 1984). Neither effect was apparent in the data. Although the model predicts smaller jnds with increasing bandwidth, it predicts better detection of an increment in the highest or lowest component than of an increment at the center of the complex.

4. Conclusion

The Moore et al. (1997) loudness model provides a good account of the increased loudness of multitone patterns with increasing bandwidth and of the perceptual weights and masked thresholds of individual components, except at the widest bandwidth. The model also provides a good account of intensity discrimination for individual components for the three narrower bandwidths, but fails to predict results at wider bandwidths. Efforts to relate differences in perceptual weight at the wider bandwidths to differences in intensity discrimination for individual components were unsuccessful.