Species richness and resource availability: A phylogenetic analysis of insects associated with trees

Abstract

The data on the number of species of insects associated with various trees in Britain have been reanalyzed to factor out possible bias from phylogenetic effects. It was found that tree availability (range and abundance) continues to provide a good predictor (r = 0.852) of insect-species richness, slightly better than straightforward cross-species analyses. Of the two components of tree availability, tree abundance gives a much better prediction than tree range. The species richness on trees of major taxa with similar trophic habits (Lepidoptera and Hymenoptera/Symphyta and the two suborders of the Homoptera—Auchenorrhyncha and Sternorrhyncha) shows positive correlations; there is thus no evidence of competitive exclusion at this taxonomic level.

The determinants of diversity are of central issue in ecology and have gained particular urgency with the escalating loss of species and habitats in recent years. Hence, the relationship of resource availability and its constituent components to species richness is a focus of long-term and continuing attention. Recently, it has been recognized that the sorts of multispecific data sets used to examine questions such as this one can be unwittingly biased because of phylogenetic relatedness among the included species (1, 2). Several reanalyses of data thought to support some “classic” ecological principles have shown that when phylogenetic relatedness is appropriately taken into account, the relationship may not, after all, be upheld (e.g., refs. 3 and 4). The relationship between the species richness of the insect herbivore community and the abundance of the tree species is commonly cited as one of the definitive indications of the relationship between resource availability and species richness (4–7). Here, we present a reanalysis of these data, taking into account the potentially confounding effect of phylogenetic relatedness.

Among other things, previous analyses established that the total number of phytophagous insect species to be found on a particular tree species correlated with a measure of the availability of that tree species (5–7). Breaking down insect species into subgroups, each containing similarly feeding, taxonomically related taxa, indicated that the relationship with tree-species availability operated at that level as well. Further, taxonomic isolation of a tree species, that is, whether a tree species has few or many close relatives in Great Britain, was found to explain a small but significant proportion of the variation in insect-species richness on the trees in their data set: the more taxonomically isolated tree species supported fewer insect species than otherwise expected. Subsequent studies have applied these findings and this methodology (i) to compare insect-species richness and host-species availability in other geographic areas and taxa (e.g., 8–11), in terms of parasitoid–host, predator–prey, and ectomycorrhizal fungi–host tree relationships (e.g., refs. 12–15); (ii) to infer causes behind the patterns of diversity in urban areas, pine plantations, and among coral communities (e.g., refs. 16–19); and (iii) to devise plans for the conservation of insectivorous bats (20). Thus, whether the findings of this earlier body of work (5, 7) are upheld by reanalysis may have repercussions considerably beyond the straightforward application of those results to insect–plant relations.

Ecology seeks to understand the causes behind ecological processes that seem to lead to similarity among species in performance and interactions. However, species may be similar simply because they are closely related and therefore will not constitute independent data points from which to infer functional ecological dynamics. Therefore, comparative methods compare step-by-step the most closely related taxa available so as to contrast species or groups in which similarity due to shared ancestry minimizes the number of differences and thus indicates possible factors that may explain the observed differences in the target variables (1). We have used this method in the study reported here.

MATERIALS AND METHODS

Data on range size and abundance of tree species in Great Britain and on the number of phytophagous insect species associated with each tree species were extracted from Kennedy and Southwood (7). Tree-species availability was represented as the product of range size and abundance for that species. To eliminate the effect of taxonomy on the relationships between tree range size, abundance, and availability and insect-species richness (the target variables), we used a method developed by Pagel (21) based on the formulation by Felsenstein (22) called “independent contrasts.” For the purpose of examining the relationships between continuous variables as we have done here, the common logarithms of the target variable for all the subtaxa within each taxon are split into two groups based on similarity in the assumed causal variable. Beginning at the species level, those species having a value for the causal variable less than the average for the node that joined those species were placed in one group; those with a value greater than the nodal average were placed in a second group. The difference in the means between the two groups provides the contrast for the variable of the node in question. Within-group averages in the dependent variable were also produced for the same two groups of species and used to calculate the contrast of the dependent variable.

In the next step, the overall average value of the dependent variable at the previous node was taken to represent the ancestral species of that node, and the same sort of contrasts were then calculated between groups joined by the next highest node, with the same criterion for dividing the constituents into two groups. This method of creating dichotomous contrasts within a taxon was performed from the lowest to the highest level of phylogenetic organization by applying the computer package caic (Comparative Analysis by Independent Contrasts; ref. 23).

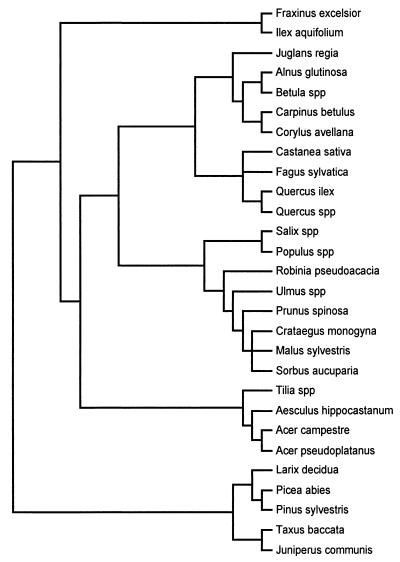
We built our phylogenetic “tree” by using both the method of Nandi (24) to define relationships among plant families and higher level taxa and that of Thorne (25) to define relationships below the level of family. Because of the particular species involved, the structure of our phylogenetic tree formed a regular dichotomizing pattern, with only 2 of 25 nodes left unresolved (with more than two taxa; Fig. 1). This high degree of resolution meant that the choice of the predictor variable used for grouping taxa to calculate contrasts was largely irrelevant to our conclusions, as the same taxa were compared with one another in all cases.

Figure 1.

Tree species used in the analysis, arranged according to phylogenetic relationships taken from Nandi (24) and Thorne (25).

The contrasts for the common logarithms of the target variables were entered into a regression forced through the origin (26). The reasoning behind this model is that if there is a functional relationship between x and y, then a lack of change in the state of x (i.e., the contrast calculated by comparing the independent variable of two closely related taxa) should be accompanied by a lack of change in y (the contrast calculated by comparing the dependent variable for the same two, phylogenetically related taxa). A significant relationship indicates a functional relationship between the two variables.

In factoring out possible biases due to phylogenetic relatedness in Kennedy and Southwood’s (7) original data, we performed several regression and regression-type analyses of independent contrasts calculated by the algorithm described above. First and foremost, we determined the relationship between Kennedy and Southwood’s (7) measure of tree-species availability in Great Britain and insect-species richness for each of those tree species (in Great Britain) by using a regression analysis that forces the intercept through the origin (as above). We also noted Kennedy and Southwood’s concern with possible effects of native vs. nonnative status of the tree species, and performed an analysis of contrasts derived from a phylogenetic tree constructed by using only native tree species.

Kennedy and Southwood (7) asked whether the degree to which a tree seems “familiar” to a phytophagous insect will affect the probability of infestation of that tree by estimating the taxonomic isolation of the tree species in their data set (following the method described in ref. 27). Noting that closely related tree species are more likely to be chemically or morphologically similar to one another, Kennedy and Southwood (7) postulated that the more close relatives a tree species has in a region, the more familiar an insect will be with those general, taxon-based characteristics and the more predisposed the insect will be to accept the target species. Kennedy and Southwood (7) found that this assay of tree familiarity to insects had a small but significant effect on insect diversity supported by a tree species.

We based the tests herein on the logic that introduced tree species, by definition, are not the tree species with which native insects have coexisted over evolutionary time and, in consequence, must present some degree of unfamiliarity to those insect species. Thus, if unfamiliarity of a tree species decreases the chance of infestation by an insect species, then an introduced tree species should support fewer insect species than does the native taxon most closely related to that introduced tree species. When considering this question, we realized however that it may at the same time be true that an introduced tree species may not have been present in Great Britain over a sufficient period of time to have reached the level of availability of its closest native relatives. Alternatively, either the native or the introduced tree species may be highly planted and could thus support more insect species than the most closely related native taxon, resulting in an anomalous contrast even though still conforming to the expected relationship between tree-species availability and insect-species richness. We therefore normalized for possible manipulated or time-dependent distributions of tree species by dividing values for insect-species richness by tree-species availability and used a sign test to compare the log₁₀ of this ratio between each introduced tree species and that of the most closely related native taxa.

With regard to another aspect of inquiry, insect behavior may affect plant–insect interactions in various ways, and different insect feeding guilds or taxa may respond differently because of differing feeding ecologies (e.g., adaptations to host-plant chemistry), because of differing mobilities (potentially altering access to widely distributed tree species), or as a result of competitive interactions between taxa. Regression analyses were therefore used to examine the relationships between host-plant availability and numbers of species in selected subgroups: the Homopteran suborders Auchenorrhyncha and Sternorrhyncha as well as the Hymenoptera/Symphyta and Lepidoptera. Two analyses were performed to determine whether a hypothesized effect of competitive exclusion between similarly feeding subgroups might be evident, through comparing the simultaneous distributions among tree species of the Auchenorrhyncha with the Sternorrhyncha, and the Symphyta with the Lepidoptera.

Kennedy and Southwood’s (7) measure of tree-species availability is a composite of two measures of plant distribution, species range size and species abundance, which are of interest in themselves for their individual roles in animal diversity and distribution and for their potential relationship to one another (28–30). Therefore, we examined the relationships of the contrasts of plant-species abundance and plant-species range size with the contrasts of insect-species richness for the independent contribution of each in determining insect diversity relative to tree-species availability. We also used regression analysis, forced through the origin, to ascertain the relationship between tree-species range size and abundance when phylogenetic relatedness has been taken into account.

The sets of contrasts of all variables for all analyses were examined for normality to verify that the Brownian-Motion model of evolutionary change assumed in Felsenstein’s original formulation of independent contrasts (22) was not violated (P. Harvey, personal communication).

RESULTS

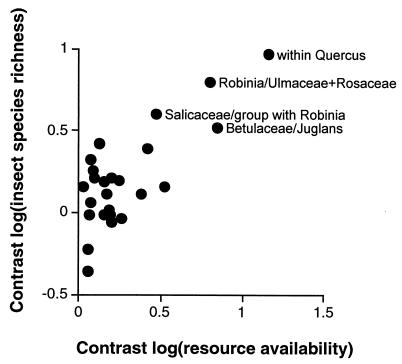
The relationship between tree-species availability and insect-species richness found in earlier studies (5–7) continued to be evident when the possible biasing effect of phylogenetic relatedness is factored out of the analysis (r = 0.852; P > 0.0001; n = 25; Fig. 2). The independent-contrasts method produced a relationship between tree availability and insect-species number explaining a greater amount of the variation than that seen in the cross-species analysis (i.e., without taking phylogeny into account; r = 0.765; P < 0.0001; n = 28), although that difference was not significant (P = 0.4562). Analyses of the independent contrasts calculated with only native tree species also produced a significant, positive relationship between availability of a tree species and the numbers of insect species to be found on it (r = 0.503; P = 0.0238; n = 19) but one similar in explanatory value to the cross-species analysis including only native tree species (where r = 0.51; P < 0.05; n = 21).

Figure 2.

Relationship between the contrasts of the log₁₀(resource availability) and the contrasts of the log₁₀(insect-species richness). The four most extreme points are labeled with the identities of the taxa used to calculate those contrasts.

However, native and introduced tree species may possess differing relationships with insect diversity. Exclusion of introduced tree species from the analysis of independent contrasts significantly reduced the explanatory value of the model (P = 0.0195). Examination of Fig. 2 suggests that the reason for this reduction may rest in the four most extreme values in the figure being attributable to contrasts including just three of the seven introduced species: Quercus ilex, Robinia pseudoacacia, and Juglans nigra. Recalculation of the regression analysis leaving out only these three species accounted for the largest part of the observed reduction in the correlation coefficient (to r = 0.570). In a similar vein, comparison between native and introduced tree species showed that insect-species richness relative to tree-species availability is greater for native tree species in six of seven contrasts (P = 0.062).

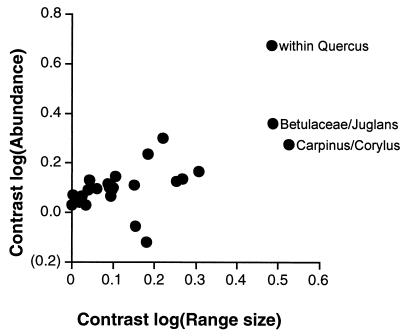
In our comparison of Kennedy and Southwood’s measures of tree-species range size and tree-species abundance for native species, an analysis not included in the original study, we find the two components to have a significant positive relationship both when phylogenetic relatedness is accounted for (r = 0.654; P = 0.0018; Fig. 3) and when it is not, in a cross-species analysis (r = 0.777; P < 0.0001). When the two components of tree-species availability are entered individually into phylogenetically controlled regression models with insect-species number as the dependent variable, tree-species abundance explains by far the greatest amount of variation in insect diversity (for tree-species abundance alone, r = 0.632, P = 0.0028; for tree-species range size alone r = 0.252, P = 0.2834). However, because these two variables are correlated with one another, the effect of one may obscure the independent effect of the other on insect diversity. It is of interest then to find that a regression of the residuals of the relationship between tree-species abundance and insect-species number against range size shows the independent effect of range size on insect diversity to be negative (β = −0.367; r = 0.208), although not significantly so (P = 0.3795), whereas the effect of tree-species abundance on insect diversity, when the effect of tree range size was held constant, continued to be positive and significant (r = 0.482; P = 0.0312).

Figure 3.

Relationship between the contrasts of the log₁₀(tree range size) and the contrasts of the log₁₀(tree abundance). The three most extreme points are labeled with the identities of the taxa used to calculate those contrasts.

Regression analyses also showed that only for two of the selected insect subgroups did a significant, positive statistical relationship exist between number of insect species and tree-species availability (Table 1). Additionally, comparisons between insect groups showed that distribution among the tree species are high or low in concert (Table 2). In general, the independent effect of tree-species abundance on insect-species number tended to follow the pattern of the effect for the composite measure of tree-species availability. However, tree-species range size had no significant independent effect in any analysis entailing insect subgroups (Table 1).

Table 1.

Relationships between various measures of resource availability and the richness of selected insect taxa for the tree species displayed in Fig. 1

Insect subgroup	Effect of resource availability on insect-species richness		Independent effects of tree-species abundance and tree range size on insect-species richness
	Independent contrasts	Cross-species	Tree-species abundance	Tree-species range size
Auchenorhyncha	r  =  −0.027; P  =  0.9104	r  =  0.451; P  =  0.403	r  =  −0.003; P  =  0.9913	r  =  −0.014; P  =  0.9518
Sternorrhyncha	r  =  0.337; P  =  0.1460	r  =  0.580; P  =  0.0058	r  =  −0.176; P  =  0.4577	r  =  −0.043; P  =  0.8579
Hymenoptera/Symphyta	r  =  0.597; P  =  0.0055	r  =  0.611; P  =  0.0033	r  =  0.382; P  =  0.0970	r  =  0.036; P  =  0.8803
Lepidoptera	r  =  0.446; P  =  0.0487	r  =  0.602; P  =  0.0039	r  =  0.462; P  =  0.0405	r  =  −0.225; P  =  0.3410

Table 2.

Relationship between selected insect taxa in species richness found on the tree species displayed in Fig. 1

Comparison	Independent contrasts	Cross-species
Auchenorhyncha vs. Sternorrhyncha	r  =  0.381; P  =  0.0973	r  =  0.646; P  =  0.0015
Hymenoptera/Symphyta vs. Lepidoptera	r  =  0.836; P  <  0.0001	r  =  0.814; P  <  0.0001

All sets of independent contrasts used in the above analyses conformed to the criterion of normality, indicating that the underlying assumption in the phylogenetically controlled analyses of a Brownian-Motion model of evolution is not violated.

DISCUSSION AND CONCLUSIONS

The relationship between species richness and resource availability, as exemplified by the number of species of insect phytophages associated with different species of tree in Britain, is supported by this comparative analysis that takes account of phylogenetic relationships among tree species. The availability of the resource, in this case the tree species, can be evaluated by a composite measure of range size and abundance as derived by Kennedy and Southwood (7) from records of distribution in 10 × 10-km and 2 × 2-km squares, respectively. In this comparative analysis with phylogenetic effects factored out, the number of insect species is strongly correlated with tree availability, as so defined. Indeed, after the exclusion of phylogenetic effects, tree-species availability is a slightly better predictor than in the straight cross-species analyses of earlier studies (5, 7), although not significantly so.

Of the two components of the measure of availability, tree abundance is the best predictor, in the statistical sense, of insect-species richness. This result is interpretable as a reflection of the encounter-frequency hypothesis (31, 32) for the determination of insect–plant associations. It draws a parallel with the development of insect resistance to pesticides, the rate of which is related to the frequency of exposure. This simple hypothesis is supported further by the lack of any evidence of competitive exclusion when the number of species of the major taxa of chewing and sap-feeding (“sucking”) phytophages are compared. Indeed, there is a positive relationship such that, for example, a tree species with a rich Lepidopteran fauna is likely also to have a rich assemblage of Symphyta; both have similar caterpillar type larvae. A different but complementary explanation is that colonies of insects on the less abundant trees are more isolated and thus the risk of extinction—in both evolutionary and geographical terms—is greater (reviewed in ref. 33).

The two components of tree availability, range size and abundance, are themselves correlated, whether phylogenetic influence is excluded or not. This correlation may be evidence for the concept that those species with the broader niche breadth (range) are also the most abundant (28, 30, 34).

It should be noted that, although the relationship between insect-species richness and tree abundance holds when only native species are considered, this relationship has much less predictive value. High predictive values in earlier studies (5, 7) have also been based on analyses including introduced (nonnative) species. This loss of predictive power may be a purely statistical effect, as there are few native trees in Britain with limited ranges and abundances. Alternatively, the loss of predictive power may reflect change in the proportions of generalist and specialist herbivores at different stages in the process of species accumulation on a tree species, a suggested difference between native and introduced trees (35, 36). The presence of truly generalist herbivore species tended to be under-recorded in the original insect data sets (5, 7).