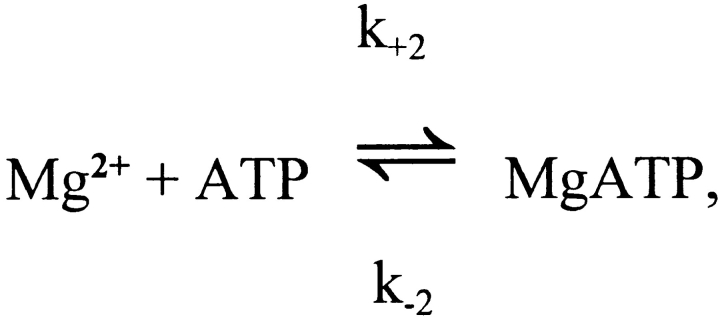Model of Sarcomeric Ca²⁺ Movements, Including ATP Ca²⁺ Binding and Diffusion, during Activation of Frog Skeletal Muscle

Abstract

Cannell and Allen (1984. Biophys. J. 45:913–925) introduced the use of a multi-compartment model to estimate the time course of spread of calcium ions (Ca²⁺) within a half sarcomere of a frog skeletal muscle fiber activated by an action potential. Under the assumption that the sites of sarcoplasmic reticulum (SR) Ca²⁺ release are located radially around each myofibril at the Z line, their model calculated the spread of released Ca²⁺ both along and into the half sarcomere. During diffusion, Ca²⁺ was assumed to react with metal-binding sites on parvalbumin (a diffusible Ca²⁺- and Mg²⁺-binding protein) as well as with fixed sites on troponin. We have developed a similar model, but with several modifications that reflect current knowledge of the myoplasmic environment and SR Ca²⁺ release. We use a myoplasmic diffusion constant for free Ca²⁺ that is twofold smaller and an SR Ca²⁺ release function in response to an action potential that is threefold briefer than used previously. Additionally, our model includes the effects of Ca²⁺ and Mg²⁺ binding by adenosine 5′-triphosphate (ATP) and the diffusion of Ca²⁺-bound ATP (CaATP). Under the assumption that the total myoplasmic concentration of ATP is 8 mM and that the amplitude of SR Ca²⁺ release is sufficient to drive the peak change in free [Ca²⁺] (Δ[Ca²⁺]) to 18 μM (the approximate spatially averaged value that is observed experimentally), our model calculates that (a) the spatially averaged peak increase in [CaATP] is 64 μM; (b) the peak saturation of troponin with Ca²⁺ is high along the entire thin filament; and (c) the half-width of Δ[Ca²⁺] is consistent with that observed experimentally. Without ATP, the calculated half-width of spatially averaged Δ[Ca²⁺] is abnormally brief, and troponin saturation away from the release sites is markedly reduced. We conclude that Ca²⁺ binding by ATP and diffusion of CaATP make important contributions to the determination of the amplitude and the time course of Δ[Ca²⁺].

introduction

During normal activation of a skeletal muscle fiber, an action potential in the transverse tubular membranes triggers the opening of Ca²⁺ release channels in the sarcoplasmic reticulum (SR).¹ The released Ca²⁺ produces an increase in the myoplasmic free [Ca] (Δ[Ca²⁺]), which activates the fiber's contractile response.

The SR calcium release channels (“ryanodine receptors”) are found primarily at triadic junctions, where the transverse tubules and the terminal cisternae membranes of the SR are closely apposed. In frog fibers, the triadic junctions are located primarily at the Z lines of the sarcomeres and surround each myofibril with a geometry that approximates an annulus (Peachey, 1965). With this anatomical arrangement, intra-sarcomeric gradients in myoplasmic free [Ca²⁺] are expected when Ca²⁺ release is active. An understanding of these gradients and the associated movements of Ca²⁺ is important in the interpretation of spatially averaged Ca²⁺ measurements of the type that have been made with a variety of Ca²⁺ indicators. They are also important in the interpretation of local Ca²⁺ measurements of the type that have been made recently with high-affinity indicators and confocal microscopy (Escobar et al., 1994; Tsugorka et al., 1995; Klein et al., 1996).

Cannell and Allen (1984) were the first to use a computer model of a half-sarcomere to estimate the binding and diffusion of Ca²⁺ after its release at the Z line in response to an action potential. A principal motivation was to compare the model predictions about the amplitude and time course of Δ[Ca²⁺] with measurements of Δ[Ca²⁺] that had been obtained from frog single fibers injected with the indicator aequorin. In this article, we describe a similar computer model developed from a similar motivation. In comparison with Cannell and Allen (1984), our model incorporates three significant differences about the myoplasmic environment and the SR Ca²⁺ release process.

First, we assume a twofold smaller diffusion constant for myoplasmic free Ca²⁺ (3 × 10⁻⁶ cm² s⁻¹ at 16°C vs. 7 × 10⁻⁶ cm² s⁻¹ at 20°C). This difference is based on the finding that the viscosity of myoplasm is approximately twofold higher than that of a simple salt solution (Kushmerick and Podolsky, 1969; Maylie et al., 1987a ,b,c).

Second, the temporal waveform that we assume for SR Ca²⁺ release in response to an action potential (half-width, 1.9 ms at 16°C) is approximately threefold briefer than that assumed by Cannell and Allen (1984) (half-width, 5.8 ms at 20°C). This difference derives from measurements of spatially averaged Δ[Ca²⁺] in frog fibers injected with lower-affinity Ca²⁺ indicators such as purpurate-di-acetic acid (PDAA; Southwick and Waggoner, 1989) or furaptra (Raju et al., 1989). These indicators, which appear to track Δ[Ca²⁺] in skeletal muscle with 1:1 stoichiometry and little or no kinetic delay (Hirota et al., 1989; Konishi et al., 1991; Zhao et al., 1996), report Ca²⁺ signals that are substantially briefer than estimated with aequorin (Cannell and Allen, 1984). Consequently, estimates of SR Ca²⁺ release with these indicators (Maylie et al., 1987b ; Baylor and Hollingworth, 1988; Hollingworth et al., 1992, 1996), which to date have been based on spatially averaged models (e.g., Baylor et al., 1983), are substantially briefer than assumed by Cannell and Allen (1984).

Third, we include the reactions of Ca²⁺ and Mg²⁺ with ATP, which is present in the myoplasm of skeletal muscle at millimolar concentration (probably 5–10 mM in a rested fiber; Kushmerick, 1985; Godt and Maughan, 1988; Thompson and Fitts, 1992). Although the fraction of ATP in the Mg²⁺-bound form (MgATP) at rest is expected to be large (∼0.9) at the free [Mg²⁺] level of myoplasm (see results), the ATP reaction kinetics (Eigen and Wilkins, 1965) are such that a significant rise in the concentration of Ca²⁺ bound to ATP (Δ[CaATP]) is predicted during activity. Furthermore, ATP is sufficiently small (mol wt, ∼500), with an expected myoplasmic diffusion constant of ∼1.4 × 10⁻⁶ cm² s⁻¹ at 16°C (Kushmerick and Podolsky, 1969), that a significant transport of Ca²⁺ along the sarcomere in the CaATP form should occur. This transport of Ca²⁺ by CaATP appears to permit a more uniform and synchronous binding of Ca²⁺ to troponin along the thin filament. These effects of ATP in skeletal muscle point to a likely role of ATP in the shaping of local Ca²⁺ gradients in other cells (cf., Zhou and Neher, 1993; Kargacin and Kargacin, 1997).

materials and methods

Overview of the Multi-compartment Model

Our computational model is similar in principle to that of Cannell and Allen (1984). We divide the myoplasmic space corresponding to a half-sarcomere of one myofibril into a number of compartments that have equal volume and radial symmetry (cf., Fig. 1, where there are six longitudinal by three radial compartments). Within each compartment, appropriate metal-binding sites for Ca²⁺ and Mg²⁺ are included at the total concentrations and with the diffusion constants listed in Table I, B and C (described below). Resting occupancies of the sites by Ca²⁺ and Mg²⁺ are based on appropriately chosen values of dissociation constants (K _d,_Ca for Ca²⁺, K _d,Mg for Mg²⁺) and resting levels of free [Ca²⁺] and free [Mg²⁺]. The time-dependent calculation is initiated by the introduction of a finite amount of total Ca²⁺, with an appropriate time course, into the compartment comprising the outermost annulus nearest the Z line (corresponding to the location of the SR release sites; see Fig. 1, downward arrow). The calculation is advanced in time by simultaneous integration of the first-order differential equations for the concentration changes of the various species (free Ca²⁺; metal-free and metal-bound sites) in all compartments. For the integration, it is assumed that: (a) Ca²⁺ and Mg²⁺ react with available binding sites according to the law of mass action; and (b) the various species move by the laws of diffusion across any immediately adjacent compartment boundary. Additionally, Mg²⁺ is assumed to be well buffered, so that possible changes in free [Mg²⁺] are neglected in all compartments.

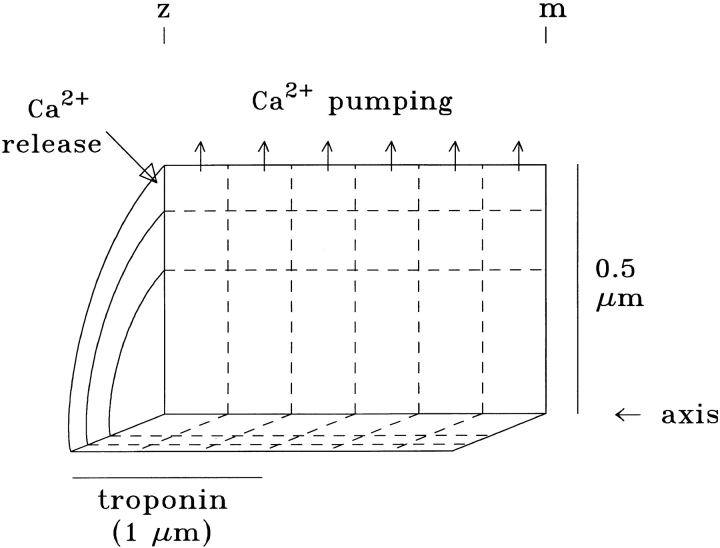
Figure 1.

Cut-away view of a portion of a half-sarcomere of one myofibril, illustrating the compartment geometry of the model. The fiber axis extends horizontally. As shown here, a typical calculation divides the myoplasm into 18 radially symmetric compartments of equal volume (six axial times three radial). Troponin is assumed to be restricted to the compartments located axially within 1 μm of the Z line (i.e., the length of the thin filaments), whereas the diffusible species (parvalbumin and ATP) are assumed to have access to all compartments. Note that the vertical and horizontal scales are different. See Table I for additional information.

Table I.

Parameters of the Standard Multi-compartment Model

A. General
Temperature		16°C
Length, z- to m-line		2.0 μm
Length of thin filament		1.0 μm
Radius of myofibril		0.5 μm
Resting free [Ca2+]		0.1 μM
Resting free [Mg2+]		1.0 mM
Longitudinal subdivisions		6
Radial subdivisions		3

B. Spatial Distributions and Diffusion Constants
Species		Location		Diffusion constant
				10−6 cm2 s−1
Free Ca2+		All compartments		3
Troponin		Compartments within  1.0 μm of the z-line
				0
Parvalbumin		All compartments		0.15
ATP		All compartments		1.4
Indicator dyes		All compartments		0.20–0.99

C. Bulk Concentrations and Ca2+ and Mg2+ Reaction Rates
Species		[Total sites]		k + 1		k − 1		K d
		μM		108 M−1 s−1		s−1		μM
Troponin (regulatory)		240		0.885		115		1.3
Parvalbumin		1,500
Ca2+				0.417		0.5		0.012
Mg2+				0.00033		3.0		91.0
ATP		8,000		0.1364*		30,000*		2,200*

D. Resting Fractional Occupancies of Sites by Ca2+ and Mg2+
		Ca2+		Mg2+
Troponin (regulatory)		0.071		—
Parvalbumin		0.410		0.541
ATP		0.000		0.909*

The table lists general information as well as typical values used for the model calculations. In B, all species are assumed to diffuse in myoplasm except for troponin, which is fixed at the location of the thin filament. In C, the site concentrations are referred to the myoplasmic water volume (cf., Baylor et al., 1983); the values of k ₊₁, k ₋₁, and K _d (= k ₋₁/k ₊₁) are for Ca²⁺ unless indicated otherwise. The asterisk in C and D denotes that the rates for Ca²⁺-ATP are effective rates in the presence of 1 mM free [Mg²⁺] and that a reduced set of equations involving the effective occupancy of ATP with Mg²⁺ was used for the majority of calculations with ATP included (see materials and methods).  

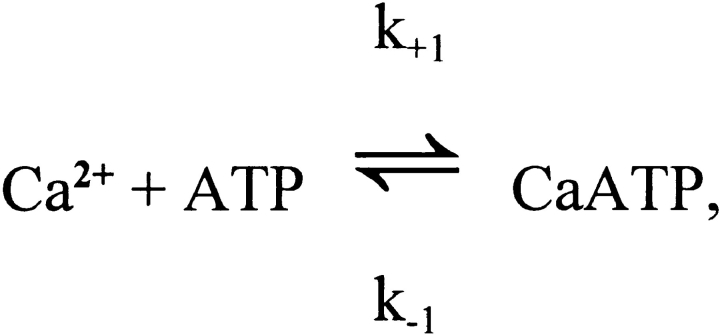
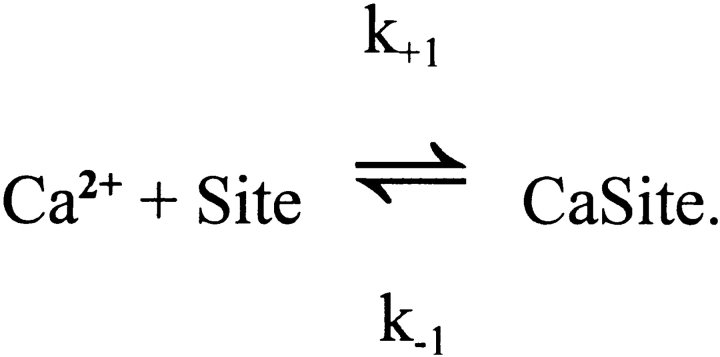
In each compartment, the binding steps are governed by a mass-action reaction of the type illustrated here for Ca²⁺:

Site and CaSite denote the metal-free and Ca²⁺-bound forms of the site, respectively, and k ₊₁ and k ₋₁ denote the on- and off-rate constants, respectively, for the reaction. The corresponding functional form used in the integration is:

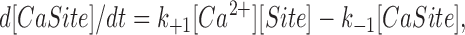1

where [Ca²⁺] denotes the free Ca²⁺ concentration (Δ[Ca²⁺] + resting [Ca²⁺]). For the sites that bind Mg²⁺ (e.g., parvalbumin; cf., Johnson et al., 1981; Gillis et al., 1982; Baylor et al., 1983), an analogous equation for Mg²⁺ is included in each compartment.

The diffusion of each species across each internal compartment boundary is calculated with an approximation from Fick's law, illustrated here for Ca²⁺:

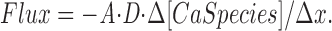2

A denotes the area of the boundary, and D denotes the relevant diffusion constant; “CaSpecies” denotes either free Ca²⁺ or one of the Ca²⁺-binding species listed in Table I; “Δ[CaSpecies]” denotes the difference in species concentration for the two compartments on either side of the boundary being crossed; and Δx denotes the center-to-center distance between the two compartments. The number of boundaries varies from two to four per compartment, according to the compartment's location (see Fig. 1). Ca²⁺'s spread within the half-sarcomere thus occurs both as diffusion of the free ion and as diffusion of Ca²⁺ bound to mobile sites (parvalbumin, ATP, and indicator but not troponin). For the integration, the number of moles of each species that moves into or out of each compartment per unit time is divided by the compartment volume to determine the effect of diffusion on the change in concentration of that species in that compartment per unit time.

The removal of Ca²⁺ from the half-sarcomere is assumed to take place only from the outermost compartments (see Fig. 1, upward arrows). This corresponds to the location of the longitudinal membranes of the SR, which extend from Z line to Z line at the periphery of a myofibril (Peachey, 1965) and contain calcium ATPase molecules (Ca²⁺ pumps) at a high density (Franzini-Armstrong, 1975).

In each compartment, a mass conservation equation is used to track the change in total Ca²⁺ concentration in that compartment (denoted Δ[Ca_T]), equal to the change in compartment Ca²⁺ concentration due to SR release (if any) minus that due to SR pumping (if any) minus the net change in concentrations due to diffusive movements out of the compartment of free Ca²⁺, Ca²⁺ bound to parvalbumin, and Ca²⁺ bound to ATP. The Δ[Ca²⁺] level in each compartment (for use in Eq. 1) is calculated as the Δ[Ca_T] of the compartment minus the change in compartment concentrations of Ca²⁺ bound to troponin, parvalbumin, and ATP (denoted Δ[CaTrop], Δ[CaParv], and Δ[CaATP], respectively). If the maximum removal rate by the Ca²⁺ pump is set to zero, the mass equations provide a check on the accuracy of the calculation, since the values of Δ[Ca_T], if summed over all compartments, should then equal the integral of the Ca²⁺ release waveform (Eq. 3, described below) after referral of both quantities to the total myoplasmic volume. This check of the model was satisfied at the level of a fraction of one percent.

Parameters of the Model

Table I gives general information about the model, including the standard dimensions of the half-sarcomere and the most common choice for the number of longitudinal and radial subdivisions. Part B lists the spatial locations of the different metal-binding species and their diffusion constants. In all cases, metal-free and metal-bound diffusion constants are assumed to be identical. The troponin sites are assumed to be fixed because of their attachment to the thin filaments, which in a frog twitch fiber extend 1.0 μm away from the Z line (Page and Huxley, 1963). The other values of the diffusion constants are half those estimated to apply to free solution at 16°C, since the viscosity of myoplasm appears to be about twice that of free solution (Kushmerick and Podolsky, 1969; Maylie et al., 1987a ,b,c).

Table I lists the assumed concentrations and reaction rate constants for the metal-binding sites on troponin, parvalbumin, and ATP. The values assumed for ATP are explained in the next section. The values for troponin are taken from “model 2” of Baylor et al. (1983), modified slightly as described in Baylor and Hollingworth (1988). The values for parvalbumin are also taken from “model 2” of Baylor et al. (1983), but with two changes. The value for the total concentration of metal sites on parvalbumin is 1,500 rather than 1,000 μM, which reflects a more recent estimate for frog twitch fibers (Hou et al., 1991). The value assumed for the parvalbumin on-rate for Ca²⁺ is threefold smaller than that assumed by Baylor et al. (1983). This latter change is related to our assumption that resting free [Ca²⁺] is 0.1 μM (cf., Kurebayashi et al., 1993; Harkins et al., 1993; Westerblad and Allen, 1996) rather than the fivefold smaller value assumed by Baylor et al. (1983). There is uncertainty in the values of the parvalbumin reaction rates (Johnson et al., 1981; Ogawa and Tanokura, 1986), and if the Ca²⁺-parvalbumin on-rate assumed by Baylor et al. (1983) is used, the fraction of the parvalbumin sites bound with Ca²⁺ at a resting [Ca²⁺] of 0.1 μM is quite large (0.676). This large fraction decreases somewhat the ability of parvalbumin to accelerate the rate of decline of Δ[Ca²⁺] after the termination of release. In any event, a threefold variation in the value assumed for the Ca²⁺-parvalbumin on-rate had only minor effects on the calculations (see results).

Table I also gives the values of K _d (dissociation constant, calculated as k ₋₁/k ₊₁). Part D lists the fractional occupancies of the metal sites in the resting state, as calculated from the values of K _d and the values assumed for resting [Ca²⁺] and [Mg²⁺].

The Reactions of Ca²⁺ and Mg²⁺ with ATP

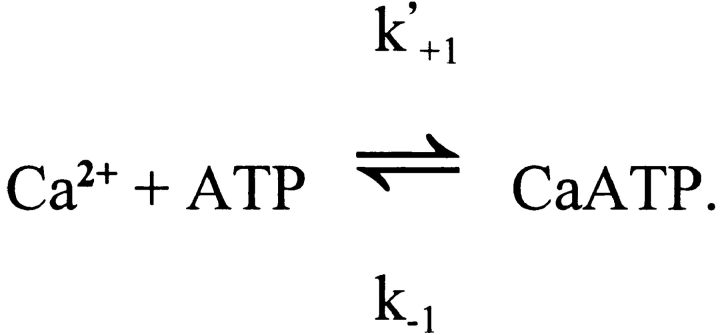
The competitive reaction of ATP with Ca²⁺ and Mg²⁺ is summarized as follows:

For ATP⁴⁻, Eigen and Wilkins (1965) report values of k ₊₁ and k ₊₂ of > 10⁹ M⁻¹ s⁻¹ and 1.3 × 10⁷ M⁻¹ s⁻¹, respectively (25°C; ionic strength, 0.1–0.2 M), whereas under similar conditions the values of K _d,Ca (= k ₋₁/k ₊₁) and K _d,Mg (= k ₋₂/k ₊₂) are approximately 60 μM and 30 μM, respectively (Botts et al., 1965; Phillips et al., 1966). Thus, k ₋₁ and k ₋₂ are calculated to be >60,000 s⁻¹ and 390 s⁻¹, respectively. At 16°C and a viscosity of 2 cP (i.e., appropriate to the model conditions), reaction rates would be smaller, with k ₋₁ and k ₋₂ values of perhaps 30,000 s⁻¹ and 150 s⁻¹, respectively. Moreover, at the pH (∼7) and K⁺ concentration (∼140 mM) of myoplasm, the effective values of K _d,Ca and K _d,Mg are elevated because of partial binding of K⁺ and H⁺ to ATP⁴⁻. Under these conditions, we estimate that K _d,Ca and K _d,Mg are ∼200 and ∼100 μM, respectively (Botts et al., 1965; Phillips et al., 1966; Martell and Smith, 1974). Thus, in the model, the values assumed for k ₊₁ (= k ₋₁/K _d) and k ₊₂ (= k ₋₂/K _d) are 1.5 × 10⁸ M⁻¹ s⁻¹ and 1.5 × 10⁶ M⁻¹ s⁻¹, respectively.

Given these reaction rates, single-compartment (i.e., spatially homogeneous) calculations were carried out to estimate the kinetic response of the ATP reactions if driven by a substantial Ca²⁺ transient. The total concentration of ATP was assumed to be 8 mM, a value near the middle of the range of values recently reported for fast-twitch fibers, 5–10 mM (Kushmerick, 1985; Godt and Maughan, 1988; Thompson and Fitts, 1992). (Note: As for the other species of this article, the ATP concentration is referred to the myoplasmic water volume; see Baylor et al., 1983; Godt and Maughan, 1988.) The free [Mg²⁺] was assumed to be 1 mM and constant.

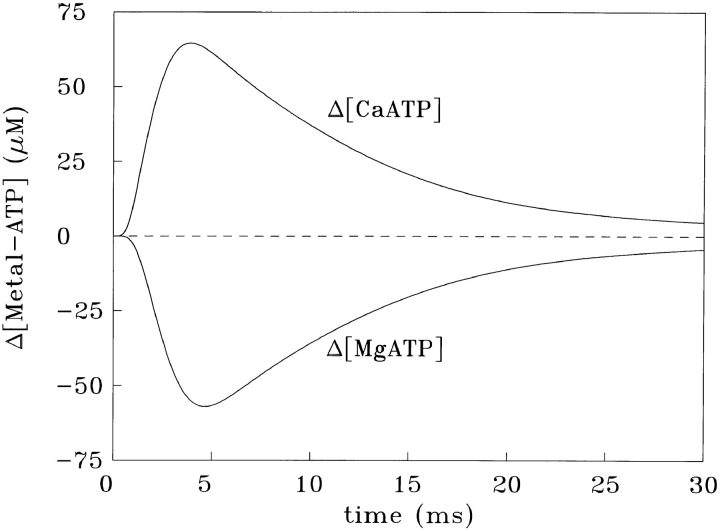
Fig. 2 shows the responses of Schemes SB and SC if driven simultaneously by a Δ[Ca²⁺] of peak amplitude 18.0 μM, a time-to-peak of 2.90 ms, and half-width of 5.90 ms, i.e., similar to that expected for the spatially averaged Δ[Ca²⁺] of a single myofibril (see results). The Δ[CaATP] response (upper trace) has a time-to-peak of 2.98 ms and a half-width of 6.09 ms; as a waveform, it is virtually indistinguishable from that of Δ[Ca²⁺] (not shown). The amplitude of Δ[CaATP], however, at 63.9 μM, is 3.6-fold larger than that of Δ[Ca²⁺]. The factor 3.6 comes from the ratio of total [ATP] (8 mM) to the effective value of K _d,Ca in the presence of 1 mM free [Mg²⁺] (2.2 mM = the actual K _d,Ca of 200 μM times the factor {1 + [Mg²⁺]/K _d,Mg}; see Scheme SB). Fig. 2 shows that, on a millisecond time scale, ATP behaves as a rapid and linear Ca²⁺ buffer, with the concentration of Ca²⁺ bound to ATP being nearly fourfold larger than that of free [Ca²⁺].

Figure 2.

Single-compartment simulation of the response of 8 mM total ATP to a [Ca²⁺] transient of peak amplitude 18.0 μM, time-to-peak 2.90 ms, and half-width 5.90 ms. Schemes SB and SC were simulated with the Ca²⁺ and Mg²⁺ reaction rates given in the text; free [Mg²⁺] was 1 mM and constant.

Scheme B.

Scheme C.

The lower trace in Fig. 2 shows the Δ[MgATP] response for the same calculation; the peak change is −53.4 μM. With a time-to-peak of 3.73 ms and half-width of 6.96 ms, the Δ[MgATP] waveform also closely tracks Δ[Ca²⁺], although not quite as faithfully as does Δ[CaATP]. Because ATP transiently releases ∼53 μM total Mg²⁺, an increase in free [Mg²⁺] would occur if the solution were not well buffered for Mg²⁺. In the case of myoplasm, any Mg²⁺ released by ATP would be buffered by phospho-creatine (primarily), which would limit the increase in free [Mg²⁺] to about one-third the increase in total [Mg²⁺] (e.g., Baylor et al., 1985). Thus, in myoplasm, spatially averaged free [Mg²⁺] would remain nearly constant, rising by only ∼2% relative to the resting level of 1 mM.

Since the Δ[CaATP] response in Fig. 2 is fast and linear and the implied increase in myoplasmic free [Mg²⁺] is small, the Δ[CaATP] response can be closely approximated by an equivalent reaction (termed here the “reduced” reaction), which omits consideration of Δ[MgATP]:

For this reaction, it is assumed that k ₋₁ has the same value as does Scheme SB, but that k′₊₁ is 11-fold smaller than k ₊₁, 1.36 × 10⁷ M⁻¹ s⁻¹ (= 1.5 × 10⁸ M⁻¹ s⁻¹/11). This decrease reflects the assumption that resting free [Mg²⁺] is 1 mM (10-fold higher than K _d,Mg), which reduces by 11-fold the fraction of total ATP that is immediately available to react with Ca²⁺. Thus, the 11-fold reduction in k ₊₁ accounts for the 11-fold increase in effective value of K _d,Ca due to 1 mM [Mg²⁺]. The response of Scheme SD to the same Δ[Ca²⁺] driving function used for Fig. 2 was also calculated (not shown). As expected, this Δ[CaATP] response was virtually identical to that of Δ[CaATP] shown in Fig. 2; it had a peak amplitude of 64.9 μM, a time-to-peak of 2.93 ms, and a half-width of 5.94 ms (vs. 63.9 μM, 2.98 ms and 6.09 ms, respectively, for Δ[CaATP] in Fig. 2). Thus, the reduced reaction (Scheme SD), which speeds and simplifies the calculations of Δ[CaATP] in the multi-compartment model, closely approximates the complete reaction system (Schemes SB and SC). Although it is possible that other constituents of myoplasm might also bind significant concentrations of Ca²⁺, our examination of the list of constituents for frog myoplasm (Godt and Maughan, 1988) indicates that ATP is the major (known) species that, to date, has not been included in kinetic models of Ca²⁺ binding in skeletal muscle. Phospho-creatine, although present in resting fibers at a concentration that is approximately four times larger than that of ATP, has, in the presence of 1 mM free [Mg²⁺], an effective value of K _d,Ca that is about 16-fold larger (36 mM vs. 2.2 mM) (cf., Smith and Alberty, 1956; O'Sullivan and Perrin, 1964; Sillen and Martell, 1964). Thus, the ability of phospho-creatine to act as a Ca²⁺ buffer is expected to be only ∼25% of that of ATP. For other compounds that are present at millimolar or near millimolar concentrations in myoplasm, e.g., inorganic phosphate and carnosine, the Ca²⁺ buffering effect is expected to be no more than a few percent of that of ATP (Sillen and Martell, 1964; Lenz and Martell, 1964; Godt and Maughan, 1988).

Scheme D.

Ca²⁺ Release from the SR

The form of the equation assumed in the multi-compartment model for SR Ca²⁺ release in response to an action potential is

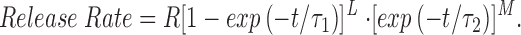3

Release rate has units of micromoles of Ca²⁺ per liter of myoplasmic water per millisecond (μM/ms) and its time course, in the absence of SR Ca²⁺ depletion, reflects the open time of the SR Ca²⁺-release channels. The choice of a product of exponentials, as given on the right-hand side of Eq. 3, is empirical. The values selected for τ₁, τ₂, L, and M (1.5 ms, 1.9 ms, 5 and 3, respectively) give a waveform of SR Ca²⁺ release that is similar to the release waveform estimated with our single-compartment model when driven with experimental measurements of Δ[Ca²⁺] (see results). With these selections, the time-to-peak and half-width of the release rate are 1.70 and 1.93 ms, respectively. The value chosen for R varied with the particular model being examined (see results) but was usually adjusted so that the peak of spatially averaged Δ[Ca²⁺] would be 18 μM, the value expected from the experimental measurements (cf., the first section of results). For the standard multi-compartment calculation with ATP (cf., Fig. 4), the value of R corresponds to a peak release rate of 141 μM/ ms. The corresponding spatially averaged total concentration of released Ca²⁺, which is given by the integral of release rate with respect to time, is 296 μM.

Figure 4.

18-compartment calculation with ATP included. A shows spatially averaged Δ[Ca²⁺]. B shows the Δ[CaTrop] responses for the nine compartments that contain troponin; a value of 446 μM on the ordinate corresponds to 100% occupancy of troponin with Ca²⁺. The three largest Δ[CaTrop] changes are from the three radial compartments adjacent to the Z line. Among these three, the peak rate of rise progressively decreases from edge to middle to center of the myofibril. A similar progression is seen for the three Δ[CaTrop] changes of intermediate size (which correspond to the middle third of the thin filament) and for the three smallest Δ[CaTrop] changes (which correspond to the thin filament regions most distant from the Z line). C shows the 18 individual Δ[CaATP] responses. Their amplitudes decrease progressively in a manner analogous to that described in B. Only the three largest Δ[CaATP] responses (from the three radial compartments adjacent to the Z line) are well resolved individually; for the other changes, the radial gradient is almost negligible, and thus, at the display gain shown, traces for the radial compartments at other axial locations are indistinguishable. D shows the 18 individual Δ[CaParv] changes, again with an analogous progression in amplitudes. The amplitude of the SR Ca²⁺ release function (cf., Eq. 3) was 141 μM/ms if referred to the myoplasmic volume of the half-sarcomere. See Table I for a summary of other model parameters.

Ca²⁺ Uptake by the SR

The form of the equation assumed for Ca²⁺ uptake from the half-sarcomere by the SR Ca²⁺ pump is

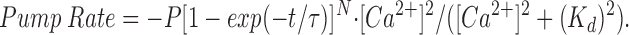4

The minus sign signifies that Ca²⁺ is removed from the myoplasm, and P gives the maximum removal rate (units of μM/ms). The choice of functional form for the remaining terms reflects the relatively short time scale of the calculations (≤30 ms) and is largely empirical. With τ and N chosen to be 1 ms and 10, respectively, the exponential term gives a small delay (2–3 ms) for pump activation after initiation of the calculation. The introduction of this delay, while somewhat arbitrary, permits the initial binding of Ca²⁺ by troponin to precede the initial pumping of Ca²⁺ by the SR Ca²⁺ pump. With the parameter P selected to be 1.5 μM/ms (concentration referred to the entire half-sarcomere) and with K _d selected to be 1 μM, the return of spatially averaged Δ[Ca²⁺] towards baseline at later times in the calculation (10–30 ms) is similar to that observed experimentally (cf., Figs. 3 and 4 A of results). Although it is possible in principle to include a reaction mechanism for the pump that explicitly calculates the concentration of Ca²⁺ bound by the pump (e.g., the 11-state cycle of Fernandez-Belda et al., 1984—for example, as implemented by Pape et al., 1990, in their single-compartment model), this approach was deemed too complicated and very unlikely to change the main conclusions of this article. As an additional simplification, the resting removal of Ca²⁺ by the SR Ca²⁺ pump and the resting leak of Ca²⁺ through the efflux channels were assumed to be zero.

Figure 3.

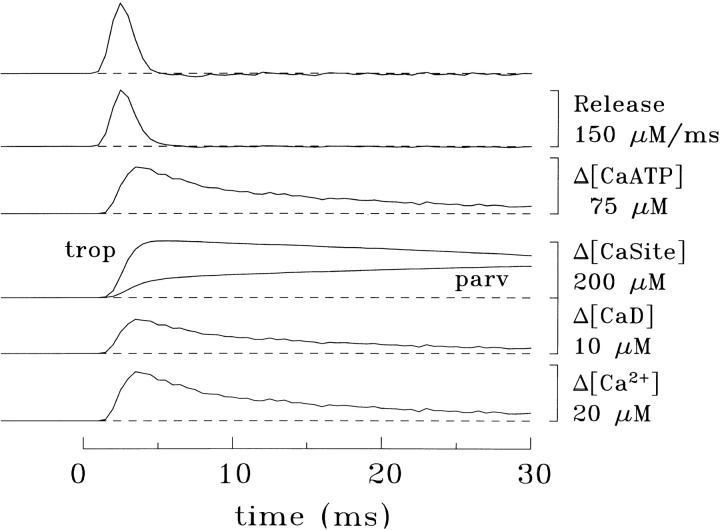
Example of the single-compartment model of Baylor et al. (1983) to estimate SR Ca²⁺ release (top two traces, referred to in the text as the dΔ[Ca_T]/dt signal); the uppermost calibration bar applies to both traces. The furaptra Δ[CaD] signal (second trace from the bottom) was measured in a frog fiber of small diameter (45 μm). Δ[Ca²⁺] (bottom trace) was estimated from the furaptra signal by the single-compartment method described in the text and used to calculate the Δ[CaTrop], Δ[CaParv],] and Δ[CaATP] responses. Fiber reference, 032896.2; sarcomere length, 3.8 μm; 16°C; furaptra concentration, 0.04 mM.

Implementation

Calculations and figure preparation were carried out on a DOS platform (100 MHz Pentium computer) with programs written in MLAB (Civilized Software, Bethesda, MD), a high-level language for differential equation solving, curve fitting, and graphics. In the 18-compartment model with ATP included, the total number of differential equations requiring simultaneous solution is ∼100. This number is close to the maximum possible number of such equations that the 1997 DOS version of MLAB can handle. Because of this constraint, the “reduced” reaction of Ca²⁺ with ATP (Scheme SD) was used in the multi-compartment calculations with ATP included.

Single Fiber Measurements

Intact single twitch fibers of semi-tendinosus or iliofibularis muscles of Rana temporaria were isolated and pressure injected with furaptra. The indicator concentration in myoplasm was sufficiently small (<0.2 mM) that the fiber's Δ[Ca²⁺] signal in response to action potential stimulation was not altered significantly by the indicator. The furaptra fluorescence signal was measured and calibrated as described previously (Konishi et al., 1991; Zhao et al., 1996).

results

Summary of Experimental Features of Δ[Ca²⁺] in Response to an Action Potential

Our previous experiments that measured spatially averaged Δ[Ca²⁺] in response to an action potential (16°C) provide an important constraint for the evaluation of the multi-compartment model of this article. Most of these experiments used intact frog twitch fibers of typical diameter (∼90 μm) and used furaptra, a lower-affinity, rapidly reacting fluorescence indicator (Konishi et al., 1991; Hollingworth et al., 1996; Zhao et al., 1996). However, any attempt to relate the properties of the furaptra fluorescence measurements to the Δ[Ca²⁺] of a single myofibril involves several complications.

First, the myoplasmic value of furaptra's K _d,Ca is uncertain. Our calibration of the furaptra fluorescence signal uses a K _d,Ca of 98 μM (16°C), which is the value obtained from a comparison of the furaptra measurements with the Δ[Ca²⁺] signal from PDAA (Konishi and Baylor, 1991; Konishi et al., 1991). Because PDAA is a rapidly reacting Ca²⁺ indicator of low-affinity (K _d,Ca ≈ 1 mM) and does not bind strongly to myoplasmic constituents, PDAA is thought to give the most reliable available estimate of Δ[Ca²⁺] (Hirota et al., 1989). The value of 98 μM for furaptra's myoplasmic K _d,Ca is about twofold higher than the 49 μM value estimated for the indicator in a salt solution (16°C, free [Mg²⁺] = 1 mM); an increased value is expected in myoplasm because of the binding of furaptra to myoplasmic constituents (Konishi et al., 1991). From the average experimental value in frog fibers (0.144) observed for the peak of furaptra's Δf _CaD signal (the change in the fraction of indicator in the Ca²⁺-bound form due to an action potential), the average value calibrated for the peak of Δ[Ca²⁺] is 16.5 μM (Hollingworth et al., 1996; Zhao et al., 1996). From the same measurements, the average values estimated for time-to-peak and half-width of Δ[Ca²⁺] are 5.0 and 9.6 ms, respectively.

Second, as noted by Konishi et al. (1991), who made simultaneous measurements of Δ[Ca²⁺] with PDAA and furaptra from the same region of the same fiber, the furaptra measurements may overestimate slightly the actual values for the time-to-peak and half-width of Δ[Ca²⁺]. This follows because the time-to-peak and half-width values measured with PDAA were slightly briefer (by about 0.3 and 1.5 ms, respectively) than the furaptra measurements.

Third, as noted by Hollingworth et al. (1996), a slightly larger and briefer Δ[Ca²⁺] signal is found in experiments with smaller-diameter frog fibers. In four such fibers (diameters 45–54 μm), the average furaptra Δ[Ca²⁺] values were 17.3 μM for peak, 4.4 ms for time-to-peak, and 8.2 ms for half-width (compared with 16.5 μM, 5.0 and 9.6 ms, respectively, for ordinary-sized fibers—mentioned above). These differences presumably arise because delays associated with radial propagation of the tubular action potential (Adrian and Peachey, 1973; Nakajima and Gilai, 1980) are smaller in smaller diameter fibers. Thus, the dispersive effects on the spatially averaged Δ[Ca²⁺] signal due to nonsynchronous activation of individual myofibrils should be smaller. We assume that if measurements could be made in the absence of any radial delays, Δ[Ca²⁺] would be slightly larger and briefer.

Based on these considerations, we expect that the following approximate values should apply to Δ[Ca²⁺] of a single myofibril at 16°C: peak amplitude, ∼18 μM; time-to-peak, ∼4 ms; half-width, ∼6 ms. In the absence of longitudinal propagation delays (appropriate for the multi-compartment model), the value for time-to-peak is expected to be ∼3 ms.

Summary of Estimates of SR Ca²⁺ Release Obtained with the Single-compartment Model

The furaptra Δ[Ca²⁺] measurements can be used as input to the single compartment model of Baylor et al. (1983) to estimate the amplitude and time course of SR Ca²⁺ release (e.g., Hollingworth et al., 1996). With this model, it is assumed that myoplasmic changes occur uniformly in space and that the change in total myoplasmic Ca²⁺ concentration due to SR release (Δ[Ca_T]) can be estimated from the summed changes of Ca²⁺ in four pools: (a) Δ[CaD] (the change in concentration of Ca²⁺ bound to furaptra, which can be directly calibrated from the measured change in indicator fluorescence, ΔF), (b) Δ[Ca²⁺] itself (calibrated as described in the previous section), (c) Δ[CaTrop], and (d) Δ[CaParv]. Given Δ[Ca²⁺] and the assumed resting [Ca²⁺] of 0.1 μM, changes c and d can be calculated from Eq. 1 (described in materials and methods) and the reaction parameters given in Table I.

Fig. 3 shows an example of this model applied to measurements from a frog fiber of small diameter (45 μm). The four lower traces show the estimated changes in Ca²⁺ concentration in the four pools described in the preceding paragraph. The next trace (Δ[CaATP]) shows the estimated concentration change in a fifth pool, that of Ca²⁺ bound to ATP (cf., Fig. 2). Two Δ[Ca_T] traces were computed (not shown). The first, which equaled the sum of the concentration changes in the original four pools, had a peak value of 291 μM and a time-to-peak of 6.5 ms; the second, which also included the contribution of Δ[CaATP], had a peak value of 339 μM and a time-to-peak of 5.5 ms. The two traces at the top of Fig. 3 show the time derivative (dΔ[Ca_T]/dt) of the two Δ[Ca_T] signals; these traces supply two estimates of the net flux of Ca²⁺ between SR and myoplasm (i.e., release rate minus uptake rate). The large early positive deflections essentially reflect the release process. The effect of Ca²⁺ uptake is apparent only at later times when, with the cessation of release, the traces go slightly negative. The smaller of the two dΔ[Ca_T]/dt signals (second from top) had a peak value of 146 μM/ms, a time-to-peak of 2.5 ms, and a half-width of 1.8 ms, whereas the larger signal (top), which includes the contribution of Δ[CaATP], had a peak value of 183 μM/ms, a time-to-peak of 2.5 ms, and a half-width of 1.8 ms.

Results similar to those in Fig. 3 were observed in a total of four small-diameter frog experiments. Without inclusion of ATP, the average values (±SEM) estimated for the Δ[Ca_T] signal were 298 ± 4 μM for peak amplitude and 6.5 ± 0.1 ms for time-to-peak; with inclusion of ATP, the values were 351 ± 9 μM and 5.6 ± 0.1 ms, respectively. For the dΔ[Ca_T]/dt signal, the average values without inclusion of ATP were 142 ± 4 μM/ms for peak amplitude, 2.9 ± 0.2 ms for time-to-peak, and 1.9 ± 0.1 ms for half-width; with ATP, the values were 176 ± 7 μM/ms, 2.9 ± 0.2 ms, and 1.9 ± 0.1 ms, respectively. All values for time-to-peak likely include a small delay, ∼1 ms, because of action potential propagation.

These calculations indicate that the inclusion of ATP, with properties as specified in Table I, in the single-compartment model of Baylor et al. (1983) increases the estimated peak value of Δ[Ca_T] by about 53 μM (18%) and that of dΔ[Ca_T]/dt by about 34 μM/ms (24%). Interestingly, these changes occur with very little change in the main time course of the dΔ[Ca_T]/dt signal, as the estimates for time-to-peak and half-width of release were unaltered. This finding supports the use of the SR Ca²⁺ release function described in materials and methods (Eq. 3) as the starting point for the calculations with the multi-compartment model.

Results of the Multi-compartment Model without Inclusion of ATP

At the outset, it is useful to note two important conceptual differences between single- and multi-compartment modeling. First, with a single-compartment model, calculations can be applied in either of two logical directions: (a) backward, from Δ[Ca²⁺] to a release waveform (e.g., as in Fig. 3) or (b) forward, from the release waveform to Δ[Ca²⁺] (not shown). In contrast, with the multi-compartment approach, only calculations in the forward direction are practical because spatially averaged Δ[Ca²⁺] results from the summed changes in a number of different compartments (e.g., 18 as in Fig. 1). The procedure adopted for the multi-compartment calculations was thus to assume an SR Ca²⁺ release waveform as driving function and evaluate its success by a comparison of calculated spatially averaged Δ[Ca²⁺] with expectations from the measurements of Δ[Ca²⁺] (cf., first section of results). This evaluation compared values for peak amplitude, time-to-peak, and half-width of Δ[Ca²⁺]. Secondly, only the multi-compartment model calculates concentrations as a function of spatial location. Thus, single-compartment calculations are expected to have errors associated with an inability to estimate local gradients in Δ[Ca²⁺] and the associated gradients in Ca²⁺ bound to nonlinear (saturable) binding sites. In consequence, inconsistencies are expected to arise between single- and multi-compartment calculations with otherwise identical parameters.

The first calculations with the multi-compartment model did not include ATP and provide a useful baseline for assessment of the effect of the inclusion of ATP (next section). The amplitude initially selected for the parameter R in the release waveform driving function (Eq. 3) corresponds to a spatially averaged release rate of 142 μM/ms, the value estimated from the single-compartment model without ATP (preceding section). A striking result of this calculation (not shown) is that spatially averaged Δ[Ca²⁺] is very different from the expectations outlined in the first section of results. Its peak amplitude, 58 μM, is about threefold larger than expected (∼18 μM), and its half-width, 3.6 ms, is markedly briefer than expected (∼6 ms). The time-to-peak (3.2 ms), however, is close to expected (∼3 ms). This large discrepancy between the single- and multi-compartment results has two possible sources. First, there might be a significant error in the dΔ[Ca_T]/dt signal used to drive the multi-compartment model, in which case the effect of other parameter selections (including the omission of ATP) becomes difficult to evaluate. Alternatively, the dΔ[Ca_T]/dt signal may be approximately correct, in which case the omission of ATP and/ or the choice of the other model parameters must be quite significant.

Although it is possible that the dΔ[Ca_T]/dt signal, which is based on the single-compartment model, may have errors in both amplitude and time course, other experimental evidence supports the conclusion that the time course of the dΔ[Ca_T]/dt signal is approximately correct. This evidence comes from action potential experiments on fibers that contained millimolar concentrations of a high-affinity Ca²⁺ buffer such as fura-2 (Baylor and Hollingworth, 1988; Hollingworth et al., 1992; Pape et al., 1993) or EGTA (Jong et al., 1995). At millimolar concentrations, these buffers rapidly bind most of the Ca²⁺ that is released from the SR, and thus their optical signal, which is proportional to the amount of bound Ca²⁺, closely tracks Δ[Ca_T]. The time derivative of this signal had a half-width of ∼3 ms. Although this value is ∼1 ms larger than that of the dΔ[Ca_T]/dt waveform defined by Eq. 3, a larger experimental half-width is expected for two reasons. First, the fibers of these experiments were of typical diameter (∼90 μm) rather than small diameter. Second, because the myoplasmic Δ[Ca²⁺] signal in these fibers was reduced and abbreviated (due to the presence of millimolar Ca²⁺ buffer), there was likely relief from the process of Ca²⁺-inactivation of SR Ca²⁺ release (Baylor et al., 1983; Schneider and Simon, 1988). This process normally serves to abbreviate the time course of SR release.

Given this support for the time-dependent part of Eq. 3, it was of interest to redo the multi-compartment calculation described above with the amplitude factor R reduced so that the peak value of spatially averaged Δ[Ca²⁺] would be 18 μM, the value expected from the experimental measurements (cf., first section of results). To achieve this result, an R value of 89 μM/ms is required (instead of 142 μM/ms). In this case, however, the half-width of Δ[Ca²⁺] is only 2.6 ms, which is even briefer than calculated initially (3.6 ms) and less than half the expected value (∼6 ms). In summary, because these calculations failed to produce a spatially averaged Δ[Ca²⁺] that is acceptable in both amplitude and time course, the multi-compartment model appears to have some important error or omission unrelated to the use of Eq. 3 as driving function.

Results of the Multi-compartment Model with Inclusion of ATP

The next calculations included ATP, with the value of R set initially to 176 μM/ms (the value estimated from the single-compartment model with ATP; see second section of results). In this case, spatially averaged Δ[Ca²⁺] (not shown) has a peak value of 27.7 μM and a half-width of 10.6 ms. Both values are substantially larger than expected from the measurements (∼18 μM peak and ∼6 ms half-width) and again imply some significant error or omission.

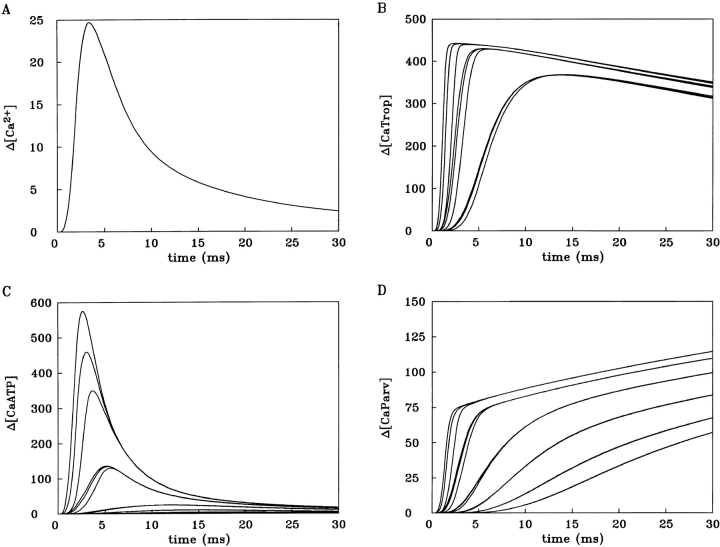
As in the preceding section, the multi-compartment calculation with ATP was then repeated but with the value of R lowered (to 141 μM/ms) so as to yield an amplitude of 18 μM for spatially averaged Δ[Ca²⁺]. The results of this calculation are shown in Fig. 4. Interestingly, spatially averaged Δ[Ca²⁺] (Fig. 4 A) has values for time-to-peak and half-width of 3.2 and 5.2 ms, respectively, which are quite close to the expected values (∼3 and ∼6 ms, respectively).

Fig. 4 B shows the associated calculations of Δ[CaTrop], which involve nine troponin-containing compartments. For Δ[CaTrop], a value of 446 μM on the ordinate corresponds to 100% occupancy of the troponin sites with Ca²⁺. (The 446 μM value is calculated from the 240 μM value given in Table I times a factor of two [since the troponin sites are located in only half of the compartments in Fig. 1] minus the resting occupancy of troponin with Ca²⁺, 34 μM [= 0.071 × 480 μM; cf., Table I].) In all nine compartments, the occupancy of troponin with Ca²⁺ reached a peak level that is close to saturation (>85%). Thus, the underlying Ca²⁺ transients in the troponin-containing compartments are of sufficient amplitude and duration to give nearly complete activation of troponin along the entire thin filament, as expected from fiber mechanical measurements (e.g., Gordon et al., 1964).

Fig. 4, C and D, shows the calculations of Δ[CaATP] and Δ[CaParv], respectively, in the 18 compartments. The calculations of Δ[Ca²⁺] for the individual compartments are not shown, but the time course and relative amplitude of these changes are closely similar to those shown in Fig. 4 C for Δ[CaATP]. This follows because (a) as mentioned in materials and methods, on the time scale shown, the Ca²⁺-ATP reaction is virtually in kinetic equilibrium with Δ[Ca²⁺], and (b) since the effective value of ATP's K _d,Ca is large (2.2 mM; Table I), the Ca²⁺-ATP reaction deviates by <10% from linearity even for Ca²⁺ transients as large as 100 μM (the amplitude of Δ[Ca²⁺] in the outer-most compartment nearest the Z line in the calculation of Fig. 4; not shown). Hence, the Δ[Ca²⁺] changes for all compartments can be closely approximated from the Δ[CaATP] changes in Fig. 4 C if the latter are scaled by the factor 1/3.6 (see materials and methods). Similarly, spatially averaged Δ[CaATP] can be closely approximated from the spatially averaged Δ[Ca²⁺] waveform shown in Fig. 4 A if scaled by the factor 3.6. For spatially averaged Δ[CaATP], the actual values of peak amplitude, time-to-peak, and half-width are 63.7 μM, 3.2 ms, and 5.3 ms, respectively.

The principal conclusion from the calculation of Fig. 4 is that, with ATP included as a diffusible Ca²⁺-binding species, spatially averaged Δ[Ca²⁺] is close to expectation if the value of R in Eq. 3 is ∼140 μM/ms. Based on (a) the fact that ATP is present in myoplasm at millimolar concentrations and presumably reacts with Ca²⁺ with reaction rate constants close to those listed in Table I, and (b) the finding of a great improvement in the agreement between calculated and measured Δ[Ca²⁺] with inclusion of ATP in the multi-compartment model, two conclusions appear to be warranted. First, ATP likely plays an important role in the binding and transport of myoplasmic Ca²⁺. Second, apart from a small time shift due to action potential propagation, the SR Ca²⁺ release function used in Fig. 4 is probably quite close to the actual SR Ca²⁺ release function of a small-diameter frog fiber.

As discussed in a later section of results, the need in Fig. 4 for an SR Ca²⁺ release function with an amplitude ∼20% smaller than that estimated from the single-compartment model with ATP included reflects errors in the single-compartment model due to its inability to calculate effects of local saturation of Ca²⁺-binding sites. The somewhat fortuitous result that the amplitude of the release function used in Fig. 4 (141 μM/ ms) is very close to that estimated in the single-compartment calculation without ATP included (142 μM/ ms; second section of results) is a related point that is also considered in a later section of results.

Role of ATP in Transporting Ca²⁺ within the Sarcomere

An additional feature of the calculation in Fig. 4 is that the diffusion of Ca²⁺ in the CaATP form is responsible for the spread of more total Ca²⁺ throughout the sarcomere than is the diffusion of free Ca²⁺. This follows from the observation that, at any myoplasmic location, Δ[CaATP] is ∼3.6-fold greater than Δ[Ca²⁺], whereas the diffusion constant of free Ca²⁺ is only 2.1-fold greater than that of ATP (Table I). Thus, the flux of Ca²⁺ across compartment boundaries will be ∼1.7-fold (= 3.6/2.1) greater for CaATP than for free Ca²⁺ (cf., Eq. 2).

To explore the importance of CaATP diffusion, it was of interest to repeat the multi-compartment calculation of Fig. 4 with the value of D _ATP reduced from 1.4 × 10⁻⁶ cm² s⁻¹ to 0. In this circumstance, the spread of Ca²⁺ depends primarily on the diffusion of free Ca²⁺. Fig. 5 shows the result, which reveals two significant points. First, a comparison of Figs. 5 B and 4 B shows that, with D _ATP reduced to 0, there is an increased occupancy of troponin with Ca²⁺ in the compartments nearest the Z line but a reduced occupancy in the compartments nearest the m-line, as well as a reduced rate of rise in the latter compartments. Thus, the transport of Ca²⁺ in the CaATP form that occurs if D _ATP = 1.4 × 10⁻⁶ cm² s⁻¹ results in a Ca²⁺-troponin occupancy that is more uniform and more synchronous. This presumably enables a more uniform and synchronous activation of fiber force.

Figure 5.

Multi-compartment calculation identical to that in Fig. 4, except that the diffusion constant of ATP was reduced from 1.4 × 10⁻⁶ cm² s⁻¹ to 0.

Second, spatially averaged Δ[Ca²⁺] in Fig. 5 A has a peak amplitude of 24.7 μM, a time-to-peak of 3.4 ms, and a half-width of 6.2 ms. Although these values are not markedly different from those in Fig. 4 A (18.0 μM, 3.2 ms, and 5.2 ms, respectively), they are substantially different from the values mentioned in the first multi-compartment calculations of results. In those calculations, with ATP omitted entirely, Δ[Ca²⁺] had a peak amplitude of 58.0 μM, a time-to-peak of 3.2 ms, and a half-width of 3.6 ms. Because the value of R in Eq. 3 was essentially identical for that calculation and the calculation of Fig. 5 (142 vs. 141 μM/ms, respectively), it follows that ATP produces a much smaller and broader Ca²⁺ transient simply through its ability to bind Ca²⁺ during the rising phase of Δ[Ca²⁺] and release it during the falling phase. Thus, independent of its ability to transport Ca²⁺, ATP acts as an important “temporal filter” of Δ[Ca²⁺].

Conclusions Based on an Examination of Changes to Other Parameters Listed in Table I

As described in the preceding sections, a significant binding and diffusive role for ATP is supported by the finding that inclusion of millimolar ATP in the model results in good agreement between the properties of calculated Δ[Ca²⁺] and those extrapolated from the measurements of Δ[Ca²⁺]. A further test of the significance of this result is to examine whether, without ATP, adjustment of one or several of the many other parameters of the model listed in Table I might produce a comparable improvement in the properties of calculated Δ[Ca²⁺]. Although it was not possible to make an exhaustive exploration of all such model adjustments, several changes were investigated that, in the absence of ATP, were designed specifically to improve the agreement between calculated and measured Δ[Ca²⁺]. None of the changes was found to make the substantial qualitative difference that resulted from the inclusion of ATP. These other changes included (a) a threefold reduction in the peak rate of SR Ca²⁺ pumping (the parameter P in Eq. 4), (b) a twofold increase in the value of the diffusion constant of free Ca²⁺ (D _Ca in Table I), (c) a threefold increase in the Ca²⁺-parvalbumin on-rate constant (k ₊₁ for parvalbumin in Table I), and (d) use of a smaller and broader SR Ca²⁺-release function. With changes a–c, whether implemented individually or simultaneously, there was no major improvement in the agreement between modeled and measured Δ[Ca²⁺]. With changes of type d, if sufficiently large, it was possible to produce a Δ[Ca²⁺] with a peak amplitude of ∼18 μM and a half-width of 5-6 ms, but these improvements were achieved only at the expense of the appearance of a slow foot on the rising phase of Δ[Ca²⁺] and a delayed time-to-peak of Δ[Ca²⁺] (∼5 ms). In sum, the inability of these changes to produce an acceptable spatially averaged Δ[Ca²⁺] further supports the idea that ATP does indeed contribute importantly to the determination of Δ[Ca²⁺].

The Possible Importance of other Myoplasmic Ca²⁺-binding Species

A related question is whether inclusion of other types of Ca²⁺-binding species in the multi-compartment model can produce improvements similar to that produced by ATP. For example, some neuronal cells appear to contain substantial concentrations of a nondiffusible, low- affinity Ca²⁺ buffer(s), which may strongly influence Δ[Ca²⁺] (Helmchen et al., 1996). This possibility was examined in our multi-compartment model by a comparison of the effects of such a hypothetical fixed buffer (HFB) with those of ATP. For these comparisons, HFB was assumed to be distributed in all myoplasmic compartments and have values of k ₊₁ and k ₋₁ identical to those listed in Table I for ATP. A further constraint for these calculations was that, for each concentration of HFB considered, the value of R (Eq. 3) was always adjusted so that the peak amplitude of Δ[Ca²⁺] would be 18 μM.

The first calculation assumed that ATP was absent but that HFB was present at a concentration of 8 mM. This situation is similar to that shown in Fig. 5, except that a smaller value of R is used (118 μM/ms) so as to yield an 18 μM Δ[Ca²⁺] transient. In this case, the values for time-to-peak and half-width of Δ[Ca²⁺] are 3.4 and 5.4 ms, respectively, which are essentially identical to those in Fig. 4 A (3.2 and 5.2 ms, respectively). Thus, in terms of the ability to generate a satisfactory Δ[Ca²⁺] response, the presence of HFB in the multi-compartment is very comparable to that of ATP. However, this calculation also reveals that, because of the inability of HFB to diffuse, there is substantially less occupancy of troponin with Ca²⁺ in the three troponin-containing compartments most distant from the Z line—on average, only 64% with HFB (vs. 86% with ATP; Fig. 4 B). As in Fig. 5, this calculation provides another demonstration of the importance of the diffusibility of a low-affinity buffer for achieving a high Ca²⁺-occupancy of troponin all along the thin filament and indicates that the calculations with HFB alone are not as satisfactory as those with ATP alone.

The second calculations with HFB assumed that ATP was present in the usual amount (8 mM) and examined how the presence of different concentrations of HFB affected the time course of Δ[Ca²⁺]. The first such calculation assumed a concentration of HFB equal to that of ATP, 8 mM. In this case, the required value of R for an 18 μM Δ[Ca²⁺] was 171 μM/ms, and the time-to-peak and half-width of Δ[Ca²⁺] were 3.6 and 9.9 ms, respectively. Since the value for half-width is substantially longer than expected (∼6 ms), it seems unlikely, given that skeletal muscle contains ∼8 mM ATP, that it also contains a similar or larger concentration of HFB.

The next step was to reduce the concentration of HFB to identify the value that would give a half-width for Δ[Ca²⁺] of 6 ms, i.e., essentially that expected from the experimental measurements. This concentration was 1.8 mM (with associated value of R = 148 μM/ms), and the value for time-to-peak of Δ[Ca²⁺] was 3.3 ms. Since the occupancy of troponin with Ca²⁺ in this calculation was also high in all of the troponin-containing compartments (>85%), the presence of this concentration of HFB in muscle seems plausible. Indeed, with 1.8 mM HFB, the time-to-peak and half-width of Δ[Ca²⁺] are in better overall agreement with the values expected from the experimental measurements than is the Δ[Ca²⁺] of Fig. 4 (time-to-peak, 3.2 ms; half-width, 5.2 ms).

In summary, these calculations indicate that it is unlikely that skeletal muscle contains a concentration of low-affinity fixed buffer (in ATP-equivalent units) as large as 10% of that postulated for nerve (Helmchen et al., 1996). However, the possibility that muscle contains a few percent of that postulated for nerve cannot be ruled out and, in fact, may be supported by the calculations.

A final calculation in this general category was to omit HFB entirely and identify what concentration of ATP alone would give values for peak and half-width of Δ[Ca²⁺] that were essentially the same as noted in the preceding paragraphs with the inclusion of 1.8 mM HFB. (Again, a constraint for these calculations was that, for each concentration of ATP considered, the value of R was readjusted to give a peak amplitude of 18 μM for Δ[Ca²⁺].) With 9.2 mM ATP and with an R of 148 μM/ms, the time-to-peak and half-width values of Δ[Ca²⁺] are 3.3 and 6.0 ms, respectively. Thus, inclusion of ATP alone at 9.2 mM (rather than 8 mM) gives a calculated Δ[Ca²⁺] that is virtually identical to that obtained with inclusion of 8 mM ATP and 1.8 mM HFB. As noted in materials and methods, the concentration of phospho-creatine found in muscle, ∼40 mM, approximates 2 mM of ATP-equivalent (diffusible) low-affinity Ca²⁺ buffer. Phospho-creatine thus provides a basis for a modest increase in the ATP-equivalent concentration used in the model.

In summary, the calculations of this section do not exclude, but also do not necessarily support, the presence of a small concentration of HFB in myoplasm. They do, however, argue against the likelihood of a concentration of HFB as large as 10% of that found in nerve.

Comparison of the Single-compartment Model without ATP and the Multi-compartment Model with ATP

In Fig. 4, the multi-compartment model with ATP was driven by an SR Ca²⁺ release function of amplitude 141 μM/ms, which is essentially identical to the 142 μM/ms value estimated from the single-compartment model without ATP (cf., Fig. 3). This similarity implies that the error in the single-compartment estimates of SR release associated with the omission of ATP are offset by other errors. Several factors appear to contribute to these other errors.

First, a single-compartment model does not consider separate myoplasmic regions with differing degrees of local saturation of binding sites. Thus, a single-compartment model will, for a given spatially averaged Δ[Ca²⁺], maximize—and thus over-estimate—the amount of Ca²⁺ captured by the intrinsic buffer sites included in the model (which are assumed to react with Ca²⁺ with a 1:1 stoichiometry). Moreover, the erroneous extra Ca²⁺ that the single-compartment model assigns to binding by the intrinsic buffers occurs early in time, when the myoplasmic gradients in [Ca²⁺] (as estimated by the multi-compartment model) are large. For example, the multi-compartment model estimates that Δ[Ca²⁺] in the compartments nearest the Z line rises rapidly to ∼100 μM, and as a result, there is rapid, local saturation of the troponin sites in these regions (Fig. 4 B). In contrast, in the other compartments, significant diffusional delays affect the rise of Δ[CaTrop]. Thus, in the single-compartment model, both kinetic and steady-state errors arise from the spatially homogeneous estimation of Ca²⁺ binding to the intrinsic buffers.

Second, calculations with the multi-compartment model show that some local saturation of furaptra with Ca²⁺ also occurs at early times near the release sites (see next section). This local saturation results in an estimate of Δ[Ca²⁺] from furaptra that has a later time-to-peak and broader half-width than does the actual Δ[Ca²⁺]. By itself, use of a delayed Δ[Ca²⁺] to drive the single-compartment model will result in an estimate of SR Ca²⁺ release that is delayed with respect to the actual release waveform.

Third, because of the same early local saturation of furaptra, the amplitude of spatially averaged Δ[Ca²⁺], if calibrated with the actual myoplasmic K _d,Ca of the indicator, will be underestimated. As discussed earlier, a value of 98 μM was assumed for furaptra's K _d,Ca so that the amplitude of Δ[Ca²⁺] calibrated from the indicator's Δf _CaD would agree with Δ[Ca²⁺] measured with PDAA. The next section shows that the 98 μM value is probably larger than the actual myoplasmic value, and its use in the single-compartment model partially compensates for the other errors that arise because of the local saturation of sites with Ca²⁺.

Characterization of Probable Error in the Previous Estimate of Furaptra's K_d,Ca and in the Single-compartment Estimates of SR Ca²⁺ Release

Fig. 6 shows several additional calculations associated with the multi-compartment model of Fig. 4. For these calculations, a nonperturbing concentration of furaptra (1 μM) was included as a separate Ca²⁺-binding species in all compartments, and the diffusion constant of furaptra was assumed to be 0.68 × 10⁻⁶ cm² s⁻¹ (Konishi et al., 1991). In Fig. 6 A, the continuous trace is identical to the spatially averaged Δ[Ca²⁺] shown in Fig. 4 A (called here “true” spatially averaged Δ[Ca²⁺], i.e., as calculated under the assumptions of the model). In Fig. 6 B, the spatially averaged Δf _CaD signal for furaptra was simulated by the multi-compartment model under two different assumptions about indicator properties. For both simulations, furaptra was assumed to have a value of k ₋₁ (Scheme SA) of 5,000 s⁻¹ (Zhao et al., 1997). For the first calculation (Fig. 6 B, dotted trace), a value of 5.1 × 10⁷ M⁻¹ s⁻¹ was assumed for k ₊₁ (thus K _d,Ca = 98 μM, as assumed by Konishi et al. [1991] and Zhao et al. [1996]); for the second calculation (Fig. 6 B, dashed trace), the k ₊₁ value was 7.1 × 10⁷ M⁻¹ s⁻¹ (K _d,Ca = 70 μM). Fig. 6 B shows that, with a K _d,Ca of 70 μM, the amplitude of Δf _CaD is significantly larger, 0.151 (vs. 0.120 if K _d,Ca is 98 μM). The 0.151 value is essentially identical to the average value of 0.150 observed for Δf _CaD in the experiments on small-diameter frog fibers (described in the first section of results). Thus, the dotted trace in Fig. 6 B indicates that the peak of ∼0.15 for furaptra's spatially averaged Δf _CaD signal cannot be explained under the assumptions that K _d,Ca is 98 μM and that the peak of spatially averaged Δ[Ca²⁺] is 18 μM. Since the peak of Δ[Ca²⁺] is thought to be close to 18 μM, we conclude that furaptra's myoplasmic K _d,Ca is likely to be closer to 70 μM than to 98 μM.

Figure 6.

Multi-compartment simulations to illustrate the effects of local furaptra saturation on the estimation of furaptra's Δ[Ca²⁺] by the single-compartment approach. The continuous trace in A is identical to that in Fig. 4 A, i.e., is “true” spatially averaged Δ[Ca²⁺] as calculated by the multi-compartment model with ATP included. The traces in B represent two multi-compartment simulations of the spatially averaged furaptra Δf _CaD signal based on two assumed values for K _d,Ca of the indicator in myoplasm (70 μM for the dashed trace, 98 μM for the dotted trace). For these calculations, a nonperturbing concentration of furaptra (1 μM) was included in each compartment in Fig. 1, but otherwise the simulation conditions were identical to those of Fig. 4. In A, the dashed trace is a single-compartment calculation of Δ[Ca²⁺], which is based on the dashed trace in B and the assumption that K _d,Ca is 98 μM.

Scheme A.

Considered as temporal waveforms, the two Δf _CaD responses in Fig. 6 B are essentially identical (times-to-peak, 3.8–3.9 ms; half-widths, 8.1–8.2 ms). Both times-to-peak are noticeably slower than the 3.2 ms time-to-peak of true spatially averaged Δ[Ca²⁺] (Fig. 4 A, continuous trace). The delay in time-to-peak of Δf _CaD is due to local saturation of furaptra with Ca²⁺ because no such delay is found if Δf _CaD is driven by Δ[Ca²⁺] in a single-compartment simulation (not shown).

The dashed trace in Fig. 6 A is a single-compartment calculation of spatially averaged Δ[Ca²⁺] based on the dashed Δf _CaD response in Fig. 6 B; for this conversion, a furaptra K _d,Ca of 98 μM was used, and the steady-state form of the 1:1 binding equation was assumed. This trace thus simulates previous experimental estimates of Δ[Ca²⁺] based on a furaptra Δf _CaD signal of typical amplitude and the previously assumed value of K _d,Ca. In Fig. 6 A, the peak amplitudes of Δ[Ca²⁺] are nearly identical (18 μM for the continuous trace, 17.5 μM for the dashed trace); this is expected since K _d,Ca for furaptra was chosen previously to make the amplitude of furaptra's Δ[Ca²⁺] agree with that of PDAA's (cf., first section of results). The time courses of the two changes in Fig. 6 A, however, are obviously different (time-to-peak of 3.2 ms and half-width of 5.2 ms for the continuous trace vs. 3.9 and 8.1 ms, respectively, for the dashed trace). As mentioned above, the fact that the time course of the simulated furaptra Δ[Ca²⁺] is slower than that of true Δ[Ca²⁺] reflects the effects of local saturation of the indicator with Ca²⁺, which the single-compartment calculation cannot take into account. This error in time course would be smaller for an indicator of lower affinity, which would undergo less local saturation. Indeed, if calculations analogous to those of Fig. 6 are carried out with PDAA (K _d,Ca ≈ 1 mM), the simulated peak amplitude of Δf _CaD is only 0.017, and the single-compartment conversion of Δf _CaD to spatially averaged Δ[Ca²⁺] yields a peak amplitude of 17.3 μM, a time-to-peak of 3.3 ms, and a half-width of 5.4 ms (calculations not shown). As expected, these values are very close to those of true Δ[Ca²⁺].

Since a number of previous publications, from this and other laboratories, have used a single-compartment model without ATP to estimate SR Ca²⁺ release parameters, it was of interest to use the multi-compartment model with ATP to characterize the likely errors in these estimates. Table II gives this information for estimates obtained with PDAA and furaptra. Column 1 (part A for Δ[Ca_T]; part B for dΔ[Ca_T]/dt) gives the information related to the release function used to drive the standard multi-compartment calculation with ATP (Fig. 4) and thus provides the “true” reference point for the comparisons in Table II. For the estimates in column 2, the dashed trace in Fig. 6 A was used as the Δ[Ca²⁺] to drive the single-compartment model. (As mentioned above, this trace simulates a furaptra Δ[Ca²⁺] signal, calibrated as in Fig. 3.) A comparison of column 2 with column 1 shows that, somewhat fortuitously, the single-compartment model without ATP provides generally accurate estimates of the true release parameters; the main error is a modest overestimation of the time-to-peak of release. Column 3 shows analogous release parameters based on use of the simulated PDAA Δ[Ca²⁺] signal mentioned above. Again, the release parameters in column 3 are in reasonable agreement with those in column 1. Overall, the SR release parameters estimated from furaptra are in slightly better agreement with the true release parameters than are those from PDAA, even though there is more error in the single-compartment estimate of spatially averaged Δ[Ca²⁺] with furaptra than with PDAA (see above and next section). This result, which is again somewhat fortuitous, indicates that the totality of errors inherent in the difference between the single-compartment model without ATP and the multi-compartment model with ATP (see preceding section) is offset slightly better with furaptra and its previous method of calibration (K _d,Ca = 98 μM) than with PDAA.

Table II.

SR Ca²⁺ Release Compared with Single- and Multi-compartment Models

		1		2		3
A. Δ[CaT]
Actual peak (μM)		296		—		—
Estimated peak (μM)		292		290		284
Time of estimated peak (ms)		5.8		6.1		5.3
B. dΔ[CaT]/dt
Peak (μM/ms)		141		141		153
Time-to-peak (ms)		1.70		2.07		2.00
Half-width (ms)		1.93		1.87		1.70

Column 1 refers to the multi-compartment model of Fig. 4, which includes ATP and is considered the reference point for the comparisons. Part B of column 1 gives the parameters of the release waveform used to drive this model. In part A, the “actual” peak is the value of this waveform integrated from time zero to infinity, whereas the “estimated” peak refers to the peak value of the waveform obtained as the sum of Δ[Ca²⁺], Δ[CaTrop], Δ[CaParv], and, in the case of column 1, Δ[CaATP]. (These waveforms reach a peak and then decay because of SR Ca²⁺ pumping.) Columns 2 and 3 give release parameters as estimated by the single-compartment model without ATP if driven by the multi-compartment simulation of spatially averaged Δ[Ca²⁺] (which was calculated for each of the indicators; see text). Column 2 is for a furaptra simulation (assumed K _d,Ca of furaptra is 70 μM for the multi-compartment simulation and 98 μM for the single-compartment reestimation of Δ[Ca²⁺]; see results). Column 3 is analogous to column 2 but is for a PDAA simulation (assumed K _d,Ca of PDAA, 1 mM for both parts of the simulation).  

General Analysis of Errors in Δ[Ca²⁺] Associated with Single-compartment Calculations

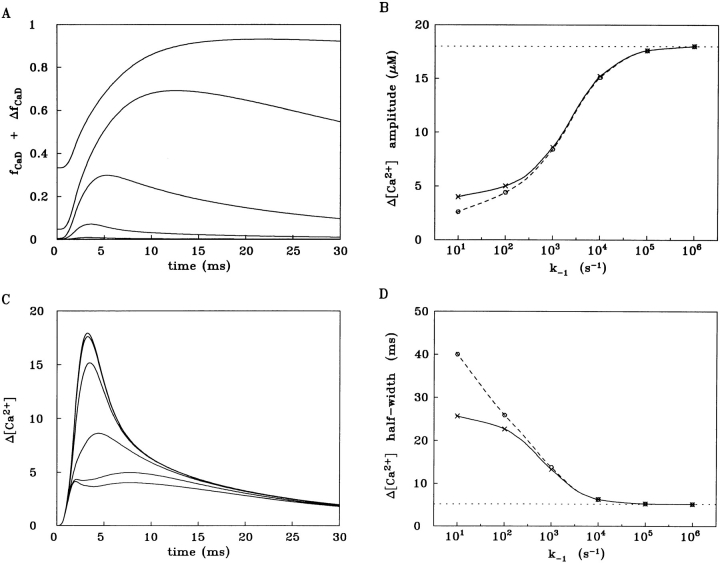
The preceding section compared single- and multi-compartment estimations of spatially averaged Δ[Ca²⁺] from furaptra and PDAA and noted several sources of error inherent in the single-compartment estimates. This section further characterizes these errors by means of analogous calculations applied to a hypothetical family of indicators. For this analysis, all indicators are assumed to react with Ca²⁺ with an identical value of k ₊₁ but with different values of k ₋₁ and hence different values of K _d,Ca. The value selected for k ₊₁, 5 × 10⁷ M⁻¹ s⁻¹, lies in the range considered for furaptra in the previous section (5–7 × 10⁷ M⁻¹ s⁻¹) and is probably also similar to that which applies to many members of the family of tetra-carboxylate Ca²⁺ indicators (cf., Tsien, 1980) when in the myoplasmic environment, e.g., indo-1, fura-2, fluo-3, calcium-orange-5N, etc. (Zhao et al., 1996). In general, these indicators bind heavily to myoplasmic constituents, and as a consequence, their rate constants for reaction with Ca²⁺ appear to be substantially reduced in comparison with those of the indicator in free solution. Six values of k ₋₁ were selected for these calculations: 10¹ s⁻¹, 10² s⁻¹,..., 10⁶ s⁻¹, with the corresponding values of K _d,Ca being 0.2 μM, 2 μM,..., 20 mM.

Fig. 7 (described in detail beginning with the next paragraph) summarizes the results of this analysis. As in the preceding section (cf., Fig. 6 and Table II), the multi-compartment model with ATP included is assumed to give the “true” results (Fig. 7, B and D, horizontal dotted lines) against which the simulated Δ[Ca²⁺] from each of the indicators can be compared. To calculate an indicator's Δ[Ca²⁺], the fraction of the indicator bound with Ca²⁺ (spatially averaged Δf _CaD plus the resting fraction, f _CaD; Fig. 7 A) was calculated by the multi-compartment model, based on a nonperturbing concentration of indicator (1 μM) included in all compartments. For simplicity, the myoplasmic diffusion constant of all indicators was fixed in the calculations at 0.25 × 10⁻⁶ cm² s⁻¹ (cf., Zhao et al., 1996). For the conversion of an indicator's f _CaD + Δf _CaD response to Δ[Ca²⁺], two different single-compartment methods were used. In the first method (Fig. 7, B and D, dashed curves), f _CaD + Δf _CaD was converted to Δ[Ca²⁺] by the steady-state form of the 1:1 binding equation, i.e., as was done for the conversion of the dashed Δf _CaD curve in Fig. 6 B to the dashed Δ[Ca²⁺] curve in Fig. 6 A. In the second method (Fig. 7, B and D, continuous curves; also traces in Fig. 7 C), the kinetic form of the 1:1 binding equation was used (see for example Baylor and Hollingworth, 1988; Klein et al., 1988; Hollingworth et al., 1992). Necessarily, the second method gives a Δ[Ca²⁺] with a larger peak amplitude and a briefer half-width than does the first method. Even though the second approach partially compensates for the kinetic lag between Δ[Ca²⁺] and Δf _CaD that arises when k ₋₁ is small, this method cannot be expected to correct for errors related to gradients in indicator saturation.

Figure 7.

Analysis of responses of a hypothetical family of Ca²⁺ indicators assumed to react with Ca²⁺ with a fixed value of k ₊₁ (5 × 10⁷ M⁻¹ s⁻¹) but with different values of k ₋₁ (order of magnitude increases from 10¹ to 10⁶ s⁻¹). All simulations started with the multi-compartment model with ATP, whereby “true” spatially averaged Δ[Ca²⁺] and “true” spatially averaged Δf _CaD of the indicators were calculated. A single-compartment model was then used to convert Δf _CaD to Δ[Ca²⁺] by one of two methods. The first approach (cf., B and D, dashed curves) used the steady-state form of the 1:1 binding equation. The second approach (cf., B and D, continuous curves) used the kinetic form of the same equation. A shows the calculation of f _CaD + Δf _CaD for the six multi-compartment simulations, whereas B shows the amplitude of spatially averaged Δ[Ca²⁺], as calculated from the f _CaD + Δf _CaD signals in the two single-compartment approaches. D is similar to B except that the half-width of Δ[Ca²⁺] is analyzed. C shows the spatially averaged Δ[Ca²⁺] traces calculated from the traces in A by the kinetic form of the 1:1 binding equation.

In Fig. 7 A, six time-dependent calculations of f _CaD + Δf _CaD are plotted, corresponding to the six different choices of k ₋₁. As k ₋₁ increases from 10¹ s⁻¹ to 10⁶ s⁻¹, f _CaD becomes progressively smaller and Δf _CaD becomes both briefer and smaller. At the largest value of k ₋₁, both f _CaD and Δf _CaD are too small to be resolved above baseline at the gain shown. With the first (steady-state) method of conversion of the traces in Fig. 7 A to Δ[Ca²⁺] (Δ[Ca²⁺] traces not shown), values of k ₋₁ approaching 10⁵ s⁻¹ or greater are required if both the peak amplitude and half-width of Δ[Ca²⁺] (Fig. 7, B and D, respectively, circle points connected by dashed curves) are to agree well with those of true Δ[Ca²⁺] (Fig. 7, B and D, horizontal dotted lines). With values of k ₋₁ ≤ 10⁴ s⁻¹, a progressively larger disparity is observed between the parameters of calculated and true Δ[Ca²⁺].

The traces in Fig. 7 C show the results of the second (kinetic) method of conversion of the traces in Fig. 7 A to Δ[Ca²⁺] and were used to calculate the second set of points in Fig. 7, B and D (cross points connected by continuous curves). As mentioned above, Δ[Ca²⁺] parameters are necessarily larger and briefer with this method and thus the continuous curves in Fig. 7, B and D, lie closer to the horizontal dotted lines than do the dashed curves. Interestingly, with this method of conversion, Δ[Ca²⁺] in Fig. 7 C becomes obviously biphasic at the two smallest values of k ₋₁ (10¹ s⁻¹ and 10² s⁻¹), and at the next larger value of k ₋₁ (10³ s⁻¹), a hump can be seen on the rising phase of Δ[Ca²⁺]. The appearance of two phases in Δ[Ca²⁺] is an artifact of local indicator saturation in combination with the use of a single-compartment kinetic correction to convert the f _CaD + Δf _CaD response to Δ[Ca²⁺]. The earlier phase, which rises to a plateau during the time of SR Ca²⁺ release, reflects effects of indicator saturation at sarcomeric regions close to the release sites. The later phase, which involves a delay in the rise of Δ[Ca²⁺] and Δf _CaD at sarcomeric locations more distant from the release sites, reflects the time required for Ca²⁺ to diffuse and bind to indicator in these locations. (Note: The biphasic response does not depend on the diffusion of indicator since it is also seen if the diffusion constant of the indicator is set to zero in the multi-compartment part of the calculation; not shown.)

Overall, the simulations in Fig. 7 indicate that, at values of k ₋₁ < 10⁴ s⁻¹, effects due to Ca²⁺ gradients and local saturation of indicator introduce significant error in single-compartment methods for estimation of true Δ[Ca²⁺]. Moreover, at k ₋₁ ≤ 10³ s⁻¹, these errors are quite severe. Thus, this analysis supports the conclusion that, to achieve an accurate estimate of true Δ[Ca²⁺] in spatially averaged measurements, it is highly desirable to use a low-affinity, rapidly responding indicator (Hirota et al., 1989).

Application of the Multi-compartment Model to Reestimate Myoplasmic Values of the Ca²⁺-fluo-3 Reaction Rates

Previous publications from this and other laboratories (e.g., Baylor and Hollingworth, 1985, 1988; Klein et al., 1988; Harkins et al., 1993; Kurebayashi et al., 1993; Pape et al., 1993; Westerblad and Allen, 1996; Zhao et al., 1996) have described single-compartment methods for estimation of Ca²⁺ reaction rates (k ₊₁ and k ₋₁) of a number of different indicators when in the myoplasmic environment. In the most common type of experiment, the same region of the same fiber was exposed to two indicators—usually a lower-affinity indicator (e.g., antipyrylazo III, furaptra, or PDAA) and a higher-affinity indicator (e.g., fura-2, fura-red, or fluo-3)—and optical measurements were made simultaneously from both indicators. The optical responses from the lower-affinity indicators (whether recorded in the same or different fibers) were usually very similar in time course, whereas the responses from the higher-affinity indicators, while somewhat variable in time course, always had significantly later times-to-peak and broader half-widths than did the lower-affinity responses. The slower responses of the higher-affinity indicators were assumed to reflect the smaller values of k ₋₁ that are inherent in these indicators being of higher-affinity, and the timing of these responses relative to the lower-affinity responses was used in single-compartment fits to estimate the values of k ₋₁ and k ₊₁ of the higher-affinity indicators. The results presented in the previous two sections, however, indicate that because of the effects of local indicator saturation, use of the single-compartment method will probably introduce significant error in the estimates of k ₋₁ and k ₊₁. It was therefore of interest to use the multi-compartment model to reestimate k ₋₁ and k ₊₁ values for one of the higher-affinity indicators, as a means of assessing the direction and magnitude of possible errors in the previous estimates.

The indicator selected for this analysis was fluo-3, which has been used in a number of recent measurements of local Ca²⁺ signals in muscle (e.g., Cheng et al., 1993; Tsugorka et al., 1995; Klein et al., 1996; Hollingworth et al., 1998). Results with the multi-compartment model were compared with the traces and single-compartment analysis of Harkins et al. (1993; cf., their Fig. 8). These authors reported average values for k ₊₁ and k ₋₁ of fluo-3 of 1.31 × 10⁷ M⁻¹ s⁻¹ and 33.5 s⁻¹, respectively (16°C), with the corresponding value of K _d,Ca being 2.56 μM. If these values are used in a multi-compartment calculation of the type shown in Fig. 6, a poor fit of the simulated data of Harkins et al. (1993) is obtained (not shown). Additional calculations were therefore carried out with the multi-compartment model to find values of k ₊₁ and k ₋₁ that gave a better fit to these data. A good fit was obtained with k ₊₁ and k ₋₁ values of 3.5 × 10⁷ M⁻¹ s⁻¹ and 55 s⁻¹, respectively (K _d,Ca of 1.57 μM). We conclude that the new estimates of k ₊₁ and k ₋₁ are probably closer to the actual rates that apply to fluo-3 in myoplasm and that the use of a single-compartment method probably underestimates the actual rates of higher-affinity indicators. Although the new estimates for fluo-3 are significantly larger than the previous estimates, they are still markedly smaller than the rates reported for the indicator in a simple salt solution (∼8 × 10⁸ M⁻¹ s⁻¹ and ∼400 s⁻¹ at 22°C and a viscosity of 1 cP; Eberhard and Erne, 1989; Lattanzio and Bartschat, 1991).

discussion

A Multi-compartment Model That Includes Ca²⁺-binding to ATP and Diffusion of CaATP

In this article, we describe a multi-compartment model of a half-sarcomere that calculates the spread of Ca²⁺ within a single myofibril of a frog twitch fiber. This model is similar to that first developed by Cannell and Allen (1994) but incorporates several important changes that reflect current knowledge of the Ca²⁺ release process and the myoplasmic environment. These changes include a smaller value for the diffusion constant of free Ca²⁺, a larger and briefer SR Ca²⁺ release event in response to an action potential, and the inclusion of ATP as a diffusible species that binds both Ca²⁺ and Mg²⁺. As shown in materials and methods, at the free Mg²⁺ concentration of myoplasm (∼1 mM), ATP behaves as a low-affinity, rapidly reacting Ca²⁺ buffer. Additionally, ATP (mol wt 507) has a diffusion constant in myoplasm (∼1.4 × 10⁻⁶ cm² s⁻¹; 16°C) that is about half that of Ca²⁺ (Kushmerick and Podolsky, 1969). Thus, there should be a significant transport of Ca²⁺ along the sarcomere in the CaATP form. Probably the most important result of our modeling is that, without ATP (or an analogous compound), the measurements of spatially averaged Δ[Ca²⁺] in frog fibers could not be adequately simulated with the SR Ca²⁺ release function that is thought to result from a single action potential (peak amplitude of ∼140 μM/ms, half-width of ∼2 ms). Thus, our calculations (a) implicate the presence of a significant concentration of a low-affinity, rapidly reacting Ca²⁺-binding species in myoplasm, and (b) show that ATP, with properties as reported in the biochemical and physiological literature, satisfies the requirements of this species. Since ATP is present in myoplasm at millimolar concentrations, is mobile, and is capable of binding Ca²⁺, it seems hard to avoid the conclusion that ATP plays a role similar to that inferred with our model. It is possible, of course, that other compounds, both mobile (e.g., phospho-creatine) and immobile (cf., Helmchen et al., 1996), also contribute to the effects that our model assigns to ATP. However, the contribution of these other compounds appears to be minor in comparison with that of ATP.

Other Implications of the Presence of ATP in the Model

The calculation in Fig. 2 shows that Δ[CaATP] is expected to be three to four times larger than, and nearly in kinetic equilibrium with, Δ[Ca²⁺]. Since the diffusion constant of ATP is about half that of free Ca²⁺ (Table I), it follows that more Ca²⁺ diffuses within myoplasm as CaATP than as free Ca²⁺. As shown by the comparison between Figs. 4 B and 5 B, the diffusion of CaATP helps synchronize the Ca²⁺-occupancy of troponin and thus activation of the myofilaments.

Given that the peak value of spatially averaged Δ[CaATP] is ∼65 μM, the question arises whether the presence of ATP requires that SR Ca²⁺ release be ∼65 μM larger than it would otherwise be if ATP were not present (or did not bind Ca²⁺). However, calculations (not shown) indicate that, to achieve a given high-level occupancy of the troponin regulatory sites, the amount of extra SR Ca²⁺ that must be released due to the presence of 8 mM ATP is only about 30 μM (∼270 μM in the absence of ATP vs. ∼300 μM in the presence of ATP). The difference between 65 and 30 μM reflects the fact that Ca²⁺ quickly dissociates from ATP when Δ[Ca²⁺] declines (cf., Figs. 2 and 4). Thus, Ca²⁺ from ATP is made available to bind to the troponin (and other) sites. Since the multi-compartment model indicates that slightly more than half of the Ca²⁺ that is bound by ATP is subsequently bound by troponin, the extra load that ATP adds to the SR release requirement is only ∼10%. For comparison, the increase in load due to the presence of parvalbumin is ∼20%. This follows from the observations that spatially averaged Δ[CaParv] also rises rapidly to about 65 μM but that the rate of Ca²⁺ dissociation from parvalbumin is very slow (cf., Table I). Thus, Ca²⁺ cannot dissociate from parvalbumin and be bound by troponin on the time scale of twitch activation.

Other Comparisons between the Single- and Multi-compartment Models

We were initially surprised that the estimate of SR Ca²⁺ release from the single-compartment model without ATP included supplied a satisfactory driving function for the multi-compartment model with ATP included. As considered in results, there appear to be several sources of offsetting error in the single-compartment model that account for this situation. As shown by the comparisons in Table II, the overall effect of these errors is offset slightly better in the case of Δ[Ca²⁺] measurements with furaptra (if calibrated with a K _d,Ca of 98 μM) than with PDAA, even though PDAA is thought to give a more reliable estimate of the actual time course of spatially averaged Δ[Ca²⁺].

Based on our multi-compartment model, we have reanalyzed the data reported by Harkins et al. (1993), who estimated the values of k ₊₁ and k ₋₁ for the Ca²⁺– fluo-3 reaction in myoplasm. While their reaction rates provide a useful empirical way to relate, in a single-compartment calculation, the Δf _CaD signal of fluo-3 to the Δ[Ca²⁺] signal of furaptra (calibrated by the method of Konishi et al., 1991), these rates may not accurately reflect fluo-3's actual myoplasmic values of k ₊₁ and k ₋₁. Indeed, our multi-compartment model indicates that because of local saturation of the indicator with Ca²⁺ near the SR release sites, there is likely to be error in the previous single-compartment analysis (as well as in comparable analyses reported elsewhere in the literature for other indicators—see results). The new values that we estimate for fluo-3's k ₊₁ and k ₋₁ are 2.7- and 1.6-fold higher, respectively, than the rates reported by Harkins et al. (1993). It should be noted, however, that in our multi-compartment model of the half-sarcomere, Ca²⁺ enters the myoplasm only at the outermost compartment nearest the Z line, whereas in reality, some Ca²⁺ may enter a myofibril from adjacent myofibrils and/or SR release sites that are not in registration with the Z line. Thus, the local Δ[Ca²⁺] gradients calculated by our model should probably be regarded as upper limits of the actual gradients. In support of this conclusion, our previous experiments with PDAA and furaptra (e.g., Fig. 6 B of Konishi et al., 1991) indicate that the difference between the time courses of spatially averaged Δ[Ca²⁺] measured with these two indicators is slightly less extreme than calculated by our multi-compartment model (Figs. 6 A and 7 D). This, in turn, suggests that the k ₊₁ and k ₋₁ values estimated for fluo-3 by the multi-compartment model may slightly overestimate the actual Ca²⁺–fluo-3 reaction rates in myoplasm.

Local indicator saturation probably also explains a finding reported by Pape et al. (1993), who used a single-compartment approach to estimate the value of k ₊₁ for fura-2 in the myoplasm of cut fibers from spatially averaged PDAA and fura-2 measurements. They found that the estimate of k ₊₁ varied with indicator concentration, increasing about twofold, from 3.5 × 10⁷ M⁻¹ s⁻¹ to 7 × 10⁷ M⁻¹ s⁻¹, as myoplasmic [fura-2] rose from 0.5 to 2 mM. Since the spatially averaged Δ[Ca²⁺] signal in these experiments was relatively large at a [fura-2] of 0.5 mM but nearly eliminated at a [fura-2] of 2 mM, it is possible that the estimate of k ₊₁ increased because local saturation of fura-2 with Ca²⁺ was substantially reduced at the higher fura-2 concentrations. This interpretation is supported by calculations (not shown) carried out with our multi-compartment model, which were designed to simulate the experiment of Pape et al. (1993). In these simulations, the estimate of k ₊₁ also increased twofold as the fura-2 concentration was increased from 0.5 to 2 mM. (Note: The value of k ₊₁ estimated for fura-2 in cut fibers is severalfold larger than the value of k ₊₁ estimated for fluo-3 in intact fibers [cf., last section of results]. Two effects probably underlie this difference. First, the binding of indicator to myoplasmic constituents has been associated with reductions in the values of indicator reaction rates, and fluo-3 appears to be more heavily bound in myoplasm than fura-2 [intact fiber measurements, summarized in Zhao et al., 1996]. Second, cut fibers appear to be ∼40% more hydrated than intact fibers [as judged by measurements of intrinsic birefringence; Irving et al., 1987]. Therefore, reaction rates, may, in general, be higher in cut compared with intact fibers.)

A related point concerns previous estimations of the resting level of myoplasmic [Ca²⁺] ([Ca²⁺]_R) with high-affinity indicators such as fluo-3 or fura-2. Often, the value estimated for [Ca²⁺]_R depends directly on an associated estimate of the myoplasmic value of the indicator's K _d,Ca. If K _d,Ca is obtained from kinetic fits of spatially averaged measurements with two Ca²⁺ indicators, the multi-compartment calculations indicate that the value of K _d,Ca is probably overestimated. For example, with fluo-3, our multi-compartment analysis implies that K _d,Ca for fluo-3 lies closer to 1.6 μM than to the 2.6 μM value estimated by Harkins et al. (1993). Corrected for this error, the fluo-3 data of Harkins et al. imply a narrower range of estimates for [Ca²⁺]_R, 0.10–0.14 μM rather than 0.10–0.24 μM. This correction helps slightly to reconcile the ∼10-fold difference for [Ca²⁺]_R (0.03–0.3 μM) reported in skeletal muscle with different techniques (cf., Baylor et al., 1994; Westerblad and Allen, 1994).

Generalizations and Speculations

Since ATP is present in most cells at millimolar concentrations, it seems likely that the significant effects of ATP deduced here on the shaping of local Ca²⁺ gradients also applies to the many other cell types that use Δ[Ca²⁺] to control their activity. For example, in secretory cells, the binding of Ca²⁺ by ATP and the diffusion of CaATP likely modify the amplitude and time course of the local Δ[Ca²⁺] signals that control vesicle release.

A final speculation relates to the possibility that Δ[CaATP] might itself serve as an intracellular signal. Since the value of K _d,Ca of ATP is large relative to Δ[Ca²⁺], Δ[CaATP] provides a rapid, local monitor of the product of Δ[Ca²⁺] and total [ATP]. Thus, a large Δ[CaATP] indicates both a substantial Δ[Ca²⁺] and a substantial total [ATP]. This signal might be used by the cell to activate novel regulatory pathways.

Abbreviations used in this paper

Δ[Ca²⁺]

free [Ca]

Δ[Ca_T]

the change in total Ca concentration

HFB

hypothetical fixed buffer

PDAA

purpurate-di-acetic acid

SR

sarcoplasmic reticulum