Setting the Stage for Molecular Dissection of the Regulatory Components of BK Channels

Despite remarkable diversity in the properties of large-conductance, calcium- and voltage-activated K⁺ channels (also termed “BK” or “maxi-K” channels) in different tissues, (McManus, 1991; Vergara et al., 1998), the defining characteristic of all BK channels is that their activation is controlled by two independent physiological stimuli, membrane voltage and cytosolic Ca²⁺ concentrations ([Ca²⁺]_i). This dual regulation by Ca²⁺ and voltage allows BK channels to play a more dynamic role in the regulation of cellular excitability than is possible with strictly voltage-gated K⁺ channel homologues, as the extent of activation during any particular depolarization is also linked to [Ca²⁺]_i. Since the initial discovery of BK channels, this dual regulation has naturally tantalized those interested in channel gating mechanisms, posing the fascinating question: what is the molecular mechanism by which two independent stimuli each influence the ability of the BK channel to open?

A priori one might imagine any of a number of mechanisms by which two distinct stimuli can regulate activation of a channel. One of the earliest proposals in this regard suggested that Ca²⁺ binding itself could be voltage-dependent (Moczydlowski and Latorre, 1983). Alternatively (but not exhaustively), Ca²⁺ and voltage-dependent steps might each act independently to promote channel activation, Ca²⁺ binding might directly influence the voltage-sensor equilibrium or Ca²⁺ might only exert its effect after movement of the voltage sensors. In the last 5 yr, there have been a stream of substantive papers that cumulatively have illuminated the mechanisms underlying regulation of BK channels by Ca²⁺ and voltage. Gratifyingly, a common theme permeates this work, whether BK channel gating has been studied with single channels (Rothberg and Magleby, 1999, 2000) or macroscopic currents (Cox et al., 1997; Cui et al., 1997), or whether gating has been probed using auxiliary β subunits (Cox and Aldrich, 2000; Nimigean and Magleby, 2000), mutations (Cui and Aldrich, 2000), Mg²⁺ (Shi and Cui, 2001; Zhang et al., 2001), Ca²⁺ (Rothberg and Magleby, 1999; Cox and Aldrich, 2000), voltage (Horrigan and Aldrich, 1999; Horrigan et al., 1999), or both Ca²⁺ and voltage (Rothberg and Magleby, 2000). All of these papers have contributed to the view that voltage and Ca²⁺ regulate allosterically BK channel activation by independently influencing the energetics of channel opening.

Yet, despite this evolving consensus, several important questions concerned with allosteric coupling between Ca²⁺ and voltage remain unresolved. Now in a compelling study in this issue of the Journal of General Physiology, Horrigan and Aldrich (2002) present an important extension of this path of investigation. Specifically, they address the question: “Does the allosteric effect of Ca²⁺ on BK channel activation occur via effects on voltage-sensor activation, channel opening, or both”? By using both gating current and ion current measurements, their work provides a more direct test of the allosteric mechanism and the linkage between ligand-binding and voltage-gating than in previous work. The conclusion from their work is that Ca²⁺ and voltage independently act to regulate channel opening, and that the interaction between Ca²⁺ binding and voltage-sensor movement is minor. This conclusion is reached through a combination of clever analytic methods and technically challenging experiments that allow detailed definition of the ionic and gating currents under conditions that independently illuminate voltage-sensor movement versus Ca²⁺-dependent channel activation.

Before highlighting the key experimental observations in this work, it is important to discuss the rationale for the use of particular allosteric models in evaluating mechanisms of Ca²⁺ and voltage dependence. A central tenet of the approach in the Horrigan and Aldrich paper is that “the simplest or most physically plausible mechanism often does not produce the fewest states.” Thus, although allosteric models seem to add complexity, by increasing the number of discrete conformational states required to describe channel function, in reality allosteric models provide a more simple and plausible physical conception of the mechanical steps involved in gating. Furthermore, as we shall see below, the elucidation of the allosteric regulation of BK channels by Ca²⁺ and voltage provided by Horrigan and Aldrich places strong constraints on the physical mechanisms that may be involved in BK gating. Before addressing such issues, it is interesting to consider this approach in light of the history of BK channel investigations.

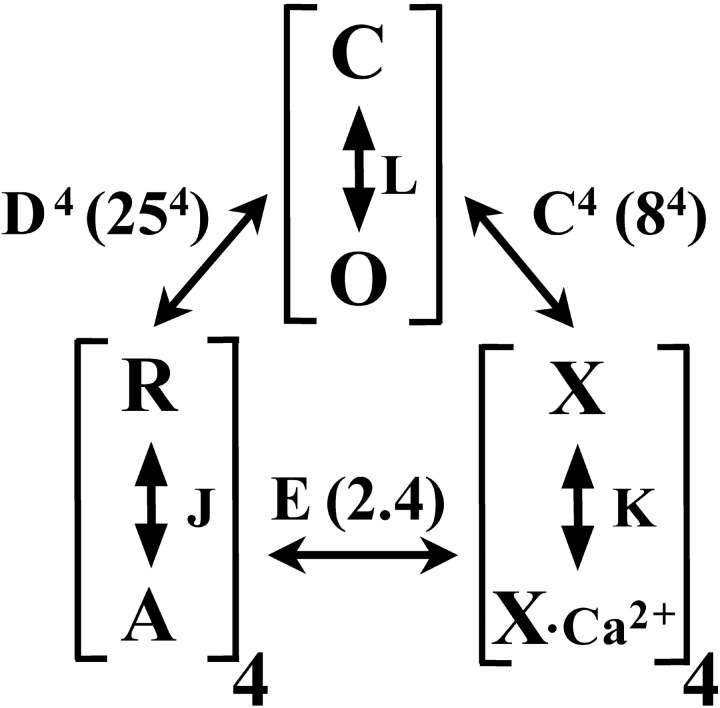
Since their initial discovery, BK channels have been particularly seductive subjects for detailed kinetic investigations. Both the large single channel conductance and the ease with which large numbers of single channel events can be obtained have facilitated an analysis of single channel behavior not possible for most other channels. Such studies have shown a complexity in channel behavior that has seemed daunting in terms of a physical interpretation of the molecular steps involved in channel gating. Historically, a focus of such studies has been the definition of Markovian state diagrams that arise from determination of the minimal number of distinct closed interval and open interval components that appear in the datasets (Moczydlowski and Latorre, 1983; McManus and Magleby, 1988, 1991) and from the dependencies among such intervals (Rothberg and Magleby, 1998). Although such studies have illuminated the complexities involved in BK gating, a major advance in understanding the role of Ca²⁺ binding and voltage gating was when functional analysis shifted to evaluation of physically plausible allosteric models that arise from consideration of structural information about the channel. In particular, the tetrameric structure of the channel suggests that each subunit within the tetramer contains both a voltage sensor and a high affinity Ca²⁺ binding site. For BK channels then, a plausible mechanism of allosteric gating arises when realizing that three processes contribute to gating: a channel opening equilibrium (C-O), Ca²⁺ binding, and voltage-sensor activation, as encapsulated in Scheme II of Horrigan and Aldrich (2002) (Fig. 1) . By definition, allosteric coupling between any two processes is then described solely by equilibrium constants for each process and an allosteric factor reflecting the coupling between the processes. Horrigan and Aldrich aimed to unravel the relative strength of interactions between each of these processes, which was accomplished through the use of novel approaches to isolate interactions between pairs of processes (e.g., channel opening and voltage-sensor movement or channel opening and Ca²⁺ binding).

Figure 1.

Diagram of Scheme II (Horrigan and Aldrich, 2002) summarizing the allosteric relationships between channel opening, voltage-sensor movement, and Ca²⁺ binding in BK channel activation. Channel opening (C-O) is defined by equilibrium constant, L; voltage-sensor movement (R-A) is defined by equilibrium constant J, with 4 voltage-sensors per channel; Ca²⁺ binding (X-X*Ca²⁺) is defined by binding constant K with four binding sites per channel. The allosteric coupling factors are given by C–E, with values estimated by Horrigan and Aldrich given in parentheses. Although Ca²⁺ binding and voltage-sensor movement relatively independently regulate the C-O transition, voltage-sensor movement is ∼100-fold more effective. When there is no coupling between two processes, the allosteric coupling factor is 1.

Several notable features of the Horrigan and Aldrich work deserve specific mention, but before these can be addressed it is necessary to describe how the effects of Ca²⁺ on BK gating often are measured. Since BK channels are activated by both Ca²⁺ and voltage, the relative effects of Ca²⁺ on gating can be expressed as an equivalent shift in voltage. For example, at 70 μM Ca²⁺, BK channels are half activated (P_o ∼0.5) at ∼15 mV. To activate the channels to the same level in 0 Ca²⁺ requires depolarization to ∼180 mV. Thus, the effect of an increase in Ca²⁺ from 0 to 70 μM on P_o is equivalent to a depolarization of 165 mV.

To determine whether channel activation by Ca²⁺ results from direct effects on the C-O equilibrium or on voltage sensor movement, the relationship between gating current and voltage was defined at both 0 and 70 μM Ca²⁺; the latter concentration should almost saturate all higher affinity Ca²⁺ binding sites. Because voltage-sensor movement is very rapid relative to channel opening, one can separate the gating current into two components, a fast component (Q_fast) resulting from voltage-sensor movement that occurs within the first 100 μs of a voltage-step and a slower component (Q_slow) with kinetics similar to channel opening. Thus, the specific effects of Ca²⁺ on voltage-sensor movement (Q_fast) can be distinguished from effects on channel opening (Q_slow). The results (and analysis) revealed an approximately −33 mV shift in the Q_fast-V relationship as Ca²⁺ was increased from 0 to 70 μM. This shift is much less than the ∼166 mV shift in the P_o-V relationship resulting from the same increase in [Ca²⁺]_i. In essence, voltage-sensor movement per se has only a small dependence on Ca²⁺, allowing Horrigan and Aldrich to conclude that the primary effect of Ca²⁺ binding is to directly modulate the C-O transition.

Another important contribution is the introduction of the term, R_o(Ca²⁺), the ratio of NP_o in the presence and absence of Ca²⁺ at extreme negative voltages, where the interpretation of the P_o-V relation is presumed to be simple (but see Andersen and Koeppe, 1992). At these potentials, P_o increases >1,000-fold in response to Ca²⁺ elevations, through a pathway that does not appear to involve voltage-sensor activation. The dependence of NP_o (where N is the number of channels in a patch) on Ca²⁺ at negative potentials therefore allows for a determination of the Ca²⁺ affinity and coupling constant between Ca²⁺ binding and channel activation more effectively than the more traditional measurements of the Ca²⁺-dependence of the voltage of half-maximal activation of conductance. The R_o-Ca²⁺ relation shows that R_o saturates at [Ca²⁺] above 100 μM, with a K_d ∼9 μM, similar to earlier estimates of the Ca²⁺ binding affinity of closed channels obtained from other means (Cox and Aldrich, 2000; Zhang et al., 2001). Most critically, the analysis shows that Ca²⁺ binding is allosterically linked to the C-O equilibrium with an approximately eightfold change in Ca²⁺ binding affinity with channel opening.

Finally, Horrigan and Aldrich extend an analysis used by Sigg and Bezanilla (1997) to estimate the voltage-sensor equilibrium for open channels (Q_o-V) relative to that for closed channels (Q_c-V). Specifically, as P_o → 0, the Q_o-V relationship can be determined solely from the voltage dependence of P_o. As expected for their allosteric model (Fig. 1), the Q_o-V relationship is similar in shape to the Q_c-V relationship, but shifted to more negative potentials. This provides an indication of the extent of interaction between the voltage sensor and the channel gate, revealing that channel opening directly increases the voltage-sensor equilibrium constant.

These results also have important implications in regards to the allosteric nature of voltage-dependent gating in other channels. In previous work, Horrigan and Aldrich showed that, in 0 Ca²⁺, voltage-sensor activation is not necessary for channel opening (Horrigan et al., 1999). The case for allosteric regulation of the C-O transition by voltage-sensor movement in voltage-gated channels now is strengthened further by the direct demonstration of the residual voltage-dependence of the C-O transition in the absence of voltage-sensor movement. Thus, voltage-sensor movement per se is not required in order for channel opening to occur, but it allosterically influences that transition.

The combination of astute analysis and measurements at extreme conditions has effectively isolated the interactions between each of the key processes involved in gating: channel opening, Ca²⁺ binding, and voltage-sensor movement. On balance, Ca²⁺ binding and voltage-sensor movement each regulate the C-O transition in a relatively independent fashion. However, this independence does not imply that Ca²⁺ and voltage are both similarly effective in regulating channel activation. Clearly, strong depolarization in the absence of Ca²⁺ drives channels to near maximal P_o. In contrast, although Ca²⁺ can increase P_o several orders of magnitude in the complete absence of voltage-sensor movement, the absolute magnitude of the limiting P_o value elicited by Ca²⁺ alone remains small. This can be understood most directly from comparison of the allosteric coupling constants of Fig. 1, in which D, the coupling constant for voltage-sensor movement to channel opening is 25, while C, the coupling constant for Ca²⁺ binding to channel opening is 8. Since each constant reflects the contribution of a single subunit, the ability of Ca²⁺ and voltage to influence channel opening reflects each factor raised to the fourth power, such that voltage-sensor movement can be considered ∼100-fold more effective at influencing the closed-open equilibrium than Ca²⁺ binding. Irrespective of this difference in the effectiveness of Ca²⁺ and voltage, the relatively independent fashion in which both Ca²⁺ and voltage regulate the C-O transition of BK channels now seems fundamentally established. Yet, this should not be taken to mean that we understand all aspects of the allosteric regulation of BK channels. At least two aspects of the results will require further consideration.

First, complexities in the Ca²⁺ dependence of rate constants for channel activation raise some question about the nature of the C-O conformational change. Horrigan and Aldrich approach this problem by using a two-barrier energy landscape in the C-O transition to explain their observations. This adequately reproduces their kinetic data, while also accounting for the energetic additivity of Ca²⁺ binding and voltage gating. However, the deduced multibarrier energy landscape in the C-O transition is functionally equivalent to a two-step process in the C-O transition, something hinted at in previous single channel observations (Rothberg and Magleby, 1998, 1999). The rapidity of movement through this energy landscape precludes straightforward examination of the fine structure of this transition, but specific physical movements in the channel protein must somehow be involved. Thus, given the independent coupling of both Ca²⁺ binding and voltage sensor movement to the C-O transition, clear definition of how Ca²⁺ and voltage influence the intermediate states in the C-O transition may be important in understanding the molecular motions underlying channel opening.

Second, another intriguing suggestion is that, based on the properties of R_o at [Ca²⁺] above 100 μM, regulation of BK channels by any lower affinity Ca²⁺ binding sites (Shi and Cui, 2001; Zhang et al., 2001) may involve direct coupling to voltage-sensor movement. The Horrigan and Aldrich work establishes procedures by which this proposal can be directly tested.

It is fitting that this paper arrives almost simultaneously with the emergence of work that is beginning to assess the molecular underpinnings of the machinery that underlies Ca²⁺ and voltage gating in BK channels. Horrigan and Aldrich have provided clear definition of the strength of coupling between ligand-binding, voltage-sensor movement, and channel opening information that will be critical as investigators attempt to unravel the physical linkages between each component of gating. By itself, however, the demonstration that either voltage or ligand regulates gating in an allosteric fashion does not identify the specific mechanical steps that underlie channel opening. Nevertheless, Horrigan and Aldrich almost certainly exclude certain classes of allosteric mechanisms. Thus, the simple fact that there appears to be little direct coupling between voltage-sensor movement and high affinity Ca²⁺ binding constrains the types of interactions that may occur between Ca²⁺ binding domains, voltage-sensors, and the gate. The key question now becomes, what are the physical mechanisms by which voltage sensor movement and Ca²⁺ binding both promote channel opening while exhibiting minimal interaction with each other?

Activation of BK channels by voltage presumably arises in a fashion similar to its Kv homologues (Bezanilla, 2000), initiated by the movement of charges in the S4 transmembrane segment (Diaz et al., 1998; Cui and Aldrich, 2000). However, despite the cloning of the Slo1 subunit ∼10 yr ago (Adelman et al., 1992; Butler et al., 1993), and evidence that one region of the cytosolic COOH-terminal tail might contribute to Ca²⁺-dependent regulation of the BK channel (Schreiber and Salkoff, 1997), progress on the sites and mechanisms by which Ca²⁺ binding regulates channel activation until recently has been slow. This situation is changing as this is written by a staggering amount of ongoing work that addresses the issue of Ca²⁺ regulation. For example, the cytosolic COOH terminus of the mammalian BK α subunit contains a sequence with homology to prokaryotic K⁺ channel sequences that encode a domain involved in regulation of K⁺ conductance (termed RCK domains) (Jiang et al., 2001). For some bacterial K⁺ channels, the RCK domain contains a consensus nucleotide binding sequence and RCK domains in other channels might provide regulatory binding sites for other signaling molecules. Now, the structure of the RCK domain of a Ca²⁺-regulated K⁺ channel (a two transmembrane channel from the archeon Methanobacterium thermoautotrophicum) provides an even more provocative view of how RCK domains may be important in BK channel function (Jiang et al., 2002). Specifically, for MthK, each subunit in the tetramer contributes one RCK domain to the structure, and another is assembled from solution, resulting in an octamer of RCK domains arranged around the axis of the pore. Startlingly, the BK channel α subunit sequence also was noted to contain two RCK domains in the COOH terminus, suggesting a similar octameric structure for BK channels. The residues in MthK thought to be involved in Ca²⁺ ligation, howeve r, are not conserved with residues in the BK RCK domain and are also quite distant from the residues that contribute to nucleotide binding in other RCK domains. Nevertheless, the presence of RCK domains in BK channel subunits suggests that the RCK domains are critical regulatory components of BK channels.

Stirring the pot even more, two recent studies show that the first RCK domain of the BK channel α subunit contains residues (different from those implicated in Ca²⁺ binding in MthK) that, when mutated together with mutation of the Ca²⁺ bowl, remove essentially all physiological regulation by Ca²⁺ (Bao et al., 2002; Xia et al., 2002). Furthermore, an additional single residue removes most of the ability of mM Ca²⁺ and Mg²⁺ to activate BK channels (Shi et al., 2002; Xia et al., 2002). These results strongly implicate the RCK structure as a key element in the ability of Ca²⁺ to regulate BK channel activation and raise the tantalizing possibility that the COOH terminus of each BK channel α subunit forms two discrete, linked (perhaps Ca²⁺ binding) RCK structures, that participate in regulating channel function. It is premature to assert where all the key Ca²⁺ binding sites in BK channels may be, but it is an intriguing possibility that multiple high affinity Ca²⁺ regulatory elements reside on each subunit. How does this relate to the work of Horrigan and Aldrich?

Although it may be necessary to consider explicitly two high affinity regulatory elements per BK channel α subunit, the key conclusion of the H/A work, that the allosteric effects of Ca²⁺ and voltage occur essentially independently, is unlikely to be affected. As such, the H/A work defines the essential framework for analyses that will guide future efforts to understand the domains involved in Ca²⁺ regulation. For example, in the case of MthK, the Ca²⁺ activation has been proposed to arise from a rotary movement of the RCK domains, which would result in mechanical forces being applied to the inner helix that contributes to the pore (Jiang et al., 2002). Movement of the S4 helix may similarly be coupled to conformational changes in the inner helix, although the steps in this coupling are unknown. A mechanism by which both the RCK domains and the S4-initiated mechanism influence the conformation of the inner helix could explain the independence between ligand binding and voltage-sensor movement. Furthermore, the H/A analysis might be interpreted to imply that the RCK domain and associated structures exhibit only minimal interaction with residues that influence voltage-sensor movement.

This recent flurry of progress on BK channels marks only a beginning and we can look forward to even more exciting and definitive results in the near future. A central question is: how do the voltage-sensing apparatus and the Ca²⁺-sensing apparatus exert their influences on the equilibrium between closed and open channel conformations? At present, this remains a poorly defined problem. However, insights into this important question are likely to have broad implications. For example, the specific mechanistic steps involved in coupling voltage-sensor movement to channel activation remain rather undefined for all voltage-dependent channels (Bezanilla, 2000). Analogous to the usefulness of BK channels in establishing the allosteric nature of voltage-gating, the dual regulation of the closed-open equilibrium in BK channels may allow new insights into the actual mechanism underlying the gating. Specifically, a key element of an allosteric mechanism is the reciprocal interactions between gate and sensor. In the case of BK channels, if Ca²⁺ or voltage affect channel opening, then channel opening will also affect Ca²⁺ binding affinity and voltage-sensor equilibrium. By taking advantage of such reciprocity, it should be possible to define more clearly the key physical linkages between voltage (or Ca²⁺ binding) and channel opening.