Termination of Ca²⁺ release during Ca²⁺ sparks in rat ventricular myocytes

Abstract

1. Confocal Ca²⁺ imaging was used to measure spontaneous release events (Ca²⁺ sparks) in fluo-3-loaded isolated rat ventricular myocytes.

2. The microscopic Ca²⁺ release flux underlying Ca²⁺ sparks was derived by adapting the methods used previously to describe macroscopic Ca²⁺ release from cell-averaged Ca²⁺ transients.

3. The magnitude of the local release fluxes varied from 2 to 5 μm ms⁻¹, depending on SR Ca²⁺ loading conditions. Following spontaneous activation, the release flux rapidly decayed (τ = 6–12 ms). The rate of termination of release flux was found to be directly related to the magnitude of the flux (r² = 0.88).

4. The rate of termination of local release flux was slowed in the presence of FK506, a compound that is known to reduce inactivation of SR Ca²⁺ channels in vitro.

5. These results suggest that termination of release flux during sparks is not due to a spontaneous stochastic decay process or local depletion of Ca²⁺ from the SR, but rather involves an active extinguishing mechanism such as Ca²⁺-dependent inactivation or adaptation.

In mammalian cardiac myocytes, the process of excitation-contraction (E-C) coupling is mediated by Ca²⁺ influx from the extracellular medium triggering Ca²⁺-induced Ca²⁺ release (CICR) from the sarcoplasmic reticulum (SR) (Bers, 1991; Stern & Lakatta, 1992). In its simplest form, CICR should involve a positive feedback of released Ca²⁺ on further release, which should result in all-or-none regenerative release. In vivo, however, the Ca²⁺ release is tightly graded with the magnitude of the Ca²⁺ influx (Cannell, Berlin & Lederer, 1987; Beuckelmann & Wier, 1988). One suggested solution to this paradox is the existence of local subdomains in which the trigger and released Ca²⁺ are morphologically and/or functionally segregated (Stern, 1992; Györke & Palade, 1993). Recently, the idea of local control of release has received direct experimental support from the discovery of Ca²⁺ sparks (Cheng, Lederer & Cannell, 1993; Lipp & Niggli, 1994). A Ca²⁺ spark is a spontaneous local increase in [Ca²⁺] (Cheng et al. 1993) that appears to reflect activation of a regenerative cluster of Ca²⁺ release channels (Lipp & Niggli, 1996). A spark is well confined in space (< 3 μm) and time (< 50 ms) and reaches a peak amplitude of 100–500 nm, depending on SR Ca²⁺ loading conditions (Cheng et al. 1993). Thus, to understand the graded nature of CICR we have to define the factors that are responsible for the early termination of Ca²⁺ release during a spark and factors that prevent Ca²⁺ released during these events from activating adjacent release sites.

In principle, termination of Ca²⁺ release during a spark could be due to any of the following mechanisms: (1) Ca²⁺ release dying off spontaneously (stochastic attrition; Stern, 1992); (2) local depletion of Ca²⁺ in the SR; and (3) Ca²⁺-dependent inactivation/adaptation of release (Fabiato, 1985; Yasui, Palade & Györke, 1994). To address the question of which of these mechanisms is responsible for termination of release during sparks, we have derived the Ca²⁺ release flux underlying sparks and correlated the rate of termination of the release flux with the magnitude of the flux under various SR Ca²⁺ loading conditions. Our results show that the rate of decay of flux is directly dependent on the amount of Ca²⁺ released during the spark, and it can be modulated by agents that affect Ca²⁺ release channel closure. Based on these results, we conclude that decay of release during a spark is caused by inactivation or adaptation of Ca²⁺ release channels.

METHODS

Cell isolation and experimental solutions

Adult Sprague-Dawley rats (200–300 g) were killed by lethal injection of Nembutal (100 mg kg⁻¹, i.p.; Abbott Laborotories, Chicago, IL, USA), and single ventricular myocytes were obtained by enzymatic dissociation as described previously (Györke, Lukyanenko & Györke, 1997). The cells were loaded with fluo-3 by a 20 min incubation with 5 μm fluo-3 AM (acetoxymethyl ester form; Molecular Probes) at room temperature.

The standard Tyrode solution contained (mm): 140 NaCl, 2 KCl, 0.5 MgCl₂, 1 CaCl₂, 10 Hepes, 0.25 NaH₂PO₄, 5.6 glucose, pH 7.3. Tetrodotoxin (Sigma), at 10 μm, was added to the bathing solution to avoid depolarization-induced Ca²⁺ release due to spontaneous action potentials. All experiments began in a bathing solution containing 1 mm Ca²⁺. Only cells that exhibited no spontaneous Ca²⁺ oscillations were selected for further measurements. To alter the SR Ca²⁺ load, extracellular [Ca²⁺] ([Ca²⁺]_o) was varied between 1 and 10 mm. In the Na⁺-free solution, Na⁺ was replaced by Li⁺ and Ca²⁺ was omitted. FK506 (generous gift from Fujisawa, Deerfield, IL, USA) was added from a 10 mm stock solution in methanol at concentrations of 10–50 μm.

Confocal microscope

Experiments were performed using the linescan mode of an Olympus Laser Scanning Confocal Microscope (LSM-GB200) as described previously (Györke et al. 1997). Fluo-3 was excited by light at 488 nm (25 mW argon laser; intensity attenuated to 1–3%). Fluo-3 fluorescence was measured at wavelengths of > 515 nm. Images were acquired at a rate of 2.1 ms per scan with the confocal detector aperture set to 75% of maximum. An analog recording of fluorescence intensity was digitized into 640 pixels, giving a nominal pixel dimension of 0.41 μm. Image processing and analysis were performed by using NIH Image (NIH, Bethesda, MD, USA) and IDL software (Research Systems Inc., Boulder, CO, USA). The local, instantaneous concentration of the calcium-dye complex was derived from fluo-3 fluorescence according to the equation:

(1)

where F(iΔr, jΔt) is the fluorescence of pixel (i, j), Δr and Δt are the spatial and temporal resolution of the microscope. The spatial co-ordinate r is the distance along the line scan. [Dye]_T is the total dye concentration. F_min is essentially zero for fluo-3. F_max was obtained from the resting level of fluorescence (F_rest) according to the equation (Cheng et al. 1993):

(2)

where K_D (740 nm; Escobar, Cifuentes & Vergara, 1995) is the dissociation constant of the indicator-dye complex, and [Ca²⁺]_rest is the resting value of calcium concentration (assumed to be 0.1 μm at 1 mm [Ca²⁺]_o). The total fluo-3 concentration, ([dye]_T ≈ 50 μm) was estimated fluorometrically by releasing the dye trapped in the cytosol with digitonin (50 μm) and extrapolation to an in vitro calibration curve. To identify Ca²⁺ sparks we used the following criteria (Györke et al. 1997): (a) the amplitude of the event had to be at least two times greater then the standard deviation of fluorescence intensity fluctuations measured in the neighbouring region (area ≈ 3 × 15 pixels); (b) the duration and image width of the Ca²⁺ signal (both measured at half-maximal amplitude) had to be within 10–100 ms and 0.5-3 μm, respectively. Averaged Ca²⁺ sparks for given experimental conditions were obtained by averaging linescan images of individual sparks synchronized at the time to half-maximal amplitude.

Calculation of the local release flux

The spatio-temporal distribution of the free cytoplasmic Ca²⁺ is described by the reaction-diffusion equation:

(3)

Here ∂Ca/∂t is the rate of change of cytosolic free Ca²⁺, D_Ca is the diffusion coefficient of Ca²⁺, and ▿²Ca is the Laplacian, q_rel is the release flux, q_rem is the removal flux and q_dye is the net rate of binding of calcium ion to the fluorescent indicator. For the binding of calcium to the dye complex, the kinetic expression is given by:

(4)

In this expression, k_d,on and k_d,off are the on- and off-rate constants, respectively, for binding to the dye. The instantaneous concentration of the free dye may be determined from the following conservation relation:

(5)

The spatio-temporal distribution of the calcium-dye complex is also given by the reaction-diffusion equation:

(6)

Within the myoplasm, spherically symmetrical diffusion of calcium and of the calcium-dye complex is assumed.

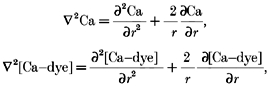(7)

Substituting eqns (4), (5), (6) and (7) into eqn (3) and solving for the release flux yields the following expression:

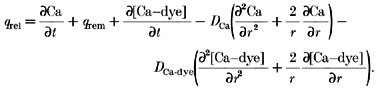(8)

Thus, the diffusion of both the free ion and complex are included. All the terms on the right-hand side can be measured experimentally, or computed from accepted kinetic expressions. The instantaneous local concentration of the fluo-3-calcium complex is obtained by measuring fluorescence intensity. The instantaneous local concentration of free Ca²⁺ is obtained by solving for it from eqn (6):

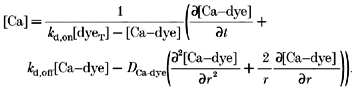(9)

The removal flux consists of three contributions (Sipido & Wier, 1991).

(10)

The quantity q_b is the removal by binding to soluble buffers, q_SR is the removal by the Ca²⁺-ATPase of the sarcoplasmic reticulum, and q_Sl is the removal by the Ca²⁺-ATPase and Na⁺-Ca²⁺ exchanger of the sarcolemma (SL). The fluxes transported by the Ca²⁺ pumps and Na²⁺-Ca²⁺ exchanger were calculated from expressions given by Tang & Othmer (1994). These are given below:

(11)

(12)

V is the maximal capacity, and K is the calcium capacity at which the transport is half-maximal for a particular process. With regard to buffering, its flux was calculated from the following equation, which lumps all the buffers into a single pool:

(13)

The rate constants for binding and dissociation are given by k_b,on and k_b,off, respectively. [B]_o is the total concentration of buffers, and [Ca-B] is the concentration of the calcium-buffer complexes. The latter is, itself, a function of time and is calculated from integration using a forward finite difference approximation.

(14)

Thus, all the quantities on the right side of eqn (8) were calculated from the experimental records. The values of the various parameters used in the calculations are given in Table 1. The equations were numerically discretized using finite differences. The time derivative was approximated by backward differences, while the first and second order spatial derivatives were approximated by central finite differences. The grid density corresponded to the resolution of our confocal microscope (Δt = 2.1 ms, Δr = 0.4 μm). The resulting set of discrete equations were coded in a three-dimensional spreadsheet in Microsoft Excel for numerical integration.

Table 1.

Parameters used to compute local SR Ca²⁺ fluxes associated with sparks

Parameter	Value	Source
DyeT (nm)	5 × 104	Experimental value
KD (nm)	740	Escobar, Cifuentes & Vergara (1995)
kd,on (nm−1 ms−1)	2.4 × 10−4	Escobar, Cifuentes & Vergara (1995)
kd,off (ms−1)	1.8 × 10−2	Escobar, Cifuentes & Vergara (1995)
VSR (nm ms−1)	2 × 103	Tang & Othmer (1994)
KSR (nm)	120	Tang & Othmer (1994)
VSL (nm ms−1)	19	Tang & Othmer (1994)
KSL (nm)	60	Tang & Othmer (1994)
B0 (nm)	2.15 × 105	Hove-Madsen & Bers (1993)
kb,on (nm−1 ms−1)	10−4	Sipido & Wier (1991)
kb,off (ms−1)	4 × 10−2	Sipido & Wier (1991)
DCa-dye (μm2 ms−1)	0.02	Harkins, Kurebayashi & Baylor (1993)
DCa (μm2 ms−1)	0.3	Kushmeric & Podolsky (1969)
Δt (ms)	2.1	Temporal resolution of confocal microscope
Δr (μm)	0.4	Spatial resolution of confocal microscope

Filtering

Because of unavoidable random noise in experimental records the raw pixel intensities and the calculated free calcium from eqn (9) were filtered in the course of the calculations. The filtering algorithm averaged the raw value at a point with its eight adjacent neighbours. That is:

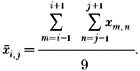(15)

This allowed clear visualization of the peaks in release flux and other relevant quantities.

Model validation

To verify the accuracy of our algorithm for reconstruction of release fluxes, we compared our numerical approach to analytical expressions. First we confirmed that the time and space derivatives were accurately estimated using the grid density of our numerical discretization scheme (Δr = 0.4 μm; Δt = 2.1 ms). We compared the analytical derivatives of the solution presented by Stern (1992) (eqn (13)) for diffusion of calcium from a point source to our numerical estimates. The estimated time and spatial derivatives superimposed upon their analytical counterparts (not shown).

Next, we applied our analysis to several simulated calcium distributions arising from known release fluxes. A simple calcium distribution, C(t, r), with an exponentially decaying release flux is described in a sphere of radius a by the following system.

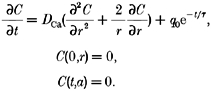(16)

The analytical solution to this system is given by Carslaw & Jaeger (1959). With the analytical solution contaminated with 10% noise, our analysis reconstructed an input time constant of 12 ms to within 5%, and an input time constant of 6 ms to within 20% (Fig. 1).

Figure 1. Reconstruction of Ca²⁺ release flux from simulated Ca²⁺ distributions arising from exponentially decaying release fluxes.

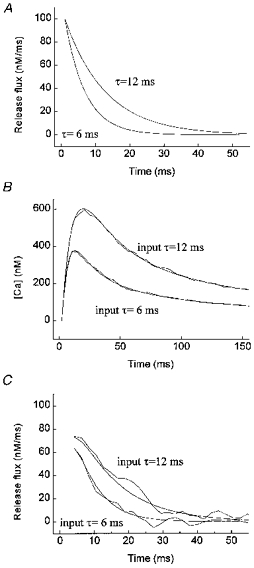
A, time courses of input release fluxes with time constants of 6 and 12 ms. B, calcium time courses resulting from input release fluxes in A, obtained by solving analytically eqn (16) (smooth lines, without noise; wavy lines, with noise and filtering). The grid density of the analytical solution was equal to the temporal and spatial resolution of the confocal microscope (Δt = 2.1 ms, Δr = 0.4 μm). Random noise equal to 10% of the local magnitude of the [Ca²⁺] was generated by adding a normally distributed random number to the [Ca²⁺] variable: C_noise(t,r) = C(t,r)(1 + 0.05n), where n is a random number between −1 and +1; the [Ca²⁺] values were then filtered using our filtering algorithm (eqn (15)). C, time courses of recovered release fluxes without noise and with noise and filtering. The release flux was calculated by solving the numerically discretized eqn (16) for q_rel, i.e. The reconstructed values of the release flux at r = a were fitted to the exponential decay function given in eqn (16). The following values for the parameters q₀ and τ were obtained for the reconstructed release fluxes with noise and filtering. Input τ (ms): 6 and 12 ms; reconstructed τ, (ms): 6.09 (r² = 0.873) and 12.58 (r² = 0.891); input q₀ (nm ms⁻¹): 100 and 100; reconstructed q₀ (nm ms⁻¹): 62 and 75.

Lastly, we reconstructed a known Ca²⁺ release flux function giving rise to a simulated Ca²⁺ spark. The theoretical linescan image of a Ca²⁺ spark was kindly provided by Dr Michael Stern (NIH, Baltimore, MD, USA). This simulation included kinetics and diffusion of the dye complex, buffering, and re-uptake, but employed a different model from the inverted model employed in our analysis. Using our analysis program we were able to recover reasonably well the wave form of the input release signal (not shown). In view of the accuracy of the reconstructions, in the presence of noise and using a model different from the inverted analysis model, the algorithm was judged sufficiently accurate and robust for estimating release fluxes from experimental records.

RESULTS

Local release flux underlying Ca²⁺ sparks

To understand the behaviour of channels underlying sparks it was important to determine the Ca²⁺ release flux associated with the sparks. SR Ca²⁺ release flux was determined by applying the general approach used for calculating release flux from cell averaged Ca²⁺ transients in skeletal and cardiac muscle (Melzer, Rios & Schneider, 1987; Sipido & Wier, 1991). According to the basic equation of Melzer and co-authors:

(17)

where q_rel is the Ca²⁺ release flux; q_rem is the Ca²⁺ removal flux due to binding to soluble buffers, extrusion by sarcolemmal pumps, and re-uptake by the sarcoplasmic reticulum; and d[Ca-dye]/dt is the net rate of binding of Ca²⁺ to the fluorescent indicator. This formulation assumes spatially uniform concentrations of Ca²⁺ and buffers. To account for spatially heterogeneous release underlying Ca²⁺ sparks, eqn (17) was modified to include diffusion terms for Ca²⁺ and the Ca-dye complex.

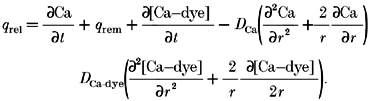(8)

Following Sipido & Wier (1991), Ca²⁺ removal flux was estimated based on the measured properties of the known cellular Ca²⁺ buffering, uptake and extrusion systems (Table. 1).

Examples of removal and release fluxes derived from a local fluorescence signal are shown in Fig. 2. The removal flux includes binding of Ca²⁺ to intracellular Ca²⁺ buffers and Ca²⁺ removal by the SR and sarcolemmal Ca²⁺-ATPase. These components contributed about 55, 44 and 1%, respectively, to the peak of the total removal flux (not shown). Local [Ca²⁺]_i changes determined by taking into consideration the kinetics of the Ca²⁺ indicator and the diffusion of Ca²⁺ and the Ca-dye complex are also presented. It can be seen that a local [Ca²⁺]_i elevation of ∼100 nm is produced by a release flux of ∼2000 nm ms⁻¹. The local release flux is clearly more confined in both space and time than the resulting Ca²⁺ spark.

Figure 2. Calculation of Ca²⁺ release flux underlying Ca²⁺ release events.

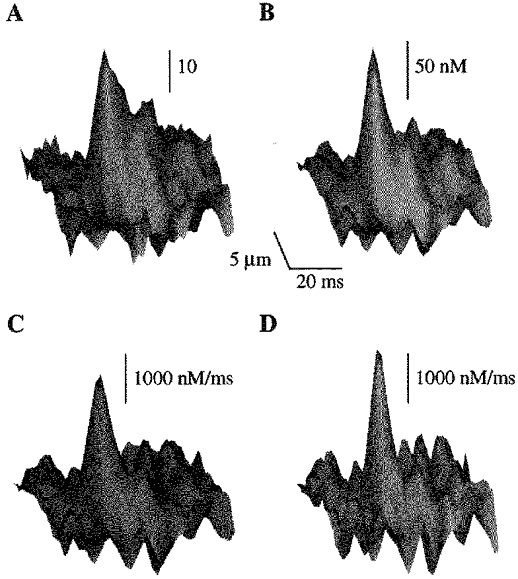
Surface plots of images of spontaneous Ca²⁺ sparks represented in arbitrary fluorescence units (A) and [Ca²⁺] (B) and their associated Ca²⁺ removal (C) and release fluxes (D). The images were obtained from a single release event measured in an extracellular solution containing 1 mm Ca²⁺. Background [Ca²⁺]_i was assumed to be 100 nm.

A potential source of error in our estimation of release fluxes is that spatially heterogeneous Na⁺-Ca²⁺ exchanger flux was homogeneously lumped with the SL Ca²⁺-ATPase flux. To assess the extent to which the calculated Ca²⁺ release flux signal is affected by removal of Ca²⁺ by the Na⁺-Ca²⁺ exchanger we carried out experiments in zero-Na⁺ medium. We found no significant differences in the magnitude and rate of termination of release fluxes measured under control vs. zero-Na⁺ conditions (peak amplitude, 2632 ± 163 nm ms⁻¹ vs. 2628 ± 161 nm ms⁻¹ and τ = 9.5 ± 0.9 ms vs. 10.4 ± 1.3 ms; means ± s.e.m.; n = 30 and 19, respectively). Thus, our estimates of Ca²⁺ release fluxes were not affected significantly by a heterogeneous Na²⁺-Ca²⁺ exchanger.

In Fig. 2, it can be seen that the local release flux rapidly increases then it decays just as rapidly. Defining the mechanism of this decay was the main goal of the remainder of this study.

Dependency of the rate of decay on the magnitude of release

To understand the mechanism of termination of Ca²⁺ release underlying a spark it is important to determine the relationship between the rate of termination of the release flux and the amount of Ca²⁺ released. Release flux magnitude was varied by means of exposing the cells to different [Ca²⁺]_o. Elevation of [Ca²⁺]_o has been shown to increase the levels of SR Ca²⁺ content in cardiac myocytes. It has also been reported to increase the magnitude of Ca²⁺ sparks, presumably due to a greater Ca²⁺ gradient across the SR membrane and an increased duration of openings of ryanodine receptors (RyRs) at higher lumenal [Ca²⁺] (Lukyanenko, Györke & Györke, 1997).

Figure 3 illustrates images of Ca²⁺ sparks, and associated with them, Ca²⁺ release fluxes measured at low and high SR Ca²⁺ loading conditions (i.e. 1 and 5 mm [Ca²⁺]_o). To quantify the effects of changing the SR Ca²⁺ load on the magnitude and duration of [Ca²⁺] and release flux signals, we measured the peak amplitude and time constant of decay of the signals (Fig. 3C). Figure 4A and Table 2 summarize these measurements. It can be seen that the magnitude of the release fluxes gradually increased as [Ca²⁺]_o was raised from 1 to 5 mm. Raising [Ca²⁺]_o to 10 mm did not cause a further increase in the magnitude of the Ca²⁺ release flux. These results could be explained by a gradual increase in the SR Ca²⁺ content until a maximal load is reached at 5 mm. The [Ca²⁺]_o dependence of the release flux decay is a mirror image of the [Ca²⁺]_o dependence of the release flux magnitude: the time constant of decay first decreases and then levels off at 5 mm [Ca²⁺]_o. These results suggest that there may be a correlation between the magnitude of Ca²⁺ release flux and its decay. Figure 4B shows a linear relationship between the peak amplitude and time constant of decay of the local Ca²⁺ release flux signals. It can be seen that these two parameters are, indeed, highly correlated. To determine to what extent these results are influenced by selection of parameter values of the model, we examined the effects of variations of parameter values on the magnitude and rate of decay of the release flux. Changing values of resting [Ca²⁺]_i (from 75 to 150 nm), [dye]_T (from 20 to 100 μm), fluo-3 Ca²⁺ affinity (from 400 to 1000 nm), maximal SR Ca²⁺ pump rate (from 500 to 3000 nm ms⁻¹) or cellular buffer concentration (from 150 to 300 μm) resulted in up to 2-fold changes in the absolute values of release flux amplitude, and decay rate. However, the relative increases in amplitude and rate of decay of flux upon elevation of SR Ca²⁺ load remained constant at all parameter values. Thus, the observed increase in the rate of Ca²⁺ flux decay at higher flux amplitudes is not affected by selection of parameter values. These results suggest that Ca²⁺ release during sparks is controlled by a negative control mechanism such as Ca²⁺-dependent inactivation or adaptation. Alternatively, Ca²⁺ termination of release fluxes could be attributed to depletion of Ca²⁺ from the SR

Figure 3. The effect of increasing SR Ca²⁺ load on Ca²⁺ sparks and the underlying Ca²⁺ release fluxes.

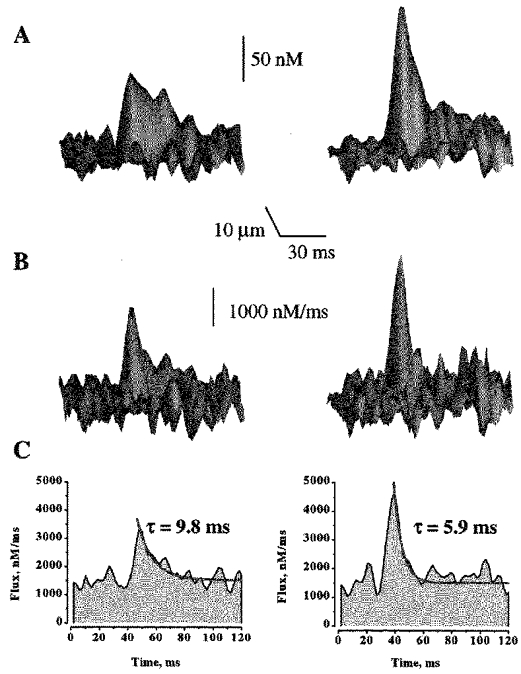
A and B, representative surface plots of Ca²⁺ sparks and associated Ca²⁺ release fluxes measured in the presence of 1 and 5 mm Ca²⁺ in the extracellular solution. The signals were obtained from single release events measured before and after raising [Ca²⁺]_o from 1 to 5 mm in the same cell. C, line plots of Ca²⁺ release fluxes through the peaks of the signals shown in B. The release flux decays were best fitted by single exponentials functions (red lines). Background [Ca²⁺]_i at 1 mm [Ca²⁺]_i was assumed to be 100 nm; background [Ca²⁺]_i at 5 mm [Ca²⁺]_o was extrapolated to be 124 nm.

Figure 4. The effect of changing SR Ca²⁺ load on the magnitude and time constant of decay of local release fluxes.

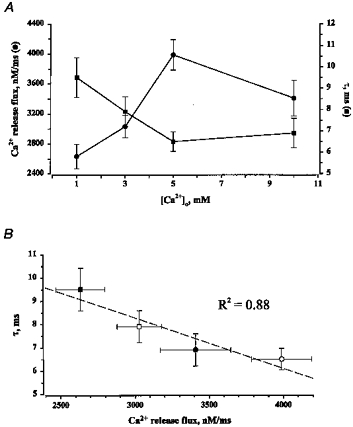
A, dependency of magnitude (•) and time of decay of local release fluxes (▪) on [Ca²⁺] in the extracellular solution. B, linear relationship between the peak amplitude of the release flux and time constant of its decay (r² = 0.88) at various [Ca²⁺] (▪, 1 mm; □, 3 mm; ○, 5 mm; •, 10 mm). The values are means (±s.e.m.) obtained from 15–30 individual sparks.

Table 2.

The effects of [Ca²⁺]_o and FK506 on the amplitude and decay of Ca²⁺ sparks and associated Ca²⁺ release fluxes

	[Ca2+]o (mm)	1	3	5	10	5
	FK506 (μm)	0	0	0	0	50
[Ca2+] signal	Amplitude (nm)	102 ± 5	126 ± 8	174 ± 12 *	154 ± 13 *	167 ± 12
	Decay time constant (ms)	16.6 ± 3.6	14.7 ± 1.4	10.8 ± 1.0 *	13.6 ± 1.2	29.5 ± 2.9 †
Release flux	Amplitude (nm ms−1)	2632 ± 163	3029 ± 150	3887 ± 202	3406 ± 236 *	3519 ± 260
	Decay time constant (ms)	9.5 ± 0.9	7.9 ± 0.7	6.5 ± 0.5 *	6.9 ± 0.7	11.2 ± 1.0 †

Data represented as means ± s.e.m. of measurements from 14–29 individual sparks. The amplitudes of the local [Ca²⁺]_i and relase flux signals were determined as differences between the background and peak values. Decay time constants were determined by fitting single exponential functions to the initial descending face of linear profiles through the peaks of the signals.

^*P < 0.05 vs. values at 1 mm [Ca²⁺]_o

^†P < 0.05 vs. values at 5 mm [Ca²⁺]_o and no FK506.

Effect of FK506

To evaluate the possibility that decay of Ca²⁺ release during sparks is caused by local depletion of SR Ca²⁺ we tested the effects of FK506 on release fluxes underlying sparks. FK506 has been shown to reduce the rate of closure of single RyRs in lipid bilayers and increase the duration of sparks in cardiac myocytes (Xiao, Valdivia, Bogdanov, Valdivia, Lakatta & Cheng, 1997). Examples of linescan images of Ca²⁺ sparks measured before and after addition of 50 μm FK506 are shown in Fig. 4A. It can be seen that the duration of sparks is increased dramatically.

The effects of FK506 on the spatio-temporal properties of sparks and associated release fluxes are illustrated in Fig. 5 and summarized in Table 2. It can be seen that the magnitudes of these signals are comparable to those under control conditions. At the same time, the duration of the [Ca²⁺] and release flux signals increased 2.6- and 1.7-fold, respectively. These experiments clearly show that normally not all the Ca²⁺ is released from the SR, and thus SR Ca²⁺ depletion is not the primary factor that determines termination of release during sparks.

Figure 5. The effect of FK506 on Ca²⁺ sparks and associated release fluxes.

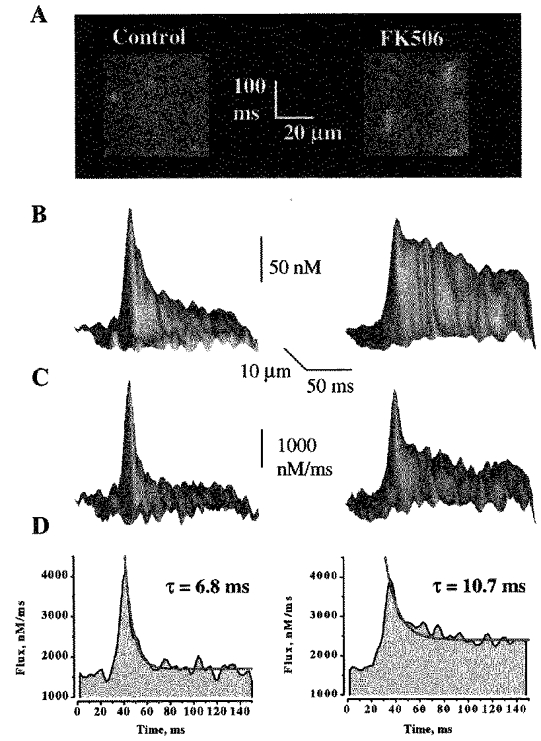
A, linescan images of Ca²⁺ sparks before and after administration to the bathing solution of 50 μm FK506. The horizontal and vertical calibration bars represent 20 μm and 100 ms, respectively. B and C, surface plots of Ca²⁺ sparks and underlying release fluxes measured in the presence and the absence of FK506. The signals were obtained by averaging 8 individual release events before and after administration of 50 μm FK506. D, time-dependent line plots of Ca²⁺ release fluxes through the peaks of the signals shown in C. The decays of the fluxes were best fitted by single exponential functions (red lines).

Interestingly, despite the significant increase in duration of the release flux, most sparks still remained spatially localized (Fig. 5A). Only in a few instances did prolonged sparks become initiation sites for propagating Ca²⁺ waves (not shown). These results suggest that rapid termination is not essential for spatial confinement of release.

DISCUSSION

Here we report on the first systematic attempt to investigate the mechanism responsible for termination of Ca²⁺ release during sparks in cardiac myocytes. Our principal finding is that the rate of decay of release flux underlying sparks is directly related to the amount of Ca²⁺ released and can be modulated pharmacologically by FK506, a compound that has been shown to alter inactivation properties of the Ca²⁺ release channel. These results suggest that termination of Ca²⁺ release during the local release events involves a negative control mechanism at the level of Ca²⁺ release channel gating.

The local Ca²⁺ release flux during Ca²⁺ sparks was derived by adapting the methods used to describe macroscopic Ca²⁺ release from cell-averaged Ca²⁺ transients in skeletal (Melzer et al. 1987) and cardiac muscle (Sipido & Wier, 1991). The main modification made to these previous methods was that in order to account for the spatial heterogeneity of the local Ca²⁺ release fluxes, we considered the diffusion of Ca²⁺ and Ca-dye complex. A similar approach was recently used to derive SR Ca²⁺ release fluxes associated with Ca²⁺ sparks in cat atrial myocytes (Blatter, Hüser & Rios, 1997). The obtained values of peak release fluxes (2–5 mm s⁻¹) are comparable to those reported for cell-averaged Ca²⁺ transients in guinea-pig ventricular myocytes (2.7-9.5 mm s⁻¹; Sipido & Wier, 1991) and for Ca²⁺ sparks in cat atrial cells (∼10 mm s⁻¹; Blatter et al. 1997).

Ultrastructural evidence suggests that about twenty-two release channels are present in a typical SR junction cleft (based on an estimated average area of 0.02 μm² for the junctional SR and a foot spacing of 30 nm; Protasi, Sun & Franzini-Armstrong, 1996). According to computations by Langer & Peskoff (1995), a Ca²⁺ influx of 9 mm s⁻¹ (comparable to 5 mm s⁻¹ found in this study) into the narrow diadic cleft increases [Ca²⁺] to 600 μm (cleft centre) −100 μm (cleft periphery). At these Ca²⁺ concentrations, all or most of the Ca²⁺ channels in the cleft should be activated. Activation of the cluster probably begins from a spontaneous opening of a single Ca²⁺ release channel, which activates a chain reaction of CICR involving the rest of the channels in the cleft (‘cluster bomb’ scenario; Stern, 1992).

After initial activation, the Ca²⁺ release flux rapidly decayed. Defining the mechanism of this decay constituted the main goal of the present study. As mentioned in the Introduction, there are three types of mechanism that could account for the termination of release flux: (1) stochastic attrition (Stern, 1992); (2) local depletion of Ca²⁺ from the SR; and, (3) an active extinguishing process involving alterations in channel gating. Stern (1992) has shown that stochastic attrition is very sensitive to the number of channels and to the amount of the positive feedback gain that sustains CICR in the regenerative cluster. Thus, greater SR Ca²⁺ fluxes would be expected to result in slower flux decays. In contrast to this expectation, our results clearly showed that termination of release flux is faster for large Ca²⁺ release fluxes than for small release fluxes. Therefore, our results are inconsistent with release termination being a result of a stochastic decay mechanism. The possibility that termination of release during sparks is primarily due to local depletion of Ca²⁺ in the SR is inconsistent with the results of our experiments with the RyR gating modulator, FK506. This compound significantly slowed down the rate of decay of the release flux, indicating that under control conditions not all available Ca²⁺ is released from the SR. However, we cannot rule out partial depletion as a contributing factor to the decay of the local release flux. Taken together, our results suggest that decay of release during a spark involves an extinguishing mechanism at the level of Ca²⁺ release channel gating. This mechanism could be Ca²⁺-dependent inactivation (Fabiato, 1985) or adaptation (Györke & Fill, 1993; Yasui et al. 1994). It also could involve the reported ability of the Ca²⁺ release channel to respond to changes in lumenal [Ca²⁺] (Sitsapeasan & Williams, 1994; Lukyanenko et al. 1997). Based on the present results, we cannot distinguish among these possibilities.

The increase in duration of sparks and associated release fluxes in the presence of FK506 is in agreement with the results of Xiao, Lakatta & Cheng (1996) regarding the effects of FK506 on Ca²⁺ sparks in rat ventricular myocytes, and are also consistent with the ability of the drug to reduce adaptive inactivation by RyRs in isolated vesicle preparations (Györke, Dettbarn & Palade, 1994) and in lipid bilayers (Xiao et al. 1997). These results support the possibility that termination of release flux during sparks involves adaptive inactivation of the release channels. McCall and co-workers (McCall, Satoh, Shannon, Blatter & Bers, 1996) found no significant effect of FK506 on duration of sparks, using of 10 μm of the drug. In our experiments, we routinely used 50 μm of FK506; however, in several preliminary experiments, 10 μm FK506 had a similar potentiating effect on spark duration. At the present time, we do not know the reason for these differences in results.

It has been suggested that Ca²⁺-dependent inactivation serves as a negative feedback control mechanism to prevent CICR from self-regeneration. Our experiments with FK506 showed that, despite the significant increase in time of decay of local release fluxes, most sparks remained localized and did not trigger release from adjacent release sites. Thus, it appears that the most important factor for preventing the spread of CICR is not inactivation, but spatial segregation of release sites, as suggested by Stern (1992). The role of release inactivation in suppressing regenerative release may become increasingly important under conditions of Ca²⁺ overload, when the tendency for generating propagating waves is augmented. This possibility is consistent with our preliminary results showing that, in Ca²⁺ overloaded myocytes, FK506 substantially increased the frequency of propagating and abortive Ca²⁺ waves. In addition, inactivation of release could play a role in preventing excessive Ca²⁺ loss from the SR during Ca²⁺ release and allowing the stores to be rapidly reloaded following release.