The influence of caffeine on intramembrane charge movements in intact frog striated muscle

Abstract

1. The influence of caffeine, applied over a 25-fold range of concentrations, on intramembrane charge movements was examined in intact voltage-clamped amphibian muscle fibres studied in the hypertonic gluconate-containing solutions that were hitherto reported to emphasize the features of q_γ at the expense of those of q_β charge.

2. The total charge, Q_max, the transition voltage, V*, and the steepness factor, k, of the steady-state charge-voltage relationships, Q(V), were all conserved to values expected with significant contributions from the steeply voltage-dependent q_γ species (Q_max ≈ 20 nC μF⁻¹, V* ≈ −50 mV, k ≈ 8 mV) through all the applications of caffeine concentrations between 0.2 and 5.0 mm. This differs from recent reports from studies in cut as opposed to intact fibres.

3. The delayed transients that have been attributed to transitions within the q_γ charge persisted at low (0.2 mm) and intermediate (1.0 mm) caffeine concentrations.

4. In contrast, the time courses of such q_γ currents became more rapid and their waveforms consequently merged with the earlier q_β decays at higher (5.0 mm) reagent concentrations. The charging records became single monotonic decays from which individual contributions could not be distinguished. This suggests that caffeine modified the kinetic properties of the q_γ system but preserved its steady-state properties. These findings thus differ from earlier reports that high caffeine concentrations enhanced the prominence of delayed transient components in cut fibres.

5. Caffeine (5.0 mm) and ryanodine (0.1 mm) exerted antagonistic actions upon q_γ charge movements. The addition of caffeine restored the delayed time courses that were lost in ryanodine-containing solutions, reversed the shift these produced in the steady-state charge-voltage relationship but preserved both the maximum charge, Q_max, and the steepness, k, of the steady-state Q(V) relationships.

6. Caffeine also antagonized the actions of tetracaine on the total available q_γ charge, but did so only at the low and not at the high applied concentrations. Thus, 0.2 mm caffeine restored the steady-state q_γ charge, the steepness of the overall Q(V) function and the appearance of delayed q_γ charge movements that had been previously abolished by the addition of 2.0 mm tetracaine.

7. In contrast, the higher applied (1.0 and 5.0 mm) caffeine concentrations paradoxically did not modify these actions of tetracaine. The total charge and voltage dependence of the Q(V) curves, and the amplitude and time course of charge movements remained at the reduced values expected for the tetracaine-resistant q_β charge.

8. These results permit a scheme in which caffeine acts directly upon ryanodine receptor (RyR)-Ca²⁺ release channels whose consequent activation then dissociates them from the tubular dihydropyridine receptor (DHPR) voltage sensors that produce q_γ charge movement, with which they normally are coupled in reciprocal allosteric contact.

There is now extensive pharmacological and kinetic evidence for the existence of at least two distinct species of intramembrane charge in striated muscle that is subject to depolarizing voltage clamp steps (Adrian & Peres, 1979; Huang, 1982; Vergara & Caputo, 1982; Hui, 1983). Of these, the striking characteristics of the q_γ charge strongly suggest an association with the excitation-contraction coupling process. This charge species is selectively distributed in the transverse tubular as opposed to the surface membranes (Huang & Peachey, 1989) and is specifically sensitive to both local anaesthetics and L-type Ca²⁺ channel blocking agents that are known to inhibit contractile activation (Huang, 1982, 1990; Chen & Hui, 1991). Its steep voltage sensitivity closely parallels the potential dependence of the release of intracellularly stored Ca²⁺ (Hui & Chandler, 1990). Such findings prompted suggestions that q_γ transients represent configurational changes in dihydropyridine receptor (DHPR) voltage sensors in their regulation of the Ca²⁺ release that activates skeletal muscle (Huang & Peachey, 1989; Huang, 1990).

However, the q_γ current shows complex kinetics that often include a delayed ‘hump’-shaped ‘on’ current at some test voltages which is nevertheless followed by monotonically decaying ‘off’ waveforms (Adrian & Peres, 1979; Huang, 1982). In contrast, uncoupled relaxations of intramembrane particles should exhibit relatively simple monotonic decays, as has been demonstrated for currents attributed to the remaining q_β charge. These observations led to suggestions that delayed q_γ currents result from Ca²⁺ release rather than voltage change (Csernoch et al. 1991). Alternative hypotheses proposed simple co-operative systems that contained varying energy barriers (Huang, 1984) or models with multiple states linked through appropriately adjusted and varying rate constants (Rios et al. 1993; Jong et al. 1995).

A recent series of studies considered a hypothesis in which q_γ charge did result from intramembrane transitions driven by the tubular field but which explained its detailed kinetics in terms of reciprocal allosteric interactions between RyR-Ca²⁺ release channels and tubular DHPR voltage sensors. Complex q_γ currents thus appear specific to skeletal muscle whose activation may well involve such direct interactions. Conversely they are absent from gating currents in either arthropod skeletal muscle or mammalian cardiac muscle which may be more indirectly triggered by extracellular Ca²⁺ entry (for review see Huang, 1993). These studies demonstrated that RyR modification by the inhibitors ryanodine, daunorubicin and tetracaine (at micromolar concentrations) modified the kinetics but preserved the steady-state voltage dependence and pharmacological identity of the q_γ and q_β species (Huang, 1996, 1997; Sarkozi et al. 1996). These findings suggested that RyRs might directly influence the tubular DHPR voltage sensors even though they themselves fall outside the tubular electric field. Conversely, the twitch potentiator perchlorate selectively shifted the activation voltages for delayed q_γ currents and antagonized both the steady-state effects of tetracaine and the kinetic effects of ryanodine and daunorubicin (Huang, 1998).

The present experiments proceeded to examine the influence of caffeine on the kinetic and steady-state properties particularly of the q_γ charge in intact amphibian muscle fibres under comparable experimental conditions and analysis procedures. Whereas perchlorate may potentiate excitation-contraction coupling by enhancing interactions between tubular and cisternal membrane events (Gonzalez & Rios, 1993; Ma et al. 1993), caffeine may act directly upon the RyR. It thus either potentiates or triggers excitation- contraction coupling even in fully polarized muscle fibres (Sandow et al. 1964; Luttgau & Oetliker, 1968; Delay et al. 1986; Klein et al. 1990). Other evidence also suggests direct actions on the calcium release mechanism (see Discussion; Miyamoto & Racker, 1982; Kim et al. 1983; Palade, 1987; Rousseau et al. 1988). The experiments described here also complement recent studies that applied caffeine to either cut (Kovacs & Szucs, 1983; Szucs et al. 1991; Shirokova & Rios, 1996) or intact fibres (Huang, 1986). Thus they used a wide, 25-fold, range of caffeine concentrations (0.2–5 mm) and investigated both charging kinetics and steady-state charge over a full voltage range between −90 to 0 mV. They also explored interactions between the effects of caffeine and those of the inhibitors tetracaine and ryanodine.

METHODS

Sartorius muscles were obtained from cold-adapted frogs (Rana temporaria: Blades Biological, Kent, UK) that had been killed by concussion followed by decapitation and pithing (UK Schedule 1 Home Office regulations). They were dissected in cold (4°C) Ringer solution, mounted in a temperature-controlled recording chamber and then stretched to give a centre fibre sarcomere length of 2.2–2.4 μm as measured through a Zeiss × 40 water immersion objective lens using an eyepiece graticule. The bathing solution was next replaced with the following isotonic solution at the same temperature: 120 mm tetraethylammonium gluconate, 2.0 mm MgCl₂, 2.5 mm RbCl, 800 μm CaCl₂, 1.0 mm 3,4-diaminopyridine, 2 × 10⁻⁷m tetrodotoxin and 3 mm Hepes buffered to pH 7.0. This was followed after 15 min with a similar solution to which 500 mm sucrose had been added. The final test solution containing the adopted concentrations of different combinations of the test agents was introduced within 10 min of commencing electrophysiological studies; the latter in turn were completed within the next 50 min under similarly cooled (2–4°C) conditions. The pharmacological agents used in the experiments were caffeine (0.2, 1.0 or 5.0 mm), ryanodine (0.1 mm) and tetracaine (2.0 mm) (all from Sigma). The above sequence of solution changes ensured that contractile activity had been suppressed by the hypertonic solutions before addition of caffeine. It was found that the application of extracellular caffeine at a concentration of 5.0 mm triggered contractures even in resting fibres bathed in isotonic as opposed to hypertonic solutions, although 0.2 or 1.0 mm caffeine did not. Further increases in caffeine concentration to 10 mm resulted in rapid fibre deterioration that precluded detailed study, as reported on earlier occasions (Luttgau & Oetliker, 1968; Shirokova & Rios, 1996).

A three-microelectrode voltage clamp using conventional 3–5 MΩ glass electrodes was applied to the pelvic ends of superficial muscle fibres directly exposed to the bathing solutions. The voltage-recording electrodes were filled with 3 m KCl and positioned at distances of l = 375 μm (voltage control electrode, V₁) and 2l = 750 μm (second voltage electrode, V₂) from the fibre end respectively. The current injection electrode I₀ was held by a shielded electrode holder designed and built around a 50 Ω SMB gold-plated coaxial cable assembly (Radio Spares, Corby, UK) by Mr B. Secker. It was filled with 2 m potassium citrate and inserted at 5l/2 = 940 μm. Signals that represented the clamp voltage V₁, the voltage difference (V₁–V₂), and the injected current I₀ (t), were filtered through 3-pole Butterworth filters set to a cut off frequency of 1.0 kHz. They were then sampled using a PDP 11/23 computer (Digital Equipment Corporation, MA, USA) with a model 502 interface (Cambridge Electronic Design, Cambridge, UK) using 12-bit analog-to-digital conversion at a 200 μs sampling interval. Five sweeps, spaced by intervals of 20 s, were averaged into each test or control record.

The experiments sought to measure changes in both the kinetic and the steady-state properties of the non-linear intramembrane charge under different conditions of applied caffeine. This involved an acquisition both of test transients obtained in response to voltage steps, of duration 124 ms, to different potentials and of stable control records with which such test transients could be compared. The application of control pulses regularly bracketed successive sets of three or four test runs. They involved the superimposition of +50 mV steps at a time 500 ms following the introduction of prepulse steps to a level of −140 mV. The steady-state values of V₁ (t), V₂ (t) and the injected current I₀ (t) were all reached well before the end of such control steps. These accordingly were determined directly from the traces without the sloping baseline corrections hitherto required for both control and test responses in some cut fibre preparations. This enabled computations of the fibre length constants, λ, internal longitudinal resistances, r_i, and membrane resistances of unit fibre length, r_m, from each individual averaged record. The fibre diameters, d, and specific membrane resistances, R_m, were calculated assuming an internal sarcoplasmic resistivity, R_i, of 391 Ω cm in 2.5 times hypertonic solution at 2°C, and a temperature coefficient of 0.73. The calculated cable constants, particularly those that began and ended each of the experimental runs, were followed to assess for significant systematic changes in fibre stability and condition over time: these are listed in the figure legends. The membrane current as a function of time t through unit fibre surface area, I_m (t), was then calculated from the equation:

Charge movements were calculated as the differences between the test traces and the control currents which were first appropriately scaled to the ratio of the respective amplitudes of the test and control voltage steps. Similar scalings and subtractions also applied to the averaged records of the test and the control voltage (V₁) steps further verified that the derived charge movements indeed reflected non-linear contributions to the electrical properties of the fibres examined. Further corrections concerned any small changes that might have taken place in the linear membrane properties over the course of each set of test voltage steps. Accordingly, the control records with which the comparisons were made were constituted from a weighted mean of the two bracketing control records, weighted using the position of the relevant test average within the bracketed test sequence. All the results of the steady-state determinations outlined here are expressed as means ± s.e.m. The steady-state charge-voltage data were described in terms of Boltzmann functions that related the steady-state charge movement, Q(V), to the maximum charge, Q_max, the transition voltage, V*, and the steepness factor, k:

Some of the data was amenable to fitting to the sum of two rather than one Boltzmann function as described by the differential sensitivities of Q_β and Q_γ charges to tetracaine:

All these functions were optimized by a Levenberg-Marquadt algorithm that performed successive least-squares minimizations of the values of each of the parameters a_j of the generalized non-linear function y(x). This was performed simultaneously over all the experimentally obtained mean values y(x_i) as obtained at each ith test voltage x_i. The successive iterations minimized the values of χ_ν² in a weighted fit derived from the mean and standard errors of the data points y_i where the number of degrees of freedom, ν, incorporates both the number of data points, n, and the number of variables, N:

The curve fits used a weighting factor w_i for each point that was determined by the inverse of its variance σ_i²; the latter was in turn normalized to the average of all such weighting factors. This precaution maximized the likelihood that the fitted functions actually represented the distributions of the parent data.

RESULTS

The experiments investigated both steady-state and kinetic properties of charge movements under a wide range of caffeine concentrations and of test voltages. The conditions and pulse protocols were comparable to those adopted in recent studies on RyR-specific agents (Huang, 1996, 1998). Fibres were studied in 3,4-diaminopyridine and tetraethylammonium-containing solutions that largely replaced extracellular Cl⁻ by gluconate and most of the Ca²⁺ by Mg²⁺. This reduced q_β relative to q_γ charge (Hui & Chen, 1992), reduced Cl⁻ contributions to the overall membrane leak conductance and minimized any time-dependent Ca²⁺ and K⁺ currents. All electrophysiological studies were performed within 50 min of the addition of caffeine to the extracellular solutions. The ‘on’ charging currents obtained under such conditions all decayed to stable baselines and are accordingly displayed following only simple direct current corrections based on the final 20 ms of the ‘on’ records. Intervening time-dependent currents, particularly the inward current phases reported to follow the slow q_γ currents (cf. Csernoch et al. 1991) were not observed in the present study. Gradually developing outward currents occurred only in some of the responses to the strongest depolarizing steps to levels around 0 mV.

Caffeine conserves both the steady-state intramembrane charge and its voltage dependence

Figure 1a displays the steady-state charge-voltage curves obtained from intact voltage-clamped fibres studied in gluconate-containing solutions to which caffeine was added in progressively increasing concentrations. The relevant experimental values are plotted as means ± s.e.m. They establish a number of points that confirm less complete reports on the effects of caffeine on charge movements in intact fibres studied in sulphate-containing solutions (Huang, 1986), but that differ in some respects from recent reports from cut fibres (Shirokova & Rios, 1996). In the absence of caffeine (○), the available charge increased with progressive depolarization to test potentials positive to −80 mV. It passed an inflexion at test potentials around −50 mV and then increased to a maximum value close to 20 nC μF⁻¹ as the test voltages became positive to −30 mV. Both the total amount of charge, Q_max, and its steepness, k, were compatible with a large contribution from the q_γ as opposed to the q_β charge species, as demonstrated in earlier reports from fibres studied in the presence of extracellular gluconate (Hui & Chen, 1992; Huang, 1994; Jong et al. 1995). Thus, Fig. 1b plots the corresponding data obtained following treatment with 2.0 mm tetracaine. This inhibited the q_γ system to leave a considerably more gradual increase in q_β charge with depolarization and a significantly reduced maximum charge of around 8 nC μF⁻¹.

Figure 1. Caffeine conserves both the steady-state distribution and the quantity of the intramembrane charge through a wide range of applied concentrations.

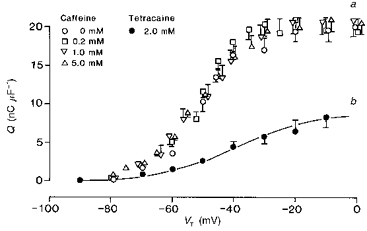
Curve a, the steady-state dependence of the overall intramembrane charge upon test potential in voltage-clamped fibres studied at a −90 mV holding potential at 0.0 mm (controls: ○), 0.2 mm (□), 1.0 mm (▿) and 5.0 mm caffeine (▵). These are compared with the charge-voltage curve obtained following the addition of 2.0 mm tetracaine to abolish selectively the q_γ charge (•; curve b). All points represented as means ± s.e.m. The continuous line represents a two-state Boltzmann function drawn through the points, defined by the parameters: Q_max = 9.2 nC μF⁻¹, V* = −37.1 mV and k = 14.1 mV. Curve a, seven control fibres studied in the absence of either perchlorate or tetracaine (controls: ○): temperature = 2.9 ± 0.31 °C, R_i = 407 ± 5.7 Ω cm. Initial cable constants: λ = 1.9 ± 0.35 mm, r_i = 12265 ± 2077 kΩ cm⁻¹, d = 71 ± 6.8 μm, r_m = 386.3 ± 72.5 kΩ cm, R_m = 8.82 ± 2.62 kΩ cm², C_m = 6.8 ± 0.39 μF cm⁻². Final cable constants: λ = 1.8 ± 0.33 mm, r_i = 11617 ± 2142 kΩ cm⁻¹, d = 72.0 ± 6.7 μm, r_m = 323.8 ± 65.6 kΩ cm, R_m = 8.13 ± 2.41 kΩ cm², C_m = 7.9 ± 0.60 μF cm⁻². Six fibres studied in 0.2 mm caffeine (□): temperature = 6.2 ± 0.14 °C, R_i = 382.7 ± 1.56 Ω cm. Initial cable constants: λ = 2.0 ± 0.15 mm, r_i = 9113.5 ± 714.5 kΩ cm⁻¹, d = 74.2 ± 3.13 μm, r_m = 363.1 ± 39.79 kΩ cm, R_m = 8.49 ± 1.08 kΩ cm², C_m = 6.3 ± 0.46 μF cm⁻². Final cable constants: λ = 1.9 ± 0.23 mm, r_i = 9040.0 ± 709.1 kΩ cm⁻¹, d = 74.6 ± 3.33 μm, r_m = 347.3 ± 43.78 kΩ cm, R_m = 8.23 ± 1.28 kΩ cm², C_m = 6.7 ± 0.45 μF cm⁻². Six fibres in 1.0 mm caffeine (▿): temperature = 5.9 ± 0.27 °C, R_i = 385.0 ± 2.62 Ω cm. Initial cable constants: λ = 2.1 ± 0.16 mm, r_i = 9405.0 ± 1199.9 kΩ cm⁻¹, d = 75.6 ± 4.55 μm, r_m = 362.0 ± 20.62 kΩ cm, R_m = 8.72 ± 0.92 kΩ cm², C_m = 9.9 ± 1.02 μF cm⁻². Final cable constants: λ = 1.8 ± 0.12 mm, r_i = 10587 ± 1424 kΩ cm⁻¹, d = 71.3 ± 4.34 μm, r_m = 312.6 ± 10.28 kΩ cm, R_m = 7.07 ± 0.59 kΩ cm², C_m = 10.5 ± 1.15 μF cm⁻². Six fibres in 5.0 mm caffeine (▵): temperature = 5.5 ± 0.21 °C, R_i = 391.0 ± 2.55 Ω cm. Initial cable constants: λ = 1.9 ± 0.13 mm, r_i = 06582.0 ± 1031.0 kΩ cm⁻¹, d = 91.0 ± 6.56 μm, r_m = 224.4 ± 24.81 kΩ cm, R_m = 6.32 ± 0.59 kΩ cm², C_m = 9.52 ± 1.56 μF cm⁻². Final cable constants: λ = 1.9 ± 0.16 mm, r_i = 6787.0 ± 1070.9 kΩ cm⁻¹, d = 91.8 ± 7.67 μm, r_m = 214.0 ± 23.14 kΩ cm, R_m = 6.05 ± 0.71 kΩ cm², C_m = 10.18 ± 1.90 μF cm⁻². Curve b, six fibres studied in the presence of 2.0 mm tetracaine (•): temperature = 5.4 ± 0.1 °C, R_i = 367 ± 1.2 Ω cm. Initial cable constants: λ = 2.39 ± 0.24 mm, r_i = 6899 ± 1566 kΩ cm⁻¹, d = 93.8 ± 12.3 μm, r_m = 334.7 ± 46.8 kΩ cm, R_m = 9.14 ± 0.79 kΩ cm², C_m = 7.13 ± 1.3 μF cm⁻². Final cable constants: λ = 2.1 ± 0.25 mm, r_i = 7691 ± 1746 kΩ cm⁻¹, d = 90.0 ± 13.0 μm, r_m = 268.7 ± 40.2 kΩ cm, R_m = 6.88 ± 0.45 kΩ cm², C_m = 7.94 ± 1.67 μF cm⁻².

However, the introduction of progressively increasing concentrations of 0.2 (□), 1.0 (▿) and 5.0 mm caffeine (▵) exerted no significant effects upon the charge-voltage (Q(V)) curves. They altered neither the position nor the overall steepness of the Q(V) functions. This observation differs from the effects reported from fibres studied under different conditions of caffeine application and extracellular solution tonicity (Shirokova & Rios, 1996). Table 1 displays the results of the least-squares curve fits of the steady-state charge-voltage data obtained at each caffeine concentration to an equation for a single two-state Boltzmann system which characterizes intramembrane charge by its maximum charge, Q_max, transition voltage, V*, and steepness factor, k. This descriptive approach has been established in previous characterizations of the contribution of the steeply voltage-dependent q_γ charge in both intact (Huang, 1994, 1996) and cut fibre preparations when these were studied in gluconate-containing solutions (Jong et al. 1995). The analysis confirmed that Q_max was conserved at close to a value of 19–21 nC μF⁻¹ through all the caffeine concentrations tested. Furthermore, the steepness factor, k, remained close to 8.0 mV in both the control and the caffeine-treated fibres. If anything, there was a slight but statistically insignificant decrease in this voltage sensitivity at the highest caffeine concentration. Nor was there any significant shift in the transition potential, V*, of the steady-state charge from its control value around −50 mV. These observations resemble recent findings on the effects of the alternative twitch potentiator perchlorate in that both agents conserved the maximum available charge, Q_max, and steepness factor, k. However, the reports differ in that, unlike caffeine, perchlorate shifted the transition voltage V* to an extent that depended on concentration up to 8.0 mm (Huang, 1998).

Table 1.

Descriptions of overall charge-voltage curves in terms of a single two-state Boltzmann relationship at different concentrations of caffeine

Experimental conditions	n	Qmax (nC μF−1)	V*(mV)	k(mV)
0 mm caffeine	7	19.3 ± 0.53	−50.5 ± 1.12	8.0 ± 1.03
0.2 mm caffeine	6	20.3 ± 0.84	−52.7 ± 0.89	7.2 ± 0.42
1.0 mm caffeine	6	19.9 ± 1.10	−51.5 ± 1.67	8.4 ± 0.72
5.0 mm caffeine	6	20.7 ± 0.76	−51.6 ± 0.91	9.0 ± 0.34

n, number of fibres; Q_max, maximum charge

^*V, transition voltage; k, steepness factor.

A closer analysis of the steady-state properties of the individual charge components scrutinized specifically the properties of the q_γ charge through these pharmacological manoeuvres. This confirmed that caffeine also conserved the steady-state properties of the q_γ system at all the tested concentrations. Table 2 summarizes a separation of individual steady-state q_β and q_γ two-state Boltzmann terms (cf. Hui & Chandler, 1990; Huang, 1996). It used values for the q_β term that made it possible to compare the present separations with earlier results which provided the following parameters for the q_β charge: Q_max = 9.2 nC μF⁻¹, V* = −37.1 mV and k = 14.1 mV (Huang, 1998). It was then possible to isolate the q_γ contribution to the experimental values of steady-state charge. The resulting calculations for total q_γ charge (Q_max = 11–13 nC μF⁻¹), steepness factors (k = 4–7 mV) and transition voltages, V* (−53 to −55 mV) closely agreed with earlier characterizations of the q_γ species (Hui, 1983: Huang & Peachey, 1989; Hui & Chandler 1990; Huang, 1996). Furthermore, it gave similar values with all the caffeine concentrations explored (0.0–5.0 mm), again with slightly lower, rather than higher voltage sensitivities at the highest reagent concentrations.

Table 2.

Extraction of Boltzmann parameters for the qγ charge from overall charge-voltage curves at different concentrations of caffeine assuming previously determined values for the parameters describing the qβ charge ^†

Experimental conditions	n	Qmax (nC μF−1)	V* (mV)	k (mV)
0 mm caffeine	7	11.3 ± 0.40	−53.5 ± 1.47	4.2 ± 1.08
0.2 mm caffeine	6	13.1 ± 0.69	−55.1 ± 1.14	5.4 ± 0.63
1.0 mm caffeine	6	12.6 ± 0.84	−54.9 ± 2.12	6.6 ± 0.99
5.0 mm caffeine	6	13.0 ± 0.47	−55.2 ± 1.36	7.0 ± 1.14

^†Q_max = 9.2 nC μF⁻¹

^*V = −37.14 mV; k = 14.08 mV (Huang, 1998).

Distinct early (q_β) and late (q_γ) charge transfers persist despite the presence of low caffeine concentrations (0.2 mm)

The conservation in steady-state properties outlined above contrasted with the effects of such varying concentrations of caffeine upon the kinetics of the charge movements. This particularly concerned the delayed (q_γ) currents. Figure 2 displays typical charge movements from voltage-clamped muscle fibres studied in the presence of 0.2 mm caffeine. The fibres were held at a fixed, −90 mV, membrane potential and were subject to applied voltage clamp steps to a series of progressively depolarized test levels, V_T. The latter were incremented in 5 mV or even 2–3 mV intervals in order closely to investigate for the existence of and changes in steeply voltage-sensitive delayed q_γ charge movement components. In addition, the records are plotted at high magnification in order specifically to emphasize smaller and delayed current components at the expense of the earlier but larger transients. The smaller voltage steps transferred relatively little charge movement; however, larger imposed depolarizations up to levels of V_T = −60 mV gave rise to the simple exponential current decays that have been previously attributed to q_β charge (Huang, 1982). Still larger voltage steps elicited the delayed transients that have been identified with q_γ charge transfer (Adrian & Peres, 1979; Huang, 1982; Hui, 1983). Such ‘on’ currents were particularly small but prolonged around their threshold voltages close to −52 and −50 mV at which they often extended the full duration (124 ms) of the ‘on’ pulse (Fig. 2: horizontal bars beneath traces). Nevertheless, they were accompanied by a marked increase in the initial amplitudes of their corresponding ‘off’ tail currents. The detailed time courses of the ‘on’ q_γ transients were sharply voltage sensitive: even small further depolarizations to levels of −48 or −45 mV gave rise to more rapid current decays that could be completely captured within the ‘on’ sampling interval. By test voltages of −43 mV and −40 mV there were marked and prominent q_γ currents that were clearly distinguishable from the earlier q_β decays. The latter records accordingly exhibited ‘hump’ waveforms that were at least as prominent, and with a voltage dependence as steep, as those that have been classically reported for the q_γ charge movement when caffeine was absent (Adrian & Peres, 1979; Huang, 1982). As reported on earlier occasions, the two component transients merged at voltages that were close or positive to −35 mV. Potential steps to levels in this region left simple exponential decays in which the two charge movement components could not be distinguished. It was only under the latter conditions (test voltages around −10 to 0 mV) that any prolonged outward ‘on’ current phases were sometimes observed. This was accordingly at potentials that were well positive to the voltages at which delayed q_γ transients took place.

Figure 2. Charge movements from fibres within 50 min of exposure to 0.2 mm caffeine show persistent delayed (q_γ) charging currents, following the application of graded depolarizing test steps (see horizontal bars below traces), that are particularly prominent between test voltages of −52 to −45 mV.

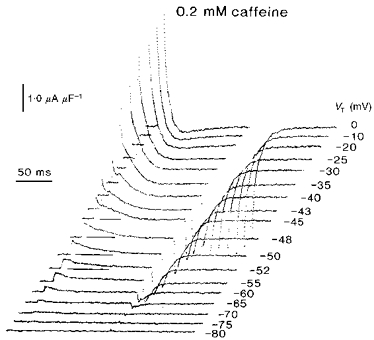
Fibre Z23 in 0.2 mm caffeine: temperature = 5.6 °C, R_i = 390 Ω cm. Initial cable constants: λ = 1.9 mm, r_i = 6992 kΩ cm⁻¹, d = 84.3 μm, r_m = 262.5 kΩ cm, R_m = 6.95 kΩ cm², C_m = 6.2 μF cm⁻². Final cable constants: λ = 1.7 mm, r_i = 8239 kΩ cm⁻¹, d = 77.6 μm, r_m = 226.3 kΩ cm, R_m = 5.52 kΩ cm², C_m = 6.2 μF cm⁻².

The q_β and q_γ waveforms merge with the application of higher (∼5.0 mm) caffeine concentrations

Higher (1.0–5.0 mm) caffeine concentrations markedly altered the kinetics of charge movements whilst still preserving their steady-state properties. Figure 3 displays typical charge movements obtained from fibres that were studied in the presence of 1 mm caffeine and subject to similarly incremented test voltage steps. The smaller voltage steps up to −55 mV elicited the expected decays of the q_β charge. The delayed q_γ currents were visible, but were less prominent and only discernible in the experimental records that were obtained at membrane potentials around −50 and −45 mV.

Figure 3. The delayed charging currents associated with transfers of q_γ charge are either absent or considerably less visible in fibres studied in the presence of 1.0 mm caffeine.

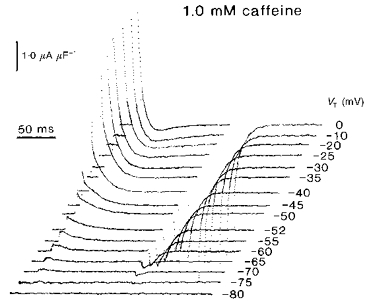
Fibre Z28 in 1.0 mm caffeine: temperature = 6.6 °C, R_i = 379.1 Ω cm. Initial cable constants: λ = 2.8 mm, r_i = 6724.0 kΩ cm⁻¹, d = 84.7 μm, r_m = 516.5 kΩ cm, R_m = 13.75 kΩ cm², C_m = 5.7 μF cm⁻². Final cable constants: λ = 2.9 mm, r_i = 5857.9 kΩ cm⁻¹, d = 90.8 μm, r_m = 491.2 kΩ cm, R_m = 14.01 kΩ cm², C_m = 6.7 μF cm⁻².

Still higher (5.0 mm) caffeine concentrations further accelerated the time courses of the q_γ decays. Figure 4 displays charge movements from fully polarized fibres that were exposed to 5.0 mm caffeine and again subject to graded depolarizing test steps. It displays the results of exploring a full potential range between −90 and 0 mV, entirely in progressive 5 mV increments. The small depolarizing steps transferred little charge. Larger voltage steps elicited progressively larger charge movements. However, these now did not show significant contributions from delayed transients anywhere within the explored potential range of test voltages even at the relatively high display magnifications adopted here. Both the ‘on’ and the ‘off’ charge movements thus consisted exclusively of monotonic decays whose amplitudes increased with the test voltage excursion until charge saturation. There was an increase in the initial amplitude of the currents, consistent with a kinetic alteration in a nevertheless conserved total quantity of charge. The responses decayed completely to leave DC baselines: only the responses to the strongest depolarizations showed any appreciable delayed outward current. The charge movements thus resembled the exponential transients that have been observed in some cut fibre preparations or the records obtained following treatment with other RyR-specific agents such as ryanodine and daunorubicin (Huang, 1996).

Figure 4. Charge movements in the presence of 5.0 mm caffeine are simple monotonic decays despite the conserved steady-state charge-voltage curves.

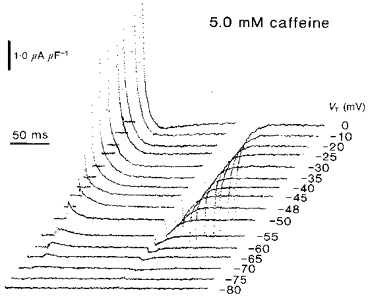
Fibre Z25 studied in 5.0 mm caffeine: temperature = 6.1 °C, R_i = 383.9 Ω cm. Initial cable constants: λ = 2.0 mm, r_i = 9734.0 kΩ cm⁻¹, d = 70.9 μm, r_m = 398.1 kΩ cm, R_m = 8.86 kΩ cm², C_m = 8.6 μF cm⁻². Final cable constants: λ = 1.9 mm, r_i = 10839 kΩ cm⁻¹, d = 67.2 μm, r_m = 335.9 kΩ cm, R_m = 7.51 kΩ cm², C_m = 8.6 μF cm⁻².

Taken together with the findings in Fig. 1, these kinetic findings suggest that the introduction of caffeine altered the time course of an otherwise conserved total available charge. The effects of caffeine in converting delayed transients into rapid decays contrast with the persistence of late charge movement components following perchlorate treatment (Huang, 1998). They agree with earlier reports in cut fibres that studied the effects of low (0.2–0.5 mm) caffeine concentrations. Caffeine then did not alter the amount or the voltage dependence of intramembrane charge, overall charging kinetics (0.2–0.5 mm caffeine: Klein et al. 1990), or the presence or otherwise specifically of distinguishable delayed, q_γ charge transfers (Kovacs & Szucs, 1983: see their Fig. 2). However, the findings contrast with studies on single cut fibres that were either exposed to 0.75 mm caffeine (Szucs et al. 1991) or studied in flow systems that rapidly introduced and withdrew high (10 mm) caffeine concentrations (Shirokova & Rios, 1996). The former study reported increased ‘hump’ currents and increased intramembrane charge particularly around the contractile threshold. The latter observed that the application of caffeine prior to and during voltage pulses increased intramembrane charge movement by 40% and also yielded larger, more prominent delayed q_γ charge movements. However, a full comparison of these results is not possible. The former study considered threshold voltages only; the latter analysis only measured charge movement that followed pulses to test voltages between −60 and −40 mV rather than the entire voltage range.

Caffeine obliterates the effects of ryanodine on intramembrane charge

A previous paper reported that the RyR antagonist ryanodine both modified q_γ ‘hump’ currents into exponential decays and shifted their corresponding steady-state charge-voltage curves in the positive direction. It nevertheless conserved both the separate pharmacological identities of the separate steady-state q_β and q_γ components and their individual steepness factors (Huang, 1996). These actions were antagonized by the twitch potentiator perchlorate and were attributed to primary actions of both these reagents at the level of a RyR allosterically coupled to the intramembrane charge (Huang, 1998). Figure 5 illustrates the results of similar experiments that explored for interactions between the effects of ryanodine and caffeine at the level of steady-state (Fig. 5A) and kinetic (Fig. 5B) features of the charge movement. The fibres were studied using concentrations of 5.0 mm caffeine and 0.1 mm ryanodine, respectively. Both of these agents, when applied by themselves at such concentrations, would have transformed the delayed q_γ components into indistinguishable simple monotonic decays yet conserved the total available intramembrane charge (Fig. 1; see also Huang, 1996). In contrast, Fig. 5B demonstrates that the reagents at least partially restored the delayed kinetic properties of the q_γ system when they were applied in combination. The delayed ‘on’ ‘hump’ currents (marked by horizontal bars beneath the records) reappeared at similar test voltages (−47 to −40 mV) as in control fibres that were exposed to neither drug. The records now showed distinguishable early q_β and later q_γ current components.

Figure 5. Caffeine (5 mm) reverses the +10 mV shift in the steady-state charge-voltage curve brought about by 0.1 mm ryanodine and restores delayed (q_γ) charging transients.

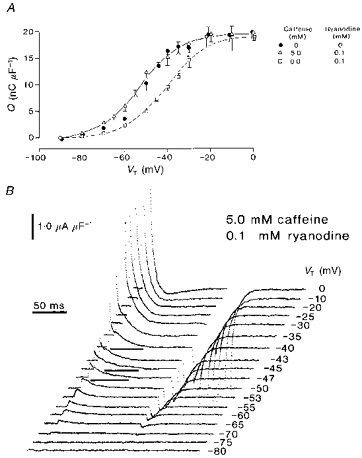
A, charge-voltage curves obtained in control fibres (•), and fibres exposed to 0.1 mm ryanodine (□) and to both 5.0 mm caffeine and 0.1 mm ryanodine (▵). B, charge movements displayed at high gain from a typical fibre exposed to both 5.0 mm caffeine and 0.1 mm ryanodine. All points represented as means ± s.e.m. The dotted line is a two-state Boltzmann function drawn through the data points obtained in the presence of ryanodine alone, characterized by: Q_max = 20.0 nC μF⁻¹, V* = −39.0 mV and k = 9.5 mV. The continuous line is a similar function fitted to data obtained in the presence of both ryanodine and caffeine, where: Q_max = 19.1 nC μF⁻¹, V* = −53.4 mV and k = 8.8 mV. A, four control fibres studied in the presence of 0.1 mm ryanodine only: temperature = 4.2 ± 0.01 °C, R_i = 407.6 ± 1.56 Ω cm. Initial cable constants: λ = 1.46 ± 0.10 mm, r_i = 14253 ± 3609 kΩ cm⁻¹, d = 65.4 ± 6.8 μm, r_m = 274.4 ± 28.9 kΩ cm, R_m = 5.41 ± 0.35 kΩ cm², C_m = 9.6 ± 2.37 μF cm⁻². Final cable constants: λ = 1.29 ± 0.04 mm, r_i = 14219 ± 3064 kΩ cm⁻¹, d = 64.7 ± 6.9 μm, r_m = 225.4 ± 33.02 kΩ cm, R_m = 4.3 ± 0.18 kΩ cm², C_m = 10.3 ± 2.3 μF cm⁻². Six fibres studied in the presence of both 5 mm caffeine and 0.1 mm ryanodine: temperature = 6.3 ± 0.05 °C, R_i = 381.5 ± 0.64 Ω cm. Initial cable constants: λ = 1.9 ± 0.23 mm, r_i = 8294 ± 1883 kΩ cm⁻¹, d = 86.1 ± 8.03 μm, r_m = 246.6 ± 23.91 kΩ cm, R_m = 6.77 ± 1.09 kΩ cm², C_m = 10.7 ± 0.99 μF cm⁻². Final cable constants: λ = 1.6 ± 0.15 mm, r_i = 9504.0 ± 2356.0 kΩ cm⁻¹, d = 81.3 ± 7.00 μm, r_m = 201.9 ± 20.23 kΩ cm, R_m = 5.12 ± 0.65 kΩ cm², C_m = 11.4 ± 1.05 μF cm⁻². B, fibre Z24 studied in the presence of both 5.0 mm caffeine and 0.1 mm ryanodine: temperature = 6.1 °C, R_i = 383.9 Ω cm. Initial cable constants: λ = 1.9 mm, r_i = 9541.0 kΩ cm⁻¹, d = 71.6 μm, r_m = 331.9 kΩ cm, R_m = 7.47 kΩ cm², C_m = 5.9 μF cm⁻². Final cable constants: λ = 1.7 mm, r_i = 10996 kΩ cm⁻¹, d = 66.7 μm, r_m = 307.5 kΩ cm, R_m = 6.44 kΩ cm², C_m = 5.8 μF cm⁻².

Total charge was conserved despite this combination of reagents and the kinetic changes that they produced. Thus, Fig. 5A demonstrates that (i) a fit of a single Boltzmann function to the charge-voltage curves yielded similar values for maximum charge (Q_max) of 20.0 ± 0.37 nC μF⁻¹ in fibres exposed to ryanodine alone and 19.1 ± 1.18 nC μF⁻¹ in fibres that were exposed to both ryanodine and caffeine. (ii) However, the inclusion of ryanodine by itself shifted the steady-state charge-voltage curves in the positive direction from a transition voltage (V*) of −50.5 ± 1.12 mV in the absence of either reagent (see Table 1) to −39.0 ± 1.41 mV (Fig. 5A, □). (iii) Nevertheless, the further introduction of caffeine (5.0 mm; Fig. 5A, ▵) to the experimental solutions completely reversed this effect. It returned the charge- voltage functions along the voltage axis to a position (V* = −53.4 ± 1.41 mV) close to that expected in either control fibres (Fig. 5A, •) or fibres that were studied in caffeine alone (See Table 1). (iv) These shifts took place in the absence of major changes in the steepness factor, k. The latter was 9.5 ± 1.29 mV with ryanodine alone and 8.8 ± 0.55 mV in fibres that were exposed to both ryanodine and caffeine, values compatible with significant contributions from intact q_γ intramembrane charge in fibres studied in the presence of gluconate (Jong et al. 1995; Huang, 1996). These findings closely resembled the earlier observations in which perchlorate similarly antagonized the effect of ryanodine on the q_γ charge movement (Huang, 1998).

The low (0.2 mm) but paradoxically not the high (1.0–5.0 mm) caffeine concentrations restore the q_γ currents previously suppressed by tetracaine

A recent paper reported that the twitch potentiator perchlorate also interacts with tetracaine in influencing the intramembrane charge. It suggested that the two agents exerted competitive actions upon a common RyR, or alternatively that these drugs acted separately upon intramembrane DHPRs and RyRs to give rise to an interaction that involved the allosteric coupling between them (Huang, 1998). Figures 6 and 7 summarize the results of experiments that similarly explored for possible interactions between the action of caffeine, applied at different concentrations, in muscle fibres that were also exposed to a fully effective, 2.0 mm, tetracaine concentration. Tetracaine by itself abolished the delayed q_γ transient to leave exponential q_β decays, as confirmed on earlier occasions (e.g. Huang, 1996). Figure 6A displays charge movement records obtained from fibres that were exposed both to the lowest (0.2 mm) caffeine concentrations considered here and to 2.0 mm tetracaine following successively larger depolarizing steps from the −90 mV holding potential. It demonstrates that 0.2 mm caffeine restored the delayed q_γ transients to families of such records. The restored q_γ transients (Fig. 6A: horizontal lines beneath traces) appeared at more positive potentials (−40 to −20 mV) than in control fibres. In contrast, the higher (1.0 or 5.0 mm) caffeine concentrations paradoxically did not restore the q_γ charge movements following tetracaine treatment. Figure 6B shows this in transients that were obtained in the presence of 5.0 mm caffeine and 2.0 mm tetracaine. The currents now consisted of the considerably reduced exponential decays that were similar to those hitherto attributed to a transfer of q_β charge alone.

Figure 6. Low (0.2 mm) but not high concentrations of caffeine reverse the action of 2.0 mm tetracaine in abolishing delayed q_γ charge movements.

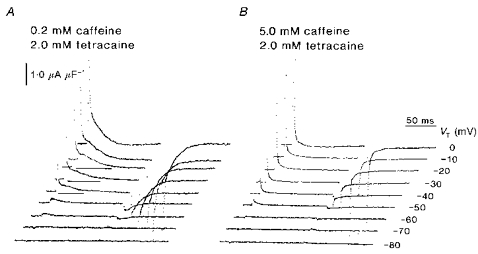
A, typical charge movements obtained in the presence of both 0.2 mm caffeine and 2.0 mm tetracaine. B, charge movements from a fibre studied in 5.0 mm caffeine and 2 mm tetracaine. A, fibre Z84 in the presence of both 0.2 mm caffeine and 2 mm tetracaine: temperature = 4.6 °C, R_i = 402.5 Ω cm. Initial cable constants: λ = 1.7 mm, r_i = 10046 kΩ cm⁻¹, d = 71.4 μm, r_m = 273.9 kΩ cm, R_m = 6.15 kΩ cm², C_m = 7.3 μF cm⁻². Final cable constants: λ = 1.7 mm, r_i = 8587 kΩ cm⁻¹, d = 77.3 μm, r_m = 249.8 kΩ cm, R_m = 6.06 kΩ cm², C_m = 8.8 μF cm⁻². B, fibre Z35 studied in 5.0 mm caffeine and 2.0 mm tetracaine: temperature = 5.0 °C, R_i = 379.4 Ω cm. Initial cable constants: λ = 1.8 mm, r_i = 6149.0 kΩ cm⁻¹, d = 90.7 μm, r_m = 203.9 kΩ cm, R_m = 5.82 kΩ cm², C_m = 10.0 μF cm². Final cable constants: λ = 1.6 mm, r_i = 4852.0 kΩ cm⁻¹, d = 102.1 μm, r_m = 120.31 kΩ cm, R_m = 3.86 kΩ cm², C_m = 12.1 μF cm⁻².

Figure 7. Low (0.2 mm) but not high (1.0–5.0 mm) concentrations of caffeine reverse the action of 2 mm tetracaine and restore the steady-state q_γ charge.

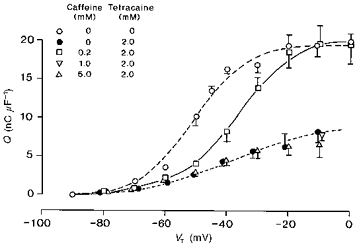
Charge-voltage curves obtained from fibres that were studied in the absence of caffeine or tetracaine (○), and fibres studied in the presence of 2.0 mm tetracaine alone (•) are included as controls. These are compared with charge-voltage curves obtained from fibres that were studied in the presence of 2.0 mm tetracaine with the further addition of 0.2 mm (□), 1.0 mm (▿) and 5.0 mm caffeine (▵). All points represented as means ± s.e.m. The continuous line represents the two-state Boltzmann function drawn through the points obtained in the presence of 0.2 mm caffeine and 2.0 mm tetracaine characterized by: Q_max = 20.5 nC μF⁻¹, V* = −37.3 mV and k = 9.3 mV. The dotted line drawn through the points obtained in the presence of 5.0 mm caffeine and 2.0 mm tetracaine is described by: Q_max = 8.3 nC μF⁻¹, V* = −46.8 mV and k = 11.3 mV. Four fibres studied in the presence of 0.2 mm caffeine and 2.0 mm tetracaine: temperature = 4.8 ± 0.27 °C, R_i = 400.3 ± 3.42 Ω cm. Initial cable constants: λ = 2.2 ± 0.52 mm, r_i = 9078 ± 1795 kΩ cm⁻¹, d = 82.8 ± 10.68 μm, r_m = 330.7 ± 68.1 kΩ cm, R_m = 9.57 ± 3.28 kΩ cm², C_m = 9.6 ± 0.85 μF cm⁻². Final cable constants: λ = 1.8 ± 0.22 mm, r_i = 8351 ± 1282 kΩ cm⁻¹, d = 81.3 ± 5.76 μm, r_m = 243.4 ± 33.06 kΩ cm, R_m = 6.31 ± 1.13 kΩ cm², C_m = 10.1 ± 0.58 μF cm⁻². Eleven fibres studied in the presence of 1 mm caffeine and 2 mm tetracaine: temperature = 4.5 ± 0.20 °C, R_i = 403.7 ± 2.48 Ω cm. Initial cable constants: λ = 1.5 ± 0.17 mm, r_i = 15809 ± 2326 kΩ cm⁻¹, d = 62.1 ± 5.45 μm, r_m = 340.8 ± 42.56 kΩ cm, R_m = 6.36 ± 0.86 kΩ cm², C_m = 8.9 ± 0.92 μF cm⁻². Final cable constants: λ = 1.42 ± 0.15 mm, r_i = 14807 ± 2831 kΩ cm⁻¹, d = 69.9 ± 10.01 μm, r_m = 264.7 ± 45.17 kΩ cm, R_m = 5.05 ± 0.635 kΩ cm², C_m = 9.3 ± 0.77 μF cm⁻². Four fibres studied in the presence of 5 mm caffeine and 2 mm tetracaine: temperature = 5.1 ± 0.22 °C, R_i = 395.3 ± 2.62 Ω cm. Initial cable constants: λ = 1.8 ± 0.13 mm, r_i = 8609 ± 1386 kΩ cm⁻¹, d = 79.1 ± 5.59 μm, r_m = 261.6 ± 20.1 kΩ cm, R_m = 6.46 ± 0.60 kΩ cm², C_m = 9.6 ± 0.65 μF cm⁻². Final cable constants: λ = 1.6 ± 0.08 mm, r_i = 6827 ± 948 kΩ cm⁻¹, d = 88.2 ± 5.59 μm, r_m = 173.5 ± 18.8 kΩ cm, R_m = 4.71 ± 0.36 kΩ cm², C_m = 11.3 ± 0.91 μF cm⁻².

Low (0.2 mm) but not high (1.0–5.0 mm) caffeine concentrations restore steady-state q_γ charge in tetracaine-treated fibres

The above effects of caffeine reflected a restoration of steady-state q_γ charge that the tetracaine treatment had previously abolished. Thus, Fig. 7 displays the corresponding effects of different caffeine concentrations upon the steady-state charge-voltage curves obtained from fibres that were treated with a fixed and fully effective (2.0 mm) tetracaine concentration. Taken together, the findings support the possibility that such agents interact and do so at the level of the intramembrane charge. The data were described in terms of their approximations to two-state Boltzmann functions (cf. Jong et al. 1995; Huang, 1996). By itself, tetracaine more than halved the available intramembrane charge and reduced its voltage dependence (Fig. 7: •; Q_max ≈ 9.0 nC μF⁻¹, k ≈ 14 mV, V* ≈ −37.1 mV: cf. Fig. 1). However, the introduction of low (0.2 mm; Fig. 7: □) caffeine concentrations restored the maximum charge, Q_max, to its full value giving Q_max = 20.5 ± 0.95 nC μF⁻¹; it also returned the full steepness of its voltage dependence, giving k = 9.3 ± 1.33 mV. Although the resulting charge-voltage curve showed a positive shift to V* = −37.3 ± 1.72 mV (compare circles and squares in Fig. 7), both the maximum available charge and the steepness factor were consistent with a full contribution from the q_γ system (cf. Jong et al. 1995; Huang, 1996). Furthermore, a separation of individual steady-state q_β and q_γ Boltzmann terms yielded values that would be expected for an isolated q_γ charge: Q_max = 11.6 ± 0.74 nC μF⁻¹, V* = −36.4 ± 2.16 mV and k = 6.6 ± 1.78 mV.

The findings concerning the effects of low (0.2 mm) caffeine concentrations thus paralleled recent reports on the interactions between perchlorate and tetracaine at the level of the intramembrane charge (cf. Huang, 1998). However, higher caffeine concentrations paradoxically failed to reverse the inhibitory actions of tetracaine. Thus in the presence of 5.0 mm caffeine (Fig. 7: ▵), the charge-voltage relationship retained the features that were expected for an isolated q_β charge by itself to give the following steady-state parameters: Q_max = 8.3 ± 1.30 nC μF⁻¹, V* = −46.8 ± 3.64 mV and k = 11.3 ± 1.73 mV. Similarly, there was no charge restoration with the introduction of 1.0 mm caffeine for which a voltage step to a −10 mV test potential only displaced 7.96 ± 0.85 nC μF⁻¹ of intramembrane charge (Fig. 7, ▿).

DISCUSSION

The present experiments extended recent studies on the actions of agents known to influence the RyR, upon the properties of intramembrane charge in intact voltage-clamped amphibian muscle. These particularly investigated alterations in the q_γ charge movement in view of its possible roles in excitation-contraction coupling (for review see Huang, 1993). Ryanodine, daunorubicin and micromolar tetracaine concentrations modified q_γ kinetics whilst conserving its pharmacological and steady-state identity (Huang, 1996, 1997; see also Sarkozi et al. 1996). Conversely, the twitch potentiator perchlorate shifted the appearance of delayed q_γ charge transfers to more negative test potentials at which prior q_β decays were actually absent. Perchlorate also antagonized both the steady-state effects of tetracaine and the kinetic effects of ryanodine and daunorubicin on q_γ charge (Huang, 1998). These findings could be reconciled to a discrete intramembrane DHPR q_γ charge acting as voltage sensor (see Huang, 1994) provided RyR modifications could exert their kinetic effect through a reciprocal allosteric coupling (Huang, 1996, 1998). Such direct interactions are consistent with the close associations of four DHPRs with each RyR in skeletal muscle triads demonstrated in morphological studies. Biochemical evidence similarly suggests allosteric links between DHPRs and RyR-I or RyR-α isoforms in mammalian and amphibian skeletal muscle triads, respectively, but not with the RyR-II isoforms in cardiac muscle (for review see Meissner, 1994; Anderson & Meissner, 1995). The features might also explain the existence of complex q_γ charge movements in striated muscle despite their absence in other contractile systems triggered more indirectly through extracellular Ca²⁺ entry (for review see Huang, 1993).

The experiments here went on to explore the actions of caffeine upon intramembrane charge under comparable conditions of pulse procedure, external solutions, osmolarity and temperature. Perchlorate may influence interactions between voltage-sensing tubular events and the opening of cisternal Ca²⁺ release channels (Luttgau et al. 1983; Gonzalez & Rios, 1993; Ma et al. 1993). In contrast, caffeine may activate RyR-Ca²⁺ release channels directly. Caffeine (6–10 mm) readily crosses cell membranes to activate contraction independently of extracellular [Ca²⁺] even in fully polarized fibres (Sandow et al. 1964; Luttgau & Oetliker, 1968; but see also Hoock et al. 1996). Subthreshold levels (< ∼0.1 mm) potentiate twitch tension and the release of intracellularly stored Ca²⁺ at a step subsequent to tubular excitation (< 2 mm: Delay et al. 1986). They shift the voltage dependence both of tension generation and of Ca²⁺ release (Luttgau & Oetliker, 1968; Klein et al. 1990), and elicit ‘sarcomeric oscillations’ under some conditions (Kumbaraci & Nastuk, 1982). Low caffeine concentrations additionally prolong Ca²⁺ release for many seconds beyond the end of short (10 ms) depolarizing pulses, suggesting direct effects upon Ca²⁺-induced Ca²⁺ release (Klein et al. 1990). Suprathreshold concentrations (6–10 mm) elicit phasic or even sustained contractures. Caffeine also reverses the inhibitory effect of tetracaine on contractile activation, suggesting related sites of antagonistic action (Luttgau & Oetliker, 1968). Finally, caffeine directly potentiates Ca²⁺-induced Ca²⁺ release in skinned skeletal muscle fibres (Endo, 1977), by isolated skeletal sarcoplasmic reticular vesicles (Miyamoto & Racker, 1982; Kim et al. 1983; Palade, 1987) and by sarcoplasmic reticular release channels from skeletal muscle (Rousseau et al. 1988).

The studies described here also compliment recent reports on the effects of caffeine itself in both cut and intact fibres. First, they studied intact rather than cut fibres under conditions that were comparable with those used in previous studies using other RyR-specific agents (cf. Huang, 1996, 1998). Second, they closely investigated a full depolarizing voltage range over a wider range of concentrations (0.2–5 mm) than used hitherto (cf. Kovacs & Szucs, 1983; Shirokova & Rios, 1996). Third, they examined for alterations both in charging kinetics and steady-state charge (cf. Huang, 1986). Finally, they also investigated the possible interactions between the actions of caffeine and those of the known RyR inhibitors tetracaine and ryanodine (cf. Huang, 1998). The findings extend recent suggestions made about the nature of the possible interactions between the DHPR voltage sensor and the RyR-Ca²⁺ release channel.

In some respects, the present findings agreed with earlier explorations that used low caffeine concentrations (0.2–0.5 mm: Kovacs & Szucs, 1983; Klein et al. 1990). Thus distinct q_β and delayed q_γ contributions persisted in experimental records in fibres that were exposed to the lowest (0.2 mm) applied concentrations, at least when studied within 50 min of adding reagent. However, the results of using 1.0–5.0 mm caffeine significantly differed from some earlier reports in cut fibres. The latter studied threshold charge in fibres exposed to 0.75 mm caffeine (Szucs et al. 1991) or fibres subject to rapid applications and withdrawals of 10 mm caffeine (Shirokova & Rios, 1996). Both reported an increased q_γ charge, the latter by as much as 40%, as well as an enhanced prominence of delayed q_γ ‘humps’ in charging records. Such results suggested that q_γ currents were a consequence of Ca²⁺ release rather than voltage change.

In contrast, the present studies have demonstrated that progressive increases in caffeine concentration do not significantly affect the maximum steady-state charge. These steady-state properties were defined by single two-state Boltzmann functions, an approach used recently to assess the persistence of the steeply voltage-dependent q_γ system in fibres studied in gluconate-containing solutions (Jong et al. 1995; Huang, 1996). Such a characterization of full charge-voltage curves suggested that the total charge Q_max neither increased nor decreased significantly through these manipulations. Furthermore, both the overall steepness factors, k, and the position, V*, of the charge-voltage curves did not significantly alter from their control values. These quantitative results were reinforced using a two-component Boltzmann analysis procedure to separate individual q_β and q_γ contributions to intramembrane charge (cf. Huang, 1996, 1998). The latter findings were directly compatible with unaltered steady-state distributions of a persistent intramembrane q_γ charge dependent upon the tubular membrane voltage (Jong et al. 1995; Huang, 1994, 1996).

The present findings also contrast with recent observations that higher caffeine concentrations increased the prominence of the delayed q_γ charging transient (Shirokova & Rios, 1996). Thus, increases in caffeine concentration, from 0.2 or 1.0 to 5.0 mm, gave faster q_γ transients that consequently tended to fuse with and become indistinguishable from the q_β contribution. The overall records now resembled simple monotonic decays. In this respect, the findings instead resemble the consequences of recent pharmacological RyR modification procedures that also preserved total charge but altered q_γ kinetics (Huang, 1996, 1997). These observations can also be reconciled with the negative shifts that caffeine produced in strength-duration curves, as these were most marked at short pulse durations rather than at the rheobase (Kovacs & Szucs, 1983): this suggests parallel changes in the activation kinetics of Ca²⁺ release. Detailed analysis of these apparent discrepancies with the previous findings is difficult. The present studies examined the full available voltage range and could therefore obtain and compare values of maximum charge, Q_max, transition voltage, V*, and steepness, k, of the complete charge-voltage relationship. However, the earlier studies each used different experimental conditions, and more restricted ranges of caffeine concentrations. In addition, Shirokova & Rios (1996) investigated charge movements following pulses to test voltages between −60 and −40 mV only. Szucs et al. (1991) considered only threshold voltages and obtained biphasic traces from comparisons of records obtained before and after caffeine treatment. The latter could as much reflect alterations in charging kinetics as increases in steady-state charge in the presence of caffeine.

The effects of caffeine described here additionally invite comparison with those of the alternative twitch potentiator perchlorate as well as with their interactions with ryanodine and daunorubicin under otherwise similar conditions. All these RyR-specific reagents conserved both the overall charge and its voltage sensitivity (Huang, 1996, 1998). This is consistent with actions upon the RyR, as the RyR falls outside the tubular field and therefore would itself not be expected to contribute voltage-sensitive intramembrane charge. However, both caffeine and perchlorate exerted major kinetic effects upon the charge movement, either when applied alone, or as reflected in their interactions with ryanodine or daunorubicin. These findings can be reconciled to a steady-state q_γ charge movement that reflects transitions within intramembrane DHPRs driven by the tubular field (Huang, 1994). However, the kinetic complexities of such q_γ charge would then be the result of their reciprocal allosteric interactions with the RyRs that might accompany the opening of the latter Ca²⁺ release channels (cf. Huang, 1996, 1998). Thus one might suggest a transition from an inactive (‘tense’) form with associated DHPR and RyR subunits to a dissociated (‘relaxed’) form in which the Ca²⁺ release channels were open (Monod et al. 1965). Such a co-operative transition would generate ‘on’ and ‘off’ kinetic features that resemble q_γ charge movement (Huang, 1983, 1984).

Perchlorate is thought to act through its powerful chaotropic actions in promoting dissociation of protein subunits in contact (Luttgau et al. 1983; Gonzalez & Rios, 1993; Ma et al. 1993). This would preserve or enhance the generation of delayed q_γ charge movements following the imposition of voltage steps that might reflect such reactions. Such a prediction was confirmed by recent results (Huang, 1998). In contrast, caffeine applied at sufficiently high concentrations (e.g. 5 mm) acts directly upon the RyR-Ca²⁺ release channel itself independently of any change in the membrane potential (see above). The present experiments thus demonstrated that caffeine eliminated the delayed character of q_γ kinetics but did not shift the position of its steady-state voltage dependence. The DHPR transitions might then be influenced in two possible ways. First, the allosteric model suggested above would predict that the resulting Ca²⁺ release channel opening would be accompanied by a dissociation of the RyR-DHPR complex. The latter delayed transition then would not be reflected in the q_γ charge movements produced by subsequent voltage steps. Charge movements would then show the simple monotonic q_γ decays as described here. However, delayed transfers of q_γ charge would persist at the lower caffeine concentrations (e.g. 0.2 and 1.0 mm) if these initially opened fewer such Ca²⁺ release channels. Secondly, applications of caffeine also could influence levels of cytosolic [Ca²⁺] (see e.g. Shirokova & Rios, 1996) that could themselves influence q_γ charging kinetics (see Jong et al. 1995).

The latter effect could at least partially explain the kinetic influences of caffeine upon intramembrane charge when this agent was applied by itself. However, there are also the interactions between caffeine (and perchlorate) and the remaining RyR-specific reagents ryanodine and tetracaine. Thus both caffeine (5.0 mm) and perchlorate (Huang, 1998) obliterated the shifts in transition potential, V*, produced by ryanodine, and restored the prolonged nature of the q_γ waveforms yet conserved both total available charge, Q_max, and its steepness factor, k. Yet a similar caffeine concentration applied alone had the opposite effect upon q_γ kinetics. Furthermore, both perchlorate and caffeine interacted with tetracaine in modifying the amount and distribution of charge in the steady state. Thus perchlorate antagonized the action of tetracaine with a concentration dependence suggestive of simple competitive models that involved separate binding sites on the same or adjacent but coupled subunits (Huang, 1998). One might suggest similar mechanisms by which low caffeine concentrations restored q_γ charge in tetracaine-treated fibres. However, the allosteric hypothesis suggested here would predict that the higher caffeine concentrations would dissociate these receptor complexes upon which such drug interactions might well depend. This would account for the contrasting effects of caffeine at the higher concentrations explored here, which paradoxically failed to restore the q_γ charge.