Skip to main content
PMC home page
The Journal of Physiology logoLink to The Journal of Physiology
. 1998 Dec 15;513(Pt 3):915–925. doi: 10.1111/j.1469-7793.1998.915ba.x

Mechanical advantage of the human parasternal intercostal and triangularis sterni muscles

André De Troyer *,†, Alexandre Legrand *,†, Pierre-Alain Gevenois ‡, Theodore A Wilson §
PMCID: PMC2231324  PMID: 9824728

Abstract

  1. Previous studies in dogs have demonstrated that the maximum change in airway pressure (ΔPao) produced by a particular respiratory muscle is the product of three factors, namely the mass of the muscle, the maximal active muscle tension per unit cross-sectional area (∼3.0 kg cm−2), and the fractional change in muscle length per unit volume increase of the relaxed chest wall (i.e. the muscle's mechanical advantage). In the present studies, we have used this principle to infer the ΔPao values generated by the parasternal intercostal and triangularis sterni muscles in man.

  2. The mass of the muscles and the direction of the muscle fibres relative to the sternum were first assessed in six cadavers. Seven healthy individuals were then placed in a computed tomographic scanner to determine the orientation of the costal cartilages relative to the sternum and their rotation during passive inflation to total lung capacity. The fractional changes in length of the muscles during inflation, their mechanical advantages, and their ΔPao values were then calculated.

  3. Passive inflation induced shortening of the parasternal intercostals in all interspaces and lengthening of the triangularis sterni. The fractional shortening of the parasternal intercostals decreased gradually from 7.7% in the second interspace to 2.0% in the fifth, whereas the fractional lengthening of the triangularis sterni increased progressively from 5.9 to 13.8%. These rostrocaudal gradients were well accounted for by the more caudal orientation of the cartilages of the lower ribs.

  4. Since these fractional changes in length corresponded to a maximal inflation, the inspiratory mechanical advantage of the parasternal intercostals was only 2.2–0.6% l−1, and the expiratory mechanical advantage of the triangularis sterni was only 1.6–3.8% l−1. In addition, whatever the interspace, parasternal and triangularis muscle mass was 3–5 and 1–3 g, respectively. As a result, the magnitude of the ΔPao values generated by a maximal contraction of the parasternal intercostals or triangularis sterni in all interspaces would be only 1–3 cmH2O.

  5. These studies therefore confirm that the parasternal intercostals in man have an inspiratory action on the lung whereas the triangularis sterni has an expiratory action. However, these studies also establish the important fact that the pressure-generating ability of both muscles is substantially smaller than in the dog.

Although the actions of most respiratory muscles on the chest wall in humans have been qualitatively described, the question of how much lung expansion (or deflation) each muscle can produce has not been answered. This is a difficult question because these muscles are inaccessible and cannot be maximally activated in isolation. However, recent theoretical studies by Wilson & De Troyer (1992, 1993) have proposed an indirect approach, based on the Maxwell reciprocity theorem. When applied to the respiratory system, this standard theorem of mechanics predicts that the potential change in airway pressure (ΔPao) produced by a particular muscle contracting alone against a closed airway is related to the mass (m) of the muscle, the maximal active muscle tension per unit cross-sectional area (σ), and the fractional change in muscle length (ΔL/L) per unit volume increase of the relaxed chest wall (ΔVL)Rel, such that:

graphic file with name tjp0513-0915-m1.jpg (1)

Thus, a muscle that shortens during passive inflation would cause a fall in Pao when it contracts; this muscle would therefore have an inspiratory effect on the lung. Conversely, a muscle that lengthens during passive inflation would cause a rise in Pao during contraction, and so have an expiratory effect.

The validity of this equation has been tested experimentally on a number of canine inspiratory and expiratory muscles, such as the interchondral portion of the internal intercostals (the so-called parasternal intercostals) (Legrand et al. 1996; De Troyer et al. 1996), the scalenes, the sternomastoids (Legrand et al. 1997), and the triangularis sterni (De Troyer & Legrand, 1998). For all these muscles, there was a unique relationship between ΔPao per unit muscle mass and [ΔL/(LΔVL)]Rel. Furthermore, the coefficient of proportionality (σ) between ΔPao/m and [ΔL/(LΔVL)]Rel was 3.0, in close agreement with values of maximal active muscle tension measured in vitro (Close, 1972; Farkas, 1991; Tao & Farkas, 1992). These observations therefore provide strong evidence in support of eqn (1), and this implies that the respiratory effect of a given muscle can be evaluated simply by measuring the mass of the muscle and its fractional change in length during passive inflation.

The present studies were undertaken on the basis of this principle to evaluate the respiratory effects of the human parasternal intercostal and triangularis sterni muscles. Previous studies in dogs have demonstrated that the fractional changes in length of these muscles during passive inflation are determined by the orientations of the muscle fibres and costal cartilages relative to the sternum and by the rotation of the cartilages during passive inflation (De Troyer et al. 1996; De Troyer & Legrand, 1998). Therefore, we have first studied human cadavers to measure the angles between the costal cartilages and the sternum and between the muscle fibres and the sternum, and to determine the relation between these two angles at end-expiratory lung volume; the mass of the muscles was also measured. We subsequently measured the rotation of the costal cartilages during passive inflation in a group of healthy individuals. By combining the two series of measurements, we then calculated the fractional changes in length of the muscles in the different interspaces and computed their respiratory effects.

METHODS

Model

Figure 1 shows a diagram of the parasternal region of the human rib cage (third interspace) and emphasizes the determinants of the fractional changes in length of the parasternal intercostal muscles during passive inflation. As shown in our previous investigation in dogs (De Troyer et al. 1996), the fractional change in length of this muscle in a given interspace (ΔL/L1) is related to the orientation of the muscle fibres relative to the sternum at end-expiration (angle β), the orientation of the costal cartilage relative to the sternum at end-expiration (angle α1), and the orientation of the cartilage after passive inflation (angle α2) such that:

graphic file with name tjp0513-0915-m2.jpg (2)

Figure 1. Model of the geometry of the parasternal region of the human rib cage.

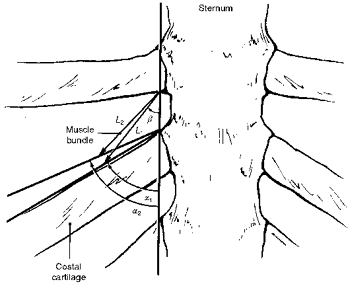
Figure 1

Open in a new tab

Parasternal intercostal muscle length is denoted L, and the angles between the sternum and the costal cartilage and between the sternum and the muscle fibres are denoted α and β, respectively. Subscripts 1 and 2 denote muscle length and angles before and after, respectively, passive inflation.

These three factors determine the fractional changes in length of the triangularis sterni muscle as well (De Troyer & Legrand, 1998), and these changes, therefore, can also be computed by using eqn (2) (Fig. 2). Indeed, since the factor (cosα2 - cosα1) in this equation is negative, the sign of ΔL/L1 only depends on the sign of sin(α1 - β), being negative (muscle shortening) when α1 > β and positive (muscle lengthening) when α1 < β.

Figure 2. Geometry of the triangularis sterni and determinants of muscle length.

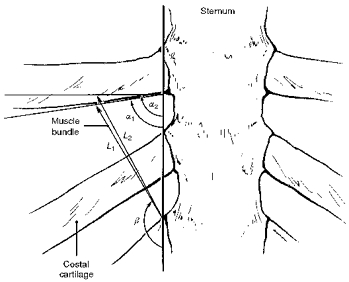
Figure 2

Open in a new tab

Same conventions as in Fig. 1.

Experiment 1

To assess the orientations of the parasternal intercostal and triangularis sterni muscles relative to the sternum at end-expiration, we examined the parasternal region of the rib cage in six fresh human cadavers. Although their medical records and identities could not be disclosed, these cadavers were selected from the pool of human bodies in the Department of Anatomy of the Brussels School of Medicine on the basis of three criteria: absence of overt malnutrition, absence of obesity or other thoracic deformity, and absence of macroscopic pulmonary emphysema at autopsy. The six subjects thus retained for the study were elderly people (> 65–70 years).

With the subjects lying on their backs, the ventral aspect of the rib cage was exposed bilaterally by reflection of the skin and the pectoralis muscles. In five of the six subjects, the costal cartilage of the sixth rib articulated with the cranial border of the cartilage below over its lateral half, and the medial half of the interspace in between did not contain any parasternal intercostal muscle. In addition, the parasternal intercostal fibres in the first interspace were commonly mixed with muscle bundles radiating from the sternal head of the clavicle and the first costal cartilage, such that the insertions of the parasternal intercostals could be readily defined only in interspaces two to five. In each interspace, the acute angle between the sternum and the direction of the muscle fibres (angle β) was then measured bilaterally by aligning the lower edge of a protractor with the sternum. Whereas this angle in the dog increases markedly and continuously from the sternum to the chondrocostal junction (De Troyer & Legrand, 1995), β in the cadavers was found to be constant over the medial three-quarters of the muscle. A moderate increase appeared only in the lateral quarter. This moderate, lateral change in β would not have made any significant difference to the computed pressure-generating ability of the parasternal intercostals overall (see Results), and it was, therefore, disregarded.

After the orientation of the parasternal intercostals was determined, the acute angle between the sternum and the costal cartilage of ribs two to six (angle α1) was measured, after which the parasternal intercostal muscles on both sides of the sternum were sectioned along their caudal insertions. The obtuse angle between the sternum and the direction of the triangularis sterni fibres thus exposed was then measured in interspaces two to six. All these measurements were made in triplicate, and in each individual subject, the measurements obtained in a given interspace on the right and left sides of the sternum were averaged. The sternum and costal cartilages were finally removed en bloc, and the two muscles were harvested bilaterally and weighed.

Data of angles and muscle mass were averaged for the subject group, and they are presented as means ±s.d. Comparison between the different interspaces was made by analysis of variance (ANOVA) with repeated measures, and multiple comparison testing of the mean values was performed, when appropriate, using Student- Newman-Keuls tests. The criterion for statistical significance was taken as P < 0.05.

Experiment 2

The rotation of the costal cartilages during passive inflation was assessed in seven healthy subjects of 29–45 years of age. The subjects were respiratory physicians and gave informed consent to the procedures, as approved by the Ethics Committee of the Brussels School of Medicine. They were all non-smokers and had normal pulmonary function tests, and their principal anthropometric characteristics and supine inspiratory capacity are listed in Table 1. Three subjects (1 to 3) had previously been subjects in many respiratory experiments and were highly trained in relaxing their respiratory muscles at different lung volumes, but four subjects (4 to 7) had little or no prior experience as respiratory subjects. Before the study, these subjects therefore underwent several practice sessions with pairs of respiratory magnetometers (Norman H. Peterson, Boston, MA, USA) placed on the abdomen and rib cage, during which they were coached to relax their respiratory muscles. At the time of the study, all subjects were consequently able to produce consistent relaxation curves of the chest wall from resting end-expiration (functional residual capacity - FRC) to total lung capacity (TLC).

Table 1.

Characteristics of the healthy subjects

Subject Sex Age (years) Height (cm) Weight (kg) Inspiratory capacity (l)
1 M 40 177 65 3.75
2 M 40 173 67 4.95
3 F 45 150 41 2.10
4 M 31 182 74 3.65
5 M 29 173 61 3.40
6 M 35 169 60 4.20
7 F 33 172 65 3.10
Open in a new tab

On the day of the study, the subject was placed supine in a computed tomographic (CT) scanner (Somaton Plus 4A, Siemens Medical Systems, Erlangen, Germany), and all restrictive garments were removed. Once positioned, the subject hyperventilated for 10–20 s and held his or her breath at FRC for 25 s, at which time a spiral data acquisition starting at the cranial border of the manubrium sterni was performed. Preliminary experiments showed that the following scanning parameters allowed precise display of the sternum and costal cartilages: 140 kV, 206 mA, 1.0 s per revolution scanning time, 3 mm collimation, and 4 mm per revolution. However, the volume swept by this acquisition covered only the cranial 10 cm of the sternum. A second acquisition starting at the caudal margin of the first acquisition and extending to the caudal portion of the rib cage was therefore performed. After this procedure was completed, the subject breathed in up to TLC, closed the glottis, and relaxed the respiratory muscles. A second set of spiral data acquisition was then performed.

Transverse CT scans at FRC and TLC were reconstructed at 2.5 mm intervals by using a 360 deg linear-interpolation algorithm and a standard kernel. A total of seventy-four successive transverse images were therefore obtained at each lung volume, and three-dimensional reformations of the thorax were then generated with the threshold (113 Hounsfield units, HU) appropriate for displaying the bony and cartilaginous structures. Anteroposterior images tangent to the sternum and costal cartilages were finally obtained and photographed (window width, 3000 HU; window level, 1100 HU), as shown in Fig. 3, and on each image, the acute angles between the sternum and the direction of the costal cartilages of ribs two to six were measured on both sides of the sternum. The two values thus obtained for each cartilage at FRC (angle α1) and TLC (angle α2) were averaged in each subject, and their statistical analysis was made with the technique described in ‘Experiment 1’.

Figure 3. CT scan image of the sternum and costal cartilages.

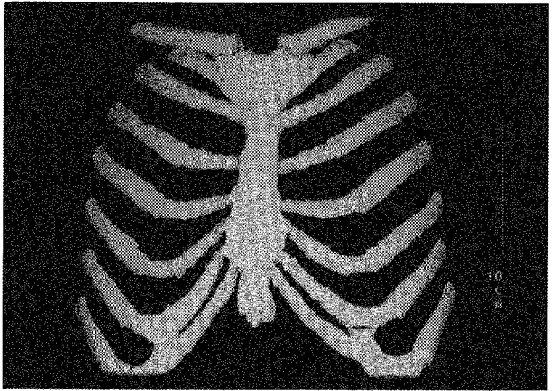
Figure 3

Open in a new tab

This image was obtained in a healthy subject during relaxation at TLC.

RESULTS

Muscle and cartilage orientation in cadavers

The angles between the sternum and the fibres of the parasternal intercostal and triangularis sterni muscles (angle β) in the six cadavers studied are shown in Fig. 4A. The acute angle between the sternum and the parasternal fibres decreased gradually from 45.1 ± 6.9 deg in the second interspace to 25.9 ± 8.0 deg in the fifth interspace (P < 0.001), and the obtuse angle between the sternum and the triangularis sterni fibres decreased from 151.9 ± 7.9 deg in the second interspace to 97.0 ± 5.5 deg in the sixth (P < 0.001). As shown in Fig. 4B, the acute angle between the sternum and the costal cartilage (angle α) also decreased continuously and markedly from the second (100.2 ± 6.3 deg) to the sixth (50.9 ± 5.6 deg) rib (P < 0.001).

Figure 4. Orientations of the parasternal intercostals, triangularis sterni, and costal cartilages in cadavers.

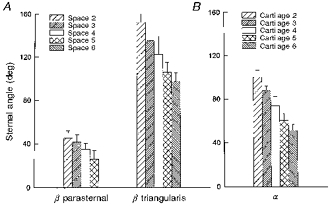
Figure 4

Open in a new tab

The values shown in A are the acute angles between the sternum and the parasternal intercostal fibres (β parasternal) in interspaces 2–5 and the obtuse angles between the sternum and the triangularis sterni fibres (β triangularis) in interspaces 2–6. The values shown in B are the acute angles (α) between the sternum and the costal cartilages of ribs 2–6. Data are mean values ±s.d. obtained from six subjects.

The values of angle β thus measured for the parasternal intercostal and triangularis sterni muscles in the different interspaces were closely related to those of angle α, as shown in Fig. 5. For the parasternal intercostals, β in each interspace was about equal to α/2, such that the linear relationship for the four interspaces studied was β= 2.6 deg + 0.50α (r= 0.960; P < 0.001). The corresponding relationship for the triangularis sterni in interspaces two to six was β= 40.3 deg + 1.10α (r= 0.998; P < 0.001).

Figure 5. Relationships between the orientations of the costal cartilages (angle α) and those of the parasternal intercostals and triangularis sterni (angle β).

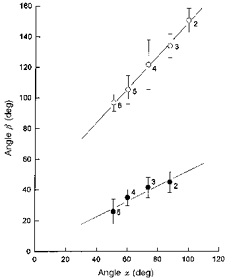
Figure 5

Open in a new tab

Values for the parasternal intercostals (•) and triangularis sterni (^) are those shown in Fig. 4 (the s.d. bars for angle α have been omitted for the sake of clarity), and the numbers next to the circles indicate the interspace number. The continuous lines indicate the linear relationships between the angle α and the angle β parasternal and between the angle α and the angle β triangularis.

Muscle mass

Figure 6 shows the values of parasternal intercostal and triangularis sterni muscle mass in the different interspaces. For the six subjects, bilateral parasternal muscle mass in interspaces two to four was about 5.0 g, whereas in interspace five, it was only 3.2 g (P < 0.01). Triangularis sterni muscle mass was smaller than parasternal muscle mass and increased gradually (P= 0.001) from 1 g in the second interspace to 3 g in the fifth interspace.

Figure 6. Mass of the parasternal intercostal and triangularis sterni muscles.

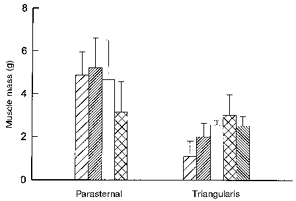
Figure 6

Open in a new tab

Data are mean values ±s.d. obtained from six cadavers on both sides of the sternum. Same conventions as in Fig. 4.

Cartilage orientation and rotation in healthy subjects

The angles measured between the sternum and the costal cartilages of ribs two to six at FRC and TLC in the seven healthy subjects are shown in Fig. 7. As in cadavers, this angle at FRC (angle α1) decreased continuously and markedly from the second (94.9 ± 2.5 deg) to the sixth (34.6 ± 6.7 deg) rib (P < 0.001). This overall distribution persisted with passive inflation to TLC, although all cartilages had rotated by 7.0–9.4 deg. However, the angles were smaller than those measured in cadavers, in particular for the cartilages of ribs five and six. It is likely, therefore, that in a given interspace, the angle between the sternum and the parasternal intercostal muscle in these healthy subjects was smaller than in the cadavers, whereas the angle between the sternum and the triangularis sterni muscle was larger.

Figure 7. Orientations of the costal cartilages in healthy subjects.

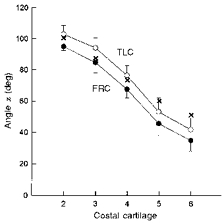
Figure 7

Open in a new tab

The circles are the angles (α) between the sternum and the costal cartilages of ribs 2–6 at FRC (•) and TLC (^). Data are mean values ±s.d. obtained from seven healthy subjects in the supine posture. The crosses are the mean values obtained in cadavers (Fig. 4B) and are shown for comparison.

Computed changes in muscle length

The mean values of β for the parasternal intercostal and triangularis sterni muscles in healthy subjects were computed by using the mean values of α1 measured in these subjects (Fig. 7) and the relationships between α and β obtained in cadavers (Fig. 5). Then, by substituting into eqn (2) the calculated values of β and the measured values of α1 and α2, we computed the mean fractional changes in length of both muscles during passive inflation from FRC to TLC.

The values thus computed are shown in Fig. 8. With passive inflation from FRC to TLC, the parasternal intercostal muscles shortened in all interspaces and the triangularis sterni lengthened. In addition, the two muscles showed clear-cut rostrocaudal gradients. Specifically, the fractional shortening of the parasternal intercostal muscles decreased gradually from 7.7% in the second interspace to only 2.0% in the fifth interspace, whereas the fractional lengthening of the triangularis sterni increased from 5.9% in the second interspace to 13.8% in the sixth.

Figure 8. Computed fractional changes in muscle length.

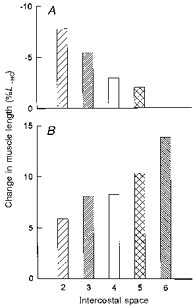
Figure 8

Open in a new tab

A, computed fractional changes in length of the parasternal intercostal muscles in interspaces 2–5 during passive inflation from FRC to TLC. B, computed fractional changes in length of the triangularis sterni muscle in interspaces 2–6 during the same inflation. The changes in muscle length are expressed as percentage changes relative to the muscle length at FRC (LFRC); negative changes in length represent muscle shortening, and positive changes in length represent muscle lengthening. Same conventions as in Fig. 4.

Computed mechanical advantages and respiratory effects

For a machine, such as a lever, ‘mechanical advantage’ is defined as the ratio of the force delivered at the load to the force applied at the handle. By analogy, the mechanical advantage of a respiratory muscle may therefore be defined as ΔPao/mσ which, according to eqn (1), can be evaluated by measuring [ΔL/(LΔVL)]Rel (Wilson & De Troyer, 1992; 1993). The mechanical advantages of the parasternal intercostal and triangularis sterni could therefore be obtained by dividing the mean fractional changes in muscle length shown in Fig. 8 by the mean inspiratory capacity (i.e. 3.59 ± 0.89 l). We then multiplied these values of mechanical advantage by the values of muscle mass measured in cadavers; assuming that σ for these muscles in humans is similar to the dog (i.e. ∼3.0 kg cm−2), we finally calculated the change in Pao that each muscle would produce during isolated, maximal contraction.

The results of these computations are summarized in Fig. 9. As anticipated from the data shown in Fig. 8, the inspiratory mechanical advantage of the parasternal intercostal muscles decreased progressively from 2.16% l−1 in the second interspace to 0.56% l−1 in the fifth interspace. Since muscle mass in the different interspaces was about the same, the respiratory effect of the muscle also decreased gradually from −0.31 cmH2O in the second interspace to only −0.05 cmH2O in the fifth. In contrast, the expiratory mechanical advantage of the triangularis sterni increased from 1.63% l−1 in the second interspace to 3.85% l−1 in the sixth, and the respiratory effect of the muscle increased from +0.05 to +0.29 cmH2O.

Figure 9. Computed mechanical advantages and respiratory effects.

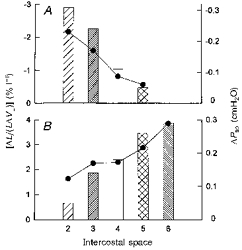
Figure 9

Open in a new tab

A, calculated mechanical advantages (•) and computed changes in airway pressure (ΔPao, bars) of the parasternal intercostal muscles in interspaces 2–5. B, mechanical advantages (•) and ΔPao (bars) of the triangularis sterni muscle in interspaces 2–6. The mechanical advantages were calculated by dividing the fractional changes in muscle length (ΔL/L) by the inspiratory capacity, and are expressed as percentage changes per litre; a negative mechanical advantage indicates an inspiratory effect, while a positive mechanical advantage indicates an expiratory effect.

DISCUSSION

When we initiated the present investigation, we attempted to measure in the same subjects the three determinants of the fractional changes in length of the parasternal intercostal and triangularis sterni muscles during passive inflation of the lungs, as outlined in the model shown in Figs 1 and 2. A number of high-resolution ultrasound linear probes (Acuson 128 Computed Sonography system, Acuson, Mountain View, CA, USA) were thus placed in the parasternal region of the rib cage in healthy subjects. The sternum, costal cartilages and parasternal intercostal muscles were clearly visualized, but the orientations of the muscle fibres could not be estimated. The triangularis sterni was not even identified, and so we elected to expose the parasternal region in fresh cadavers. The orientations of both muscle fibres relative to the sternum could therefore be measured precisely. On the other hand, the intercostal muscles thus exposed had some degree of rigor mortis, and the effect of this alteration on the orientation of the costal cartilages is rather difficult to predict. In addition, the cadavers were significantly older than our healthy subjects, and it is well known that lung recoil pressure in man decreases with increasing age (Turner et al. 1968; Gibson et al. 1976). The result of this loss in lung recoil pressure is an increase in FRC, and indeed the acute angles between the sternum and the costal cartilages were greater in cadavers than in the healthy subjects at end-expiration (Fig. 7). Consequently, the angles measured in cadavers between the sternum and the orientations of the muscle fibres could not be directly introduced into eqn (2) to compute the fractional changes in muscle length in healthy subjects. However, the orientations of the parasternal intercostal and triangularis sterni muscles in the different interspaces were closely related to the orientations of the costal cartilages (Fig. 5), and there is no reason to believe that these relationships would be altered by rigor mortis or age. The orientations of the muscle fibres in healthy subjects could therefore be estimated with reasonable accuracy.

Electromyographic recordings from intercostal muscles in normal humans have previously established that the parasternal intercostals are invariably active during the inspiratory phase of the breathing cycle, including when the increase in lung volume is very small (Taylor, 1960; De Troyer & Sampson, 1982; Whitelaw & Feroah, 1989). These recordings have also shown that the triangularis sterni in supine humans is usually inactive during resting breathing but always contracts during expiratory efforts (De Troyer et al. 1987). It was anticipated, therefore, that inflation of the relaxed chest wall would lead to a shortening of the parasternal intercostals and a lengthening of the triangularis sterni. As shown in Fig. 8, the results of the current studies have fully confirmed this prediction.

Although passive inflation did induce shortening of the parasternal intercostals and lengthening of the triangularis sterni in all interspaces, the magnitude of the changes in muscle length varied from the second interspace caudally. Specifically, the fractional shortening of the parasternal intercostals decreased, whereas the fractional lengthening of the triangularis sterni concomitantly increased. The mechanism of these opposite rostrocaudal gradients is primarily related to the orientation of the costal cartilages, as illustrated in Fig. 10. In this figure, the fractional changes in muscle length during passive inflation, calculated from eqn (2) for the mean values of α1 and α2 measured for the cartilage of the third rib in healthy subjects, are plotted as a function of β (filled circles). Since α1 for this cartilage is 85 deg, passive inflation would cause shortening (negative ΔL/L1) of all muscle fibres with β < 85 deg and lengthening (positive ΔL/L1) of all muscle fibres with β > 85 deg. Furthermore, the curve is nearly antisymmetric about β= 85 deg, and the magnitude of both maximum fractional shortening and lengthening is about 8%. These values are very close, respectively, to the parasternal intercostal shortening computed for the second interspace and the triangularis sterni lengthening calculated for the third interspace. Figure 10 also shows the fractional changes in muscle length calculated for the mean values of α1 and α2 obtained for the cartilage of the sixth rib (open circles). The angle α1 for this cartilage was 35 deg, and so muscle fibres with β= 35 deg would not show any change in length. More importantly, the curve calculated for these values is clearly asymmetric, such that the maximum fractional shortening and lengthening are 2 and 19%, respectively. In other words, the parasternal intercostal in the second interspace shortens by about as much as the triangularis sterni in the third interspace lengthens because the cartilage of the third rib extends out from the sternum at about a right angle; and the shortening of the parasternal intercostal and lengthening of the triangularis sterni muscles become gradually smaller and greater, respectively, as one moves down the rib cage because the costal cartilages slant more and more caudally from the sternum.

Figure 10. Mechanism of rostrocaudal gradients of parasternal intercostal shortening and triangularis sterni lengthening.

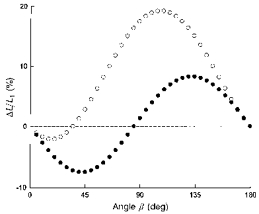
Figure 10

Open in a new tab

The two curves are relationships between the fractional changes in muscle length during passive inflation (ΔL/L1) and the angle β. The curve represented by the filled circles was calculated from eqn (2) for the mean values of α1 (85 deg) and α2 (94 deg) measured for the costal cartilage of the third rib in healthy subjects, whereas the curve represented by the open circles was calculated for the mean values of α1 (35 deg) and α2 (42 deg) measured for the costal cartilage of the sixth rib. Because the former cartilage meets the sternum at about a right angle, the potential shortening of a muscle that attaches to the cartilage from above (parasternal intercostal) is about equal to the potential lengthening of a muscle that attaches to the cartilage from below (triangularis sterni). On the other hand, because the cartilage of the sixth rib slopes caudally from the sternum, the potential muscle lengthening is larger than the potential muscle shortening.

The length response of the parasternal intercostal and triangularis sterni muscles to passive inflation in man is thus, by and large, similar to that previously demonstrated in the dog (De Troyer et al. 1996; De Troyer & Legrand, 1998), yet there are quantitative differences between the two species. To make this comparison, the size of the subjects must be taken into account. Indeed, one would expect that in any particular species, the fractional change in length of a given respiratory muscle over the inspiratory capacity would be independent of the subject's size. Consequently, the fractional change in muscle length per unit increase in lung volume (i.e. the mechanical advantage of the muscle) would be inversely proportional to inspiratory capacity. To the extent that muscle mass is proportional to body mass, values of ΔPao during muscle contraction (eqn (1)) would therefore be independent of body mass.

The dogs studied in our previous investigations (De Troyer et al. 1996) had body masses between 15 and 25 kg and an inspiratory capacity of 1–1.5 l, and with a 1 l passive inflation, the parasternal intercostals in the second and fifth interspace shortened by 10.0 and 7.0%, respectively. In contrast, when the subjects of this study inspired from FRC to TLC and increased lung volume by 3.6 l, the muscles in the second and fifth interspace shortened, respectively, by 7.7 and 2.0%. Similarly, whereas the triangularis sterni in similar dogs lengthened by 18% with a 1 l passive inflation (De Troyer & Legrand, 1998), the triangularis sterni in our subjects lengthened by 8 to 13% over the inspiratory capacity. Thus the fractional changes in length of both muscles are smaller in man than in the dog, and indeed measurements of rib displacement during passive inflation from FRC to TLC have previously shown that the bucket handle component of rib rotation is smaller in man (Wilson et al. 1987; Margulies et al. 1989). Furthermore, in the dog the cranial displacement of the chondrocostal junction during passive inflation is substantially greater than that of the sternum (De Troyer et al. 1996), and this difference leads to an additional rotation of the costal cartilages. In humans, therefore, the rotation of the costal cartilages over the inspiratory capacity is smaller than in the dog, and so the mechanical advantages of the parasternal intercostal and triangularis sterni muscles are smaller than anticipated on the basis of inspiratory capacity alone.

The parasternal intercostal and triangularis sterni muscles in man are also different from those of the dog with respect to muscle mass. In our previous studies in dogs with body masses of 15–25 kg, the mass of parasternal intercostal muscle in any given interspace was found to be about 11 g (Legrand et al. 1996; De Troyer et al. 1996), and triangularis sterni muscle mass ranged between 2.2 and 4.5 g (De Troyer & Legrand, 1998). If the mass of these muscles was similarly related to body mass in man, then parasternal intercostal and triangularis sterni muscle mass in 60 kg subjects should be about 35 and 10 g, respectively. Instead, parasternal intercostal muscle mass per interspace did not exceed 5 g, and the largest triangularis sterni muscle mass in the fifth interspace was only 3 g. In addition, whereas in the dog parasternal intercostal muscles are present in all interspaces from the first to the seventh, the cadavers of this study did not have any muscle below the fifth interspace. When related to body mass, total parasternal intercostal and triangularis sterni muscle mass is therefore substantially smaller in man than in the dog, and this difference suggests that the mechanical effect of these muscles on the human respiratory system is also smaller.

The computations in Fig. 9 are consistent with this concept. According to eqn (1), the maximum change in Pao produced by a particular respiratory muscle can be calculated by multiplying mechanical advantage by muscle mass and maximum active stress. In vitro studies on a variety of limb and respiratory muscles from different animal species, including the parasternal intercostals in primates, have shown that for any muscle, maximum active stress is ∼3.0 kg cm−2 (Close, 1972; Farkas, 1991; Tao & Farkas, 1992; Edwards & Faulkner, 1995). A similar value has been found in vivo for the canine parasternal intercostals and triangularis sterni (De Troyer et al. 1996; De Troyer & Legrand, 1998), and so it seemed reasonable to assume that maximum active stress for these muscles in humans is also 3.0 kg cm−2. However, because both the inspiratory mechanical advantage and the mass of the human parasternal intercostals were smaller than in the dog, the computed ΔPao values in the different interspaces ranged between −0.05 and −0.31 cmH2O (Fig. 9); that is, they were about 10 times less negative than the values previously measured during maximal stimulation of the canine parasternal intercostals (De Troyer et al. 1996; Legrand et al. 1996). Similarly, whereas maximal contraction of the canine triangularis sterni in one interspace causes a mean rise in Pao of 1.75 cmH2O (De Troyer & Legrand, 1998), the computed ΔPao values for the human triangularis sterni were only +0.05 to +0.29 cmH2O (Fig. 9). If the pressures generated by these muscles in the different interspaces in man are additive as they are in the dog (Legrand et al. 1998), the maximal ΔPao generated by all the human parasternal intercostals contracting together or by the entire triangularis sterni would therefore amount to only −1 and +1 cmH2O, respectively.

These values might be underestimated for two reasons. First, the Maxwell reciprocity theorem and eqn (1) are obtained by modelling the chest wall as a linear elastic system, whereas, in fact, the chest wall is neither perfectly linear nor perfectly elastic. Because the CT measurements in these studies were designed to minimize the amount of irradiation and yet provide the greatest signal-to-noise ratio in the assessment of cartilage rotation during passive inflation, no image was obtained between FRC and TLC. It is most likely, however, that the relationships between lung volume and parasternal intercostal or triangularis sterni muscle length are curvilinear such that for a given volume increase, the changes in muscle length are larger near FRC than near TLC. Consequently, the computed mechanical advantages of the two muscles were probably underestimated. Secondly, even though the cadavers in this study were carefully selected and did not show any evidence of overt undernutrition, their parasternal intercostal and triangularis sterni muscles may have been somewhat atrophied or damaged. However, if one assumes that the fractional changes in muscle length at lower lung volumes are 1.5 times greater than those herein computed and that muscle mass in young healthy individuals is 2 times greater than in cadavers, the computed value for the maximal ΔPao generated by the human parasternal intercostals or triangularis sterni muscles contracting in all interspaces simultaneously would still be only −3 and +3 cmH2O, respectively.

Because of the inaccessibility of the parasternal intercostal and triangularis sterni muscles in man, the pressure-generating ability of these muscles has never been measured. This ability has never been estimated either, and so there are no data with which the present results can be compared. However, previous evaluations of subjects with complete, bilateral paralysis of the diaphragm have reported that such subjects generate ΔPao values between −9 and −40 cmH2O during maximum static inspiratory efforts (Kreitzer et al. 1978; Celli et al. 1987; Stradling & Warley, 1988; Laroche et al. 1988). Although the muscles of the neck and the external intercostals are known to be involved, it is conventionally thought that these pressure changes are predominantly contributed by the action of the parasternal intercostals. By showing that these muscles can generate only 2–3 cmH2O, the current studies therefore imply that the pressure-generating ability of the external intercostals, or of the scalenes and sternomastoids, or of both muscle groups is substantially greater than that of the parasternal intercostals.

Acknowledgments

The authors are grateful to Professor M. Rooze for allowing them to study the parasternal region in human cadavers and to the National Heart, Lung and Blood Institute (USA) for its financial support (grant HL-45 545).

References

  1. Celli BR, Rassulo J, Corral R. Ventilatory muscle dysfunction in patients with bilateral idiopathic diaphragmatic paralysis: reversal by intermittent external negative pressure ventilation. American Review of Respiratory Disease. 1987;136:1276–1278. doi: 10.1164/ajrccm/136.5.1276. [DOI] [PubMed] [Google Scholar]
  2. Close RI. Dynamic properties of mammalian skeletal muscles. Physiological Reviews. 1972;52:129–197. doi: 10.1152/physrev.1972.52.1.129. [DOI] [PubMed] [Google Scholar]
  3. De Troyer A, Legrand A. Inhomogeneous activation of the parasternal intercostals during breathing. Journal of Applied Physiology. 1995;79:55–62. doi: 10.1152/jappl.1995.79.1.55. [DOI] [PubMed] [Google Scholar]
  4. De Troyer A, Legrand A. Mechanical advantage of the canine triangularis sterni. Journal of Applied Physiology. 1998;84:562–568. doi: 10.1152/jappl.1998.84.2.562. [DOI] [PubMed] [Google Scholar]
  5. De Troyer A, Legrand A, Wilson TA. Rostrocaudal gradient of mechanical advantage in the parasternal intercostal muscles of the dog. The Journal of Physiology. 1996;495:239–246. doi: 10.1113/jphysiol.1996.sp021588. [DOI] [PMC free article] [PubMed] [Google Scholar]
  6. De Troyer A, Ninane V, Gilmartin JJ, Lemerre C, Estenne M. Triangularis sterni muscle use in supine humans. Journal of Applied Physiology. 1987;62:919–925. doi: 10.1152/jappl.1987.62.3.919. [DOI] [PubMed] [Google Scholar]
  7. De Troyer A, Sampson MG. Activation of the parasternal intercostals during breathing efforts in human subjects. Journal of Applied Physiology. 1982;52:524–529. doi: 10.1152/jappl.1982.52.3.524. [DOI] [PubMed] [Google Scholar]
  8. Edwards RHT, Faulkner JA. Structure and function of the respiratory muscles. In: Roussos C, editor. The Thorax. 2. New York, USA: Marcel Dekker; 1995. pp. 185–217. [Google Scholar]
  9. Farkas GA. Mechanical properties of respiratory muscles in primates. Respiration Physiology. 1991;86:41–50. doi: 10.1016/0034-5687(91)90038-k. 10.1016/0034-5687(91)90038-K. [DOI] [PubMed] [Google Scholar]
  10. Gibson GJ, Pride NB, O'Cain C, Quagliato R. Sex and age differences in pulmonary mechanics in normal nonsmoking subjects. Journal of Applied Physiology. 1976;41:20–25. doi: 10.1152/jappl.1976.41.1.20. [DOI] [PubMed] [Google Scholar]
  11. Kreitzer SM, Feldman NT, Saunders NA, Ingram RH., Jr Bilateral diaphragmatic paralysis with hypercapnic respiratory failure. American Journal of Medicine. 1978;65:89–95. doi: 10.1016/0002-9343(78)90697-6. 10.1016/0002-9343(78)90697-6. [DOI] [PubMed] [Google Scholar]
  12. Laroche CM, Carroll N, Moxham J, Green M. Clinical significance of severe isolated diaphragm weakness. American Review of Respiratory Disease. 1988;138:862–866. doi: 10.1164/ajrccm/138.4.862. [DOI] [PubMed] [Google Scholar]
  13. Legrand A, Ninane V, De Troyer A. Mechanical advantage of sternomastoid and scalene muscles in dogs. Journal of Applied Physiology. 1997;82:1517–1522. doi: 10.1152/jappl.1997.82.5.1517. [DOI] [PubMed] [Google Scholar]
  14. Legrand A, Wilson TA, De Troyer A. Mediolateral gradient of mechanical advantage in the canine parasternal intercostals. Journal of Applied Physiology. 1996;80:2097–2101. doi: 10.1152/jappl.1996.80.6.2097. 10.1063/1.363102. [DOI] [PubMed] [Google Scholar]
  15. Legrand A, Wilson TA, De Troyer A. Rib cage muscle interaction in airway pressure generation. Journal of Applied Physiology. 1998;85:198–203. doi: 10.1152/jappl.1998.85.1.198. [DOI] [PubMed] [Google Scholar]
  16. Margulies SS, Rodarte JR, Hoffman EA. Geometry and kinematics of dog ribs. Journal of Applied Physiology. 1989;67:707–712. doi: 10.1152/jappl.1989.67.2.707. [DOI] [PubMed] [Google Scholar]
  17. Stradling JR, Warley ARH. Bilateral diaphragm paralysis and sleep apnea without diurnal respiratory failure. Thorax. 1988;43:75–77. doi: 10.1136/thx.43.1.75. [DOI] [PMC free article] [PubMed] [Google Scholar]
  18. Tao HY, Farkas GA. Predictability of ventilatory muscle optimal length based on excised dimensions. Journal of Applied Physiology. 1992;72:2024–2028. doi: 10.1152/jappl.1992.72.5.2024. [DOI] [PubMed] [Google Scholar]
  19. Taylor A. The contribution of the intercostal muscles to the effort of respiration in man. The Journal of Physiology. 1960;151:390–402. doi: 10.1113/jphysiol.1960.sp006446. [DOI] [PMC free article] [PubMed] [Google Scholar]
  20. Turner JM, Mead J, Wohl ME. Elasticity of human lungs in relation to age. Journal of Applied Physiology. 1968;25:664–671. doi: 10.1152/jappl.1968.25.6.664. [DOI] [PubMed] [Google Scholar]
  21. Whitelaw WA, Feroah T. Patterns of intercostal muscle activity in humans. Journal of Applied Physiology. 1989;67:2087–2094. doi: 10.1152/jappl.1989.67.5.2087. [DOI] [PubMed] [Google Scholar]
  22. Wilson TA, De Troyer A. Effect of respiratory muscle tension on lung volume. Journal of Applied Physiology. 1992;73:2283–2288. doi: 10.1152/jappl.1992.73.6.2283. [DOI] [PubMed] [Google Scholar]
  23. Wilson TA, De Troyer A. Respiratory effect of the intercostal muscles in the dog. Journal of Applied Physiology. 1993;75:2636–2645. doi: 10.1152/jappl.1993.75.6.2636. [DOI] [PubMed] [Google Scholar]
  24. Wilson TA, Rehder K, Krayer S, Hoffman EA, Whitney CG, Rodarte JR. Geometry and respiratory displacement of human ribs. Journal of Applied Physiology. 1987;62:1872–1877. doi: 10.1152/jappl.1987.62.5.1872. [DOI] [PubMed] [Google Scholar]

Articles from The Journal of Physiology are provided here courtesy of The Physiological Society

RESOURCES