Abstract
Although the relationship between exocytosis and calcium is fundamental both to synaptic and nonneuronal secretory function, analysis is problematic because of the temporal and spatial properties of calcium, and the fact that vesicle transport, priming, retrieval, and recycling are coupled. By analyzing the kinetics of sea urchin egg secretory vesicle exocytosis in vitro, the final steps of exocytosis are resolved. These steps are modeled as a three-state system: activated, committed, and fused, where interstate transitions are given by the probabilities that an active fusion complex commits (α) and that a committed fusion complex results in fusion, p. The number of committed complexes per vesicle docking site is Poisson distributed with mean n. Experimentally, p and n increase with increasing calcium, whereas α and the p n ratio remain constant, reducing the kinetic description to only one calcium-dependent, controlling variable, n. On average, the calcium dependence of the maximum rate (Rmax) and the time to reach Rmax (Tpeak) are described by the calcium dependence of n. Thus, the nonlinear relationship between the free calcium concentration and the rate of exocytosis can be explained solely by the calcium dependence of the distribution of fusion complexes at vesicle docking sites.
Keywords: cytoplasmic vesicles, membrane fusion, sea urchins, secretion, neurosecretion
INTRODUCTION
The exquisite physiological regulation of local calcium concentration at the active zone of a presynaptic terminal supports the long-debated hypothesis that synaptic plasticity depends heavily on the modulation of the relationship between calcium concentration and transmitter release. This fundamental relationship is observed in temporally diverse secretory systems including neurons, other secretory cells, and eggs. However, a single analytical description for the action of calcium at the synapse that includes the many sites of modulation and control has yet to be developed. Such a description would be equally valuable in synthesizing and testing the molecular basis of synaptic function, since calcium must interact with ensembles of proteins to effect release, and kinetic parameters need to be assigned to the underlying molecular events. Here, we present an analytical description for the final steps of calcium-triggered exocytosis that relies, on average, only upon the calcium dependence of fusion complexes at vesicle docking sites and may be applicable in describing calcium-regulated exocytosis over the entire temporal range observed from eggs to neurons.
To understand the modulation of calcium-regulated release, it is essential to fully understand the origin of the nonlinear relationship between calcium concentration (external, internal, and local) and calcium-triggered exocytosis (amplitude and rate of response). Dodge and Rahamimoff 1967 postulated that the cooperative action of four calcium ions is involved in transmitter release at the frog neuromuscular junction. This observation was based on the calcium concentration in the external bathing solution. Efforts to relate the external, internal, and local calcium concentrations have focused attention on multiple steps in the exocytotic process that involve calcium. Calcium entry through channels complicates the relationship between internal and external calcium concentrations and, ultimately, the interpretation of power-law dependencies (Llinas et al. 1976, Llinas et al. 1981; Charlton et al. 1982; Augustine et al. 1985; Augustine and Charlton 1986; Schneggenburger et al. 1999). Calcium diffusion and the dynamics and density of channels, and their location relative to the fusion site are predicted to influence the exocytotic response (Chad and Eckert 1984; Simon and Llinas 1985; Zucker and Fogelson 1986; Yamada and Zucker 1992; Bertram et al. 1996; Klingauf and Neher 1997). In fact, direct measurements of free calcium concentrations reveal nonlinear relationships in a variety of calcium-triggered systems including neurosecretory cells and neurons support the existence of power-law dependencies (Zucker et al. 1991; Augustine and Neher 1992; Thomas et al. 1993; Heidelberger et al. 1994; Heinemann et al. 1994; Huang and Neher 1996; Tse et al. 1997; Schneggenburger et al. 1999; Bollman et al. 2000; Schneggenburger and Neher 2000). Models of the exocytotic pathway that incorporate multiple binding reactions at one or more key steps describe many of the kinetic features observed in these preparations (Bittner and Holz 1992; Heinemann et al. 1993, Heinemann et al. 1994; Neher and Zucker 1993; Thomas et al. 1993; von Ruden and Neher 1993). The fraction of vesicles available for release and the calcium dependence and dynamics of this pool are important aspects of these models (von Ruden and Neher 1993; Heinemann et al. 1993, Heinemann et al. 1994; Gillis et al. 1996; Rosenmund and Stevens 1996; Murthy et al. 1997; Stevens and Wesseling 1998; Schneggenburger et al. 1999; Wu and Borst 1999). Overall, the nonlinear relationship between calcium concentration and exocytosis has proven to be a consistent finding whether the calcium is measured as an external calcium concentration, a channel-dependent calcium influx, or an intracellular free calcium concentration.
Preparations that isolate selected steps in the exocytotic pathway reduce the complexity of the overall process, and are useful in developing an analytical description of the secretory process. Cortical vesicle exocytosis in the isolated planar cortex of the sea urchin egg, an example of calcium-triggered exocytosis, is an attractive system for directly studying the relationship between calcium and exocytosis. The final fusion steps of exocytosis are preserved in isolation from kinetically confounding processes, such as the temporal and spatial properties of the calcium signal, vesicle transport and priming, and membrane retrieval and recycling. The magnitude of the free calcium concentration is the single controlling variable determining which fraction of vesicles fuse (Blank et al. 1998a,Blank et al. 1998b). Consequently, the kinetics of exocytosis in the sea urchin cortex reflects the relationship between calcium triggering and the final fusion steps of the exocytotic process.
Previously, we investigated the consequences of having multiple fusion complexes at fusion sites and proposed that the calcium-triggered exocytotic behavior observed in this preparation can be explained by the hypothesis that an increase in the free calcium concentration increases the average number of participating fusion complexes (Vogel et al. 1996). For simplicity, fusion complex activation was assumed to be instantaneous, but this assumption led to limitations regarding the calcium dependence of the fusion probability, and a major difference between the observed and predicted initial time courses. In this paper, the analysis assumes that, upon calcium-dependent activation, the following exists: (1) a probability in time that an active fusion complex enters a committed state; and (2) a probability in time that a committed fusion complex enters a fusion-competent state. Either of these probabilities may be a function of the calcium concentration, but neither is assumed to be a function of either time or vesicle location. The probability of mediating a calcium-triggered fusion event (the fusion efficacy) and the relationship between this probability and the number of fusion complexes has been determined here. The fusion efficacy is linearly correlated with the number of fusion complexes. This correlation supports the hypothesis that, in the absence of other kinetic processes, the calcium dependence of multiple fusion complexes at vesicle docking sites is sufficient to explain the nonlinear relationship between the calcium concentration and the rate of exocytosis. Our analysis will show that Poisson-distributed fusion complexes may be operant in neuronal systems under conditions in which large numbers of vesicles in an ensemble of boutons are released from the most readily releasable pool.
MATERIALS AND METHODS
All materials and methods related to the study of exocytosis in the sea urchin, Strongylocentrotus purpuratus are described in Blank et al. 1998a. Curve fitting was performed using the Levenburg-Marquardt algorithm with Pearson minimization as implemented in Table Curve2D software (Windows v2.0; Jandel Scientific). Fusion curves were fit using the three-parameter kinetic model. Several criteria were established for evaluating goodness of fit upon minimization. These criteria are parameter standard errors, magnitude and distribution of the residuals, and convergence from different initial values in parameter space. The residuals were randomly distributed about zero (zero mean Gaussian) and, typically, showed <1% deviation (Fitted − Observed)/Observed. The mean coefficients of variation for fitting the three parameters n, α, and p were 0.4 ± 0.1%, 1.2 ± 0.2%, and 3.1 ± 0.5% (n = 73, mean ± SEM), respectively. To further test the goodness of fit upon minimization a small sample, resampling analysis was performed. In this procedure, 90% of the data was randomly selected without replacement and the process was repeated 10 times. These 10 datasets were then individually fit for the three parameters, and parameter values and error estimates were calculated from the mean and standard deviation of the 10 values obtained for each parameter. The estimated errors for all parameters were, both from each separate trial and calculated from the 10 trials, smaller than that obtained in the original dataset and strongly argues for a global minimum in the fitting. The results described were obtained from fitting 73 different fusion curves (n = 3, 19, 25, 20, and 6 for pCa 4.12, 4.46, 4.62, 4.84, and 4.98; and n = 10, 11, and 3 for pCa 4.46, 4.62, and 4.84, for single and double challenge experiments, respectively), a subset of the 111 egg preparations studied previously (Blank et al. 1998a). Experiments involving calcium concentrations below threshold, in which fusion did not occur, were not fit (n = 12). Of the 99 fusion curves available for fitting, 26 are not included in this analysis. These fusion curves were not amenable to fitting because the data were too noisy, or corrupted by the transient lifting of the plasma membrane or passage of air bubbles during solution exchange. Derivative curves were calculated from the percent fusion versus time using third order forward differences of data smoothed using a low-pass filter as implemented in SigmaPlot (Windows v2.0; Jandel Scientific) or Savitsky-Golay smoothing (1–5%) as implemented in TableCurve2D software. Absence of a calcium-dependent trend in α and the p n ratio was evaluated using a one-factor analysis of variance (ANOVA) on both the data and a log transformation of the data. Differences between the parameters describing single and double challenge kinetics were evaluated using a two-sample t test done at each calcium level; significance was evaluated at the P = 0.05 level. Population estimates are presented as the mean ± SEM for n experiments. Note, the error (SEM) of the population estimates for the parameters n, α, and p, represents the observed biological variability and is larger than the fitting errors associated with the determination of parameter values from a single experiment.
RESULTS
A Three-parameter Kinetic Analysis of Exocytosis
We have developed a three-parameter kinetic description for calcium-triggered exocytosis by including an explicit transition between a calcium-triggered precursor and a committed state, to address the major incongruity between the observed initial time course of fusion and the initial time course predicted by our previous analysis (Vogel et al. 1996). The active fusion complex was redefined as the calcium-triggered precursor of the committed fusion complex, where the committed fusion complex is defined as the minimal set of components that can cause the fusion of a vesicle with the plasma membrane. Triggered exocytosis of a population of vesicles was treated as a survival process in which the population of vesicles decreases by fusion. Let t equal the time in an arbitrary dimensionless time interval that, for example, could be scaled to 1 s, n is an integer representing the number of committed fusion complexes per docking site, α is the probability per time interval that an active fusion complex becomes a committed fusion complex, and p is the probability per time interval that a committed fusion complex mediates a fusion event between a vesicle and the plasma membrane. The survival of a single vesicle having a time dependent appearance of n committed fusion complexes isSt,n,α,t,p=1−p t nα,t.
In general, n(α, t) = n · L(α, t), where L(α, t) allows for the introduction of a lag phase signifying that the appearance of committed fusion complexes does not occur instantaneously. The lag phase is a characteristic feature of exocytotic kinetics. The deterministic function L(α, t) = [1 − (1 − α)t] was used because it is the simplest function that described the observed transition. If higher temporal resolution data provides evidence for multiple transitions in the lag phase, then a more detailed function describing these transitions would be necessary. Both the appearance of n committed fusion complexes and the disappearance (fusion) of a vesicle have been formulated as Bernoulli trials (Papoulis 1984). The Poisson-weighted sum of individual vesicle survival curves is:
 |
n represents the average number of committed complexes per docking site. Fusion, Ft,n,α,p=1−St,n,α,p. The rate of fusion, Rt,n,α,p is:
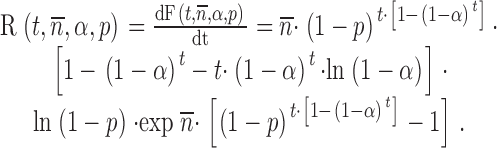 |
As described previously, the extent of fusion is:
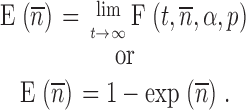 |
In the limit of small α and p (≤ 0.1), (1 − α)t and (1 − p)t approach exp(−αt) and exp(−pt), respectively. In this limit, an alternative expression for the Poisson-weighted sum of individual vesicle survival curves that is continuous in time is:
 |
Now, α and p represent rate constants for the specified transition. For the same transition probability (for example, 0.1) but different time intervals (millisecond and second) the characteristic time constants for the transitions τα = 1/α and τP = 1/p, are 10 ms and 10 s, respectively. Using a discrete time parameter, represented by a sequence of independent trials, is analogous to approximating a one-dimensional random walk using interval transition probabilities to describe movement to the left or right. The discrete and continuous expressions for the Poisson-weighted sum of individual vesicle survival curves describe the kinetics with statistically indistinguishable parameters. Both analytical expressions eliminate the need to fit data to exponential sums, a notoriously difficult analysis requiring data of high precision over a wide range (Istratov and Vyvenko 1999).
Kinetic Analysis of the Calcium Dependence of Exocytosis
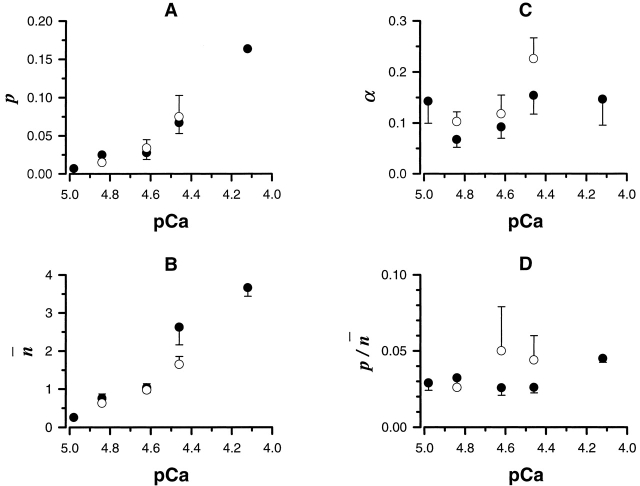
We have used multiple calcium challenges to investigate submaximal exocytotic responses of isolated planar cortices of the sea urchin S. purpuratus at suboptimal calcium concentrations (Blank et al. 1998a,Blank et al. 1998b; Coorssen et al. 1998). In a sequence of two challenges, one to an intermediate level of calcium followed by a second higher concentration (double challenge), the same extent of fusion as a single challenge at the higher concentration was observed (Blank et al. 1998a). Our analysis describes the time course of cortical vesicle exocytosis triggered by calcium using multiple calcium challenges (Fig. 1). The calcium dependence of the model parameters p, n, and α is shown in Fig. 2. There is no statistical difference between the parameters describing either single or double challenge kinetics: this analysis describes both responses equally well (Fig. 1). Note, in the present study, n is estimated from a fitting parameter and not calculated from the extent of fusion. Over the ranges of calcium concentrations studied, the p n ratio and the parameter α were found to be independent of pCa (−log [Ca2+]free) (Fig. 2). Both the p n ratio and the log transformation of p n, log (p n), are independent of pCa with p n = 0.029 ± 0.002 (mean ± SEM, n = 73 experiments; F = 0.514, df = 5, 67, P = 0.765, and F = 0.671, df = 5, 67, P = 0.647, ANOVA). The invariance of α with [Ca2+]free (α = 0.113 ± 0.013 [mean ± SEM], n = 73 experiments; F = 1.722, df = 5, 67, P = 0.141, ANOVA; Fig. 2 C) is consistent with either calcium-independent conversion of activated to committed complexes or calcium-dependent conversion saturating at ∼5 μM calcium. In particular, as neither the ANOVA nor the within subset, two-sample t tests were significant, the hypothesis that no significant trend was detected in either α or the p n ratio for both single and double challenges is supported. The correlation between p and n is consistent with a rate constant proportional to n. Since p represents a bounded quantity in the discrete expression, the invariance of p n with [Ca2+]free (Fig. 2 D) suggests that this is a limiting relationship of the form p n = κ, a characteristic constant. This was tested by fitting all pairs of p and n with p = 1 − exp(−κn), which approaches κn and 1 for small and large values of the exponent, respectively. The value of κ was identical to that obtained by ANOVA of p n with [Ca2+]free. For 0 < n < 0.2, >98% of vesicles have zero or one activated complex, suggesting that at low [Ca2+]free, p has a limiting value whose distribution is presently unknown. Substituting α = 0.113 and p = 0.029 n ignores the distribution of α and κ, but reduces the analysis to one parameter that should, on average, describe the kinetics of fusion. This was tested using a reduced kinetic analysis with only one controlling variable (pCa) derived using the relationship between the extent of fusion and n and the empirical relationship (log-normal cumulative distribution with midpoint M = 18.2 μM and width W = 0.23), which describes the extent of fusion as a function of [Ca2+]free (Blank et al. 1998a):
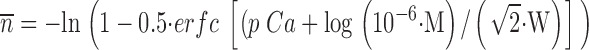 |
Figure 1.
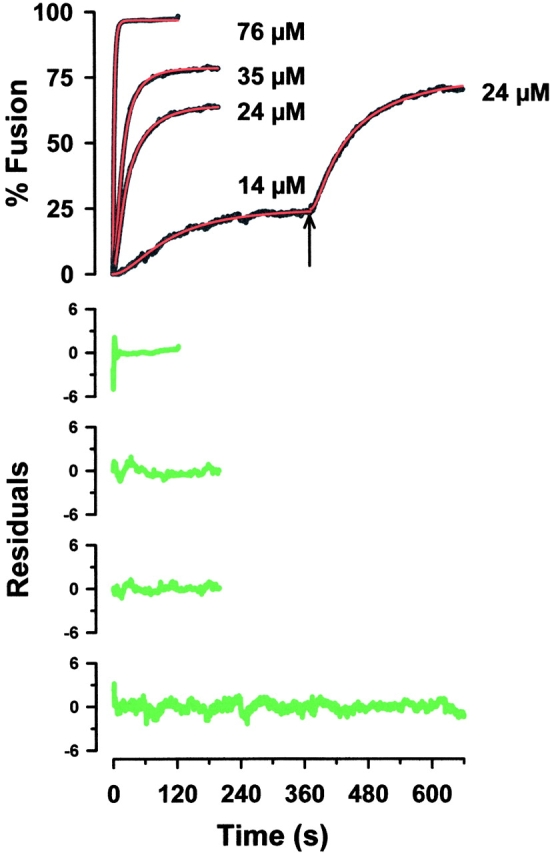
Typical fusion curves resulting from single and double challenge experiments at four different calcium concentrations are represented (14, 24, 35, and 76 μM). The data were collected using a sample time of 0.1 s The second fusion curve of a double challenge experiment was fit piecewise using a modification of the model. A fourth scaling parameter was used to represent the extent of fusion at the time of the second challenge. The data are black, the fitted curves are red, and the difference between the two (residuals) is green.
Figure 2.
Dependence of p, n, α, and p n on calcium concentration (mean ± SEM; A–D). Open symbols represent the fitting results of double challenge experiments using the same final calcium concentration, as indicated by the matching closed symbols, of single challenge experiments.
The maximum rate and the time to reach the maximum rate were calculated as a function of pCa and compared with measured values (Fig. 3). Note this is not a fit to the average data, but rather it is the predicted behavior following reduction to one controlling variable, the calcium concentration. The maximum rate is a quadratic function of n, (Rmax ∼ n a; a = 1.93 ± 0.25); this is exactly the dependence predicted when p is proportional to n. The relationship between the maximum rate and n suggests that n represents the local concentration of fusion complexes. Furthermore, these predicted characteristic relationships strongly support the hypothesis that understanding the properties of the local and stochastic concentration of fusion complexes is important for a full kinetic description of calcium-triggered exocytosis. The nonlinear relationship between the maximum rate of exocytosis and the free calcium concentration can be described by the calcium dependence of n and two characteristic constants of the system, α and κ.
Figure 3.
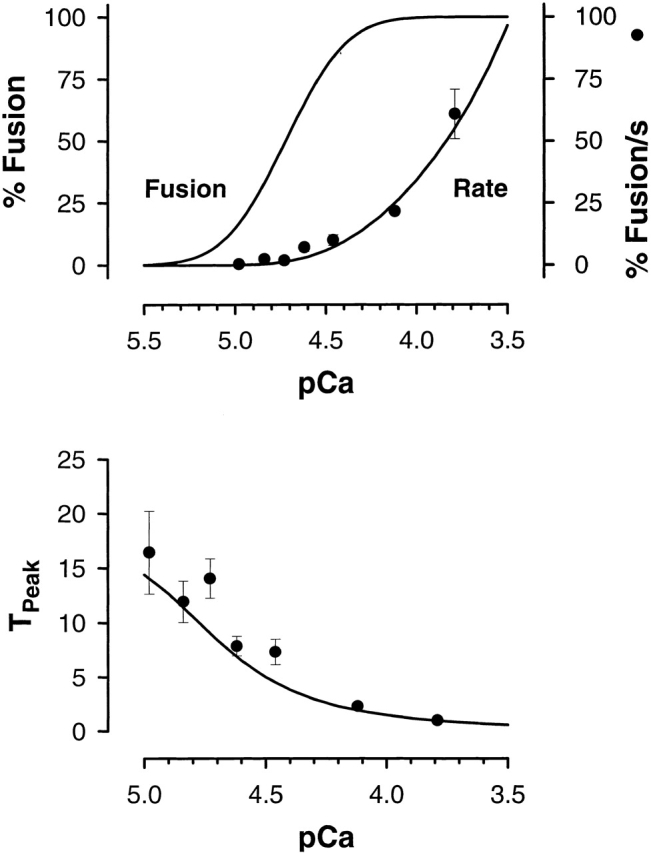
The maximum fusion rate and the time to reach the maximum rate (TPeak) vary with calcium. Using the relationship between the extent of fusion (pCa and n), the experimentally derived constants for α, and the correlation between n and p, specifies the kinetic response as a function of calcium. The solid lines indicate these relationships, in agreement with the observed behavior of both the maximum rate and the time to reach the maximum rate.
Extension of the Analysis to the Millisecond Time Scale
Variations in the model parameters can capture kinetic features observed in both “egglike” and “neuronal-like” calcium-triggered systems (Fig. 4). Calcium concentrations eliciting n ∼ 5 will trigger fusion of >99% of all vesicles present. Changing the time constants from the second to millisecond time scale and decreasing n results in limited depletion of the available vesicle pool with fusion occurring on a millisecond time scale. Both the extent of vesicle release and the maximum rate of release can be approximated by a [Ca2+]4 power-law dependence (Fig. 5A and Fig. B). Calcium-dependent variations in the lag (time to first fusion event) and time to peak rate (Fig. 5 C) are similar to those observed in neurons (Bollman et al. 2000; Schneggenburger and Neher 2000). Poisson-distributed fusion complexes, or reserve capacity (n), coupled with variations in the kinetic time constants and distribution of calcium thresholds is therefore sufficient to explain the wide range in rates and extents of fusion observed in other calcium-triggered exocytotic systems.
Figure 4.
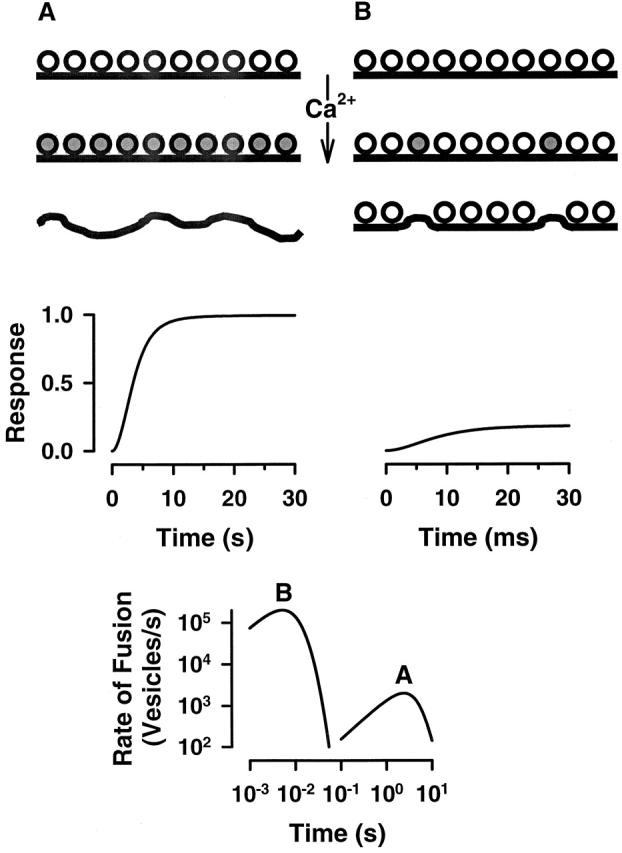
A and B represent the calcium-triggered response in two systems showing egglike and neuronlike kinetic behaviors with parameters n = 5, τα = 10 s, and τP = 7 s, and n = 0.2, τα = 10 ms, and τP = 7 ms, respectively. The rates were calculated using a total releasable pool of 1,000 vesicles. Note, the kinetics of the neuronlike system is ∼100× faster than that of the egglike system.
Figure 5.
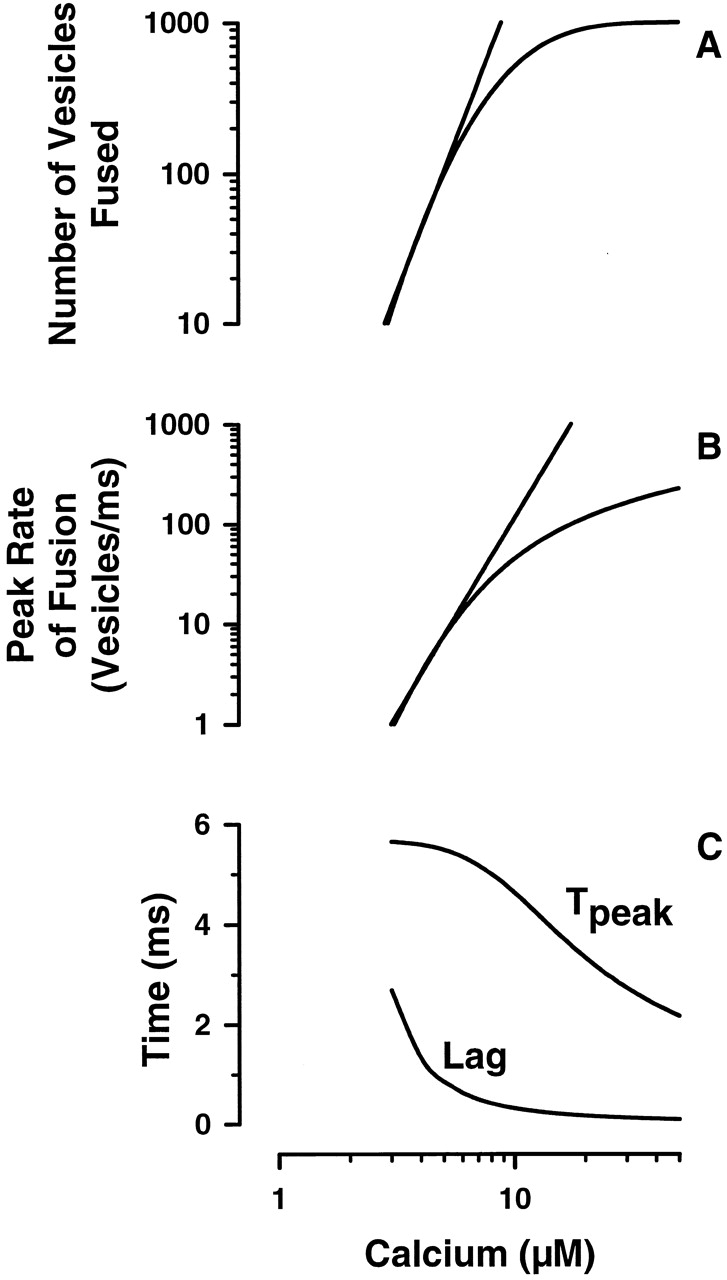
A and B are the log-log representations for the calcium dependence of the extent and maximum fusion rate predicted for the neuronlike system with τα = 10 ms and τP = 7 ms. The n-calcium relationship was established using midpoint M = 10 μM and width W = 0.23 in the cumulative log-normal distribution. The straight lines are nth order fits to the linear portions of the curve (3–7 μM calcium); the extent and maximum rate are approximated by [Ca2+]4.0 and [Ca2+]3.9, respectively. C is the predicted calcium dependence for the time of the first fusion event (Lag) and the time to reach the maximum rate (Tpeak).
DISCUSSION
What factors contribute to the observed nonlinear relationship between exocytosis and calcium concentration? We present an analytical description for the final steps of calcium-triggered exocytosis that relies, on average, only upon the calcium dependence of Poisson-distributed fusion complexes at vesicle docking sites. The calcium dependence of distributed fusion complexes is sufficient to explain the nonlinear relationship observed in sea urchin cortical vesicle exocytosis. This analysis may be applicable in describing experimental features of calcium-triggered exocytosis that are observed in diverse systems covering a wide temporal range.
Kinetic Properties of Exocytosis in Eggs Are Similar to Those of Other Calcium-triggered Systems
How do the rates of exocytosis in neurons and other secretory cells compare with those observed in the egg? An 80-μm-diam S. purpuratus egg has a density of ∼0.9 vesicles/μm2 based on ∼1.8 × 104 vesicles/egg (Schroeder 1979). An 80-μm-diam planar cortex is expected to have ∼4.5 × 103 vesicles, which is in agreement with measured values (Zimmerberg et al. 1985). Although kinetically unresolved in this study, fusion rates, calculated from the initial slope, plateau at ∼200% fusion/s (∼104 vesicles/s) when challenged with saturating calcium concentrations (>1 mM) that activate all fusion complexes. As a function of [Ca2+]free, the maximum rates calculated from the analysis of the resolved fusion kinetics correspond to ∼20–2,700 vesicles/s. In the urchin cortex, such rates are primarily a consequence of the large number of releasable vesicles rather than the underlying kinetic rate constant (less than ∼10 s−1 maximum based on the time to peak rate occurring in 0.1–0.2 s at saturating [Ca2+]free). The releasable pool in other preparations is estimated to be ∼10–1,000 vesicles which, at the highest rates, are released on a ∼1–10-ms time scale (for review see Zucker 1996). Upon fertilization, the intact egg releases ∼104 vesicles at a maximum rate of ∼2,000 vesicles/s (Vogel et al. 1996). Cortical vesicle release rates (events per second) are therefore comparable to rates reported in other calcium-triggered systems such as melanotrophs (Thomas et al. 1993), chromaffin cells (Augustine and Neher 1992; Heinemann et al. 1994; Parsons et al. 1995; Voets et al. 1999), neutrophils (Nusse et al. 1998), and bipolar neurons (von Gersdorff and Matthews 1994; although Heidelberger et al. 1994 report higher rates). However, estimates of the maximal time constant describing the final fusion steps vary over three orders of magnitude depending upon the preparation. For example, in orders of magnitude, the rate limiting time constant in egg is ∼100 ms, in melanotrophs and neutrophils ∼10 ms (Thomas et al. 1993; Nusse et al. 1998), in chromaffin cells ∼1 ms (Heinemann et al. 1994), and in neurons ∼0.1 ms (Heidelberger et al. 1994). Both the transition to the committed state (α) and the coupling between the fusion probability and the number of committed complexes (fusion efficacy [κ]) must be sites of modulation if an underlying conserved mechanism, captured in this analysis, is responsible for the final fusion pathway in such temporally diverse systems.
Are Poisson-distributed Fusion Complexes with Distributed Calcium Thresholds a Conserved Feature of Calcium-triggered Exocytosis?
One major feature of sea urchin cortical vesicle exocytosis is submaximal secretion at suboptimal stimulation, also observed in a wide variety of permeabilized preparations (for reviews see Knight and Scrutton 1986; Knight and Baker 1987). This has been reported more recently in the high Ca2+ affinity granule population of human neutrophils (Nusse et al. 1998), PC12 cells (Kasai et al. 1996), and mast cells (stimulated with compound 48/80; Hide et al. 1993). Neuronal preparations also have been shown to produce submaximal responses to suboptimal stimulation. However, these results are consistent with an underlying kinetic process that scales with the number of vesicles undergoing release, and this number is a function of the effective calcium concentration. In cultured CA1 hippocampal neurons, reducing the release probability 20-fold did not affect the time course of the averaged AMPA receptor EPSC (Diamond and Jahr 1995). In calyces of Held, the time course of the averaged EPSC at low and physiological calcium concentrations is similar, whereas the amplitude depended strongly upon [Ca2+]free (Borst and Sakman 1996). If synaptic vesicles have reserve capacity then the prediction of our analysis is that the calcium concentration under these conditions did not reach levels sufficient to activate more than one complex per synaptic vesicle docking site. This does not imply that multiple complexes do not exist at synaptic vesicle docking sites, but rather that the reserve capacity was not used under these stimulus conditions. For example, in the urchin, the maximum number of activated complexes (n Max) is approximately seven to nine (Vogel et al. 1996; Coorssen et al. 1998), whereas the [Ca2+]free present during fertilization activates only approximately four to five complexes (Vogel et al. 1996). Calcium-triggered systems requiring fast cyclic patterns of release may be designed to work in a regime where only one complex is activated so as to prevent massive depletion of releasable vesicles, and thereby preventing vesicle transport, maturation, and pool refilling from becoming rate limiting. Since the release of synaptic vesicles is part of a cyclic process, comparison with the urchin system requires that the release probability of the most readily releasable pool be considered.
The calcium dependence of this readily releasable pool was determined in calyces of Held where 20% of 700 quanta (vesicles) are released, on average, by a single presynaptic action potential under physiological conditions (Schneggenburger et al. 1999). A releasable pool of ∼20% suggests that when stimulation is low, synaptic transmission operates with n < 0.2; vesicles have only one activated complex. We predict that manipulations decreasing the available calcium decrease n further and results in a kinetic response that normalizes to a common time course in which the number of participating quanta varies with [Ca2+]free. Do synaptic vesicles utilize multiple fusion complexes with distributed calcium thresholds under conditions of higher calcium concentrations then those observed during a single action potential?
A single presynaptic action potential is capable of depleting the readily releasable pool in calyces of Held, provided the external calcium concentration is high enough (Schneggenburger et al. 1999). In the same preparation, rapid photolysis of caged calcium producing a [Ca2+]free of ∼10 μM released ∼100% of the releasable pool while the release rate continued to increase with free calcium concentrations greater than the minimum concentrations required to release ∼100% of the releasable pool (Bollman et al. 2000; Schneggenburger and Neher 2000). The variability in the sensitivity to calcium and the increased rate with manipulations that increase [Ca2+]free are observations consistent with synaptic vesicles using multiple fusion complexes with distributed calcium thresholds. In addition, a decrease in the release probability of recently recruited releasable vesicles was observed in the same preparation (Wu and Borst 1999). Although this decrease in release probability of recently recruited vesicles may be due to vesicle location relative to the calcium channel, these results are consistent also with a population of synaptic vesicles having a decreased sensitivity to calcium. Calcium-dependent modulation of the release probability may occur through both the distribution of sensitivities and the number of complexes activated. However, the neuronal data are also consistent with other kinetic schemes; multiple calcium binding steps predict similar behavior (Heinemann et al. 1993, Heinemann et al. 1994). Although synaptic transmission may operate in the regime where less than one complex, on average, is activated, the system may have the capacity to operate in the regime where multiple complexes are activated. This may represent another site for synaptic modulation. As n increases, corresponding to the appearance of vesicles with two or more activated complexes, additional terms in the kinetics begin to appear and may manifest as poorer fits with a single exponential. The failure to rigorously describe synaptic release kinetics with one scalable process is predicted to increase with increasing concentrations of calcium and may provide evidence for the existence of reserve capacity on synaptic vesicles.
Does n Max Vary in Different Calcium-triggered Systems?
The size of an exocytotic vesicle may limit the maximum average number of complexes n Max present at vesicle docking sites. For example, n Max on synaptic vesicles may be fractional such that the proportion of vesicles with two or more complexes is small. One consequence of n Max = 0.5–0.1 is that only 8% to <1% of the vesicles present will have two or more complexes, and that 61–90% of the vesicles present will be incapable of fusion at any calcium concentration. The pool of inactive vesicles may represent inefficiency in the process of creating the readily releasable/recycling pool and may not be detected using techniques that rely solely on vesicle release. Such a large pool of inactive vesicles is consistent with the observations that, even under strong stimulus conditions, only ∼10% of the total synaptic vesicle population in a terminal participates in neurotransmitter release and recycling (Murthy et al. 1997; Murthy and Stevens 1999). Our analysis remains valid at fractional values of n Max because of the hypothesis that the overall population of complexes is described by an underlying distribution of thresholds defined by the derivative of the calcium activity curve (Blank et al. 1998a,Blank et al. 1998b). In this regime, vesicles either fuse under the action of one committed complex, or they are inactive. An alternative analysis, that fixes the number of complexes on every vesicle at one, would require calcium-dependent rate constants and a threshold function defined by the calcium activity curve to be consistent with the existing data. With α and p representing the described previously rate constants, and Thresh(pCa) describing the normalized calcium activity curve, an alternative formulation for the fusion process is:
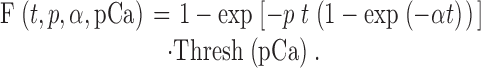 |
Although this expression is not appropriate for the urchin, where reserve capacity is well established (Vogel et al. 1996; Blank et al. 1998a,Blank et al. 1998b; Coorssen et al. 1998), it may be descriptive of systems where the number of complexes is fixed at one and the population of complexes has an underlying distribution of calcium thresholds. In a sequential, homogeneous model based on a single, linear reaction, calcium is postulated to influence the rate of exocytosis via calcium-dependent rate constants; both the number and binding characteristics of the calcium-dependent transitions are explicitly specified. Although these models can be highly parameterized and may not statistically differentiate between the numbers and characteristics of the calcium-binding site, the data appear to be best described with three or four calcium-binding steps (Heidelberger et al. 1994; Heinemann et al. 1994). In the present analysis, the detailed relationship between calcium binding and the conversion of inactive to active fusion complexes is unspecified. However, even in the absence of a specified calcium activation process, the reduced kinetic analysis describes the same dependence on calcium as that approximated by a [Ca2+]4 power function for Rmax and extent of fusion, respectively.
Issues in Identifying the Molecular Basis of Calcium-triggered Exocytosis
Understanding the interactions of calcium with ensembles of proteins is a key step in identifying the molecular basis of calcium-triggered exocytosis. The conserved multigene families of SNARE proteins, VAMP, SNAP-25, and syntaxin, have homologues present in the cortical membranes of sea urchin eggs (Avery et al. 1997; Conner et al. 1997; Tahara et al. 1998). These homologues form the same complexes as those observed in other systems (Coorssen et al. 1998; Tahara et al. 1998). Although the exact roles for these and other proteins have yet to be elucidated, the correspondence and conservation among proteins present from yeast, to urchin, to man suggests a conserved pathway for exocytosis; specific steps in this pathway can be directly probed in sea urchin egg preparations (Vogel et al. 1996; Blank et al. 1998a,Blank et al. 1998b; Coorssen et al. 1998; Zimmerberg et al. 1999, Zimmerberg et al. 2000). It may be premature to couple kinetic properties to identified proteins without knowing the relationship between calcium, triggering, and fusion. The kinetic coupling between multiple identified and unknown steps in cyclic processes can result in the incorrect association of a protein or ensemble of proteins with specific kinetic transitions. The stage specific preparations of the sea urchin simplify this difficult problem (Zimmerberg et al. 1999, Zimmerberg et al. 2000).
Conclusion
The nonlinear relationship between [Ca2+]free and the rate of exocytosis is a characteristic feature of calcium-triggered secretory systems. The final steps of calcium-triggered vesicle exocytosis are described by an analysis using three parameters: (1) the interval probability that an active fusion complex becomes committed (α); (2) the average number of committed complexes (n); and (3) the interval probability that a committed fusion complex results in fusion (p). This analysis is based on the hypothesis that exocytotic vesicle docking sites have multiple fusion complexes (reserve capacity), and that increasing the free calcium concentration increases the average number of participating fusion complexes. In the sea urchin planar cortex, the average kinetic behavior of submaximal exocytosis at suboptimal free calcium concentrations, including maximum (peak) rate and time to reach maximum rate, is described by the calcium dependence of the participating fusion complexes. The reserve capacity may be limited in other systems when the rapid release of only a small number of vesicles is required. In contrast, the egg requires that essentially every cortical vesicle fuse after fertilization and utilizes reserve capacity to ensure that this level of calcium-triggered exocytosis occurs.
Acknowledgments
We thank Drs. Jens R. Coorssen and Nevin A. Lambert for stimulating discussions and critically reading our manuscript.
S.S. Vogel has received support from the National Institutes of Health grant No. NS41055.
References
- Augustine G.J., Charlton M.P. Calcium dependence of presynaptic calcium current and post-synaptic response at the squid giant synapse. J. Physiol. 1986;381:619–640. doi: 10.1113/jphysiol.1986.sp016347. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Augustine G.J., Neher E. Calcium requirements for secretion in bovine chromaffin cells. J. Physiol. 1992;450:247–271. doi: 10.1113/jphysiol.1992.sp019126. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Augustine G.J., Charlton M.P., Smith S.J. Calcium entry and transmitter release at voltage-clamped nerve terminals of squid. J. Physiol. 1985;367:163–181. doi: 10.1113/jphysiol.1985.sp015819. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Avery J., Hodel A., Whitaker M. In vitro exocytosis in sea urchin eggs requires a synaptobrevin-related protein. J. Cell Sci. 1997;110:1555–1561. doi: 10.1242/jcs.110.14.1555. [DOI] [PubMed] [Google Scholar]
- Bertram R., Sherman A., Stanley E.F. Single-domain/bound calcium hypothesis of transmitter release and facilitation. J. Neurophys. 1996;75:1919–1931. doi: 10.1152/jn.1996.75.5.1919. [DOI] [PubMed] [Google Scholar]
- Bittner M.A., Holz R.W. Kinetic analysis of secretion from permeabilized adrenal chromaffin cells reveals distinct components. J. Biol. Chem. 1992;267:16219–16225. [PubMed] [Google Scholar]
- Blank P.S., Cho M.S., Vogel S.S., Kaplan D., Kang A., Malley J., Zimmerberg J. Submaximal responses in calcium-triggered exocytosis are explained by differences in the calcium sensitivity of individual secretory vesicles J. Gen. Phys. 112 1998. 559 567a [DOI] [PMC free article] [PubMed] [Google Scholar]
- Blank P.S., Vogel S.S., Cho M.S., Kaplan D., Bhuva D., Malley J., Zimmerberg J. The calcium sensitivity of individual secretory vesicles is invariant with the rate of calcium delivery J. Gen. Phys. 112 1998. 569 576b [DOI] [PMC free article] [PubMed] [Google Scholar]
- Bollman J.H., Sakman B., Borst J.G.G. Calcium sensitivity of glutamate release in a calyx-type terminal. Science. 2000;289:953–957. doi: 10.1126/science.289.5481.953. [DOI] [PubMed] [Google Scholar]
- Borst J.G.G., Sakman B. Calcium influx and transmitter release in a fast CNS synapse. Nature. 1996;383:431–434. doi: 10.1038/383431a0. [DOI] [PubMed] [Google Scholar]
- Chad J.E., Eckert R. Calcium domains associated with individual channels can account for anomalous voltage relations of Ca-dependent responses. Biophys. J. 1984;45:993–999. doi: 10.1016/S0006-3495(84)84244-7. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Charlton M.P., Smith S.J., Zucker R.S. Role of presynaptic calcium ions and channels in synaptic facilitation and depression at the squid giant synapse. J. Physiol. 1982;323:173–193. doi: 10.1113/jphysiol.1982.sp014067. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Conner S., Leaf D., Wessel G. Members of the SNARE hypothesis are associated with cortical granule exocytosis in the sea urchin egg. Mol. Reprod. Dev. 1997;48:106–118. doi: 10.1002/(SICI)1098-2795(199709)48:1<106::AID-MRD13>3.0.CO;2-Z. [DOI] [PubMed] [Google Scholar]
- Coorssen J.R., Blank P.S., Tahara M., Zimmerberg J. Biochemical and functional studies of cortical vesicle fusionthe SNARE complex and Ca2+ sensitivity. J. Cell Biol. 1998;143:1845–1857. doi: 10.1083/jcb.143.7.1845. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Diamond J.S., Jahr C.E. Asynchronous release of synaptic vesicles determines the time course of the AMPA receptor-mediated EPSC. Neuron. 1995;15:1097–1107. doi: 10.1016/0896-6273(95)90098-5. [DOI] [PubMed] [Google Scholar]
- Dodge R.A., Rahamimoff R. Co-operative action of calcium ions in transmitter release at the neuromuscular junction. J. Physiol. 1967;193:419–432. doi: 10.1113/jphysiol.1967.sp008367. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Gillis K.D., Mossner R., Neher E. Protein kinase C enhances exocytosis from chromaffin cells by increasing the size of the readily releasable pool of secretory granules. Neuron. 1996;16:1209–1220. doi: 10.1016/s0896-6273(00)80147-6. [DOI] [PubMed] [Google Scholar]
- Heidelberger R., Heinemann C., Neher E., Mathews G. Calcium dependence of the rate of exocytosis in a synaptic terminal. Nature. 1994;371:513–515. doi: 10.1038/371513a0. [DOI] [PubMed] [Google Scholar]
- Heinemann C., von Ruden L., Chow R.H., Neher E. A two-step model of secretion control in neuroendocrine cells. Pflügers Arch. 1993;424:105–112. doi: 10.1007/BF00374600. [DOI] [PubMed] [Google Scholar]
- Heinemann C., Chow R.H., Neher E., Zucker R.S. Kinetics of the secretory response in bovine chromaffin cells following flash photolysis of caged Ca2+ Biophys. J. 1994;67:2546–2557. doi: 10.1016/S0006-3495(94)80744-1. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Hide I., Bennett J.P., Pizzey A., Boonen G., Bar-Sagi D., Gomperts B.D., Tatham P.E.R. Degranulation of individual mast cells in response to Ca2+ and guanine nucleotidesan all-or-none event. J. Cell Biol. 1993;123:585–593. doi: 10.1083/jcb.123.3.585. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Huang L.-Y.M., Neher E. Ca2+-dependent exocytosis in the somata of dorsal root ganglion neurons. Neuron. 1996;17:135–145. doi: 10.1016/s0896-6273(00)80287-1. [DOI] [PubMed] [Google Scholar]
- Istratov A.A., Vyvenko O.F. Exponential analysis in physical phenomena. Rev. Sci. Instr. 1999;70:1233–1257. [Google Scholar]
- Kasai H., Takagi H., Ninomiya Y., Kishimoto T., Ito K., Yoshida A., Yoshioka T., Miyashita Y. Two components of exocytosis and endocytosis in phaeochromocytoma cells studied using caged Ca2+ compounds. J. Physiol. 1996;494:53–65. doi: 10.1113/jphysiol.1996.sp021475. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Klingauf J., Neher E. Modeling buffered Ca2+ diffusion near the membraneimplications for secretion in neuroendocrine cells. Biophys. J. 1997;72:674–690. doi: 10.1016/s0006-3495(97)78704-6. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Knight D.E., Scrutton M.C. Gaining access to the cytosolthe technique and some applications of electropermeabilization. Biochem J. 1986;234:497–506. doi: 10.1042/bj2340497. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Knight D.E., Baker P.F. Exocytosis from the vesicle view-pointan overview. Ann. NY Acad. Sci. 1987;493:504–523. doi: 10.1111/j.1749-6632.1987.tb27237.x. [DOI] [PubMed] [Google Scholar]
- Llinas R., Steinberg I.Z., Walton K. Presynaptic calcium currents and their relation to synaptic transmissionvoltage clamp study in squid giant synapse and theoretical model for the calcium gate. Proc. Natl. Acad. Sci. USA. 1976;73:2913–2922. doi: 10.1073/pnas.73.8.2918. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Llinas R., Steinberg I.Z., Walton K. Relationship between presynaptic calcium current and postsynaptic potential in squid giant synapse. Biophys. J. 1981;33:323–351. doi: 10.1016/S0006-3495(81)84899-0. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Murthy V.N., Stevens C.F. Reversal of synaptic vesicle docking at central synapses. Nat. Neurosci. 1999;2:503–507. doi: 10.1038/9149. [DOI] [PubMed] [Google Scholar]
- Murthy V.N., Sejnowski T.J., Stevens C.F. Heterogeneous release properties of visualized individual hippocampal synapses. Neuron. 1997;18:599–612. doi: 10.1016/s0896-6273(00)80301-3. [DOI] [PubMed] [Google Scholar]
- Neher E., Zucker R.S. Multiple calcium-dependent processes related to secretion in bovine chromaffin cell. Neuron. 1993;10:21–30. doi: 10.1016/0896-6273(93)90238-m. [DOI] [PubMed] [Google Scholar]
- Nusse O., Serrander L., Lew D.P., Krause K.-H. Ca2+-induced exocytosis in individual human neutrophilshigh- and low-affinity granule populations and submaximal responses. EMBO J. 1998;17:1279–1288. doi: 10.1093/emboj/17.5.1279. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Papoulis A. Probability, Random Variables, and Stochastic Processes 1984. McGraw-Hill Inc; New York: pp. 576 pp [Google Scholar]
- Parsons T.D., Coorssen J.R., Horstmann H., Almers W. Docked granules, the exocytic burst, and the need for ATP hydrolysis in endocrine cells. Neuron. 1995;15:1085–1096. doi: 10.1016/0896-6273(95)90097-7. [DOI] [PubMed] [Google Scholar]
- Rosenmund C., Stevens C.F. Definition of the readily releasable pool of vesicles at hippocampal synapses. Neuron. 1996;16:1197–1207. doi: 10.1016/s0896-6273(00)80146-4. [DOI] [PubMed] [Google Scholar]
- Schneggenburger R., Neher E. Intracellular calcium dependence of transmitter release rates at a fast central synapse. Nature. 2000;406:889–893. doi: 10.1038/35022702. [DOI] [PubMed] [Google Scholar]
- Schneggenburger R., Meyer A.C., Neher E. Released fraction and total size of a pool of immediately available transmitter quanta at a calyx synapse. Neuron. 1999;23:399–409. doi: 10.1016/s0896-6273(00)80789-8. [DOI] [PubMed] [Google Scholar]
- Schroeder T.E. Surface area change at fertilizationResorption of the mosaic membrane. Dev. Biol. 1979;70:306–326. doi: 10.1016/0012-1606(79)90030-7. [DOI] [PubMed] [Google Scholar]
- Simon S.M., Llinas R.R. Compartmentalization of the submembrane calcium activity during calcium influx and its significance in transmitter release. Biophys. J. 1985;48:485–498. doi: 10.1016/S0006-3495(85)83804-2. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Stevens C.F., Wesseling J.F. Activity-dependent modulation of the rate at which synaptic vesicles become available to undergo exocytosis. Neuron. 1998;21:415–424. doi: 10.1016/s0896-6273(00)80550-4. [DOI] [PubMed] [Google Scholar]
- Tahara M., Coorssen J.R., Timmers K., Blank P.S., Whalley T., Scheller R., Zimmerberg J. Calcium can disrupt the SNARE protein complex on sea urchin egg secretory vesicles without irreversibly blocking fusion. J. Biol. Chem. 1998;273:33667–33673. doi: 10.1074/jbc.273.50.33667. [DOI] [PubMed] [Google Scholar]
- Thomas P., Wong J.G., Lee A.K., Almers W. A low affinity Ca2+ receptor controls the final steps in peptide secretion from pituitary melanotrophs. Neuron. 1993;11:93–104. doi: 10.1016/0896-6273(93)90274-u. [DOI] [PubMed] [Google Scholar]
- Tse F.W., Tse A., Hille B., Horstmann H., Almers W. Local Ca2+ release from internal stores controls exocytosis in pituitary gonadotrophs. Neuron. 1997;18:121–132. doi: 10.1016/s0896-6273(01)80051-9. [DOI] [PubMed] [Google Scholar]
- Voets T., Neher E., Moser T. Mechanisms underlying phasic and sustained secretion in chromaffin cells from mouse adrenal slices. Neuron. 1999;23:607–615. doi: 10.1016/s0896-6273(00)80812-0. [DOI] [PubMed] [Google Scholar]
- Vogel S.S., Blank P.S., Zimmerberg J. Poisson distributed active fusion complexes underlie the control of the rate and extent of exocytosis by calcium. J. Cell Biol. 1996;134:329–338. doi: 10.1083/jcb.134.2.329. [DOI] [PMC free article] [PubMed] [Google Scholar]
- von Gersdorff H., Matthews G. Dynamics of synaptic vesicle fusion and membrane retrieval in synaptic terminals. Nature. 1994;367:735–739. doi: 10.1038/367735a0. [DOI] [PubMed] [Google Scholar]
- von Ruden L., Neher E. A Ca-dependent early step in the release of catecholamines from adrenal chromaffin cells. Science. 1993;262:1061–1065. doi: 10.1126/science.8235626. [DOI] [PubMed] [Google Scholar]
- Wu L.-G., Borst J.G.G. The reduced release probability of releasable vesicles during recovery from short-term synaptic depression. Neuron. 1999;23:821–832. doi: 10.1016/s0896-6273(01)80039-8. [DOI] [PubMed] [Google Scholar]
- Yamada W.M., Zucker R.S. Time course of transmitter release calculated from simulations of a calcium diffusion model. Biophys. J. 1992;61:671–682. doi: 10.1016/S0006-3495(92)81872-6. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Zimmerberg J., Sardet C., Epel D. Exocytosis of sea urchin egg cortical vesicles in vitro is retarded by hyperosmotic sucrosekinetics of fusion monitored by quantitative light-scattering microscopy. J. Cell Biol. 1985;101:2398–2410. doi: 10.1083/jcb.101.6.2398. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Zimmerberg J., Coorssen J.R., Vogel S.S., Blank P.S. Sea urchin egg preparations as systems for the study of calcium-triggered exocytosis. J. Physiol. 1999;520:15–21. doi: 10.1111/j.1469-7793.1999.00015.x. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Zimmerberg J., Blank P.S., Kolosova I., Cho M., Tahara M., Coorssen J.R. A stage-specific preparation to study the Ca(2+)-triggered fusion steps of exocytosisrationale and perspectives. Biochimie. 2000;82:303–314. doi: 10.1016/s0300-9084(00)00215-7. [DOI] [PubMed] [Google Scholar]
- Zucker R.S., Fogelson A.L. Relationship between transmitter release and presynaptic calcium influx when calcium enters through discrete channels. Proc. Natl. Acad. Sci. USA. 1986;83:3032–3036. doi: 10.1073/pnas.83.9.3032. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Zucker R.S., Delaney K.R., Mulkey R., Tank D.W. Presynaptic calcium in transmitter release and posttetanic potentiation. Ann. NY Acad. Sci. 1991;635:191–207. doi: 10.1111/j.1749-6632.1991.tb36492.x. [DOI] [PubMed] [Google Scholar]
- Zucker R.S. Exocytosisa molecular and physiological perspective. Neuron. 1996;17:1049–1055. doi: 10.1016/s0896-6273(00)80238-x. [DOI] [PubMed] [Google Scholar]