Two Stable, Conducting Conformations of the Selectivity Filter in Shaker K⁺ Channels

Abstract

We have examined the voltage dependence of external TEA block of Shaker K⁺ channels over a range of internal K⁺ concentrations from 2 to 135 mM. We found that the concentration dependence of external TEA block in low internal K⁺ solutions could not be described by a single TEA binding affinity. The deviation from a single TEA binding isotherm was increased at more depolarized membrane voltages. The data were well described by a two-component binding scheme representing two, relatively stable populations of conducting channels that differ in their affinity for external TEA. The relative proportion of these two populations was not much affected by membrane voltage but did depend on the internal K⁺ concentration. Low internal K⁺ promoted an increase in the fraction of channels with a low TEA affinity. The voltage dependence of the apparent high-affinity TEA binding constant depended on the internal K⁺ concentration, becoming almost voltage independent in 5 mM. The K⁺ sensitivity of these low- and high-affinity TEA states suggests that they may represent one- and two-ion occupancy states of the selectivity filter, consistent with recent crystallographic results from the bacterial KcsA K⁺ channel. We therefore analyzed these data in terms of such a model and found a large (almost 14-fold) difference between the intrinsic TEA affinity of the one-ion and two-ion modes. According to this analysis, the single ion in the one-ion mode (at 0 mV) prefers the inner end of the selectivity filter twofold more than the outer end. This distribution does not change with internal K⁺. The two ions in the two-ion mode prefer to occupy the inner end of the selectivity filter at low K⁺, but high internal K⁺ promotes increased occupancy of the outer sites. Our analysis further suggests that the four K⁺ sites in the selectivity filter are spaced between 20 and 25% of the membrane electric field.

INTRODUCTION

The pore in potassium channels has the remarkable, apparently contradictory, properties of high selectivity and high ion throughput. Adding to the complexity of this process is the presence of several ion binding sites in the selectivity filter and inner pore structures, many of which may be simultaneously occupied.

The high resolution solution of the bacterial channel KcsA crystal structure has considerably illuminated the permeation process. The conserved, K⁺ channel selectivity filter provides coordination of four K⁺ ions at sites, labeled 1–4, from the extracellular surface (Morais-Cabral et al., 2001). These authors suggest that, usually, two K⁺ ions occupy the selectivity filter, either in the 1,3 or 2,4 configuration, each with an intervening water molecule. Optimal throughput is achieved if there is little energy difference between these two sets of occupancy states (Morais-Cabral et al., 2001). External TEA may have difficulty blocking the pore when the outermost site is occupied by K⁺ and so the voltage dependence of external TEA block of Shaker K⁺ channels may reflect the voltage-dependent equilibrium between the 1,3 and 2,4 configurations (Thompson and Begenisich, 2003a).

The crystallographic data suggest that when the KcsA channel is bathed in low K⁺ solutions, the selectivity filter tends to be occupied by a single K⁺ ion and, in fact, adopts a very different conformation. It has been suggested that the low K⁺ conformation is nonconducting (Zhou et al., 2001) and that the two conformations interconvert on the millisecond timescale of gating. These authors emphasize that this interconversion between the nonconducting, one-ion and the conducting, two-ion conformations is an important mechanism for maintaining high throughput in the face of high selectivity in K⁺ channels.

We have previously shown that the voltage dependence of external TEA block of Shaker K⁺ channels likely reflects K⁺ occupancy of the selectivity filter (Thompson and Begenisich, 2003a). Thus, it may be possible to use external TEA block to functionally test the suggestions from the crystallographic data that low K⁺ conditions promote a nonconducting, single ion occupancy state of the selectivity filter.

We measured external TEA block of Shaker K⁺ channels in solutions with internal K⁺ concentrations from 2 to 135 mM. We found that the concentration dependence of external TEA block in low internal K⁺ solutions could not be described by a single TEA binding affinity. The data were well described by a two-component binding scheme as if there were two populations of channels: one with a high and one with a low affinity for external TEA. To exhibit two components of TEA block, the interconversion between the high- and low-affinity states must be slow compared with the speed of external TEA block and both states must be conducting. We suggest that the populations with low and high TEA affinity represent one-ion and two-ion K⁺ occupancy modes of the pore selectivity filter, respectively. The voltage sensitivity of TEA block of the two states is consistent with this assignment. The differences in TEA affinity of these two states may represent a conformational difference between the two states of the channel. Thus, the functional data on Shaker K⁺ channels appear to support the K⁺ concentration control over selectivity filter occupancy modes indicated by the crystallographic data but, in contrast to the suggestion made from the crystallographic analysis, both modes readily conduct K⁺ ions.

MATERIALS AND METHODS

K Channel Constructs and Expression

The experiments reported here were done on the inactivation-deletion version of Shaker B, ShB Δ6-46 (Hoshi et al., 1990). Frog (Xenopus laevis) maintenance, oocyte isolation, and RNA injection used standard methods (Goldin, 1992) and have been previously described in detail (Thompson and Begenisich, 2000, 2001). Frogs were anaesthetized with 0.2% tricaine (Sigma-Aldrich). Isolated ovarian lobes were defolliculated by incubation for 60–90 min with 2 mg/ml of collagenase Type IA (Sigma-Aldrich). The procedures for animal handling, maintenance, and surgery were approved by the University of Rochester Committee on Animal Resources.

Electrophysiological Recordings

Potassium channel currents were recorded 1–5 d after RNA injection. Electrophysiological recordings were done at room temperature (20–22°C) with excised inside/out or outside/out macropatches using an Axopatch 200B amplifier (Axon Instruments). Patch pipettes had tip diameters of ∼3–6 μm constructed from Corning 7052 glass (Garner Glass Co.), GC-150 glass (Warner Instruments Inc.), or quartz (Garner Glass Co.), the latter particularly useful for minimizing electrode capacitance artifacts in the experiments with relatively small ionic currents in low internal K⁺ solutions. Data acquisition was performed using a 12 bit analogue/digital converter controlled by a personal computer. Current records were filtered at 5 kHz.

In this study we examined block of Shaker K channels by external TEA in solutions with a range of internal and external K⁺ concentrations. These (and other) K channels undergo a slow, voltage-dependent inactivation process termed slow, or C-type inactivation (Hoshi et al., 1991). This process is exacerbated by solutions with low internal and external K⁺ (Lopez-Barneo et al., 1993; Baukrowitz and Yellen, 1996) and is inhibited by external TEA (Grissmer and Cahalan, 1989; Choi et al., 1991). Thus, it is important to assure that these properties of the C-type inactivation process did not affect our results.

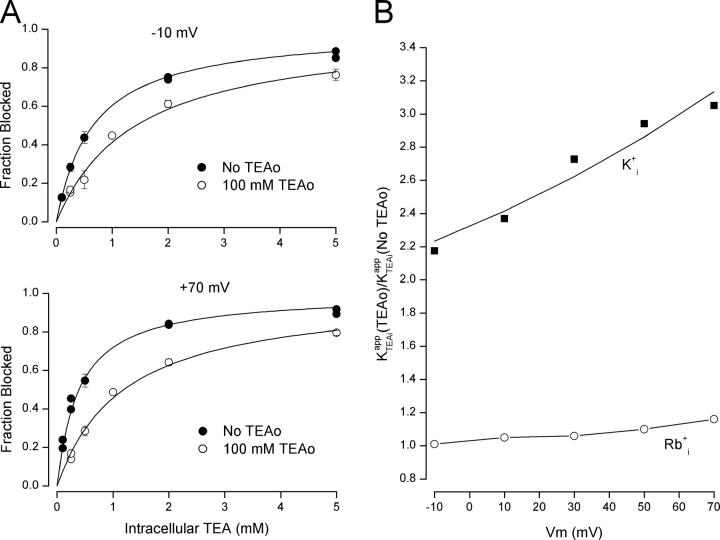
C-type inactivation is easily observed in two ways: (1) a slow decline in current levels during a sustained depolarization and (2) a decline in current level from one depolarizing test pulse to the next. The former is caused by the onset of this inactivation process and even in low K⁺ solutions is quite slow (tens or hundreds of milliseconds; Baukrowitz and Yellen, 1996). The latter is caused by the incomplete removal of slow inactivation during the interpulse interval. An example of the time course of the development of C-type inactivation can be seen in the raw currents in Fig. 1. Even at +70 mV with 5 mM internal K⁺ (Fig. 1 B, bottom) this aspect of the inactivation process was too slow to compromise our results. Note that we measured current levels just after channel opening before significant inactivation could develop.

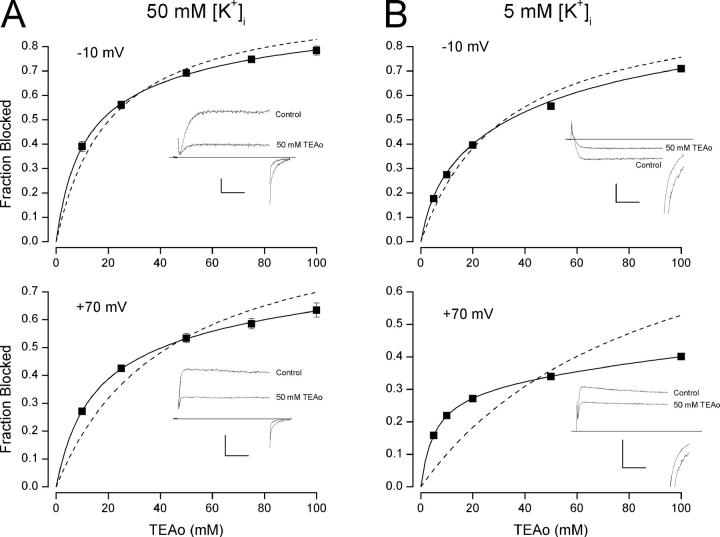
Figure 1.

Low internal K⁺ reveals two components of external TEA block. Fraction of Shaker K⁺ channel current blocked by external TEA in 50 mM (A) and 5 mM (B) internal K⁺ at −10 mV (top) and +70 mV (bottom). Data points are the mean values from 3 to 6 measurements with standard error limits for those whose errors are larger than the symbol size. Insets show raw currents in the absence (Control) and presence of 50 mM external TEA. Calibrations: (A) −10 mV, 50 pA/10 ms; +70 mV, 0.2 nA/10 ms; (B) −10 mV, 50 pA/10 ms; +70 mV, 0.1 nA/10 ms. Dashed lines are fits of Eq. 1 to the data with K _app values of (A) −10 mV, 20 mM; +70 mV, 43 mM; (B) −10 mV, 32 mM; +70 mV, 89 mM. Solid lines are fits of Eq. 2 to the data with the A, K _app ^low, and K _app ^high parameter values shown in Fig. 2.

Since C-type inactivation is voltage dependent, it is minimized with very negative holding potentials. In addition, the rate of removal of slow inactivation produced by a depolarizing pulse is faster at more hyperpolarized potentials (Levy and Deutsch, 1996). Thus, when using solutions of internal K⁺ of 20 mM or less, we used an 8-s interpulse interval in combination with a holding potential of −90 mV instead of the −70 mV value used with higher internal K⁺. The −90 mV holding potential removed the pulse-to-pulse decline caused by the incomplete removal of C-type inactivation in channel current seen in low K⁺ solutions with a −70 mV holding potential (not depicted). In a set of five control experiments with 5 mM external and internal K⁺, we examined channel currents at +50 mV and block of these currents by 50 mM external TEA with holding potentials of −90 and −110 mV. There was no difference between the currents recorded with the −90 and −110 mV holding potentials; the measured current ratio was 0.97 ± 0.03 (SEM). This result confirmed that the −90-mV holding potential and long interpulse interval used in these experiments were sufficient to assure minimization of C-type inactivation. Block by 50 mM TEA at the +50 mV test pulse was 35.6 ± 0.85% and 35.4 ± 0.36% with the −90 and −110 mV holding potential, respectively, likewise not significantly different. These results demonstrate that C-type inactivation did not compromise our findings, even with very low K⁺ levels.

The standard external solution contained (in mM) 5 KCl, 135 NMDG-Cl, 2 CaCl₂, 2 MgCl₂, 10 mM HEPES, pH of 7.2 (with NMDG). External TEA was added to this solution by equimolar replacement of NMDG. The standard internal solution consisted of (in mM) 110 KCl, 25 KOH, 10 EGTA, 10 HEPES, pH 7.2 (with HCl). In some experiments, Rb⁺ completely replaced the K⁺ in the internal solution. The K⁺ or Rb⁺ content in the external or internal solutions was altered by equimolar replacement with NMDG. The junction potentials between the relevant combinations of these solutions did not exceed 4 mV and so corrections for these were not made.

Data Analysis

Current block by different concentrations of TEA were fit by single-, or two-component Langmuir equations:

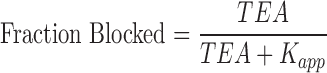(1)

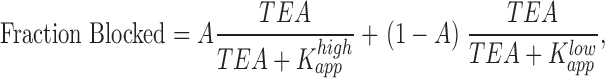(2)

where A represents the fraction of current blocked with high affinity.

Shaker channel activation gating occurs between about −60 and −10 or −20 mV (Yellen et al., 1991). Thus, in order to minimize any apparent voltage dependence coupled to channel gating, we analyzed TEA block of currents obtained at membrane potentials between −10 and +70 mV. In one set of experiments (see Fig. 2 insets), we determined external TEA block of instantaneous currents at potentials from −40 to −10 mV following a 5-ms activation voltage of +20 mV. The capacity transient in these experiments was eliminated with a standard P/4 protocol (Armstrong and Bezanilla, 1977; Begenisich and Cahalan, 1980).

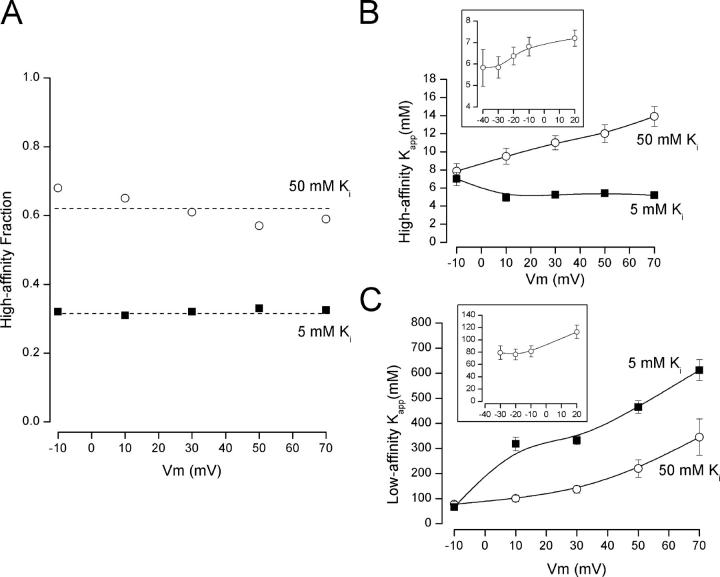
Figure 2.

Voltage dependence of the two components of external TEA block. (A) The fraction of current block by external TEA with high affinity (the parameter A in Eq. 2) at 50 mM (○) and 5 mM (▪) internal K⁺. (B) The K _app ^high value from Eq. 2 determined in 50 mM (○) and 5 mM (▪) internal K⁺. (C) The K _app ^low value from Eq. 2 determined in 50 mM (○) and 5 mM (▪) internal K⁺. Insets: K _app ^high and K _app ^low values obtained at negative potentials from block of current tails with an activation voltage of +20 mV (see materials and methods). The values at +20 mV were from block of steady-state current during the activation pulse.

Fits of equations to the data were done using the Levenberg-Marquardt algorithm as implemented in Origin 6.1 (OriginLab Corp.). Error limits for the fitted parameters are the estimated errors from the fitting routine.

RESULTS

As noted in an earlier study (Thompson and Begenisich, 2003a), the concentration dependence of external TEA block in low internal K⁺ solutions is not well described by a single TEA binding affinity. Examples of this are illustrated in Fig. 1. We measured external TEA block of steady-state Shaker K⁺ channel currents over the voltage range from −10 to +70 mV. Shown in Fig. 1 A is the fraction of channel current with 50 mM internal K⁺ blocked by external TEA at −10 (top) and +70 mV (bottom). The dotted line in each figure represents the best fit of a standard, one-component TEA block relation (Eq. 1) to the data (see materials and methods). At −10 mV (top), the discrepancy is small and could, in some conditions, be accounted for by statistical or experimental errors. However, at +70 mV (bottom) the discrepancy between the data points and a one-component block relation is well beyond any reasonable error. Fig. 1 B shows that the discrepancy at positive potentials is substantially increased when the internal K⁺ concentration is reduced to 5 mM.

Also shown in Fig. 1 (solid lines) are best fits of a two-component TEA block relation (Eq. 2). These fits clearly provide a very accurate representation of the data. The voltage-dependent discrepancy between the data and the one-component relation could arise from a voltage-dependent change in the relative fraction of channels blocked with high and low affinity. That is, at negative potentials, there would be mostly one component and depolarization would increase the contribution of a second component not prevalent at negative voltages. However, the two-component analysis (Eq. 2) did not support this mechanism; the high-affinity fraction (parameter A in Eq. 2) from the fit to the 50 mM K⁺ data in Fig. 1 A is 0.68 and 0.59 at −10 and +70 mV, respectively, and for the 5 mM K⁺ data (Fig. 1 B), the values were 0.32 for both the −10 and +70-mV data. This analysis showed that the voltage-dependent change in shape of the TEA block relationship was due to a difference in the voltage sensitivity of the high- and low-affinity K _app values. In 50 mM K⁺, the depolarization from −10 to +70 mV increased the high-affinity K _app slightly from 8.9 to ∼13 mM, but the low-affinity value almost tripled from 95 mM at −10 mV to 276 mM at +70 mV. The fits to the 5 mM K⁺ data indicated a slight decrease in the high-affinity K _app from 6.6 to 5.3 mM but a very large increase in the low-affinity value from 67 to 575 mM.

We performed this two-component analysis for several voltages between −10 and +70 mV for 5 and 50 mM internal K⁺, and the results are illustrated in Fig. 2. Part A shows that, as indicated above, the fraction of current blocked with high affinity was relatively independent of membrane voltage but was sensitive to internal K⁺. The dashed lines in the figure represent the average fraction of current blocked with high affinity, and these values were 0.62 and 0.32 for 50 and 5 mM internal K⁺, respectively. That is, in 50 mM internal K⁺, 62% of the channel current was blocked by external TEA with a relatively high apparent affinity; the other 38% was blocked with a much lower affinity. The high-affinity fraction was reduced to only ∼32% in 5 mM internal K⁺.

To provide accurate estimates for the high- and low-affinity values of external TEA block in the 50 mM and 5 mM K⁺ solutions, we fit the dose–response relation (Eq. 2) using the average value of the high-affinity fraction parameter A as determined in Fig. 2 A. This increases the reliability of the estimate for this parameter and for the high- and low-affinity K _app values. The resulting best-fit values for these apparent affinity constants at the various membrane potentials are illustrated in Fig. 2 (B and C).

The K _app value for the high-affinity TEA block was clearly voltage dependent in 50 mM K⁺ (Fig. 2 B, ○) over the range from −10 to +70 mV. As described in materials and methods, we used instantaneous current measurements to extend our estimate of this parameter to more negative potentials (Fig. 2 B, inset). The high-affinity K _app value acquired a minimum value near 5.5 mM at these negative potentials. The high-affinity K _app value for TEA block in 5 mM K⁺ was relatively voltage insensitive with a value between 5 and 6 mM (▪), very close to the limiting, minimal value at negative potentials seen with 50 mM K⁺.

The estimated K _app values for the low-affinity TEA block in 5 mM and 50 mM K⁺ solutions are illustrated in Fig. 2 C (▪ and ○, respectively). In both internal K⁺ solutions, the low-affinity K _app values were voltage dependent and, at least for 50 mM K⁺, appeared to reach a limiting value near 70 mM at negative potentials (Fig. 2 C, inset). The low-affinity K _app values in 5 mM K⁺ were generally much larger than those in 50 mM K⁺ but may be approaching an asymptotic value similar to that in 50 mM internal K⁺. However, this conclusion is weakened by the “complex” nature of the K _app values in 5 mM K⁺ near 0 mV, the reversal potential for the channel current in these conditions. This may be due to some contribution of driving force direction on external TEA block or simply the result of the small current size at potentials near the current reversal potential.

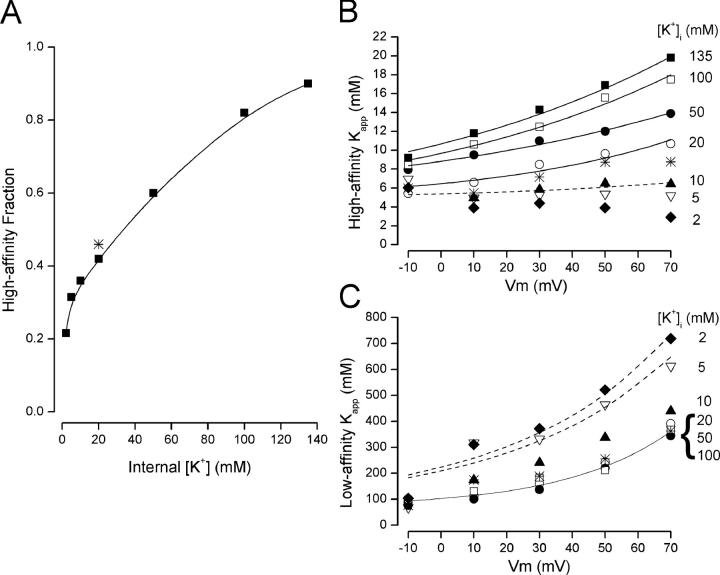
We performed this type of two-component analysis of external TEA block data with internal K⁺ concentrations from 2 mM to 135 mM over the −10 to +70 mV range. The results of all these analyses are illustrated in Fig. 3. Part A shows the internal K⁺ concentration dependence of the high-affinity fraction of TEA block (averaged over membrane voltage for each internal K⁺ level as illustrated in Fig. 2 A). Increasing internal K⁺ increased the fraction of current blocked by TEA with high affinity and this relationship had a midpoint near 30 mM. This more complete analysis confirmed the result noted above that the relative amount of high- and low-affinity block depended only on internal K⁺ concentration and not on membrane potential.

Figure 3.

Properties of the two components of external TEA block. (A) Fraction of high-affinity block as a function of internal K⁺ with 5 mM external K⁺ (▪) except for the 2 mM data, which were obtained with 2 mM external K⁺. Data were also obtained with 20 mM external and 20 mM internal K⁺ (*). The solid line is a fitted spline function and serves only to connect the data points. (B) Voltage dependence of the K _app ^high values at the indicated internal K⁺ concentrations (different symbol for each internal K⁺ level) with 5 mM external K⁺ except for the 2 mM data, which were obtained with 2 mM external K⁺. Data were also obtained with 20 mM external and 20 mM internal K⁺ (*). The solid lines are fits of Eq. 3a to the data all with a K _T2 value of 5 mM and the δ ₂ and K _eq2 (0) values shown in Fig. 5. (C) Voltage dependence of the K _app ^low values at the indicated internal K⁺ levels with 5 mM external K⁺ (same symbols as in B) except for the 2 mM data, which were obtained with 2 mM external K⁺. Data were also obtained with 20 mM external and 20 mM internal K⁺ (*). Solid line is from Eq. 3b with K _T1, δ ₁, and K _eq1 (0) values of 70 mM, 0.78, and 0.47, respectively. The dashed line associated with the 5 mM K⁺ data (▿) is from Eq. 3b with K _T1, δ ₁, and K _eq1 (0) values of 70 mM, 0.5, and 2, respectively. The dashed line associated with the 2 mM K⁺ data (♦) is from Eq. 3b with K _T1, δ ₁, and K _eq1 (0) values of 70 mM, 0.52, and 2.1, respectively.

Fig. 3 B shows the voltage dependence of the high-affinity, apparent equilibrium constant for TEA block at the indicated internal K⁺ concentrations. The K _app values in 135 mM K⁺ solutions increased markedly at depolarized potentials. As the internal K⁺ concentration was decreased there appeared to be a smooth, gradual reduction in the apparent voltage dependence. The K _app values with 10 mM internal K⁺ (▴) increased just slightly with depolarization. As previously illustrated in Fig. 2 B, the high-affinity K _app value in 5 mM K⁺ (▿) had no consistent voltage dependence. With 2 mM internal K⁺, the K _app value actually appeared to decrease slightly with depolarization (♦).

In contrast to the smoothly graded change in the high-affinity K _app values with changes in internal K⁺, the low-affinity values appeared more complex. Internal K⁺ over the range of 20–100 mM had little or no effect on these low-affinity K _app values (○, •, and □) but these were considerably lower than those with 2 and 5 mM K⁺ (♦ and ▿). Data with 10 mM internal K⁺ (▴) appear intermediate between these two groups but closer to the other higher K⁺ values. All the low-affinity K _app values appear to be equally voltage dependent with some complexity in the shape of the relationship in the lowest two K⁺ values near 0 mV.

The experimental conditions for the 2 and 5 mM data differed from those at higher concentrations: all the high internal K⁺ concentrations were paired with an external solution that contained 5 mM K⁺ (see materials and methods); the 2 and 5 mM K⁺ internal solutions were paired with external solutions of the same 2 and 5 mM K⁺ concentrations, respectively. Thus, a K⁺ concentration gradient was present in experiments with K⁺ concentrations 10 mM and greater but not with the two low K⁺ solutions. To test whether it was the gradient or the internal K⁺ that was responsible for the differences in the data, we measured TEA block with 20 mM internal K⁺ paired with an external 20 mM K⁺ solution. These data also exhibited two components of block with about the same high-affinity fraction (Fig. 3 A, *) as with 5 mM external K⁺. In addition, the high (Fig. 3 B, *) and low (Fig. 3 C, *) K _app values with these 20 mM//20 mM K⁺ solutions were quite similar to those with 5 mM//20 mM K⁺ and quite distinct from the data with 5 mM//5 mM K⁺. Thus, the most important determinant of the sensitivity of Shaker K⁺ channels to external TEA block was internal K⁺, not external K⁺, and not the K⁺ gradient.

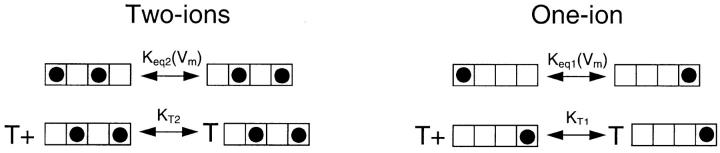
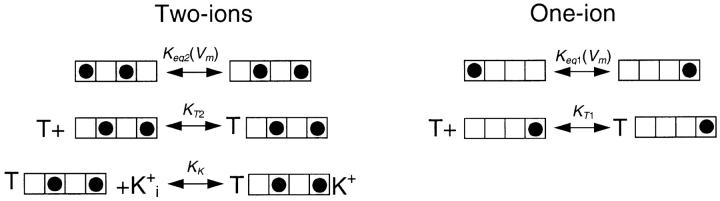
We have previously shown (Thompson and Begenisich, 2003a) that the voltage dependence of external TEA block all but disappears when Rb⁺ replaces K⁺ ions in these types of experiments, indicating that this voltage dependence has more to do with the activity of permeant ions within the pore than TEA moving through the membrane electric field. Viewed in this way, the two components of TEA block shown here indicate two separate, relatively stable states of pore occupancy, both of which conduct ions. If these two states interconverted faster than TEA block, there would be only a single TEA block component. Internal K⁺ concentration appears to control the relative amount of the low and high-affinity components (Fig. 3 A), favoring the low-affinity state at low K⁺ concentrations. Therefore, it seems reasonable to consider that the low TEA–affinity state represents a selectivity filter with a low occupancy of K⁺ ions and the high-affinity state a high-occupancy one. Following the lead of the crystallographic analyses (Morais-Cabral et al., 2001; Zhou and MacKinnon, 2003; see also Berneche and Roux, 2001), we consider the high- and low-occupancy states to represent two-ion and one-ion occupancy of the selectivity filter:

Figure .

The four-component boxes are meant to represent the four K⁺ occupancy sites in the selectivity filter with the external solution on the left. In the two-ion mode, the pair of ions likely move together and occupy sites 1,3 and 2,4. Since the selectivity filter is within the membrane electric field, the equilibrium constant controlling occupancy of these sites will, as indicated, be voltage dependent. In these schemes, external TEA (T) cannot bind to the channel when the permeant ions occupy their most externally oriented positions. The single-ion occupancy mode has been simplified to consider only the two extreme positions. Adding more single-ion occupancy states, even if these can be blocked by external TEA, does not change the qualitative expectations from such a scheme. The equilibrium constant and TEA affinity values in the simplified one-ion model used here represent aggregate parameters composed of, in the more complicated version, “weighted” values for the equilibrium constants and TEA affinities of the various occupancy states.

The state diagrams above do not include ion occupancy of sites outside the selectivity filter. These need to be included in the model if there are significant changes in the occupancy of such sites under the conditions of our experiments. In the original study of the crystallized KcsA K⁺ channel (Doyle et al., 1998), the authors used difference electron density maps to localize permeant ions in the pore. This study did not reveal any K⁺ ion binding sites external to the selectivity filter; however, a more recent study using a Fab antibody to improve crystallographic resolution revealed an electron density near the outer entrance to the selectivity filter (Zhou et al., 2001), a position labeled S₀ in order to distinguish this site from the four (S₁–S₄) in the selectivity filter itself. The fact that this site escaped detection in the original study and has not been described in newer studies on ion occupancy in KcsA crystals (M. Zhou and MacKinnon, 2003, 2004a,b) suggests that this site may have a low occupancy probability. However, molecular dynamics free energy simulations of KcsA (Berneche and Roux, 2001) indicate conditions under which significant K⁺ occupancy of the S₀ site could occur. Another molecular dynamics simulation of the KcsA structure (Crouzy et al., 2001) suggests that external TEA blocks the pore by binding near this site, indicating the possibility for a competition between external K⁺ ions and TEA. We have previously tested external TEA block of Shaker K⁺ channels with external K⁺ concentrations from 0.2 to 40 mM (Thompson and Begenisich, 2001, 2003a), and neither the apparent affinity nor the voltage dependence of TEA block is affected. Thus, the experimental data on the Shaker pore indicates that K⁺ occupancy of sites external to the selectivity filter is unlikely to be significant for the conditions of our experiments and so is not included in the models above.

The crystallographic analyses of KcsA indicate considerable K⁺ occupancy of a site interior to the selectivity filter, the so-called “cavity site” (Doyle et al., 1998; Zhou et al., 2001; Zhou and MacKinnon, 2004). K⁺ occupancy of this site may be overestimated in these analyses because the “closed” form of the crystallized channel likely traps K⁺ ions in this spot. Nevertheless, increasing internal K⁺ from 20 to 100 to 400 mM significantly decreases internal TEA block of KcsA channels (Kutluay et al., 2005), indicating significant occupancy of the inner end of the KcsA channels by K⁺ ions at these concentrations. In contrast, internal K⁺ concentrations from 20 to 140 mM have little or no effect on internal TEA block of Shaker K⁺ channels (Thompson and Begenisich, 2001), demonstrating only very transient occupancy of these internal sites by K⁺ in conducting channels. However, internal K⁺ ions significantly interfere with internal TEA block of Shaker K⁺ channels when these channels are made nonconducting via block by external TEA (Thompson and Begenisich, 2001, 2003b).

Thus, internal K⁺ ions likely occupy sites internal to the Shaker selectivity filter when the channels are blocked by external TEA, and these need to be included in any description of external TEA block. However, as discussed above, the methodology of this previous work included changes in the internal K⁺ concentration, which, as we have shown here, alters the proportion of channels operating in the one-ion and two-ion modes. In the face of this new information, it is necessary to consider carefully the issue of K⁺ occupancy of internal sites in each of the two modes.

If external TEA promoted significant occupancy of inner pore sites, the apparent affinity for block would not be independent of internal K⁺ levels. The data in Fig. 3 C (○, •, □, and ▪) show that the low-affinity K _app values for external TEA block are independent of internal K⁺ from 100 down to 20 mM. So either K⁺ does not significantly occupy internal pore sites in this concentration range or there is some other internal K⁺-dependent process that exactly compensates for such occupancy. With no data to suggest otherwise, we will consider the simpler solution and not include K⁺ occupancy of inner sites in our theoretical analysis.

In contrast to the insensitivity of low-affinity TEA block to internal K⁺ concentration, the high-affinity component was quite sensitive to internal K⁺ from 20 to 135 mM (Fig. 3 B). This and the previous work described above shows that it is necessary to include K⁺ occupancy of inner pore sites with external TEA block. Thus, we will have the following diagrams showing occupancy states of the one-ion and two-ion selectivity filters:

Figure .

where K _K represents the apparent affinity for inner pore occupancy by K⁺ ions when the pore is occluded by external TEA. These schemes can be readily solved to yield expressions for the apparent affinity for external TEA block.

Two-ions:

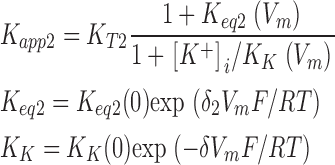(3a)

One-ion:

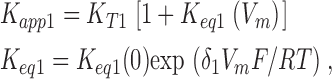(3b)

where K _K(0) represents the affinity of the internal site for K⁺ ions at 0 mV and R, T, and F have their usual thermodynamic meanings. The δ parameter provides for the voltage dependence of the apparent K⁺ binding affinity to the inner pore. It could represent the fraction of the membrane electric field that internal K⁺ ions cross in getting from the internal solution to the binding site but it can also include the voltage-dependent movement of any other K⁺ ions that move in order for the binding site to be occupied.

δ₁ and δ₂ represent the effective fraction of the electric field crossed by the K⁺ ions when they move from their outermost to innermost positions in the selectivity filter. Since the two-ion mode involves the movement of two ions, the actual electrical distance will be half of the δ₂ parameter. K _eq1 (0) and K _eq2 (0) represent the zero voltage equilibrium positions of the ions. For example, a unity value for this parameter means that, at zero voltage, the ions in the selectivity filter spend equal time in their outermost and innermost positions. Note that a very small zero-voltage equilibrium value (<<1) implies site-occupancy energies strongly favoring inner site occupation and, in these conditions, the voltage dependence would only be revealed at very large depolarizations.

It is our goal to test the applicability of this model for describing the high- and low-affinity modes of external TEA block. To this end, we would fit the equations for K _app2 and K _app1 to the high- and low-affinity TEA block data of Fig. 3 (B and C). However, the large number of free parameters (K _T2, K _eq2 (0), δ ₂, K _K(0), and δ) in the set of equations describing block of the two-ion mode compromises this task. The reliability of the analysis would be greatly improved if values for some of these parameters could be independently determined. We have previously studied the properties of the internal K⁺ site (K _K(0) and δ) by examining the ability of external TEA to promote site occupancy by K⁺ ions and so protect from block by internal TEA (Thompson and Begenisich, 2000, 2001). For external TEA concentrations much larger than the TEA affinity, this protection has a simple form (see Thompson and Begenisich, 2001):

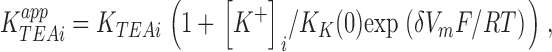(4)

where K _TEAi ^app is the apparent affinity for internal TEA block in high (saturating) external TEA and K _TEAi is the affinity for internal TEA block in the absence of external TEA. Since 90% of the channels are in the high-affinity (two-ion selectivity filter) state at 135 mM internal K⁺ (Fig. 3 A), good estimates for K _K(0) and δ can be obtained from the application of Eq. 4 to the voltage dependence of the external TEA-mediated protection from internal TEA block in 135 mM K⁺ solutions as illustrated in Fig. 4.

Figure 4.

Determination of the affinity and apparent voltage dependence for K⁺ binding to an internal site. (A) Fraction of current at −10 mV (top) and +70 mV (bottom) blocked by the indicated internal TEA concentrations in the absence (•) and presence (○) of 100 mM external TEA with 135 mM internal K⁺. Symbols with standard error limits represent mean values from 3–4 measurements. Symbols without error bars represent individual measurements. Solid lines are fits of Eq. 1 to the data with K _app values at −10 mV of 0.65 and 1.4 mM and at +70 mV of 0.39 and 1.2 mM in the absence and presence of external TEA, respectively. (B) The ratio of the K _app values for internal TEA block in the presence and absence of external TEA with 135 mM internal K⁺ (▪) and with the internal K⁺ replaced by Rb⁺ (○). The solid line with the internal K⁺ data is the best fit of Eqs. 3 and 4 to the data with K _K(0) and δ values of 102 mM and 0.17, respectively. The line connecting the Rb⁺ data has no theoretical meaning.

Fig. 4 A (top) shows internal TEA block of Shaker K⁺ channels at −10 mV in the absence (•) and presence (○) of 100 mM external TEA with 135 mM internal K⁺. External TEA protected from block by internal TEA as seen by the 2.2-fold increase in the apparent affinity for internal TEA block from 0.65 mM in the absence of external TEA to 1.42 mM with 100 mM external TEA. The protection was increased at +70 mV to a 3.1-fold effect (Fig. 4 A, bottom). Fig. 4 B (▪) presents the membrane potential dependence of the increase in apparent internal TEA affinity. Fitting these data with Eq. 4 reveals an approximate affinity of 100 mM for the internal K⁺ site (K _K(0)) and a voltage dependence represented by a δ of 0.17. The protection and voltage dependence was lost when Rb⁺ replaced the internal K⁺ (Fig. 4 B, ○).

TEA High-affinity Block/Two-ion Selectivity Filter

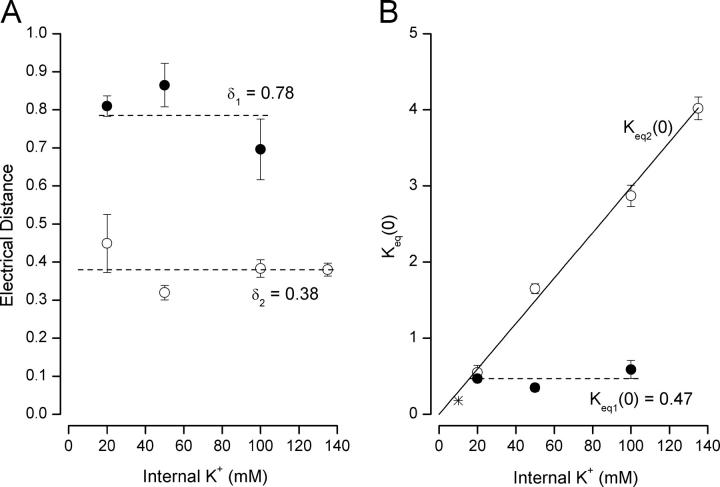
Using these values for K _K(0) and δ we fit the Eq. 3a set to the high-affinity TEA apparent equilibrium constant data in Fig. 3 B for internal K⁺ concentrations from 20 to 135 mM (solid lines). At low internal K⁺ concentrations and very negative potentials, the K _app2 value should approach K _T2, the intrinsic binding affinity for TEA. Inspection of Fig. 3 B suggests that this value may be near 5 mM (see also inset of Fig. 2 B). Thus, we fixed K _T2 at 5 mM, and the resulting fits of the equation set produced estimates for the electrical distance, δ ₂, and zero-voltage equilibrium, K _eq2 (0), values. It is apparent that the fits (Fig. 3 B, lines) were of good quality and the estimated values of δ ₂ and K _eq2 (0) values are shown in Fig. 5. The electrical distance δ ₂ value (Fig. 5 A, ○) appeared to change little, if at all, with internal K⁺ concentration over the range of 20–135 mM. The dashed line in Fig. 5 A represents a δ₂ value of 0.38, the mean of the individual fit values.

Figure 5.

Properties of selectivity filter one-ion and two-ion occupancy modes. Electrical distance and equilibrium values from fits of Eqs. 3a or 3b to the relevant data in Fig. 3. (A) Internal K⁺ and the electrical distance for one-ion (δ ₁, •) and two-ion (δ ₂, ○) occupancy modes. Dashed lines represent the mean of the electrical distance parameters. (B) Internal K⁺ and the equilibrium values (at 0 mV) for the one-ion (K _eq1 (0), •) and two-ion (K _eq2 (0), ○) occupancy modes for internal K⁺ levels ≥20 mM. * represents K _eq2 (0) at 10 mM internal K⁺. The dashed line represents the mean of the K _eq1 (0) values.

The K _eq2 (0) values from the fits of Eq. 3a to the data at different internal K⁺ concentrations are shown in Fig. 5 B (○). These suggest a linear relationship between the internal K⁺ concentration and the zero-voltage equilibrium value: high concentrations of internal K⁺ producing large values of K _eq2 (0). That is, the decrease in the apparent external TEA affinity in high internal K⁺ concentrations appears to arise from a shift in selectivity filter ion occupancy favoring the more external sites which, in the context of the model, are not blockable. This could be due, through electrostatic repulsion, to K⁺ occupancy of a site internal to the selectivity filter. The linear nature of the concentration dependence could reflect occupancy of a very low-affinity site consistent with earlier estimates for K⁺ occupancy of internal pore sites in conducting Shaker channels (Thompson and Begenisich, 2001, 2003a).

This analysis shows that the loss of the voltage dependence of the high-affinity TEA block seen in Fig. 3 B is likely not through the loss of some intrinsic voltage dependence of external TEA block. For example, the high-affinity K _app values in 20 mM K⁺ (Fig. 3 B, ○) appear to be less voltage dependent that those in 135 K⁺, but the analysis reveals the same δ₂ value near 0.38 as seen with the higher K⁺ levels. What makes the voltage dependence of the high-affinity K _app appear to decrease in low K⁺ solutions is a reduction in the K _eq2 (0) values (Fig. 5 B). This point can be emphasized by examining the high-affinity K _app values in 10 mM internal K⁺ (Fig. 3 B, ▴). These data appear to have almost no voltage dependence, and yet the same δ₂ value of 0.38 provides an excellent representation of these data (Fig. 3 B, dashed line) with value for K _eq2 (0) consistent with the trend of the results for the higher concentrations (Fig. 5 B, *). Thus, the high-affinity TEA block data can be accurately described by the two-ion selectivity filter model from 135 down to 10 mM K⁺.

TEA Low-affinity Block/One-ion Selectivity Filter

As noted above, the low-affinity K _app values did not exhibit the smoothly graded behavior with internal K⁺ seen with the high-affinity values. Rather, these values clustered in two groups: one for K⁺ concentrations between 20 and 100 mM and the other for the lowest internal K⁺ concentrations of 2 and 5 mM (Fig. 3 C). The very low fraction of low-affinity block in 135 mM K⁺ (less than 10%, see Fig. 3 A) precluded obtaining a reliable estimate for the affinity constant in this solution.

We fit Eq. 3b to the data with 20–100 mM K⁺ in order to obtain estimates for the voltage sensitivity and magnitude of K⁺ occupancy in the one-ion selectivity filter state. As suggested by the data at very negative potentials, we used a value of 70 mM for K _T1, the intrinsic TEA affinity for the one-ion occupancy state of the selectivity filter. The results of these fits are illustrated in Fig. 5 (•). The voltage sensitivity of the selectivity filter K⁺ equilibrium in the one-ion mode (δ ₁) appears to have a relatively constant value near 0.78, independent of internal K⁺, rather larger than the 0.38 value obtained from the high-affinity data (○). The K _eq1 (0) values (Fig. 5 B, •) are also independent of internal K⁺ levels with a constant value near 0.47, another property of the one-ion mode that differs significantly from its counterpart in the two-ion mode. The solid line in Fig. 3 C is Eq. 3b with these average values for δ ₁ and K _eq1 (0) and clearly provides a reasonable description of the data at internal K⁺ levels from 20 to 100 mM.

The complex shape of the 5 and 2 mM K⁺ data in Fig. 3 C precludes accurate estimates of the K _T1, δ₁, and K _eq1 (0) values for these very low K⁺ conditions. However, these data with 5 and 2 mM K⁺ are clearly very different than those obtained with 20–100 mM K⁺, so the low K⁺ must produce a large change in some property of the channel pore. For example, the dashed lines in Fig. 3 C are fits to the 5 and 2 mM K⁺ data with the K _T1 value set to 70 mM with fitted δ₁ values of 0.5 and 0.52, respectively, and K _eq1 (0) values of 2 and 2.1, respectively, all considerably different than those obtained from data with higher K⁺ levels.

DISCUSSION

The results of the study presented here show that the pore in Shaker K⁺ channels is blocked by external TEA in a rather complex manner. In high internal K⁺, almost all the current can be blocked as if there is mostly a single population of TEA binding sites with a relatively high affinity. Lower internal K⁺ promotes the presence of a population of channels with a much lower TEA affinity. The proportion of the high- and low-affinity states appears to be governed by the internal K⁺ concentration and not by external K⁺ or membrane voltage. The voltage dependence of the apparent affinities for external TEA block is sensitive to the internal K⁺ concentration. All these findings are summarized in Fig. 3. This dual population phenomenon appears restricted to external TEA block as the dose–response relations for internal TEA are well described by a single blockable component at least for intracellular K⁺ levels down to 20 mM (Thompson and Begenisich, 2001, 2003b).

The existence of two components of external TEA block may not be unique to Shaker K⁺ channels. From the results of their study of TEA block of Kv2.1 channels, Immke et al. (1999) suggested that external TEA block is sensitive to K⁺ occupancy of the selectivity filter. Low internal K⁺ reduces the apparent external TEA efficacy of Kv2.1 channels with little effect on potency (Immke and Korn, 2000). These data were interpreted to represent two components of block: a single component with a high TEA affinity (near 2 mM) and a second, unblockable fraction. It is possible that the unblockable fraction is, instead, a second blockable component with a very low TEA affinity. Indeed, the amount of block did not fully saturate even up to the 100 mM TEA levels used. These experiments were reported only at 0 mV and the experimental solutions were somewhat different from those used in the present study, so a more detailed comparison is not possible. In addition, the Kv2.1 data were fit with a concentration–response relation that included a variable Hill coefficient. The fact that the Hill coefficients, especially in low K⁺ solutions, were considerably less than unity is consistent with the presence of two blockable components.

As noted in introduction, one of the important issues in K⁺ channel permeation has been to understand how very high ion throughput can exist in the face of a very high affinity of the pore for K⁺ ions. Several general mechanisms have been proposed to account for these apparently contradictory behaviors. One of these proposes that the “first” K⁺ ion binds to the pore with high affinity and may not support high throughput. A second K⁺ ion associates with the pore and induces a conformational change to a form that allows high flux rates. Some support for this idea has come from recent crystallographic analyses of the bacterial KcsA K⁺ channel (Morais-Cabral et al., 2001; Zhou et al., 2001; Zhou and MacKinnon, 2003). These studies suggested that the selectivity filter occupancy changes with the ion concentration in the solutions bathing the protein: predominantly two ions at high ion concentrations and only a single ion with solutions of low permeant ion concentration. The selectivity filter part of the pore attains a different conformation with one-ion occupancy, a conformation said to be nonconducting “because it is essentially pinched shut” (Zhou et al., 2001). Because of the large difference between high- and low-ion selectivity filter structures, it was argued that “their rate of interconversion occurs more on the timescale of gating (milliseconds) than that of ion conduction (nanoseconds)” (Zhou et al., 2001).

The studies described here were designed to functionally test the generality of the conclusions made from the crystallographic analyses. The presence of two components of external TEA block of Shaker K⁺ channels, regulated by internal K⁺, is certainly consistent with two conformations as suggested by the crystallographic analyses and likely represents the two-ion and one-ion occupancy modes. However, different apparent TEA affinities does not immediately imply different conformations since it is clear that external TEA block is sensitive to ion occupancy of the selectivity filter (Korn and Ikeda, 1995; Immke and Korn, 2000; Thompson and Begenisich, 2003a). Thus, to quantitatively test whether the low-affinity TEA block in low internal K⁺ was indeed a new pore conformation or merely a redistribution of ions within the pore, we quantitatively analyzed the predictions from the two occupancy states (Eqs. 3a and 3b). These equations show how the apparent TEA affinity is related to some of the fundamental properties of the channel pore. Our analysis suggests that the intrinsic external TEA affinity of the two-ion pore is relatively high at ∼5 mM and that of the one-ion pore is more than an order of magnitude lower, near 70 mM. This supports the suggestion from the crystallographic studies for a structural difference between pores occupied by two or by a single permeant ion. Our data also support the crystallographic prediction that these two conformations are relatively stable, at least much slower that the external TEA block kinetics. If these conformations interchanged faster than TEA block, there would be only a single component of block.

The structure of the KcsA protein changes significantly in the outer pore region when the K⁺ in the crystallization solutions is reduced to very low (mM) values (Zhou et al., 2001; Zhou and MacKinnon, 2003). In particular, under these conditions, the selectivity filter appears too narrow to support conduction. While we find, in agreement with the crystallographic analyses, the presence of two different, stable conformations of the outer region of the Shaker K⁺ channel, both conformations of Shaker channels are conducting.

There are, of course, many possible reasons for the apparent difference between KcsA crystallography and Shaker functionality. It is possible that the KcsA protein could attain an altered, but conducting, conformation in low K⁺ under “normal” conditions but the liquid nitrogen temperatures necessary for the crystallographic studies might favor the observed, apparently nonconducting one. In addition, there are several experimental differences between our functional studies and the crystallographic ones. One of these is that we used NMDG as the K⁺ replacement and many of the crystallographic experiments used Na⁺ as the replacement. However, Morais-Cabral et al. (2001) note that they obtained the same structures with either Na⁺ or NMDG replacing K⁺. Lastly, of course, it may be that these (and other) K⁺ channels have some differences as well as many similarities in the selectivity filter design.

It should be noted that our study seemed to show two versions of the low-affinity TEA state. As seen in Fig. 3 C, the TEA-binding properties of the low-affinity state were relatively independent of internal K⁺ down to 20 mM but changed rather significantly for 5 and 2 mM K⁺. As described above, we cannot unambiguously determine exactly what is different between these two low TEA–affinity states, but there appears to be significant differences in TEA affinity or in K⁺ occupancy. Both states, however, must be conducting.

Our two-component analysis tacitly assumes equal ionic currents through the two different TEA-sensitive conformations. With that assumption, the A factor in Eq. 2 represents the fraction of channels in the high-affinity conformation. If the two conformations have different conductances, then this factor A is a more complex parameter that includes both the relative fraction of channels in each conformation and their respective single channel currents. We certainly have insufficient data to address the question of the conductivity of the low-affinity conformation; however, if the conductance was very much lower than the high-affinity conformation, the A parameter would be expected to have a particularly steep dependence on internal K⁺. This does not appear to be the case (see Fig. 3 A), at least not for internal K⁺ levels ≥20 mM. Thus, we tentatively suggest that the low-affinity TEA conformation for internal K⁺ concentrations down to 20 mM is readily conducting, comparable to the high-affinity TEA conformation. Since the A parameter data in Fig. 3 A appear to have a particularly steep dependence on internal K⁺ for levels <20 mM, it is possible that the second low-affinity conformation in K⁺ concentrations in the low mM range may have a somewhat lower conductance than the conformation at 20 mM and above.

The model used to describe the high- and low-affinity states in terms of two-ion and one-ion occupancy modes of the selectivity filter is a simplified version of models of KcsA channel selectivity filter occupancy (Morais-Cabral et al., 2001; Kutluay et al., 2005). Nevertheless, this simplified version was able to provide an excellent description of the complex nature of external TEA block in the different internal K⁺ solutions. In addition to providing quantitative estimates on the intrinsic TEA affinity of the one- and two-ion occupancy states, our analysis also revealed information about the distribution of ions within the selectivity filter and on the relationship between the sites and the membrane electric field. The occupancy equilibrium value for the one-ion occupancy state was near 0.5 and not particularly dependent on internal K⁺ (see Fig. 5 B). This value means that the ion spends about twice as much time toward the inner end of the selectivity filter compared with the external end. Positive potentials shift the occupancy to increasingly favor the outer sites which, of course, accounts for the voltage dependence of external TEA block seen in Fig. 3 C. In contrast to the behavior of the one-ion state, the ion occupancy of the two-ion pore changes linearly with internal K⁺ (Fig. 5 B). At very low K⁺, the two ions preferentially occupy sites 2 and 4 near the inner end of the pore, but with increased internal K⁺, the equilibrium shifts to favor the 1, 3 orientation. In physiological K⁺ levels, the occupancy of the 1, 3 sites is preferred over the 2, 4 configuration by over fourfold. The internal K⁺ dependence of the equilibrium ion positions may occur because occupancy of a site (or sites) just internal to the selectivity filter causes (through electrostatic repulsion) the ions in the selectivity filter to prefer sites nearer the external entrance. The linear relationship between the equilibrium constant and internal K⁺ may suggest that such a site in the cavity would have a very low affinity, consistent with our previous studies.

The voltage dependence of the equilibrium constant for selectivity filter site occupancy provides information about the relationship between the sites and the membrane electric field. In the single ion occupancy state, the ion moves between site 4 toward the inner end of the filter and the outermost site 1 with a voltage dependence of 0.78. This equates to an approximate drop of 25% of the electric field across each of the selectivity filter sites. In the two-ion mode, the voltage dependence was ∼0.38 (Fig. 5 A) or each of the two ions crossing almost 20% of the electric field. Thus, we estimate that there is a 20–25% change in the electric field across each site within the selectivity filter.

The results reported here demonstrate that the magnitude and voltage dependence of external TEA block reflects K⁺ occupancy of the selectivity filter. The intrinsic TEA affinity under physiological conditions is near 5 mM, and the measured, higher values (often near 17 mM) reflect a particular ion occupancy state of the selectivity filter. External TEA block has little or no intrinsic voltage dependence: the observed voltage sensitivity appears to be due to a voltage-dependent rearrangement of ions in the selectivity filter. In addition, these results support the idea that the selectivity filter is predominantly occupied by two ions in normal intracellular K⁺ and that low K⁺ promotes a one-ion occupancy mode. These one- and two-ion modes appear to have different conformations in at least the outer part of the pore responsible for TEA binding. Both forms of the selectivity filter are readily conducting. The fact that the one-ion mode of the selectivity filter is conducting argues against the classical conformational change mechanism for high-throughput with tight binding.