Heat production in human skeletal muscle at the onset of intense dynamic exercise

Abstract

1. We hypothesised that heat production of human skeletal muscle at a given high power output would gradually increase as heat liberation per mole of ATP produced rises when energy is derived from oxidation compared to phosphocreatine (PCr) breakdown and glycogenolysis.

2. Five young volunteers performed 180 s of intense dynamic knee-extensor exercise (≈80 W) while estimates of muscle heat production, power output, oxygen uptake, lactate release, lactate accumulation and ATP and PCr hydrolysis were made. Heat production was determined continuously by (i) measuring heat storage in the contracting muscles, (ii) measuring heat removal to the body core by the circulation, and (iii) estimating heat transfer to the skin by convection and conductance as well as to the body core by lymph drainage.

3. The rate of heat storage in knee-extensor muscles was highest during the first 45 s of exercise (70-80 J s⁻¹) and declined gradually to 14 ± 10 J s⁻¹ at 180 s. The rate of heat removal by blood was negligible during the first 10 s of exercise, rising gradually to 112 ± 14 J s⁻¹ at 180 s. The estimated rate of heat release to skin and heat removal via lymph flow was < 2 J s⁻¹ during the first 5 s and increased progressively to 24 ± 1 J s⁻¹ at 180 s.

4. The rate of heat production increased significantly throughout exercise, being 107 % higher at 180 s compared to the initial 5 s, with half of the increase occurring during the first 38 s, while power output remained essentially constant.

5. The contribution of muscle oxygen uptake and net lactate release to total energy turnover increased curvilinearly from 32 % and 2 %, respectively, during the first 30 s to 86 % and 8 %, respectively, during the last 30 s of exercise. The combined energy contribution from net ATP hydrolysis, net PCr hydrolysis and muscle lactate accumulation is estimated to decline from 37 % to 3 % comparing the same time intervals.

6. The magnitude and rate of elevation in heat production by human skeletal muscle during exercise in vivo could be the result of the enhanced heat liberation during ATP production when aerobic metabolism gradually becomes dominant after PCr and glycogenolysis have initially provided most of the energy.

Quantification of energy fluxes in contracting muscle in man is difficult. In isometric contractions with a well-defined muscle group this has been attempted when the force of the contraction causes a complete mechanical hindrance to the blood flow, i.e. when aerobic energy yield plays no role (Edwards et al. 1972; Saugen & Vøllested, 1995). In intense dynamic exercise, however, oxidation is the primary energy-liberating pathway after ∼60 s of exercise, while at the onset of dynamic exercise anaerobic energy production predominates. At the whole body level oxygen consumption can be precisely determined in humans, but it is more of a problem to measure the amount of oxygen used by a specific muscle or muscle group. To, at least in part, overcome this problem the one-legged knee-extensor exercise model was developed, confining the dynamic contractions largely to the quadriceps muscle (Andersen et al. 1985; Ray & Dudley, 1998; Richardson et al. 1998). Blood flow to or from this muscle can be measured, as can the arterial-venous (a-v) difference for oxygen, giving the oxygen uptake by the Fick principle. During steady-state submaximal dynamic knee-extension exercise, the mechanical efficiency estimated according to the traditional concept (i.e. the ratio between power output and the sum of the caloric equivalent of oxygen consumption and power output; Benedict & Cathcart, 1913) was found to be ∼25 % (Andersen & Saltin, 1985), which is in the upper range of what has been observed at the whole body level with cycle ergometer exercise (Åstrand, 1960). With more intense exercise and at the onset of exercise not only the oxygen uptake, but also the anaerobic energy liberation has to be determined in order to obtain an estimate of energy turnover. Bangsbo et al. (1990) have made such an attempt using the knee-extensor model. The anaerobic processes were estimated from the reductions of muscle ATP and PCr, the accumulation of glycolytic intermediates and lactate, and the release of lactate. Although there was an acceptable match between this estimate of the anaerobic energy yield and the oxygen deficit (total energy turnover – aerobic energy turnover) it did not provide sufficient insight since total energy turnover during exercise remained unknown.

The appropriate approach, novel in humans performing dynamic exercise, would be to measure heat production, power output and aerobic and anaerobic energy turnover. This approach has a long and successful history in muscle energetics in the in vitro study of muscle fibres (Wilkie, 1960; Hill & Woledge, 1962; Kushmerick et al. 1969), in vivo in exercising animals (Ardevol et al. 1998) and in man performing isometric contractions (Edwards et al. 1972, 1975; Saugen & Vøllested, 1995, 1996). Furthermore, early work of Barcroft & Edholm (1942) and Pennes (1948) established the theoretical basis for the quantification of heat transfer in perfused tissue. Despite the complexity of the various tissues, muscle fibre and motor unit recruitment (Lexell et al. 1983), the knee-extensor model could provide a means to precisely determine total heat production in dynamic exercise by measuring heat stored in the contracting muscles and that dissipated from the muscle. If heat capacity and muscle mass are known, it is possible to determine heat storage via thermosensors placed in the various active muscle portions. Heat dissipation from dynamically contracting muscles consists of heat transfer to the core of the body (limb blood flow × v-a temperature difference according to the Fick principle) and to surrounding tissues or environment. Heat conductance through tissues in the human body is a slow process (Hensel & Bock, 1955) and in knee-extensor exercise it can be estimated based on temperature measurements of inactive tissues within the thigh, including the subcutis. Heat exchange with the surroundings of the exercising thigh (convection and conduction) can be minimised by a thermostat isolation system.

Thus, the aim of this study was to quantify energy liberation at the onset of and during short intense dynamic exercise by continuously measuring the rate of heat production and power output by the knee-extensors. This was done to test the hypothesis that in man the efficiency of conversion of chemical energy to mechanical power is high in the transition from rest to exercise, and then gradually declines corresponding to the change in source of ATP production. This hypothesis is based on the findings from in vitro studies that heat liberation during ATP utilisation varies from 35 to 72 kJ (mol ATP)⁻¹ depending upon whether PCr, glycolysis or oxidative phosphorylation provide the energy for ATP resynthesis (Wilkie, 1968; Curtin & Woledge, 1978; Woledge & Reilly, 1988). Thus, an increase in heat production in the order of a factor of two would be anticipated with a shift in ATP resynthesis from pure PCr splitting to pure oxidative phosphorylation. Continuous measurements were performed of the aerobic metabolism whereas data on the anaerobic contribution could not be obtained simultaneously due to technical restraints. However, such information is available from parallel studies using the same exercise model and of the same intensity and duration.

METHODS

Subjects

The five healthy, recreationally active males participating in this study possessed a mean age of 24 years (range 22–25 years), mean body weight of 76.6 kg (55-92 kg) and a mean height of 180 cm (169-192 cm). The peak oxygen uptake of the quadriceps muscle of one leg was 0.89 l min⁻¹ (0.59-1.05 l min⁻¹) (Table 1). The study was approved by the Ethics Committee of Copenhagen and Frederiksberg communities and was carried out in accordance with the Declaration of Helsinki. Subjects gave written informed consent before participating.

Table 1. Subject characteristics.

Subject no.	Age (years)	Weight (kg)	Height (cm)	Mean power output (W)	Quadriceps and tensor fasciae latae (kg)	Hamstrings, sartorious and adductors (kg)	Skin, subcutaneous fat, connective tissue (kg)	Femur (kg)	Total thigh (kg)	Peak V̇O2 thigh (l min−1)
1	24	74	178	87	2.6	2.8	1.1	0.7	7.2	0.99
2	24	90	188	72	3.0	3.7	2.1	0.9	9.8	0.78
3	25	55	169	66	2.1	2.8	0.8	0.7	6.5	0.59
4	22	92	192	109	3.3	3.6	1.7	1.0	9.6	1.02
5	24	72	172	80	2.4	2.5	1.2	0.7	6.7	1.05
Mean	24	77	180	83	2.7	3.0	1.4	0.8	7.9	0.89
±s.d.	1	15	10	17	0.5	0.6	0.5	0.2	1.6	0.20

Mechanical power output

Power output was continuously recorded during exercise. The mean force produced by the knee-extensor muscles during each kick was estimated by integrating the area under the curve (over the active angle ∼80-170 deg of the kicking cycle) obtained from the changes in voltage detected by a strain-gauge placed between the ankle and the metal rod connecting the leg to the fly-wheel (Andersen et al. 1985). The strain-gauge was calibrated by placing weights of known mass (5 and 20 kg) on the metal rod. The duration of the kick was measured by optical encoders on the cranks of the cycle ergometer. Power output was calculated by estimating the external work done on the ergometer as well as the work done to lift the lower leg.

Oesophageal, arterial, skin and muscle temperatures

Commercially available thermistors were used to continuously monitor skin and oesophageal temperatures (MHC-40050-A, Ellab A/S, Rødovre, Denmark) and for muscle and vessel blood temperatures (Edslab, TD probe 94-030-2.5F).

Thigh blood flow

Femoral venous blood flow was measured by a modified version of the thermodilution technique originally described by Andersen & Saltin (1985). With the same experimental set-up, we obtained continuous measures of the infusate and femoral venous blood temperatures, allowing the calculation of femoral venous blood flow second by second during the time of infusion of cold saline (20 s; 1–3°C) after an initial stabilisation period of 5 s. This was achieved by connecting the thermistors (Edslab, TD probe 94-030-2.5F) via a custom-made interface to a Macintosh Performa computer using a MacLab 8:s data acquisition system (ADInstruments, Sydney, Australia). The data sampling frequency was 100 Hz. Before and during the second 3 min exercise bout, femoral venous blood flow (index of thigh blood flow; TBF) was measured at rest, during the passive acceleration phase, and at the time intervals 0–10, 40-55, 80–95, 120–135 and 170–180 s during the exercise. Infusate temperature was measured at the site where the infusate entered the venous catheter (∼8 cm from the tip of the catheter). The set-up was tested under in vitro conditions to determine the linearity of the system up to blood flows of 10 l min⁻¹. The in vitro experiment was also used to determine the elevation in the infusate temperature occurring as the infusate travels from the measuring point to the tip of the catheter. Infusate temperature was corrected accordingly by 0.6°C with infusion rates of 1.866 ml s⁻¹ (exercise conditions) and by 1.0°C with infusion rates of 0.766 ml s⁻¹ (resting conditions and passive acceleration phase). TBF (expressed in ml s⁻¹) was calculated according to the following formula, derived from a heat balance equation (Ganz & Swan, 1974; Andersen & Saltin, 1985):

where V_I represents infusate rate (ml s⁻¹), T_B is blood temperature before saline infusion (°C), T_I is temperature of infusate, T_M is temperature of blood-saline mixture during steady state (after 5 s infusion), S_I and S_B are specific gravities of infusate and blood (1.005 and 1.045 g cm⁻³), respectively, and C_I and C_B are specific heats of infusate and blood (4.173 and 3.600 J g⁻¹°C⁻¹), respectively.

Under the present experimental conditions (non-steady state), the reference blood temperature increased 0.05-0.09°C during the 20 s of infusion. Furthermore, although venous blood temperature was restored in less than 1 s upon termination of the infusion period, repeated blood flow measurements reduced the venous blood temperature by 0.10-0.15°C after 3 min of exercise compared to that observed during the first bout (no blood flow measurements). Notwithstanding, correction for this effect only increased thigh blood flow values by < 1 %. This effect is therefore negligible compared to the oscillations of limb blood flow from kick to kick (see Results).

Data sampling

Muscle and blood temperatures as well as power output were recorded with a sampling frequency of 100 Hz. In addition to the thermistors in the blood vessels (femoral artery and vein) each of the muscle thermistors were connected via another custom-made interface and A/D converter to an IBM computer board. Data were stored on a hard disk and displayed on-line, using LabVIEW 4.0 software (National Instruments, Austin, TX, USA). Temperature data were then averaged per second and expressed in degrees Celsius. The seven skin thermistors and the oesophageal thermistor were connected to an eight-channel temperature monitor (Ellab CTF 9008 Precision thermometer; Ellab A/S) interfaced with an IBM-AT computer. Data were stored on a hard disk every 15 s and also simultaneously displayed on-line using the data acquisition software PCLINK92 (Ellab A/S).

Muscle mass

Muscle mass was determined by magnetic resonance imaging (MRI) performed on a Siemens 1.5 T MAGNETOM vision scanner (Siemens, Germany). For each subject, 30–33 parallel axial T₁-weighted images (cross-sections) of the right thigh (i.e. from the anterior superior iliac spine to the patellar ligament) were obtained with a multi-slice spin-echo FLASH sequence (T₁ is the spin-lattice relaxation time; repetition time (T_R) = 500 ms, echo time (T_E) = 15 ms) using a standard body coil. Slice thickness was 3 mm with 12 mm interslice distance. Pixel size was 1.2 mm². This setting was selected to optimise image quality in order to most clearly separate muscle, bone, fat, and connective tissue.

Definitions of anatomical cross-sections were performed manually and the area calculations made using NIH Image software (Rasband & Bright, 1995). The proximal portion of the patella was considered as the zero-reference slice for all the subjects. The volume of the compartments was calculated as the sum of each anatomical cross-sectional area times the distance covered by each slice (1.5 cm). Volume values were converted into mass values using the specific density of each compartment (1.043 for muscle, 0.9 for fat, 1.791 for femur, 1.053 for skin, and 1.045 for soft tissue; von Döbeln, 1956). The anatomical compartments investigated were: (1) quadriceps plus tensor fasciae latae muscles (active during knee-extensor exercise), (2) hamstring, sartorious and adductor muscles complex (inactive), (3) bone (femur) and (4) skin, subcutaneous fat and connective tissue complex (Fig. 1A and B).

Figure 1. Anatomical compartments of the thigh and quantification of the knee-extensor muscle mass.

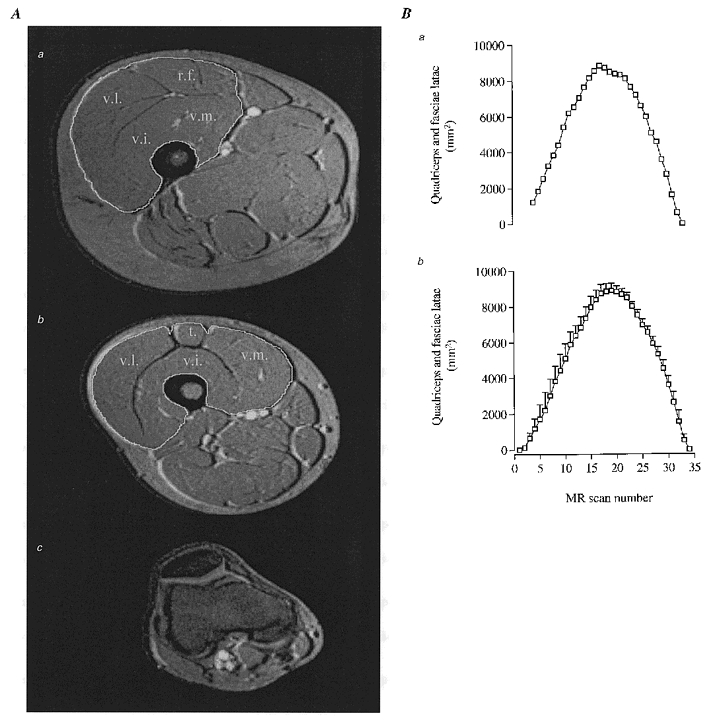
A, MRI of cross-sections of upper-thigh (a) and mid-thigh (b) with the white line indicating borders of quadriceps femoris muscle, including vastus lateralis (v.l.), vastus intermedius (v.i.), vastus medialis (v.m.), rectus femoris (r.f.) and tendon of quadriceps femoris muscle (t). A cross-section of the distal end is shown in c. It was sometimes difficult to exactly determine the origin of the muscles at the proximal end (a). This was solved by plotting the serial individual cross-sections and determining the origin by extrapolation (see Ba). Mean values ±s.e.m. for all subjects’ serial cross-sections of the thigh are shown in Bb.

Procedures and protocol

In preparation for this study the subjects were familiarised with the exercise model by training at a desired cadence while minimising the involvement of the hamstring and gluteal muscles, and thereby confining the work to the knee-extensor muscles. In the preliminary trials they also became accustomed to a thermistor placed in the oesophagus at the level of the heart while exercising.

On the morning of the experiment, subjects arrived after a light breakfast. Three catheters were placed by the Seldinger technique at the level of the inguinal ligament. In the resting leg, a catheter was placed in the femoral artery for blood sampling. In the exercising leg, catheters were placed in both the femoral vein and artery. Through these latter two catheters, thermistors (Edslab probe 94-030-2.5F) were placed just at the tip of the catheters for the measurements of arterial-venous differences for temperature (°C). Following the placement of the arterial and venous catheters, seven thermistors of the same type were inserted into the thigh muscles through a venflon catheter (18G/32 mm). The tip of the thermistor probe used in the muscle was cut 2–3 mm beyond the thermistor location, which was advanced into the muscle 0.5-0.8 cm beyond the catheter tip (approximately 3.0-4.0 cm into the thigh, depending upon muscle group and size of subjects). Different inclinations (30, 45 and 60 deg) with respect to the length direction of the muscle fibres were used when the thermistors were inserted. This minimised their movement, reduced the risk of damaging the muscle or the thermistors and minimised the discomfort of subjects. Three thermistors were inserted in the vastus lateralis (v.l., proximal, medial and distal portions), two in the rectus femoris (r.f., medial and distal portions), one in the distal portion of the vastus medialis (v.m.), and one in the medial portion of the biceps femoris. The flexible venflon catheter was left in place to provide protection for the thermistor probe. The thermistor probes and the venflon catheters were fastened to the skin with tape. Due to the difficulty reaching the vastus intermedius (v.i.) muscle, which is located underneath the rectus femoris (Fig. 1A), thermistors were only placed in this muscle group in two separate additional experiments. Next to each of the seven muscle thermistor probes, a skin thermistor was placed and secured to the skin with tape. Thereafter, the oesophageal thermistor was inserted through the nostril down to the level of the heart.

To minimise the heat losses to the environment surrounding the exercising leg via convection and conductance, local muscle, skin, and blood temperatures were equalised to the core temperature (õesophageal ≈ 37°C) by perfusing a cuff surrounding the thigh with warm water (∼41°C). This procedure lasted 45–60 min until muscle and tissue temperatures were ∼37°C (Fig. 3). Thereafter, the temperature of the water was reduced to 37.5°C to minimise heat transfer to or from the surroundings.

Figure 3. Thigh temperature during the thermal equilibration procedure prior to exercise.

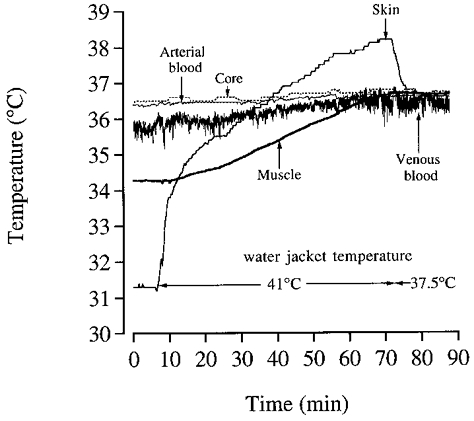
The tissue temperatures of the thigh are depicted when warming it with a water-perfused wrapping set at 41 °C for ≈70 min to equalise these temperatures with the core temperatures (≈37 °C). Thereafter, the water temperature was adjusted to 37.5 °C for the remainder of the experiment.

The subject performed the 3 min knee-extensor exercise with the right leg in a recumbent position at a mean power output of 83 ± 9 W (mean kicking frequency of 1.03 ± 0.02 s⁻¹) for 3 min. The work was close to exhaustive for the subject. During exercise, the left leg was resting. Over a period of 30 s prior to the voluntary exercise, the lower part of the right leg was accelerated passively up to the target cadence to ensure that the subject did not use extra energy to accelerate the fly-wheel. During the 3 min exercise period a cuff just below the knee was inflated to 240 mmHg to avoid any transport of heat to or from this part of the leg. Additionally, blood samples were withdrawn from the femoral artery and vein at rest, during passive exercise and at 13, 31, 50, 75, 128 and 176 s of the first exercise bout, for later analysis of oxygen saturation and haemoglobin concentration (OSM-3 Hemoximeter, Radiometer, Copenhagen, Denmark) as well as blood lactate (lactate analyser; Yellow Springs Instruments, Yellow Springs, OH, USA).

To determine the thigh blood flow the subjects repeated the same exercise after 1 h of recovery during which femoral venous blood flow was measured using the thermodilution method, as explained above. The reason for measuring blood flow during this second bout of exercise was to avoid the confounding effect of infusing cold saline on venous and arterial blood temperatures. Previous results from this laboratory indicate that the pattern and the magnitude of femoral venous blood flow are the same during a first and a second bout of intense knee-extensor exercise, separated by 1 h of recovery (Bangsbo et al. 1992). After each experiment all thermistors were immediately calibrated within the temperature range 35–40°C against a mercury thermometer having a precision of 0.01°C. The response time of the thermistors in the muscles and in the blood vessels, being Teflon imbedded, was ≤ 0.8 s.

Calculations (see Fig. 2)

Figure 2. Schematic model used for calculation of total energy turnover.

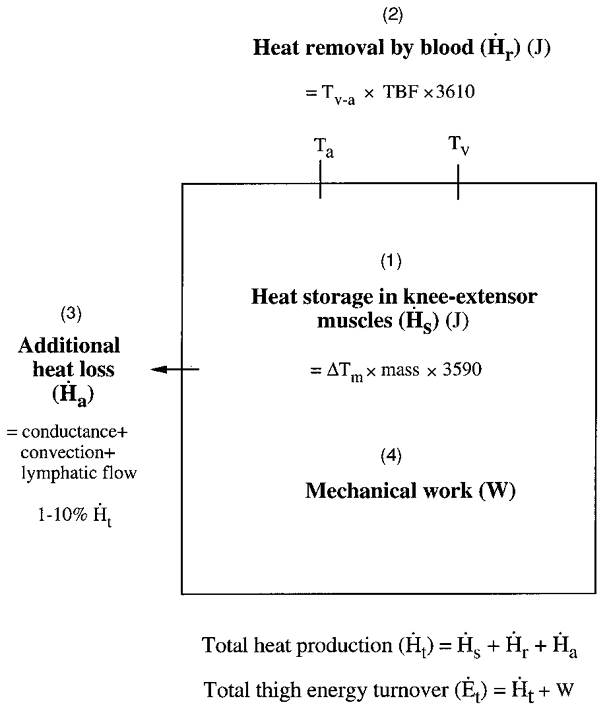
T_a and T_v, arterial and venous temperature. T_v-a, venous-arterial temperature difference. ΔT_m, mean increase in temperature of all muscles.

Rate of heat storage in active muscles

The rate of heat storage (H_s) in the active muscles (i.e. quadriceps and tensor of fasciae latae) was calculated for 5 s intervals by multiplying the mean increase in temperature of all muscle portions in 5 s (ΔT_m;°C) by the muscle mass (m; kg) and the specific heat of the muscle at 37.5°C (c_m= 3590 J kg⁻¹°C⁻¹), assuming a 23 % solid content (based on an average water content in quadriceps muscle of 77 %). The resulting value was divided by 5 to express the rate of heat storage in joules per second. The active muscle mass was corrected by the estimated increases in muscle volume observed during intense knee-extensor exercise (Bangsbo et al. 1992; Ray & Dudley, 1998). The same principle was used to determine the rate of heat storage in the hamstrings muscle:

(1)

Rate of heat removal by the blood

The mean thigh blood flow (TBF; l s⁻¹) during each 5 s interval was estimated from the individual curve fitting applying a 3rd-order polynomial model (r²= 0.84-0.93). The rate of heat removal by the blood (H_r) was calculated for 5 s intervals by multiplying the mean v-a temperature gradient (T_v-a;°C) by TBF and the specific heat of the blood at 37.5°C (blood specific heat (c_b) = 3610 J l⁻¹°C⁻¹; haemotocrit ∼45 %):

(2)

Additional heat loss

The additional rate of heat loss (H_a) was calculated by adding the estimated heat loss to the surrounding skin by the processes of conductance and convection as well as the heat transfer to the body core by the lymph drainage.

(1) Rate of heat loss by conductance. The heat transfer from the knee-extensor muscles to the skin through conductance (H_c) was estimated every 5 s by multiplying the temperature gradient between the muscle and skin (ΔT_m-sk;°C) by the thermal conductivity of human muscle (c_h= 4.8 J s⁻¹ cm⁻¹°C⁻¹; Hensel & Bock, 1955) and the mean distance through which heat is conducted in the anterior thigh (l =∼3 cm):

(3)

(2)Rate of heat loss by convection. Heat loss from the skin of the thigh by convection (H_cv) was calculated every 5 s by multiplying the estimated thigh skin blood flow (SkBF = 0.005 l s⁻¹ measured in the saphenous vein) by the temperature gradient between the arterial blood and saphenous venous blood (equal to skin temperature under these conditions; T_a-v,°C) and the specific heat of the blood (c_b= 3610 J l⁻¹°C⁻¹):

(4)

(3) Rate of heat loss by the lymph flow. Heat loss by the lymph flow (H_l) was estimated by multiplying the estimated lymph flow (LF = 0.003 l s⁻¹) by the arterial-to-lymph temperature gradient, assuming that the lymph leaving the thigh has the same temperature as the venous blood and the same specific heat as plasma (c_p= 3930 J l⁻¹°C⁻¹):

(5)

Rate of heat production

Total rate of heat production (H_t) was calculated by adding the rate of heat storage in the active muscles, the rate of heat removal by the blood and the rate of heat transfer to the skin by conductance and convection as well as to the body core by the lymph drainage:

(6)

Total energy turnover (E_t) was calculated by summing H_t and mechanical power output (W):

(7)

Mechanical efficiency

Mechanical efficiency (MEf) was calculated by dividing the mechanical power output by the total energy turnover:

(8)

Aerobic and anaerobic heat liberation

Oxygen uptake (V̇_O2 expressed in ml s⁻¹) of the exercising thigh was obtained by multiplying the a-v O₂ difference by thigh blood flow (Fick principle), and converted into moles of O₂ assuming that 1 mole O₂ equals 25.4-25.5 l O₂ when temperature ranges from 37 to 38°C. V̇_O2 was then converted into moles of ATP assuming a P:O ratio of 3.0 throughout exercise. Resting V̇_O2 was subtracted from exercise V̇_O2 to compare aerobic heat liberation to total energy turnover. Net PCr hydrolysis and lactate accumulation estimations per 30 s interval are based on the initial and final biopsy mean values (vastus lateralis) obtained in parallel studies with the same experimental protocol (J. Bangsbo, P. Krustrup, J. González-Alonso & B. Saltin, unpublished observations; ΔPCr 16.3 mmol (kg wet wt)⁻¹ and Δlactate 22.3 mmol (kg wet wt)⁻¹) and the kinetics described by Gollnick & Hermansen (Fig. 3, p. 12; 1973) and Sinclair et al. (1999). Lactate concentrations were converted into ATP concentrations by multiplying lactate concentrations by a factor of 1.5. Heat produced per mole of ATP used was assumed to be 35 kJ for ATP and PCr hydrolysis, 65 kJ for glycolysis and 72 kJ for oxidation (Curtin & Woledge, 1978; Woledge & Reilly, 1988).

Analysis

The mean response time for total heat production was estimated by fitting a two-component exponential model to the data, using a (non-linear) least squares regression technique.

Statistics

A one-way repeated measures analysis of variance (ANOVA) was performed to test significance over time. When the F value was significant, pairwise differences were identified using Tukey's honestly significant difference post hoc procedure. The significance level was set at P < 0.05. Data are presented as means ±s.e.m. unless otherwise stated.

RESULTS

Pre-exercise thermal equilibration

The use of a water-perfused jacket was quite successful in equalising temperatures at ∼37.0°C within the thigh before the exercise (see Fig. 3). This was true for the various muscles except for the hamstrings. At the start of the exercise, the hamstrings had a slightly higher temperature than ∼37.0°C (i.e. 37.3-37.4°C), probably due to the direct contact with, and pressure of the posterior side of the thigh on, the water jacket.

Thigh size

The mean mass of the knee-extensor muscle including the tensor fasciae latae was 2.68 ± 0.46 kg (±s.d.) (Table 1). The mass of m. rectus femoris was ∼0.2-0.3 kg with the other three portions of the quadriceps being of similar weight (0.6-0.8 kg). After 180 s of knee-extension exercise, the active muscle volume is estimated to increase by 0.26 ± 0.04 kg to 2.94 ± 0.50 kg due to fluid gain.

Muscle temperature

At the onset of the voluntary dynamic exercise the temperature in the quadriceps muscles was promptly elevated at all sites, i.e. after the first kick (Fig 4A and Fig 5A, B). However, there were some differences in the magnitude of increase in temperature among the different muscle portions (v.l., r.f. and v.m.) and subjects. A faster increase was observed in rectus femoris with 0.26-0.31°C elevation over the first 30 s compared to the vastus medialis and vastus lateralis which reached 0.14-0.19°C over the same time interval (P < 0.05; Fig. 5A). At the end of the 3 min exercise bout, the temperature rise ranged between 0.89 and 1.03°C in v.l., v.m. and r.f. In two additional experiments in which thermistors were placed in the v.i. portion of the quadriceps femoris muscle and in the tensor fasciae latae, the temperature elevation was similar to that observed in the other portions of the knee-extensor muscles (Fig. 6A). This rendered the basis for using a mean value of temperature in v.l., v.m. and r.f. for the entire knee-extensor muscles. When comparing the rate of rise in muscle temperature, it was observed that knee-extensor muscle temperature increased 0.04 ± 0.01°C during the first 5 s to decline progressively to 0.01 ± 0.01°C during the last 5 s of exercise (P < 0.05). In contrast to the knee-extensor muscles, the temperature in the hamstrings barely increased during the first 120 s of exercise (Figs 5A and 6B), but was at the end elevated by 0.19°C (range 0.13-0.42°C). Half of this elevation occurred during the last 30 s of exercise (Fig. 5A). In the additional experiments, it was observed that anterior thigh subcutaneous tissue temperature and hamstrings temperature in three different sites only increased slightly during exercise (Fig. 6B).

Figure 4. Mean quadriceps muscle temperature, arterial and femoral blood temperature (A) and thigh blood flow (B) for all subjects at rest and during passive exercise, dynamic exercise and recovery.

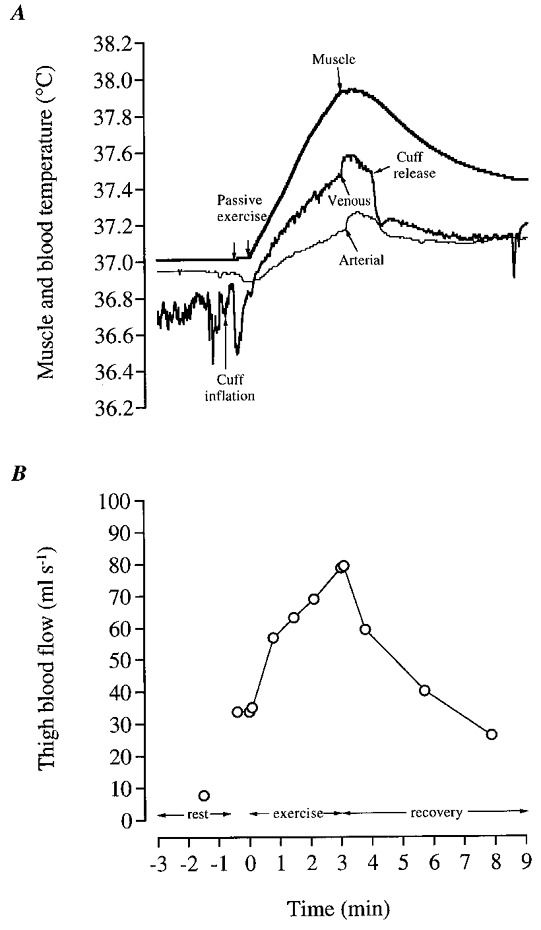
Of note is the observation that the decline in muscle temperature during recovery can be accounted for almost completely by the on-going convective heat removal to the body core.

Figure 5. Temperature and thigh blood flow during intense dynamic knee-extensor exercise.

A, mean values (n = 5) for temperature increases in the rectus femoris (r.f.; 2 sites), vastus medialis (v.m.; 1 site) and vastus lateralis (v.l.; 2 sites) of the quadriceps muscle and hamstrings muscle (1 site; biceps femoris medial) during dynamic knee-extensor exercise. s.e.m. bars are not included for reasons of clarity (s.e.m. range 0.00-0.10 °C; mean s.e.m. values 0.04-0.07 °C for all muscle portions). B, the mean (±s.e.m.) increases (n = 5) in arterial and venous blood temperatures are given from start to end of the exercise. For comparison mean (±s.e.m.) quadriceps, oesophageal (core) and skin temperature are given as well. C, mean (±s.e.m.) values for thigh blood flow during exercise are presented. The mean elevation (n = 5) observed during the exercise fits the polynomial equation:

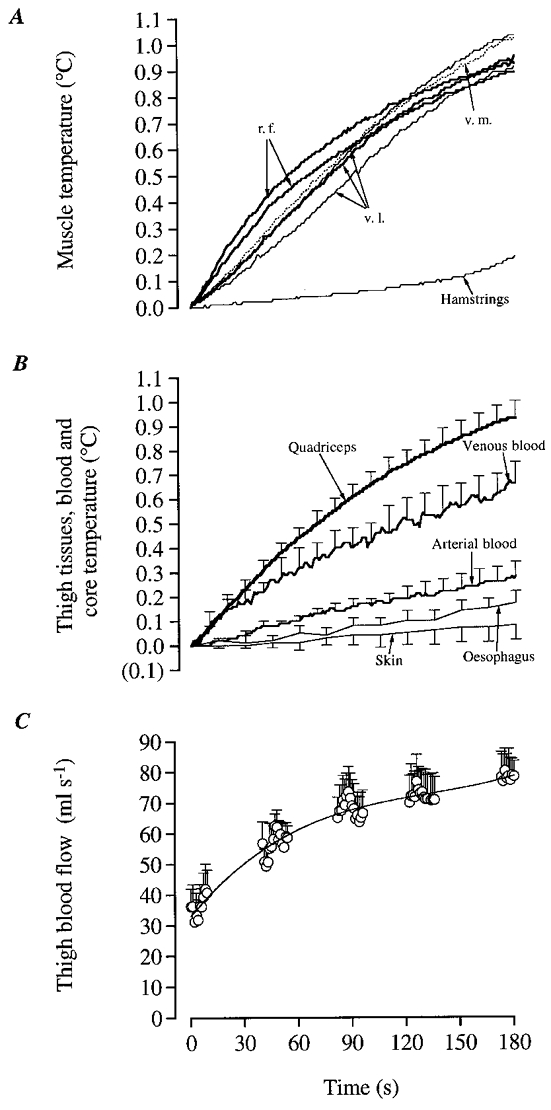
Figure 6. Temperature in various knee-extensor and hamstring muscles as well as subcutaneous tissue during intense knee-extensor exercise.

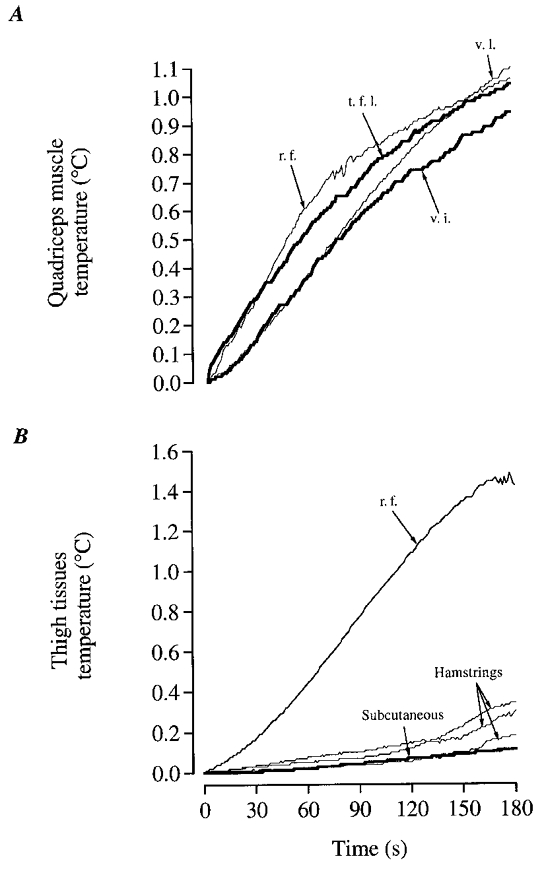
A, mean increases in the two additional experiments when thermistors were placed in vastus intermedius (v.i.) and tensor fasciae latae (t.f.l.) in addition to other knee-extensors. B, data from another additional experiment. Three thermistor probes were placed in different hamstring muscles (biceps femoris, semitendinosus and semimembranosus), and one in the subcutaneous fat (≈5 mm under the skin) next to a thermistor probe placed in the rectus femoris (depth ≈3 cm).

Blood temperature

Femoral venous temperature increased after the first kick with the arterial temperature lagging behind (Figs 4A and 5B). The v-a temperature difference was nil during the first 5 s but increased to 0.09 ± 0.06°C after 15 s (Fig. 5B). Thereafter, v-a temperature difference increased further to reach 0.39 ± 0.04°C at the end of exercise. The absolute values at the termination of exercise for femoral artery and venous blood temperatures were 37.65 ± 0.06 and 37.21 ± 0.08°C, respectively. The rate of rise in oesophageal temperature (T_oes) was only slightly lower than that of femoral artery temperature, reaching a value of 37.1 ± 0.1°C at the end of exercise (Fig. 5B). The observation that the rise in T_oes (index of body core or central blood temperature) throughout exercise was similar to that of arterial temperature, indicates that countercurrent heat exchange between the femoral vein and femoral artery, if it indeed occurred, was very small.

Thigh blood flow

Although the passive acceleration of the leg did not cause any alteration in temperature of the muscle or the arterial blood (Fig. 4A), it increased the thigh blood flow from its resting level of 7.7 ± 1.5 ml s⁻¹ to 33.7 ± 1.2 ml s⁻¹ (Figs 4B and 5C). A fast further elevation occurred in the blood flow during the voluntary exercises reaching 56.7 ± 10.3 ml s⁻¹ at 45 s and 76.7 ± 16 ml s⁻¹ at the end of the exercise.

Heat production

The rate of heat storage in the knee-extensors (H_s) was largest early in the exercise, amounting to 70–80 J s⁻¹ after the first 30 s, after which it gradually declined to 14 ± 10 J s⁻¹ for the last 30 s of exercise (Fig. 7). The rate of heat removal by the blood (H_r) was not significant until after 10 s of exercise, then being 8 ± 10 J s⁻¹. Thereafter, convective heat removal increased progressively to reach a value of 112 ± 14 J s⁻¹ during the last 5 s of exercise (Fig. 7). The decline in H_s was less than the elevation in H_r, especially during the first 60–75 s of exercise, in keeping with an increased heat production during the exercise (Fig. 7). The rate of heat production was 70 ± 10 J s⁻¹ during the first 5 s of the exercise, and approached a value just above 100 J s⁻¹ at 60–75 s (P < 0.05). Thereafter, the increase in the rate of heat production was linear reaching 126 ± 26 J s⁻¹ at the end of the exercise. Therefore, heat production was 77 % higher (P < 0.05) during the last compared to the first 5 s. After 180 s of exercise, total heat storage in the knee-extensors amounted to 9276 J (range 7822–11776 J), whereas total heat removal by the blood amounted to 10993 J (range 6808–20403 J).

Figure 7. Heat production during dynamic knee-extensor exercise.

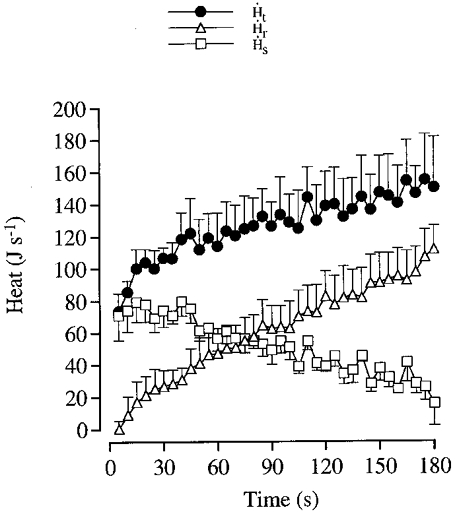
Mean values (±s.e.m.; n = 5) of total heat production (H_t) are depicted for each 5 s period of the exercise as well as its subdivision in terms of storage in the quadriceps muscle (H_s) and removal by the blood from the thigh (H_r).

Additional heat loss

To the above reported heat production should be added the small heat losses by convection, conduction and convection via the lymph flow (H_a). The amount of heat conducted to the skin of the anterior thigh during exercise was estimated to range from ∼2 J s⁻¹ during the first 5 s of exercise to 6 J s⁻¹ during the last 5 s of exercise, with almost no difference among subjects. The corresponding calculation for the posterior portion of the thigh demonstrated that it can be neglected due to the temperatures in the hamstring and the skin being similar. With no temperature gradient, heat transfer to the thigh skin through convection was estimated to be zero at the beginning of exercise, increasing progressively throughout exercise to a value of 12 ± 4 J s⁻¹. Heat transfer to the body core via the lymph might amount to a maximum of 6 J s⁻¹. Therefore, during the first 5 s of exercise, H_a was negligible (< 2 J s⁻¹), and increased throughout exercise to a value of 24 J s⁻¹ (range 6–48 J s⁻¹) at 180 s. The total value for H_a is 2446 J (range 334–3096 J), which represents a maximum of 10 % of H_t (22726 J; range 16612–33878 J) or 6 % of E_t (37652 J; range 31650–44769 J). When including H_a, H_t during the last 5 s of exercise was twice as high as that observed during the first 5 s of exercise (P < 0.05) (Fig. 7), with half of the increase occurring during the first 38 ± 12 s.

Mechanical power output

During the first 30 s of exercise the mean power output was 83 W (range 58–114 W). During the subsequent 90 s, power output was within 1 % of this level (Fig. 8C). Overall there was a trend for a reduction in power output which was due to a progressive decline in kicking frequency from 1.08 ± 0.03 s⁻¹ during the first 5 s to 0.96 ± 0.03 s⁻¹ during the last 5 s (P < 0.05; Fig. 8A and B). After 120 s of exercise, power output dropped somewhat more in two subjects. This coincided with a progressive increase in the work performed by the hamstrings as evidenced by the force tracing.

Figure 8. Mechanical power output during dynamic knee-extensor exercise.

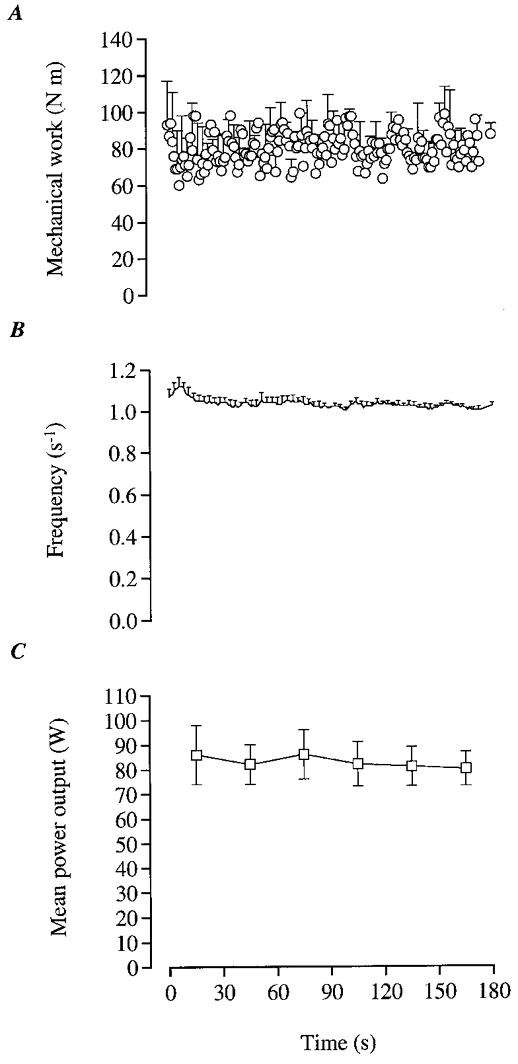
Mean values for mechanical work per kick (A) and the kicking frequency (B) as well as the mean power output over 30 s intervals (C) for n = 5.

Mechanical efficiency

With heat production doubling over the 180 s of the exercise while power output was in essence constant (Fig. 8C), the estimated mechanical efficiency declined from an initial value of 53 ± 6 % to 36 ± 5 % at the end of exercise (P < 0.05).

Oxygen consumption and lactate release

Thigh V̇_O2 increased in a curvilinear fashion from 0.055 ± 0.023 mmol s⁻¹ immediately before exercise to 0.464 ± 0.035 mmol s⁻¹ at the end of exercise, with one-half of the increase occurring during the first 28 ± 3 s (Fig. 9A). Net lactate release from the thigh increased from 0.01 ± 0.01 mmol s⁻¹ immediately before exercise to 0.15 ± 0.03 mmol s⁻¹ (P < 0.05) after 70 s and 0.21 ± 0.02 mmol s⁻¹ at the end of exercise (P < 0.05; Fig. 9B).

Figure 9. Oxygen consumption (A) and lactate release (B) during exercise.

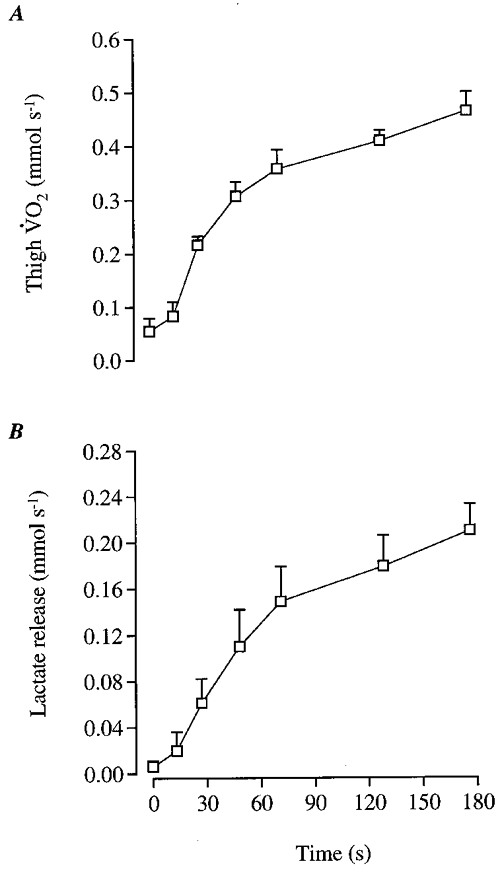
Data are means ±s.e.m. for 5 subjects.

DISCUSSION

The major finding of this study was that heat production by contracting human skeletal muscle doubled over 3 min of intense dynamic exercise at essentially constant power output. Half of this increase in rate of heat production occurred during the first 38 s of exercise. This elevated heat production in contracting skeletal muscle appears to be tightly coupled with changes in heat liberation during ATP production in the metabolic reactions involved early in exercise.

Measurement in man of heat production in dynamically contracting muscle with a free blood flow is complex, requiring a high time resolution and precise measurements. This was accomplished in this study and one major finding was that after 60 s of exercise, when aerobic metabolism provided 82–89 % of the ATP resynthesis, the match between total energy turnover (sum of rate of heat production and power output) and total metabolic input (sum of net PCr and net ATP hydrolysis, glycolysis and oxidative phosphorylation) was quite close. However, the critical time period is the very first minute of exercise where the rate of heat production is much smaller than in the later phase of the exercise bout. Multiple thermistors were placed in all major muscle portions developing the force as well as in one adjacent inactive muscle and temperature was recorded continuously. As evidenced in Figs 4A and 5A, an elevation in temperature in the active muscle can be observed during the first few contractions (1-3 s). The observation that passive exercise did not elevate quadriceps muscle temperature and that knee-extensor exercise did not increase hamstring temperature early in exercise indicates that movement friction of the thermistor did not add to the heat gain in the muscle. Therefore, the precise muscle temperature and volume measurements in the present study provided highly valid estimates of heat accumulated in the muscle, including the initial phase of the exercise.

Some uncertainty, however, is involved in the estimation of the heat removal from the muscle by the blood flow. The inflow and outflow temperatures are obtained with the same time resolution and sensitivity as the measurements of the changes in muscle temperature and have an error of ∼0.01°C. The largest uncertainty in the measurement of heat removal by the blood is the blood flow determinations. The thermodilution technique does not allow for continuous measurements. Thus, thigh blood flow in the present study was measured for 20 s every ∼30 s and mean thigh blood flow during each 5 s interval was estimated using a curve-fitting polynomial model. The present rate of rise and absolute values in thigh blood flow correspond closely with continuous Doppler measurements using the same exercise model and exercise intensity (Rådegran & Saltin, 1998). In this study, a rapid increase in thigh blood flow was found with the first contractions. However, in this time interval the v-a temperature difference was very small, indicating that heat removal by the blood was negligible and that even a large error in blood flow measurement would only constitute a very small error in the estimation of convective heat removal. Furthermore, in the present experimental conditions with no or small temperature gradients between muscle and skin during the first 30 s of exercise, the additional heat loss to the skin by convection and conductance as well as to the body core by lymph drainage amounted to < 3 % of total heat production. Taken together, these findings indicate that most (> 90 %) of the heat produced during the first 10 s of exercise accumulated in the contracting muscles whereas, at the end of exercise, most of the heat produced was transported by the blood to the body core (see Fig. 7). Therefore, it is very unlikely that the heat accounting during the initial phase of the exercise bout is missing significant amounts of heat and vice versa for the later part of the exercise bout. Consequently, since work output is maintained nearly constant throughout the exercise, the overall efficiency of the muscle work performed is decreasing proportionally to the additional heat output.

There are several possible mechanisms explaining our observation at the onset of exercise of progressive increasing rate of heat production during generally maintained power output. One likely explanation is based on Wilkie's work in the 1960 s (Wilkie, 1968) and that of Woledge & Reilly (1988). They studied the heat produced during muscle contraction when the ATP resynthesis was powered by a net breakdown of PCr and found that the immediate heat liberated was only ∼35 kJ per mole of ATP used due to the near-equilibrium state of the creatine kinase reaction (Wilkie, 1968; Woledge & Reilly, 1988) as compared to the ∼72 kJ per mole of ATP used when ATP is resynthesised via oxidation (Curtin & Woledge, 1978). ATP resynthesis from the anaerobic usage of glycogen (glucose) appears to have an intermediate value for heat liberation compared to PCr breakdown and aerobic metabolism (i.e. ∼65 kJ per mole of ATP used; Curtin & Woledge, 1978). We measured thigh V̇_O2 and lactate release and observed that oxidation accounted for ∼30 % of the total energy turnover during the first 30 s of exercise, increasing to > 82 % after 60 s of exercise. PCr degradation and lactate accumulation in muscle were not assessed in this study; however, these parameters have been measured repeatedly in the same model with similar work rate and exercise regimen in other studies (Bangsbo et al. 1990; Sinclair et al. 1999). Based upon measured absolute values in parallel studies and well-documented PCr and lactate kinetics (Gollnick & Hermansen, 1973; Meyer, 1988; Bangsbo et al. 1990; Sinclair et al. 1999), a likely model can be described for the energy contribution of these pathways (see Table 2). Accordingly, PCr and anaerobic glycogenolysis would account for 15 and 25 % of the total energy turnover, respectively, and 20 and 33 % of the total ATP production, respectively, during the first 30 s of exercise. During this 30 s interval the anaerobic processes therefore provided approximately two-thirds of the ATP resynthesis (Table 2; see also Fig. 10 for an indirect account of the anaerobic heat liberation). The observation that the metabolic rate of heat liberation was 53–68 % higher in all the 30 s intervals following the initial 30 s of exercise strongly suggests lesser heat liberation per ATP provided by ATP-PCr hydrolysis and glycogenolysis compared to ATP provided by oxidative phosphorylation.

Table 2. Muscle energetics during 3 min of intense dynamic kneeextension exercise.

	Time interval (s)
Energy source	0–30	30–60	60–90	90–120	120–150	150–180	0–180
(1) Oxygen consumption (J s−1)	57.6 ± 7.5	132.8 ± 9.7	168.0 ± 16.9	176.8 ± 22.0	182.0 ± 22.6	196.7 ± 19.6	152.3 ± 15.3
(2) Net PCr hydrolysis (J s−1)a	27.0	15.0	6.3	1.7	0.8	0.3	8.6
(3) Net ATP hydrolysis (J s−1)b	0.4	0.6	0.6	0.6	0.6	0.6	0.6
(4) Lactate accumulation (J s−1)a	40.6	64.3	12.6	7.9	6.3	5.0	21.7
(5) Lactate release (J s−1)	4.1 ± 1.2	11.4 ± 2.1	17.1 ± 2.6	19.0 ± 2.9	18.2 ± 2.7	18.4 ± 2.4	14.7 ± 2.2
Σ(1–5) Total metabolic input (J s−1)	133.8	224.1	204.6	206.0	207.9	221.0	197.9
Total energy turnover (J s−1)c	181.9 ± 13.6	197.2 ± 16.3	211.0 ± 17.4	216.0 ± 21.0	220.8 ± 20.4	228.1 ± 23.2	209.2 ± 15.3

Data represent mean rates for 30 s time intervals and the overall mean for 180 s. Measured data are depicted as means ±s.e.m. for 5 subjects. ^aNet PCr hydrolysis and lactate accumulation estimations are based on the initial and final values observed in biopsy samples from vastus lateralis obtained in parallel studies with the same experimental protocol (J. Bangsbo, P. Krustrup, J. Gonz.alezález-Alonso & B. Saltin, unpublished; ΔPCr 16.3 mmol (kg wet wt)⁻¹ and Δlactate 22.3 mmol (kg wet wt)⁻¹; see also Bangsbo et al. 1990, for similar measurements) and the kinetics described by Gollnick & Hermansen (Fig. 3, p. 12; 1973) and Sinclair et al. (1999). Heat produced per mole of ATP used was assumed to be 35 kJ for ATP and PCr hydrolysis, 65 kJ for glycogenolysis and 72 kJ for oxidation (Curtin & Woledge, 1978; Woledge & Reilly, 1988). P:O ratio was assumed to be constant throughout exercise and equal to theoretical 3.0. Total metabolic input was calculated as the sum of the energy input from oxygen consumption, net ATP and net PCr hydrolysis and lactate production. ^bNet ATP hydrolysis estimation (ΔATP 1.1 mmol (kg wet wt)⁻¹) is based on data from Hellsten et al. (1999). ^cTotal energy turnover was calculated by summing power output and rate of heat production.

Figure 10. Total and aerobic energy turnover during dynamic exercise.

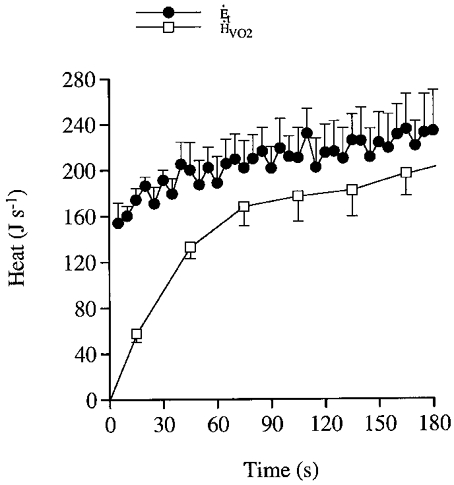
Total energy turnover (E_t) vs. aerobic heat liberation (H_VO2) during 180 s of exercise. Note that the difference in heat between E_t and H_VO2 is accounted for by anaerobic heat liberation.

Oxygen uptake of the thigh gradually increased throughout the exercise period. However, it did not reach a plateau value, although the work rate and thus energy turnover was in each individual above the aerobic capacity. To what extent this continuous elevation in oxygen uptake is due to (i) altered motor unit and fibre type recruitment, (ii) declining mitochondrial P:O ratio, (iii) reduced free energy (ΔG) from the hydrolysis of ATP, (iv) declining crossbridge cycling efficiency, and (v) increasing non-crossbridge ATPase activity (Crow & Kushmerick, 1982; Curtin & Woledge, 1991, 1993; Barclay et al. 1993; Willis & Jackman, 1994; Barclay, 1996) requires further investigations. The possibility cannot be excluded that one or all five of these mechanisms could play a role. The knee-extensor muscles are mixed with regard to their fibre type distribution, usually containing similar amounts of type I and type II muscle fibres (Saltin et al. 1977; Lexell et al. 1983). As the efficiency of type I muscle fibres is likely to be greater than that of type II fibres when contraction velocity is < 25 % of the maximal value (Crow & Kushmerick, 1982; Curtin & Woledge, 1991, 1993; Aagaard et al. 1994; Barclay, 1996), the question arises as to whether a shift in fibre recruitment and/or enhanced motor unit recruitment contributed to the observed increases in oxygen uptake and heat production. Based on T₂-weighted MRI images obtained before and after exercise and the glycogen depletion pattern, all knee-extensor muscles are engaged when the intensity of contractions is high (Bangsbo et al. 1992; Richardson et al. 1998; Ray & Dudley, 1998). The rapid increase in muscle temperature in all the locations in the knee-extensor muscles after the first contractions is consistent with this notion. The glycogen depletion pattern reveals a recruitment of all fibre types at this intensity of exercise (Bangsbo et al. 1992). Thus, it is most likely that the relative role of type I fibres is enhanced rather than reduced in the present experiment. Therefore, it appears unlikely that an altered motor unit recruitment pattern influenced to a significant extent the slow increase in muscle V̇_O2 and heat production during exercise.

The free energy (ΔG) for ATP hydrolysis will decrease from rest to maximal exercise, since the contribution from the RTlnK′ term of the equation ΔG =ΔG°+RTlnK′ (where ΔG° is the free energy under standard conditions), will decline with the increase in free ADP, P_i and H⁺, occurring during contraction. It may be estimated that the K′ will typically increase by a factor of 300 or more, comparing resting conditions and end-exercise conditions (see e.g. Ratkevicius et al. 1998), corresponding to a decrease in ΔG by about 15 kJ mol⁻¹. Whether or not the P:O ratio declines with intense exercise is unknown at present; however, in vitro measurements do not suggest that this is the case (Hinckle & Yu, 1979; Willis & Jackman, 1994). Whether a decreasing efficiency of the crossbridge coupling during maximal exercise occurs is also unknown. Judging from NMR measurements of the cost of contraction (moles of ATP hydrolysis per watt of power output) under anaerobic conditions, this does not appear to be the case (Y. Nakagawa, A. Ratkevicius, M. Mizuno & B. Quistorff, unpublished observations). Finally, the possibility cannot be excluded that the non-crossbridge ATPase activity could contribute to the observed elevated heat production (Barclay et al. 1993; Barclay, 1996).

In conclusion, the total skeletal muscle heat production in humans performing intense work was accurately quantified during dynamic knee-extension exercise by summing: (a) heat storage in the contracting muscles, (b) heat removal to the body core by the circulation, and (c) heat release to the skin and environment. At constant power output, heat production increased progressively throughout exercise. This meant that heat production was ∼100 % larger during the final as compared to the initial 5 s of exercise, with half of the increase occurring during the first 38 s. This allows the comparison of metabolic energy input with heat production. Concomitant measurements of aerobic energy metabolism and net lactate release supplemented with data on PCr depletion and muscle lactate accumulation in similar exercise made possible the accurate estimation of the magnitude of energy yield from these three metabolic pathways throughout exercise. The match was found to be very close provided correct values for the heat liberated from the breakdown of PCr, anaerobic glycolysis and oxidative phosphorylation were applied.