Abstract
The Hoffmann (H-) reflex is an electrical analogue of the monosynaptic stretch reflex, elicited by bypassing the muscle spindle and directly stimulating the afferent nerve. Studying H-reflex modulation provides insight into how the nervous system centrally modulates stretch reflex responses.
A common measure of H-reflex gain is the slope of the relationship between H-reflex amplitude and EMG amplitude. To examine soleus H-reflex gain across a range of EMG levels during human locomotion, we used simulated reduced gravity to reduce muscle activity. We hypothesised that H-reflex gain would be independent of gravity level.
We recorded EMG from eight subjects walking (1.25 m s−1) and running (3.0 m s−1) at four gravity levels (1.0, 0.75, 0.5 and 0.25 G (Earth gravity)). We normalised the stimulus M-wave and resulting H-reflex to the maximal M-wave amplitude (Mmax) elicited throughout the stride to correct for movement of stimulus and recording electrodes relative to nerve and muscle fibres.
Peak soleus EMG amplitude decreased by ≈30% for walking and for running over the fourfold change in gravity. As hypothesised, slopes of linear regressions fitted to H-reflex versus EMG data were independent of gravity for walking and running (ANOVA, P > 0.8). The slopes were also independent of gait (P > 0.6), contrary to previous studies. Walking had a greater y-intercept (19.9%Mmax) than running (-2.5%Mmax; P < 0.001). At all levels of EMG, walking H-reflex amplitudes were higher than running H-reflex amplitudes by a constant amount.
We conclude that the nervous system adjusts H-reflex threshold but not H-reflex gain between walking and running. These findings provide insight into potential neural mechanisms responsible for spinal modulation of the stretch reflex during human locomotion.
The H-reflex is similar to the monosynaptic stretch reflex, but is evoked by electrically stimulating the afferent nerve instead of mechanically stretching the muscle spindle. It has been extensively studied in humans, especially regarding its modulation for different tasks and different phases of a task (for recent reviews see Brooke et al. 1997; Capaday, 1997; Zehr & Stein, 1999). Studying the H-reflex instead of the stretch reflex has both limitations and advantages. One limitation is that it does not reflect changes in fusimotor activation or musculotendon mechanics during movement because it bypasses the muscle spindle. As a result, it is possible that H-reflex modulation is not always behaviourally relevant (Andersen & Sinkjaer, 1999; Kearney et al. 1999). However, circumventing changes in fusimotor activation and musculotendon mechanics can be advantageous because it allows for an examination of spinally mediated reflex modulation.
Previous studies on reflex modulation during human locomotion have concluded that soleus H-reflex gain is greater for walking than for running (Capaday & Stein, 1987a; Edamura et al. 1991). Measurements of H-reflex gain are not calculated directly but are inferred based on an operational definition of reflex gain. H-reflex gain is calculated as the slope of the relation between H-reflex amplitude and electromyography (EMG) amplitude (Capaday & Stein, 1986, 1987a; Edamura et al. 1991). During a given task, a higher level of EMG will coincide with a higher H-reflex amplitude because of an increase in excitability of the motor neuron pool at the same reflex gain (Pierrot-Deseilligny, 1997). Comparing slopes for different tasks is a way to compare the increase in H-reflex amplitude for a given increase in EMG amplitude. Past studies that have examined H-reflex gain in this manner have found that walking has a much higher slope than running even though both gaits have y-intercepts at the origin (Capaday & Stein, 1987a; Edamura et al. 1991). This suggests that there is a greater difference in reflex responses between walking and running when muscle activity is high. When muscle activity is low, walking and running have similar reflex responses.
A limitation of past studies has been the inability to make H-reflex measurements over a range of EMG levels during locomotion. It is advantageous to make H-reflex measurements over a large range of EMG levels because the accuracy of gain calculations increases with the range of muscle activation levels. Furthermore, measuring H-reflex amplitudes at different muscle activation levels is necessary to test the hypothesis that H-reflex gain is independent of muscle recruitment during locomotion. Edamura et al. (1991) attempted to increase the range of muscle activation levels for their H-reflex measurements by examining humans walking and running at multiple speeds. However, in addition to increasing muscle activation, increasing locomotion speed also increased H-reflex inhibition (Edamura et al. 1991; Brooke et al. 1997). No study has been able to examine human H-reflex gain for different levels of muscle recruitment at the same locomotion speed.
The purpose of this study was to assess soleus H-reflex gain during walking and running over a range of muscle activity levels. We used simulated reduced gravity to reduce soleus muscle recruitment during human locomotion. At lower levels of gravity, soleus EMG would presumably be less than soleus EMG at normal earth gravity due to reduced body weight load. This protocol allowed us to examine the relationship between H-reflex amplitude and EMG amplitude at the same locomotion speed but lower muscle activation levels. We hypothesised that soleus H-reflex gain would be independent of gravity level. We based this hypothesis on the observation that changes in muscle activity level do not directly alter reflex gain during non-locomotory tasks (Gottlieb et al. 1970; Capaday, 1997; Pierrot-Deseilligny, 1997). Thus, changes in muscle activity level should not directly alter reflex gain during locomotion.
METHODS
Subjects
Eight healthy male subjects between the ages of 21 and 42 years participated in this study (mean body mass 76.2 kg, standard deviation 6.3 kg). The local committee for the protection of human subjects gave approval for the study, the procedures conformed to The Declaration of Helsinki, and subjects provided written consent.
Experimental design
All subjects walked at 1.25 m s−1 and ran at 3.0 m s−1 on a motorised treadmill at four gravity levels (1.0, 0.75, 0.50 and 0.25 G). We randomised the order of gravity levels for each subject. Subjects walked before they ran for half of the levels of gravity and ran before they walked for the other half. All subjects walked or ran at each gravity level for a minimum of 2 min before we collected data. Previous studies have shown that this is sufficient time to familiarise subjects with the reduced gravity simulator and produce reliable and consistent locomotion mechanics (Donelan & Kram, 1997). Each subject completed all trials in a single recording session.
Reduced gravity simulator
To simulate reduced gravity on the subject’s centre of mass, a series of rubber springs provided a nearly constant upward force to each subject’s trunk via a modified rock climbing harness (Fig. 1). We adjusted the level of gravity by turning a winch attached to the opposite end of the springs, stretching the springs and increasing the upward force on the subject. The support force fluctuations were very small compared to the level of simulated gravity because the total displacement of the springs from resting length was much larger than the vertical movements of the subjects during locomotion. A strain gauge force transducer in series with the springs revealed that the support force fluctuations during each stride were within ±0.03 G for all support levels. Thus, although the simulator did not affect the gravity level acting on the swinging limbs, it did provide a reasonable approximation of reduced gravity on the centre of mass. Our hypothesis focuses on H-reflex gain during the stance phase of locomotion, so the lack of reduced gravity on the swinging limbs is not a major concern for our study. More detailed descriptions of this type of reduced gravity simulator can be found in a number of previous studies (He et al. 1991; Farley & McMahon, 1992; Donelan & Kram, 1997, 2000; Kram et al. 1997; Griffin et al. 1999; Chang et al. 2000). The compliant support mechanism for the reduced gravity simulator did not restrict the vertical position of the subject and allowed normal centre of mass dynamics as subjects walked and ran (He et al. 1991; Donelan & Kram, 1997, 2000; Griffin et al. 1999). Biomechanical and metabolic results from this type of simulator are similar to those found using horizontal suspension (Davis & Cavanagh, 1993), underwater immersion (Newman et al. 1994), and parabolic flight (Cavagna et al. 1998) to simulate reduced gravity.
Figure 1. Reduced gravity simulator.
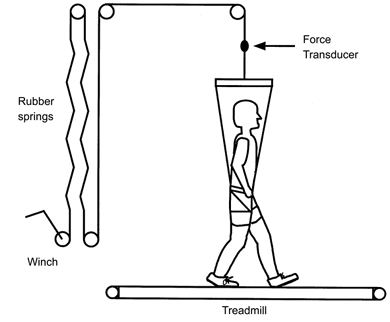
The subjects wore a modified rock climbing harness attached to a series of rubber springs via a metal cable and multiple pulleys. Upward support force fluctuations were small within each stride (± 0.03 G).
Foot switches
Two electrical foot switches were taped to the bottom of each subject’s shoe, one under the forefoot and one under the heel. The heel signal provided a consistent signal indicating the instant of foot contact at the beginning of stance, allowing us to synchronise data for multiple strides. Decreased plantar pressure during the second half of stance at low gravity levels often caused the foot switch under the forefoot to signal the end of contact before the foot was actually off the treadmill. As a result, we could not directly measure the ground contact time for subjects at all gravity levels. Multiple studies with similar simulators have found that ground contact time changes only slightly over this range of gravity levels (He et al. 1991; Farley & McMahon, 1992; Donelan & Kram, 1997). Duty factors for humans at these speeds and gravity levels are typically around 0.6 for walking and 0.4 (1.0 G) to 0.3 (0.25 G) for running (He et al. 1991; Donelan & Kram, 1997; Chang et al. 2000). The duty factor for running decreases under reduced gravity because ground contact time slightly decreases and stride period slightly increases (He et al. 1991; Farley & McMahon, 1992; Chang et al. 2000).
Goniometer
We placed a twin axis electrogoniometer (Penny & Giles M-series, 1000 Hz recording frequency) on the lateral side of the ankle joint to monitor ankle position for five of the eight subjects. The goniometer broke during testing of the sixth subject. After data collection, we normalised individual strides of ankle joint position data to total stride time and averaged seven to 10 consecutive strides for each gravity and gait. We low-pass filtered the averaged position data using a fourth-order zero-lag Butterworth filter with an 8 Hz cut-off frequency. We calculated the amount of ankle dorsiflexion during stance by subtracting the minimum ankle angle during mid-stance (most dorsiflexed) from the initial peak ankle angle at beginning of stance (most plantarflexed). We also calculated the amount of plantarflexion by subtracting the minimum ankle angle during mid-stance (most dorsiflexed) from the following most plantarflexed ankle angle.
Electromyography
To examine the effect of reduced gravity on muscle activation patterns, we placed bipolar surface electrodes on four leg muscles (soleus, medial gastrocnemius, tibialis anterior and vastus lateralis). The inter-electrode distance was 2 cm. Prior to electrode placement, we shaved the skin and prepared the electrode sites with fine sandpaper and alcohol. We placed the electrodes over the approximate centre of each muscle belly for all muscles except the soleus. For the soleus, we placed the electrodes over the distal third of the soleus muscle below the insertion of the gastrocnemius muscle onto the Achilles’ tendon in order to selectively record from the soleus. We connected small custom-built preamplifiers to the electrodes and wrapped elastic bandages around each subject’s leg to minimise movement artifacts. Each subject’s electrodes remained in place for all trials without being moved or replaced. The custom-built EMG amplifiers (gain 1000) had a frequency response of 20 Hz to 10 Hz. A personal computer sampled each EMG channel at 1000 Hz via an analog-to-digital board. We verified that cross-talk was negligible by visual inspection of the EMG signals. A previous study describes the EMG equipment and collection methods in more detail (Simonsen et al. 1995).
After data collection, we processed the EMG signals by full wave rectification, normalisation to total stride time, and averaging seven to 10 consecutive strides for each gravity level and gait. We also used a fourth-order zero-lag Butterworth low-pass filter with a cut-off frequency of 10 Hz on the rectified averaged EMG to form linear envelopes. As a measure of relative activity between gravity levels, we took the mean of the rectified averaged EMG over the stride period (mEMG). We normalised mean EMG for each subject to the maximum value recorded for that subject and muscle at all gravity levels regardless of gait to reduce intersubject variability (Yang & Winter, 1984).
H-reflex measurements
We elicited the soleus H-reflex by stimulating the tibial nerve with an AgCl cathode on the skin in the popliteal fossa (Medicotest Q-10-A) and a 4 cm diameter anode placed over the patella. We located the optimum site of nerve stimulation with a hand-held electrode using the criterion that soleus Ia afferents could be selectively stimulated at low stimulus intensities. The stimulus was a 1 ms square pulse delivered by a custom-built constant current stimulator. We measured the maximal evoked muscle response (maximal M-wave amplitude: Mmax) and the maximal H-reflex amplitude while subjects stood motionless.
During locomotion, a custom-written computer program controlled the electrical stimulator and recorded the resulting H-reflex amplitudes (Dyhre-Poulsen et al. 1991). The program sent a stimulus approximately every 2 s so that it was slightly out of phase with the gait cycle. This simulation interval dispersed the stimuli randomly over the gait cycle. We divided the gait cycle into 16 equal time slices and averaged the H-reflex measurements within each time slice to match previous H-reflex studies (Capaday & Stein, 1987a; Edamura et al. 1991; Simonsen & Dyhre-Poulsen, 1999). There were a minimum of four, and typically eight to twelve measurements, averaged together within each time slice.
We measured the peak-to-peak amplitude of the M-wave produced by the stimulus to monitor the effective stimulus strength. The stimulating electrode can move relative to the nerve so it is necessary to monitor M-wave amplitude to ensure that a constant stimulus intensity reaches the nerve. The computer calculated the stimulus intensity necessary to evoke an M-wave that was 25% of Mmax based on M-wave amplitudes from previous stimuli. As the muscle contracts during locomotion, the muscle fibres move relative to the recording electrodes. This relative movement can change the magnitude of Mmax throughout a stride of locomotion (Simonsen & Dyhre-Poulsen, 1999). As a result, stimulating to produce a constant absolute M-wave amplitude results in the recruitment of fewer motor axons if Mmax has increased. In contrast, stimulating to produce an M-wave that is a constant percentage of Mmax results in the recruitment of approximately the same number of motor axons. Recruiting approximately the same number of motor axons indicates that stimulus intensity to the afferent nerve is also constant.
The electrical stimulator delivered a second supramaximal stimulus 60 ms after the first stimulus in order to measure Mmax throughout the gait cycle. We determined supramaximal stimulus intensity by doubling the stimulus required to produce Mmax during quiet standing. Additional standing tests on one of the subjects demonstrated that this stimulus intensity was more than high enough to yield Mmax even with the leg flexed throughout the range of motion normally occurring during locomotion. Furthermore, the absence of an H-reflex after the second M-wave during all locomotion tests also verified that we had successfully elicited Mmax. If the stimulus did not produce a true Mmax, the H-reflex would not have been occluded from antidromic nerve activity. The program calculated the ratio of the M-wave immediately preceding the H-reflex to the Mmax immediately following the H-reflex. We only accepted H-reflex measurements where the M-wave was 25 ± 10% of the corresponding Mmax.
Although a measurement of Mmax at the exact instant of the H-reflex measurement would have been preferable to a measurement 60 ms later, it is not possible to stimulate both at a submaximal level and a supramaximal level at the same point in time. Offline analysis of H-reflex data where the Mmax measurement had been shifted 60 ms earlier produced no detectable differences in H-reflex modulation (Simonsen & Dyhre-Poulsen, 1999). We also re-analysed a sample of the H-reflex data from this study by shifting Mmax values one time slice and using that Mmax value to select M-waves that were 25%Mmax. These re-calculated H-reflex mean amplitudes were always within 1 standard deviation of the previous H-reflex means. Repeated supramaximal stimulation can depress Mmax and H-reflex amplitudes when measured during rest (Crone et al. 1999), but this depression does not occur during locomotion as demonstrated by comparisons of H-reflex data collected with and without a second supramaximal stimulus (Simonsen & Dyhre-Poulsen, 1999). In addition, we compared H-reflex amplitudes measured with and without a second supramaximal stimulus in one subject at different gravity levels and found no effect of the second supramaximal stimulus.
The computer program used a ‘learning algorithm’ to adjust the stimulus intensity during locomotion (Simonsen & Dyhre-Poulsen, 1999). At each stimulation, the computer program measured Mmax and calculated the target M-wave amplitude (i.e. 25%Mmax). If the M-wave collected immediately before the Mmax measurement was not within 25 ± 10% of the corresponding Mmax then the program adjusted the stimulus intensity either up or down for the next H-reflex measurement taken during that time slice of the gait cycle. This program allowed us to adjust stimulus strength based on fluctuations in Mmax during the gait cycle and avoid subject fatigue by reducing total experimental time.
H-reflex gain calculations
We calculated H-reflex gain in a manner similar to past studies (Capaday & Stein, 1987a; Edamura et al. 1991) with two changes. For each of the 16 time slices during the gait cycle, we calculated the mean of the rectified averaged EMG during that time slice. Subsequently, we plotted a data point for each time slice with the mean H-reflex on the y-axis and the mean rectified averaged EMG on the x-axis. We fitted a linear least-squares regression to the data points at each gravity level and gait for each subject. The first difference from past studies is that we normalised H-reflex amplitudes and mean EMG measurements to the Mmax for that time slice. This corrected for changes in H-reflex and EMG values due to movement of the muscle fibres relative to the recording electrodes (Gerilovsky et al. 1989; Simonsen & Dyhre-Poulsen, 1999). As a result of the normalisation, the slope of the H-reflex versus EMG linear regression was unitless. This slope served as our measure of H-reflex gain. We also compared the y-intercept of the linear regressions between gaits and gravity levels.
The second difference concerns the exclusion of data with low level EMG amplitude from the linear regressions. The relationship between H-reflex amplitude and EMG level can only be defined unambiguously when there is a non-zero level of EMG (Edamura et al. 1991). As a result, we set the following guidelines for including data points in the linear regressions. We included data points starting with the first time slice at the beginning of the stance phase. We stopped including time slice data points when the EMG time slice value fell below three times the lowest EMG time slice value for that subject and condition. Using four times the lowest EMG time slice value as the cutoff criterion produced virtually identical results due to the sharp decrease in soleus EMG at the end of stance (see Fig. 7). Using twice the lowest EMG time slice value as the cutoff would have included many low level EMG data points that were similar in magnitude to EMG levels from time slices 10-15 (i.e. during the swing phase). The EMG levels in time slices 10-15 presumably represent background noise in the EMG because the soleus muscle is not active during the major part of swing (see Fig. 7). Thus, we rejected twice the lowest EMG time slice value as the cutoff criterion because it would have included many EMG data points with magnitudes similar to background noise levels. Although the noise level did not change substantially between different conditions, using a condition-specific cutoff corrected for any inter-trial variation in background noise.
Figure 7. Mean rectified EMG amplitudes for soleus during walking and running at each gravity level for all subjects.
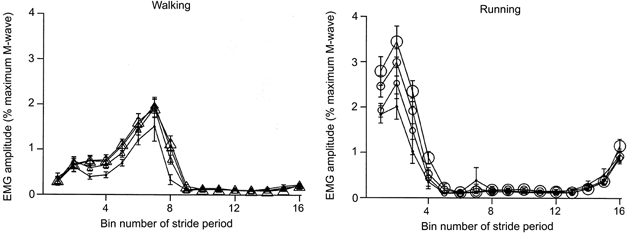
We broke the stride period into 16 equal bins. Each data point represents the average for a bin. The size of the symbol reflects the gravity level (i.e. largest symbols are 1.0 G, smallest symbols are 0.25 G). Bin number 1 is the first time slice after heel strike. Error bars are standard errors of the mean.
Our choice of cutoff criterion is different from past studies as other researchers have included data points with EMG values close to zero (see Fig. 4 in Capaday & Stein, 1987a and Fig. 2 in Edamura et al. 1991). This could have potentially forced the linear regressions through the origin and altered the calculations of H-reflex gain (i.e. linear regression slope). The choice of the present cutoff criterion prevents this effect without altering the calculated slope between H-reflex amplitude and EMG at higher levels of muscle activation.
Figure 4. Rectified averaged low-pass-filtered EMG (cutoff frequency 10 Hz) for a typical subject walking (A) and running (B) at 1.0 and 0.25 G.
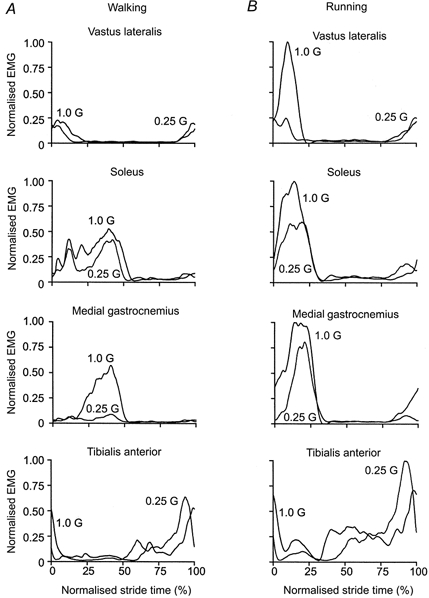
Heel strike is at 0% normalised stride time. A, during walking, vastus lateralis activity was very similar for both gravity levels. The 75% decrease in gravity resulted in only a slight reduction in soleus activity but a substantial reduction in medial gastrocnemius activity. Tibialis anterior activity was similar for both gravity levels. B, during running, vastus lateralis activity was much lower at 0.25 G than at 1.0 G. Soleus and medial gastrocnemius activity showed similar reductions for the 75% decrease in gravity level. Although this subject demonstrated a slight increase in tibialis anterior activity under simulated reduced gravity, tibialis anterior activity remained constant for most subjects.
Figure 2. Typical ankle movement patterns for a single subject walking and running at 1.0 G and 0.25 G.
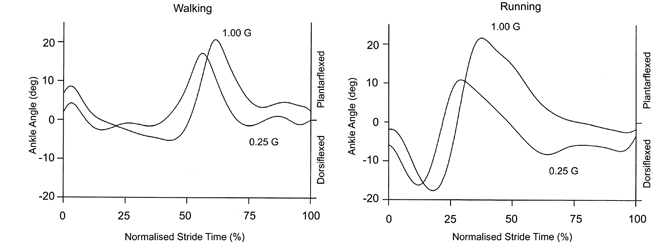
The ankle movement patterns were very similar at all gravity levels, but there was slightly less ankle dorsiflexion during the first part of the stance phase under simulated reduced gravity. We defined 0 deg as each subject’s standing posture. The curves represent the average of seven to 10 strides at each condition. Heel strike is at 0% normalised stride time.
The exclusion of data points that have EMG values close to zero is necessary because EMG does not necessarily reflect the excitability of the motor neuron pool when there is no muscle activity. The mean value of rectified EMG reflects the overall excitability of the motor neuron pool when the muscle is active (Capaday, 1997). When the muscle is inactive, sub-threshold changes in the resting potential of the motor neuron pool cannot be detected through EMG. For example, increased postsynaptic inhibition of an inactive motor neuron pool would decrease the resting potential and, as a result, decrease the amplitude of the H-reflex (Capaday & Stein, 1987b, 1989). Because the muscle is inactive, the change in resting potential of the motor neuron pool would not be detected. This would lead to an erroneous calculation of H-reflex gain assessed by the relationship between EMG and H-reflex amplitudes.
Statistical tests
We used a repeated measures ANOVA to test for significant differences between gaits and across gravity within each gait for ankle joint kinematics, mean EMG, linear regression slopes, and linear regression y-intercepts (JMP IN software, SAS Institute, Inc.). We also used a one-sample Student’s t test to determine if Mmax during locomotion was significantly different from the standing Mmax value. Significance was set at P < 0.05 for all tests.
RESULTS
Simulated reduced gravity did not substantially alter the movement pattern as locomotion kinematics were similar for all gravity levels. Stride frequency was independent of gravity during walking (P > 0.05) and decreased only slightly for the lower gravity levels during running (P < 0.01). Stride frequency was 0.93 ± 0.004 Hz for walking at 1.0 G and 0.90 ± 0.02 Hz for walking at 0.25 G (mean ±s.e.m.). For running, stride frequency decreased from 1.37 ± 0.03 Hz at 1.0 G to 1.12 ± 0.03 Hz at 0.25 G, an 18% reduction. Goniometer data revealed that ankle movement followed a similar pattern across gravity levels (Fig. 2), but joint flexion slightly decreased under simulated reduced gravity (Fig. 3). The amount of ankle dorsiflexion during the first part of the stance phase decreased from 15.1 to 7.5 deg for walking and from 15.8 to 7.7 deg for running (P < 0.05). The amount of subsequent ankle plantarflexion did not change significantly with gravity level (Fig. 3). For walking it was 25.6 deg at 1.0 G and 27.4 deg at 0.25 G (P > 0.05). For running it was 40.9 deg at 1.0 G and 32.0 deg at 0.25 G (P > 0.05).
Figure 3. Mean peak ankle displacements for walking and running at four gravity levels.
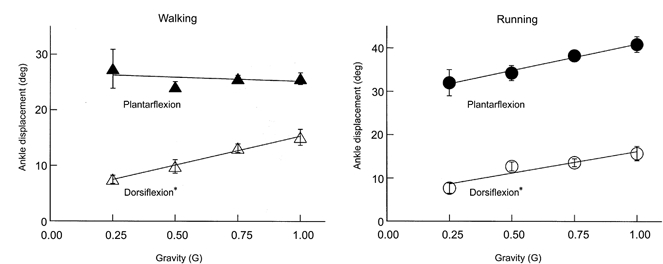
During the first part of the stance phase the ankle joint dorsiflexes and during the second part of the stance phase the ankle joint plantarflexes. Although dorsiflexion slightly decreased under reduced gravity (*P < 0.05), plantarflexion was statistically independent of gravity level (P > 0.05). Error bars are standard errors of the mean and are sometimes small enough to be hidden by the symbol.
The muscle activation patterns were also similar across gravity levels although EMG amplitude decreased under reduced gravity for most muscles. Figure 4 depicts the averaged low-pass filtered EMG for a typical subject during walking and running at 1.0 G and 0.25 G. For all muscles, the patterns of the low-pass filtered EMG were remarkably similar between 1.0 G and 0.25 G conditions. The mean EMG decreased with reduced gravity in most instances (Fig. 5). Vastus lateralis mean EMG decreased by 58% between 1.0 G and 0.25 G during running (P < 0.01) but was independent of gravity during walking (P > 0.05). Soleus mean EMG decreased by 15% during walking and by 32% during running between 1.0 G and 0.25 G (P < 0.01). Medial gastrocnemius mean EMG decreased by 54% during walking and 39% during running between 1.0 G and 0.25 G (P < 0.01). Tibialis anterior mean EMG was independent of gravity during both walking and running (P > 0.10).
Figure 5. Mean EMG for walking and running at four gravity levels for all subjects.
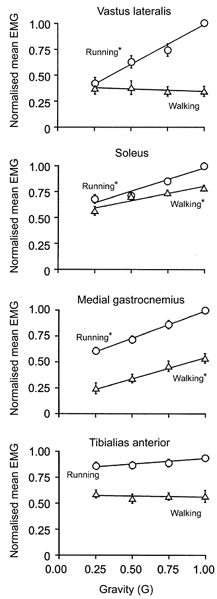
We took the mean rectified averaged EMG over the complete stride for each subject and then normalised to the maximum value recorded for that subject regardless of gait. We used a repeated measures ANOVA to determine if gravity level had a significant effect on mean EMG for each gait (*P < 0.05). Error bars are standard errors of the mean and are sometimes small enough to be hidden by the symbol.
For both walking and running, the maximal M-wave amplitude (Mmax) for the soleus changed during the gait cycle but was similar across gravity levels. Figure 6 shows subjects’ mean Mmax throughout the gait cycle. Walking and running Mmax values were significantly different from the standing Mmax value (P < 0.001). Locomotion Mmax values were highest near the end of the stance phase during walking and near the middle to end of the stance phase during running. Soleus EMG and peak H-reflex amplitudes decreased with reduced gravity for both walking and running (Fig. 7 and 8). Figure 7 shows the subjects’ mean rectified averaged EMG values throughout the gait cycle for the soleus. Peak EMG values for the rectified normalised time slice averages decreased by 27% for walking and by 36% for running over the fourfold reduction in gravity level (P < 0.05). Figure 8 shows the mean H-reflex amplitudes throughout the gait cycle. The H-reflex pattern was similar for all gravity levels but peak amplitudes were lowest for the 0.25 G conditions.
Figure 6. Mean maximum M-wave amplitudes (Mmax) during walking and running at each gravity level for all subjects.
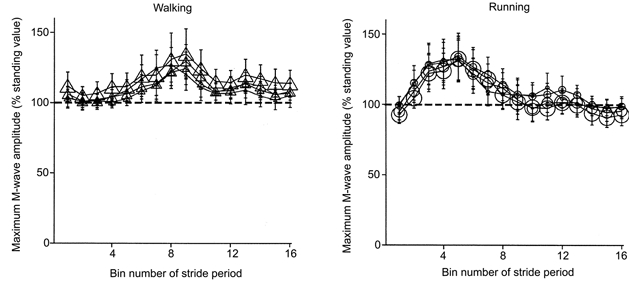
We broke the stride period into 16 equal bins. Each data point represents the average for a bin. The size of the symbol reflects the gravity level (i.e. largest symbols are 1.0 G, smallest symbols are 0.25 G). The dashed line is 100% of Mmax standing value. Bin number 1 is the first time slice after heel strike. Error bars are standard errors of the mean.
Figure 8. Mean H-reflex amplitudes during walking and running at each gravity for all subjects.
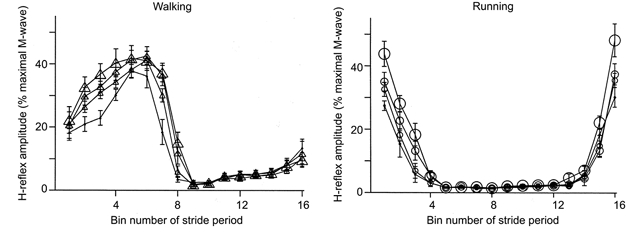
We broke the stride period into 16 equal bins. Each data point represents the average for a bin. The size of the symbol reflects the gravity level (i.e. largest symbols are 1.0 G, smallest symbols are 0.25 G). Bin number 1 is the first time slice after heel strike. Error bars are standard errors of the mean.
H-reflex gain was independent of both gravity and gait but there were differences between gaits in y-intercepts of the linear regressions (Figs 9 and 10). Figure 9 shows an example of data and linear regressions for one subject. At the same level of EMG, walking H-reflex amplitudes were higher than running H-reflex amplitudes. The higher H-reflex amplitudes for walking were due to a vertical shift of the H-reflex versus EMG relationship and not due to a change in slope of the relationship (i.e. H-reflex gain) (Fig. 10). The slopes of the linear regressions were the same for both walking and running (P > 0.6). The y-intercepts were significantly higher for walking compared to running (P < 0.001). Mean slopes for all gravity levels combined were 11.1 for walking and 12.0 for running. Mean y-intercepts for all gravity levels combined were 19.9%Mmax for walking and -2.5%Mmax for running. Both slopes and y-intercepts were independent of gravity for walking and running (P > 0.4).
Figure 9. Example of linear regression data for one subject.
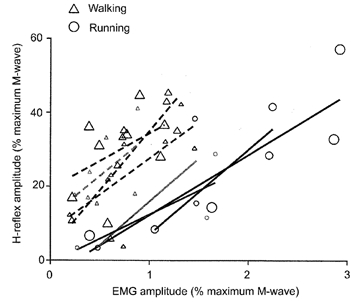
Triangles denote walking data and circles denote running data. Each data point reflects data for a single time bin. The size of the symbol reflects the gravity level (i.e. largest symbols are 1.0 G, smallest symbols are 0.25 G). We fitted a linear least-squares regression to data points for each gravity level and gait for each subject. The calculated slope is a measure of H-reflex gain.
Figure 10. Slope and y-intercept of the linear regressions at each gravity levels for all subjects.
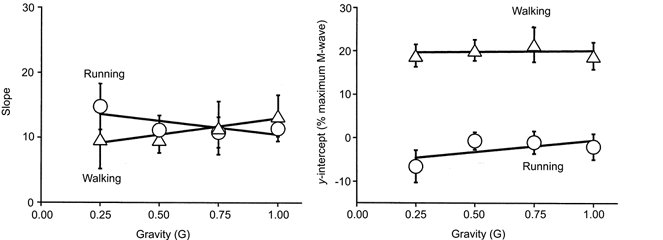
Gravity level did not significantly change either linear regression slope (i.e. H-reflex gain) or linear regression y-intercept for either gait (P > 0.05). Slope was not significantly different for walking or running (P > 0.6), but y-intercept was significantly higher for walking compared to running (P < 0.001). Triangles denote walking data and circles denote running data. Error bars are standard errors of the mean and lines are linear least-squares regressions.
DISCUSSION
As predicted by our hypothesis, H-reflex gain (i.e. the slope of the linear regressions) was independent of gravity level (Fig. 10). H-reflex modulation during human locomotion does not depend on muscle activity level. This indicates that the neural mechanisms responsible for setting H-reflex gain during walking and running are not influenced by changes in motor neuron recruitment. It also validates the premise used in past H-reflex studies that H-reflex gain is independent of EMG amplitude during human locomotion.
H-reflex gain or threshold?
Contrary to previous studies (Capaday & Stein, 1987a; Edamura et al. 1991), we found that H-reflex gain was the same for walking and running. H-reflex amplitudes were lower for running than for walking at similar EMG levels. However, the difference between gaits was a constant reduction of H-reflex amplitude regardless of EMG level and not a change in the slope of the relationship between H-reflex amplitude and EMG. There are two reasons why our results could differ from the results in previous studies.
The first difference between our study and past studies is that Stein and colleagues (Capaday & Stein, 1987a; Edamura et al. 1991) did not correct for systematic changes in the maximal evoked muscle response (i.e. maximal M-wave amplitude: Mmax) throughout a stride of locomotion. Because the stimulating electrode can move relative to the nerve during locomotion, it is necessary to monitor the M-wave amplitude that results from directly stimulating the efferent nerve (Capaday, 1997). By adjusting stimulus intensity to maintain the M-wave within a certain percentage of Mmax, it is possible to ensure that the number of motor axons stimulated remains relatively constant. If the number of motor axons stimulated remains relatively constant, then stimulus intensity to the afferent nerve also remains relatively constant. The recording electrodes can also move relative to the muscle fibres during locomotion. Changes in muscle geometry induced by muscle contraction influence myoelectrical amplitude (Gerilovsky et al. 1989). Measuring Mmax throughout a stride of locomotion allows for the correction of this factor by scaling the amplitude of the M-wave to the Mmax at each instant throughout the stride (Simonsen et al. 1995; Simonsen & Dyhre-Poulsen, 1999). Scaling the amplitude of the M-wave to Mmax at each instant throughout the stride ensures that a similar percentage of motor axons are stimulated. If a similar percentage of motor axons are stimulated, then the stimulus intensity to the afferent nerve is the same. Past studies assessing H-reflex gain (Capaday & Stein, 1987a; Edamura et al. 1991) used the standing Mmax to scale the direct M-wave during locomotion. Thus, they used the same absolute value M-wave while we used the same percentage of the Mmax at each instant throughout the stride.
Changes in Mmax during locomotion may have contributed to differences in the results because not correcting for an increased Mmax could result in a greater slope for the walking linear regression. The greatest difference between Mmax during standing and Mmax during walking or running is at the end of the stance phase when EMG amplitude is low and decreasing (Fig. 6 and 7). Without correcting for the increased Mmax during locomotion, the stimulus level would be decreased relative to the rest of the stride. A lower relative stimulus could result in lower H-reflex amplitudes during the end of stance when EMG values are already low. The lower H-reflex amplitudes would probably not alter H-reflex gain calculations during running because H-reflex amplitudes at low EMG levels are already close to zero and the linear regressions have y-intercepts near the origin (Capaday & Stein, 1987a; Edamura et al. 1991). However, lower H-reflex values at low EMG levels during walking would result in calculation of greater slopes for the linear regressions because the linear regressions would have y-intercepts closer to the origin.
A second difference between our study and past studies is related to the inclusion of data for the linear regressions. Past studies have included data points in the linear regressions with very low level EMG amplitudes and very low level H-reflex amplitudes (see Fig. 4 in Capaday & Stein, 1987a and Fig. 2 in Edamura et al. 1991). As we discussed in the ‘H-reflex gain calculations’ section of Methods, inclusion of these data can force the linear regressions through the origin. To test the possibility that this difference in methods was the primary cause of the difference in slopes between walking and running, we reanalysed our data by progressively including more time slices with low EMG amplitudes. For every subject, we found that adding data points could produce linear regressions with significantly higher slopes for walking than for running. Including the additional data points from late in the stance phase and early in the swing phase also caused the y-intercepts for walking and running to both occur at the origin. These reanalysed results are in agreement with the results from previous studies (Capaday & Stein, 1987a; Edamura et al. 1991).
What is the significance of a change in y-intercept instead of a change in slope? Capaday & Stein (1986, 1987a,b) have previously used the x-intercept of the line relating H-reflex amplitude and EMG amplitude as a measure of reflex threshold. The x-intercept theoretically represents the minimum level of EMG required for an H-reflex response. A positive x-intercept (and thus, a negative y-intercept) indicates that some pre-existing muscle activation is required to produce an H-reflex response for the given afferent stimulus. A negative x-intercept (and thus, a positive y-intercept) indicates that no pre-existing muscle activation is required to produce an H-reflex response for the given afferent stimulus. Our data show no difference in slope between walking and running, but there is a difference in y-intercept. Two lines that have the same slope but different y-intercepts also have different x-intercepts. We conclude that the primary difference in H-reflex modulation between walking and running is a difference in H-reflex threshold and not a difference in H-reflex gain. Walking has a lower H-reflex threshold than running. Thus, a given afferent signal from soleus muscle spindles will produce a greater efferent response during walking compared to running, but the difference between gaits does not depend on muscle recruitment level.
Neural mechanisms for H-reflex modulation
Identifying the differences in H-reflex modulation between gaits provides an insight into the neural mechanisms responsible for H-reflex modulation during locomotion. Stein et al. (1993) found a change in H-reflex threshold without a change in H-reflex gain when they used the drug baclofen to increase presynaptic inhibition of Ia afferents in a decerebrate cat. Baclofen is a drug that selectively affects γ-aminobutyric acid receptors that are insensitive to bicuculline (i.e. GABAB receptors). GABAB receptors are present on Ia afferent terminals and act to control entry of calcium ions into the presynaptic terminal (Curtis et al. 1997). Baclofen produces a prolonged inhibition of the monosynaptic stretch reflex in cats by decreasing excitatory postsynaptic potentials (Curtis et al. 1997; Curtis & Lacey, 1998). Administering baclofen decreased soleus H-reflex amplitude by a constant amount at all levels of muscle activity (Stein et al. 1993), similar to the differences between walking and running observed in our study.
Additional studies examining fictive locomotion in cats also suggest that GABAB receptors are involved in H-reflex depression during walking and running. When spinal locomotor circuits are active during fictive locomotion, they activate interneurons responsible for presynaptic inhibition of motor neurons (Menard et al. 1999). A recent study revealed that the interneurons produce both a tonic inhibition and a phasic inhibition of Ia afferent excitatory postsynaptic potentials (Gosgnach et al. 1999). The authors suggested that the tonic inhibition may be acting through GABAB receptors due to their longer-lasting depression effects while the phasic inhibition may be acting through GABAA receptors (i.e. those sensitive to bicuculline) due to their shorter depression effects (Gosgnach et al. 1999).
This hypothesis regarding GABA receptors would fit well with soleus H-reflex modulation in humans. H-reflex amplitude is lower during walking than during standing at the same level of muscle activation (Capaday & Stein, 1986). Although the original data of Capaday & Stein (1986) indicated differences in both H-reflex gain and threshold between standing and walking, our data suggest there may not be a change in H-reflex gain between the two tasks. Capaday & Stein (1986) found that standing had a positive, non-zero y-intercept and walking had a y-intercept at the origin. Our results indicate that walking actually has a y-intercept on the positive y-axis and not at the origin. The difference in H-reflex modulation between standing and walking could solely be an increase in threshold for walking via the GABAB receptors. The difference between walking and running H-reflex modulation would then be a further increase in H-reflex threshold for running via the GABAB receptors. The phase-dependent modulation of H-reflex amplitude during walking and running could be explained by spinal locomotor circuits acting through GABAA receptors, although there is some evidence against this possibility (Misiaszek et al. 2000). Alternatively, it has been suggested that phase-dependent and gait-dependent H-reflex depression during locomotion may be caused by proprioceptive feedback related to leg movement (Brooke et al. 1997) and not by centrally generated motor patterns. However, recent studies limiting knee joint movement during human walking found no difference in H-reflex modulation compared to control conditions (Garrett et al. 1999; Schneider et al. 2000). Future research needs to further explore the relative importance of centrally generated motor patterns and peripheral feedback on H-reflex modulation during locomotion.
Although our results support an increase in presynaptic inhibition of the H-reflex for running compared to walking, the physiological advantages for the increased inhibition are still unclear. Capaday & Stein (1987a) have suggested that depression of the H-reflex during running might be necessary to prevent saturation of the total motor output. A direct comparison of H-reflex amplitude between walking and running indicates that the absolute H-reflex amplitude is similar for the two gaits in spite of much higher muscle activation for running (Simonsen & Dyhre-Poulsen, 1999). The nervous system might use presynaptic inhibition during locomotion to produce a relatively constant H-reflex response independent of muscle activation and rely on fusimotor activity to adjust stretch reflex responses for different gaits. Available data suggest that the soleus stretch reflex response contributes substantially to muscle activation during running (Dietz et al. 1979; Dietz, 1981), so the increase in H-reflex inhibition does not appear to attenuate the stretch reflex response enough to prevent it from contributing during running. A conclusive advantage for an increase in H-reflex threshold during running remains to be found.
Muscle co-ordination and function
Studying soleus H-reflex modulation during movement may aid in revealing principles of intermuscular co-ordination. Soleus group I afferents have monosynaptic and polysynaptic connections to multiple leg muscles in addition to their autogenic pathways (Pierrot-Deseilligny et al. 1981; Fournier et al. 1984; Mao et al. 1984; Meunier et al. 1993). Conversely, stimulation of group I afferents in other leg muscles can produce excitation and inhibition in the soleus H-reflex (Pierrot-Deseilligny et al. 1981; Mao et al. 1984; Meunier et al. 1990, 1993). Both group Ia and Ib afferents contribute to this complex network of autogenic and heterogenic reflexes (Pierrot-Deseilligny et al. 1981; Burke et al. 1984) and more recent studies indicate that cutaneous afferents play an important role in intermuscular co-ordination as well (Zehr & Stein, 1999). A confounding factor, however, is that both autogenic and heterogenic reflex pathways are highly state dependent. Their magnitude and direction (i.e. excitation vs. inhibition) change to accommodate the muscular demands of the task, phase of the task, and mechanics of the environment (Nichols, 1989; Pearson & Ramirez, 1997; Zehr & Stein, 1999). Deciphering general principles of intermuscular co-ordination is not easy, but identifying reflex function and modulation in the context of musculoskeletal mechanics is an important step (Nichols et al. 1999).
The changes in muscle activity under simulated reduced gravity provide insight into the role of the leg muscles during human locomotion. In spite of a fourfold change in gravity level, there was only a modest decrease in soleus muscle activity. This is not entirely unexpected given that the soleus is made up of predominantly slow-twitch muscle fibres (Edgerton et al. 1975). The nervous system normally recruits slow-twitch muscle fibres before fast-twitch muscle fibres and de-recruits fast-twitch fibres before slow-twitch fibres. Medial gastrocnemius mean EMG decreased in parallel with soleus mean EMG for running but decreased twice as much as soleus mean EMG for walking (Fig. 5). Thus, the two muscles may be recruited as a functional unit during running but appear to be more independent during walking.
It was surprising that the vastus lateralis mean EMG was independent of gravity level for walking. This finding suggests that the vastus lateralis does not act to support body weight during initial stance as is often assumed (Skinner et al. 1985). If the vastus lateralis was acting to support body weight, vastus lateralis mean EMG should have decreased when body weight was reduced under simulated reduced gravity. Our results alternatively suggest that quadriceps primarily decelerates the limb before ground contact to attenuate impact forces (Jefferson et al. 1990) and/or acts to balance hamstring moments about the knee to control the direction of the ground reaction force vector during walking (Jacobs & van Ingen Schenau, 1992; Simonsen et al. 1997). In contrast to the walking results, there was a large decrease in vastus lateralis mean EMG during running. This is in agreement with its assumed role as an anti-gravity muscle, primarily generating force to counter bodyweight (Farley & McMahon, 1992).
As might have been predicted from musculoskeletal mechanics, tibialis anterior mean EMG amplitude was not affected by gravity level during walking or running. The tibialis anterior is primarily active during swing. The simulator did not affect the gravity acting on the swinging limbs. Thus, the mechanical demand placed on the tibialis anterior probably did not change significantly across simulated gravity levels. However, while it may be biomechanically appropriate, a constant tibialis anterior activation holds important neural implications for soleus H-reflex modulation. The tibialis anterior has strong reciprocal and presynaptic inhibitory pathways onto the soleus (Lavoie et al. 1997; Pierrot-Deseilligny, 1997) and is probably involved in some manner with the phase-dependent changes in H-reflex modulation during human locomotion (Schneider et al. 2000). It would be interesting to know if tibialis anterior activity decreased under actual reduced gravity (e.g. on the moon) and if that reduction produced a concomitant change in H-reflex modulation.
The muscle activity results are the first published EMG recordings for human walking and running under simulated reduced gravity that we could locate in the literature. Some previous studies have examined EMG during walking with partial body weight support in humans with neurological deficits and in healthy subjects (Finch et al. 1991; Dietz et al. 1997; Harkema et al. 1997; Stephens & Yang, 1999). However, the body weight support devices used in those studies had extremely stiff support mechanisms that raised the subject’s centre of mass and restricted vertical movement of the trunk and pelvis (Finch et al. 1991; Harkema et al. 1997; Stephens & Yang, 1999) or massive counterweight support mechanisms that increased inertial forces during walking (Dietz et al. 1997). The reduced gravity simulator used in this study had a compliant elastic support mechanism (i.e. rubber springs) that allowed similar centre of mass movement regardless of support level and did not increase inertial forces (He et al. 1991; Griffin et al. 1999). This difference in support mechanics and additional differences in walking speeds between studies make it difficult to directly compare results. The most notable difference between our results and those from the partial body weight support studies is in vastus lateralis muscle activity. Our study was unique in finding that vastus lateralis mean EMG was independent of body weight load during walking. We suggest that additional altered gravity studies including biomechanical analyses could yield further insight into the function and control of leg muscles during human locomotion.
Acknowledgments
The authors would like to thank Kailine Liang for her invaluable assistance with data analysis. This research was supported by grants from NASA (NGT-51416), National Institutes of Health (R29 AR44008), and the Danish Sports Research Council.
References
- Andersen JB, Sinkjaer T. The stretch reflex and H-reflex of the human soleus muscle during walking. Motor Control. 1999;3:151–157. doi: 10.1123/mcj.3.2.151. [DOI] [PubMed] [Google Scholar]
- Brooke JD, Cheng J, Collins D, MacIlroy WE, Misiaszek J, Staines WR. Sensori-sensory afferent conditioning with leg movement: gain control in spinal reflex and ascending paths. Progress in Neurobiology. 1997;51:393–421. doi: 10.1016/s0301-0082(96)00061-5. [DOI] [PubMed] [Google Scholar]
- Capaday C. Neurophysiological methods for studies of the motor system in freely moving human subjects. Journal of Neuroscience Methods. 1997;74:201–218. doi: 10.1016/s0165-0270(97)02250-4. [DOI] [PubMed] [Google Scholar]
- Capaday C, Stein RB. Amplitude modulation of the soleus H-reflex in the human during walking and standing. Journal of Neuroscience. 1986;6:1308–1313. doi: 10.1523/JNEUROSCI.06-05-01308.1986. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Capaday C, Stein RB. Difference in the amplitude of the human soleus H reflex during walking and running. Journal of Physiology. 1987a;392:513–522. doi: 10.1113/jphysiol.1987.sp016794. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Capaday C, Stein RB. A method for simulating the reflex output of a motoneuron pool. Journal of Neuroscience Methods. 1987bg;21:91–104. doi: 10.1016/0165-0270(87)90107-5. [DOI] [PubMed] [Google Scholar]
- Capaday C, Stein RB. The effects of postsynaptic inhibition on the monosynaptic reflex of the cat at different levels of motorneuron pool activity. Experimental Brain Research. 1989;77:577–584. doi: 10.1007/BF00249610. [DOI] [PubMed] [Google Scholar]
- Cavagna GA, Willems PA, Heglund NC. Walking on Mars. Nature. 1998;393:636. doi: 10.1038/31374. [DOI] [PubMed] [Google Scholar]
- Chang Y-H, Huang H-W C, Hamerski CM, Kram R. The independent effects of gravity and inertia on running mechanics. Journal of Experimental Biology. 2000;203:229–238. doi: 10.1242/jeb.203.2.229. [DOI] [PubMed] [Google Scholar]
- Crone C, Johnsen LL, Hultborn H, Orsnes GB. Amplitude of the maximum motor response (Mmax) in human muscles typically decreases during the course of an experiment. Experimental Brain Research. 1999;124:265–270. doi: 10.1007/s002210050621. [DOI] [PubMed] [Google Scholar]
- Curtis DR, Gynther BD, Lacey G, Beattie DT. Baclofen: reduction of presynaptic calcium influx in the cat spinal cord in vivo. Experimental Brain Research. 1997;113:520–533. doi: 10.1007/pl00005604. [DOI] [PubMed] [Google Scholar]
- Curtis DR, Lacey G. Prolonged GABA-B receptor-mediated synaptic inhibition in the cat spinal cord: an in vivo study. Experimental Brain Research. 1998;121:319–333. doi: 10.1007/s002210050465. [DOI] [PubMed] [Google Scholar]
- Davis BL, Cavanagh PR. Simulating reduced gravity: a review of biomechanical issues pertaining to human locomotion. Aviation, Space and Environmental Medicine. 1993;64:557–566. [PubMed] [Google Scholar]
- Dietz V. Contribution of spinal stretch reflexes to the activity of leg muscles in running. In: Taylor A, Prochazka A, editors. Muscle Receptors and Movement. London: Macmillan; 1981. pp. 339–346. [Google Scholar]
- Dietz V, Leenders KL, Colombo G. Leg muscle activation during gait in Parkinson’s disease: influence of body unloading. Electroencephalography and Clinical Neurophysiology. 1997;105:400–405. doi: 10.1016/s0924-980x(97)00042-8. [DOI] [PubMed] [Google Scholar]
- Dietz V, Schmidtbleicher D, Noth J. Neuronal mechanisms of human locomotion. Journal of Neurophysiology. 1979;42:1212–1222. doi: 10.1152/jn.1979.42.5.1212. [DOI] [PubMed] [Google Scholar]
- Donelan JM, Kram R. The effect of reduced gravity on the kinematics of human walking: a test of the dynamic similarity hypothesis for locomotion. Journal of Experimental Biology. 1997;200:3193–3201. doi: 10.1242/jeb.200.24.3193. [DOI] [PubMed] [Google Scholar]
- Donelan JM, Kram R. Exploring dynamic similarity in human running using simulated reduced gravity. Journal of Experimental Biology. 2000;203:2405–2415. doi: 10.1242/jeb.203.16.2405. [DOI] [PubMed] [Google Scholar]
- Dyhre-Poulsen P, Simonsen EB, Voigt M. Dynamic control of muscle stiffness and H reflex modulation during hopping and jumping in man. Journal of Physiology. 1991;437:287–304. doi: 10.1113/jphysiol.1991.sp018596. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Edamura M, Yang JF, Stein RB. Factors that determine the magnitude and time course of human H-reflexes in locomotion. Journal of Neuroscience. 1991;11:420–427. doi: 10.1523/JNEUROSCI.11-02-00420.1991. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Edgerton VR, Smith JL, Simpson DR. Muscle fibre type populations of human leg muscles. Histochemical Journal. 1975;7:259–266. doi: 10.1007/BF01003594. [DOI] [PubMed] [Google Scholar]
- Farley CT, MacMahon TA. Energetics of walking and running: insights from simulated reduced-gravity experiments. Journal of Applied Physiology. 1992;73:2709–2712. doi: 10.1152/jappl.1992.73.6.2709. [DOI] [PubMed] [Google Scholar]
- Finch L, Barbeau H, Arsenault B. Influence of body weight support on normal human gait: development of a gait retraining strategy. Physical Therapy. 1991;71:842–855. doi: 10.1093/ptj/71.11.842. [DOI] [PubMed] [Google Scholar]
- Fournier E, Katz R, Pierrot-Deseilligny E. A re-evaluation of the pattern of group I fibre projections in the human lower limb on using randomly alternated stimulations. Experimental Brain Research. 1984;56:193–195. doi: 10.1007/BF00237457. [DOI] [PubMed] [Google Scholar]
- Garrett M, Kerr T, Caulfield B. Phase-dependent inhibition of H-reflexes during walking in humans is independent of reduction in knee angular velocity. Journal of Neurophysiology. 1999;82:747–753. doi: 10.1152/jn.1999.82.2.747. [DOI] [PubMed] [Google Scholar]
- Gerilovsky L, Tsvetinov P, Trenkova G. Peripheral effects on the amplitude of monopolar and bipolar H-reflex potentials from the soleus muscle. Experimental Brain Research. 1989;76:173–181. doi: 10.1007/BF00253634. [DOI] [PubMed] [Google Scholar]
- Gosgnach S, Quevedo J, Fedirchuk B, Stecina K, McCrea D. Prolonged presynaptic inhibition of monosynaptic Ia EPSPs following fictive locomotion. Society for Neuroscience Abstracts. 1999;25:1154. [Google Scholar]
- Gottlieb GL, Agarwal GC, Stark L. Interactions between voluntary and postural mechanisms of the human motor system. Journal of Neurophysiology. 1970;33:365–381. doi: 10.1152/jn.1970.33.3.365. [DOI] [PubMed] [Google Scholar]
- Griffin TM, Tolani NA, Kram R. Walking in simulated reduced gravity: mechanical energy fluctuations and exchange. Journal of Applied Physiology. 1999;86:383–390. doi: 10.1152/jappl.1999.86.1.383. [DOI] [PubMed] [Google Scholar]
- Harkema SJ, Hurley SL, Patel UK, Requejo PS, Dobkin BH, Edgerton VR. Human lumbosacral spinal cord interprets loading during stepping. Journal of Neurophysiology. 1997;77:797–811. doi: 10.1152/jn.1997.77.2.797. [DOI] [PubMed] [Google Scholar]
- He JP, Kram R, McMahon TA. Mechanics of running under simulated low gravity. Journal of Applied Physiology. 1991;71:863–870. doi: 10.1152/jappl.1991.71.3.863. [DOI] [PubMed] [Google Scholar]
- Jacobs R, van Ingen Schenau GJ. Control of an external force in leg extensions in humans. Journal of Physiology. 1992;457:611–626. doi: 10.1113/jphysiol.1992.sp019397. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Jefferson RJ, Collins JJ, Whittle MW, Radin EL, O’Connor JJ. The role of the quadriceps in controlling impulsive forces around heel strike; Journal of Engineering in Medicine, Proceedings of the Institution of Mechanical Engineers. Part H; 1990. pp. 21–28. [DOI] [PubMed] [Google Scholar]
- Kearney RE, Lortie M, Stein RB. Modulation of stretch reflexes during imposed walking movements of the human ankle. Journal of Neurophysiology. 1999;81:2893–2902. doi: 10.1152/jn.1999.81.6.2893. [DOI] [PubMed] [Google Scholar]
- Kram R, Domingo A, Ferris DP. Effect of reduced gravity on the preferred walk-run transition speed. Journal of Experimental Biology. 1997;200:821–826. doi: 10.1242/jeb.200.4.821. [DOI] [PubMed] [Google Scholar]
- Lavoie BA, Devanne H, Capaday C. Differential control of reciprocal inhibition during walking versus postural and voluntary motor tasks in humans. Journal of Neurophysiology. 1997;78:429–438. doi: 10.1152/jn.1997.78.1.429. [DOI] [PubMed] [Google Scholar]
- Mao CC, Ashby P, Wang M, McCrea D. Synaptic connections from large muscle afferents to the motoneurons of various leg muscles in man. Experimental Brain Research. 1984;56:341–350. doi: 10.1007/BF00236290. [DOI] [PubMed] [Google Scholar]
- Menard A, Leblond H, Gossard JP. The modulation of presynaptic inhibition in single muscle primary afferents during fictive locomotion in the cat. Journal of Neuroscience. 1999;19:391–400. doi: 10.1523/JNEUROSCI.19-01-00391.1999. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Meunier S, Penicaud A, Pierrot-Deseilligny E, Rossi A. Monosynaptic Ia excitation and recurrent inhibition from quadriceps to ankle flexors and extensors in man. Journal of Physiology. 1990;423:661–675. doi: 10.1113/jphysiol.1990.sp018046. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Meunier S, Pierrot-Deseilligny E, Simonetta M. Pattern of monosynaptic heteronymous Ia connections in the human lower limb. Experimental Brain Research. 1993;96:534–544. doi: 10.1007/BF00234121. [DOI] [PubMed] [Google Scholar]
- Misiaszek JE, de Serres SJ, Stein RB, Jiang W, Pearson KG. Stretch and H reflexes in triceps surae are similar during tonic and rhythmic contractions in high decerebrate cats. Journal of Neurophysiology. 2000;83:1941–1950. doi: 10.1152/jn.2000.83.4.1941. [DOI] [PubMed] [Google Scholar]
- Newman DJ, Alexander HL, Webbon BW. Energetics and mechanics for partial gravity locomotion. Aviation, Space and Environmental Medicine. 1994;65:815–823. [PubMed] [Google Scholar]
- Nichols TR. The organization of heterogenic reflexes among muscles crossing the ankle joint in the decerebrate cat. Journal of Physiology. 1989;410:463–477. doi: 10.1113/jphysiol.1989.sp017544. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Nichols TR, Cope TC, Abelew TA. Rapid spinal mechanisms of motor coordination. Exercise and Sport Sciences Reviews. 1999;27:255–284. [PubMed] [Google Scholar]
- Pearson KG, Ramirez J-M. Sensory modulation of pattern-generating circuits. In: Stein PSG, Grillner S, Selverston AI, Stuart DG, editors. Neurons Networks, and Motor Behavior. Cambridge, MA, USA: MIT Press; 1997. pp. 225–235. [Google Scholar]
- Pierrot-Deseilligny E. Assessing changes in presynaptic inhibition of Ia afferents during movement in humans. Journal of Neuroscience Methods. 1997;74:189–199. doi: 10.1016/s0165-0270(97)02249-8. [DOI] [PubMed] [Google Scholar]
- Pierrot-Deseilligny E, Morin C, Bergego C, Tankov N. Pattern of group I fibre projections from ankle flexor and extensor muscles in man. Experimental Brain Research. 1981;42:337–350. doi: 10.1007/BF00237499. [DOI] [PubMed] [Google Scholar]
- Schneider C, Lavoie BA, Capaday C. On the origin of the soleus H-reflex modulation pattern during human walking and its task-dependent differences. Journal of Neurophysiology. 2000;83:2881–2890. doi: 10.1152/jn.2000.83.5.2881. [DOI] [PubMed] [Google Scholar]
- Simonsen EB, Dyhre-Poulsen P. Amplitude of the human soleus H reflex during walking and running. Journal of Physiology. 1999;515:929–939. doi: 10.1111/j.1469-7793.1999.929ab.x. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Simonsen EB, Dyhre-Poulsen P, Voigt M. Excitability of the soleus H reflex during graded walking in humans. Acta Physiologica Scandinavica. 1995;153:21–32. doi: 10.1111/j.1748-1716.1995.tb09830.x. [DOI] [PubMed] [Google Scholar]
- Simonsen EB, Dyhre-Poulsen P, Voigt M, Aagaard P, Fallentin N. Mechanisms contributing to different joint moments observed during human walking. Scandinavian Journal of Medicine and Science in Sports. 1997;7:1–13. doi: 10.1111/j.1600-0838.1997.tb00110.x. [DOI] [PubMed] [Google Scholar]
- Skinner SR, Antonelli D, Perry J, Lester DK. Functional demands on the stance limb in walking. Orthopedics. 1985;8:355–361. doi: 10.3928/0147-7447-19850301-07. [DOI] [PubMed] [Google Scholar]
- Stein RB, Yang JF, Belanger M, Pearson KG. Modification of reflexes in normal and abnormal movements. Progress in Brain Research. 1993;97:189–196. doi: 10.1016/s0079-6123(08)62277-3. [DOI] [PubMed] [Google Scholar]
- Stephens MJ, Yang JF. Loading during the stance phase of walking in humans increases the extensor EMG amplitude, but does not change the duration of step cycle. Experimental Brain Research. 1999;124:363–370. doi: 10.1007/s002210050633. [DOI] [PubMed] [Google Scholar]
- Yang JF, Winter DA. Electromyographic amplitude normalization methods: improving their sensitivity as diagnostic tools in gait analysis. Archives of Physical Medicine and Rehabilitation. 1984;65:517–521. [PubMed] [Google Scholar]
- Zehr EP, Stein RB. What functions do reflexes serve during human locomotion? Progress in Neurobiology. 1999;58:185–205. doi: 10.1016/s0301-0082(98)00081-1. [DOI] [PubMed] [Google Scholar]


