Skip to main content
PMC home page
The Journal of Physiology logoLink to The Journal of Physiology
. 2002 Apr 15;540(Pt 2):635–646. doi: 10.1113/jphysiol.2001.013459

In vivo muscle fibre behaviour during counter-movement exercise in humans reveals a significant role for tendon elasticity

Y Kawakami 1, T Muraoka 1, S Ito 1, H Kanehisa 1, T Fukunaga 1
PMCID: PMC2290252  PMID: 11956349

Abstract

Six men performed a single ankle plantar flexion exercise in the supine position with the maximal effort with counter movement (CM, plantar flexion preceded by dorsiflexion) and without counter movement (NoCM, plantar flexion only) produced by a sliding table that controlled applied load to the ankle (40 % of the maximal voluntary force). The reaction force at the foot and ankle joint angle were measured using a force plate and a goniometer, respectively. From real-time ultrasonography of the gastrocnemius medialis muscle during the movement, the fascicle length was determined. The estimated peak force, average power, and work at the Achilles’ tendon during the plantar flexion phase in CM were significantly greater than those in NoCM. In CM, in the dorsiflexion phase, fascicle length initially increased with little electromyographic activity, then remained constant while the whole muscle-tendon unit lengthened, before decreasing in the final plantar flexion phase. In NoCM, fascicle length decreased throughout the movement and the fascicle length at the onset of movement was longer than that of the corresponding phase in CM. It was concluded that during CM muscle fibres optimally work almost isometrically, by leaving the task of storing and releasing elastic energy for enhancing exercise performance to the tendon.

The force exerted by skeletal muscle fibres is transmitted to the tendinous tissues (tendon and aponeurosis) before producing torque around a joint. The tendinous tissues-sheet-like structures such as aponeuroses in particular-possess elastic characteristics (Alexander & Bennet-Clark, 1977; Proske & Morgan, 1987; Lieber et al. 1991; Kawakami & Lieber, 2000) and are hence a major constituent of the series elastic component (SEC) of a muscle model initially proposed by Hill (1951). Previous studies revealed muscle deformation upon contraction: muscle fibres shorten even during fixed-end isometric contractions while tendinous tissues are elongated to take up muscle fibre shortening (Griffiths, 1991; Fukunaga et al. 1997; Kawakami et al. 1998; Maganaris & Paul, 1999). Therefore, although the force-producing capability of a muscle fibre itself is a function of its length and velocity of shortening or lengthening (Katz, 1939; Hill, 1951; Close, 1972; Edman et al. 1978), the force generated by a muscle-tendon unit is not a simple function of its length and velocity as a whole. This makes it difficult to estimate muscle fibre behaviour solely from observation of joint performance (Fukunaga et al. 1997).

Shortening of muscle preceded by active lengthening has been shown to be more powerful than that resulting from shortening alone (Cavagna et al. 1965). Such a counter-movement action is frequently used in animal locomotion for efficient power production (Alexander & Bennet-Clark, 1977; Morgan et al. 1978). Enhanced performance of human movement accompanied by a preceding counter action has also been widely reported (Thys et al. 1972; Komi, 1984, 1992; Wilson et al. 1992). Mechanisms for the enhancement of performance of the final action have been proposed, including the potentiation of force exerted by prestretched muscle fibres and the additional power provided by the elastic potential of the muscle-tendon unit (Huijing, 1992; Komi, 1992). In the latter case, tendinous tissues have been thought to act as a spring (Alexander & Bennet-Clark, 1977; Morgan et al. 1978; Hof et al. 1983; Griffiths, 1991; Biewener et al. 1998). In fact, direct observation of fascicle length change in walking cats (Hoffer et al. 1989) and running turkeys (Roberts et al. 1997) in vivo has revealed significant elastic recoil of tendinous structures that contributes to joint performance. In humans, however, muscle-tendon unit length has only been estimated from ‘external’ joint kinematics (e.g. Komi, 1992); therefore, it has not been possible to separately observe the behaviour of contractile and elastic components, and the interactions between muscle fibres and tendinous tissues in counter movement have not been observed.

The gastrocnemius muscle, one of the major plantar flexors in humans, has long tendons and aponeuroses that possess substantial compliance (Alexander & Bennet-Clark, 1977; Soest et al. 1995; Kawakami et al. 1998). In the present study, we investigated the muscle fibre behaviour of the gastrocnemius during a plantar flexion exercise in humans, by directly measuring changes in the fascicle length in vivo. The tasks with and without counter movement were compared. We hypothesized that the interactions between muscle fibres and tendinous tissues are different between tasks, and that with counter movement, tendinous tissues, not muscle fibres, behave as elastic structures capable of energy storage and release, thereby enhancing the joint performance compared with the task without counter movement.

METHODS

Subjects

Six healthy men (24–45 years old, 171 ± 5 cm, 72 ± 6 kg, mean ±s.d.) participated in this study as subjects. All the subjects were in good health, with no orthopaedic abnormalities. The nature and possible consequences of the study were explained to each subject before informed, written consent was obtained. The experiments were carried out according to the guidelines laid down by the Ethical Committee of Department of Life Sciences, University of Tokyo (on the use of human subjects), and the Declaration of Helsinki.

Experimental settings and parameters measured

The subject lay on the sliding table of a weight-training apparatus (VR-4100, Cybex, USA), and was secured at the trunk. The table was designed to slide with minimum friction with a constant load through a steel cable connected to adjustable weights. A force plate (Model 9281B, Kistler, Switzerland) was mounted firmly onto the footplate of the machine. A wooden block was attached to the force plate and the subject placed the ball of his right foot on the block with his knee fully extended (Fig. 1). The position of the table was controlled by unilateral plantar flexions of the right ankle. The joint angle of the right ankle was measured with an electric goniometer (Model 4513, NEC Medical Systems, Japan). Electromyographic (EMG) activity was recorded from the midbellies of the medial and lateral gastrocnemii (MG and LG, respectively) and soleus (Sol) muscles in the right leg using surface electrodes with a diameter of 5 mm and an interelectrode distance of 20 mm. The midbellies of the muscles were confirmed from a B-mode ultrasonogram as described previously (Kawakami et al. 2000), and care was taken not to place electrodes over the peripheries of muscles to minimize EMG crosstalk between muscles. The force (a component of the reaction force perpendicular to the force plate), angle and EMG signals were amplified and A/D converted (MacLab/8s, ADInstruments, Japan) at a sampling rate of 1 kHz and transmitted to a computer (Power Macintosh, Apple Computer, USA) for later analysis.

Figure 1. Schematic diagram of experimental setup.

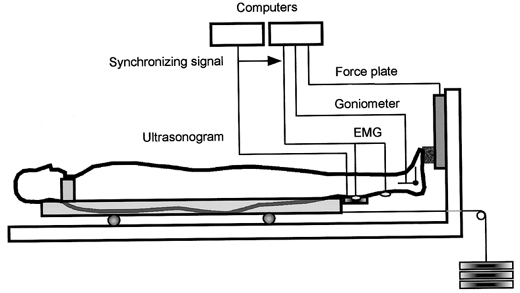
Figure 1

Open in a new tab

The subject was secured on a sliding table with the knee extended. The table was connected to adjustable weights with a cable. The subject performed maximal unilateral plantar flexion movement with and without a counter movement (CM and NoCM, respectively). The force at the ball of the foot perpendicular to the foot plate, ankle joint angle, electomyogram (EMG) from the medial and lateral gastrocnemius and soleus muscles were recorded. Real-time ultrasonic images were also taken to measure fascicle length of the medial gastrocnemius muscle.

Longitudinal sectional images of the gastrocnemius muscle (medial head) were obtained using a real-time ultrasound apparatus (SSD-5000, Aloka, Japan). A linear array probe with a scanning frequency of 7.5 MHz was fixed firmly onto the right leg (at 30 % of the distance between popliteal crease and the centre of lateral malleolus, starting from the popliteal crease), over the midbelly of MG, using foam pads and elastic tapes. The images were obtained at a rate of 65 images s−1 and the images during the exercise were transmitted to a computer (Power Macintosh, Apple Computer, USA). Recordings of images were manually terminated by an electrical switch and its signal was fed into the computer for synchronization of ultrasonic images with other data (force, angle and EMG). For each image, MG fascicles were traced along their length from the superficial and deep aponeuroses (Kawakami et al. 1998) (Fig. 2), and the length of a fascicle was measured using a public domain National Institutes of Health (NIH) Image program (written by Wayne Rasband at the NIH and available from the Internet by anonymous ftp from zippy.nimh.nih.gov). Care was taken to visualize fascicles along their lengths from the superficial to the deep aponeuroses so that the plane of the ultrasonogram was parallel to the fascicles (Kawakami et al. 1993), otherwise the fascicle length would be overestimated (Scott et al. 1993). The fascicle length measurement was repeated two to three times for each image and the averaged values were used. The reliability and reproducibility of the present fascicle length measurement technique have been confirmed elsewhere with the coefficient of variation of 0–2 % (Fukunaga et al. 1997; Kawakami et al. 1995, 1998). We regarded the fascicle length equivalent to muscle fibre length, based on our previous observation of tendon-to-tendon fibre arrangement within a fascicle (Kawakami et al. 2000).

Figure 2. Serial ultrasonic images of the medial gastrocnemius muscle under CM conditions.

Figure 2

Open in a new tab

The muscle belly of the medial gastrocnemius was scanned (top). The fascicles of the gastrocnemius (the line in each image was drawn along the fascicle in the centre of image) can be seen between the superficial (proximal) and deep (distal) aponeuroses (white horizontal echoes). The images were recorded at 65 Hz. In this figure, serial images were curtailed and arranged sequentially, the value to the right of each image shows the time before toe-off (end of movement). To the far right images of the foot position in relation to the recordings are also shown. It can be seen that fascicle length is longest at transition from dorsiflexion to plantar flexion.

After warm-up and practice submaximal contractions, the weight cable was fixed to the end of the table, and the subject performed maximal isometric plantar flexions (maximum voluntary contraction, MVC) for 3–5 s with the ankle positioned at 90 deg (anatomical position, increasing angle refers to plantar flexion). A load equivalent to 40 % of the maximal force at this ankle position was determined by adjusting the number of weight plates, and the maximal range of ankle motion with this load was determined for each subject.

The subject then performed a single plantar flexion exercise with the load described above, with and without a counter movement (CM and NoCM conditions, respectively). The determination of the load was based on pilot experiments that had revealed that the ankle joint angle change performed by subjects were most reproducible with this load. In NoCM, the subject initially kept the ankle position maximally dorsiflexed, and supported the load at this position. Then the subject started ankle movement until the ankle was fully plantar flexed and the toe lifted away (toe-off) from the wooden block. In CM, the ankle was initially positioned at maximal plantar flexion (supporting the load at this position). Then the subject relaxed plantar flexor muscles. This caused the table to slide resulting in passive dorsiflexion of the ankle. The subject then started to develop plantar flexion force until the foot came to a stop at maximal dorsiflexion (the same position as the initial position in NoCM), and rebounded to start plantar flexion until the toe finally lifted away from the wooden block. In both conditions, the subject was instructed to perform the plantar flexion phase of the exercise as fast as possible.

Analyses

The EMG was full-wave rectified and averaged for the duration of the plantar flexion phase of the exercise before being normalized to the average EMG amplitude during the maximal isometric contraction at 90 deg. The torque around the ankle joint (Tq) was estimated from the following equation:

graphic file with name tjp0540-0635-mu1.jpg

where Ff is the force at the ball of the foot tangential to the ankle joint rotation arc, and LF is the distance from the estimated centre of ankle joint to the ball of the foot (measured for each subject) (Fig. 3). Approximation of Ff was obtained by dividing the measured force (Fp; component of Ff perpendicular to the force plate) by the cosine of the ankle joint angle, i.e.

graphic file with name tjp0540-0635-mu2.jpg

Figure 3. Schematic diagram of the leg.

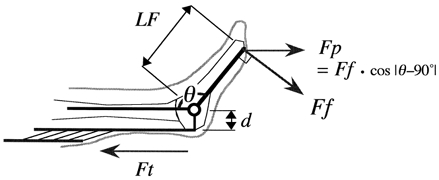
Figure 3

Open in a new tab

This diagram was used to estimate the Achilles' tendon force (Ft). The force at the ball of the foot tangential to the ankle joint rotation arc (Ff) was approximated by dividing the measured force perpendicular to the force plate (Fp) by cos |θ− 90 deg| where θ was the ankle joint angle. Then Ff was multiplied by the distance from the joint centre to the ball of the foot (LF) to obtain torque around the ankle joint. Then the torque was divided by the moment arm length (d) to calculate Ft.

Then the Achilles’ tendon force (Ft) was estimated by the following equation:

graphic file with name tjp0540-0635-mu3.jpg

where d is the moment arm length of the Achilles’ tendon. The moment arm length as a function of the ankle joint angle was derived from a previous report (Rugg et al. 1990). The linear velocity of the gastrocnemius muscle-tendon unit (MTU) was determined from the estimated length change of the gastrocnemius muscle as a function of ankle joint angles (Grieve et al. 1978), and the power delivered to the Achilles’ tendon by MG MTU was calculated from the tendon force and MTU velocity. Work provided by MG MTU was further estimated by the time integral of MTU power over the plantar flexion phase.

Statistics

Values are presented as individual values as well as means and standard deviations unless otherwise stated. The differences between conditions (NoCM and CM) were tested using Student's paired t test for fascicle and MTU lengths and fascicle velocity and MTU power and work. A two-way analysis of variance (ANOVA) with repeated measures was used to analyse the difference in the normalized average EMG amplitudes in the plantar flexion phase (3 × 2, muscles × exercise conditions). The probability level accepted for statistical significance was P < 0.05.

RESULTS

Figure 4 illustrates typical results in CM and NoCM, where changes in ankle joint angle, force perpendicular to the force plate, EMG, and fascicle length of MG are shown from the onset through to the end of movement. There was no significant difference between CM and NoCM in the maximal dorsiflexion angle and the plantar flexion angle at toe-off (Table 1). Maximal reaction force was observed at the onset of the plantar flexion phase both in CM and NoCM (Fig. 4), and the estimated maximal Achilles’ tendon force was greater in CM than in NoCM (Table 1). The maximal angular velocity in the plantar flexion phase was also higher in CM than in NoCM. There was no significant effect of conditions or muscles on EMG amplitudes (Table 1).

Figure 4. Records of ankle joint angle, fascicle length of MG, reaction force at the foot perpendicular to the force plate, and EMGs from the medial gastrocnemius (MG) muscle.

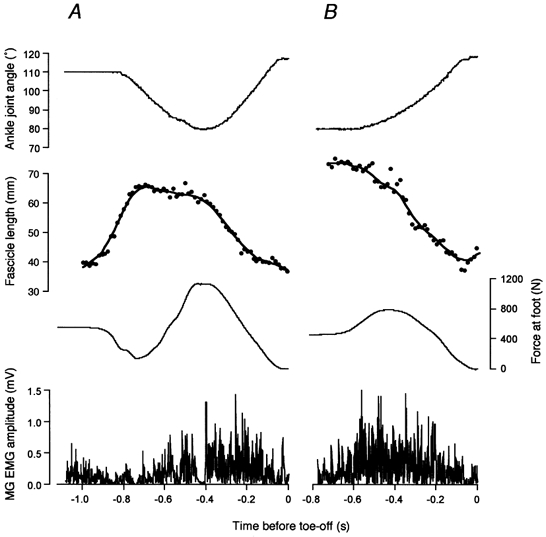
Figure 4

Open in a new tab

In A (CM), the subject initially supported the load with the ankle kept in a plantar flexed position (in this case at 110 deg), then relaxed the muscles to start dorsiflexion. Fascicle length started to increase (passive lengthening) then remained constant for the most part of dorsiflexion phase (in this subject, fascicle length slightly decreased), then began to decrease in the plantar flexion phase. In B (NoCM), the subject initially supported the load with the ankle in a dorsiflexed position (in this case at 80 deg), then started to perform plantar flexion. The fascicle length kept decreasing throughout the movement.

Table 1.

Measured and estimated variables (n = 6)

CM NoCM
Maximal angular velocity (deg s−1) 318.7 ± 95.3* 274.1 ± 86.2
Maximal plantar flexion angle (deg) 138.4 ± 12.7 135.0 ± 10.0
Maximal dorsiflexion angle (deg) 79.4 ± 7.3 78.5 ± 8.7
Maximal Achilles' tendon force (N) 4055 ± 655* 3081 ± 667
Maximal Achilles' tendon power (W) 408.0 ± 144.1* 331.8 ± 108.6
Mean Achilles' tendon power (W) 235.6 ± 87.8* 157.9 ± 47.7
Work during plantar flexion phase (J) 75.2 ± 16.1* 62.9 ± 17.4
Mean EMG amplitude of MG (%) † 104.5 ± 18.8 104.5 ± 29.1
Mean EMG amplitude of LG (%) † 112.9 ± 18.0 108.1 ± 17.0
Mean EMG amplitude of Sol (%) † 103.7 ± 27.6 105.1 ± 30.4
Mean fascicle length of MG in plantar flexion phase (mm) 41 ± 4* 46 ± 6
Maximal fascicle shortening velocity of MG (mm s−1) 186 ± 45 212 ± 53
Mean fascicle shortening velocity of MG in plantar flexion phase (mm s−1) 73 ± 25 56 ± 12
Open in a new tab

Values are expressed as means ±s.d.

*

Significantly different from NoCM

†

EMG of the medial (MG) lateral (LG) gastrocnemius and soleus (Sol) (averaged over the plantar flexion phase) is expressed percentage of maximal isometric contraction.

In NoCM, the fascicle length systematically decreased during the exercise (Fig. 4). In CM, on the other hand, the fascicle length increased at the onset of joint movement (dorsiflexion), then remained constant as the ankle was dorsiflexed (in the case of the subject in Fig. 4, it slightly decreased). When the ankle was finally plantar flexing, the fascicle length decreased. The increase in fascicle length when the ankle was dorsiflexing was accompanied by a decrease in EMG amplitude in all muscles. Figure 5 shows individual data of fascicle lengths from six subjects, plotted against ankle joint angles. In all subjects, there was a phase in CM where the fascicle length was almost constant while the ankle was dorsiflexed, which was subsequently followed by fascicle shortening with plantar flexion before toe-off. It was also observed in all subjects that the rate of fascicle length change, immediately after the onset of shortening, was greater than that of the whole muscle both in CM and NoCM. This was apparent in Fig. 5 as differences in the rate of change in length between MTU and fascicles (different slopes of joint angle-length relationships) in the plantar flexion phase.

Figure 5. The relationships between ankle joint angles and fascicle length and MTU length change of MG.

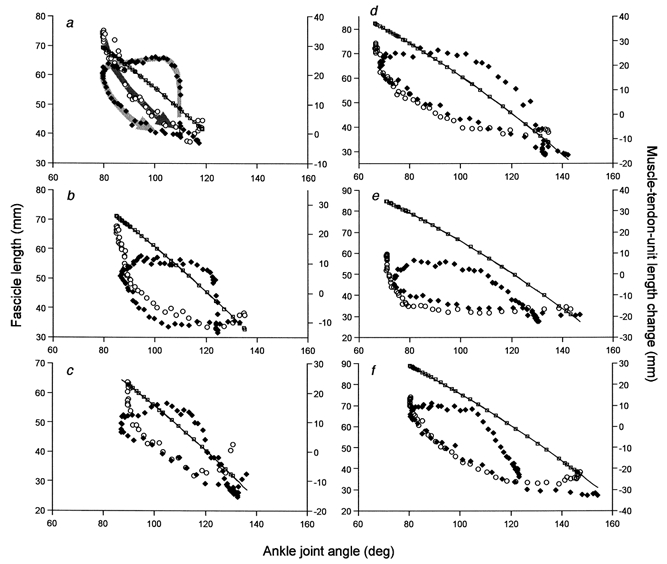
Figure 5

Open in a new tab

Results from six subjects (a-f). Fascicle length (left ordinate): ♦, CM; ○, NoCM. The line represents the estimated MTU length change in CM and squares (□) along the line represent the estimated MTU length change in NoCM (right ordinate). Both estimates are along the same line because they were based on the same equation. Arrows are drawn in a as a guide to the directions of movement. There is a dorsiflexion phase (ankle joint angle decreasing) in CM followed by a plantar flexion phase (the arrow in a for CM changes its direction at the top left corner). NoCM consists of only a plantar flexion phase (the arrow direction is from top left to bottom right). The slopes of the curves made by the arrows for the early plantar flexion phase are higher both for CM and NoCM than that of MTU length changes in all subjects, indicating a higher fascicle shortening velocity than that of MTU.

Figure 6 and Figure 7 illustrate changes in Achilles’ tendon force and MTU power, plotted against the fascicle length and ankle joint angle, respectively. The Achilles’ tendon force was greatest at the onset of the plantar flexion phase, and CM resulted in greater peak force compared with NoCM (Fig. 6). Once again, it was obvious that in CM, fascicles developed force almost isometrically in the late dorsiflexion phase (the part indicated by a thin bi-directional arrow in Fig. 6), which was preceded by passive fascicle lengthening (force decreasing as the fascicle was lengthened). The MTU power showed a similar pattern with CM demonstrating greater values when the ankle started plantar flexion (Fig. 7). Table 1 shows measured and estimated variables in CM and NoCM. Significant differences between CM and NoCM were observed for the maximal force, mean and maximal power and work, and mean fascicle length, but not the mean or peak fascicle shortening velocity in the plantar flexion phase.

Figure 6. The relationship between MG fascicle length and estimated Achilles' tendon force.

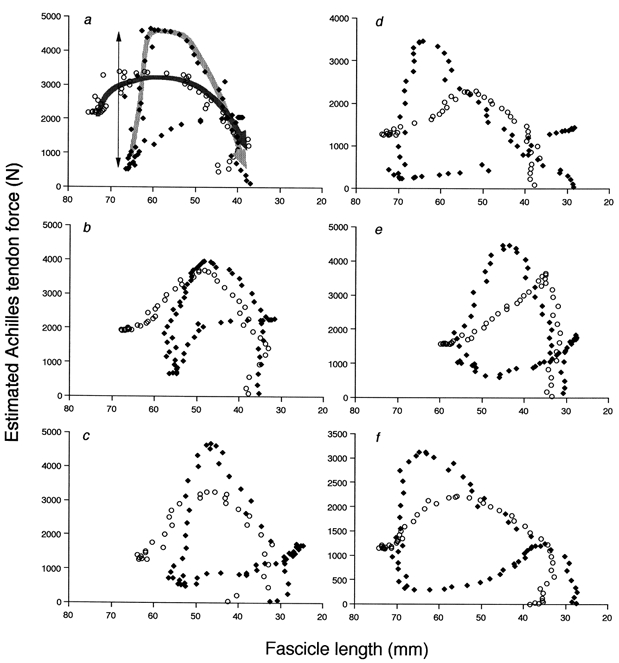
Figure 6

Open in a new tab

Results from six subjects (a-f). ♦, CM; ○, NoCM. Arrows are drawn in a as a guide to the directions of movement (for CM, the arrow is not drawn for the early dorsiflexion phase where tendon force is decreasing). The late dorsiflexion phase in CM is indicated by a thin bi-directional arrow. Note: the direction of the abscissa is reversed (plantar flexion phase from left to right) for comparison with Fig. 7. In all subjects, the fascicle length was almost constant in the late dorsiflexion phase of CM (MTU length increasing) (for subjects a-e, fascicle length slightly decreased), then started to decrease (shorten) with greater tendon force than in NoCM.

Figure 7. The relationship between ankle joint angle and estimated MTU power.

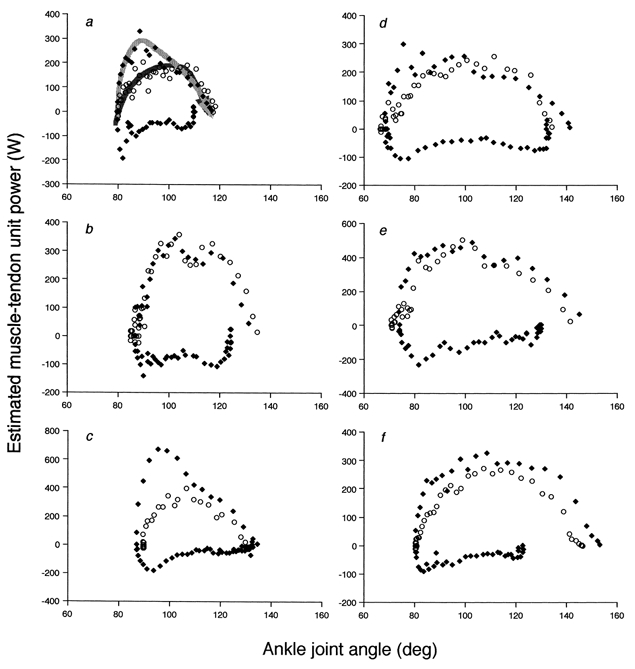
Figure 7

Open in a new tab

Results from six subjects (a-f). ♦, CM; ○, NoCM. Arrows are drawn in a as a guide to the directions of movement in the fascicle shortening phase (for CM, the arrow is not drawn for the dorsiflexion phase). The plots with negative power represent fascicle lengthening. The MTU power was greater in CM than in NoCM at the onset of the shortening phase in all subjects except for b.

DISCUSSION

Muscle shortening immediately preceded by lengthening is called a stretch-shortening cycle (Komi, 1984). Muscle fibres in a stretch-shortening cycle produce greater work during the shortening phase (Cavagna et al. 1965, 1985). Joint power enhancement during a stretch-shortening cycle seen in human movement with preceding counter action has often been attributed to such a potentiation mechanism within stretched muscle fibres (Bosco et al. 1982; Komi, 1992). Some researchers, however, have also proposed the role of elasticity of SEC (Hof & van den Berg, 1986; Huijing, 1992). In the present study, we compared fascicle behaviour with and without a stretch-shortening cycle (i.e. counter movement of the ankle joint). We hypothesized that the interactions between contractile (muscle fibres) and elastic (tendinous tissues) components of MTU are different between tasks, and that with counter movement, tendinous tissues, not muscle fibres, behave as elastic structures capable of energy storage and release, thereby enhancing the joint performance compared with the task without counter movement.

One of the major findings of the present study was that changes in joint angle and fascicle length did not match in either CM or NoCM. Such mismatching of muscle and fascicle lengths has already been observed in animal locomotion (Hoffer et al. 1989; Griffiths, 1991) and recently in human walking (Fukunaga et al. 2001). A number of studies on the stretch-shortening cycle in humans have investigated counter movement exercises (e.g. jumping and running) and separated the movement into ‘eccentric’ (muscle lengthening) and ‘concentric’ (muscle shortening) phases based on joint angles and reaction forces (e.g. Bosco et al. 1982; Komi, 1984, 1992). The present study showed that in an ‘eccentric’ phase of CM where the ankle joint was being dorsiflexed, fascicle length was almost constant when the muscle was activated. Even in NoCM, fascicle length and joint angles did not match. These results clearly indicate the existence of interactions between muscle fibres and tendinous tissues that are task specific (CM and NoCM), which supports our first hypothesis.

The tendon force at the onset of plantar flexion phase was greater in CM than in NoCM. There were no differences between CM and NoCM in this phase for the maximal and mean fascicle shortening velocities and EMG amplitudes. Considering also no active lengthening of fascicles in CM (dorsiflexion phase), the greater force in CM does not appear to be due to the force-velocity characteristics of muscle fibres, as predicted from a model (Bobbert et al. 1986) or different levels of muscle activation (Bosco et al. 1982; Komi, 1992) in CM and NoCM. Alternatively, the result could be attributed to the difference in fascicle lengths in this phase. Our recent study (Kawakami et al. 2000) showed that the MG fascicle length corresponding to the optimal sarcomere lengths was ∼50 mm. At the onset of NoCM, fascicle length was 64 ± 10 mm, suggesting that the sarcomeres were on the descending limb of the length-tension curve and started contraction in this disadvantageous condition. In CM, on the other hand, fascicle length was 55 ± 9 mm at the transition from dorsiflexion to plantar flexion, thus the sarcomeres would be closer to the optimal length for greater force development. However, this is purely speculative at this moment, and we cannot entirely rule out the possibility of facilitated greater activation of stretched (even if only passively) muscle fibres. Small changes in activation might have gone undetected by the small number of subjects and by surface EMG that is a noisy average of neural activation. The fascicle shortening velocity tended to be, although not significantly, higher in CM compared with NoCM, which might also have been operative in power enhancement. We do, however, feel justified in concluding that the greater force combined with elastic recoil of tendinous tissues is the principal mechanism of power enhancement in CM, which supports our second hypothesis.

Based on the present findings, muscle fibre kinetics during CM and NoCM can be formulated as follows (Fig. 8). For CM, initially muscle fibres were contracting isometrically with the ankle most plantar flexed, sustaining the load applied to the subject. In this situation, tendinous tissues were stretched by the tension provided by muscle fibres and hence, fibres were more shortened than in the resting state at the same joint angle. Then the subject relaxed the muscle, and the torque around the ankle decreased and the ankle began to be dorsiflexed. Muscle fibres were stretched almost passively by the tension from elongated tendinous tissues (Fig. 4, a rapid increase in fascicle length at the onset of dorsiflexion). The subject then started to activate the muscle to resist the inertial load until dorsiflexion finally stopped at the most dorsiflexed position. In this phase, muscle fibres were isometrically contracting, while tendinous tissues were being lengthened. In fact, in most subjects, fascicles slightly shortened in this phase, supporting the idea of ‘concerted contraction’ by Hof & van den Berg (1986). After the transition from dorsiflexion to plantar flexion, both muscle fibres and tendinous tissues started to shorten until the toe lifted away from the block. This made powerful plantar flexion like a ‘catapult action’ (Hof et al. 1983). In NoCM, initially muscle fibres were contracting isometrically with the ankle most dorsiflexed, sustaining the load. At the onset of plantar flexion, muscle fibres started to develop greater force to launch the ankle for plantar flexion against the inertial load. At this instance, muscle fibres stretched tendinous tissues a certain amount, thus the shortening velocity of muscle fibres was greater than that of MTU (Fig. 5, a greater decrease in fascicle length than that of MTU). Towards the take-off of the toe, not only muscle fibres but tendinous tissues shortened; although the amount of power provided by tendinous tissues would be much smaller than that of CM. This model can explain differences in peak Achilles’ tendon force and power estimated from a theoretical muscle-tendon model (Bobbert et al. 1986; Hof & van den Berg, 1986; Voigt et al. 1995), and this was actually observed during hopping and squat jump through direct measurement of Achilles’ tendon force in vivo (Fukashiro et al. 1995). Biewener et al. (1998) reported from their study on wallabies, that muscle fibres showed little change in length during a stance phase of hopping. They concluded that the primary role of the gastrocnemius muscle fibres was to provide isometric force for the tendinous tissues to effectively convert elastic energy to mechanical work. The present result indicates that this is also the case for a ballistic exercise with counter movement in humans: muscle fibres optimally work almost isometrically, by leaving the task of storing and releasing elastic energy for enhancing exercise performance to the tendon.

Figure 8. Models of the MG MTU during the present exercises.

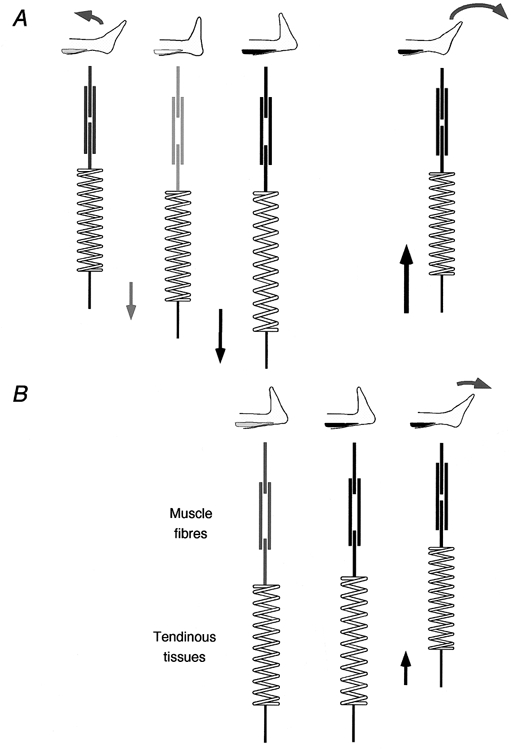
Figure 8

Open in a new tab

The diagrams in A (CM) and B (NoCM) illustrate the schematic time course of changes in MTU from the onset through to the end of movement. Thick lines represent muscle fibres (the overlap of lines represents the amount of overlap of thick and thin filaments). The darkness of lines represents different activation levels (darker for more activated). The spring represents tendinous tissues. In A, MTU length increases in the dorsiflexion phase, with almost no change in muscle fibre length later in this phase when the muscle is actively contracting. In the plantar flexion phase, both muscle fibres and tendinous tissues shorten, resulting in greater MTU power than in NoCM (B) where the length change of tendinous tissues is much shorter.

To carry out the present study, we made the following assumptions. Firstly, we studied MG length change while in fact, other muscles such as LG and Sol also contribute to ankle plantar flexion, and the Achilles’ tendon force and MTU power by a combination of contractions of all these muscles. The behaviour of these other muscles during CM and NoCM might be different from that of MG. However, EMG activity in the plantar flexion phase of CM and NoCM showed no significant inter-muscle differences, suggesting that the three muscles were equally activated during the exercises. However, fascicle behaviour of other muscles should also be tested in future studies. Secondly, we measured fascicle length from only one position within MG, assuming that the measurement represented the fascicle behaviour of the whole muscle. This assumption is based on our recent study that revealed identical fascicle length around the midbelly of MG at rest and during contractions (Kawakami et al. 2000). In addition, an identical position was scanned for CM and NoCM to make viable comparisons between conditions.

In summary, fascicle behaviour during a counter-movement plantar flexion revealed almost isometric, not lengthening, action of the gastrocnemius muscle fibres in the ‘eccentric’ phase of the joint. This fascicle behaviour was associated with greater muscle power and elastic recoil of tendinous tissues in the muscle shortening phase for greater work production compared with an exercise without a counter movement. The near-isometric fascicle behaviour in the muscle lengthening phase is in line with our recent study on human walking. Future studies will reveal a significant role for the muscle-tendon interactions in other human movements such as running and jumping.

REFERENCES

  1. Alexander RM, Bennet-Clark HC. Storage of elastic strain energy in muscle and other tissues. Nature. 1977;265:114–117. doi: 10.1038/265114a0. [DOI] [PubMed] [Google Scholar]
  2. Biewener AA, Konieczynski DD, Baudinette RV. In vivo muscle force-length behavior during steady-speed hopping in tammar wallabies. Journal of Experimental Biology. 1998;201:1681–1694. doi: 10.1242/jeb.201.11.1681. [DOI] [PubMed] [Google Scholar]
  3. Bobbert MF, Huijing PA, Schenau GJ. A model of the human triceps surae muscle-tendon complex applied to jumping. Journal of Biomechanics. 1986;19:887–898. doi: 10.1016/0021-9290(86)90184-3. [DOI] [PubMed] [Google Scholar]
  4. Bosco C, Viitasalo JA, Komi PV, Luhtanen P. Combined effect of elastic energy and myoelectrical potentiation during stretch-shortening cycle exercise. Acta Physiologica Scandinavica. 1982;114:557–565. doi: 10.1111/j.1748-1716.1982.tb07024.x. [DOI] [PubMed] [Google Scholar]
  5. Cavagna GA, Mazzanti M, Heglund NC, Citterio G. Storage and release of mechanical energy by active muscle: A non-elastic mechanism? Journal of Experimental Biology. 1985;115:79–87. doi: 10.1242/jeb.115.1.79. [DOI] [PubMed] [Google Scholar]
  6. Cavagna GA, Saibene FP, Margaria R. Effect of negative work on the amount of positive work performed by an isolated muscle. Journal of Applied Physiology. 1965;20:157–158. doi: 10.1152/jappl.1965.20.1.157. [DOI] [PubMed] [Google Scholar]
  7. Close R. Dynamic properties of mammalian skeletal muscles. Physiological Reviews. 1972;52:129–197. doi: 10.1152/physrev.1972.52.1.129. [DOI] [PubMed] [Google Scholar]
  8. Edman KAP, Elzinga G, Noble MIM. Enhancement of mechanical performance by stretch during tetanic contractions of vertebrate skeletal muscle fibres. Journal of Physiology. 1978;281:139–155. doi: 10.1113/jphysiol.1978.sp012413. [DOI] [PMC free article] [PubMed] [Google Scholar]
  9. Fukashiro S, Komi PV, Jarvinen M, Miyashita M. In vivo Achilles tendon loading during jumping in humans. European Journal of Applied Physiology. 1995;71:453–458. doi: 10.1007/BF00635880. [DOI] [PubMed] [Google Scholar]
  10. Fukunaga T, Ichinose Y, Ito M, Kawakami YS, Fukashiro S. Determination of fascicle length and pennation in a contracting human muscle in vivo. Journal of Applied Physiology. 1997;82:354–358. doi: 10.1152/jappl.1997.82.1.354. [DOI] [PubMed] [Google Scholar]
  11. Fukunaga T, Kubo K, Kawakami Y, Fukashiro S, Kanehisa H, Maganaris CN. In vivo behaviour of human muscle tendon during walking. Proceedings of the Royal Society. 2001;268B:229–233. doi: 10.1098/rspb.2000.1361. [DOI] [PMC free article] [PubMed] [Google Scholar]
  12. Grieve DW, Pheasant S, Cavanagh PR. Prediction of gastrocnemius length from knee and ankle joint posture. In: Asmussen E, Jorgensen K, editors. Biomechanics VI-A. Baltimore: University Park Press; 1978. pp. 405–413. [Google Scholar]
  13. Griffiths RI. Shortening of muscle fibres during stretch of the active cat medial gastrocnemius muscle: the role of tendon compliance. Journal of Physiology. 1991;436:219–236. doi: 10.1113/jphysiol.1991.sp018547. [DOI] [PMC free article] [PubMed] [Google Scholar]
  14. Hill AV. The mechanics of voluntary muscle. The Lancet. 1951;24:947–951. doi: 10.1016/s0140-6736(51)91922-8. [DOI] [PubMed] [Google Scholar]
  15. Hof AL, Geelen BA, van den Berg JW. Calf muscle moment, work and efficiency in level walking: role of series elasticity. Journal of Biomechanics. 1983;16:523–537. doi: 10.1016/0021-9290(83)90067-2. [DOI] [PubMed] [Google Scholar]
  16. Hof AL, van den Berg JW. How much energy can be stored in human muscle elasticity? Human Movement Science. 1986;5:107–114. [Google Scholar]
  17. Hoffer JA, Caputi AA, Pose IE, Griffiths RI. Roles of muscle activity and load on the relationship betweenmuscle spindle length and whole muscle length in the freelywalking cat. In: Allum JHJ, Hulliger M, editors. Progress in Brain Research. Vol. 80. Amsterdam: Elsevier Science; 1989. pp. 75–85. [DOI] [PubMed] [Google Scholar]
  18. Huijing PA. Elastic potential of muscle. In: Komi PV, editor. Strength and Power in Sport. Oxford: Blackwell Scientific; 1992. pp. 151–168. [Google Scholar]
  19. Katz B. The relation between force and speed in muscular contraction. Journal of Physiology. 1939;96:45–64. doi: 10.1113/jphysiol.1939.sp003756. [DOI] [PMC free article] [PubMed] [Google Scholar]
  20. Kawakami Y, Abe T, Fukunaga T. Muscle-fiber pennation angles are greater in hypertrophied than in normal muscles. Journal of Applied Physiology. 1993;74:2740–2744. doi: 10.1152/jappl.1993.74.6.2740. [DOI] [PubMed] [Google Scholar]
  21. Kawakami Y, Abe T, Kuno S, Fukunaga T. Training-induced changes in muscle architecture and specific tension. European Journal of Applied Physiology. 1995;72:37–43. doi: 10.1007/BF00964112. [DOI] [PubMed] [Google Scholar]
  22. Kawakami Y, Ichinose Y, Fukunaga T. Architectural and functional features of human triceps surae muscles during contraction. Journal of Applied Physiology. 1998;85:398–404. doi: 10.1152/jappl.1998.85.2.398. [DOI] [PubMed] [Google Scholar]
  23. Kawakami Y, Kumagai K, Huijing PA, Hijikata T, Fukunaga T. The length-force characteristics of human gastrocnemius and soleus muscles in vivo. In: Herzog W, editor. Skeletal Muscle Mechanics: From Mechanisms to Function. Chichester: John Wiley & Sons; 2000. pp. 327–341. [Google Scholar]
  24. Kawakami Y, Lieber RL. Interaction between series compliance and sarcomere kinetics determines internal sarcomere shortening during fixed-end contraction. Journal of Biomechanics. 2000;33:1249–1255. doi: 10.1016/s0021-9290(00)00095-6. [DOI] [PubMed] [Google Scholar]
  25. Komi PV. Physiological and biomechanical correlates of muscle function: effects of muscle structure and stretch-shortening cycle on force and speed. In: Terjung RL, editor. Exercise and Sport Sciences Reviews. Lexington: Collamore Press; 1984. pp. 81–121. [PubMed] [Google Scholar]
  26. Komi PV. Stretch shortening cycle. In: Komi PV, editor. Strength and Power in Sport. Oxford: Blackwell Scientific; 1992. pp. 169–179. [Google Scholar]
  27. Lieber RL, Leonard ME, Brown CG, Trestik CL. Frog semitendinosis tendon load-strain and stress-strain properties during passive loading. American Journal of Physiology. 1991;261C:86–92. doi: 10.1152/ajpcell.1991.261.1.C86. [DOI] [PubMed] [Google Scholar]
  28. Maganaris CN, Paul JP. In vivo human tendon mechanical properties. Journal of Physiology. 1999;521:307–313. doi: 10.1111/j.1469-7793.1999.00307.x. [DOI] [PMC free article] [PubMed] [Google Scholar]
  29. Morgan DL, Proske U, Warren D. Measurements of muscle stiffness and the mechanism of elastic storage of energy in hopping kangaroos. Journal of Physiology. 1978;282:253–261. doi: 10.1113/jphysiol.1978.sp012461. [DOI] [PMC free article] [PubMed] [Google Scholar]
  30. Proske U, Morgan DL. Tendon stiffness: methods of measurement and significance for the control of movement. A review. Journal of Biomechanics. 1987;20:75–82. doi: 10.1016/0021-9290(87)90269-7. [DOI] [PubMed] [Google Scholar]
  31. Roberts TJ, Marsh RL, Weyand PG, Taylor CR. Muscular force in running turkeys: the economy of minimizing work. Science. 1997;275:1113–1115. doi: 10.1126/science.275.5303.1113. [DOI] [PubMed] [Google Scholar]
  32. Rugg SG, Gregor RJ, Mandelbaum BR, Chiu L. In vivo moment arm calculation at the ankle using magnetic resonance imaging (MRI) Journal of Biomechanics. 1990;23:495–501. doi: 10.1016/0021-9290(90)90305-m. [DOI] [PubMed] [Google Scholar]
  33. Scott SH, Engstrom CM, Loeb GE. Morphometry of human thigh muscles. Determination of fascicle architecture by magnetic resonance imaging. Journal of Anatomy. 1993;182:249–257. [PMC free article] [PubMed] [Google Scholar]
  34. Soest AJ, van Huijing PA, Solomonow M. The effect of tendon on muscle force in dynamic isometric contraction: a simulation study. Journal of Biomechanics. 1995;28:801–807. doi: 10.1016/0021-9290(94)00131-m. [DOI] [PubMed] [Google Scholar]
  35. Thys H, Faraggiana T, Margaria R. Utilization of muscle elasticity in exercise. Journal of Applied Physiology. 1972;32:491–494. doi: 10.1152/jappl.1972.32.4.491. [DOI] [PubMed] [Google Scholar]
  36. Voigt M, Simonsen EB, Dyhre-Poulsen P, Klausen K. Mechanical and muscular factors influencing the performance in maximal vertical jumping after different prestretch loads. Journal of Biomechanics. 1995;28:293–307. doi: 10.1016/0021-9290(94)00062-9. [DOI] [PubMed] [Google Scholar]
  37. Wilson GJ, Elliott BC, Wood GA. Stretch shorten cycle performance enhancement through flexibility training. Medicine and Science in Sports and Exercise. 1992;24:116–123. [PubMed] [Google Scholar]

Articles from The Journal of Physiology are provided here courtesy of The Physiological Society

RESOURCES