Length-dependent activation in three striated muscle types of the rat

Abstract

The process whereby sarcomere length modulates the sensitivity of the myofilaments to Ca²⁺ is termed length-dependent activation. Length-dependent activation is a property of all striated muscles, yet the relative extent of length-dependent activation between skeletal muscle and cardiac muscle is unclear. Although length-dependent activation may be greater in fast skeletal muscle (FSM) than in slow skeletal muscle (SSM), there has not been a well controlled comparison of length-dependent activation between skeletal muscle and cardiac muscle (CM). Accordingly, we measured sarcomere length-dependent properties in skinned soleus (SSM), psoas (FSM) and ventricular trabeculae (CM) of the rat under carefully controlled conditions. The free Ca²⁺-force relationship was determined at sarcomere lengths (SL) of 1.95 μm, 2.10 μm and 2.25 μm and fitted to a modified Hill equation. FSM and SSM were more sensitive to Ca²⁺ than CM. Length-dependent activation was ordered as CM > FSM > SSM. Cooperativity as measured by the Hill coefficient of the Ca²⁺-force relationship was not significantly different between CM and FSM, both of which exhibited greater cooperativity than SSM. SL did not significantly alter this parameter in each muscle type. To establish whether the observed differences can be explained by alterations in interfilament spacing, we measured myofilament lattice spacing (LS) by synchrotron X-ray diffraction in relaxed, skinned muscle preparations. LS was inversely proportional to SL for each muscle type. The slope of the SL-LS relationship, however, was not significantly different between striated muscle types. We conclude that (1) length-dependent activation differs among the three types of striated muscle and (2) these differences in the length-dependent properties among the striated muscle types may not solely be explained by the differences in the response of interfilament spacing to changes in muscle length in relaxed, skinned isolated muscle preparations.

Studies of the relationship between sarcomere length and tension development in striated muscle have been an important tool for revealing the complex molecular events that occur in the sarcomere upon activation by Ca²⁺ ions. Whereas skeletal muscle exhibits both a steep and a shallow portion of the ascending limb of this relationship, cardiac muscle does not display such a biphasic response (Allen & Moss, 1987). In skinned preparations at maximal activation, the length-tension relationship of both skeletal and cardiac muscle more closely resembles the shallow portion of the ascending limb seen in skeletal muscle (Fabiato & Fabiato, 1975; ter Keurs et al. 1978; Allen & Kentish, 1985). During submaximal levels of activation, the length-tension relationship of cardiac muscle becomes quite steep compared to that of skeletal muscle (Fabiato & Fabiato, 1975; ter Keurs et al. 1978; Allen & Kentish, 1985). These differences have been attributed to a greater length dependence of tension development in cardiac muscle. This phenomenon, known as length-dependent activation, has as a cellular mechanism an increase in the sensitivity of the myofilaments to Ca²⁺ at greater sarcomere lengths (Hibberd & Jewell, 1982; Kentish et al. 1986) rather than enhanced release of sarcoplasmic Ca²⁺ with increases in length (Allen & Kurihara, 1982; Allen & Kentish, 1985). In the intact heart, increases in ventricular volume, the ventricular counterpart to muscle length, lead to an increase in ventricular output, independent of arterial resistance. Myofilament length-dependent activation thus provides for the cellular basis for the Frank-Starling law of the heart.

The ways in which length-dependent activation differs among various skeletal muscle types are currently unclear. It has been suggested that the magnitude of length-dependent activation is greater in skinned slow-twitch than fast-twitch skeletal fibres in both the rat (Stephenson & Williams, 1982) and hamster (Gulati et al. 1990). Contrary to these results, McDonald et al. (1997) found that the magnitude of the length effect was greater in rabbit fast-twitch muscle than rat slow-twitch muscle (McDonald et al. 1997). When length-dependent activation in cardiac muscle and skeletal muscle has been directly compared, the interpretation of the results is similarly unclear. Both McDonald et al. (1995), who compared rat cardiac myocytes to rat soleus, and Gulati et al. (1990), who compared hamster trabeculae to hamster soleus, reported that length-dependent activation in cardiac muscle was similar to that of slow skeletal muscle. However, if the data reported by McDonald et al. (1995) are expressed in terms of molar Ca²⁺ rather than in pCa units, length-dependent activation is seen to be greater in cardiac muscle compared to slow-twitch skeletal muscle, a direct result of differences in overall Ca²⁺ sensitivity between the muscle types studied. In addition, Gulati et al. (1990) reported a reduction in the effect of length in fast skeletal versus cardiac muscle, which is not consistent with the observation by McDonald et al. (1997) that fast skeletal muscle exhibits a greater length-dependent activation than slow skeletal muscle. Although these differences may be attributed to the isoform-specific genetic variations among the species studied, the inconsistencies between these various studies may also result from alternative ways of data representation and interpretation, and clearly need to be resolved.

Another important unanswered question is the nature of the molecular mechanism whereby information concerning muscle length is transmitted to the myofilaments giving rise to length-dependent activation. Recently, a prominent, straightforward, hypothesis has emerged to explain this mechanism (McDonald & Moss, 1995; McDonald et al. 1997; Fuchs & Smith, 2001). It proposes that the lateral spacing between the myofilaments influences cross-bridge reactivity independent of Ca²⁺ concentration. In intact skeletal and cardiac muscle, constant lattice volume forces the thin and thick filaments to move closer together as the muscle is stretched to longer lengths, a phenomenon that is also seen in muscle in which the membranes have been chemically removed (Rome, 1968; Matsubara & Elliott, 1972; Matsubara & Millman, 1974; Irving et al. 2000). As the interfilament spacing is reduced with increased sarcomere length, it is assumed that the probability of strong-binding cross-bridges is enhanced at subsaturating Ca²⁺ concentrations thereby increasing the sensitivity of the myofilaments to Ca²⁺.

In order to clarify the current state of uncertainty concerning the relative effect of length on Ca²⁺ sensitivity in various muscle types, we compared length-dependent activation in three striated muscle types from the rat: soleus (slow-twitch), psoas (fast-twitch) and cardiac trabeculae, under well controlled conditions. As part of the study, we also directly tested whether measurements of lattice spacing in relaxed skinned muscle preparations, by synchrotron X-ray diffraction, correlated with the mechanical observations of length-dependent activation. We show that length-dependent activation was greatest in cardiac trabeculae, less in psoas, and least in soleus muscle. In contrast, the relative change in lattice spacing in response to an incremental change in sarcomere length was similar in all three striated muscle types, suggesting that interfilament spacing alone may not be sufficient to explain these differences in length-dependent activation between striated muscle types.

METHODS

Cardiac and skeletal muscle preparation

Rats (LBNF-1 220–280 g) were anaesthetized (sodium pentobartbital 50 mg (kg body weight)⁻¹i.p. injection) and the hearts were rapidly excised and retrogradely perfused with a modified Krebs-Henseleit (K-H) solution with propranolol (5 μM), to block non-specific β-adrenergic activation, and carbamyl choline (10 μM), to enhance phosphatase activity (Gupta et al. 1994). 2,3-Butanedione monoxime (20 mm) was used to inhibit contraction presumably through the energetic stabilization of the unattached state of the myosin molecule (Backx et al. 1994). Thin, uniform, and unbranched trabeculae were dissected from the right ventricular (RV) free wall and transferred to an ice-cold standard relaxing solution ([EGTA], 10 mm; ionic strength, 180 mm) containing 1 % Triton X-100 for a minimum of 2 h to allow solubilization of all membranous structures. The fibres were used within 24 h of dissection. Single skeletal fibres were prepared as described previously with slight modifications (McDonald et al. 1997). The abdominal (descending) aorta was cannulated allowing perfusion of the peripheral tissues with the K-H solution referred to above. The rat soleus (slow skeletal) was dissected followed by removal of a large portion of the rat psoas (fast skeletal) muscle. Upon excision, each muscle was placed in a 50 % (v/v) glycerol/standard relaxing solution on ice. Muscle bundles of approximately 100 fibres were prepared from each muscle group and stored at −20 °C for no more than 7 days. Prior to data collection, single fibres were pulled free from the ends of the bundles and placed in a standard relaxing solution containing 1 % Triton X-100 to further remove any remaining membranous structures. Trabeculae (at SL = 2.1 μm) were 2–3 mm in length, 141 ± 0.02 μm in width and 108 ± 0.02 μm in thickness; skeletal fibres were 2–3 mm in length, 115 ± 0.01 μm (FSM) and 80 ± 0.01 μm (SSM) in width, and 91 ± 0.02 μm (FSM) and 74 ± 0.01 μm (SSM) in thickness.

Experimental apparatus

Cardiac trabeculae and skeletal fibres

The experimental apparatus for mechanical measurements of single skinned skeletal fibres or cardiac trabeculae was similar to that described previously (Janssen & de Tombe, 1997). The fibre was attached to the apparatus via aluminum T-clips to stainless steel hooks that extended from a high-speed servomotor (Cambridge model 308; ≈1 ms 90 % step response) and a modified silicon strain gauge force transducer (model AE801, SensoNor, Horten, Norway), both of which were attached to X-Y-Z manipulators mounted on a movable microscope stage. This allowed the suspended muscle to be lowered into a muscle trough (≈200 μl) milled from an anodized aluminum block that was temperature controlled (15 ± 0.1 °C). Force was recorded by a strip chart recorder and a personal computer (Apple PowerPC) equipped with an A/D converter using custom software written in LabVIEW (National Instruments; Austin, TX, USA) for off-line analysis (10 kHz per channel sampling frequency).

Sarcomere length was measured in a central segment of the muscle preparation by laser diffraction as described previously (ter Keurs et al. 1980; de Tombe & ter Keurs, 1990). Briefly, the fibre segment was illuminated by a 10 mW helium-neon laser beam. Because of damage inflicted on the ends of each muscle type during preparation, multiple populations of sarcomeres may shorten non-uniformly. To circumvent this problem an area no larger than 400 μm was illuminated by the laser in a central segment of the preparation where sarcomere length was kept constant (see below). The first-order diffraction band was focused onto a 512 element photodiode array (model RC 105, Reticon, Sunnyvale, CA, USA). The system was calibrated by placing gratings of known spacing in the same position of the muscle prior to each experiment. Spatial resolution was 10 nm.

Experimental protocol

Cardiac trabeculae and skeletal fibres

The Ca²⁺ sensitivity of force as a function of sarcomere length was determined as described previously (Janssen & de Tombe, 1997) with slight modifications. Prior to activation, the relaxing solution in the muscle bath was exchanged with a preactivating solution (see Solutions). Using an exchange volume that was three to four times the trough volume (3-4 min for each exchange) ensured complete exchange of the fluid. Ca²⁺-dependent force was determined by activating the muscle during a series of pre-activating- activating- relaxation cycles using a range of free [Ca²⁺] in the activating solutions selected in random order. During each activation, measurements were made at each of the three SLs, again selected in random order. To compensate for end-compliance created during preparation of the sample (Pollack & Krueger, 1976; ter Keurs et al. 1980; de Tombe & ter Keurs, 1990), SL was kept constant by appropriately adjusting muscle length via a micromanipulator during maximal and submaximal activations. The amount of SL shortening in our preparations during activation was about 0.05-0.1 μm as we have reported previously for isolated skinned cardiac muscle (de Tombe & Stienen, 1995; Wannenburg et al. 2000; Dobesh et al. 2002). The amount of stretching that was required to maintain SL in the central segment of either cardiac or skeletal muscle was about 10 % in this study, comparable to our previous studies in skinned cardiac fibres (Dobesh et al. 2002). After selection of each SL, force was allowed to reach a new steady state prior to recording any measurements; steady state force was reached within ≈10 s. Following the determination of force at the three SLs, a quick release prior to fibre relaxation was used to identify the zero force level. The length of the step release was not consistent within or between each experimental preparation but instead depended on muscle type and [Ca²⁺]. The step size of the quick release was the minimum step that allowed accurate determination of both steady state tension and the zero force level. Furthermore, this step release was not accompanied by restretch to the initial SL. This protocol was followed in order to minimize myofilament damage brought about by too large a step release or by restretch of an actively contracting muscle fibre. Because of the variable length of the step release, the force traces were used only for determination of active and passive force development and not as a measure of the rate of force recovery or the rate of force redevelopment. Subsequently, the amount of active force generated at each [Ca²⁺] was calculated as the difference between total force and relaxed, passive force that was assessed by slackening the fibre at each sarcomere length while it was in the relaxed state. To determine any decline in force-generating capability, the fibre was maximally activated at the beginning and at the end of the protocol. If the fibres did not maintain 90 % of initial maximal force then the fibre data were discarded. Furthermore, during activation, the fibre was scanned with the laser beam by using a movable stage to assess homogeneity of the fibre and uniformity of the sarcomeres. If sarcomere length was not uniform (within 0.05 μm) across the main portion of the muscle fibre (that is, excluding the area under and close to the T-clips) or if any fibre lost a visible first-order laser diffraction band at any point in the experimental protocol, it was discarded. Note that the damaged end (about 0.05-0.1 mm on either side of the muscle preparation) was not included in the SL control. Because the experimental criteria were strict, approximately 90 % of the muscle preparations were discarded.

X-ray diffraction experiments

The overall experimental arrangement has been described in detail previously (Irving et al. 2000). Briefly, experiments were performed on the BioCAT undulator-based beamline at the Advanced Photon Source, Argonne National Laboratory. The beam size at the sample position was collimated to about 0.3 × 0.8 mm, and about 0.040 × 1 mm (vertical × horizontal) at the detector and contained a maximum incident flux of ≈3 × 10¹² photons s⁻¹. Exposure times were 1 s. The small-angle camera had a 3 m sample to detector distance with a wavelength of 1.03 Å. For the X-ray studies, trabeculae were mounted between a force transducer and a servo motor in a small trough which allowed simultaneous collection of the X-ray patterns and viewing of the striation pattern using a long working distance objective (×40) of an inverted microscope) equipped with a CCD video camera. During the experiment, ≈20 ml of bathing solution was continuously pumped through the chamber using a peristaltic pump, except during a 2 s period during the digitization of the video striation images which were used to estimate sarcomere length. Low angle X-ray diffraction patterns were collected on a CCD-based X-ray detector. Spacings between the 1,0 and 1,1 equatorial reflections in the diffraction pattern were converted to d₁₀ lattice spacings using Bragg's Law, which can then, in turn, be converted to the inter-thick filament spacing by multiplying d₁₀ by .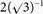

Individual trabeculae were stretched to arbitrary sarcomere lengths between 1.9 and 2.4 μm at the beginning of the experiment (skeletal fibres could be stretched to sarcomere lengths up to 4 μm). Sarcomere lengths were determined from the video image as described previously (Irving et al. 2000) and checked both before and immediately after the X-ray exposure. Fibre length was then systematically increased or decreased to collect at least 10–12 (at least every 50 nm) consecutive data points describing the relationship between sarcomere length and interfilament lattice spacing. Next, an additional two to four data points were collected at various sarcomere lengths back along the curve to check for reproducibility or hysteresis. The level of passive tension in the lattice was recorded when the X-ray exposure was taken.

Solutions

The K-H solution contained (mm): NaCl 118.5; KCl 5; MgSO₄ 1.2; NaH₂PO₄ 2; d(+)-glucose 10; NaHCO₃ 25; CaCl₂ 0.2. Three bathing solutions were used: a relaxing solution, a preactivating solution, and an activating solution. The ionic composition of these solutions is shown in Table 1. The ionic strength of the solutions was kept at 180 mm by adding the appropriate amount of potassium propionate. In addition, all solutions contained the following (mm): phosphocreatine 10; N,N-bis(2-hydroxyethyl)-2-aminoethanesulfonic acid (BES) 100; leupeptin 0.1; phenylmethylsulfonyl fluoride (PMSF) 0.1; dithiothreitol (DTT) 1, and 4 u ml⁻¹ creatine phosphokinase. The free Mg²⁺ and Mg-ATP concentration were calculated at 1 and 5 mm, respectively. Relaxing and activating solutions were appropriately mixed to obtain a range of free [Ca²⁺] (temperature kept constant at 15 °C). The pH was adjusted to 7.0 at 15.0 °C with KOH (see also Table 1).

Table 1.

Composition of relaxing, activating, and preactivating solutions

Solution	MgCl2	Na2ATP	EGTA	HDTA	Ca-EGTA	KProp
Relaxing	6.41	5.95	10	—	—	50.25
Preactivating	6.25	5.95	0.25	9.75	—	50.51
Activating	6.20	6.08	—	—	10	29.98

Ca-EGTA was made by mixing equimolar amounts of CaCl₂ and EGTA. In addition, all solutions contained the following (mM): phosphocreatine 10, N,N-bis(2-hydroxyethyl)-2-aminoethanesulfonic acid (BES) 100, leupeptin 0.1, phenylmethylsulfonyl fluoride (PMSF) 0.1, dithiothreitol (DTT) 1, and 4 u ml⁻¹ creatine phosphokinase. Free Mg²⁺ and Mg-ATP concentration was 1 and 5 mM, respectively. Relaxing and activating solutions was mixed to obtain the desired range of free [Ca²⁺] assuming an apparent stability constant of the Ca²⁺—EGTA complex of 10^6.39 at 15 °C. The preactivating solution with low Ca²⁺-buffering capacity containing 1,6-diaminohexane-N,N,N',N',-tetraacetic acid (HDTA) was used prior to the activating solution.

Data analysis

Force in submaximally activating solutions was expressed as fractions (F_rel) of the maximum force (F₀) at the same sarcomere length. The F₀ value used to normalize submaximal force was obtained by linear interpolation between successive maximal activations. Each individual Ca²⁺-force relationship was fitted to a modified Hill equation where F_rel=[Ca²⁺]ⁿ/((EC₅₀)ⁿ+[Ca²⁺]ⁿ), F_rel= relative force, EC₅₀=[Ca²⁺] at which force is half-maximal, n= slope of the Ca²⁺-force relationship (Hill coefficient). The ΔEC₅₀ was calculated as the difference in EC₅₀ at SL = 1.95 μm and 2.25 μm for each experiment. Likewise, the ΔLS was calculated as the difference in LS calculated at SL = 1.95 μm and 2.25 μm for each experiment. The differences between EC₅₀, ΔEC₅₀, LS and ΔLS for each group at each SL were analysed with a one-way ANOVA followed by Student's t test with a post hoc Bonferroni correction to assess differences among mean values. All data are shown as means ±s.e.m.

RESULTS

Ca²⁺ sensitivity of force

To assess the effect of sarcomere length (SL) on myofilament Ca²⁺ sensitivity, Ca²⁺-force relationships were determined at three sarcomere lengths (1.95 μm, 2.10 μm and 2.25 μm) in rat cardiac trabeculae, soleus (slow-twitch) and psoas (fast-twitch) muscles. The experimental conditions and solutions were identical for each group of fibres studied. Original recordings of force from a typical experiment are illustrated in Fig. 1. Steady state force was measured at each SL during each activating cycle; the zero force level was identified by instituting a quick ramp shortening in muscle length just before introduction of relaxing solution into the bath (active force was calculated as the difference between total force and passive, relaxed force at each SL). Figure 1A shows a series of such quick length-release steps recorded at varying levels of activation ([Ca²⁺] in micromolar indicated on the right of each tracing) for each muscle type: cardiac trabeculae (CM), psoas (FSM), and soleus (SSM) muscles (SL = 2.10 μm), indicated at the top of each column of raw data tracings. A final maximal activation was administered to assess muscle preparation integrity. The relationship between active force development and Ca²⁺ concentration ([Ca²⁺]) for each muscle type was fitted to the Hill equation (see Methods) as illustrated in Fig. 1B. Although not clear from these tracings, maximal developed force at this particular SL, on average was 16 % and 20 % greater in SSM and FSM over CM, respectively. It is clear, however, that the force developed at submaximal concentrations depended on muscle type and the [Ca²⁺], as evidenced by the Ca²⁺-force relationships in Fig. 1B and by the pooled data in Fig. 2.

Figure 1. Method used to measure active force development under maximal and submaximal activation in rat cardiac trabeculae (CM), rat psoas (FSM), and soleus (SSM) muscle fibres.

A, original recordings of force, normalized as force (unit area⁻¹, at the indicated [Ca²⁺] (in μM) to the right of the tracings for each striated muscle type (SL = 2.10 μm). A quick release was used to determine zero force level (see Methods). The quick release step size depended on muscle type and [Ca²⁺]; it was 10–20 % of muscle length. Calibrations as indicated. B, Ca²⁺-force relationship, constructed from data obtained as illustrated in A. The continuous lines indicate the Hill fit to the data obtained from the indicated muscle type (above each data column); the dotted lines represent the Hill fit obtained from CM (middle panel), or CM and FSM (right panel). C, typical CCD images of X-ray diffraction patterns obtained from each muscle type at the same SL (SL = 2.10 μm) indicative of the change in lateral separation of the myofilaments in each muscle. The 1,0 and 1,1 equatorial reflections are as indicated.

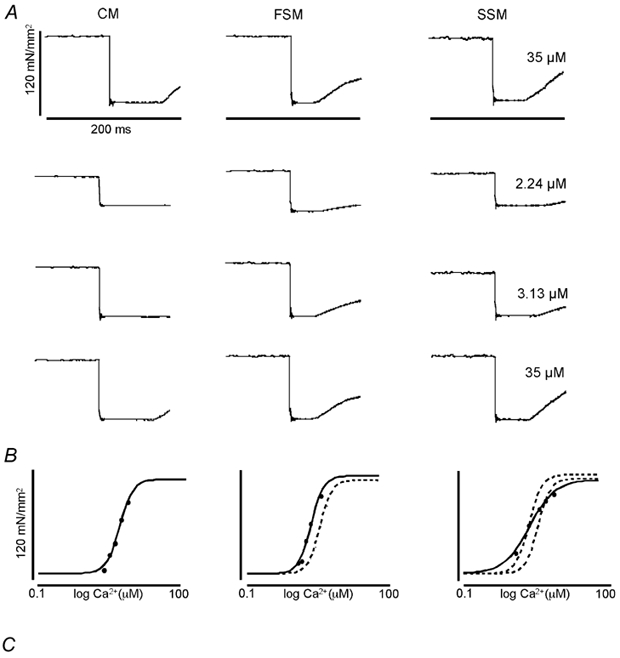
Figure 2. Ca²⁺-dependent force development in skinned cardiac trabeculae, psoas and soleus muscle fibres.

A, Ca²⁺-force relationship at three sarcomere lengths (1.95 μm (circles), 2.10 μm (squares), 2.25 μm (triangles)) of psoas (FSM; open symbols) and soleus (SSM; filled symbols) single muscle fibres. B, Ca²⁺-force relationship at the same three sarcomere lengths as in A of psoas (FSM; open symbols) and cardiac trabeculae (CM; filled symbols). The data were normalized to Ca²⁺-saturated (maximum) force at each SL.

The average parameters of the Hill fit obtained in each individual trabecula are summarized in Table 2. Increasing SL from 1.95 μm (circles on Fig. 2) to 2.10 μm (squares) to 2.25 μm (triangles) caused a leftward shift of the Ca²⁺-force relationship and a decrease in the EC₅₀ ([Ca²⁺] at which tension is half-maximal) for each experimental group. Comparing the Ca²⁺-force relationships for FSM and CM, it is apparent that the Ca²⁺ sensitivity of tension development was greater corresponding with a reduced EC₅₀ in FSM than CM at all three SLs studied (Fig. 2B and Table 2). In addition, neither muscle type (CM or FSM, only) nor changes in SL had an appreciable, significant, effect on the steepness of the Ca²⁺-force relationship (Hill coefficient; Table 2). Ca²⁺ sensitivity of tension development in SSM was also greater than that of CM over most of the concentration range. Previous studies have employed pCa₅₀ (-log[Ca²⁺]) to index Ca²⁺ sensitivity (Stephenson & Williams, 1982; McDonald et al. 1997). Employing this method also revealed a statistically significant increase in Ca²⁺ sensitivity in FSM and SSM when compared to that of CM. From the Ca²⁺-force relationships, it is also apparent that although the overall Ca²⁺ sensitivity of SSM was not very different from FSM at the SLs studied, the slope of the Ca²⁺-force relationship (Hill coefficient) in SSM was markedly decreased when compared to CM and FSM (Table 2).

Table 2.

Average values of Hill fit to Ca²⁺-force curves for CM, FSM and SSM muscle fibres at sarcomere lengths of 1.95, 2.10 and 2.25 μm

	CM (n = 7)			FSM (n = 8)			SSM (n = 8)
	1.95	2.10	2.25	1.95	2.10	2.25	1.95	2.10	2.25
EC50 (μM)	3.40 ± 0.15	3.10 ± 0.16	2.75 ± 0.16	2.45 ± 0.12*	2.24 ± 0.11*	2.03 ± 0.10*	2.36 ± 0.13*	2.22 ± 0.14*	2.11 ± 0.15*
pCa50 (μM)	5.47 ± 0.02	5.51 ± 0.02	5.56 ± 0.03	5.61 ± 0.02*	5.65 ± 0.02*	5.70 ± 0.02*	5.63 ± 0.03*	5.66 ± 0.03*	5.68 ± 0.03*
Hill coeff.	6.50 ± 0.29	6.01 ± 0.59	5.40 ± 0.31	5.63 ± 0.76	5.82 ± 0.30	5.61 ± 0.57	2.57 ± 0.17*	2.39 ± 0.19*	2.34 ± 0.21*
				CM	FSM	SSM
			ΔEC50	0.65 ± 0.04	0.42 ± 0.05*	0.25 ± 0.04*†
			ΔpCa50	0.09 ± 0.01	0.08 ± 0.01	0.05 ± 0.01*†

Each column indicates the averaged EC₅₀, pCa₅₀, and Hill coefficient values of the Ca²⁺-force curves obtained from rat cardiac trabeculae (CM), rat psoas (FSM), and soleus (SSM) muscle fibers at the SLs listed. The lower part of the table indicates the average length-dependent changes in Ca²⁺-sensitivity (ΔEC₅₀ or ΔpCa₅₀) for the three muscle types calculated as the difference in EC₅₀ and pCa₅₀ between SL = 2.25 μm and 1.95 μm

^*P < 0.01 vs.CM

^†P < 0.01 vs.FSM).

To quantify the magnitude of the effect of length change on the Ca²⁺ sensitivity of force in each muscle type studied, i.e. length-dependent activation, we defined ΔEC₅₀ as the difference in the [Ca²⁺] at which force is half-maximal (EC₅₀) at the long (2.25 μm) and the short (1.95 μm) sarcomere lengths for which there are data in all three muscle types and which encompass most of the working range of the heart. So defined, length-dependent activation was greater in CM (0.65 ± 0.04 μM) than in FSM (0.42 ± 0.05 μM) that, in turn, was greater than in SSM (0.25 ± 0.04 μM) (Table 2). However, when EC₅₀ values were converted to pCa₅₀, ΔpCa₅₀ determined from CM was not significantly different from ΔpCa₅₀ determined from FSM. Both of these values were significantly greater than the ΔpCa₅₀ determined from SSM (Table 2).

Figure 3 shows that within the working range of SLs in the heart, there was an inverse, linear relationship between SL and Ca²⁺ sensitivity (EC₅₀). The slope of the SL-EC₅₀ relationship was steepest in cardiac muscle (-2.15 ± 0.14 μmol (μm SL)⁻¹), less in fast skeletal muscle (-1.40 ± 0.16 μmol(μm SL)⁻¹; P < 0.01 vs. CM) and least in slow skeletal muscle (-0.83 ± 0.13 μmol(μm SL)⁻¹; P < 0.01 vs. CM and FSM) demonstrating the relative degree of length-dependent activation in the three muscle types.

Figure 3. Relationship between Ca²⁺ sensitivity (EC₅₀) and sarcomere length in skinned cardiac (CM), psoas (FSM), and soleus (SSM) muscle fibres.

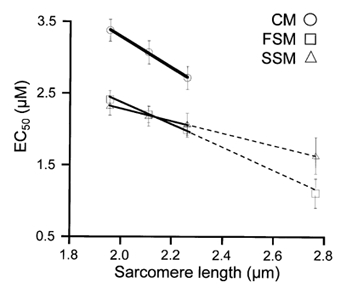
The relationship in each muscle type was linear over the range of sarcomere lengths studied (1.95 μm, 2.10 μm and 2.25 μm) representing the working range of the heart. The slope of these relationships reflects the magnitude of length-dependent activation; the steeper the slope, the greater the shift in Ca²⁺ sensitivity with changes in length. The dashed line represents the linear extrapolation of these data to SL = 2.75 μm consistent with the Ca²⁺ sensitivity (EC₅₀) obtained in a separate group skeletal muscle fibres at that length.

It has been previously demonstrated that in both cardiac (Fabiato & Fabiato, 1978) and skeletal muscle (Endo, 1972) the Ca²⁺ sensitivity of tension increases at SLs well beyond optimal filament overlap. Here we demonstrate that there was a further, linear decrease (as indicated by extrapolation of the best fit line calculated for the short SL data) in the EC₅₀ at a high SL (SL = 2.75 μm) determined in psoas (1.16 ± 0.20 μM; n= 3) and soleus (1.68 ± 0.25 μM; n= 3) muscle fibres (Fig. 3). It should be noted that a small, but significant passive force was generated at this longer SL, which was subtracted from total force to derive developed force. Although we attempted to eliminate this confounding factor by accurate SL control at this high SL, the level of confidence of Ca²⁺ sensitivity at this high SL in skinned isolated skeletal muscle was lower than that at the shorter SL range.

Ca²⁺-saturated, maximum force (P₀) decreased 20 %, on average, with a reduction in SL from 2.25 to 1.95 μm in CM (data not shown) consistent with previous reports (ter Keurs et al. 1980; Dobesh et al. 2002). In contrast, the decrease in maximum force with the same reduction in SL was 11 % for both FSM and SSM. The decrease in maximum Ca²⁺-saturated force with a length change from 2.25 to 2.10 μm in both FSM and SSM was 3.5 %, while the decrease in maximum force resulting from a reduction in sarcomere length from 2.10 to 1.95 μm was 7.5 %. Though the length-tension relationship was not extensively examined in this study, these findings are consistent with previous studies on the length-tension relationship in skeletal and cardiac muscle reports (Gordon et al. 1966; ter Keurs et al. 1980). Maximally activated cardiac muscle maintains a proportional relationship between length and tension over the working SLs of the heart (1.9-2.3 μm) whereas skeletal muscle exhibits a significant plateau phase corresponding to optimal filament overlap at SLs between ≈2.10 and 2.20 μm (Gordon et al. 1966; ter Keurs et al. 1978; Allen & Kentish, 1985; Allen & Moss, 1987). Nevertheless, the differences between the muscle types at this sarcomere length range are small, suggesting a similar contribution of physical factors due to myofilament geometry on cross-bridge availability (Allen & Kentish, 1985).

Relationship between lattice spacing and sarcomere length

Although there have been measurements of the relationship between lattice spacing and SL in both skeletal (Rome, 1968; Bagni et al. 1994) and cardiac (Irving et al. 2000) muscle, there has not been a comparison between both muscle types from the same animal under identical conditions. Therefore, we simultaneously measured myofilament lattice spacing (LS) and SL in relaxed skinned cardiac trabeculae, single soleus and psoas muscle fibres using synchrotron X-ray diffraction (Irving et al. 2000; Fig. 4). In each muscle type, lattice spacing showed a similar inverse dependence on sarcomere length consistent with previous results (Rome, 1968; Bagni et al. 1994; Irving et al. 2000). Despite a significant difference in the elevation of the relationship in each muscle group (LS determined at SL = 2.10 μm was (nm) 42.57 ± 0.21, 44.26 ± 0.46, and 45.21 ± 0.33 in CM, SSM, and FSM, respectively), the slope of the SL-LS relationship was not significantly different among the experimental groups (-5.35 ± 0.70, −5.11 ± 0.23 and −5.26 ± 0.65 in CM, SSM and FSM, respectively).

Figure 4. Averaged lattice spacing as a function of sarcomere length.

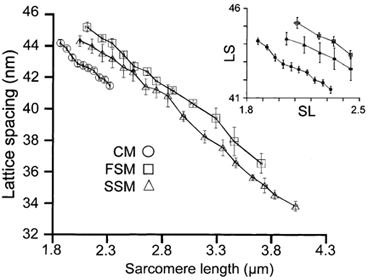
Shown are the sarcomere length-lattice spacing relationships determined from the three striated muscle types studied. These relationships were obtained by placing the sarcomere length on 0.05 μm (CM) or 0.10 μm (FSM and SSM) intervals into bins and averaging the average value per fibre bin. Inset, sarcomere length-lattice spacing relationships scaled to the working range of cardiac muscle.

We defined ΔLS to be the change in lattice spacing over the same range of SLs used to determine the ΔEC₅₀ in order to quantify the sensitivity of LS to an incremental change in SL. The ΔLS when the SL was increased from 1.95 to 2.25 μm was not significantly different between muscle types (1.55 ± 0.17, 1.54 ± 0.05 and 1.58 ± 0.21 in CM, SSM and FSM, respectively). The working range of cardiac muscle is limited to a SL range of 1.85 to 2.3 μm. Therefore, to facilitate comparison, the inset to Fig. 4 shows the relationship between SL and lattice spacing of the three muscle types over just this range. It should be noted that the resting SL of single skeletal fibres used in this study was consistently greater than 2.25 μm whereas the resting SL of cardiac trabeculae was commonly 1.85 μm. Thus, there were no lattice spacing measurements in soleus and psoas muscle < 2.10 μm (Fig. 4, inset).

DISCUSSION

While previous studies of the differences in the length- tension relationships between cardiac muscle and skeletal muscle suggested that length-dependent activation was more pronounced in cardiac muscle than in skeletal muscle (Gordon et al. 1966; Fabiato & Fabiato, 1975; ter Keurs et al. 1980; Allen & Kentish, 1985) other studies (Stephenson & Williams, 1982; Gulati et al. 1990; McDonald & Moss, 1995; McDonald et al. 1997) concerning the relative degree of length dependent activation in cardiac and fast and slow skeletal muscle were mutually inconsistent, possibly due to isoform-specific genetic variations among the species studied (see Introduction). Moreover, in several of these studies (Stephenson & Williams, 1982; Gulati et al. 1990; McDonald & Moss, 1995; McDonald et al. 1997) the range of sarcomere lengths over which length-dependent activation was studied was considerably higher (SLs 1.8 to >> 2.3 μm), well within the descending limb of the length-tension relationship. In addition, strict length control methods were not employed in some of these studies. Here we attempted to resolve the discrepancies by directly comparing the length-dependent properties of each muscle type in a single species (rat) under identical experimental conditions with strict sarcomere length control. Our data demonstrate that length-dependent activation as indexed by the ΔEC₅₀ parameter (difference in half-maximal tension at the long and short sarcomere lengths) was greatest in cardiac muscle followed by fast-twitch muscle and least in slow-twitch skeletal muscle. However, when the data were converted to pCa, despite consistency in relative Ca²⁺ sensitivities of the muscle types studied, length-dependent activation as indexed by the ΔpCa50 parameter indicated no significant difference between FSM and CM. Nevertheless, length-dependent activation was significantly reduced in SSM compared to both CM and FSM, independent of the choice of indexing parameter. The effect of parameter choice to index Ca²⁺ sensitivity and length-dependent activation has similarly been seen to affect data interpretation. McDonald et al. (1995) reported a similar effect of length change in slow skeletal muscle when compared to cardiac muscle. However, if the values for Ca²⁺ sensitivity and, consequently, length-dependent activation are expressed in terms of molar [Ca²⁺] rather than in pCa units, length-dependent activation is seen to be greater in cardiac muscle compared to slow-twitch skeletal muscle. It has yet to be determined which of the two parameters to index Ca²⁺ sensitivity is more appropriate.

Consistent with previous studies, the level of cooperativity as indexed by the slope of the Ca²⁺-force relationship (Hill coefficient) was greater in fast skeletal and less in slow skeletal muscle. However, the Hill coefficient values obtained for skinned cardiac trabeculae were similar to our previous findings (Dobesh et al. 2002) and were larger than values published by other investigators (Gulati et al. 1990; Gao et al. 1994; McDonald et al. 1995). Incidentally, the cooperativity measured in cardiac trabeculae was similar to that of fast skeletal muscle in contrast to previous experiments (Morimoto & Ohtsuki, 1994). In addition, although this was not the primary focus of our study, sarcomere length did not appear to affect the apparent level of cooperativity in either muscle type. This observation is consistent with previous studies in skeletal muscle (Stephenson & Williams, 1982; Gulati et al. 1990; McDonald et al. 1997) and a recent detailed study in skinned cardiac muscle by our laboratory (Dobesh et al. 2002). Apparently, the molecular mechanisms of length-dependent activation do not include modulation of cooperativity of myofilament activation.

In contrast, the values obtained for the level of cooperativity for fast skeletal muscle in this study were lower than previously reported (Moss et al. 1991; McDonald et al. 1997). However, a direct comparison between this study and the aforementioned studies is difficult because of differences in the determination of the Hill coefficients (see below). Because contracting muscle is operating at a non-equilibrium stationary state, the Hill coefficient may be greater than the number of interacting sites for the system (Shiner & Solaro, 1984). Therefore, a more accurate representation of apparent cooperativity is the slope of the lines fitted to the data following Hill plot transformation (McDonald & Moss, 1995). In general, this type of analysis yields a biphasic relationship with the slope of the upper phase less than the slope of the lower phase (McDonald & Moss, 1995). Such was the case in the previous studies in which the lower phase had slopes that were much greater than the Hill coefficients determined in this study (Moss et al. 1991; McDonald et al. 1997). Despite a well-defined Ca²⁺-force relationship and EC₅₀ (the primary focus of this study), there were too few data points to provide reliable statistical analysis following the Hill plot transformation to allow the precise determination of the steep force-calcium relationship in skeletal muscle. Hence, the level of cooperativity in that muscle type may have been underestimated in our study.

The significance of these data relates to the fact that there is a single Ca²⁺ regulatory site on cardiac troponin C (Holroyde et al. 1980) compared to the two Ca²⁺ regulatory sites on fast skeletal troponin C (Potter & Gergely, 1975). Consequently, in light of the necessity of cooperative interaction along the thin filament for full myofilament activation (Bremel & Weber, 1972; Brenner, 1988; Lehrer, 1994), the similarity in levels of cooperativity indicates that the influence of strong-binding cross-bridges on the binding of additional cross-bridges is similar in cardiac and fast skeletal muscle. However, recent studies indicate that the influence of strong-binding cross-bridges may be greater in cardiac muscle than fast skeletal muscle since NEM-S1 (a strong-binding, non-tension-generating derivative of the myosin head) appears to potentiate activation of the thin filament and additional cross-bridge binding more in cardiac muscle (Fitzsimons et al. 2001a,b). Furthermore, it has been shown that the rate of force redevelopment after release-restretch protocols varies more in fast skeletal muscle than in cardiac muscle over a similar range of [Ca²⁺] (Metzger & Moss, 1990; Wolff et al. 1995; Campbell, 1997). It has been postulated that the mechanism of such an observation can be explained by greater cooperative interactions in fast skeletal muscle than cardiac muscle especially at low levels of activation (Moss, 1992; Campbell, 1997). Fitzsimons et al. (2001a) also suggest that despite similar rate constant of force redevelopment (k_tr) values for both fast skeletal and cardiac muscle (Wolff et al. 1995; McDonald et al. 1997), k_tr may not be maximal in fast skeletal fibres. Thus, in fast skeletal muscle, the model of cooperativity must include near-neighbour effects in addition to an increase in cross-bridge kinetics (Fitzsimons et al. 2001a).

One must account, then, for the significant differences in length-dependent activation in fast skeletal, slow skeletal and cardiac muscle despite similar levels of cooperativity in cardiac and fast skeletal muscle. The ability to increase tension development with an increase in sarcomere length depends on the recruitment of strong-binding cross-bridges. Support for this has been provided by studies in which the strong binding of cross-bridges was promoted by NEM-S1 (Swartz & Moss, 1992; Fitzsimons & Moss, 1998; Fukuda et al. 2000) or a decrease in ionic strength (Brenner et al. 1982; Smith & Fuchs, 1999) that reversibly eliminated the length dependence of Ca²⁺ sensitivity. The mechanism by which longer lengths potentiate the binding of strong cross-bridges is thought to involve changes in the lateral separation of the thick and thin filament with changes in muscle length, a phenomenon observed many years previously (Elliott et al. 1963; Rome, 1968). Consequently, as the interfilament spacing is reduced the probability of strongly bound cross-bridges is assumed to be greater (McDonald & Moss, 1995; Fuchs & Wang, 1996). If this mechanism is correct one would expect a parallel correlation between the slopes of the SL-EC₅₀ relationship with the slopes of the LS-SL relationships. Whereas there were significant differences in the slopes of the length-EC₅₀ relationship (Fig. 3) in accord with the extent of length-dependent activation in each muscle type, the slopes of the relationships between sarcomere length and lattice spacing were all comparable (Fig. 4). Consequently, this observation suggests that the differences in the effect of SL change on Ca²⁺ sensitivity (EC₅₀) between muscle types cannot simply be explained by the absolute changes in interfilament spacing following similar length changes. Hence, these data support the notion that the change in the resting myofilament lattice spacing in itself is not sufficient to impart the changes of Ca²⁺ sensitivity with length and, thus, cannot explain the differences in length-dependent activation between striated muscle types. In addition to these findings, we have recently challenged the role of interfilament spacing as an absolute modulator of Ca²⁺ sensitivity in cardiac muscle (Konhilas et al. 2002).

Our study compared the relationship of active Ca²⁺-dependent force development at three SLs to the resting myofilament lattice spacing at the same (and other) SLs. It has been illustrated in skeletal muscle that myofilament lattice spacing decreases with increasing isometric tension (Brenner & Yu, 1985). Similarly, skinned fibres in a rigor state have a reduced interfilament spacing compared to the same fibre under relaxed conditions (Kawai et al. 1993). The suggestion is, therefore, that the measured lattice spacing in relaxed muscle at a particular SL may not functionally be equivalent to activated fibres at the same SL. Thus, whether the steepness, asymmetry, or SL dependence of the Ca²⁺-force relationship is affected by changes in interfilament spacing during the contraction cannot be determined or excluded based on the data collected in this study. Also, our study employed the use of skinned preparations in all protocols. It has been observed that the effect of SL on Ca²⁺ sensitivity appears to be less in intact myocardium compared with skinned myocardium (Komukai & Kurihara, 1997), despite the similar impact of SL changes on interfilament spacing (Irving et al. 2000). Hence, there appear to be differences between intact and skinned muscle, such that our results on skinned muscle preparations may not be applicable to intact muscle.

Given the role of thin filament proteins during myofilament activation (Solaro & Rarick, 1998), it is likely that communication among these players is an important determinant of the length-dependent characteristics of the various muscle types. Because of the differences in specific protein isoforms among striated muscle types, these data suggest that, although a role for the interfilament spacing cannot be completely excluded, thin filament regulatory components must be important in adjusting the gain of the Ca²⁺-binding-thin filament activation-transduction process with changes in muscle length. It was previously hypothesized that the thin filament protein troponin C (TnC) was responsible for the length-dependent properties of striated muscle (Babu et al. 1988; Gulati et al. 1990). However, evidence presented by the Moss group strongly suggested that TnC may not be involved in the primary mechanism of this phenomenon (Moss et al. 1991; McDonald et al. 1995). In addition, length-dependent activation whether indexed by a ΔEC₅₀ or ΔpCa50 parameter was greater in CM than SSM, both of which have the identical isoform (cardiac) of TnC. Recent evidence indicates another thin filament component, troponin I (TnI), as a potential regulatory of length-dependent activation. Substitution of the cardiac isoform of TnI with the slow-skeletal isoform reduced the length-dependent shift in Ca²⁺ sensitivity (Arteaga et al. 2000). Moreover, phosphorylation via the cAMP-dependent protein kinase pathway either reduced (Kajiwara et al. 2000) or enhanced the length-dependent properties of cardiac muscle (Komukai & Kurihara, 1997; Konhilas et al. 2000). The importance of additional sarcomeric proteins in imparting the length-dependent characteristics of striated muscle has recently been demonstrated; these include titin (Le Guennec et al. 2000; Cazorla et al. 2001; Granzier & Labeit, 2002) and troponin T (Akella et al. 1995). Further experiments are necessary to examine the role of individual thin and thick filament protein isoforms in length-dependent activation.

In summary, the current study defined the length-dependent properties of slow-twitch, fast-twitch skeletal, and cardiac muscle under identical conditions. It was shown that length-dependent activation was greatest in cardiac muscle and least in slow skeletal muscle. In addition, cooperativity, as determined by the Hill coefficient was similar in cardiac and fast skeletal muscle, both of which were greater than the cooperativity in slow skeletal fibres. The differences in the length-dependent properties among the striated muscle types may not solely be explained by the differences in the response of interfilament spacing to changes in muscle length in relaxed, skinned isolated muscle preparations.