Experience-dependent, asymmetric expansion of hippocampal place fields

Abstract

Theories of sequence learning based on temporally asymmetric, Hebbian long-term potentiation predict that during route learning the spatial firing distributions of hippocampal neurons should enlarge in a direction opposite to the animal’s movement. On a route AB, increased synaptic drive from cells representing A would cause cells representing B to fire earlier and more robustly. These effects appeared within a few laps in rats running on closed tracks. This provides indirect evidence for Hebbian synaptic plasticity and a functional explanation for why place cells become directionally selective during route following, namely, to preserve the synaptic asymmetry necessary to encode the sequence direction.

Rat hippocampal neurons fire in a spatially selective fashion (1). Recently it was proposed that the hippocampus might store route information through Hebbian (2) asymmetric strengthening of synapses between cells with overlapping place fields on a route (3–7). A prediction of this asymmetric synaptic enhancement was an expansion of place fields and a shift in their centers of mass in a direction opposite to the direction of the route. The present study was designed to investigate whether the predicted asymmetric place field expansion indeed occurs.

Evidence for the reactivation of sequence information has been demonstrated in hippocampal neuronal ensembles during slow-wave sleep following repetitive, unidirectional traversal of a closed route (8). There is also abundant evidence, from experiments conducted under conditions of artificial, electrical stimulation, that a potential mechanism for experience-dependent synaptic change, with the necessary associative (9) and asymmetric properties, exists (10–12). The observation of the predicted, asymmetric expansion of place fields would provide strong indirect evidence that such processes are actually at work during the registration of sequences of spatial experience.

MATERIALS AND METHODS

Seven Fischer-344 rats were pretrained to run for food reinforcement at fixed locations on triangular (60 cm per side) or rectangular (66 cm × 37 cm) tracks (each 6 cm wide) situated in a moderately illuminated room with fixed landmarks. Populations of hippocampal CA1 pyramidal cells were simultaneously recorded using multiple “tetrodes” as the rats performed the task, using methods described previously (13). The position and orientation of two head-mounted diode arrays (separated by 15 cm) was measured by a video camera (0.41 cm per pixel sampled at 20 Hz). Data were analyzed (i) from one session each from seven rats, in which they ran unidirectionally around the apparatus, and (ii) from three additional sessions in two of the rats, in which they followed the same route on consecutive days. Data were also analyzed from four sessions in three animals that ran on a novel track immediately after running on the familiar one.

Because place-specific discharge appears primarily during movement, and because the rats stopped at the food locations, place fields around food locations were eliminated from the analysis. Some cells had two or more place fields, which were treated independently. For the main experiment a total of 186 place fields were analyzed from 172 place cells from seven rats in a total of 10 recording sessions. Repeated measures ANOVA was used to assess changes in place field size and location over laps. All rats ran at least 17 laps per session, all of which were included in the primary analysis. Additional analyses were performed on the first and last lap of each session. The closed linear tracks were mapped onto straight lines, with increasing coordinates in the rat’s direction of motion. The location of each spike was defined as the component of the rat’s head position on this line at the time of spike occurrence. A place field was defined as a region of high average firing rate. Its boundaries were defined by the points where the mean firing rate fell to <10% of the peak rate for 20 pixels. Subsequent measurements of field characteristics and dynamics were confined to this region. For each lap, the location-specific firing rate was computed by evaluating the number of spikes at each location divided by the time the rat spent there. The location of the center of each place field was computed by finding the center of mass of its spatial firing rate distribution, averaged over the 17 laps. Fig. 1 shows the distributions of spatial firing relative to the place field center for all 186 place fields for lap 1 and lap 17. There is an increase in the size of the mean place field and an overall backward shift in the distribution of spikes.

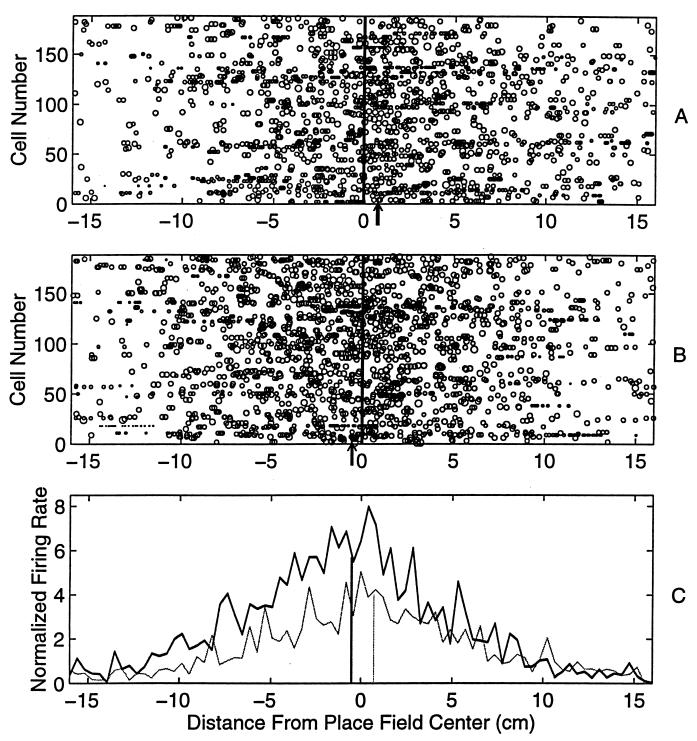
Figure 1.

The location of each spike (measured relative to the respective average place field center) during the first (A) and the seventeenth (B) laps, is shown for all the 186 fields recorded from seven rats in 10 sessions. The size of each circle is proportional to the log of the instantaneous firing rate at that location during the lap. (C) The histograms of the total firing rate at each location during the first and the last laps are also shown (light curve, lap 1; solid curve, lap 17. Bin width = 0.41 cm, which corresponds to the resolution of the position tracking camera). The center of mass of the firing rate distribution (indicated by arrows in A and B, and by vertical lines in C) of all the spikes in the last lap is shifted backwards (by ≈1.4 cm) compared with that during the first lap. There was a 66% increase in the total firing rate. Thus, with experience, the location of the place fields shifted backwards and the place cells fired more spikes.

RESULTS AND DISCUSSION

Lap-specific place field size was defined as the sum of the firing rates for all the spikes within the field boundaries. This corresponds to the integral of the firing rate distribution. For noisy, single-trial data containing few spikes this measure is far more robust than others, such as the distance between the first and last spikes. Moreover, it is consistent with the more traditional estimates of size involving some arbitrary cutoff points, as can be verified by applying an arbitrary threshold to the distributions in Fig. 1, and estimating the distances between the intersections of the distribution with these lines. The lap-specific place field location was defined as the location of the center of mass of the lap-specific firing rate distribution relative to the center of the average place field. To characterize the behavior of the population of place fields, and to ensure that the results were not dominated by cells with large place field size, the lap-specific place field size was normalized by the the average size of the respective place field. As shown in Fig. 2A, the place field size increased rapidly and asymptotically with experience (F_169,16 = 4.00, P < 0.0001). As the place fields expanded, their centers of mass shifted backwards (Fig. 2B; F_112,16 = 2.97, P < 0.0001). For the position analysis, df for cells is less than for the size analysis because position could not be defined in cases where a cell fired no spikes on one or more laps. To estimate the consistency of these phenomena, a similar analysis was performed separately for all cells within each data set, in which the first and last laps (ranging from 17 to 90) run by the rat were compared. In all 10 cases, the average place field size on the last lap was larger than on the first, and its center of mass was shifted backwards (P < 0.0001 and P < 0.001, respectively, paired Student’s t test).

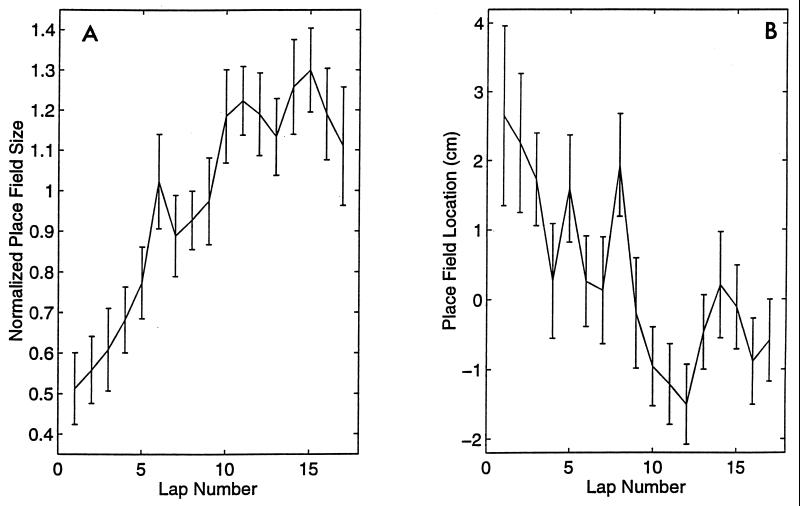
Figure 2.

(A) The relative place field size (mean ± SE) of the 186 place fields increased significantly and asymptotically over the 17 laps on the track (P < 0.0001). (B) The location of each place field on each lap (for which there was at least one spike) was calculated relative to the center of mass of the corresponding average place field for all 17 trials. The place field location (mean ± SE) for 186 place fields is shown as a function of lap number. There was a significant, asymptotic backward shift in the relative field locations over the 17 laps on the track (P < 0.0001). The location parameter was significantly inversely correlated (r = −0.9) with field size.

Each rat had been trained on the respective apparatus for several weeks prior to these recording sessions. Thus, the observed changes must occur during each session and must fade away (at least to a large extent) between recording sessions (i.e., at least within 24 h). This was confirmed by data from two of the rats that were recorded from on three consecutive days (i.e., six data sets). Each data set exhibited the asymmetric place field expansion.

Several tests were conducted to minimize the possibility that the effects merely reflect excitability changes due to recent activity of the cells or some other nonspecific factor. In a separate set of recordings, three rats were allowed to run on a relatively unfamiliar route (a different track adjacent to the familiar one) immediately after running the familiar route. As expected from other studies (14), some of the cells (43 out of 72) that fired on the first route also fired on the second (although in an unrelated order). For these cells, the place field sizes were 64% smaller on the first lap on the novel route than their final size on the last lap on the familiar route (t₅₈ = 3.79, P < 0.0002) and again increased rapidly (F_33,16 = 4.82, P < 0.0001), and the place field location shifted backward (F_10,16 = 3.23, P < 0.001) with experience (Fig. 3). The overall expansion for these cells was 100% and 124% in the familiar and novel routes, respectively. Thus, for cells whose fields had already expanded and shifted in the first route, these changes did not transfer between routes. Therefore, the changes are unlikely to be due to the recent history of either locomotion or spike discharge per se. Nor can the increase in firing rate in the place fields be accounted for by changes in running velocity. It is well documented that firing rate increases with running speed (15). On average, the running speed decreased (nonsignificantly) over the 17 laps by ≈10%, which would result in an insignificant decrease in place field size.

Figure 3.

(A) Relative place field size (mean ± SE) in the second environment, for 43 place cells that had a place cells in the first environment. The place field size again increased asymptotically by 124% in 17 laps (P < 0.0001) and (B) the place field location (mean ± SE) again shifted backwards by 2.5 cm (P < 0.001), thus indicating that the effect is environment specific and could not be attributed to general excitability changes.

A possible explanation of the backward shift in place field location might be that the angle of head tilt increased, which, because of the elevation of the tracking diodes above the rat’s head, would lead to a spurious backward shift in the apparent place field location. This possible source of error was ruled out by an analysis of the apparent distance between two tracking diode arrays. The apparent distance between the diodes did not change significantly over the 17 laps. Furthermore, the fluctuations in the distance between the diodes was uncorrelated with the amount of shift in the place field location (r = 0.1).

The results can be understood as follows. The place cells are activated in the same temporal sequence every time the rat follows the same route. Experimentally induced long-term potentiation of hippocampal synapses is associative (9) and temporally asymmetric (10–12), occurring only if the postsynaptic neuron is depolarized after and within a short duration of depolarization of the presynaptic neuron. Thus, synapses between place cells may be strengthened asymmetrically, i.e., the synapses from the cells that fire earlier on the maze onto those that fire later on the maze would become stronger; however the reciprocal connections would be relatively less affected. Such an asymmetric strengthening would induce the postsynaptic neurons to fire earlier and more robustly, thereby enlarging the place fields and moving their average locations backwards.

A natural question is whether the observed asymmetry would cancel out if the rat ran repeatedly back and forth along a route in both directions. It turns out that this experiment is not possible because, under such circumstances, the hippocampus encodes the forward and return journeys using different sets of place cells (15–17). Whereas, in random foraging situations, place cells fire in the place field irrespective of the direction of travel of the rat, on a fixed route the place field distributions in the two directions are orthogonal. Neither the mechanism nor purpose of this change in representation mode have been adequately explained. If sequence information is actually being encoded by the presumed asymmetric synaptic changes underlying the observed place field expansion, it would be necessary to encode the forward and return routes using different populations in order preserve the asymmetry.

In a sparsely coded neuronal network with noisy elements, increased place field size would lead to an improved signal to noise ratio and hence increased accuracy of the hippocampal population code, as was observed in rats exploring a novel environment by Wilson and McNaughton (18); however, we find that the place field size increases in the familiar environments too, although perhaps to a smaller degree than in a novel one.

The asymmetric synaptic enhancement that is suggested by the observed enlargement and shift of place fields with experience could also provide the basis for the reactivation of recently experienced states of neuronal correlation and correlation sequences that occur during quiet waking and slow wave sleep immediately following the experience (8, 19, 20).

To conclude, the present results show that place fields increase in size and shift backwards relative to the rat’s direction of motion, within a few traversals of a route. If some of these cells exhibit place fields on a novel route after having just expanded their fields on the familiar one, the new fields again start out small and expand with experience. Thus, the expansion is specific either to the route or to the pattern of neuronal interactions experienced along it. The results provide indirect evidence for asymmetric Hebbian synaptic strengthening, which may underlie learning of sequences.