Abstract
Preliminary measurements of the representation in the cochlear nucleus (CN) of harmonic tones, harmonic tones with mistuned components, and double harmonic tones are reported. These data indicate that, unlike auditory nerve fibers and IC neurons, neurons in the CN may exhibit one of several qualitatively-different response patterns when stimulated with mistuned tones. Primarylike neurons synchronized their discharges to 2–3 individual stimulus components, much like auditory nerve fibers do. Chopper neurons tended to respond with the periodicity of envelopes produced by interactions between adjacent stimulus components but exhibited little or no response synchronized to individual stimulus components. A small proportion of CN neurons exhibited complex slowly-modulated discharge patterns similar to those that are commonly observed in the inferior colliculus (IC). The patterns obtained from CN neurons with different pure-tone discharge patterns were generally consistent with expectations based on previous studies with other stimuli. The measurements provided additional insight into the hierarchical processing stages that result in the highly patterned responses of IC neurons to harmonic and mistuned tones.
Keywords: cochlear nucleus, chinchilla, complex sounds, spectral segregation, auditory scene analysis
Introduction
Humans and non-human animals usually process sounds in environments in which multiple sound-generating objects are present. In that case signals often overlap in time and frequency, but the normal auditory system automatically and effortlessly segregates information arising from each of the individual sources so that the original sound-generating objects can be accurately perceived. Segregation is part of the process described by terms such as auditory scene analysis (Bregman 1990) or sound-source determination (Yost 1993; Yost and Sheft 1993). In his influential review of the anatomy and physiology of the auditory brainstem, Dexter Irvine (1986) noted that sound source determination is an essential function of the auditory system. However, at the time Irvine’s book was written, neurophysiological studies of the processing of simultaneous sounds had not been reported. Although several studies of the neural representation of simultaneous sounds have now been carried out, the neural mechanisms that underlie sound-source determination remain incompletely understood. We have studied these mechanisms by measuring neural responses to harmonic tones, harmonic tones with mistuned components, and double harmonic tones. These sounds were chosen because they may be perceived by humans as originating from one or two sound sources, depending on details of their harmonic structure (Bregman 1990; Yost and Sheft 1993; Darwin and Carlyon 1995; Carlyon 2004; Darwin 2005; Roberts 2005). These stimuli engage the same neural mechanisms for sound source determination as more complex mixtures, but are simple enough to be practical for use in neurophysiological studies.
Psychophysical studies of the perception of sounds with single mistuned components have provided extensive information about segregation based on harmonicity (Hartmann 1988; Moore et al. 1986; Lin and Hartmann 1998). Harmonics of a fundamental frequency (f0) tend to be grouped and perceived as a single sound with a pitch determined by f0 and a timbre determined by the pattern of component amplitudes; voiced speech sounds and the sounds produced by musical instruments are examples of harmonic sounds. If the spectrum includes a component that deviates from the harmonic series (a “mistuned” component), that component can be segregated and perceived as a separate sound with a different pitch and timbre. Although most studies of mistuning have used strictly harmonic sounds as their starting point, Roberts and colleagues have shown that listeners can also segregate components that deviate from other forms of harmonic structure, including inharmonic sounds with regular component spacing and sounds with “stretched” spacing (Roberts and Brunstrom 1998, 2001).
In real-world listening situations, it is likely that a competing sound will consist of harmonics of a different f0. Two vowels with identical f0 that differ in timbre can be identified with accuracy greater than chance (Assmann and Summerfield 1989), but accuracy improves dramatically if the f0s of the individual vowels differ by as little as 3% (Assmann and Summerfield 1990; Chalikia and Bregman 1989, 1993; de Cheveigné 1993, 1997, 1999). Pairs of non-speech harmonic tones can also be separated, presumably by similar processes; however, these sounds have been studied less often (Beerends and Houtsma 1989; Carlyon and Shackleton 1994; Carlyon 1996; Micheyl et al. 2006).
We have previously studied the representation of harmonic and mistuned tones in the auditory nerve and the inferior colliculus (IC) of the chinchilla (Sinex et al. 2002, 2003, 2005; Sinex 2005). We have also described the representation of pairs of harmonic tones with different fundamental frequencies in the IC (Sinex and Li in press). The discharge patterns of IC neurons are strongly affected by the presence of components that depart from a simple harmonic series and lead to the perception of a second sound source. In IC responses to mistuned tones and double harmonic tones, stimulus components at and above f0 are represented weakly or not at all in the temporal discharge pattern. Instead, the response spectrum includes prominent low-frequency components that are not present in the stimulus. These components correspond to beat frequencies produced by adjacent components in the stimulus, and they may also reflect higher-ordered interactions between beats. In addition, perceptually-double sounds may elicit greater discharge rates than single sounds.
In contrast, at the level of the auditory nerve, there is no qualitative difference between the representation of a purely-harmonic tone, and a tone with a mistuned component. Auditory nerve fibers represent individual components in the spectra of complex sounds in discharge synchrony (Sinex et al. 2003). The particular stimulus components that are represented in the response do not depend on whether components are harmonically related. For example, a fiber that synchronizes to the fourth and fifth harmonics of a harmonic tone will continue to synchronize to the same numbered components after one of them has been mistuned. The exact frequencies represented in discharge synchrony change when the frequency of a component is changed, of course. A similar result was obtained by Palmer (1990), who studied the representation of double vowels with different f0; he found that auditory nerve fibers could synchronize to any component within their frequency response areas, from either vowel or both vowels.
Together, these results demonstrate that a major transformation in the temporal representation of harmonic complex sounds occurs in the lower brainstem. Where and how this transformation occurs cannot be determined from the data reported so far. To begin to establish how the characteristic discharge patterns of IC neurons are created, preliminary measurements of the responses of neurons in the cochlear nucleus (CN) of the chinchilla to harmonic and mistuned tones have been made. These preliminary results indicate that CN neurons exhibit a range of discharge patterns, including some that resemble those of auditory nerve fibers, some that resemble the more-complex responses of IC neurons, and some that fall between these patterns.
Methods
Animal preparation
Responses were obtained from units in the CN of the chinchilla with methods identical to those described previously (Sinex et al. 2005, Sinex and Li in press). Briefly, chinchillas were deeply anesthetized by injection of a mixture of 36 mg/kg ketamine and 4 mg/kg xylazine, and supplemental injections of ketamine or ketamine/xylazine were given as required. The animal was placed in a stereotaxic instrument (David Kopf Instruments, Tujunga CA) in a double-walled sound-attenuating booth (IAC, Brooklyn NY). The posterior fossa was opened, and a portion of the cerebellum was aspirated to expose the dorsal surface of the CN. Recording electrodes were placed above the ventral CN (VCN) or dorsal CN (DCN) under visual control, then advanced from outside the sound booth with a hydraulic microdrive (David Kopf Instruments, Tujunga CA). Responses were recorded from well-isolated single units with glass micropipettes filled with 2M NaCl. All procedures were approved by the Institutional Animal Care and Use Committee at Utah State University.
Stimuli
The stimuli were single harmonic tones, mistuned tones, and double harmonic tones. A single harmonic tone was generated by summing the first 8 harmonics of 250 Hz. A mistuned tone was produced by shifting the frequency of a single component by 3–12% (Sinex et al. 2002, 2003, 2005; Sinex 2005). A double harmonic tone was generated by summing the waveforms of two single harmonic tones with different f0s (Sinex and Li in press). Typically, one f0 was 250 Hz, and the second f0 was 255, 260, 270, or 280 Hz. Tones were 650 msec long, with 5-msec linear rise-fall time, and all components had identical onset times and durations. Tones were presented once per second at levels between 10–70 dB SPL per sine-wave component. Individual components were always synthesized in sine phase. The amplitude of each individual component was adjusted during synthesis to compensate for the non-uniform transfer function of the acoustic system. After compensation, individual components had equal SPL at the eardrum.
Data collection and analysis
Stimulus generation, stimulus presentation, and data collection were controlled by computer. Waveforms were digitally synthesized, passed through digital-analog converters, programmable attenuators and antialiasing filters (all from TDT, Alachua, FL), then delivered to a closed acoustic system incorporating ER2A insert earphones and an ER7 probe-tube microphone (Etymotic Research, Elk Grove Village, IL). The acoustic system was calibrated for each experiment. Tones were always delivered monaurally to the ipsilateral ear, and were typically presented 75 times.
When a neuron was isolated, a detailed frequency-response map was obtained with an automated procedure that presented tones at multiple frequencies and levels in a random sequence (Nuding et al. 1999). Responses to tone bursts at CF were also obtained and used to classify each neuron according to the shape of its peristimulus time (PST) histogram and first-spike latency (Bourk 1976; Young et al. 1988). The preliminary results shown here were obtained from primarylike, chopper, and buildup units, all of which are common in the CN (Bourk 1976; Rhode and Greenberg 1992; Feng et al. 1994).
Responses elicited by complex tones are displayed as PST histograms, or as cycle histograms constructed from spikes occurring between 50–650 msec after tone onset. Cycle histograms were generated with a 200-msec cycle; 200 msec was the shortest interval over which every complex tone was periodic. To determine the “response spectrum”, the discrete Fourier Transform of a 4096-bin histogram constructed from all the spikes in the window from 50–650 msec after onset was calculated. The resolution of the resulting spectrum was 1.667 Hz. The response at each frequency was expressed as the Synchronization Index (SI), the magnitude of the Fourier component normalized by the average discharge rate (Johnson 1980; Young and Sachs 1979). The locations of individual peaks in the response spectrum are referred to as response frequencies or response components.
Results
The results presented here illustrate the range of responses elicited by complex tones from 30 CN neurons recorded from 5 chinchillas.
Representation of mistuned tones
The discharge pattern of one CN primarylike neuron to harmonic and mistuned tones is shown in Fig. 1. The response elicited by a harmonic tone is shown as a PST histogram in Fig. 1A. As is typical of CN primarylike neurons responding to pure tones, discharge probability was highest at stimulus onset, adapted over the first few msec, and was maintained at an approximately constant level for the remaining duration of the complex stimulus. The overall discharge rate was 134 spikes/sec, and it can be seen that the harmonic tone elicited a response with a regular temporal pattern.
Figure 1. Temporal discharge pattern of a CN primarylike neuron to a single harmonic tone. Neuron 610–19, CF=1.2 kHz, threshold at CF=10 dB SPL, primarylike PST for tones at CF.
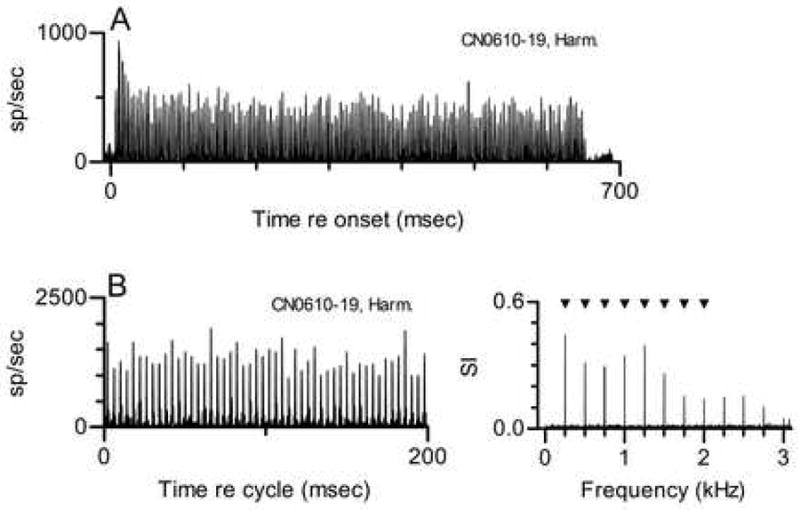
A. PST histogram. The abscissa represents time relative to the onset of the stimulus. The complex tone stimulus included the first 8 harmonics of 250 Hz, was presented at 30 dB SPL per component, and had a duration of 650 msec. The ordinate scale is in spikes/second per 1-msec bin.
B, left panel. Same data as A shown as a cycle histogram so that the temporal discharge pattern can be seen more clearly. The ordinate scale is in spikes/second per 0.147-msec bin. See text for details of histogram generation. In this and other cycle histograms, extremely high discharge rates reflect precise spike timing and the use of small binwidths.
B, right panel. Response spectrum. The ordinate scale is Synchronization Index, which is described in the text. Triangles mark the frequencies of the 8 stimulus components.
Details of this pattern can be seen more clearly in the cycle histogram and accompanying response spectrum shown in Fig. 1B. Discharge rate peaks separated by 4 msec were prominent in the cycle histogram; 4 msec was the fundamental period of the harmonic tone with f0=250 Hz. These features of the response were quantified in the response spectrum, which included components at integer multiples 250 Hz. The response with the periodicity of f0 may be a direct response to the f0 component in the stimulus, but that is not easily distinguished from a response locked to the 250-Hz beat or envelope created by interaction between responses synchronized to any pair of adjacent harmonics. After the response at 250 Hz, the largest response components occurred at 1000 and 1250 Hz; these harmonics were closest to the neuron’s CF, 1.2 kHz. Although they cannot be seen in the cycle histogram, smaller peaks with shorter separation were also present in the discharge pattern. The presence of these peaks and of short interspike intervals (not shown) confirmed that the neuron’s discharges did synchronize to the components near its CF.
Response components were also observed at higher frequencies not present in the stimulus. These are likely to be artifacts of the analysis that arise because of the rectification inherent in cochlear transduction (Young and Sachs 1979). Consistent with this possibility, some of these response components occurred at frequencies outside the neuron’s response area.
Figure 2 shows the responses of the same primarylike neuron to three tones in which Component 4 was mistuned by different amounts. The response to the tone in which Component 4 was mistuned by 3%, from 1000 to 1030 Hz, is shown in Fig. 2A. This change in the stimulus produced a corresponding change in the discharge pattern that is most easily understood by examining the response spectrum. The synchronized response followed the stimulus change, so that the largest response components occurred at 1030 and 1250 Hz. These were the same-numbered stimulus components that elicited large responses in the data shown in Fig. 1, even though one component was no longer a harmonic of f0. In addition, a new low-amplitude response component appeared at 220 Hz. The frequency of this component is consistent with its being produced by an interaction between the responses synchronized to stimulus Components 4 and 5. These responses could beat to produce an envelope at 1250 – 1030 = 220 Hz. The same interaction may have occurred in the response to the harmonic tone. As described previously, in that case the beat would have had the same frequency as an actual stimulus component, 250 Hz.
Figure 2. Responses of the same neuron shown in Fig. 1 to mistuned tones. Cycle histograms and response spectra are as described for Fig. 1B.
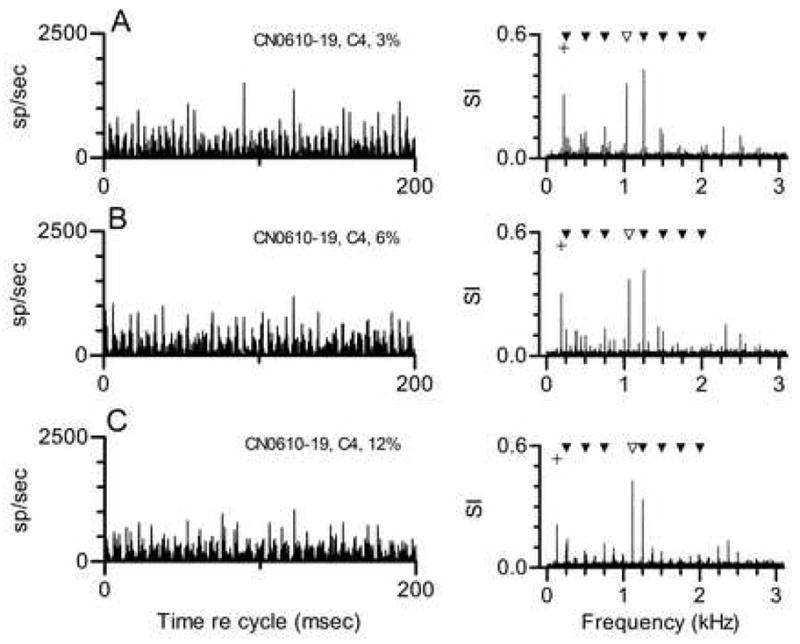
A. Responses to a tone in which Component 4 was mistuned by 3% (from 1000 to 1030 Hz). The unfilled symbol marks the frequency of the mistuned component. The + symbol marks the new envelope frequency that could be produced by interaction between Components 4 and 5.
B. Same as Fig. 2A, when Component 4 was mistuned by 6% (from 1000 to 1060 Hz).
C. Same as Fig. 2A, when Component 4 was mistuned by 12% (from 1000 to 1120 Hz).
The same general description can be applied to the data in Fig. 2B. In this example, Component 4 was mistuned by 6%, from 1000 to 1060 Hz. As in the previous example, Components 4 and 5 dominated the response, and a small component whose frequency corresponded to the difference between those two frequencies was also observed. In this case the difference frequency was 190 Hz. In the data in Fig. 2C, Component 4 was mistuned by 12% to 1120 Hz. Again, the largest response components corresponded to stimulus Components 4 and 5, and to the difference between those frequencies, 130 Hz. Overall, the primarylike neuron always produced a response synchronized to a small number of stimulus components near its CF and to a low frequency that matched the difference between those components. Mistuning changed the particular frequencies at which synchrony was observed, but produced no qualitative change in the response. Not surprisingly, the characteristics of these responses mimic those we have reported for auditory nerve fibers (Sinex et al. 2003).
The responses of a chopper neuron to the same stimuli are shown in Fig. 3. This neuron’s CF, 1.1 kHz, closely matched that of the neuron whose responses were shown in Figs. 1 and 2, although it was recorded in a different animal. In response to the harmonic tone (Fig. 3A), the largest component in the response was at 250 Hz, which was f0 and also the frequency difference between any pair of adjacent harmonics. When Component 4 was mistuned by 3%, the amplitude of the response component at 250 Hz decreased, but new response components appeared at 220 and 30 Hz. The 220-Hz component likely was produced by interactions between responses synchronized to Component 5, at 1250 Hz, and the mistuned Component 4, at 1030 Hz. The component at 30 Hz could be produced if response components at 220 and 250 Hz interact; we have previously referred to interactions of this type as second-order envelopes when describing the responses of IC neurons to mistuned tones (Sinex et al. 2002, 2005). As in Fig. 3A, this neuron’s response components at frequencies greater than 250 Hz are likely to be artifacts due to rectification; for example, a component was observed at 1000 Hz even though the mistuned tone had no component at that frequency.
Figure 3. Responses of a CN chopper neuron to harmonic and mistuned tones. Neuron 603-6, CF=1.1 kHz, threshold at CF=30 dB SPL, chopper PST for tones at CF.
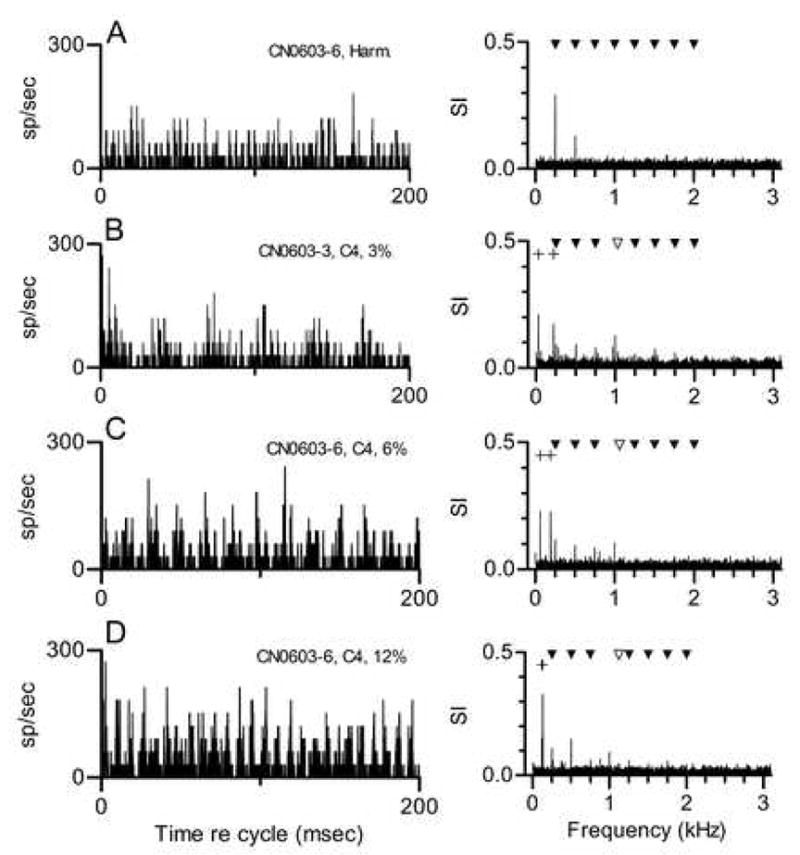
A. Responses to a single harmonic tone with f0=250 Hz, presented at 40 dB SPL per component. See Fig. 1B for additional description.
B. Responses to a harmonic tone in which Component 4 was mistuned by 3% (from 1000 to 1030 Hz). The unfilled symbol marks the frequency of the mistuned component. The + symbols mark the new envelope frequencies that could be produced by Components 3 and 4, or by Components 4 and 5. C. Same as Fig. 3B, when Component 4 was mistuned by 6% (from 1000 to 1060 Hz). D. Same as Fig. 3B, when Component 4 was mistuned by 12% (from 1000 to 1120 Hz).
The same neuron’s response to a tone in which Component 4 was mistuned by 6% is shown in Fig. 3C. In this case, the largest response components occurred at 190 and 60 Hz. The interpretation of this pattern is the same as that given for the data in Fig. 3B. The response component at 190 Hz corresponds to the difference frequency between Components 4 and 5 in the stimulus. The response at 60 Hz could be produced by second-order beats between responses synchronized to 190 and 250 Hz. Similarly, the frequency of the largest response component in Fig. 3D, 130 Hz, matches the difference between Component 5 at 1250 Hz and the mistuned Component 4 at 1120 Hz. Overall, this neuron’s synchronized responses are best described as following the envelope produced by interactions between unresolved stimulus components near its CF. It did not exhibit synchrony at the frequencies of the components themselves, as the primarylike neuron did.
The responses of a buildup neuron to the same set of complex tones are shown in Fig. 4. This neuron’s CF was much higher than the CF of the neurons in the previous example, so it is not possible to say whether the features of these discharge patterns are a consequence of CF, of the type of neuron, or both. However, this example is included here because the temporal discharge patterns strongly resembled those that have been observed in the IC (Sinex et al. 2002, 2005).
Figure 4. Responses of a CN buildup neuron to harmonic and mistuned tones. Neuron 610-4, CF=5 kHz, buildup PST for tones at CF.
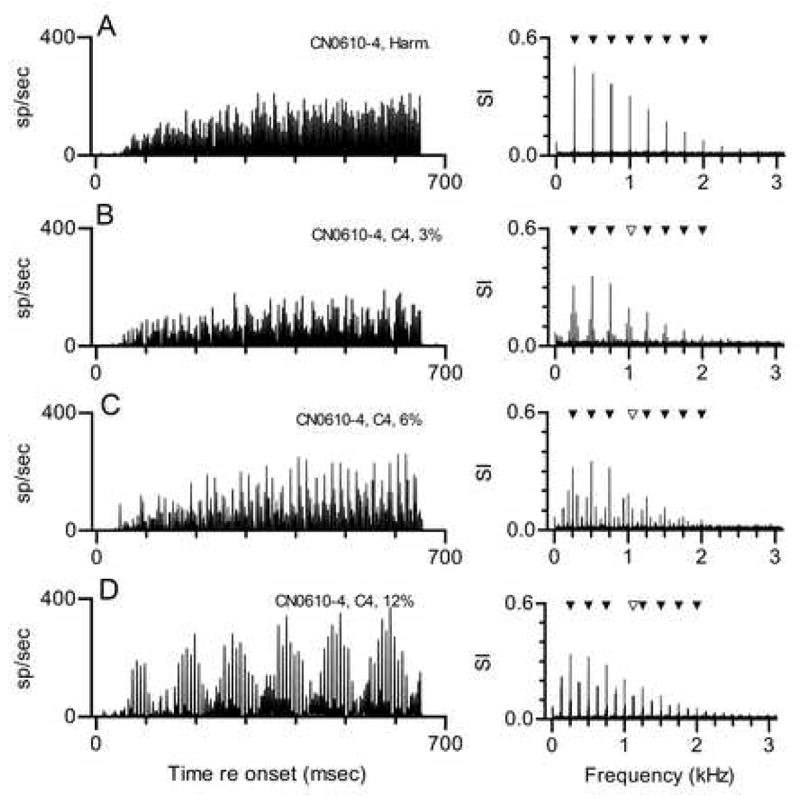
A. Response to a single harmonic tone with f0=250 Hz, presented at 50 dB SPL per component, shown as a PST histogram. See Fig. 1A for description.
B. Responses to a tone in which Component 4 was mistuned by 3% (from 1000 to 1030 Hz), shown as a PST histogram. The unfilled symbol in the response spectrum marks the frequency of the mistuned component.
C. Same as Fig. 4B, when Component 4 was mistuned by 6% (from 1000 to 1060 Hz).
C. Same as Fig. 4B, when Component 4 was mistuned by 12% (from 1000 to 1120 Hz).
In response to the harmonic tone, the neuron exhibited the same buildup pattern obtained with pure tones at CF. The response was tightly locked to the stimulus envelope, which accounts for the large component at 250 Hz in the response spectrum. Response components at 500 Hz and other integer multiples of 250 Hz were clearly artifactual in this example; interspike intervals occurred at 4 msec and at integer multiples of 4 msec, but intervals shorter than 4 msec that would indicate synchrony to frequencies greater than 250 Hz were never seen.
Responses to tones in which Component 4 was mistuned by 3, 6 and 12% are shown in Figs. 4B-D. In the response to each mistuned tone, large components at 250 Hz and its harmonics were observed. As in Fig. 4A, those components above 250 Hz were likely to be rectifier distortion products; for example, a response component occurred at 1000 Hz even though none of the mistuned stimuli included a component at that frequency. The most obvious consequence of mistuning was the appearance of sidebands above and below the large responses attributable to f0.
The sideband response components can be seen more clearly in Fig. 5, which displays the first 400 Hz of the same response spectra with higher resolution. An interaction between responses synchronized to Components 4 and 5 would produce the beat with a frequency less than 250 Hz, and an interaction between Components 3 and 4 would produce the beat with a frequency greater than 250 Hz. In each response spectrum, the frequencies of the sidebands correspond to those difference frequencies: 220 and 280 Hz in Fig. 4B, 190 and 310 Hz in Fig. 4C, and 130 and 370 Hz in Fig. 4D. One possible interpretation of these patterns is that the neuron responded in a simple way to a beat produced by those interactions. However, if that were the case, one might expect to see response components at integer multiples of those frequencies, as is seen for the response component at 250 Hz. Examination of the full response spectra in Fig. 4 shows that was not the case. Instead, the response components at higher frequencies occurred at frequencies that maintained the same spacing around each harmonic of 250 Hz. That pattern more closely resembles the spectral change that would be produced by amplitude modulation at 30, 60, or 120 Hz.
Figure 5.
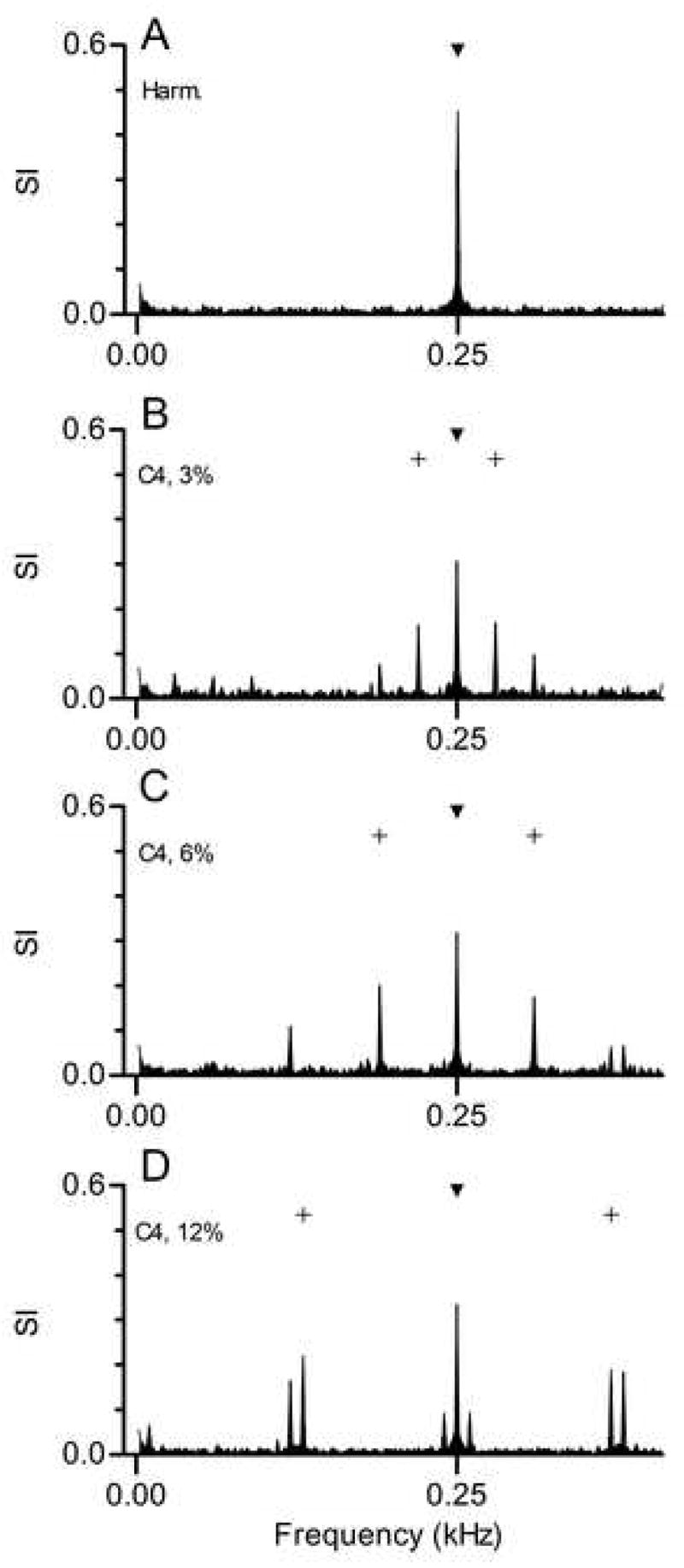
Response spectra from the previous figure shown with higher resolution. The filled symbol marks the f0 component of the stimulus, 250 Hz. In panels B-D, the + symbols mark the new envelope frequencies produced by mistuning. Envelopes at 250 Hz could occur in both harmonic and mistuned stimuli.
The discharge pattern shown in Fig. 4D is of particular interest because, although it differed from the patterns described here for other CN neurons, it strongly resembled the pattern that we have consistently observed in the IC and described in detail (Sinex et al. 2005). The resemblance can be seen in Fig. 6, which directly compares the cycle histogram of the CN buildup neuron’s response to the cycle histogram obtained with the same stimulus from an IC neuron with CF = 3 kHz. Each histogram exhibited slow modulation with a period of 100 msec, and a fine structure in which peaks were separated by approximately 8 msec. Smaller peaks interspersed between those peaks were also present, but only during a portion of the cycle. These features are common in the IC but in the preliminary data collected so far, rare in the CN.
Figure 6.
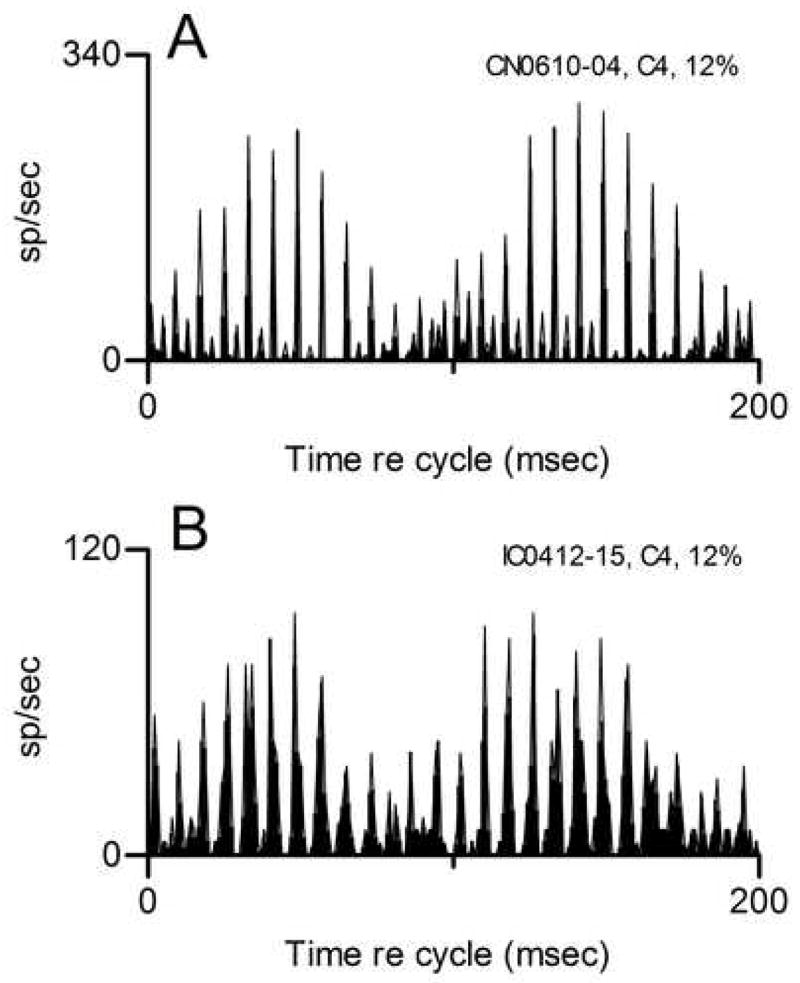
Comparison of responses elicited by the same mistuned tone from the CN buildup neuron and from a typical IC neuron. Panel A: Same data shown in Fig. 4D replotted as a cycle histogram. Panel B: Responses of IC neuron 412–15, CF=3 kHz. The stimulus was the same as in panel A, except that the level was 40 dB SPL per component. For this figure only, cycle histograms were generated with 1-msec bins.
Representation of double harmonic tones
Responses to double harmonic tones have been obtained from 8 CN neurons. Examples of responses from two neurons are shown in Fig. 7. In each case, the stimulus was a double harmonic tone with f0s of 250 and 260 Hz. The responses of a primarylike neuron (Fig. 7A) synchronized to a small number of stimulus components nearest its CF, 0.8 kHz. For this double harmonic tone, the largest response frequencies were 750 and 780 Hz, the third harmonics of each single tone. This pattern was qualitatively similar to the patterns elicited by mistuned tones shown in Figs. 1 and 2 for a different primarylike neuron, in that the response spectrum reflected the frequencies of the stimulus components that fell within the neuron’s frequency response area. The same general pattern was observed in the response to other double harmonic tones in which f0 of the second tone was varied (not shown). In each case the synchronized response included the components from each harmonic tone that fell near CF.
Figure 7. Responses of two CN neurons to double harmonic tones. In each panel, f0 for the first tone was 250 Hz, f0 for the second tone was 260 Hz, and each tone was presented at 40 dB SPL per component. Cycle histograms were generated and response spectra were calculated as in Fig. 2.
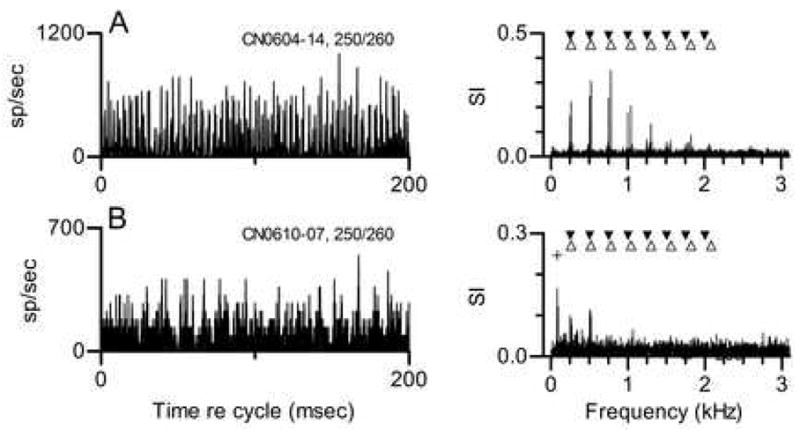
A. Neuron 604-14, CF=0.8 kHz, threshold at CF=30 dB SPL, primarylike PST for tones at CF. Filled inverted triangles mark the frequencies of the 8 harmonics of 250 Hz. Unfilled triangles mark the frequencies of the 8 harmonics of 260 Hz.
B. Same as A for chopper Neuron 610-7, CF=2.4 kHz, threshold at CF=20 dB SPL. The + symbol marks the envelope frequency that would be created by interactions between the 8th harmonics of each individual tone.
The responses of a chopper neuron with CF=2.4 kHz to the same stimulus are shown in Fig. 7B. The largest component in this neuron’s response spectrum was at 80 Hz. Smaller response components representing responses synchronized to the first two harmonics of each single tone were also seen. For this double harmonic tone, the stimulus components nearest to this neuron’s CF were at 2000 and 2080 Hz, which would beat at 80 Hz, the frequency that was prominent in the response spectrum. As in the previous example, a qualitatively similar pattern was observed in response to other double harmonic tones. The chopper neuron’s response followed changes in envelope frequencies when the f0 of the second tone was changed. This pattern also bears some similarity to the pattern elicited by mistuned tones from a different chopper shown in Fig. 3, in that the temporal discharge pattern reflected the frequency difference between two components.
Discussion
Responses of neurons in the CN of the chinchilla to harmonic complex tones, the same tones with mistuned components, and pairs of complex tones with different f0 were presented. Although these data are preliminary, they illustrate that these manipulations of the original harmonic tones have qualitatively different effects on the discharge patterns of CN neurons of different types. We had previously measured the representation of the same stimuli in the IC, and a goal of the ongoing study is to determine how the distinctive responses of IC neurons are generated. Most CN neurons respond to these tones in a way that does not resemble the representation of the same tones in the IC. However, in a few neurons, responses very much like IC responses were obtained.
The defining feature of the responses of CN primarylike neurons to complex tones was synchrony to a small number of individual stimulus components. When a component was mistuned, there was no effect of beyond the simple change in response frequency. This pattern was also observed in true primary neurons by Sinex et al. (2003). Primarylike neurons also represented double harmonic tones in a straightforward manner, exhibiting synchrony to components near CF, including components that originated with either or both of the original harmonic tones. This is also consistent with previous observations of primary and primarylike neurons. For example, Palmer (1990) examined the representation of simultaneous vowels with different f0s in the responses of auditory nerve fibers. He reported that components from each individual vowel could be identified in the population synchronized response. CF was a major determinant of the particular components that accounted for the synchronized response, as in the present results. Keilson et al. (1997) obtained responses to stimuli that were like double vowels from neurons in the CN. Consistent with the present results and those of Palmer, they reported that primarylike neurons exhibited synchrony to components near their CFs.
The responses of chopper neurons were qualitatively different from the responses of primarylike neurons, in that their temporal discharge patterns reflected the frequencies of beats produced by adjacent stimulus components, rather than the frequencies of the components themselves. For harmonic tones, choppers responded at the frequency of the fundamental period. This was true even for neurons tuned to frequencies well above f0, indicating that some or all of these responses were locked to the envelope that would be produced by any pair of adjacent unresolved components. For a stimulus with f0=250 Hz, the envelope is periodic over 4 msec. When a component is mistuned, two new envelope frequencies are produced in the frequency region of the mistuning, one with frequency lower than f0 and one with a higher frequency. These new beat frequencies were observed in the response of the chopper neuron shown in Fig. 3. Chopper neurons exhibited analogous responses to double harmonic tones, in that components at envelope frequencies were more prominent in the response spectra. When synchrony to components was observed, it was only at the lowest frequencies (Figs. 3 and 7B). This behavior is also consistent with previous reports that CN choppers do not synchronize well to the waveforms of other complex sounds, but do provide a good representation of envelopes (Blackburn and Sachs 1990; Keilson et al. 1997; Shofner 1999).
The buildup neuron whose responses to a mistuned tone were shown in Fig. 4 exhibited a discharge pattern that was unlike those shown for primarylike and chopper neurons, but bore a strong resemblance to the patterns elicited from IC neurons by the same stimulus (Sinex et al. 2002, 2005). We have previously hypothesized that this pattern might be created in the IC by converging excitatory and inhibitory inputs that originate in different frequency regions (Sinex et al. 2005; Sinex 2005). The data shown in Fig. 4 make it clear that this kind of complex modulated pattern does not originate exclusively in the IC, although the data collected so far suggest that this pattern will be much less common in the CN. Histological confirmation of this buildup neuron’s anatomical location is not available, but it was recorded in a track aimed at the DCN, and the buildup pattern is commonly observed in the DCN of anesthetized animals (Rhode and Greenberg 1992). It is well known that many neurons in the DCN have integrative properties similar to those that we believe account for the representation of these complex tones in the IC. For example, principal cells of the DCN integrate excitatory and inhibitory input across frequency (Spirou and Young 1991; Young and Davis 2002; Rhode and Smith 1986).
Given the similarity between the representation of the mistuned tone by the buildup neuron and the representation of the same tone in the IC (Fig. 6), and the fact that the DCN provides a major input to the IC, it is reasonable to ask whether the stereotypical discharge pattern observed in IC is simply relayed from a more peripheral level. Although the discharge pattern of the presumed DCN neuron was similar to those recorded in the IC, it was not identical to the stereotypical response seen in the IC. The most conspicuous difference is that many IC neurons recorded in the same species under identical conditions of anesthesia and data collection equipment and software begin to exhibit complex temporal discharge patterns after a latency that is shorter than the latency of this buildup neuron. Buildup neurons by definition respond only after a delay, so they cannot account for the initial part of the responses observed in the IC. For now, the working hypothesis continues to be that the discharge patterns observed in the IC are largely created within the IC by across-frequency interactions between neurons carrying envelope information. As the present data show, some neurons in more peripheral structures may generate similar patterns, if they receive converging inputs of the type hypothesized to occur more centrally. The extent to which DCN neurons influence the representation in the IC, and to which IC responses to these complex sounds are influenced by other brainstem nuclei, is a matter for further study.
Acknowledgments
Supported by DC00341 from NIDCD. Some of these results were presented at “The Auditory Brain - A tribute to Dexter Irvine”, a Satellite Meeting to IBRO, Lorne, Victoria 3232, Australia, July 2007.
Abbreviations
- CF
characteristic frequency
- CN
cochlear nucleus
- DCN
dorsal cochlear nucleus
- f0
fundamental frequency
- IC
inferior colliculus
- PST
peristimulus time
- SI
Synchronization Index
- VCN
ventral cochlear nucleus
Footnotes
Publisher's Disclaimer: This is a PDF file of an unedited manuscript that has been accepted for publication. As a service to our customers we are providing this early version of the manuscript. The manuscript will undergo copyediting, typesetting, and review of the resulting proof before it is published in its final citable form. Please note that during the production process errors may be discovered which could affect the content, and all legal disclaimers that apply to the journal pertain.
References
- Assmann PF, Summerfield Q. Modeling the perception of concurrent vowels: vowels with different fundamental frequencies. J Acoust Soc Am. 1990;88:680–97. doi: 10.1121/1.399772. [DOI] [PubMed] [Google Scholar]
- Assmann PF, Summerfield Q. Modeling the perception of concurrent vowels: vowels with the same fundamental frequency. J Acoust Soc Am. 1989;85:327–38. doi: 10.1121/1.397684. [DOI] [PubMed] [Google Scholar]
- Beerends JG, Houtsma AJ. Pitch identification of simultaneous diotic and dichotic two-tone complexes. J Acoust Soc Am. 1989;85:813–9. doi: 10.1121/1.397974. [DOI] [PubMed] [Google Scholar]
- Blackburn CC, Sachs MB. The representations of the steady-state vowel sound /eh/ in the discharge patterns of cat anteroventral cochlear nucleus neurons. J Neurophysiol. 1990;63:1191–212. doi: 10.1152/jn.1990.63.5.1191. [DOI] [PubMed] [Google Scholar]
- Bourk TR. PhD Thesis. Cambridge, MA: MIT; 1976. Electrical responses of neural units in the anteroventral cochlear nucleus of the cat. [Google Scholar]
- Bregman AS. Auditory Scene Analysis. Cambridge, MA: MIT Press; 1990. [Google Scholar]
- Carlyon RP. Encoding the fundamental frequency of a complex tone in the presence of a spectrally overlapping masker. J Acoust Soc Am. 1996;99:517–24. doi: 10.1121/1.414510. [DOI] [PubMed] [Google Scholar]
- Carlyon RP. How the brain separates sounds. Trends Cogn Sci. 2004;8:465–71. doi: 10.1016/j.tics.2004.08.008. [DOI] [PubMed] [Google Scholar]
- Carlyon RP, Shackleton TM. Comparing the fundamental frequencies of resolved and unresolved harmonics: Evidence for two pitch mechanisms? J Acoust Soc Am. 1994;95:3541–3554. doi: 10.1121/1.409970. [DOI] [PubMed] [Google Scholar]
- Chalikia MH, Bregman AS. The perceptual segregation of simultaneous auditory signals: pulse train segregation and vowel segregation. Percept Psychophys. 1989;46:487–96. doi: 10.3758/bf03210865. [DOI] [PubMed] [Google Scholar]
- Chalikia MH, Bregman AS. The perceptual segregation of simultaneous vowels with harmonic, shifted, or random components. Percept Psychophys. 1993;53:125–33. doi: 10.3758/bf03211722. [DOI] [PubMed] [Google Scholar]
- Darwin C. Pitch and auditory grouping. In: Plack CJ, Oxenham AJ, Fay RR, Popper AN, editors. Pitch: Neural coding and perception. New York: Springer; 2005. pp. 278–305. [Google Scholar]
- Darwin C, Carlyon R. Auditory grouping. In: Moore BC, editor. Hearing. San Diego: Academic; 1995. pp. 387–424. [Google Scholar]
- de Cheveigné A. Concurrent vowel identification: III. A neural model of harmonic interference cancellation. J Acoust Soc Am. 1997;101:2857–2865. [Google Scholar]
- de Cheveigné A. Separation of concurrent harmonic sounds: Fundamental frequency estimation and a time-domain cancellation model of auditory processing. J Acoust Soc Am. 1993;93:3271–3290. [Google Scholar]
- de Cheveigné A. Vowel-specific effects in concurrent vowel identification. J Acoust Soc Am. 1999;106:327–40. doi: 10.1121/1.427059. [DOI] [PubMed] [Google Scholar]
- Feng JJ, Kuwada S, Ostapoff EM, Batra R, Morest DK. A physiological and structural study of neuron types in the cochlear nucleus. I. Intracellular responses to acoustic stimulation and current injection. J Comp Neurol. 1994;346:1–18. doi: 10.1002/cne.903460102. [DOI] [PubMed] [Google Scholar]
- Hartmann WM. Pitch perception and the segregation and integration of auditory entities. In: Edelman G, Gall W, Cowan W, editors. Auditory Function: Neurobiological Bases of Hearing. New York: John Wiley & Sons; 1988. pp. 623–645. [Google Scholar]
- Irvine DRF. The auditory brainstem: A review of the structure and function of auditory brainstem processing mechanisms. Berlin: Springer-Verlag; 1986. [Google Scholar]
- Johnson DH. The relationship between spike rate and synchrony in responses of auditory-nerve fibers to single tones. J Acoust Soc Am. 1980;68:1115–22. doi: 10.1121/1.384982. [DOI] [PubMed] [Google Scholar]
- Keilson SE, Richards VM, Wyman BT, Young ED. The representation of concurrent vowels in the cat anesthetized ventral cochlear nucleus: evidence for a periodicity-tagged spectral representation. J Acoust Soc Am. 1997;102:1056–71. doi: 10.1121/1.419859. [DOI] [PubMed] [Google Scholar]
- Lin JY, Hartmann WM. The pitch of a mistuned harmonic: evidence for a template model. J Acoust Soc Am. 1998;103:2608–17. doi: 10.1121/1.422781. [DOI] [PubMed] [Google Scholar]
- Micheyl C, Bernstein JG, Oxenham AJ. Detection and F0 discrimination of harmonic complex tones in the presence of competing tones or noise. J Acoust Soc Am. 2006;120:1493–505. doi: 10.1121/1.2221396. [DOI] [PubMed] [Google Scholar]
- Moore BC, Glasberg BR, Peters RW. Thresholds for hearing mistuned partials as separate tones in harmonic complexes. J Acoust Soc Am. 1986;80:479–83. doi: 10.1121/1.394043. [DOI] [PubMed] [Google Scholar]
- Nuding S, Chen G-D, Sinex D. Monaural response properties of single neurons in the chinchilla inferior colliculus. Hear Res. 1999;131:89–106. doi: 10.1016/s0378-5955(99)00023-4. [DOI] [PubMed] [Google Scholar]
- Palmer AR. The representation of the spectra and fundamental frequencies of steady-state single- and double-vowel sounds in the temporal discharge patterns of guinea pig cochlear-nerve fibers. J Acoust Soc Am. 1990;88:1412–26. doi: 10.1121/1.400329. [DOI] [PubMed] [Google Scholar]
- Rhode WS, Smith PH. Physiological studies on neurons in the dorsal cochlear nucleus of cat. J Neurophysiol. 1986;56:287–307. doi: 10.1152/jn.1986.56.2.287. [DOI] [PubMed] [Google Scholar]
- Rhode WS, Greenberg S. Physiology of the cochlear nuclei. In: Popper AN, Fay R, editors. The Mammalian Auditory Pathway: Neurophysiology. New York: Springer-Verlag; 1992. pp. 94–152. [Google Scholar]
- Roberts B. Spectral pattern, grouping, and the pitches of complex tones and their components. Acta Acustica. 2005;91:945–957. [Google Scholar]
- Roberts B, Brunstrom JM. Perceptual fusion and fragmentation of complex tones made inharmonic by applying different degrees of frequency shift and spectral stretch. J Acoust Soc Am. 2001;110:2479–90. doi: 10.1121/1.1410965. [DOI] [PubMed] [Google Scholar]
- Roberts B, Brunstrom JM. Perceptual segregation and pitch shifts of mistuned components in harmonic complexes and in regular inharmonic complexes. J Acoust Soc Am. 1998;104:2326–38. doi: 10.1121/1.423771. [DOI] [PubMed] [Google Scholar]
- Shofner WP. Responses of cochlear nucleus units in the chinchilla to iterated rippled noises: analysis of neural autocorrelograms. J Neurophysiol. 1999;81:2662–74. doi: 10.1152/jn.1999.81.6.2662. [DOI] [PubMed] [Google Scholar]
- Sinex DG. Spectral processing and sound source determination. In: Malmierca M, Irvine D, editors. Auditory spectral processing. San Diego: Elsevier; 2005. pp. 371–398. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Sinex DG, Li H. Responses of inferior colliculus neurons to double harmonic tones. J Neurophysiol. doi: 10.1152/jn.00516.2007. in press. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Sinex DG, Guzik H, Li H, Henderson Sabes J. Responses of auditory nerve fibers to harmonic and mistuned complex tones. Hear Res. 2003;182:130–9. doi: 10.1016/s0378-5955(03)00189-8. [DOI] [PubMed] [Google Scholar]
- Sinex DG, Li H, Velenovsky DS. Prevalence of stereotypical responses to mistuned complex tones in the inferior colliculus. J Neurophysiol. 2005;94:3523–37. doi: 10.1152/jn.01194.2004. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Sinex DG, Sabes JH, Li H. Responses of inferior colliculus neurons to harmonic and mistuned complex tones. Hear Res. 2002;168:150–62. doi: 10.1016/s0378-5955(02)00366-0. [DOI] [PubMed] [Google Scholar]
- Spirou GA, Young ED. Organization of dorsal cochlear nucleus type IV unit response maps and their relationship to activation by bandlimited noise. J Neurophysiol. 1991;66:1750–68. doi: 10.1152/jn.1991.66.5.1750. [DOI] [PubMed] [Google Scholar]
- Yost WA. Overview: Psychoacoustics. In: Yost W, Popper A, Fay R, editors. Human Psychophysics. New York: Springer-Verlag; 1993. pp. 1–12. [Google Scholar]
- Yost WA, Sheft S. Auditory Perception. In: Yost WA, Popper AN, Fay RR, editors. Human Psychophysics. New York: Springer-Verlag; 1993. pp. 193–236. [Google Scholar]
- Young ED, Sachs MB. Representation of steady-state vowels in the temporal aspects of the discharge patterns of populations of auditory-nerve fibers. J Acoust Soc Am. 1979;66:1381–1403. doi: 10.1121/1.383532. [DOI] [PubMed] [Google Scholar]
- Young ED, Davis KA. Circuitry and function of the dorsal cochlear nucleus. In: Oertel D, Fay RR, Popper AN, editors. Integrative functions in the mammalian auditory pathway. New York: Springer; 2002. pp. 160–206. [Google Scholar]
- Young ED, Robert J-M, Shofner WP. Regularity and latency of units in ventral cochlear nucleus: implications for unit classification and generation of response properties. J Neurophysiol. 1988;60:1–29. doi: 10.1152/jn.1988.60.1.1. [DOI] [PubMed] [Google Scholar]


