The feeding dynamics of broiler chickens

Abstract

Contrary to a commonly held belief that broiler chickens need more space, there is increasing evidence that these birds are attracted to other birds. Indeed, commercially farmed birds exhibit a range of socially facilitated behaviours, such as increased feeding and preening in response to the presence of other birds. Social facilitation can generate feedback loops, whereby the adoption of a particular behaviour can spread rapidly and suddenly through the population. Here, by measuring the rate at which broiler chickens join and leave a feeding trough as a function of the number of birds already there, we quantify social facilitation. We use these measurements to parameterize a simulation model of chicken feeding behaviour. This model predicts, and further observations of broiler chickens confirm, that social facilitation leads to excitatory and synchronized patterns of group feeding. Such models could prove a powerful tool in understanding how feeding patterns depend on broiler house design.

1. Introduction

The modern commercial broiler (meat-type) chicken experiences highly social living conditions. These birds are typically kept at densities between 22 and 42 kg m⁻² (between 8.8 and 17 birds per m²) in the EU (European Commission 2000), but may be as high as 46 kg m⁻² (Dawkins et al. 2004). The EU is working towards the adoption of standards for broiler housing that will eliminate the highest densities, and provide an upper limit based on the performance of the flock and the level of environmental control provided, an approach supported by the work of Dawkins et al. (2004). In considering the welfare of these birds, what an animal wants can be of equal importance to what it needs (Dawkins 2004). Thus, in designing houses that maximize welfare, we need to understand more about the response of birds to their environment and, in particular, their social interactions with other birds.

It is a widely held belief that crowding animals together is a major cause of poor welfare (e.g. Appleby 2004). However, recent work has established that commercial broiler chickens are distributed non-randomly within the broiler house, and rather than attempting to get away from each other, these birds space themselves closer together than predicted by a random model (Febrer et al. in press). Indeed, fowl are naturally social birds. McBride et al. (1969) noted that if a single bird (Gallus gallus) located grain spread near a hide, then the other birds would join it very quickly. Groups of hens often feed simultaneously (Hughes 1971; Appleby 1991), as many other species of domestic animals do, including pigs (Nielsen et al. 1996) and sheep (Rook & Penning 1991). Webster & Hurnik (1994) suggest that behavioural synchronization could reach super-normal levels in commercial systems, as a consequence of both low environmental complexity reducing behavioural diversity, and the close proximity of other animals, serving to magnify the prominence of social stimuli.

Synchronized feeding of domestic fowl could arise from social facilitation. Social facilitation is an initiation, or increase in behaviour frequency in response to others engaged in the same behaviour (Clayton 1978). For instance, dustbathing in laying hens is thought to be motivated, at least in part, by external factors, such as the visual stimulus of others dustbathing (Lundberg & Keeling 2003). Similarly, Barber (2001) found that laying hens were more highly motivated to feed (measured by the time a bird took to pass through a narrow gap to reach food) when in the presence of feeding companions. Despite the numerous studies on social feeding behaviour in humans (e.g. De Castro 1990), birds (e.g. Barber 2001) and other animals (e.g. Sweeting et al. 1985; Adessi & Visalberghi 2001), social facilitation of feeding is yet to be shown in broiler chickens.

In order to better understand the feeding dynamics of commercially farmed animals, a more precise quantification of how these animals react to the behaviour of conspecifics is needed. Such detailed information about individual interactions can be used to build mathematical models of group behaviour (Camazine et al. 2001; Couzin & Krause 2003; Sumpter 2006). For example, in insect societies, where interactions of society members can produce complex cooperative patterns, experimental studies combined with mathematical models have helped us to understand how group behaviour results from individual interactions (Bonabeau et al. 1997; Sumpter & Beekman 2003; Sumpter & Pratt 2003). Similar approaches have been applied to the movement of fish schools (e.g. Reynolds 1987; Couzin et al. 2002), cockroach aggregations (e.g. Amé et al. 2004), spiderling aggregations (Jeanson et al. 2004), synchronized firefly flashing (e.g. Buck & Buck 1976) and even human crowds (e.g. Helbing et al. 2000; Farkas et al. 2003). These studies have demonstrated that aggregations, though manifest at the population level, are mediated at the level of the individuals as a result of feedback loops (Flierl et al. 1999; Detrain & Deneubourg 2002). This approach could allow us to develop predictive models of the dynamics of animals displaying socially facilitated behaviour, ultimately providing a better understanding of how their living environment affects their interactions.

In this paper, we provide a quantitative analysis of social facilitation of commercially farmed broiler chickens. We first measure the probability of leaving, joining and moving along the feeder by the birds. We then use these measurements to parameterize a simulation model. We test the model predictions against separate measurements of group level dynamics, showing that social facilitation does lead to excitatory, synchronized bursts of feeding.

2. Material and methods

2.1 Data collection

We first collected data to measure how the rate at which chickens joined, left and moved along a feeder depended on the local density of feeding birds. To make these measurements, the distribution of numbers of birds along linear feeding troughs was recorded using videos from six commercial broiler houses that had been manipulated to contain broiler chickens stocked to three different stocking densities (30, 42 and 46 kg m⁻²). The simulation was tested against data taken from a further five houses (with stocking densities of 30, 34, 42 and 46 kg m⁻²).

Houses were chosen for their feeder type. Only the houses containing chain-feeding apparatus (linear troughs) were used because these houses had far less occlusion on the video tapes than those with circular pan feeders. Chain feeders are typically automated and span the length of the broiler house in a series of parallel lines. Commercial broiler houses can house up to 70 000 birds. The areas visible in the video recordings used in this study typically contained up to 200 birds. Food was provided before and throughout the entire experimental period in accordance with normal husbandry practices on the farms. The homogeneous nature of the broiler house and the continuous and constant supply of food—equal at all points of the feeder—ensured that there were no consistent environmental biases to any particular part of the feeder.

Video cameras were set up at an angle of 60–80° to the horizontal and 1.55 m above the ground to film the birds (see Dawkins et al. (2004) for further details on video setup). Each feeding trough image was divided into ‘sections’ using a clear acetate grid placed over a 19 in. television screen. Each section (x, where x=1, …, n, and 17≥n≤20) was two feeding bird-widths in length, scaled by eye to counter the effects of camera distortion (i.e. section width was approx. 0.6 m and its length was 0.2 m). The size of the section meant that an absolute maximum of five birds could feed at any one section of the feeder: two birds on either side of the feeder and one bird on top of the feeder. However, in practice, there were few observations where more than three birds were feeding at any one time at a particular section, since for two birds to occupy the same side of the feeder would require them to stand side by side, each occupying precisely half of the feeder section. Every 5 s for 10 min per house, the number of birds feeding and their body position relative to the trough (i.e. left side, right side or sitting on top of the trough) was recorded at each section, x, along the feeder.

2.2 Calculating the moving average

We denote the total number of chickens (the sum of chickens on both sides of the trough) at section x, measured along the feeder at time t as C(x,t), where 5t is the number of seconds since observations began and x is a section along the feeder. In order that the probabilities of leaving and joining a particular section at the feeder account for an average of recent activity at the feeder, rather than simply the instantaneous number of chickens at the feeder, we calculated a moving average of C(x,t). We defined

$$A(x,t)=\frac{{\sum }_{i=x-s/2}^{x+s/2}{\sum }_{j=t-b}^{t}C(i,j)}{(s+1)(b+1)},$$ (2.1)

where s and b are the spatial and the temporal ranges, respectively. These ranges were determined by first finding the spatial and the temporal autocorrelation function of C(x,t) for all the possible values for s and b, respectively. For example, the temporal autocorrelation function for a temporal range b is defined as

$$\frac{\text{cov}(C(x,t),C(x,t-b))}{\sqrt{\text{var}(C(x,t))\text{var}(C(x,t-b))}}.$$

We chose the temporal range to be the smallest value of b for which this function is equal or less than 0. Using this technique, we obtained s=2 and b=5, corresponding to a spatial range of 0.6 m and temporal range of 30 s.

2.3 Probability of joining, leaving and moving left and right along the trough

To calculate the change in number of birds at the feeder through time, we first calculated the matrix defined by I(x,t)=C(x,t)−C(x,t−1). We then calculated the number of birds moving left along the feeder by setting

$$L(x,t)=\{\begin{array}{c}\text{min}(I(x,t),-I(x+1,t))\\ \text{if}I(x,t)>0\text{and}I(x+1,t)<0\\ 0\text{otherwise}\end{array}.$$

Likewise, the number of birds moving right along the feeder was set to be

$$R(x,t)=\{\begin{array}{c}\text{min}(I(x,t),-I(x-1,t))\\ \text{if}I(x,t)>0\text{and}I(x-1,t)<0\\ 0\text{otherwise}\end{array}.$$

Here, a bird described as moving to the left is moving to the left of the screen (i.e. x decreases, where 1≤x≤20), which does not necessarily correspond to the bird's own left side. For birds moving to the right, they are moving to the right of the screen (i.e. x increases). Hence, birds feeding on opposite sides of the same feeding trough may move in opposite directions (one moving to its own left and the other to its own right side), but this results in both birds moving in the same direction relative to the feeder and the observer. The number of birds leaving and joining from the resting area is then

$$B(x,t)=I(x,t)+L(x,t)-L(x-1,t)+R(x,t)-R(x+1,t).$$

Here, a positive entry denotes a bird joining and a negative entry denotes a bird leaving the feeding trough.

In order to test the accuracy of the above approach, which does not directly measure the arrivals at and departures from the feeder, we measured the arrivals of individual birds for two out of our six datasets by eye. Comparing these measurements with B(x, t), we found that our method produced approximately 0.056 false positive arrivals per 1 real arrival and failed to detect 0.112 arrivals out of every arrival: an accuracy of approximately 90%.

The frequency of joining, leaving and moving left and right was then calculated as a function of the moving average, A(x, t). We calculated these frequencies for values of A(x, t), grouped together in segments [0,1/3), [1/3,2/3), through [8/3, 3). We excluded the values of A(x, t) for which there were less than 50 observations in a particular segment (i.e. less than 0.75% of the 6454 observations). This cut-off meant that only observations where an average of three or fewer chickens at any section on the feeder was used. This corresponded with our initial observation that usually only three birds (in total from both sides of the trough) feed in a section of 0.6×0.2 m.

We then fitted the Hill function numerically to these frequencies:

$$P_J(i)=s_J+(m_J-s_J)\frac{a^{k_J}}{T_J^{k_J}+a^{k_J}},$$

where $P_J(i)$ is the probability of arriving at the trough, a is the moving average number of chickens local to a section (a=1/6,3/6,5/6,7/6,9/6,11/6,13/6,15/6,17/6) and s_J, m_J, T_J and k_J are constants. Similar constants (s_D, m_D, T_D and k_D) were calculated for fitting the frequency of leaving events and (s_move, m_move, T_move and k_move) as the frequency of moving left or right (table 1). All the calculations were done in Matlab v. 6.1 release 12.1 (The Mathworks, Inc.).

Table 1.

Parameter values for calculation of equation (2.2)

parameter	value	represents
sJ	0.032	probability of spontaneous arrival in absence of other chickens
mJ	0.176	maximum probability of arrival at low feeding activity levels
TJ	0.640	threshold response level for arrival
kJ	1.76	steepness of response: must be greater than 1
sD	0.406	spontaneous departure in absence of other chickens
mD	0.102	minimum probability of leaving
TD	0.230	threshold response level for departure
kD	1.78	steepness of response: must be greater than 1
smove	0.216	spontaneous movement to left or right in absence of other chickens
mmove	0.066	minimum probability of moving left or right
Tmove	0.709	threshold response level for moving left or right
kmove	2.09	steepness of response: must be greater than 1

We tested the null hypothesis that the number of birds feeding had no effect on the number of birds joining, leaving or changing positions at the feeder. We performed regression analyses on untransformed probabilities of joining, leaving and moving to test whether slope=0. We used a two-sample t-test to test whether, on average (across all local densities of feeding birds), broilers that change position at the feeder go to the left or to the right. All statistical analyses were carried out using Minitab for Windows release 12.23 (Minitab Ltd, Pennsylvania, USA).

2.4 Simulation model

A simulation model was created to enable a thorough exploration of feeding behaviour dynamics in broiler chickens. The model describes how the number of chickens at a particular section changes through time. In the simulation, the probability of a chicken entering a section is set equal to the probability of joining given the number of chickens currently at the feeder measured using the method described above. Similarly, the probability of leaving and moving left and right in the simulation was also determined by the functions measured from the data. This gives rise to a cellular-automata-like model where the probability of individuals arriving and leaving sections of the feeder depends on the local density of feeding chickens.

Formally, let C(x, t) be the number of chickens at the feeder at time t and position x and let A(x, t) be the moving average as in equation (2.1). At the start of the simulation, we have each of the C(x, 1 : 6) binomially distributed with p=0.2 and N=5, so that chickens are initially distributed at random. We then update on each time-step:

$$C(x,t+1)=C(x,t)+J(x,t+1)-D(x,t+1)+L(x+1,t+1)-L(x,t)+R(x-1,t+1)-R(x,t)$$

where J, D, L and R are the events that there is; an arrival, departure, left and right move, respectively. If after updating C(x, t+1)>5 or C(x, t+1)<0, we set it within these limits, which reflect the maximum and the minimum number of chickens that can feed at one section. The probability of arrival and departure events are calculated from the measured frequencies. For example, the probability that J(x, t+1)=1 is

$$s_J+(m_J-s_J)\frac{A{(x,t)}^{k_J}}{T_J^{k_J}+A{(x,t)}^{k_J}},$$ (2.2)

where s_J, m_J, T_J and k_J are the constants measured above. The simulations had periodic boundary conditions, so chickens leaving the left-hand side of the feeder would reappear on the right-hand side. This had the effect of simulating a segment of a long continuous feeder. Simulations ran for 20 000 time-steps and equilibrium distributions for the last 10 000 time-steps were taken.

2.5 Comparing clustering seen in model and data

Data for comparing the model predictions with the data were measured in the same way as described in §2.1, though less frequently. The data were recorded once every 30 s for 10 min. The mean number of chickens per feeding position for the five datasets was 0.436, 0.333 and 0.322, 1.012 and 0.681. As well as qualitatively comparing the model predictions with the data, we tested whether the distribution of the chickens differed from that of a Poisson distribution. If broilers found crowds of other feeding birds aversive, we expected that birds would cluster less often than predicted by a Poisson distribution, while if attracted to crowds, the birds would tend to form local clusters along the feeding trough. The Poisson distribution was chosen as the reference distribution for this simulation as the number of birds approaching a trough to feed may be considered as an event in both time and space, and the expected number of such events in a given time period or area can thus be calculated.

3. Results

3.1 Probability of joining, leaving and moving left and right

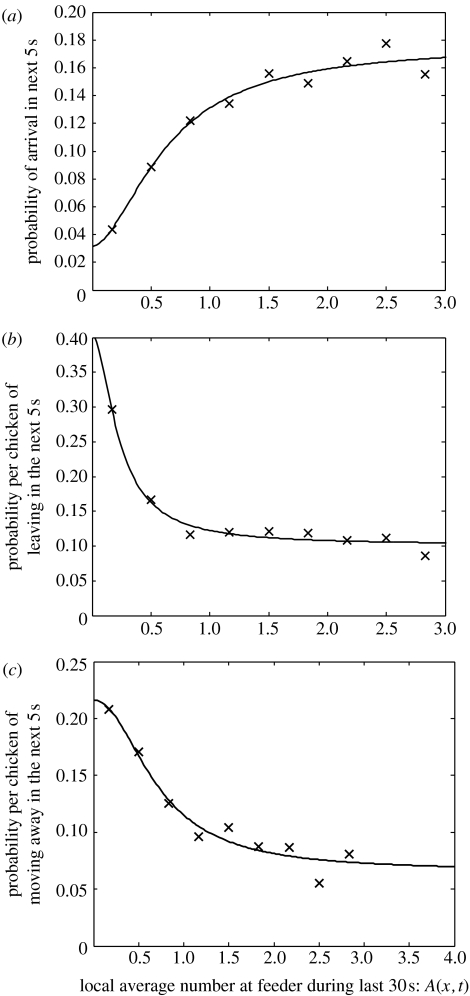
The probability of a chicken leaving a section along the feeder was found to be a decreasing function of the average number of birds feeding at that section (figure 1a). Regression analysis showed that the probability of leaving the feeder was effected by the number of birds feeding (H₀: slope=0; F_1,7=7.61, p<0.05). The probability of a chicken joining at a section in space along the feeder was an increasing function of the number at the feeder (figure 1b; F_1,7=22.38, p<0.01). The probability of a chicken moving along the feeder was a decreasing function of the number of birds feeding nearby (figure 1c; F_1,7=27.89, p<0.01). Birds were equally likely to move to the left or to the right (mean left=0.0642, s.e.=0.0143; mean right=0.0491, s.e.=0.0051; T=0.99, d.f.=9, p>0.05). Rather than being a simple attraction of hungry birds to areas at the trough with lots of feeding birds, the observation that broilers both arrive more frequently and stay for longer periods (though not necessarily consuming more) when in the presence of conspecifics is strong support for social facilitation of feeding.

Figure 1.

Measured frequencies of (a) arrival, (b) leaving and (c) moving along the feeder as a function of a moving average of the local density at a section on the feeder. Fitted functions are those in equation (2.2).

All of the above results were found to be robust to changes in the range of spatial, s, and temporal, b, averaging. Specifically, provided s≥2 and b>3, then similar relationships are observed between the number of birds at a section of the feeder and the joining, leaving and moving rates as those shown in figure 1. The lack of robustness of the results for s<2 indicates that it is the density of chickens over at least a 0.6 m length of the feeder, rather than simply the 0.2 m section occupied by one or two chickens, which induces social facilitation. When s>6 or b>30, the relationship between number of birds at the feeder and joining, leaving and moving rates is random. Once the averaging range becomes too large, then the averaging fails to capture the local interactions that determine chicken behaviour.

3.2 Simulation model

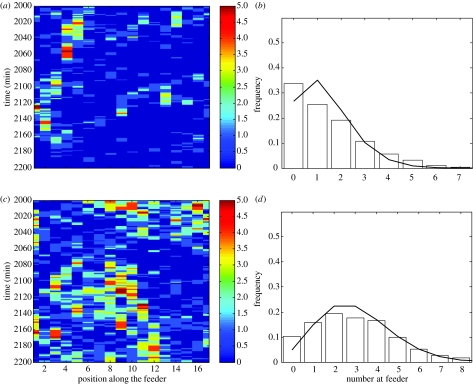
The model was parameterized by the measurements of joining, leaving and moving probabilities (table 1), though the probability of joining s_J and m_J were set to different levels to mimic the effect of high- and low-feeding activity levels (i.e. high and low probability of approaching the feeder in the absence of other feeding birds). For low-joining probabilities, the distribution of chickens at the feeder was clustered (figure 2a). In particular, the distribution of the number of feeding birds differed from a Poisson distribution (figure 2b), with higher than expected occurrences of zero and four or more chickens. For higher joining probabilities, the distribution was more similar to, though not exactly the same as, that of a Poisson distribution, predicting that at high densities the number at the feeder is less clustered. The less clustered nature of the distribution in this last case is due to the feeder reaching near carrying capacity. Local spatial and temporal clustering cannot occur because the feeder is near to maximum capacity.

Figure 2.

Results from simulation model. Example of simulated number of birds feeding at different sections along the feeder through 200 simulated minutes for (a) an average density of 0.44 and (c) an average density of 1.02 chickens per feeding position. These two densities were obtained by setting the spontaneous arrival probability, s_J, to 0.037 and 0.050, respectively, and the maximum arrival probability, m_J, to 0.151 and 0.204, respectively. All the other parameters are as measured and shown in table 1. The distribution of number of chickens feeding per three adjacent feeding sections over 10 000 simulated minutes are given in (b) and (d), respectively. Fitted lines show the distribution of number of chickens assuming a Poisson distribution.

3.3 Comparing clustering seen in model and data

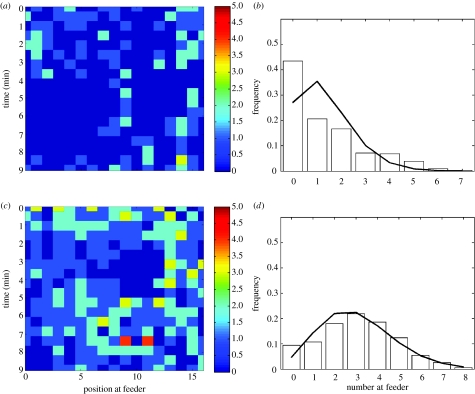
The model makes the qualitative prediction that when the local density of chickens at the feeder is low, we expect a clustered distribution of feeding birds, but when local density is high, we expect the distribution to be closer to random (figure 2). Of the five sets of observations used to validate the model, the three lower local density observations exhibited clustered distributions of chickens at the feeders (e.g. figure 3a,b), while the two high local density trials exhibited a lower degree of clustered feeding (e.g. figure 3c,d). There is a qualitative similarity, in terms of the degree of clustering, between the distributions predicted by the model and those seen in the data (i.e. compare figures 2 and 3).

Figure 3.

Results from observations of bird feeding patterns. Example of activity at different sections along the feeder through 10 min for (a) an average density of 0.44 and (c) an average density of 1.02 chickens per feeding position. Distribution of number of chickens feeding per three adjacent feeding sections over the 10 min is given in (b) and (d), respectively. Fitted lines show the distribution of number of chickens given a Poisson distribution.

The lower density observations exhibited a greater degree of clustering than the higher density observations. The three lowest local density trials differed significantly from a Poisson distribution (Χ₇²=120.2, p<0.001; Χ₆²=90.3, p<0.001; Χ₇²=148.0, p<0.001), while the highest local density trial differed significantly, but to a lesser degree than at low densities (Χ₈²=21.7, p=0.006) and the second highest density trial did not differ from a Poisson distribution (Χ₇²=7.6, p=0.37). The average local density of birds at the feeding trough did not covary with the stocking density within the broiler houses (F_1,4=0.08, p=0.794).

4. Discussion

We have shown that broiler house chickens exhibit social facilitation in feeding and measured the functional form of the response of chickens to conspecifics in this context. Our results show that single birds staying for short intervals at a particular place along the feeder do not induce other birds to arrive. Above a threshold density of one bird feeding for a 15 s period, the probability of an arrival by another bird more than doubles (figure 1a). This probability saturates once two or more birds are at the feeder for 30 s, with the probability of arrival now four times that of an unoccupied section along the feeder. Likewise, the response threshold for chickens leaving the feeding trough, and for moving left or right along the trough, rapidly decreases with the number of chickens at the feeder.

The behaviour and choices that animals make, either in an experimental set-up or in their home environment, can be highly informative about what they want (i.e. are highly motivated to have, do, or get away from) or need in their daily lives (Dawkins 2004). The response of the birds to conspecifics may tell us what broiler chickens want and the qualities they have been bred for. Broilers are bred for rapid growth and approach a feeding trough up to 50 times in 24 h (Weeks et al. 2000). The low threshold measured from the data, above which another bird will approach a feeder already occupied by a bird, gives rise to the possibility that this breeding may have led to increased social facilitation rather than, or perhaps in addition to, a simple baseline increase in feeding tendency. Increasing trough space relative to stocking density may actually reduce the number of visits that chickens make to the trough. How this would impact upon consumption patterns, however, is a matter for further study.

In terms of the welfare of the birds in a commercial broiler house, our results suggest that birds will cluster at the feeder independent of stocking density. These results are further supported by the conclusions of Febrer et al. (in press), who found that regardless of stocking density, broiler chickens form local clusters in open areas away from feeding and drinking equipment in broiler houses (their study did not look at feeding/drinking areas). Our data and model predict that at lower densities birds will cluster at the feeders, so that they experience similar local densities regardless of the global stocking density. These results could help explain Dawkins et al. (2004) conclusion that stocking density may not have as much of a direct impact on broiler chicken welfare as other environmental factors.

Our model predicted the sudden synchronized bursts of feeding seen in broiler chickens feeding at lower densities (see figures 2b and 3b), suggesting that these bursts arise through social facilitation. Once a threshold number of birds is exceeded at any particular section on the feeder, a positive feedback loop begins as more birds aggregate at that section. However, if feeding troughs are difficult to move away from when in high use, the aggregative behaviour of the birds may lead to crowding even at lower stocking densities. Numerous studies have shown that thwarting access to food leads to ‘frustration’-related behaviours in domestic hens (e.g. Duncan & Wood-Gush 1972; Zimmerman et al. 2000). Since local high densities of birds could thwart other birds' access to food, the clustering dynamics we have observed here should thus be taken into account in the design of the broiler home environment.

Our current model and parameter measurements should prove a basis for more realistic models that could inform the design of modern commercial animal housing, which minimizes crowding and frustration by maximizing even access to food and water. This type of model has already been applied to the dynamics of pedestrians in crowded situations, such as during escape panic (reviewed in Helbing 2001). These models have shown that in crisis situations, herding behaviour normally results in entire groups moving in the same direction, which can lead to dangerous levels of overcrowding (Helbing et al. 2000). Thus, birds whose behaviour shows a high degree of social facilitation are exactly the type of individuals who would do worst in situations involving crowding and panic. However, there are ways to counteract the problems of herding. For example, Helbing et al. (2000, supplement at http://angel.elte.hu/panic/) showed that by placing a pillar in front of an emergency exit, the rate of passage through the exit was much higher than when there was no pillar, when the probability of crowding was high. We hope that models where social behaviour of individual animals is accurately captured can lead to similar improvements in the design of animal housing.