Changes in body temperature influence the scaling of ${\dot{V}}_{{\text{O}}_{2\text{max}}}$ and aerobic scope in mammals

Abstract

Debate on the mechanism(s) responsible for the scaling of metabolic rate with body size in mammals has focused on why the maximum metabolic rate (${\dot{V}}_{{\text{O}}_{2\text{max}}}$) appears to scale more steeply with body size than the basal metabolic rate (BMR). Consequently, metabolic scope, defined as ${\dot{V}}_{{\text{O}}_{2\text{max}}}$/BMR, systematically increases with body size. These observations have led some to suggest that ${\dot{V}}_{{\text{O}}_{2\text{max}}}$ and BMR are controlled by fundamentally different processes, and to discount the generality of models that predict a single power-law scaling exponent for the size dependence of the metabolic rate. We present a model that predicts a steeper size dependence for ${\dot{V}}_{{\text{O}}_{2\text{max}}}$ than BMR based on the observation that changes in muscle temperature from rest to maximal activity are greater in larger mammals. Empirical data support the model's prediction. This model thus provides a potential theoretical and mechanistic link between BMR and ${\dot{V}}_{{\text{O}}_{2\text{max}}}$.

1. Introduction

A mechanistic explanation for differences in the scaling of basal and maximum metabolic rates with body size remains elusive (Bishop 1999). The basal metabolic rate (BMR or B) is generally taken to scale to the 3/4 power of the body size (i.e. $B\propto M^{0.75}$; Savage et al. 2004; Weibel et al. 2004; Farrell-Gray & Gotelli 2005; but see White & Seymour 2005). However, the maximum metabolic rate (${\dot{V}}_{{\text{O}}_{2\text{max}}}$ or V) scales with an exponent closer to 0.80, with values ranging between 0.79 and 0.87 (Taylor et al. 1981; Bishop 1999; Savage et al. 2004; Weibel et al. 2004; White & Seymour 2005). As a result, the aerobic scope (V/B) increases with body size by about two- to fourfold from 25 g mice to 500 kg horses. However, because aerobic scope also varies substantially between athletic and non-athletic species of similar size, this relationship applies only to animals of similar athletic ability (Weibel et al. 1987).

Differences in the scaling exponents reported for B and V have led some researchers to suggest that these processes are controlled by fundamentally different mechanisms (e.g. Bishop 1999; Weibel et al. 2004). These differences have also led some (Bishop 1999; Darveau et al. 2002; Hochachka et al. 2003; Weibel et al. 2004) to discount the generality of models that claim to derive a scaling exponent of 3/4 for metabolic rate based on the optimization of the architecture of delivery networks (e.g. West et al. 1997; Banavar et al. 1999). Here, we propose a model, based on principles of allometry and biochemical kinetics, that relates the scaling of BMR to that of ${\dot{V}}_{{\text{O}}_{2\text{max}}}$ for mammals of similar athletic ability. The model is derived based on the observation that the change in muscle temperature from rest to maximal activity is greater in larger mammals, perhaps due to differences in the rates of heat dissipation.

2. Model development

Our model is an extension of the one presented by Gillooly et al. (2001), which accounts for substantial variation in metabolic rate within and among ectotherms and endotherms, including endotherms in hibernation and torpor. The model predicts variation in basal (i.e. minimum) metabolic rate, B (W), based on the combined effects of the body size, M (kg), and body temperature, T_B (K),

$$B=b_0{M}^{3/4}{\text{e}}^{-E/k{T}_{\text{B}}},$$ (2.1)

where b₀ is a normalization constant (W kg^−3/4), independent of the body size and the temperature, that may vary among taxa and environments. The 3/4-power scaling exponent for the body size term has been attributed to biophysical constraints on the delivery of resources through distribution networks (West et al. 1997). The exponential increase in metabolic rate with temperature is described by the Boltzmann–Arrhenius factor, $e^{-E/k{T}_{\text{B}}}$, where E is the average activation energy of the respiratory complex (approx. 0.6–0.7 eV); k is Boltzmann's constant (8.62×10⁻⁵ eV K⁻¹); and T_B is the body temperature of the organism in the basal state in Kelvin (Gillooly et al. 2001).

To predict ${\dot{V}}_{{\text{O}}_{2\text{max}}}$ and aerobic scope in mammals, we must account for the increase in body temperature that accompanies increases in metabolic rate. The increase in temperature from BMR to ${\dot{V}}_{{\text{O}}_{2\text{max}}}$, T_B to T_V, which is presumably a consequence of increased heat production, can be incorporated into equation (2.1) to characterize its effects on ${\dot{V}}_{{\text{O}}_{2\text{max}}}$,

$$V=v_0{M}^{3/4}{\text{e}}^{-E/k{T}_{\text{V}}}\approx v_0{M}^{3/4}{\text{e}}^{(E/k{T}_{\text{B}}^2)\mathrm{\Delta }T(M)-E/k{T}_{\text{B}}}.$$ (2.2)

Here, v₀ is a normalization constant (W kg^−3/4), independent of the body size and the temperature, and $\mathrm{\Delta }T(M)=T_{\text{V}}-T_{\text{B}}$. Combining this expression with equation (2.1) yields an expression for aerobic scope,

$$\frac{V}{B}=\left(\frac{v_0}{b_0}\right){\text{e}}^{-(E/k)((1/T_{\text{V}})-(1/T_{\text{B}}))}\approx \left(\frac{v_0}{b_0}\right){\text{e}}^{(E/k{T}_{\text{B}}^2)\mathrm{\Delta }T(M)}.$$ (2.3)

The approximations in equations (2.2) and (2.3) differ from the exact expressions by less than 1% when ΔT(M)<6 K and E=0.65 eV.

Equations (2.2) and (2.3) indicate that two mechanisms contribute to the increase in metabolic rate from BMR to ${\dot{V}}_{{\text{O}}_{2\text{max}}}$, and thus to aerobic scope. First, increases in the normalization constant from b₀ to v₀ occur due to changes in the structure and function of the organism, including the facultative ones. Second, increases in body temperature, denoted by ΔT(M), have an exponential effect on the metabolic rate. These increases are best characterized using muscle temperatures because muscles consume more than 90% of oxygen at ${\dot{V}}_{{\text{O}}_{2\text{max}}}$ (Weibel et al. 2004). Empirical data indicate that respiration rates of isolated muscle mitochondria, and of muscle tissues measured in vivo, exhibit the same temperature dependence as whole-organism metabolic rate (i.e. an activation energy E≈0.6–0.7 eV; Brooks et al. 1971; Ranatunga 1998; Binzoni et al. 2002).

Equation (2.2) predicts that ${\dot{V}}_{{\text{O}}_{2\text{max}}}$ will scale with body size with an exponent higher than 3/4 if the body temperature at ${\dot{V}}_{{\text{O}}_{2\text{max}}}$ increases with body size. We predict this exponent based on the empirical observation (see §4) that the change in body temperature during strenuous activity increases with body size as

$$\mathrm{\Delta }T(M)=\alpha \text{ln}(M)+\beta ,$$ (2.4)

where α (K kg⁻¹) and β (K) represent the slope and the intercept, respectively. Equations (2.1)–(2.4) predict that the maximum metabolic rate will increase with body size as

$$V\propto M^{(3/4)+(E/k{T}_{\text{B}}^2)\alpha },$$ (2.5)

and that aerobic scope will increase with body size as

$$\frac{V}{B}\propto M^{(E/k{T}_{\text{B}}^2)\alpha }.$$ (2.6)

Equations (2.5) and (2.6) can be used to evaluate our hypothesis that size-dependent changes in muscle temperature, characterized by α, contribute to the size dependence of ${\dot{V}}_{{\text{O}}_{2\text{max}}}$ and aerobic scope. This hypothesis is consistent with the research showing that muscle temperatures increase as metabolic rate increases with strenuous exercise (Hodgson et al. 1993; Weishaupt et al. 1996).

Our model makes one testable, quantitative prediction. If changes in body temperature from B to V increase with body mass, then after correcting for these temperature changes, the logarithm of ${\dot{V}}_{{\text{O}}_{2\text{max}}}$, $\text{ln}(V{\text{e}}^{-(E/k{T}_{\text{B}}^2)\mathrm{\Delta }T(M)})$, should be a linear function of the logarithm of body size, $\ln \left(M\right)$, with a slope equal to 3/4. Support for this prediction would indicate that ${\dot{V}}_{{\text{O}}_{2\text{max}}}$ scales with body mass with an exponent higher than 3/4 due to changes in muscle temperature with body size.

3. Material and methods

We evaluated the size dependence of temperature change from BMR to ${\dot{V}}_{{\text{O}}_{2\text{max}}}$ using data compiled from the literature for nine species of mammals (n=17) that range in size from 0.02 to 565 kg (see table 1 and the electronic supplementary material). We assessed the model prediction using the ${\dot{V}}_{{\text{O}}_{2\text{max}}}$ data of Taylor et al. (1981), because it is the largest compilation of data (n=20) where all animals were measured in the same manner at or near the same environmental temperature.

Table 1.

Data compiled from the literature. (See electronic supplementary material for a listing of the full references.)

species	n	body mass (kg)	temperature (°C)
			ambient	resting	$V_{{\text{O}}_{2\text{max}}}$	location	reference
deer mouse	48	0.024	20	38.0	38.6	colon	Chappell & Hammond (2004)
rat	6	0.198	28	36.3	37.0	muscle	Ardevol et al. (1998)
rat	6	0.198	28	36.7	37.0	blood	Ardevol et al. (1998)
rat	13	0.302	24	37.5	39.3	colon	Tanaka et al. (1988)
antelope	1	15.2	25	38.9	43.8	blood	Taylor & Lyman (1972)
dog	5	17.2	22	39.2	42.4	muscle	Kruk et al. (1985)
dog		19.6	21	39.4	41.9	muscle	Greenleaf et al. (1995)
dog	9	26	—	38.0	41.7	blood	Hsia et al. (1995)
human	12	70.4	20	35.6	39.0	muscle	Febbraio et al. (1994)
human	7	73.1	25	35.4	38.8	muscle	Koga et al. (1997)
goat	3	71	23	38.9	41.3	blood	Baker & Nijland (1993)
pony	8	167	20	37.3	40.8	blood	Manohar et al. (1992)
steer	3	449	—	39.0	42.4	blood	Jones et al. (1989)
standardbred horse	4	469	—	37.3	42.1	muscle	Jones et al. (1989)
thoroughbred horse	6	511	22	37.8	43.9	muscle	Hodgson et al. (1993)
thoroughbred horse	6	511	22	38.0	42.5	blood	Hodgson et al. (1993)
standardbred horse	2	565.5	16	37.6	43.3	muscle	Weishaupt et al. (1996)

4. Results

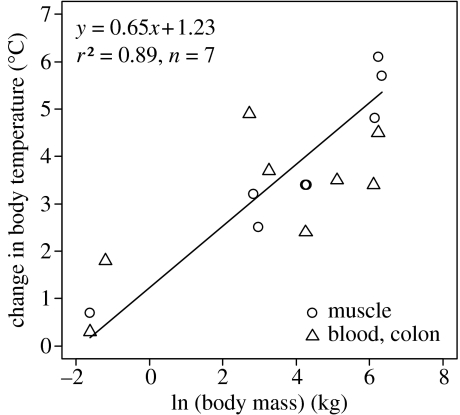
Data show that the change in body temperature from BMR to ${\dot{V}}_{{\text{O}}_{2\text{max}}}$ increases with body size (figure 1). The relationship can be described by a linear relationship that explains 89% of the variation. The difference in $\mathrm{\Delta }T(M)$ between small and large mammals is substantial: the muscle temperature of a 0.2 kg rat increases by less than 1°C, whereas that of a 511 kg horse increases by about 6°C. The linear relationship shown in figure 1 is fitted using only temperature data from muscles (n=7), but colon and blood temperatures show a qualitatively similar trend with body size, albeit with more scatter and a somewhat shallower slope (see electronic supplementary material).

Figure 1.

The change in core body temperature from BMR to at or near exercise-induced ${\dot{V}}_{{\text{O}}_{2\text{max}}}$ as a function of body size in mammals. The regression line is only fit to data where muscle and environmental temperatures were measured (table 1).

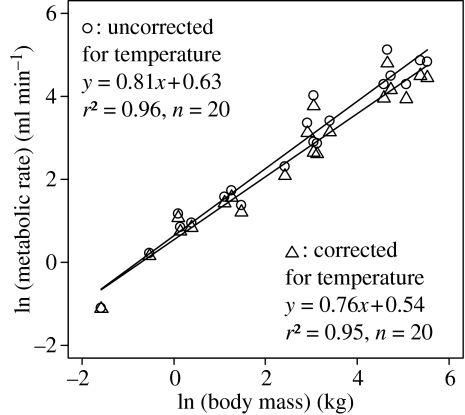
Data also show that increases in $\mathrm{\Delta }T(M)$ with body size account for differences in the scaling exponents between BMR and ${\dot{V}}_{{\text{O}}_{2\text{max}}}$. Based on the relationship between changes in muscle temperature and body size (figure 1), and using an activation energy E=0.65 eV and a body temperature of T_B=311 K, equation (2.5) predicts that ${\dot{V}}_{{\text{O}}_{2\text{max}}}$ should show a scaling exponent of 0.80. The scaling exponent for ${\dot{V}}_{{\text{O}}_{2\text{max}}}$ of 0.81 in Taylor et al. (1981) supports this prediction (95% CI 0.73–0.90). To demonstrate this, after correcting for the effect of temperature in these data, the scaling exponent for ${\dot{V}}_{{\text{O}}_{2\text{max}}}$ decreases from 0.81 to 0.76 (95% CI 0.68–0.85; figure 2). Thus, $\mathrm{\Delta }T(M)$ accounts for the difference between the 0.81 scaling exponent observed by Taylor et al. (1981) and the predicted value of 3/4. Owing to the size dependence of changes in temperature, the temperature correction results in a 1% change in metabolic rate for a 0.2 kg rat and a 51% change for a 511 kg horse.

Figure 2.

The exercise-induced ${\dot{V}}_{{\text{O}}_{2\text{max}}}$ as a function of body size in mammals. Data are from Taylor et al. (1981). The original data are corrected for temperature using equation (2.2) and values of $\mathrm{\Delta }T(M)$ estimated from the relationship in figure 1.

5. Discussion

The model and data presented here indicate that changes in muscle temperature from rest to maximal activity are greater in larger mammals (figure 1), and that this relationship could potentially explain differences in the scaling of BMR and ${\dot{V}}_{{\text{O}}_{2\text{max}}}$ with body size. Systematic increases in muscle temperature with body size can account for the difference between the predicted scaling exponent of 3/4 and the higher exponents observed for ${\dot{V}}_{{\text{O}}_{2\text{max}}}$ by Taylor et al. (1981) and Bishop (1999) (exponent of 0.79). However, the higher scaling exponents observed for ${\dot{V}}_{{\text{O}}_{2\text{max}}}$ in other studies (e.g. Savage et al. 2004; Weibel et al. 2004; White & Seymour 2005), which use much of the same data, range between 0.83 and 0.87. In these studies, our model would account for 45–65% of the difference resulting from 3/4-power scaling. The remaining difference may be due to greater numbers of larger, more athletic species, longer exercise (or ‘warm-up’) periods for larger species, and perhaps most importantly, due to differences in the environmental temperatures at which BMR and ${\dot{V}}_{{\text{O}}_{2\text{max}}}$ were measured (see electronic supplementary material). The importance of accounting for environmental temperature is supported by studies showing that metabolic scope is greater for larger rodents and marsupials when ${\dot{V}}_{{\text{O}}_{2\text{max}}}$ is exercise-induced rather than cold-induced (Hinds 1992; Hinds et al. 1993). Our model suggests that this observation is attributable to increases in muscle temperature that occur during exercise-induced ${\dot{V}}_{{\text{O}}_{2\text{max}}}$, but not during cold-induced ${\dot{V}}_{{\text{O}}_{2\text{max}}}$.

In conclusion, our model potentially provides a mechanistic link between BMR and ${\dot{V}}_{{\text{O}}_{2\text{max}}}$ in mammals. In so doing, it presents a hypothesis that makes the general models of West et al. (1997) and others (e.g. Banavar et al. 1999), which predict 3/4-power scaling for the metabolic rate, compatible with the steeper scaling exponents reported for ${\dot{V}}_{{\text{O}}_{2\text{max}}}$. However, the range of mammalian body sizes over which the model can reasonably be applied is limited to the size range considered here. At larger body sizes, the change in body temperature cannot continue to increase. A 500 kg horse approaches near-lethal body temperatures of approximately 45°C at ${\dot{V}}_{{\text{O}}_{2\text{max}}}$ (Hodgson et al. 1993). This suggests that metabolic scope in mammals reaches a maximum near the body size of a horse. It also suggests that the scaling relationships of BMR and ${\dot{V}}_{{\text{O}}_{2\text{max}}}$ cannot continue to diverge much beyond this size. Overall, this study highlights the need for further research on how environmental temperature, muscle temperature and exercise duration combine to influence the size dependence of ${\dot{V}}_{{\text{O}}_{2\text{max}}}$ and aerobic scope.