Applicability of the glomerular size distribution coefficient in assessing human glomerular volume: the Weibel and Gomez method revisited

Abstract

Changes in glomerular volume (V_glom) play an important role in the initiation and progression of various glomerulopathies. Estimation of V_glom in the normal kidney provides baseline values for studies of glomerular hypertrophy in disease. The traditional model-based method of Weibel and Gomez is widely applied to estimate V_glom in clinical biopsy specimens. Assumptions of glomerular size distribution and shape required by this method are potential sources of bias that have not been verified. We evaluated the applicability of the glomerular size distribution coefficient in estimating V_glom in human kidneys. V_glom of 720 non-sclerotic glomeruli in histologically normal kidneys of 24 males (20–69 years) was estimated by the unbiased disector/Cavalieri approach. Accurate glomerular diameters were calculated from Cavalieri estimates of V_glom assuming glomerular sphericity. The coefficients of variation (CV) of glomerular diameters were compared with the corresponding values of the size distribution coefficient predicted by the Weibel and Gomez method. Mean (SD) glomerular diameter was 201 (28) mm (range 110–276 mm). The CV of glomerular diameter within each kidney ranged from 4.9 to 14.6%. Corresponding glomerular size distribution coefficients predicted by the formula of Weibel and Gomez ranged from 1.00 to just 1.03. The value of the size distribution coefficient required by the Weibel and Gomez technique when estimating V_glom in normal human kidneys is remarkably constant. This is despite large variations in V_glom. Future studies should examine the extent of bias introduced by the glomerular shape assumptions of this method.

Introduction

Accurate estimation of glomerular volume (V_glom) has become increasingly important because V_glom varies significantly in normal human kidneys (Samuel et al. 2005), and because alterations in V_glom may play a significant pathophysiological role in the development or progression of a range of nephropathies (Bilous et al. 1989; Keller et al. 2003). Glomerular enlargement precedes glomerulosclerosis in idiopathic focal segmental glomerulosclerosis (FSGS), diabetic nephropathy, obesity-related glomerulopathy, HIV nephropathy, reflux nephropathy, pre-eclampsia, sickle cell nephropathy and transplant glomerulopathy (El-Khatib et al. 1987; Fogo et al. 1990; Pardo et al. 1991; Bathena, 1993; Nochy et al. 1994; Bertram et al. 1998; Praga et al. 2001). Furthermore, changes in V_glom that occur with different degrees of glomerulosclerosis in conditions characterized by FSGS may be of prognostic significance (Bertram et al. 1998; Fogo et al. 1990). V_glom can be reduced in ischaemic renal injury and may be associated with long-term passive smoking (Moran et al. 1992; Dundar et al. 2004).

Unbiased design-based stereological methods are currently the preferred approach for estimation of V_glom (Bertram, 1995; Madsen, 1999). However, the model-based stereological method of Weibel and Gomez (Weibel, 1980), which originally attempted to estimate glomerular number based on the relationship between volume and mean cross-section area – which depend on glomerular shape and a size distribution factor, is still widely applied to measure V_glom (Bertram et al. 1998). This method requires only a single random section through each glomerulus and is therefore well suited to clinical biopsy specimens with a limited number of glomerular profiles. The Weibel and Gomez method is also more time efficient over the ‘gold standard’ unbiased Cavalieri principle (Gundersen & Jensen, 1987; Bertram, 1995).

The Cavalieri method requires exhaustive sectioning of the glomerulus in large kidney tissue samples and requires sizing glomerular profiles in consecutive serial sections of complete glomeruli (Samuel et al. 2005). Unlike the Cavalieri principle, the method of Weibel and Gomez (Weibel, 1980) requires knowledge or assumptions about the shape and size of glomeruli. To the extent that these assumptions differ from true values, estimates of V_glom will be biased. According to the Weibel and Gomez formula, V_glom = glomerular profile area^1.5 × β/K, where β is a shape coefficient of 1.38 for a sphere and K is a size distribution coefficient. Values for K vary between 1 and 1.05 for glomerular size distributions with standard deviations of less than 20% of the mean within a single specimen of kidney tissue. It is well known that glomeruli vary in size and shape within the same kidney (Samuel et al. 2005), and therefore any bias introduced in the estimation of V_glom using the method of Weibel and Gomez needs to be determined.

The aim of this investigation was to assess the applicability of the Weibel and Gomez glomerular size distribution coefficient K, when estimating V_glom within normal human kidneys.

Materials and methods

The study population consisted of 24 North American males (12 African Americans and 12 Caucasians) aged 20–69 years who had died suddenly of non-renal causes (Samuel et al. 2005). Post-mortem kidney specimens were collected at the University of Mississippi Medical Center (Jackson, MS). Ethical approval for the use of autopsy tissue for clinical research was obtained by informed consent from the first of kin and approved by the Internal Review Board of the University of Mississippi Medical Center. Exclusion criteria included a history of kidney disease, significant size asymmetry between the right and left kidney, and histologically proven glomerular or tubulointerstitial disease. Details of tissue processing and estimates of individual glomerular volumes have previously been reported (Samuel et al. 2005). Briefly, one mid-sagittal half of each perfusion-fixed right kidney was selected randomly for analysis. A block of tissue was cut from the mid-hilar region of the kidney, embedded in glycolmethacrylate (Technovit 7100, Heraeus Kulzer Gmbh, Germany) and serially sectioned at 10 µm. Glycolmethacrylate was preferred to paraffin as the embedding medium because the dimensional changes associated with tissue processing for glycolmethacrylate embedding are significantly smaller than those associated with paraffin embedding (Bertram, 1995). Glomeruli were sampled with equal probability regardless of their shape or size using disectors (Sterio, 1984; Bertram, 1995). Thirty non-sclerotic glomeruli were sampled per kidney. Each glomerulus was exhaustively sectioned at a known section thickness, t (10 µm). The glomerular profile tuft area of every second section was measured by the point counting method of Henning (Henning & Meyer-Arendt, 1963) using an orthogonal grid system with points 1 cm apart at a final magnification of ×320. On average 12 sections were measured from each glomerulus.

The area of each glomerular profile (A_glom) was determined using A_glom = ∑ P × a(p), where ∑ P was the number of points hitting the glomerular profile and a(p) was the area associated with each test point on the grid. Glomerular tuft volume was estimated using the Cavalieri principle: V_glom = ∑ A_glom × 2(t). The radius (r) and diameter of each of the 30 sampled glomeruli per kidney were calculated from V_glom = 4/3 × πr³, assuming glomerular sphericity. The mean diameter (d) of the 30 glomeruli and the standard deviation (SD) of the diameter were used to calculate the coefficient of variation (CV) of glomerular diameter per kidney, CV = SD/d. The calculated values for CV were compared with the corresponding values for K predicted by the Weibel and Gomez method (Weibel, 1979).

Results

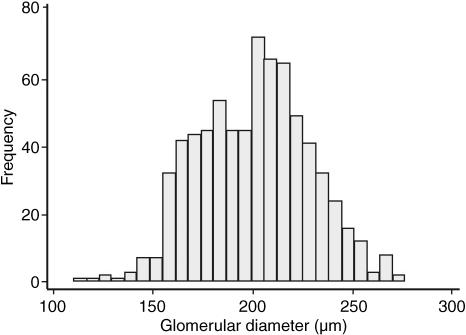
The diameters of each of 30 non-sclerotic glomeruli per kidney were calculated from previous Cavalieri estimates of V_glom in 24 male subjects (Samuel et al. 2005). The frequency distribution of the diameters of a total of 720 glomeruli is shown in Fig. 1. Glomeruli were of varying diameters with a 2.5-fold range. The diameter of the largest glomerulus was 276 µm and the smallest 110 µm. Mean (± SD) glomerular diameter was 201 ± 28 µm.

Fig. 1.

Distribution of diameters of 720 non-sclerotic glomeruli in 24 males aged 20–69 years. The normal distribution was confirmed using the Shapiro Wilk normality test.

The CV of glomerular diameter within each kidney was less than 15% with a three-fold range from 4.9 to 14.6% (Table 1). However, the corresponding glomerular size distribution coefficients (K) predicted by the formula of Weibel and Gomez (Weibel, 1980) were quite constant, ranging from 1.00 to 1.03 (Table 1).

Table 1.

Glomerular diameters, coefficients of variation (CV) of glomerular diameters derived from Cavalieri estimates of glomerular volume, and the Weibel and Gomez glomerular size distribution constant (K) in kidneys of 24 males aged 20–69 years

Subject	Age (years)	Race	No. of glomeruli analysed	Minimum glomerular diameter (µm)	Maximum glomerular diameter (µm)	Mean (SD) glomerular diameter (µm)	CV of glomerular diameter CV (%)	Size distribution coefficient (K)*
1	20	African American	30	153	201	172 (11)	6.6	1.00
2	21	African American	30	138	205	176 (17)	9.6	1.00
3	21	Caucasian	30	195	250	219 (13)	5.7	1.00
4	22	African American	30	188	231	207 (10)	4.9	1.00
5	22	Caucasian	30	149	205	178 (13)	7	1.00
6	22	Caucasian	30	155	202	176 (12)	6.6	1.00
7	25	African American	30	160	252	208 (22)	10.7	1.01
8	28	African American	30	127	248	209 (31)	14.6	1.03
9	29	African American	30	184	268	221 (22)	9.8	1.01
10	29	Caucasian	30	136	193	163 (10)	6.1	1.00
11	30	Caucasian	30	132	219	191 (19)	9.9	1.01
12	30	Caucasian	30	149	276	221 (26)	11.8	1.02
13	51	African American	30	163	237	218 (14)	6.5	1.00
14	51	African American	30	184	268	214 (16)	7.6	1.00
15	51	African American	30	165	249	224 (22)	10	1.01
16	56	African American	30	175	275	237 (28)	12	1.02
17	61	African American	30	158	265	229 (21)	9.4	1.00
18	63	Caucasian	30	159	220	181 (14)	7.6	1.00
19	65	African American	30	156	224	186 (18)	9.8	1.01
20	65	Caucasian	30	163	244	202 (17)	8.2	1.00
21	67	Caucasian	30	127	237	204 (23)	11.2	1.01
22	67	Caucasian	30	110	209	177 (23)	13	1.02
23	68	Caucasian	30	159	227	193 (18)	9.6	1.00
24	69	Caucasian	30	142	246	213 (19)	9	1.00
Mean (SD)	43 (19.3)					201 (28)	9.1

^*Values of K for a given value of CV were obtained from the estimates of Weibel and Gomez.

Discussion

The development of unbiased design-based stereological techniques over the past 20 years has revolutionized the quantification of three-dimensional cell, tissue and organ structure (Nyengaard, 1999; Gundersen et al. 1988a,b; Bertram et al. 1992; Bertram, 1995). Unfortunately, these methods are not suitable for estimation of glomerular dimensions in limited clinical core biopsy specimens. However, the unbiased techniques provide a mechanism whereby traditional model-based and potentially biased stereological methods that are more practical in clinical application, such as the method of Weibel and Gomez (Weibel, 1980), can be validated. In the present study, we utilized the gold standard disector/Cavalieri combination, to assess the applicability of the Weibel and Gomez method to estimate V_glom in normal human kidneys.

‘True’ glomerular diameters were derived using estimates of V_glom obtained with the unbiased Cavalieri method in glomeruli sampled with the disector method and embedded in glycolmethacrylate. This approach overcame several potential sources of bias and error associated with alternative methods for estimating glomerular diameter. First, all glomeruli had the same chance of being included in the sample, because of the use of the disector sampling approach. This overcame the problem of preferentially sampling large glomeruli. Second, there was no need to estimate diameter in maximal glomerular profiles. And finally, tissue shrinkage and deformations were minimized with the use of glycolmethacrylate as the embedding medium.

In a previous study from our laboratory (Bertram et al. 1998) the method of Weibel and Gomez was found to overestimate mean V_glom of 17 initial transplant biopsy specimens by 23% compared with Cavalieri estimates based on 238 glomeruli. Paraffin sections were analysed in this earlier study. It should be noted that the use of glycolmethacrylate as the embedding medium in the present study of a larger sample of 720 glomeruli very likely contributed to the good agreement between the CV of glomerular diameters derived from the Cavalieri principle and the Weibel and Gomez estimates of glomerular size distribution. Tissue embedded in glycolmethacrylate undergoes far less shrinkage and distortion than tissue processed for embedding in paraffin (Miller & Meyer, 1990).

Another critical parameter to consider in studies of this kind is section thickness. Macleod et al. (2000) demonstrated that when using the Cavalieri principle, section intervals greater than 20 µm could result in a significant increase in the variance of the estimate of V_glom in biopsies of diabetics. This is particularly relevant as various disease processes can distort both glomerular size and shape, thereby creating a different distribution of glomerular size. Glomeruli deviate from the shape of a sphere and can be ellipsoidal even in the normal kidney (Abrams et al. 1963).

We have recently addressed the other important consideration of how many glomerular profiles must be measured to obtain reliable estimates of mean glomerular areas in human renal biopsies and found that estimates based on random sampling of five or more glomerular profiles per biopsy reliably estimated the ‘true’ population mean of a group of at least 30 biopsies (Hoy et al. 2006). The remaining challenge is to determine the values of the glomerular shape coefficient (β) of the Weibel and Gomez approach to estimation of V_glom in normal and diseased human kidneys.

In conclusion, the present findings indicate the remarkable stability of the values of the size distribution coefficient (K) when estimating glomerular volume in normal human kidneys using the method of Weibel and Gomez (Weibel, 1980).