Morphometrics and inertial properties in the body segments of chimpanzees (Pan troglodytes)

Abstract

Inertial characteristics and dimensions of the body and body segments form an integral part of a biomechanical analysis of motion. In primate studies, however, segment inertial parameters of non-human hominoids are scarce and often obtained using varying techniques. Therefore, the principal aim of this study was to expand the existing chimpanzee inertial property data set using a non-invasive measuring technique. We also considered age- and sex-related differences within our sample. By means of a geometric model based on Crompton et al. (1996); Am J Phys Anthropol 99, 547–570) we generated inertial properties using external segment length and diameter measurements of 53 anaesthetized chimpanzees (Pan troglodytes). We report absolute inertial parameters for immature and mature subjects and for males and females separately. Proportional data were computed to allow the comparison between age classes and sex classes. In addition, we calculated whole limb inertial properties and we discuss their potential biomechanical consequences. We found no significant differences between the age classes in the proportional data except for hand and foot measures where juveniles exhibit relatively longer and heavier distal segments than adults. Furthermore, most sex-related differences can be directly attributed to the higher absolute segment masses in male chimpanzees resulting in higher moments of inertia. Additionally, males tend to have longer upper limbs than females. However, regarding proportional data we discuss the general inertial properties of the chimpanzee. The described segment inertial parameters of males and females, and of the two age classes, represent a valuable data set ready for use in a range of biomechanical locomotor models. These models offer great potential for improving our understanding of early hominin locomotor patterns.

Introduction

One of the most distinctive characters of human locomotion is habitual bipedal walking. Evidence from fossil remains and footprints suggest that human ancestors started walking on two legs rather than four between 7 and 4 million years ago (Lovejoy, 1988; Fleagle, 1999; Senut et al. 2001). However, the origins, the early evolution and adaptive context of human bipedality remain poorly understood. As experimental studies on extinct species are impossible, comparative biomechanical research on extant species, for example primates, can provide more insight. Through inverse dynamics we can calculate unknown muscle forces from given morphological, kinematic and kinetic data (Crompton et al. 1998; Kramer, 1999; Wang et al. 2004). Likewise, forward dynamic simulation can be a powerful approach to investigate movements in a predictive manner (Kimura et al. 1978; Nagano et al. 2005). Using this methodology it is possible to compile the available knowledge (of skeletal information, muscles, etc.) into a single model, to generate potential walking movements of our ancestors.

It has long been clear that there is a close relationship between locomotor behaviour and proportions of body segments (Zihlman, 1984; Morbeck & Zihlman, 1989; Winter, 1990; Preuschoft & Gunther, 1994; Myers & Steudel, 1997; Raichlen, 2004, 2005a, 2006). For instance, a more proximal mass distribution in the limbs may reduce the energy cost during swinging in bipedal or quadrupedal gaits (but see Taylor et al. 1974; Raichlen, 2006). The intermembral index (IMI, length of the anterior extremity divided by the length of the posterior extremity) increases with body size in primates, possibly because competence in vertical climbing is to be maintained (for details see Jungers & Susman, 1984). Both biomechanical (forward and inverse modelling) approaches mentioned above require data concerning lengths, mass distributions, positions of centre of mass and moments of inertia of involved segments. Various methods have been developed to measure these segment parameters, for example the pendulum method (Dempster, 1955), scanning systems (Mungiole & Martin, 1990; Zatsiorsky et al. 1990), video-imaging (Baca, 1996) and mathematical methods (Hanavan, 1964; Hatze, 1980). Estimates of segment inertial properties are frequently based on data and procedures developed for humans (e.g. Clauser et al. 1969; Chandler et al. 1975).

For primates valuable segment inertial data have been published occasionally. Reynolds (1974) measured segment masses, centres of gravity and principal moments of inertia of Papio using a single pendulum technique whereas Raichlen (2005b) studied the ontogeny of limb mass distribution in four living infant baboons (Papio cynocephalus) using external measurements. Vilensky (1979) and Cheng & Scott (2000) report centres of gravity and moments of inertia in Macaca mulatta and comparable data are published for one Lemur fulvus (Wells & DeMenthon, 1987).

Unfortunately, ape cadavers are extremely rare and difficult to obtain, limiting the currently available hominoid inertial data. This has led to a need for developing alternative approaches for measuring inertial properties more directly on living subjects. Crompton et al. (1996) presented a geometric model based on external measurements of length and diameters of body segments considering that segments have elliptical cross-sections and that the profiles of real segments are generally curved. This promising technique is appropriate for all (anaesthetized) primates and has already been adopted in several other studies (Raichlen, 2005b; Isler et al. 2006; Wall-Scheffler et al. 2006). In the study mentioned above Crompton et al. published inertial properties derived from four Pan troglodytes specimens and a Pongo pygmaeus using the double pendulum technique and external measurements. Isler et al. (2006) built on the work of Crompton et al. (1996) but added inertial data of five segmented hominoid cadavers and eight intact hominoid specimens. These studies provide extremely valuable data on chimpanzees and other hominoids but the limited number of individuals considered does not permit the assessment of interindividual variation. Therefore, biomechanical analyses of hominoid locomotion would greatly benefit from a more extensive data set of hominoid non-human primate morphometrics and inertial parameters.

The major aim of this study is to present a large data set of chimpanzee morphometric and segment inertial parameters in order to provide information about age- and sex-related differences and intraspecific variation. More specifically, these data are applicable in chimpanzee and locomotion studies and in the investigation of potential locomotor patterns of hominin ancestors through forward dynamic modelling.

Materials and methods

Subjects

Fifty-three anaesthetized chimpanzees (Pan troglodytes), 23 males and 30 females, were measured in this study. The individuals were kept at the Biomedical Primate Research Centre (BPRC), Rijswijk, the Netherlands. The animals were housed in large open-air cages provided with facilities for exercise. During the annual medical check-up no visible musculo-skeletal abnormalities were found. For all individuals, age, total body mass (TBM), stature length (this is the summation of trunk, thigh and shank length) and in most cases subspecies were known. Forty-one chimpanzees were thoroughbred Pan troglodytes verus and 11 were a mixture of Pan troglodytes verus and Pan troglodytes troglodytes, or a mixture of Pan troglodytes verus and a mother or father from unknown subspecies. One individual was a pure Pan troglodytes troglodytes. Pan troglodytes schweinfurthii, a subspecies known for its smaller dimensions compared with other Pan troglodytes (but with the same limb proportions, Morbeck & Zihlman, 1989), was not represented in this study. Because no apparent differences between subspecies were found, no subdivision in subspecies was made but we did account for size differences in all analyses.

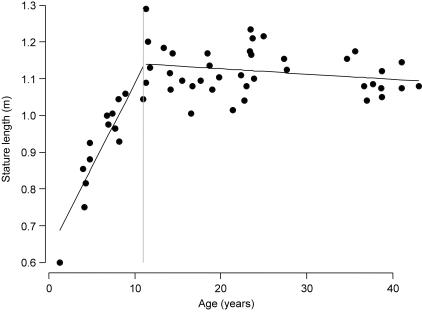
The numbers of young individuals were not sufficient to permit an elaborate division according to age. Following Hamada & Udono (2002), who state that body length maturation occurs at the age of 12 years and does not differ with sex, our data set was separated into one group of individuals under 12 years old (immature) and a second group of subjects above 12 years (mature). Figure 1 demonstrates that stature length increases linearly until the age of approximately 12 years after which the chart flattens and no further growth of the stature length is observed.

Fig. 1.

Stature length vs. age in a Pan troglodytes sample of 53 subjects. Stature length increases linearly until the age of approximately 12 years (dashed line represents partition between age classes) and remains even at older ages.

Segment-inertial model

External simple linear measurements of eight segments were taken: head, trunk, upper arm, forearm, hand, thigh, shank and foot. For each segment, the segment length as well as proximal, medial and distal diameters in both the sagittal and the frontal plane were measured. To obtain reliable measurements it is of great importance that clear landmark points are used. Therefore, details are given in the Appendix.

A geometric model based on Crompton et al. (1996) was applied to determine the inertial properties of the body segments. Using the external measurements, segment mass and location of the centre of mass relative to segment length with respect to the more proximal joint were calculated. Two principal moments of inertia were computed, the first (I_x) around the coronal axis which lies in the frontal plane and extends horizontally from side to side. The movements of flexion and extension take place about this axis in a sagittal plane. The second (I_y) is around the sagittal axis which lies in the sagittal plane and extends horizontally from front to back. The movements of abduction and adduction take place about this axis in a frontal plane. A third principal moment of inertia around the longitudinal axis was not considered here because it is rather sensitive to errors and of smaller interest for ape locomotion studies. Density was assumed to be 10³ kg m⁻³ for all segments.

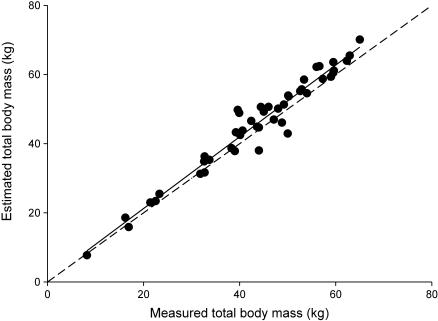
To enable comparisons between sex and age classes, segment length and mass were calculated as percentages of stature and total body mass (Fig. 2, see also Discussion), respectively. Likewise, the segment radius of gyration expressed as a percentage of segment length was used to normalize moments of inertia with body size. This parameter is defined as:

where I is the moment of inertia, m the segment mass and l the segment length.

Fig. 2.

Correlation between total body mass estimated by the model and measured total body mass. The solid line is the least-squares regression (R² = 0.95) and the dashed line is the line of identity (R² = 1).

With these limb segment inertial properties whole limb inertial parameters were calculated including the limb natural pendular period (NPP) for outstretched limbs with the position of the foot at 90° to the shank segment and the hand positioned in a straight line with the upper- and forearm as in knuckle-walking. The NPP can be found using the following equation:

where MI is the limb's moment of inertia about the proximal joint, m is the limb's mass, CoM is the distance of the limb's centre of mass from the proximal joint and g is gravitational acceleration.

Statistics

As the absolute segment inertial parameters increased with age during growth, they were modelled via linear regressions for the immature class in order to quantify their age dependency. As there were no apparent sex differences in these linear relationships, both sexes were pooled to gain estimation accuracy of the relationships. For the full-grown individuals of the mature age class, means and standard errors of each parameter were calculated for each sex, and sex differences were tested in a one-way anova model. Concerning the relative proportions, means and standard errors were calculated for both immature and mature individuals, and differences between sexes and age classes were tested in a one-way anova.

All statistic analyses were performed in SAS 8.02 for Windows. Significance of the tests was corrected for the number of traits considered with a sequential Bonferroni method (Rice, 1989).

Results

Comparison of age classes

In Table 1 the absolute segment inertial parameters are reported for both age categories (immature and mature). For the immature individuals the slopes (a) and intercepts (b) of the linear regression models are shown to describe the growth pattern. All corresponding slope P values are smaller than 0.05, implying that all slopes differ significantly from zero and that all parameters are obviously age dependent. For the mature individuals the means and standard errors for each parameter are given for both sexes separately.

Table 1.

Segment inertial parameters represented by slopes (a) and intercepts (b) of the linear regression models for immature chimpanzees and with means and standard errors for mature male and female chimpanzees

	Immature		Mature
	F/M 14		F 23		M 16
Sex sample size	ax + b	P-value of slope	mean	SE	mean	SE	P-value sex related
age	–	–	27.534	2.050	22.511	2.390	0.121
stature (m)	0.0459age +0.5824	< 0.0001	1.103	0.013	1.146	0.015	0.0314
body mass (kg)	4.0206age −0.4385	< 0.0001	47.700	1.437	56.050	1.792	0.0058
Length (m)
head	0.00823age +0.1513	< 0.0001	0.237	0.003	0.253	0.004	0.0037
trunk	0.02071age +0.2969	0.0004	0.549	0.008	0.568	0.007	ns
upper arm	0.01399age +0.1464	< 0.0001	0.288	0.004	0.303	0.006	0.0256
forearm	0.01504age +0.1455	< 0.0001	0.290	0.004	0.307	0.004	0.0055
hand	0.00926age +0.1481	0.0008	0.237	0.003	0.253	0.006	0.0164
thigh	0.01298age +0.1455	< 0.0001	0.283	0.004	0.293	0.005	ns
shank	0.01224age +0.1399	< 0.0001	0.271	0.004	0.286	0.006	ns
foot	0.01005age +0.1376	< 0.0001	0.242	0.003	0.249	0.003	ns
Mass (kg)
head	0.1497age +0.7218	< 0.0001	2.743	0.092	3.314	0.170	0.0028
trunk	2.3542age −0.5419	< 0.0001	27.894	1.015	32.109	1.090	0.0090
upper arm	0. 1897age −0.3052	< 0.0001	1.913	0.097	2.274	0.117	< 0.0001*
forearm	0.1299age −0.1499	< 0.0001	1.331	0.059	1.655	0.079	0.0020
hand	0.0573age +0.0450	< 0.0001	0.640	0.022	0.823	0.035	ns
thigh	0.3586age −0.6405	< 0.0001	3.635	0.183	4.041	0.178	ns
shank	0.1423age −0.1494	< 0.0001	1.418	0.056	1.758	0.080	0.0010
foot	0.0708age +0.0269	0.0002	0.771	0.026	0.889	0.049	0.0276
Ix (kg m2)
head	0.00126age −0.0021	0.0014	0.01179	0.00063	0.01649	0.00124	0.0007
trunk	0.06691age −0.1479	< 0.0001	0.79844	0.04506	0.99144	0.06288	0.0148
upper arm	0.00164age −0.0055	< 0.0001	0.01323	0.00071	0.01764	0.00136	0.0041
forearm	0.00100age −0.0033	< 0.0001	0.00887	0.00058	0.01219	0.00077	0.0013
hand	0.00031age −0.0005	0.0003	0.00276	0.00013	0.00398	0.00031	0.0003*
thigh	0.00245age −0.0071	< 0.0001	0.02333	0.00144	0.04306	0.01572	ns
shank	0.00093age −0.0025	< 0.0001	0.00851	0.00050	0.01162	0.00085	0.0019
foot	0.00037age −0.0008	0.0002	0.00336	0.00017	0.00420	0.00032	0.0169
Iy (kg m2)
head	0.00107age −0.0008	< 0.0001	0.01289	0.00071	0.01657	0.00143	ns
trunk	0.05866age −0.1310	< 0.0001	0.69131	0.03859	0.84893	0.05251	0.0184
upper arm	0.00175age −0.0059	< 0.0001	0.01417	0.00080	0.02762	0.00857	ns
forearm	0.00111age −0.0034	< 0.0001	0.00914	0.00060	0.01257	0.00083	0.0016
hand	0.00035age −0.0006	0.0002	0.00302	0.00015	0.00441	0.00032	0.0002*
thigh	0.00305age −0.0095	< 0.0001	0.02902	0.00206	0.03352	0.00197	ns
shank	0.00102age −0.0028	< 0.0001	0.00921	0.00055	0.01244	0.00092	0.0030
foot	0.00034age −0.0007	0.0002	0.00308	0.00016	0.00383	0.00028	0.0230

^*Significant after sequential Bonferroni correction.

To allow a better comparison between the different age classes, relative segment inertial parameters (means and standard errors) for both categories are calculated and listed in Table 2. Compared with those for the mature age class, the segment length proportions relative to stature length of the head, forearm, hand and foot were significantly larger in the immature group. Details of length growth and development of the chimpanzee have been described by Schultz (1940) in a more elaborate study. In this study Schultz reports a similar decrease in relative hand and foot length with advancing age.

Table 2.

Relative segment inertial parameters for immature and mature male and female chimpanzees

	Immature		Mature
	F/M 14		F 23		M 16
Sex sample size	mean	SE	mean	SE	mean	SE	P-value sex related	P-value age related
Rel. length (%)
head	23.22	0.53	21.52	0.31	22.07	0.47	ns	0.0082
trunk	48.84	0.51	49.77	0.40	49.57	0.43	ns	ns
upper arm	27.08	0.43	26.19	0.30	26.42	0.42	ns	ns
forearm	27.75	0.32	26.43	0.26	26.80	0.34	ns	0.0042
hand	23.57	0.40	21.60	0.28	22.03	0.42	ns	0.0003*
thigh	26.17	0.25	25.67	0.23	25.54	0.22	ns	ns
shank	24.98	0.34	24.56	0.24	24.89	0.28	ns	ns
foot	23.04	0.23	21.98	0.20	21.77	0.17	ns	< 0.0001*
Rel. mass (%)
head	6.63	0.46	5.52	0.17	5.74	0.22	ns	0.0068
trunk	55.76	0.79	55.63	0.98	54.66	1.27	ns	ns
upper arm	3.51	0.18	3.85	0.16	3.81	0.21	ns	ns
forearm	2.66	0.06	2.68	0.09	2.78	0.11	ns	ns
hand	1.59	0.06	1.30	0.05	1.43	0.06	ns	0.0009
thigh	6.29	0.31	7.20	0.24	7.11	0.31	ns	0.0147
shank	2.92	0.10	2.85	0.09	3.10	0.11	ns	ns
foot	1.84	0.06	1.54	0.05	1.57	0.06	ns	< 0.0001*
CoM (%)
head	46.70	0.33	46.08	0.26	47.09	0.36	ns	ns
trunk	51.29	0.22	50.98	0.25	51.60	0.28	ns	ns
upper arm	51.97	0.49	52.50	0.38	52.08	0.41	ns	ns
forearm	55.08	0.38	55.51	0.23	54.67	0.29	0.0293	ns
hand	51.93	0.33	51.94	0.25	52.11	0.47	ns	ns
thigh	55.79	0.42	56.02	0.34	55.30	0.37	ns	ns
shank	55.08	0.39	54.61	0.22	53.97	0.43	ns	ns
foot	53.53	0.39	54.58	0.32	53.77	0.56	ns	ns
RGx (%)
head	28.33	0.67	27.70	0.16	28.17	0.49	ns	ns
trunk	30.35	0.12	30.66	0.15	30.79	0.10	ns	0.0436
upper arm	29.32	0.18	28.21	0.12	32.06	3.99	ns	ns
forearm	28.21	0.14	29.05	0.21	28.85	0.18	ns	ns
hand	27.22	0.09	27.86	0.12	27.96	0.16	ns	ns
thigh	28.78	0.18	27.48	0.11	27.46	0.14	ns	ns
shank	28.60	0.11	28.41	0.08	28.26	0.13	ns	ns
foot	27.15	0.04	27.07	0.12	27.35	0.10	ns	ns
RGy (%)
head	28.56	0.19	28.91	0.14	28.12	0.41	ns	ns
trunk	28.28	0.11	28.54	0.13	28.50	0.12	ns	ns
upper arm	30.05	0.14	30.01	0.12	34.01	4.12	ns	ns
forearm	28.57	0.15	28.27	0.10	28.36	0.15	ns	ns
hand	28.64	0.11	28.71	0.08	28.95	0.15	ns	ns
thigh	31.04	0.14	31.35	0.30	31.04	0.20	ns	ns
shank	29.53	0.14	29.53	0.08	29.23	0.16	ns	ns
foot	27.15	0.04	27.07	0.12	27.35	0.10	ns	ns

Length is relative to stature length, mass is relative to total body mass, CoM is the location of the CoM relative to segment length. with respect to the proximal joint, RG_x and RG_y are the radii of gyration expressed as a percentage of segment length.

^*Significant after sequential Bonferroni correction.

Furthermore, the distribution of mass between the segments differed slightly between age classes. Namely, more mass was concentrated in the head, hand and foot of the immature group, whereas more mass was found in the thigh of the mature class. The decrease in relative head mass (and length) was expected as few proportions undergo as rapid and far-reaching alterations as relative head size, which is in line with other studies on chimpanzees (Schultz, 1940) and humans (Jensen, 1993).

No age-dependent difference was found for the location of the centre of mass relative to segment length, indicating that the mass distribution within a segment remains similar regardless of age. The radii of gyration in the two axes were not significantly different in the two age categories.

The same conclusions were obtained when relating the various segment mass and length proportions to age as a continuous variable (i.e. regression analysis, data not shown).

Comparison of sex classes

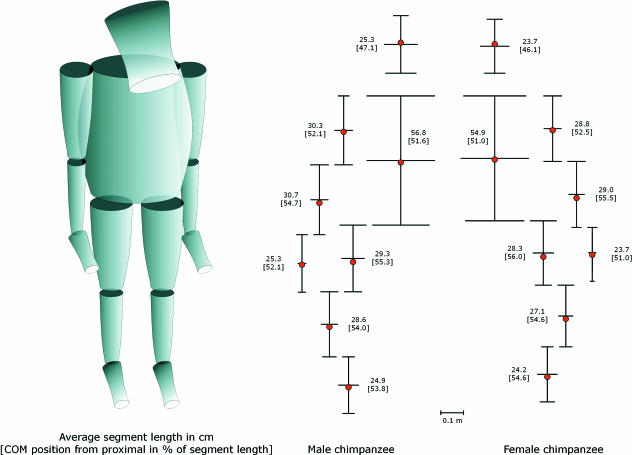
The means and standard errors for each parameter for both sexes separately are given in Table 1. All absolute measurements were higher in male than in female individuals allthough these are not always significantly different. A schematical comparison of male and female body build is given representing average segment length and proximal, medial and distal diameter in frontal plane in Fig. 3.

Fig. 3.

Comparison of body build of male and female Pan troglodytes with average segment length and proximal, medial and distal diameter in frontal plane. Red dots indicate the position of the centre of mass.

Stature length did not differ significantly according to sex. Hamada & Udono (2002) report total body lengths of 1.44 and 1.38 m for, respectively, males and females. Their total body length measurements include head height and are therefore higher than our stature length; nevertheless, they find a sex ratio (male body length)/(female body length) of 104.1% which is comparable with our 103.9%. Sex differences in absolute segment lengths were observed. Head and forelimb segment lengths were significantly longer in males while trunk and hindlimb segment lengths appeared similar among sexes. IMIs were 104.3 for females and 105.4 for males. Schultz (1930) found a similar intermembral index (107.5) in males and females whereas our study found a higher IMI for males because of the longer forelimbs. Schultz's male specimens had longer fore- and hindlimbs than his female individuals, but these parameters were not tested statistically. More striking is the dissimilarity in the length of the radius vs. forearm length and tibia vs. shank length, in which both skeletal measures (Schultz, 1930; Zihlman, 1984; Morbeck & Zihlman, 1989) are smaller than the bodily measures (Crompton et al. 1996; Isler et al. 2006; this study) while humerus/upper arm length and femur/thigh length appear quite similar. This inconsistency between studies is probably due to measurement techniques. Although we started measuring forearm length at the level of the epicondyle of the humerus the actual beginning of the radius is more distal. Likewise, for the shank length we began measurements at the level of the condyl whereas the tibia starts more distally.

For total body mass males were significantly heavier than females. This sexual dimorphism is easily discernible and is in concordance with several other studies (Schultz, 1940; Hamada & Udono, 2002). All body segment masses were significantly higher in males than in females with the exception of hand and thigh mass where no sex difference was determined. The higher masses can be expected as males have a significantly higher total body mass.

The two moments of inertia differed between sexes for all segments except the head, upper arm and thigh (for details see Table 1). Segment moments of inertia were directly proportional to their mass and to the mass distribution along the axis of rotation, and therefore higher moments of inertia are expected in heavier and longer male segments.

Relative segment inertial parameters (means and standard errors) for both sexes are listed in Table 2. No significant sex differences were found in relative segment lengths, masses, positions of centre of mass or radii of gyration. Crompton et al. (1996) reported segment inertial data obtained from double-pendulum experiments on four segmented common chimpanzees. Compared with their study our centre of mass positions were estimated more distally, especially in hand and foot segments. Differences in measurement techniques may be the source of this deviation. After segmentation the segment ends were often curved whereas the modelled segments had straight ends. The position of the centre of mass, which is a percentage of total segment length, depends on where measuring starts along this curved line. In this way, a difference of 2.8 cm in, for example, an average thigh can result in approximately 10% in centre of mass position.

The radii of gyration were, except for the head, similar to Crompton et al.'s (1996) measurements.

Whole limb inertial properties

In order to discuss overall limb shape and the consequences of limb muscle mass distribution on locomotor capacity, whole limb inertial properties for immature and male and female mature chimpanzees are described. The NPP, the period at which a given pendulum (here limb) requires the least amount of energy to maintain its swing, is also considered.

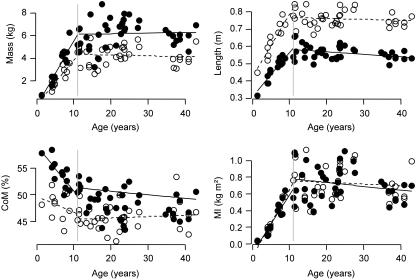
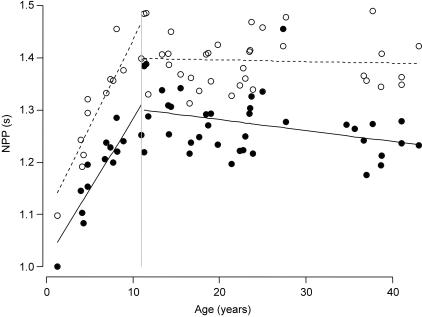
For the immature individuals the slopes (a) and intercepts (b) of the linear regression models are shown to describe the growth pattern. All corresponding slope P values are smaller than 0.05 (except for the centre of mass of the forelimb), implying that these slopes differ significantly from zero and that these parameters are age dependent (Table 3). For the mature individuals the means and standard errors for each parameter are given for both sexes separately (Table 3). From Fig. 4 it is clear that limb length and limb mass increased with age untill approximately 12 years. The relationship between age and limb length was equivalent in fore- and hindlimbs. For limb mass, however, there was a difference between limbs, with hindlimbs showing a larger increase. The increase of hindlimb mass was probably due to an increase of proportional thigh mass (Table 2), which can be related to more dependence upon motor behaviours involving hindlimbs during development. This, together with the decrease in proportional feet mass (Table 2), explains the more pronounced proximal shift of the hindlimb centre of mass (Fig. 4). The limbs’ moments of inertia showed a comparable increase with age (Fig. 4). The NPP increased with age and the relationship between age and NPP did not differ between forelimb and hindlimb (Fig. 5).

Table 3.

Whole limb inertial properties of immature chimpanzees represented by slopes (a) and intercepts (b) of the linear regression models and mature male and female chimpanzees represented by means and standard errors

	Immature				Mature
	F/M 14				F 23				M 16
sex sample size	ax + b fore	P-value of slope	ax + b hind	P-value of slope	mean fore		mean hind		mean fore		mean hind
Length (m)	0.04age +0.40	< 0.0001	0.03age +0.29	< 0.0001	0.71	0.0093	0.55	0.0082	0.78	0.0103	0.58	0.0097
Mass (kg)	0.38age −0.41	< 0.0001	0.57age −0.76	< 0.0001	3.53	0.1592	5.82	0.2422	4.75	0.1780	6.69	0.2840
CoM (%)	−0.31age +50.03	0.31430	−0.84age +59.91	0.0005	45.48	0.5143	50.30	0.3941	46.06	0.5750	50.56	0.4622
MI (kg m2)	0.076age −0.21	< 0.0001	0.069age −0.18	< 0.0001	0.57	0.0390	0.64	0.0392	0.87	0.0436	0.83	0.0459
NPP (s)	0.033age +1.07	< 0.0001	0.027age +0.98	< 0.0001	1.38	0.0102	1.26	0.0120	1.42	0.0114	1.30	0.0140

Fig. 4.

Whole limb length, mass, centre of mass from the proximal end and moment of inertia vs. age for Pan troglodytes. Forelimb is represented by open circles and hindlimb by filled circles.

Fig. 5.

NPP vs. age for Pan troglodytes. Forelimb is represented by open circles and hindlimb by filled circles.

Discussion

Method validity

We are aware that caution is needed in using captive apes to determine inertial properties because they often tend to obesity and fat accumulation especially in the trunk. Therefore, we compared TBM of our sample group with TBM from wild chimpanzees (Pan troglodytes troglodytes) shot by Major H. B. Powel-Cotton and colleagues between 1904 and 1932 (reported in Jungers & Susman, 1984). Mean TBM of these chimpanzees was 60 kg (range 50–70 kg) for males and 47.4 kg (range 42.3–50 kg) for females, compared with 55 and 47.7 kg for, respectively, males and females in our adult sample. Because the mean TBM and personal observations did not indicate obesity we considered our chimpanzee group to be reliable and we therefore calculated mass proportions using total body mass. In addition, studying wild (or captive) animals that have died from natural causes may be not completely representative given that they may have suffered from disease before death.

Apart from subspecies or sexual dimorphism, there is considerable individual variation in body shape within an ape species. We have been able to deal with this by using mean values obtained from a broad sample of specimens.

To verify the model, total body mass, deduced from the summed segment masses, was compared with measured total body mass. Both masses are highly correlated (Fig. 2). Moreover, the regression line relating these variables does not differ significantly from the line of identity. These results support the use of the model for calculating inertial properties. In addition, this model has been tested and used repeatedly in other studies (Crompton et al. 1996; Raichlen, 2005b; Isler et al. 2006; Wall-Scheffler et al. 2006) and is considered a practical and in vivo alternative for double-pendulum experiments. However, Isler et al. (2006) found that for segments with a complex shape such as hands and feet the model used here does not describe segment geometry accurately enough to allow a valid estimation of their inertial properties, even if the mass can be estimated correctly. Estimates of the CoM show a higher amount of variation for hands and feet and are estimated more distally. The assumed mass density of 10³ kg m⁻³ in the model might induce some degree of error due to different mass densities according to the distribution of bone, muscle and fat tissue. Yet, errors are relatively small compared with the extent of interindividual variation. Nevertheless, the data obtained using the double-pendulum technique are intrinsically more accurate than those obtained through external measurements alone, especially for hand and foot segments (Isler et al. 2006).

Age-related differences

Most notable in the age class comparison is the higher proportional of hand and foot mass at young ages (Table 2). These distally concentrated masses correspond to muscles that control manual and pedal grasping. A shift from distal to proximal limb mass distribution during ontogeny seems to be connected with the transition from dependent to independent locomotion. Chimpanzee infants depend on dorsal and ventral riding on their mothers at young ages (Preuschoft et al. 1992) and gradually develop the ability of independent (quadrupedal) locomotion both arboreal, in which juvenile African apes climb and use suspension more often than adults (Doran, 1992,1997; Hunt, 1992; Remis, 1995), and terrestrial. So it appears that the changing functional demands placed on the limbs during ontogeny explain the changes in limb mass distribution. In addition, quadrupedal locomotion requires propulsive forces mainly delivered by the hindlimbs (Kimura et al. 1977; Turnquist & Wells, 1994). This is reflected in the increase with age of proportional thigh mass in this study (Table 2) and can therefore be related to more reliance upon motor behaviours involving hindlimbs. Naturally, grasping abilities remain important during arboreal behaviours in adult chimpanzees and limb masses remain relatively distally concentrated compared with, for example, humans. These findings are supported by earlier studies reporting ontogenetic changes of segment mass distribution in macaques (Turnquist & Wells, 1994) and baboons (Raichlen, 2005b). Both studies found an alteration of limb mass distribution from distal to proximal with increasing age and correlated this morphological change with locomotor development (from grasping onto their mother to independent locomotor propulsion).

In a related study, Raichlen (2005a) was able to demonstrate a shift in the kinematics as the limb mass distributions changed during ontogeny in Papio cynocephalus. He found relatively low stride frequencies together with relatively long stride lengths and stance durations at very young ages when their limb mass was most distally located. The relationship between limb mass distribution and kinematics in chimpanzees could not be examined in this study because of the absence of sufficient kinematic data.

Furthermore, it is important to consider the changes in proportional segment masses within the context of changes in segment lengths. In our chimpanzee sample proportional foot and hand lengths decrease with age (Table 2). The decrease in proportional foot and hand mass may therefore be due to a decrease in dimensionless segment length with increasing age. Therefore, the precise reason why infants have more distally concentrated limb masses remains vague. The nature of our data set (cross-sectional data), the small sample size and the fact that we are examining only one species does not allow further analysis of the ontogenetic relationship between limb mass distribution and locomotion type. More ontogenetic data on mass distribution and locomotor mode of a wide range of infant primates is necessary to elucidate the aforementioned relationship (Raichlen, 2005b).

Sex-related differences

Sexual dimorphism in body mass and forelimb length is repeatedly believed to be a consequence of sexual selection (excluding rivals from mating) and predation defence (winning fights) in hominoids (Wrangham & Peterson, 1996; Plavcan, 2001). However, other researchers such as Reno et al. (2003) state that despite the chimpanzee's polygynous reproductive strategy their skeletal and body mass dimorphism is rather small, thus challenging the aforementioned theory.

In a more biomechanical context, Jungers (1985) has shown that forelimb length increases with body weight in all simians. Indeed, relatively longer forelimbs facilitate vertical climbing on tree trunks, which is disproportionally more costly for heavier individuals (Cartmill, 1972,1974; Jungers & Susman, 1984). The longer the distance between handhold and foothold, the smaller the resultant external force at hand/substrate contact point. If the arm is kept more or less in line with the external force, the sum of rotating moments is at a minimum (for details see Preuschoft et al. 1992), thus saving muscle force, which is extremely important for large-bodied animals that can utilize relatively less muscle force (Demes & Günther, 1989). This probably explains the difference in forelimb length found between the chimpanzee sexes (Table 1).

Locomotor consequences of the limb NPP

The NPP may be used as a qualitative predictor of a limb's swing period and is thought to be an important energy-saving mechanism especially during walking (Hildebrand, 1985; Myers & Steudel, 1997). This means that if the limb's actual swing period differs from ¹/2NPP (half NPP since swing phase is only a forward swing whereas NPP is a complete oscillation of forward and backward swing), the cost of locomotion increases. When comparing the actual swing phase duration during chimpanzee quadrupedal locomotion [average swing period for an adult chimpanzee = 0.37 s for forelimbs and 0.48 s for hindlimbs (Kimura, 1987); note that this variable is velocity dependent) with ¹/2NPP, the actual swing phase proved to be considerably lower. This suggests that there is more than mere passive pendulum-like swinging. The limb's swing phase in chimpanzees is probably driven by considerable muscular action (Whittlesey et al. 2000). Indeed, several researchers have shown muscle activity in the shoulder and hip during the swing phase in quadrupedal animals (Basmajian, 1978; Larson & Stern, 1989; Whitehead & Larson, 1994).

In addition, the NPP of all four limbs in a quadrupedal animal should converge in order to obtain maximal potential and kinetic energy exchange (Meyers & Steudel, 1997; Raichlen, 2004). Our data do not show convergence between forelimb and hindlimb NPP (an average difference of 8.5%, see Table 3 and Fig. 5) in contrast with what is expected for quadrupeds. The calculated values for the hindlimbs are lower than the NPP of the forelimbs. This is in concordance with the data of Isler et al. (2006), where the NPP of the forelimbs was always larger than the NPP of the hindlimbs for all hominoids. As our NPPs are calculated for extended limbs while the limb joints flex during locomotion, it is likely that our values are overestimated especially for the hindlimb where knee flexion can be particularly large (D'Août et al. 2002). Therefore, the NPP of a flexed adult chimpanzee hindlimb at mid-swing was calculated to form an idea of the impact of flexed limbs on the NPP. Because of limb flexion a decrease of the hindlimb NPP of 6% (i.e. for an average NPP of 1.27 s toward an average NPP of 1.20 s) was found through which the difference between fore- and hindlimb NPPs was even more pronounced.

The difference between NPP and swing period, together with the dissimilarity between the fore- and hindlimb NPPs, suggest that the chimpanzee's limbs are not optimized for efficient quadrupedal walking. However, chimpanzee fore- and hindlimb NPPs are more similar than those of other hominoids, suggesting that chimpanzee limb inertial properties are more consistent with efficient quadrupedal gait than other hominoids (see also Isler et al. 2006). Indeed, we deduced from Isler et al. (2006) that differences between forelimbs and hindlimb NPPs are higher in Gorilla gorilla (±10.6%), Hylobates (±12.8%) and Pongo pygmaeus (±15.9%). Chimpanzee limb NPPs are less similar than those of other quadrupeds. Raichlen (2004) reported a convergence of fore- and hindlimb NPPs for Papio cynocephalus. The average difference he found for baboons was 2.4%, which is comparable with Myers & Steudel's (1997) canid sample, despite the differences in limb mass distribution.

The reason why limbs of African apes are not optimized for pendular motion during quadrupedal locomotion can be found in their morphology, which reflects a compromise between adaptations to various locomotor modes. For example, chimpanzees have relatively long arms and heavy distal segments (for grasping abilities) that are advantageous for arboreal locomotion. At the same time they have limb properties that are beneficial enough to engage in quadrupedal locomotion.

General conclusions

No large differences are observed between age classes. Most notable is that juvenile chimpanzees exhibit relatively longer and heavier hand and foot segments than adults. Unfortunately, only a small number of young individuals were available, limiting our comparative study on age effects.

Furthermore, most sex-related differences can be reduced to a dissimilarity in absolute mass and the resulting moments of inertia, both being higher in male chimpanzees. Additionally, males tend to have longer upper limbs than females.

In general, we provide a data set valuable for locomotor biomechanical modelling including means of absolute measures of males, females and two different age classes. Moreover, when we consider the proportional inertial data we can conclude that these parameters are very much alike in the different age and sex classes, suggesting that we can speak of the relative inertial properties of chimpanzees (Pan troglodytes).

To conclude, we encourage publication of more, similar data for as many species as possible, either from double-pendulum experiments or through external measurements, to achieve a future data set that will allow us to make sound comparisons of inertial properties between other primate species.

APPENDIX

Measurement protocol and used landmarks

Because the measurements were typically made during brief anaesthesia for medical reasons, and consequently had to be made quickly, we adopted a strict protocol. We started with the most important measures (for our purposes) but were able to complete the whole protocol for most subjects.

First, the subject was weighed on a precision scale. Then, the subject was layed on a table and the lengths of all body segments were measured using a tape measure (for the trunk) or digital calipers (for the other segments). Next, the required input measures for the model were determined (i.e. frontal and saggital widths proximally, in the middle of the segment, and distally). We followed the next order: thigh, shank, foot, trunk, upper arm, lower arm, hand, head.

Below, we describe and comment on the anatomical landmarks that were used to take the measurements as input in the Crompton et al. (1996) model. It is not always self-evident which landmark is best, as purely anatomically accurate landmarks (e.g. the malleoli) will not necessarily result in a model that represents the overall shape of the segment best. The landmarks described below were considered to be both anatomically unequivocal and good proxies for the estimation of the segment's shape. This is supported by the good correspondence between estimated and measured body mass (see Method validity).

Frontal and saggital were defined with respect to normal quadrupedal (knuckle-walking) posture. The ‘middle’ positions are exactly halfway between the proximal and distal positions, unless stated otherwise.

Table A1.

Landmarks used for the segment length measurements

Segment	Length measured
Trunk	From acromion of scapula to greater trochanter of femur
Head	From occiput to incisors
Thigh	From greater trochanter of femur to palpated knee joint cleft
Shank	From palpated knee joint cleft to lateral malleolus
Foot	From heel (tuber calcanei) to tip of 3rd toe
Upper arm	From acromion to palpated elbow joint position
Lower arm	From palpated elbow joint position to palpated wrist joint
Hand	From palpated wrist joint to tip of 3rd finger

Table A2.

Landmarks used for the segment cross-sectional measurements

Segment	Measure	Frontal	Saggital
Trunk	Proximal	from left to right armpit	body height when lying flat on a table, at level of armpits
	Distal	from lef to right palpated anterior superior iliac spine (ASIS)	body height when lying flat on a table, at level of ASIS
	Medial	from left to right body side at level of greatest rib cage circumference (corresponding well with the middle of the trunk)	body height when lying flat on a table, at level of greatest rib cage circumference
Head	Proximal	*†	*†
	Distal	at level of nostrils†	at level of nostrils†
	Medial	frontal width at level of eyebrows (corresponding well with the middle of the head)	from eyebrows to edge of mandible, perpendicular to long axis of the head
Thigh	Proximal	from medial thigh surface at the level of the groin to lateral side, perpedicular to long axis of thigh	from ventral thigh surface at the level of the groin to dorsal side, perpedicular to long axis of thigh
	Distal	from medial to lateral condyle of femur	from ventral surface of the thigh (just above patella) to dorsal surface, perpendicular to long axis of thigh
	Medial	orientation as in proximal and distal measures, midway between the proximal and distal positions	orientation as in proximal and distal measures, midway between the proximal and distal positions
Shank	Proximal	distal measurements of thigh were used	distal measurements of thigh were used
	Distal	frontal-view width, just proximal to the malleoli	layteral-view width at the level of the malleoli
	Medial	orientation as in proximal and distal measures, midway between the proximal and distal positions	orientation as in proximal and distal measures, midway between the proximal and distal positions
Foot	Proximal	frontal-view with immediately below the malleoli	from the lateral malleolus to the foot sole, perpedicluar to the long axis of the foot
	Distal	toes II–IV†	toes II–IV†
	Medial	orientation as in proximal and distal measures, midway between the proximal and distal positions	orientation as in proximal and distal measures, midway between the proximal and distal positions
Upper arm	Proximal	from armpit to lateral arm surface (measured perpendicular to the long axis of the upper arm)	position as in the frontal measurement, but perpendicular to it and to the long axis of the upper arm
	Distal	from medial to lateral epicondyle of humerus	position as in the frontal measurement, but perpendicular to it and to the long axis of the upper arm
	Medial	same orientation as proximal and distal	same orientation as proximal and distal
Lower arm	Proximal	distal measurements of upper arm were used	distal measurements of upper arm were used
	Distal	frontal-view width at the level of the palpated wrist joint (knuckle-walking posture, i.e. in approximately neutral position)	position as in the frontal measure, but perpendicular to it and to the long axis of the lower arm
	Medial	same orientation as proximal and distal	same orientation as proximal and distal
Hand	Proximal	distal measurements of lower arm were used	distal measurements of lower arm were used
	Distal	frontal-view width of fingers II–IV (proximal phalangi)**	saggital-view width of fingers II–IV (proximal phalangi)†
	Medial	same orientation as proximal and distal	same orientation as proximal and distal

^*These variables are very hard to measure unequivocally, and, knowing how the model works, we have focused on taking a measure that would result in a realistic fit of the model with respect to the real segment shape.

^†Theoretically these measures should be zero, but we took the measures reported here as a better representation for the distal shape of the segment, which would result in a better model fit.